Kinematic-geometry of lines with special trajectories in spatial kinematics

1.   Introduction

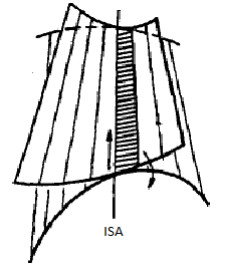
Line geometry possesses a close relationship to spatial kinematics and has thence found implementations in robot kinematics and mechanisms ^[1,2,3,4]. Spatial kinematics considers the intrinsic properties of the line trajectory from the notions of ruled surfaces in differential line geometry. As we know, in spatial kinematics, the instantaneous screw axis $(\mathbb{ISA}$) of a moveable body forms a pair of ruled surfaces, i.e., the moveable and stationary axodes, with $\mathbb{ISA}$ as their generating line in the moveable space and in the stationary space, respectively. In the movement the axodes roll and slide relative to each other in a private way such that the tangential contact between the axodes is constantly maintained over the entire length of the two matting rulings (one being in each axode), which together define the $\mathbb{ISA}$ at any instant. It is considerable that not only does a private movement give rise to a unique set of axodes, but the converse also applies. This means that, should the axodes of any movement be known, the particular movement can be created without knowledge of the physical members of the mechanism, their configuration, specific dimensions, or the means by which they are fastened. The use of axodes in the process of synthesis becomes apparent when the axodes are intermediary between the physical mechanism and the actual movement of its members^[5,6,7,8,9].

Rather unexpectedly, dual numbers have been utilized to consider the movement of a line space; they appear even to be the more convenient tools for this end. In dual number and screw algebra, the E. Study map concludes that the family of the dual points on the dual unit sphere in the dual 3-space $\mathbb{D}^{3}$ is in one-to-one correspondence with the family of all oriented lines in Euclidean 3-space $\mathbb{E}^{3}$. According to this map, a one parameter family of points (a dual curve) on the dual unit sphere matches to a one-parameter family of oriented lines (ruled surface) in $\mathbb{E}^{3}$. Additional features of the E. Study's map can be found in ^{[10,11,12,13,14,15,16,17,18,19]}.

In this work, based on the axodes, the invariants and the features of a line trajectory in spatial kinematics are investigated. Meanwhile, a new height dual function is defined and utilized to extend the planar and spherical results to spatial kinematics. The classical results of spatial kinematics are obtained in this way, as well as new loci of lines which instantaneously generate special trajectories. The torsion line congruence is established and researched in detail, and the invariants of the axodes are utilized for establishing a new proof of the Disteli formulae.

2.   Elements of screw calculus

In this section, we give a brief outline of the theory of dual numbers and dual vectors ^{[1,2,3,4,5,10,11,12,13,14,15,16,17,18]}. If $x, \;$and $x^{\ast }$ are real numbers, the number $\widehat{x} = x+\varepsilon x^{\ast }$ is named a dual number. Here, $\varepsilon$ is a dual unit subject to $\varepsilon \neq 0, \; \varepsilon ^{2} = 0, \; \varepsilon.1 = 1.\varepsilon = \varepsilon.$ The set of dual numbers, $\mathbb{D}$, creates a commutative ring having the numbers $\varepsilon x^{\ast }(x^{\ast }\in \mathbb{R}$) as divisors of zero, not a field. No number $\varepsilon x^{\ast }$ has inverse in the algebra. However, the other laws of the algebra of dual numbers are the same as of the complex numbers. The set

together with the Euclidean scalar product

forms the so named dual 3-space $\mathbb{D}^{3}$. This yields

where $\widehat{\mathbf{f}}_{1}$, $\widehat{\mathbf{f}}_{2},$ and $\widehat{ \mathbf{f}}_{3}$, are the dual base at the origin point $\widehat{\mathbf{0}} \left(0, 0, 0\right)$ of the dual 3-space $\mathbb{D}^{3}$. Then, a dual vector $\widehat{\mathbf{x}} = (\widehat{x}_{1}, \widehat{x}_{2}, \widehat{x} _{3})^{t}$ has coordinates $\widehat{x}_{i} = (x_{i}+\varepsilon x_{i}^{\ast })\in \mathbb{D}$. If $\mathbf{x}\neq \mathbf{0}$, the norm $\left \Vert \widehat{\mathbf{x}}\right \Vert$ of $\widehat{\mathbf{x}}$ is defined by

Then, the vector $\widehat{\mathbf{x}}$ is named a dual unit vector if $\left \Vert \widehat{\mathbf{x}}\right \Vert ^{2}\mathbf{ = }1$. It is evident that

The six components $x_{i}, \; x_{i}^{\ast }(i = 1, 2, 3)$ of $\mathbf{x}\;$and $\mathbf{x}^{\ast }$ are named the normed Plücker coordinates of $\widehat{\mathbf{x}}$. The dual unit sphere is:

Via this we have the E. Study map^[1,2,3,4,5]: The set of points on dual unit sphere $\mathbb{K}$ in the dual 3-space $\mathbb{D}^{3}$ are in one-to-one correspondence with the set of oriented lines in the Euclidean 3-space $\mathbb{E}^{3}$.

The representation of oriented lines in $\mathbb{E}^{3}$ by dual unit vectors brings sundry advantages, and from now on we do not distinguish between oriented lines and their corresponding dual unit vectors.

2.1.   One-parameter dual spherical movements

Let $\mathbb{K}_{m}$ and $\mathbb{K}_{f}$ be two dual unit spheres with $\widehat{\mathbf{0}}$ as a mutual center in $\mathbb{D}^{3}$. Let $\{ \widehat{\mathbf{e}}\} = \{ \widehat{\mathbf{0}}$; $\ \widehat{\mathbf{e}} _{1}, \; \widehat{\mathbf{e}}_{2}, \; \widehat{\mathbf{e}}_{3} \}$ and $\{ \widehat{\mathbf{f}}\} = \{ \widehat{\mathbf{0}}$; $\; \widehat{\mathbf{f}}_{1}$, $\; \widehat{\mathbf{f}}_{2}$, $\; \widehat{\mathbf{f}}_{3} \}$ be two orthonormal dual frames rigidly related with $\mathbb{K}_{m}$ and $\mathbb{K} _{f}$, respectively. If we set $\{ \widehat{\mathbf{f}}\}$ as stationary, whereas the elements of set $\{ \widehat{\mathbf{e}}\}$ are functions of a real parameter $t\in \mathbb{R}$ (say, the time), then we may say that $\mathbb{K}_{m}$ moves with respect to $\mathbb{K}_{f}$. This movement is named a one-parameter dual spherical movement and will be denoted by $\mathbb{K}_{m}/\mathbb{K}_{f}$. According to the E. Study map, if $\mathbb{K}_{m}$ and $\mathbb{K}_{f}$ represent to the line spaces $\mathbb{L}_{m}$ and $\mathbb{L}_{f}$, respectively, then $\mathbb{K}_{m}/\mathbb{K}_{f}$ represents the one-parameter spatial movement $\mathbb{L}_{m}/\mathbb{L}_{f}.$ Therefore, $\mathbb{L}_{m}$ is the moveable space with respect to the fixed space $\mathbb{L}_{f}$ in $\mathbb{E}^{3}$.

Let us now introduce a further dual unit sphere $\mathbb{K}_{r}$ addressed by the orthonormal dual frame $\{ \widehat{\boldsymbol{\zeta }}\} = \{ \widehat{ \mathbf{0}}; \; \widehat{\boldsymbol{\zeta }}_{1}, \; \widehat{\boldsymbol{\zeta }} _{2}, \; \widehat{\boldsymbol{\zeta }}_{3}\}$, which is considered by the 1st order instantaneous properties of the movement as follows: $\widehat{\boldsymbol{ \zeta }}_{1}(t) = \zeta _{1}(t)+\varepsilon \zeta _{1}^{\ast }(t)$ as the $\mathbb{ISA}$ of the movement $\mathbb{L}_{m}/ \mathbb{L}_{f}$, and $\widehat{\boldsymbol{\zeta }}_{2}(t): = \zeta _{2}(t)+\varepsilon \zeta _{2}^{\ast }(t) = \frac{d\widehat{ \boldsymbol{\zeta }}_{1}}{dt}\left \Vert \frac{d\widehat{\boldsymbol{\zeta }}_{1}}{dt }\right \Vert ^{-1}$ as the mutual central normal of two detached screw axes. A third dual unit vector is $\widehat{\boldsymbol{\zeta }}_{3}(t) = \widehat{ \boldsymbol{\zeta }}_{1}\times \widehat{\boldsymbol{\zeta }}_{2}$. $\{ \widehat{ \boldsymbol{\zeta }}\}$ is named the relative Blaschke frame, and the lines $\widehat{\boldsymbol{\zeta }}_{1}, \; \widehat{\boldsymbol{\zeta }}_{2},$ and $\widehat{\boldsymbol{\zeta }}_{3}$ intersect at the mutual striction (central) point $\mathbf{s}$ of $\pi _{i} \; (i = m, \; f)$ ^[5,6,7,8,9]. We have $d\widehat{s} _{i} = ds_{i}+\varepsilon ds_{i}^{\ast } = \left \Vert \frac{d\widehat{\boldsymbol{ \zeta }}_{1}}{dt}\right \Vert dt = \widehat{p}(t)dt$ as the dual arc length of $\widehat{\boldsymbol{\zeta }}_{1}(t)$. Since $\widehat{p}(t) = p+\varepsilon p^{\ast }$ contains only first derivatives of $\widehat{\boldsymbol{\zeta }} _{1}(t)$, it is a first order estimate of the movement $\mathbb{K}_{m}/\mathbb{ K}_{f}$, in specific, its dual speed. We let $d\widehat{s} = ds+\varepsilon ds^{\ast }$ to designate $d\widehat{s}_{i}$, since they are equal to each other. The tangent vector of the striction curve $\mathbf{s}(s)$ is given by ^{[10,11,12,13,14,15,16]}:

The distribution parameter of $\pi _{i}$ is

Proposition 1. In the movement $\mathbb{L}_{m}/\mathbb{L}_{f}$ the axodes have the $\mathbb{ISA}$ of the position in common, that is, the moveable axode is fastened to the stationary axode over the $\mathbb{ISA}$ in the 1st order at any instant $t$ (see Figure 1).

Furthermore, the dual arc-length derivative of $\mathbb{K}_{r}/\mathbb{K} _{i}$ is governed by ^{[11,12,13,14,15]}:

where

is the dual spherical curvature of $\pi _{i}$. Via Eq (2.7), the Disteli-axis (evolute or curvature axis) of $\pi _{i}$ is given by

Let $\widehat{\phi }_{i}(\widehat{s}) = \phi _{i}+\varepsilon \phi _{i}^{\ast }$ be the radius of curvature between $\widehat{\boldsymbol{\zeta }}_{1}$ and $\widehat{\mathbf{b}}_{i}$. Then,

where

Thus, we obtain

This is the dual equivalent of a well-known formula of the Euler-Savary equation from ordinary spherical kinematics^{[1,2,3,4,5,6]}. This dual form is a relationship between the two axodes of the movement $\mathbb{L}_{m}/\mathbb{L}_{f}$. From the real and the dual parts of Eq (2.8), respectively, we get

and

Equation (2.13) together with (2.14) are new Disteli formulae for the axodes of the movement $\mathbb{L}_{m}/\mathbb{L}_{f}$.

Now, let us assume that the relative Blaschke frame is fixed in $\mathbb{K} _{m}$. Then,

where $\widehat{\boldsymbol{\omega }}\mathbf{: = }\widehat{\boldsymbol{\omega }}_{f} \mathbf{-}\widehat{\boldsymbol{\omega }}_{m} = \widehat{\omega }\widehat{\boldsymbol{ \zeta }}_{1}$ is the relative angular vector. $\left \Vert \widehat{\boldsymbol{ \omega }}\right \Vert = \widehat{\omega } = \widehat{\omega }+\varepsilon \widehat{\omega }^{\ast } = \gamma _{r}+\varepsilon \left(\Gamma _{r}-\mu \gamma _{r}\right)$ is the relative dual spherical curvature. It follows that $\omega = \gamma _{f}-\gamma _{m}$ and $\omega ^{\ast } = \Gamma _{f}-\Gamma _{m}-\mu \left(\gamma _{f}-\gamma _{m}\right)$ are the rotational angular speed and translational angular speed of the movement $\mathbb{L}_{m}/\mathbb{L}_{f}$, as well as being both invariants in kinematics, respectively.

Proposition 2. In the movement $\mathbb{L}_{m}/\mathbb{L}_{f}$, at any instant $t\in \mathbb{R}$, the pitch $h(s)$ is given by

In this study, we disregard the pure translational movements, that is, $\omega ^{\ast }\neq 0$. Also, we omit zero divisors $\omega = 0$. Therefore, we shall think about only non-torsional movements, so that the axodes are skew ruled surfaces ($\mu \neq 0$).

3.   Lines with special trajectories

For one-parameter spatial movement $\mathbb{L}_{m}/\mathbb{L}_{f}$, each stationary line $\widehat{\mathbf{x}}$ linked with the movable axode, in general, will describe a ruled surface $(\widehat{x})$ in the stationary space $\mathbb{L}_{f}$. In kinematics, this ruled surface is referred to as line trajectory. Since all kinematic-geometric characteristics can then be derived with the invariants of the axodes of the movement $\mathbb{L}_{m}/ \mathbb{L}_{f}$. Then, the line trajectory can be obtained in terms of these invariants. Therefore, we consider a dual unit vector $\widehat{ \mathbf{x}}$ such that its coordinates are

where

The velocity $\widehat{\mathbf{x}}^{^{\prime }}$ and the acceleration $\widehat{\mathbf{x}}^{^{\prime \prime }}$ of $\widehat{\mathbf{x}}$ stationary in $\mathbb{L}_{m}$, respectively, are

and

Then,

The dual arc length $d\widehat{u} = du+\varepsilon du^{\ast }$ of $\widehat{ \mathbf{x}}(\widehat{s})$ is

The distribution parameter of $(\widehat{x})$ is

In order to consider the features of $(\widehat{x})$, the Blaschke frame is determined as:

The dual unit vectors $\widehat{\mathbf{x}}$, $\widehat{\mathbf{t}}$ and $\widehat{\mathbf{g}}$ match to three concurrent mutually orthogonal lines in $\mathbb{E}^{3}$. Their mutual point is the central point $\mathbf{c}$ on the ruling $\widehat{\mathbf{x}}$. $\widehat{\mathbf{g}}(\widehat{u})$ is the mutual perpendicular to $\widehat{\mathbf{x}}(\widehat{u})$ and $\widehat{\mathbf{x}}(\widehat{u}+d\widehat{u})$, and it is considered the central tangent of $(\widehat{x})$ at the central point. The trajectory of the central point is the striction curve. The line $\widehat{\mathbf{t}}$ is the central normal of $(\widehat{x})$ at the central point. The Blaschke formulae are

where

is the dual spherical curvature of $\widehat{\mathbf{x}}(\widehat{u})$. The tangent of the striction curve is given by:

The Serret-Frenet frame of $\widehat{\mathbf{x}}(\widehat{u})$ is established through a rotation of ($\widehat{\mathbf{x}}$, $\widehat{\mathbf{g }}$) according to

with a certain dual angle $\widehat{\phi } = \phi +\varepsilon \phi ^{\ast }$, where $\widehat{\mathbf{b}}$ is the binormal, and $\widehat{\mathbf{n}}$ is the principal normal. It is clear that $\widehat{\mathbf{b}}$ is the Disteli-axis (striction axis or curvature axis) of the ruled surface $(\widehat{x})$. Thereby, the dual Serret-Frenet formulae are

where $\widehat{\kappa }(\widehat{u})$ is the dual curvature, and $\widehat{ \tau }(\widehat{u})$ is the dual torsion of the dual curve $\widehat{\mathbf{ x}}(\widehat{u})\in \mathbb{K}_{f}$. Also, we may write the following relationships:

3.1.   Height dual functions

In analogy with ^[18,19], a dual point $\widehat{\mathbf{b}}_{0}$ of $\mathbb{K}_{f}$ will be said to be a $\widehat{\mathbf{b}}_{k}$ evolute of the dual curve$\ \widehat{\mathbf{x}}(\widehat{u})\in \mathbb{K}_{f}$; for all $i$ such that $1\leq i\leq k$, $< \widehat{\mathbf{b}}_{0}, \widehat{ \mathbf{x}}^{i}(\widehat{u}) > = 0$, but $< \widehat{\mathbf{b}}_{0}, \widehat{ \mathbf{x}}^{k+1}(\widehat{u}) > \neq 0$. Here, $\widehat{\mathbf{x}}^{i}$ indicates the i-th derivatives of $\widehat{\mathbf{x}}$ with respect to the dual arc length of$\mathbf{\ }\widehat{\mathbf{x}}(\widehat{u})\in \mathbb{K} _{f}$. For the first evolute $\widehat{\mathbf{b}}$ of$\ \widehat{\mathbf{x}} (\widehat{u})$, we have $< \widehat{\mathbf{b}}, \widehat{\mathbf{x}} ^{^{\prime }} > = \pm < \widehat{\mathbf{b}}, \widehat{\mathbf{t}} > = 0$, and $< \widehat{\mathbf{b}}, \widehat{\mathbf{x}}^{^{\prime \prime }} > = \pm < \widehat{ \mathbf{b}}, \widehat{\mathbf{x}}\mathbf{+}\widehat{\gamma }\widehat{\mathbf{g }} > \neq 0$. So, $\widehat{\mathbf{b}}$ is at least a $\widehat{\mathbf{b}} _{2}$ evolute of $\widehat{\mathbf{x}}(\widehat{u})\in \mathbb{K}_{f}$.

We now address a differentiable dual function $\widehat{h}:I\times \mathbb{K} _{f}\rightarrow \mathbb{D}$, by $\widehat{h}(\widehat{u}, \widehat{\mathbf{b}} _{0}) = < \widehat{\mathbf{b}}_{0}, \widehat{\mathbf{x}} >$. We call $\widehat{h}$ a height dual function on $\widehat{\mathbf{x}}(\widehat{u})\in \mathbb{K} _{f}$. We use the notation $\widehat{h}(\widehat{u}) = \widehat{h}(\widehat{u}, \widehat{\mathbf{b}}_{0})$ for any stationary point $\widehat{\mathbf{b}} _{0}\in \mathbb{K}_{f}$.

Proposition 3. Under the above notations, the following holds:

(1) $\widehat{h}$ will be stationary in the 1st approximation if and only if $\widehat{\mathbf{b}}_{0}\in Sp\{ \widehat{\mathbf{x}}$, $\widehat{\mathbf{g}} \mathbf{\}}$, that is,

for some dual numbers $\widehat{a}_{1}, \widehat{a}_{2}\in \mathbb{D},$ and $\widehat{a}_{1}^{2}+\widehat{a}_{2}^{2} = 1$.

(2) $\widehat{h}$ will be stationary in the 2nd approximation if and only if $\widehat{\mathbf{b}}_{0}$ is $\widehat{\mathbf{b}}_{2}$ evolute of $\widehat{ \mathbf{x}}(\widehat{u})\in \mathbb{K}_{f}$, that is,

(3) $\widehat{h}$ will be invariant in the 3rd approximation if and only if $\widehat{\mathbf{b}}_{0}$ is $\widehat{\mathbf{b}}_{3}$ evolute of $\widehat{ \mathbf{x}}(\widehat{u})\in \mathbb{K}_{f}$, that is,

(4) $\widehat{h}$ will be stationary in the 4th approximation if and only if $\widehat{\mathbf{b}}_{0}$ is $\widehat{\mathbf{b}}_{4}$ evolute of $\widehat{ \mathbf{x}}(\widehat{u})\in \mathbb{K}_{f}$, that is,

Proof. For the 1st derivative of $\widehat{h}$ we get:

So, we get

for some dual numbers $\widehat{a}_{1}, \; \widehat{a}_{2}\in \mathbb{D},$ and $\widehat{a}_{1}^{2}+\widehat{a}_{2}^{2} = 1$, the result is clear.

(2) Derivative of Eq (3.18) leads to

By using Eq (3.19), we have

(3) Derivative of Eq (3.20) leads to

Hence, we have

(4) By similar arguments, we can also have

The proof is completed.

Via the above proposition, we have the following:

(a) The osculating circle $\mathbb{S}$ of $\widehat{\mathbf{x}}(\widehat{u})\in \mathbb{K}_{f}$ is determined by the equations

which are obtained from the status that the osculating circle should have contact of at least 3rd order at $\widehat{\mathbf{x}}(\widehat{u}_{0})$ if and only if $\widehat{\gamma }^{^{\prime }}\neq 0$.

(b) The osculating circle $\mathbb{S}$ and the curve $\widehat{\mathbf{x}}(\widehat{u})\in \mathbb{K}_{f}$ have at least 4th order at $\widehat{\mathbf{ x}}(\widehat{u}_{0})$ if and only if $\widehat{\gamma }^{^{\prime }} = 0$, and $\widehat{\gamma }^{^{\prime \prime }}\neq 0$.

In this way, by considering the evolutes of $\widehat{\mathbf{x}}(\widehat{u})\in \mathbb{K}_{f}$ we can get a sequence of evolutes $\widehat{\mathbf{b}} _{2}$, $\widehat{\mathbf{b}}_{3}$, ..., $\widehat{\mathbf{b}}_{n}$. The properties and the relationships among these evolutes and their involute are very interesting problems. For example, it is easy to see that when $\widehat{\mathbf{b}}_{0}\mathbf{ = }\pm \widehat{\mathbf{b}}$, and $\widehat{ \tau }(\widehat{u}) = 0$($\widehat{\gamma }^{^{\prime }} = \widehat{\phi } ^{^{\prime }} = 0$), $\widehat{\mathbf{x}}(\widehat{u})\in \mathbb{K}_{f}$ is located at $\widehat{\phi } = dual \; const$. relative to $\widehat{\mathbf{b}} _{0}$.

3.2.   Torsion line congruence

We now examine the line trajectories which are the spatial equivalent of the cubic of stationary curvature^[1,2,3,4,5]. It is obvious that for all points with $\widehat{\tau }(\widehat{u}) = 0$, their trajectories lie on a dual great circle up to third order. Then,

Therefore, the spatial equivalent of the cubic of stationary curvature is defined by (a) the line complex defined by the torsion cone $C: \; \tau (u) = 0$, and (b) the line complex defined by the related plane of lines $\pi : \; \tau ^{\ast }(u) = 0$. All the family of lines $\widehat{\mathbf{x}}$ of the movable space $\mathbb{L}_{m}$ and also in the plane satisfy $\pi : \; \tau ^{\ast }(u) = 0$ initiating the torsion line congruence. Therefore, the torsion line congruence consists of a family of planes $\pi$, each of which is related with a direction of the inflection cone $C$. Hence, we have proved the following theorem:

Theorem 1. In one-parameter spatial movement $\mathbb{L}_{m}/ \mathbb{L}_{f}$, consider a family of related lines of the movable axode, such that each one of these lines has analog of a cubic of stationary curvature. Then, this family of lines forms a torsion line congruence which is common lines of the two line complexes $\tau (u) = 0$, and $\tau ^{\ast }(u) = 0$.

However, from Eq (3.13), we have

This means that $\phi = c(real\; const.)$, and$\; \phi ^{\ast } = c^{\ast }(real\; const.)$. Hence, each ruled surface in the torsion line congruence is a stationary Disteli-axis ruled surface. Furthermore, from Eq (3.9) we have the ordinary differential equation; ODE, $\widehat{\mathbf{t}}^{^{^{^{\prime \prime }}}}+ \widehat{\kappa }^{2}\widehat{\mathbf{t}} = \mathbf{0}$. Without loss of generality, we may take $\widehat{\mathbf{t}}(0) = (0, 0, 1)$, and the general solution of the ODE becomes

for dual constants $\widehat{a}_{1}$, $\widehat{a}_{2}$ and $\widehat{a} _{3}$. Since $\left \Vert \widehat{\mathbf{t}}\right \Vert ^{2} = 1$, we get $\widehat{a}_{3} = 0$, and $\widehat{a}_{1}^{2}+\widehat{a}_{2}^{2} = 1$. It follows that $\mathbf{x}(\widehat{u})$ is given by

where $\widehat{b}_{1}$ and $\widehat{b}_{3}$ are dual constants satisfying $\widehat{a}_{1}\widehat{b}_{2}+\widehat{a}_{2}\widehat{b}_{2} = 0$. We can change the coordinates by

Then $\widehat{\mathbf{x}}(\widehat{u})$ turns into

for a dual constant $\widehat{a}_{2}\widehat{b}_{1}+\widehat{a}_{1}\widehat{b }_{2} = \cos \widehat{\vartheta }$. This means that $\vartheta = c_{1}(real\; const.)$, and$\; \vartheta ^{\ast } = c_{1}^{\ast }(real\; const.)$. Here, $\widehat{\varphi } = \varphi +\varepsilon \varphi ^{\ast }$ is the dual angle among the projection of $\widehat{\mathbf{x}}$ on the plane $Sp\{ \widehat{\boldsymbol{\zeta }}_{2}, \widehat{\boldsymbol{\zeta }}_{3}\}$. This shows that a helical movement of angle $\varphi$ on the $\mathbb{ISA}$ and distance $\varphi ^{\ast }$ over it turns $\widehat{\boldsymbol{\zeta }}_{3}$ to be the central normal $\widehat{\mathbf{t}}$ of $\widehat{\mathbf{x}}$ (Figure 2). According to Eqs (3.11) and (3.28) we instantly find that:

Consequently, from Eqs (3.11) and (3.29) we get

where

4.   Plücker coordinates

For more analysis of the torsion line congruence, from Eq (3.28), the Plücker coordinates of $\widehat{\mathbf{x}}$ are:

Let $\eta (\eta _{1}, \eta _{2}, \eta _{3})$ indicate a point on $\widehat{\mathbf{x}}$. Since $\mathbf{x}^{\ast } = \eta \mathbf{ \times x}$ we have the system of linear equations in $\eta _{i}$ for i = 1, 2, 3 ($\eta _{is}$ are the coordinates of $\eta$):

The matrix of coefficients of unknowns $\eta _{i}$ is the skew symmetric matrix

and thus its rank is 2 with $\vartheta \neq 2\pi k$ ($k$ is an integer). The rank of the augmented matrix

is also 2. Thereby, this system has infinite solutions given by

Since $\eta _{1}$can be set arbitrarily, we may set $\eta _{1} = \varphi ^{\ast }$. In this case, Eq (4.3) reduces to

If we set $\varphi ^{\ast } = h\varphi$ and $\varphi$ as the movement parameter, then $(\widehat{x})$ is a ruled in $\mathbb{L}_{f}$-space. We now simply find the base curve as

It can be shown that $< η^{^{\prime }}, \mathbf{x}^{^{\prime }} > = 0$, ($^{\prime } = \frac{d}{d\varphi }$), so the base curve $η (\varphi)$ of ($\widehat{x}$) is its striction curve $\mathbf{c}$. The curvature $\kappa _{c}(\varphi)$ and torsion $\tau _{c}(\varphi)$ of $\mathbf{c}(\varphi)$ can be given by

which means that $\mathbf{c}(\varphi)$ is a circular helix. From Eqs (3.11), (3.29) and (4.5) it can be found that

Let $y (y_{1}, y_{2}, y_{3})$ be a point on the oriented line $\widehat{\mathbf{x}}$. Then,

The constants $h$, $\vartheta$ and $\vartheta ^{\ast }$ can control the shape of the surface $(\widehat{x})$. Hence, the major geometrical characteristics of $(\widehat{x})$ can be described as follows: $(\widehat{x})$ is a stationary Disteli-axis ruled surface, $< \mathbf{c}^{^{\prime }}, \mathbf{x} > = \Gamma$ is constant, $< \mathbf{c}^{^{\prime }}, \mathbf{g} > = \lambda$ is constant, and $\mathbf{c}(\varphi)$ is a circular helix.

Theorem 2. Let $(\widehat{x})$ be any non-developable ruled surface in Euclidean 3-space $\mathbb{E}^{3}$. Then, $(\widehat{x})$ is a ruled Weingarten surface if and only if $(\widehat{x})$ is contained in a torsion line congruence.

However, via Eq (4.6), the ruled surface can be classified into four types according to the forms of their striction curves.

(1) Archimedes helicoid when its striction curve is a circular helix; for $h = 1, \vartheta ^{\ast } = 1, \vartheta = \frac{\pi }{4}$, $-2.5\leq v\leq 2.5$, and $0\leq \varphi \leq 2\pi$ (see Figure 3).

(2) One-sheeted hyperboloid when its striction curve is a circle; for $h = 0, \vartheta^{\ast } = 1, \vartheta = \frac{\pi }{4}, -2.5\leq \; v\leq 2.5$, and $0\leq \varphi \leq 2\pi$ (see Figure 4).

(3) Right helicoid when its striction curve is a line; for $h = 1$, $\vartheta ^{\ast } = 0$, $\vartheta = \frac{\pi }{2}$, $-2.5\leq v\leq 2.5$, and $0\leq \varphi \leq 2\pi$ (see Figure 5).

(4) Circular cone when its striction curve is a fixed point; for $h = \vartheta ^{\ast } = 0$, $\vartheta = \frac{\pi }{4}$, $-2.5\leq v\leq 2.5$, and $0\leq \varphi \leq 2\pi$ (see Figure 6).

5.   Straightforward equation of the torsion line congruence

In order to have a straightforward equation of the torsion line congruence, from Eqs (3.10), (3.13) and (3.28) we can write the equation

Hence, at any instant, it is apparent that

where

The real part of Eq (5.1) characterizes the torsion cone for the spherical part of the movement $\mathbb{L}_{m}/\mathbb{L}_{f}$ and is given by

The intersection of the torsion cone with a unit sphere fastened at the cone's apex gives a spherical curve. Linked with the direction of a line $L$ on the torsion cone, there is a plane $\pi$ defined by the dual part of Eq (5.1). This plane is

So, for each direction of a line $L$ of the torsion cone, there is an associated plane of lines parallel to $L$. The torsion cone and plane of lines defines the torsion line congruence. Hence, if Eq (5.3) is solved with respect to the angle $\vartheta$, then we obtain

Then, from Eqs (4.1) and (5.5) it follows that

From Eqs (4.6) and (5.5), we can also obtain that

In the case of $0\leq \varphi \leq 2\pi$, and $\vartheta ^{\ast }\neq 0$, we get:

where $\Lambda = \vartheta ^{\ast }\left(a\csc \varphi +b\sec \varphi \right)$, and $Y_{1} = y_{1}-h\varphi$. Then, $(\widehat{x})$ is a one-parameter family of one-sheeted hyperboloids. The intersection of each hyperboloid and the corresponding plane $y_{1} = \varphi ^{\ast }$ is one-parameter family of circular cylinders $(c)$: $y_{2}^{2}+y_{3}^{2} = \vartheta ^{\ast 2}$ which is the envelope of $(\widehat{x})$. According to Eqs (5.6) and (5.7) we have the following:

(1) Spherical torsion curve with its torsion ruled surface: for $a = 1, b = 0.5, \vartheta ^{\ast } = 3, h = 1, 0\leq \; \varphi \leq 2\pi, 0\leq v\leq 4$ (Figures 7 and 8).

(2) Spherical torsion curve with its torsion ruled surface: for $a = 1$, $b = -0.5$, $\vartheta ^{\ast } = 3$, $h = 1, 0\leq \; \varphi \leq 2\pi, 0\leq v\leq 4$ (Figures 9 and 10).

5.1.   Disteli formulae of a line trajectory

In 1914 Disteli^[9] created a curvature axis for a ruled surface and generalized the planar Euler-Savary equation to line trajectories. There are several papers which deal with Euler-Savary formulae and classical geometric invariants of ruled surfaces for several kinds of geometry^[4,5,6,7]. The Disteli formula may be given immediately as follows. From Eqs (3.10), (3.13) and (3.28), we obtain

Equation (5.9) shows the correlations between $\widehat{\mathbf{x}}(\widehat{u})\in \mathbb{K}_{f\text{, }}$ which corresponds to the ruled surface ($\widehat{x}$), and its osculating dual cone, which is corresponding to the Disteli-axis, at any instant. It is comparable to the Euler-Savary equation of a point trajectory in planar and spherical movements in form^[1,2,3,4,5]. By separating the real and the dual parts, we get

and

Equation (5.10) detects the correlations among the locations of the ruling $\widehat{\mathbf{x}}$ in the movable space $\mathbb{L}_{m}$ and the Disteli-axis $\widehat{\mathbf{b}}$. Equation (5.11) depicts the distance from $\widehat{\mathbf{x}}$ to the Disteli-axis $\widehat{\mathbf{b}}$. Their geometrical explanations are shown in Figure 2. The sign of $\phi ^{\ast }$(- or +) indicates that the location of the Disteli-axis $\widehat{\mathbf{b}}$ is on the negative or positive orientation of the central normal $\widehat{\mathbf{t}}$ of $(\widehat{x})$. Equations (5.10) and (5.11) are new Disteli formulae of a line trajectory in the movement $\mathbb{L}_{m}/ \mathbb{L}_{f}$. Since the central points of the ruled surfaces are on the normal plane, when the direction of their rulings is defined by the dual unit vector $\widehat{\mathbf{x}}$ with $(\widehat{\varphi }, \widehat{ \vartheta })$ according to Eq (3.28), the Disteli fomulae (5.10) and (5.11) can be displayed in the plane $\pi :Sp\{ \boldsymbol{\zeta }_{1}, \widehat{\mathbf{t}} \}$. Hence, any arbitrary point $\mathbf{c}(\varphi ^{\ast }, \vartheta ^{\ast })$ on the plane $\pi$ is defined as central point of $(\widehat{x})$ whose ruling is the oriented line $\widehat{\mathbf{x}}$, and the radius $\vartheta ^{\ast }$ is the line segment from the point $\mathbf{q}$ to the point $\mathbf{c}$ on the plane $\pi$. Also, the vector from $\mathbf{q}$ to $\mathbf{c}$ is in the positive (resp. negative) orientation of $\widehat{ \mathbf{t}}$ if $\vartheta ^{\ast } > 0$ (resp. $\vartheta ^{\ast } < 0$). The central point $\mathbf{c}(\varphi ^{\ast }, \vartheta ^{\ast })$ can be on the $\mathbb{ISA}$ if $\vartheta ^{\ast } = 0$ ($\alpha ^{\ast } = -\varphi ^{\ast })$ and on the Disteli-axis $\widehat{\mathbf{b}}$ if$\ \phi ^{\ast } = 0\Leftrightarrow \alpha ^{\ast } = \vartheta ^{\ast }$. In the latter case the central point $\mathbf{c}(\varphi ^{\ast }, \vartheta ^{\ast })$ can be determined by setting $\phi ^{\ast } = 0$ in Eq (5.11) which is clarified as

Equation (5.12) is linear in the position coordinates $\varphi ^{\ast }$and $\vartheta ^{\ast }$ of $\widehat{\mathbf{x}}$. Hence, in one-parameter spatial movement $\mathbb{L}_{m}/\mathbb{L}_{f}$ the fixed lines in $\mathbb{ L}_{m}$ lie on a plane. The line $L$ will change its location if the parameter $\vartheta ^{\ast }$ is defined as a varying value, but $\varphi ^{\ast } =$constant. However, a set of lines envelops a curve on the plane $\pi$. Meanwhile, the $\pi$ has several positions if the parameter $\varphi ^{\ast }$ of a line has several values, but $\vartheta ^{\ast } =$constant. Therefore, the set of all lines $L$ defined by Eq (5.12) is a line congruence for all values of $(\varphi ^{\ast }, \vartheta ^{\ast })$.

On the other hand, we can derive other dual version of the Euler-Savary equation as follows: Substituting Eq (3.5) into Eq (3.21), we have

Thus, from Eqs (3.1), (3.30) and (5.13), one finds that:

Substituting Eq (3.28) into Eq (5.14), we obtain

This is exactly a second dual extension of the Euler-Savary equation. From the real and the dual parts, respectively, we get:

and

Once the angles $\alpha$ and $\vartheta$ are known, Eq (5.17) gives the correspondence between $\alpha ^{\ast }$ and $\vartheta ^{\ast }$ in terms of ($\varphi$, $\varphi ^{\ast }$) and the 2nd order invariant $\frac{\omega }{ \sin \varphi }\left(h-\mu \right)$. The spherical Euler-Savary Eq (5.16) and (5.17) are new Disteli formulae of spatial kinematics.

6.   Conclusions

For one-parameter spatial movement $\mathbb{L}_{m}/\mathbb{L}_{f}$, based on E. Study, expressions of the axodes and their invariants were derived, and then the geometric–kinematic meanings were revealed. By employing the Blaschke frames, the properties of a line trajectory and its Disteli axis were researched. Interestingly, the results slightly explain the analogies between point geometry of spherical curves in Euclidean 3-space $\mathbb{E}^{3}$ and point geometry of dual spherical curves in dual 3-space $\mathbb{D}^{3}$. Hence, the invariants of the axodes were utilized for obtaining new proof of the Disteli-formulae for a line trajectory in spatial kinematics.

The study of spatial kinematics in Euclidean 3-space $\mathbb{E}^{3}$ via the geometry of lines may be adopted to research some problems and conclude new applications. For future research, we will attract with the design of ruled surfaces as tooth flanks for gears with skew axes, as offered in ^[7].

Conflict of interest

The authors declare that there is no conflict of interests regarding the publication of this paper.