2-complex symmetric weighted composition operators on Fock space

Hong-bin Bai; Zhi-jie Jiang; Xiao-bo Hu; Zuo-an Li; Hong-bin Bai; Zhi-jie Jiang; Xiao-bo Hu; Zuo-an Li

doi:10.3934/math.20231111

AIMS Mathematics

2023, Volume 8, Issue 9: 21781-21792. doi: 10.3934/math.20231111

Previous Article Next Article

Research article

2-complex symmetric weighted composition operators on Fock space

1.
School of Mathematics and Statistics, Sichuan University of Science and Engineering, Zigong, Sichuan, 643000, China
2.
South Sichuan Center for Applied Mathematics, Sichuan University of Science and Engineering, Zigong, Sichuan, 643000, China
3.
Department of Normal Education, Meishan Vocational and Technical College, Meishan, Sichuan, 620010, China

Received: 23 April 2023 Revised: 19 June 2023 Accepted: 29 June 2023 Published: 10 July 2023
MSC : 30H10, 47B38

The aim of the present paper is to completely characterize 2-complex symmetric weighted composition operators $W_{e^{\overline{p}z, az+b}}$ with the conjugations $C$ and $C_{r, s, t}$ defined by $Cf(z) = \overline{f(\bar{z})}$ and $C_{r, s, t}f(z) = te^{sz}\overline{f(\overline{rz+s})}$ on Fock space by building the relations between the parameters $a$ , $b$ , $p$ , $r$ , $s$ and $t$ . Some examples of such operators are also given.

Keywords:

Citation: Hong-bin Bai, Zhi-jie Jiang, Xiao-bo Hu, Zuo-an Li. 2-complex symmetric weighted composition operators on Fock space[J]. AIMS Mathematics, 2023, 8(9): 21781-21792. doi: 10.3934/math.20231111

Related Papers:

[1]	Meshari Alesemi . Innovative approaches of a time-fractional system of Boussinesq equations within a Mohand transform. AIMS Mathematics, 2024, 9(10): 29269-29295. doi: 10.3934/math.20241419
[2]	Azzh Saad Alshehry, Humaira Yasmin, Ali M. Mahnashi . Exploring fractional Advection-Dispersion equations with computational methods: Caputo operator and Mohand techniques. AIMS Mathematics, 2025, 10(1): 234-269. doi: 10.3934/math.2025012
[3]	Shabir Ahmad, Aman Ullah, Ali Akgül, Fahd Jarad . A hybrid analytical technique for solving nonlinear fractional order PDEs of power law kernel: Application to KdV and Fornberg-Witham equations. AIMS Mathematics, 2022, 7(5): 9389-9404. doi: 10.3934/math.2022521
[4]	Abdul Samad, Imran Siddique, Fahd Jarad . Meshfree numerical integration for some challenging multi-term fractional order PDEs. AIMS Mathematics, 2022, 7(8): 14249-14269. doi: 10.3934/math.2022785
[5]	Mamta Kapoor, Nehad Ali Shah, Wajaree Weera . Analytical solution of time-fractional Schrödinger equations via Shehu Adomian Decomposition Method. AIMS Mathematics, 2022, 7(10): 19562-19596. doi: 10.3934/math.20221074
[6]	Abdul Samad, Imran Siddique, Zareen A. Khan . Meshfree numerical approach for some time-space dependent order partial differential equations in porous media. AIMS Mathematics, 2023, 8(6): 13162-13180. doi: 10.3934/math.2023665
[7]	M. S. Alqurashi, Saima Rashid, Bushra Kanwal, Fahd Jarad, S. K. Elagan . A novel formulation of the fuzzy hybrid transform for dealing nonlinear partial differential equations via fuzzy fractional derivative involving general order. AIMS Mathematics, 2022, 7(8): 14946-14974. doi: 10.3934/math.2022819
[8]	Asif Khan, Tayyaba Akram, Arshad Khan, Shabir Ahmad, Kamsing Nonlaopon . Investigation of time fractional nonlinear KdV-Burgers equation under fractional operators with nonsingular kernels. AIMS Mathematics, 2023, 8(1): 1251-1268. doi: 10.3934/math.2023063
[9]	Muhammad Imran Liaqat, Sina Etemad, Shahram Rezapour, Choonkil Park . A novel analytical Aboodh residual power series method for solving linear and nonlinear time-fractional partial differential equations with variable coefficients. AIMS Mathematics, 2022, 7(9): 16917-16948. doi: 10.3934/math.2022929
[10]	Aliaa Burqan, Mohammed Shqair, Ahmad El-Ajou, Sherif M. E. Ismaeel, Zeyad AlZhour . Analytical solutions to the coupled fractional neutron diffusion equations with delayed neutrons system using Laplace transform method. AIMS Mathematics, 2023, 8(8): 19297-19312. doi: 10.3934/math.2023984

Abstract

1. Introduction

Mathematical methods have been used to represent a variety of real-world issues. For example, we can approximate a body's speed for a given distance and time by using the concept of rate of change. Specifically, we use the notion of differential calculus. Many complicated phenomena, such as chaos, solitons, asymptotic properties, singular formation, and others, are either poorly projected or have not yet been discovered ^[1,2,3]. Furthermore, the notion of calculus with differential operators and integrals is essential for describing physical phenomena and divining natural events connected to variation and changes. However, while researching issues with hereditary characteristics or memory, numerous researchers found numerous shortcomings and restrictions in integer-order calculus ^[4,5]. Later, new operators defined with the aid of fractional-order were proposed by mathematicians and physicists. Many researchers are drawn to fractional calculus (FC) while looking at different models ^[6,7,8].

In many branches of physical science and engineering, fractional calculus, which deals with arbitrary order derivatives and integrals, is crucial ^[9]. Fractional calculus and its applications have developed rapidly in the last few years ^[10,11]. Significant issues in acoustics, fluid mechanics, electromagnetic, analytical chemistry, signal processing, biology, and many other engineering and physical science branches are modeled by nonlinear and linear partial differential equations (PDEs) ^[12]. Both nonlinear and linear FDEs have been solved analytically and numerically in recent years using a variety of techniques, including the Yang-Laplace transform ^[13], the Adomian decomposition method ^[14], the homotopy analysis method ^[15], and the Laplace decomposition method ^[16]. Furthermore, nonlinear and linear FDEs are also subjected to the local fractional variational iteration approach ^[17,18], the fractional complex transform method ^[19], the modified Laplace decomposition approach ^[16,20], and the cylindrical-coordinate method ^[21].

Mathematical models known as fractional partial differential equations (FPDEs) depict physical processes that exhibit complicated dynamics and non-local effects. FPDEs represent an expansion of the traditional theory of partial differential equations. They enable non-integer orders of differentiation, which more accurately capture the non-local and nonlinear characteristics of an extensive range of physical systems ^[22,23]. These formulas are being used more and more frequently in several fields, such as biology, engineering, economics, and physics. These formulas are valuable resources for constructing intricate systems and examining the behavior of those operations. This article explores the concept of fractional-order partial differential equations, their applications, and the challenges associated with their analysis and numerical solution ^[24,25,26].

In 2013, Al-Smadi proposed the Residual Power Series Method (RPSM) ^[27]. It is generated from the residual error function mixed with the Taylor series. The solution to the problem is an infinite convergence series ^[28,29,30]. Many DEs have inspired fresh RPSM algorithms ^[31,32,33]. Among these DEs are several Boussinesq DEs, fuzzy DEs, and KdV Burger's equation, among many others. These systems are built to generate exact and efficient approximations. We provide a technique to investigate the approximation of solutions to fractional PDEs and systems of PDEs using RPSM in the Mohand transform (MT) formulation. The computational series finds the exact solution after a few iterations ^{[34,35,36,37]}.

The computational complexity and effort necessary to implement the methods that were previously discussed are among the most significant constraints. The Mohand distinguishes our work transform iterative methodology (MTIM), which we developed as an iterative approach to addressing fractional PDEs and systems of PDEs. This technique is highly effective in reducing the amount of computational work and complexity necessary due to integrating the MT with the new iterative process.

In this study, the Mohand residual power series method (MRPSM) and MTIM are used to solve fractional PDEs and systems of PDEs. The numerical values produced by these techniques are more precise when compared to those of other numerical procedures. This study includes a comparison study of the numerical data. A strong indicator of the efficacy and reliability of these methods is the fact that the results of the many approaches presented are compatible with one another. The attractiveness of fractional-order derivatives grows in direct correlation with their worth. Because of this, the algorithms can withstand spikes in computational error, are easy to use, and are quick and accurate. Discovering this will make solving many partial differential equations much easier for mathematicians.

2. Mohand transformation

The portions that follow will cover the basic elements and ideas of the MT, providing the foundation for this operation.

Definition 2.1. The MT of the function $\mathfrak{P}(\mathfrak{t})$ is defined as ^[38]

$M[\mathfrak{P}(\mathfrak{t})] = R(s) = s^2\displaystyle{\int}_{0}^{\mathfrak{t}}\mathfrak{P}(\mathfrak{t})e^{-s\mathfrak{t}}d{\mathfrak{t}},\ \ {k}_{1}\leq s \leq{k}_{2}.$

The inverse Mohand transform (IMT) is defined as

$M^{-1}[R(s)] = \mathfrak{P}(\mathfrak{t}).$

Definition 2.2. (^[39]). The derivative of fractional-order in the framework of MT is defined as

$M[\mathfrak{P}^{\mathfrak{F}}(\mathfrak{t})] = s^{\mathfrak{F}}R(s)-\sum\limits_{k = 0}^{n-1}\frac{\mathfrak{P}^{k}(0)}{s^{k-(\mathfrak{F}+1)}},\ 0 < \mathfrak{F}\leq n.$

Definition 2.3. The properties of MT are given as follows:

(1) $M[\mathfrak{P}'(\mathfrak{t})] = sR(s)-s^{2}R(0)$ .

(2) $M[\mathfrak{P}''(\mathfrak{t})] = s^{2}R(s)-s^{3}R(0)-s^{2}R'(0)$ .

(3) $M[\mathfrak{P}^{n}(\mathfrak{t})] = s^{n}R(s)-s^{n+1}R(0)-s^{n}R'(0)-\cdots-s^{n}R^{n-1}(0)$ .

Lemma 2.4. Suppose there exists a function represented by $\mathfrak{P}({{\mathfrak{R}}}, {{\mathfrak{t}}})$ , having exponential order. $M[R(s)] = \mathfrak{P}({{\mathfrak{R}}}, {{\mathfrak{t}}})$ denotes the MT in this case:

$\begin{equation} M[D_{{{\mathfrak{t}}}}^{r{\mathfrak{F}}}\mathfrak{P}({{\mathfrak{R}}}, {{\mathfrak{t}}})] = {s}^{r{\mathfrak{F}}}R(s)-\sum\limits_{j = 0}^{r-1}{s}^{{\mathfrak{F}}(r-j)-1}D_{{{\mathfrak{t}}}}^{j{\mathfrak{F}}}\mathfrak{P}({{\mathfrak{R}}},0), 0 < {\mathfrak{F}}\leq 1, \end{equation}$

(2.1)

where ${{\mathfrak{R}}} = ({{\mathfrak{R}}}_{1}, {{\mathfrak{R}}}_{2}, \cdots, {{\mathfrak{R}}}_{\mathfrak{F}})\in \mathbb{R}^{\mathfrak{F}}$ , $\mathfrak{F}\in \mathbb{N}$ , and $D_{{{\mathfrak{t}}}}^{r{\mathfrak{F}}} = D_{{{\mathfrak{t}}}}^{{\mathfrak{F}}}.D_{{{\mathfrak{t}}}}^{{\mathfrak{F}}}.\cdots.D_{{{\mathfrak{t}}}}^{{\mathfrak{F}}}(r-times)$ .

Proof. To validate Eq (2.4), we use the induction method. Taking $r = 1$ in Eq (2.4):

$M[D_{{{\mathfrak{t}}}}^{2{\mathfrak{F}}}\mathfrak{P}({{\mathfrak{R}}}, {{\mathfrak{t}}})] = {s}^{2{\mathfrak{F}}}R(s)-{s}^{2{\mathfrak{F}}-1}\mathfrak{P}({{\mathfrak{R}}},0)-{s}^{{\mathfrak{F}}-1}D_{{{\mathfrak{t}}}}^{{\mathfrak{F}}}\mathfrak{P}({{\mathfrak{R}}},0).$

Equation (2.4) is true for $r = 1$ on the basis of Definition 2.2. Now, put $r = 2$ in Eq (2.4) to obtain the following outcome:

$\begin{equation} M[D_{r}^{2{\mathfrak{F}}}\mathfrak{P}({{\mathfrak{R}}}, {{\mathfrak{t}}})] = {s}^{2{\mathfrak{F}}}R(s)-{s}^{2{\mathfrak{F}}-1}\mathfrak{P}({{\mathfrak{R}}},0)-{s}^{{\mathfrak{F}}-1}D_{{{\mathfrak{t}}}}^{{\mathfrak{F}}}\mathfrak{P}({{\mathfrak{R}}},0). \end{equation}$

(2.2)

We obtain the next result from the LHS of Eq (2.2):

$\begin{equation} L.H.S = M[D_{{{\mathfrak{t}}}}^{2{\mathfrak{F}}}\mathfrak{P}({{\mathfrak{R}}}, {{\mathfrak{t}}})]. \end{equation}$

(2.3)

We may also write Eq (2.3) as

$\begin{equation} L.H.S = M[D_{{{\mathfrak{t}}}}^{{\mathfrak{F}}}D_{{{\mathfrak{t}}}}^{{\mathfrak{F}}}\mathfrak{P}({{\mathfrak{R}}}, {{\mathfrak{t}}})]. \end{equation}$

(2.4)

Assume

$\begin{equation} z({{\mathfrak{R}}}, {{\mathfrak{t}}}) = D_{{{\mathfrak{t}}}}^{{\mathfrak{F}}}\mathfrak{P}({{\mathfrak{R}}}, {{\mathfrak{t}}}). \end{equation}$

(2.5)

Putting Eq (2.5) in Eq (2.4),

$\begin{equation} L.H.S = M[D_{{{\mathfrak{t}}}}^{{\mathfrak{F}}}z({{\mathfrak{R}}}, {{\mathfrak{t}}})]. \end{equation}$

(2.6)

Using the derivative of Caputo, Eq (2.6) becomes

$\begin{equation} L.H.S = M[J^{1-{\mathfrak{F}}}z^{'}({{\mathfrak{R}}}, {{\mathfrak{t}}})]. \end{equation}$

(2.7)

Applying the RL integral on Eq (2.7),

$\begin{equation} L.H.S = \frac{M[z^{'}({{\mathfrak{R}}}, {{\mathfrak{t}}})]}{{s}^{1-{\mathfrak{F}}}}. \end{equation}$

(2.8)

The derivative property of MT is applied on Eq (2.8) to obtain the following result:

$\begin{equation} L.H.S = {s}^{{\mathfrak{F}}}Z({{\mathfrak{R}}},{s})-\frac{z({{\mathfrak{R}}},0)}{{s}^{1-{\mathfrak{F}}}}. \end{equation}$

(2.9)

Using Eq (2.5), we obtain

$Z({{\mathfrak{R}}},{s}) = {s}^{{\mathfrak{F}}}R(s)-\frac{\mathfrak{P}({{\mathfrak{R}}},0)}{{s}^{1-{\mathfrak{F}}}}.$

As $M[z({{\mathfrak{t}}}, {{\mathfrak{R}}})] = Z({{\mathfrak{R}}}, {s})$ , we can write Eq (2.9) as

$\begin{equation} L.H.S = {s}^{2{\mathfrak{F}}}R(s)-\frac{\mathfrak{P}({{\mathfrak{R}}},0)}{{s}^{1-2{\mathfrak{F}}}}-\frac{D_{{{\mathfrak{t}}}}^{{\mathfrak{F}}}\mathfrak{P}({{\mathfrak{R}}},0)}{{s}^{1-{\mathfrak{F}}}}. \end{equation}$

(2.10)

Assume that Eq (2.4) is true for $r = K$ . Taking $r = K$ in Eq (2.4),

$\begin{equation} M[D_{{{\mathfrak{t}}}}^{K{\mathfrak{F}}}\mathfrak{P}({{\mathfrak{R}}}, {{\mathfrak{t}}})] = {s}^{K{\mathfrak{F}}}R(s)-\sum\limits_{j = 0}^{K-1}{s}^{{\mathfrak{F}}(K-j)-1}D_{{{\mathfrak{t}}}}^{j{\mathfrak{F}}}D_{{{\mathfrak{t}}}}^{j{\mathfrak{F}}}\mathfrak{P}({{\mathfrak{R}}},0),\ 0 < {\mathfrak{F}}\leq1. \end{equation}$

(2.11)

Next, we will have to show that Eq (2.4) for $r = K+1$ holds. Taking $r = K+1$ in Eq (2.4),

$\begin{equation} M[D_{{{\mathfrak{t}}}}^{(K+1){\mathfrak{F}}}\mathfrak{P}({{\mathfrak{R}}}, {{\mathfrak{t}}})] = {s}^{(K+1){\mathfrak{F}}}R(s)-\sum\limits_{j = 0}^{K}{s}^{{\mathfrak{F}}((K+1)-j)-1}D_{{{\mathfrak{t}}}}^{j{\mathfrak{F}}}\mathfrak{P}({{\mathfrak{R}}},0). \end{equation}$

(2.12)

From the left-hand side of Eq (2.12), we derive

$\begin{equation} L.H.S = M[D_{{{\mathfrak{t}}}}^{K{\mathfrak{F}}}(D_{{{\mathfrak{t}}}}^{K{\mathfrak{F}}})]. \end{equation}$

(2.13)

Letting $D_{{{\mathfrak{t}}}}^{K{\mathfrak{F}}} = g({{\mathfrak{R}}}, {{\mathfrak{t}}}),$ Eq (2.13) gives us

$\begin{equation} L.H.S = M[D_{{{\mathfrak{t}}}}^{{\mathfrak{F}}}g({{\mathfrak{R}}}, {{\mathfrak{t}}})]. \end{equation}$

(2.14)

Applying Caputo's derivative and the RL integral on Eq (2.14),

$\begin{equation} L.H.S = {s}^{{\mathfrak{F}}}M[D_{{{\mathfrak{t}}}}^{K{\mathfrak{F}}}\mathfrak{P}({{\mathfrak{R}}}, {{\mathfrak{t}}})]-\frac{g({{\mathfrak{R}}},0)}{{s}^{1-{\mathfrak{F}}}}. \end{equation}$

(2.15)

On the basis of Eq (2.11), we can write Eq (2.15) as

$\begin{equation} L.H.S = {s}^{r{\mathfrak{F}}}R(s)-\sum\limits_{j = 0}^{r-1}{s}^{{\mathfrak{F}}(r-j)-1}D_{{{\mathfrak{t}}}}^{j{\mathfrak{F}}}\mathfrak{P}({{\mathfrak{R}}},0). \end{equation}$

(2.16)

Equation (2.16) can also be written as

$L.H.S = M[D_{{{\mathfrak{t}}}}^{r{\mathfrak{F}}}\mathfrak{P}({{\mathfrak{R}}},0)].$

Using mathematical induction, Eq (2.4) is true for $r = K+1$ . Hence, it is proved that for all positive integers, Eq (2.4) holds. □

Lemma 2.5. Let assume that there exists an exponential-order function $\mathfrak{P}({{\mathfrak{R}}}, {{\mathfrak{t}}})$ . $M[\mathfrak{P}({{\mathfrak{R}}}, {{\mathfrak{t}}})] = R(s)$ denotes the MT of $\mathfrak{P}({{\mathfrak{R}}}, {{\mathfrak{t}}})$ . The multiple fractional power series (MFPS) in MT is given as

$\begin{equation} R(s) = \sum\limits_{r = 0}^{\infty}\frac{\hbar_{r}({{\mathfrak{R}}})}{s^{r{\mathfrak{F}}+1}}, s > 0, \end{equation}$

(2.17)

where, ${{\mathfrak{R}}} = (s_{1}, {{\mathfrak{R}}}_{2}, \cdots, {{\mathfrak{R}}}_{\mathfrak{F}})\in\mathbb{R}^{\mathfrak{F}}, \ \mathfrak{F}\in \mathbb{N}$ .

Proof. Let us consider the Taylor series

$\begin{equation} \mathfrak{P}({{\mathfrak{R}}}, {{\mathfrak{t}}}) = \hbar_{0}({{\mathfrak{R}}})+\hbar_{1}({{\mathfrak{R}}})\frac{{{\mathfrak{t}}}^{{\mathfrak{F}}}}{\Gamma[{\mathfrak{F}}+1]}++\hbar_{2}({{\mathfrak{R}}})\frac{{{\mathfrak{t}}}^{2{\mathfrak{F}}}}{\Gamma[2{\mathfrak{F}}+1]}+\cdots. \end{equation}$

(2.18)

MT is subjected to Eq (2.18) to obtain

$M\left[\mathfrak{P}({{\mathfrak{R}}}, {{\mathfrak{t}}})\right] = M\left[\hbar_{0}({{\mathfrak{R}}})\right]+M\left[\hbar_{1}({{\mathfrak{R}}})\frac{{{\mathfrak{t}}}^{{\mathfrak{F}}}}{\Gamma[{\mathfrak{F}}+1]}\right]+M\left[\hbar_{1}({{\mathfrak{R}}}) \frac{{{\mathfrak{t}}}^{2{\mathfrak{F}}}}{\Gamma[2{\mathfrak{F}}+1]}\right]+\cdots.$

Utilizing the features of MT, we derive

$M\left[\mathfrak{P}({{\mathfrak{R}}}, {{\mathfrak{t}}})\right] = \hbar_{0}({{\mathfrak{R}}})\frac{1}{s}+\hbar_{1}({{\mathfrak{R}}})\frac{\Gamma[{\mathfrak{F}}+1]}{\Gamma[{\mathfrak{F}}+1]}\frac{1}{s^{{\mathfrak{F}}+1}}+\hbar_{2}({{\mathfrak{R}}}) \frac{\Gamma[2{\mathfrak{F}}+1]}{\Gamma[2{\mathfrak{F}}+1]}\frac{1}{s^{2{\mathfrak{F}}+1}}\cdots.$

Thus, a new Taylor series form is obtained. □

Lemma 2.6. If $M[\mathfrak{P}(\mathfrak{R}, \mathfrak{t})] = R(s)$ denotes MT, then the new Taylor series form in MFPS is given as

$\begin{equation} \hbar_{0}({{\mathfrak{R}}}) = \lim\limits_{s\rightarrow \infty}sR(s) = \mathfrak{P}({{\mathfrak{R}}},0). \end{equation}$

(2.19)

Proof. Assume the Taylor's series

$\begin{equation} \hbar_{0}({{\mathfrak{R}}}) = sR(s)-\frac{\hbar_{1}({{\mathfrak{R}}})}{s^{{\mathfrak{F}}}}-\frac{\hbar_{2}({{\mathfrak{R}}})}{s^{2{\mathfrak{F}}}}-\cdots. \end{equation}$

(2.20)

When the limit in Eq (2.19) is calculated and simplified, we get Eq (2.20). □

3. Mohand residual power series method

In this part, we construct the framework of the proposed method for the solution of fractional PDEs.

Step 1. Let us assume the fractional PDE

$\begin{equation} D_{{{\mathfrak{t}}}}^{{\mathfrak{F}}}\mathfrak{P}({{\mathfrak{R}}}, {{\mathfrak{t}}})+\mathfrak{K}({{\mathfrak{R}}})N(\mathfrak{P})-\delta({{\mathfrak{R}}},\mathfrak{P}) = 0. \end{equation}$

(3.1)

Step 2. Applying the MT on both sides of Eq (3.1),

$\begin{equation} M[D_{{{\mathfrak{t}}}}^{{\mathfrak{F}}}\mathfrak{P}({{\mathfrak{R}}}, {{\mathfrak{t}}})+\mathfrak{K}({{\mathfrak{R}}})N(\mathfrak{P})-\delta({{\mathfrak{R}}},\mathfrak{P})] = 0. \end{equation}$

(3.2)

On the basis of Lemma 2.4, we derive

$\begin{equation} R(s) = \sum\limits_{j = 0}^{q-1}\frac{D_{{{\mathfrak{t}}}}^{j}\mathfrak{P}({{\mathfrak{R}}},0)}{{s}^{j{\mathfrak{F}}+1}}-\frac{\mathfrak{K}({{\mathfrak{R}}})Y({s})}{{s}^{j{\mathfrak{F}}}}+\frac{F({{\mathfrak{R}}},{s})}{{s}^{j{\mathfrak{F}}}}, \end{equation}$

(3.3)

where, $M[\delta({{\mathfrak{R}}}, \mathfrak{P})] = F({{\mathfrak{R}}}, {s}), M[N(\mathfrak{P})] = Y({s})$ .

Step 3. The subsequent result is derived from Eq (3.3):

$R(s) = \sum\limits_{r = 0}^{\infty}\frac{\hbar_r({{\mathfrak{R}}})}{{s}^{r{\mathfrak{F}}+1}}, \ {s} > 0.$

Step 4. To obtain series form solution use the following procedure step by step:

$\hbar_0({{\mathfrak{R}}}) = \lim\limits_{{s}\rightarrow \infty}{s} R(s) = \mathfrak{P}({{\mathfrak{R}}}, 0).$

Subsequently, we obtain

$\begin{array}{*{20}{c}} {\hbar_{1}({{\mathfrak{R}}}) = D_{{{\mathfrak{t}}}}^{{\mathfrak{F}}}\mathfrak{P}({{\mathfrak{R}}}, 0),}\\ {\hbar_{2}({{\mathfrak{R}}}) = D_{{{\mathfrak{t}}}}^{2{\mathfrak{F}}}\mathfrak{P}({{\mathfrak{R}}}, 0),}\\ {\vdots}\\ {\hbar_{w}({{\mathfrak{R}}}) = D_{{{\mathfrak{t}}}}^{w{\mathfrak{F}}}\mathfrak{P}({{\mathfrak{R}}}, 0).} \end{array}$

Step 5. To obtain $R(s)$ as a $K^{th}$ truncated series, we use

$R_{K}(s) = \sum\limits_{r = 0}^{K}\frac{\hbar_r({{\mathfrak{R}}})}{{s}^{r{\mathfrak{F}}+1}}, \ {s} > 0,$

$R_{K}(s) = \frac{\hbar_{0}({{\mathfrak{R}}})}{{s}}+\frac{\hbar_{1}({{\mathfrak{R}}})}{{s}^{{\mathfrak{F}}+1}}+\cdots+\frac{\hbar_{w}({{\mathfrak{R}}})}{{s}^{w{\mathfrak{F}}+1}}+\sum\limits_{r = w+1}^{K} \frac{\hbar_r({{\mathfrak{R}}})}{{s}^{r{\mathfrak{F}}+1}}.$

Step 6. The Mohand residual function (MRF) from (3.3) is solved separately from the $K^{th}$ -truncated Mohand residual function

$MRes({{\mathfrak{R}}}, {s}) = R(s)-\sum\limits_{j = 0}^{q-1}\frac{D_{{{\mathfrak{t}}}}^{j}\mathfrak{P}({{\mathfrak{R}}},0)}{{s}^{j{\mathfrak{F}}+1}}+\frac{\mathfrak{K}({{\mathfrak{R}}})Y({s})}{{s}^{j{\mathfrak{F}}}}-\frac{F({{\mathfrak{R}}},{s})}{{s}^{j{\mathfrak{F}}}},$

and

$\begin{equation} MRes_{K}({{\mathfrak{R}}}, {s}) = R_{K}(s)-\sum\limits_{j = 0}^{q-1}\frac{D_{{{\mathfrak{t}}}}^{j}\mathfrak{P}({{\mathfrak{R}}},0)}{{s}^{j{\mathfrak{F}}+1}}+\frac{\mathfrak{K}({{\mathfrak{R}}})Y({s})}{{s}^{j{\mathfrak{F}}}}-\frac{F({{\mathfrak{R}}},{s})}{{s}^{j{\mathfrak{F}}}}. \end{equation}$

(3.4)

Step 7. In Eq (3.4), use $R_{K}(s)$ in place of its expansion form:

$\begin{equation} \begin{split} MRes_{K}({{\mathfrak{R}}}, {s})& = \Big(\frac{\hbar_{0}({{\mathfrak{R}}})}{{s}}+\frac{\hbar_{1}({{\mathfrak{R}}})}{{s}^{{\mathfrak{F}}+1}}+\cdots+\frac{\hbar_{w}({{\mathfrak{R}}})}{{s}^{w{\mathfrak{F}}+1}}+\sum\limits_{r = w+1}^{K} \frac{\hbar_r({{\mathfrak{R}}})}{{s}^{r{\mathfrak{F}}+1}}\Big)\\&-\sum\limits_{j = 0}^{q-1}\frac{D_{{{\mathfrak{t}}}}^{j}\mathfrak{P}({{\mathfrak{R}}},0)}{{s}^{j{\mathfrak{F}}+1}}+\frac{\mathfrak{K}({{\mathfrak{R}}})Y({s})}{{s}^{j{\mathfrak{F}}}} -\frac{F({{\mathfrak{R}}},{s})}{{s}^{j{\mathfrak{F}}}}. \end{split} \end{equation}$

(3.5)

Step 8. Multiplying ${s}^{K{\mathfrak{F}}+1}$ with Eq (3.5),

$\begin{equation} \begin{split} {s}^{K{\mathfrak{F}}+1}MRes_{K}({{\mathfrak{R}}}, {s}) & = {s}^{K{\mathfrak{F}}+1}\Big(\frac{\hbar_{0}({{\mathfrak{R}}})}{{s}}+\frac{\hbar_{1}({{\mathfrak{R}}})}{{s}^{{\mathfrak{F}}+1}}+\cdots+\frac{\hbar_{w}({{\mathfrak{R}}})}{{s}^{w{\mathfrak{F}}+1}}+\sum\limits_{r = w+1}^{K} \frac{\hbar_r({{\mathfrak{R}}})}{{s}^{r{\mathfrak{F}}+1}}\\&-\sum\limits_{j = 0}^{q-1}\frac{D_{{{\mathfrak{t}}}}^{j}\mathfrak{P}({{\mathfrak{R}}},0)}{{s}^{j{\mathfrak{F}}+1}}+\frac{\mathfrak{K}({{\mathfrak{R}}})Y({s})}{{s}^{j{\mathfrak{F}}}} -\frac{F({{\mathfrak{R}}},{s})}{{s}^{j{\mathfrak{F}}}}\Big). \end{split} \end{equation}$

(3.6)

Step 9. Taking the limit ${s}\rightarrow \infty$ of Eq (3.6),

$\begin{equation*} \begin{split} \lim\limits_{{s}\rightarrow \infty}{s}^{K{\mathfrak{F}}+1}MRes_{K}({{\mathfrak{R}}}, {s})& = \lim\limits_{{s}\rightarrow \infty}{s}^{K{\mathfrak{F}}+1}\Big(\frac{\hbar_{0}({{\mathfrak{R}}})}{{s}}+\frac{\hbar_{1}({{\mathfrak{R}}})}{{s}^{{\mathfrak{F}}+1}}+\cdots+\frac{\hbar_{w}({{\mathfrak{R}}})}{{s}^{w{\mathfrak{F}}+1}}+\sum\limits_{r = w+1}^{K} \frac{\hbar_r({{\mathfrak{R}}})}{{s}^{r{\mathfrak{F}}+1}}\\&-\sum\limits_{j = 0}^{q-1}\frac{D_{{{\mathfrak{t}}}}^{j}\mathfrak{P}({{\mathfrak{R}}},0)}{{s}^{j{\mathfrak{F}}+1}}+\frac{\mathfrak{K}({{\mathfrak{R}}})Y({s})}{{s}^{j{\mathfrak{F}}}} -\frac{F({{\mathfrak{R}}},{s})}{{s}^{j{\mathfrak{F}}}}\Big). \end{split} \end{equation*}$

Step 10. The values of $\hbar_K({{\mathfrak{R}}})$ are obtained by solving the following expression:

$\lim\limits_{{s}\rightarrow \infty}({s}^{K{\mathfrak{F}}+1}MRes_{K}({{\mathfrak{R}}}, {s})) = 0,$

where $K = 1+w, 2+w, \cdots.$

Step 11. Put $\hbar_K({{\mathfrak{R}}})$ in Eq (3.3).

Step 12. To determine the required solution, take IMT to obtain $R_{K}(s)$ as $\mathfrak{P}_K({{\mathfrak{R}}}, {{\mathfrak{t}}})$ .

Mohand transform iterative method

Suppose the PDE

$\begin{equation} D_{{{\mathfrak{t}}}}^{{\mathfrak{F}}}{{\mathfrak{P}}}({{\mathfrak{R}}},{{\mathfrak{t}}}) = \Upsilon\Big({{\mathfrak{P}}}({{\mathfrak{R}}},{{\mathfrak{t}}}),D_{{{\mathfrak{R}}}}^{\eta}{{\mathfrak{P}}}({{\mathfrak{R}}},{{\mathfrak{t}}}) ,D_{{{\mathfrak{R}}}}^{2\eta}{{\mathfrak{P}}}({{\mathfrak{R}}},{{\mathfrak{t}}}),D_{{{\mathfrak{R}}}}^{3\eta}{{\mathfrak{P}}}({{\mathfrak{R}}},{{\mathfrak{t}}})\Big),\ 0 < {\mathfrak{F}},\eta\leq1, \end{equation}$

(3.7)

with IC's

$\begin{equation} {{\mathfrak{P}}}^{(k)}({{\mathfrak{R}}},0) = h_{k},\ k = 0,1,2,\cdots,m-1, \end{equation}$

(3.8)

where ${{\mathfrak{P}}}({{\mathfrak{R}}}, {{\mathfrak{t}}})$ is a function to be determined and $\Upsilon\Big({{\mathfrak{P}}}({{\mathfrak{R}}}, {{\mathfrak{t}}}), D_{{{\mathfrak{R}}}}^{\eta}{{\mathfrak{P}}}({{\mathfrak{R}}}, {{\mathfrak{t}}}), D_{{{\mathfrak{R}}}}^{2\eta}{{\mathfrak{P}}}({{\mathfrak{R}}}, {{\mathfrak{t}}}), D_{{{\mathfrak{R}}}}^{3\eta}{{\mathfrak{P}}}({{\mathfrak{R}}}, {{\mathfrak{t}}})\Big)$ is operator of ${{\mathfrak{P}}}({{\mathfrak{R}}}, {{\mathfrak{t}}}), D_{{{\mathfrak{R}}}}^{\eta}{{\mathfrak{P}}}({{\mathfrak{R}}}, {{\mathfrak{t}}}), D_{{{\mathfrak{R}}}}^{2\eta}{{\mathfrak{P}}}({{\mathfrak{R}}}, {{\mathfrak{t}}})$ and $D_{{{\mathfrak{R}}}}^{3\eta}{{\mathfrak{P}}}({{\mathfrak{R}}}, {{\mathfrak{t}}})$ . Equation (3.7) is subjected to MT to obtain

$\begin{equation} {M} [{{\mathfrak{P}}}({{\mathfrak{R}}},{{\mathfrak{t}}})] = \frac{1}{{s}^{{\mathfrak{F}}}}\Big(\sum\limits_{k = 0}^{m-1}\frac{{{\mathfrak{P}}}^{(k)}({{\mathfrak{R}}},0)}{{s}^{1-{\mathfrak{F}}+k}}+{M} \Big[\Upsilon\Big({{\mathfrak{P}}}({{\mathfrak{R}}},{{\mathfrak{t}}}),D_{{{\mathfrak{R}}}}^{\eta}{{\mathfrak{P}}}({{\mathfrak{R}}},{{\mathfrak{t}}}),D_{{{\mathfrak{R}}}}^{2\eta}{{\mathfrak{P}}}({{\mathfrak{R}}},{{\mathfrak{t}}}),D_{{{\mathfrak{R}}}}^{3\eta}{{\mathfrak{P}}}({{\mathfrak{R}}},{{\mathfrak{t}}})\Big)\Big]\Big). \end{equation}$

(3.9)

The IMT gives us

$\begin{equation} {{\mathfrak{P}}}({{\mathfrak{R}}},{{\mathfrak{t}}}) = {M}^{-1}\Big[\frac{1}{{s}^{{\mathfrak{F}}}}\Big(\sum\limits_{k = 0}^{m-1}\frac{{{\mathfrak{P}}}^{(k)}({{\mathfrak{R}}},0)}{{s}^{1-{\mathfrak{F}}+k}}+{M} \Big[\Upsilon\Big({{\mathfrak{P}}}({{\mathfrak{R}}},{{\mathfrak{t}}}),D_{{{\mathfrak{R}}}}^{\eta}{{\mathfrak{P}}}({{\mathfrak{R}}},{{\mathfrak{t}}}),D_{{{\mathfrak{R}}}}^{2\eta}{{\mathfrak{P}}}({{\mathfrak{R}}},{{\mathfrak{t}}}),D_{{{\mathfrak{R}}}}^{3\eta}{{\mathfrak{P}}}({{\mathfrak{R}}},{{\mathfrak{t}}})\Big)\Big]\Big)\Big]. \end{equation}$

(3.10)

The solution via the MTIM technique is represented as

$\begin{equation} {{\mathfrak{P}}}({{\mathfrak{R}}},{{\mathfrak{t}}}) = \sum\limits_{i = 0}^{\infty}{{\mathfrak{P}}}_{i}. \end{equation}$

(3.11)

The decomposition of the operator $\Upsilon\Big({{\mathfrak{P}}}, D_{{{\mathfrak{R}}}}^{\eta}{{\mathfrak{P}}}, D_{{{\mathfrak{R}}}}^{2\eta}{{\mathfrak{P}}}, D_{{{\mathfrak{R}}}}^{3\eta}{{\mathfrak{P}}}\Big)$ is

$\begin{equation} \begin{split} \Upsilon \Big({{\mathfrak{P}}},D_{{{\mathfrak{R}}}}^{\eta}{{\mathfrak{P}}},&D_{{{\mathfrak{R}}}}^{2\eta}{{\mathfrak{P}}},D_{{{\mathfrak{R}}}}^{3\eta}{{\mathfrak{P}}}\Big) = \Upsilon \Big({{\mathfrak{P}}}_{0},D_{{{\mathfrak{R}}}}^{\eta}{{\mathfrak{P}}}_{0},D_{{{\mathfrak{R}}}}^{2\eta}{{\mathfrak{P}}}_{0},D_{{{\mathfrak{R}}}}^{3\eta}{{\mathfrak{P}}}_{0}\Big)\\&+ \sum\limits_{i = 0}^{\infty} \left( \Upsilon \Big(\sum\limits_{k = 0}^{i}\Big({{\mathfrak{P}}}_{k},D_{{{\mathfrak{R}}}}^{\eta}{{\mathfrak{P}}}_{k},D_{{{\mathfrak{R}}}}^{2\eta}{{\mathfrak{P}}}_{k},D_{{{\mathfrak{R}}}}^{3\eta}{{\mathfrak{P}}}_{k}\Big)\Big) -\Upsilon \Big(\sum\limits_{k = 1}^{i-1}\Big({{\mathfrak{P}}}_{k},D_{{{\mathfrak{R}}}}^{\eta}{{\mathfrak{P}}}_{k},D_{{{\mathfrak{R}}}}^{2\eta}{{\mathfrak{P}}}_{k},D_{{{\mathfrak{R}}}}^{3\eta}{{\mathfrak{P}}}_{k}\Big)\Big)\right). \end{split} \end{equation}$

(3.12)

Putting Eqs (3.11) and (3.12) into Eq (3.10), we obtain

$\begin{equation} \begin{split} \sum\limits_{i = 0}^{\infty}{{\mathfrak{P}}}_i({{\mathfrak{R}}},{{\mathfrak{t}}})& = {M}^{-1}\Big[ \frac{1}{{s}^{{\mathfrak{F}}}}\Big( \sum\limits_{k = 0}^{m-1}\frac{{{\mathfrak{P}}}^{(k)}({{\mathfrak{R}}},0)}{{s}^{2-{\mathfrak{F}}+k}}+{M}[\Upsilon ({{\mathfrak{P}}}_{0},D_{{{\mathfrak{R}}}}^{\eta}{{\mathfrak{P}}}_{0},D_{{{\mathfrak{R}}}}^{2\eta}{{\mathfrak{P}}}_{0},D_{{{\mathfrak{R}}}}^{3\eta}{{\mathfrak{P}}}_{0})]\Big)\Big]\\&+{M}^{-1}\Big[ \frac{1}{{s}^{{\mathfrak{F}}}}\Big({M}\Big[ \sum\limits_{i = 0}^{\infty}\Big(\Upsilon \sum\limits_{k = 0}^{i}({{\mathfrak{P}}}_{k},D_{{{\mathfrak{R}}}}^{\eta}{{\mathfrak{P}}}_{k},D_{{{\mathfrak{R}}}}^{2\eta}{{\mathfrak{P}}}_{k},D_{{{\mathfrak{R}}}}^{3\eta}{{\mathfrak{P}}}_{k})\Big)\Big]\Big)\Big]\\&-{M}^{-1}\Big[ \frac{1}{{s}^{{\mathfrak{F}}}}\Big({M}\Big[\Big(\Upsilon \sum\limits_{k = 1}^{i-1}({{\mathfrak{P}}}_{k},D_{{{\mathfrak{R}}}}^{\eta}{{\mathfrak{P}}}_{k},D_{{{\mathfrak{R}}}}^{2\eta}{{\mathfrak{P}}}_{k},D_{{{\mathfrak{R}}}}^{3\eta}{{\mathfrak{P}}}_{k})\Big)\Big]\Big)\Big] \end{split} \end{equation}$

(3.13)

$\begin{equation} \begin{split} &{{\mathfrak{P}}}_0({{\mathfrak{R}}},{{\mathfrak{t}}}) = {M}^{-1}\Big[ \frac{1}{{s}^{{\mathfrak{F}}}}\Big( \sum\limits_{k = 0}^{m-1}\frac{{{\mathfrak{P}}}^{(k)}({{\mathfrak{R}}},0)}{{s}^{2-{\mathfrak{F}}+k}}\Big)\Big],\\ &{{\mathfrak{P}}}_1({{\mathfrak{R}}},{{\mathfrak{t}}}) = {M}^{-1}\Big[ \frac{1}{{s}^{{\mathfrak{F}}}}\Big( {M}[\Upsilon ({{\mathfrak{P}}}_{0},D_{{{\mathfrak{R}}}}^{\eta}{{\mathfrak{P}}}_{0},D_{{{\mathfrak{R}}}}^{2\eta}{{\mathfrak{P}}}_{0},D_{{{\mathfrak{R}}}}^{3\eta}{{\mathfrak{P}}}_{0})]\Big)\Big],\\ \vdots\\ &{{\mathfrak{P}}}_{m+1}({{\mathfrak{R}}},{{\mathfrak{t}}}) = {M}^{-1}\Big[ \frac{1}{{s}^{{\mathfrak{F}}}}\Big({M}\Big[ \sum\limits_{i = 0}^{\infty}\Big(\Upsilon \sum\limits_{k = 0}^{i}({{\mathfrak{P}}}_{k},D_{{{\mathfrak{R}}}}^{\eta}{{\mathfrak{P}}}_{k},D_{{{\mathfrak{R}}}}^{2\eta}{{\mathfrak{P}}}_{k},D_{{{\mathfrak{R}}}}^{3\eta}{{\mathfrak{P}}}_{k})\Big)\Big]\Big)\Big]\\&-{M}^{-1}\Big[ \frac{1}{{s}^{{\mathfrak{F}}}}\Big({M}\Big[\Big(\Upsilon \sum\limits_{k = 1}^{i-1}({{\mathfrak{P}}}_{k},D_{{{\mathfrak{R}}}}^{\eta}{{\mathfrak{P}}}_{k},D_{{{\mathfrak{R}}}}^{2\eta}{{\mathfrak{P}}}_{k},D_{{{\mathfrak{R}}}}^{3\eta}{{\mathfrak{P}}}_{k})\Big)\Big]\Big)\Big],\ m = 1,2,\cdots. \end{split} \end{equation}$

(3.14)

The general solution of Eq (3.7) is given as

$\begin{equation} {{\mathfrak{P}}}({{\mathfrak{R}}},{{\mathfrak{t}}}) = \sum\limits_{i = 0}^{m-1}{{\mathfrak{P}}}_{i}. \end{equation}$

(3.15)

4. Uses of proposed methods

Example 4.1. $\bullet$ Implementation of MRPSM

Let us consider the fractional PDE

$\begin{equation} \begin{split} &D_{\mathfrak{t}}^{\mathfrak{F}} {\mathfrak{P}}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},\mathfrak{t})+\frac{\partial ^2{\mathfrak{P}}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},\mathfrak{t})}{\partial\mathfrak{R}^2}+\frac{\partial ^2{\mathfrak{P}}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},\mathfrak{t})}{\partial\mathfrak{K}^2}+\frac{\partial ^2{\mathfrak{P}}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},\mathfrak{t})}{\partial\mathfrak{Z}^2} = 0,\\& \text { where }\ 0 < \mathfrak{F} \leq 1. \end{split} \end{equation}$

(4.1)

The initial condition is

$\begin{equation} \begin{split} &{\mathfrak{P}}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},0) = e^{\mathfrak{R}+\mathfrak{K}+\mathfrak{Z}},\\ \end{split} \end{equation}$

(4.2)

with exact solution

$\begin{equation} \begin{split} &{\mathfrak{P}}(\mathfrak{R},\mathfrak{K},\mathfrak{t}) = e^{\mathfrak{R}+\mathfrak{K}+\mathfrak{Z}-3\mathfrak{t}}.\\ \end{split} \end{equation}$

(4.3)

Equation (4.1) is subjected to MT, and using Eq (4.2) we get the following result:

$\begin{equation} \begin{split} &{\mathfrak{P}}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},s)-\frac{e^{\mathfrak{R}+\mathfrak{K}+\mathfrak{Z}}}{s}+\frac{1}{s^{\mathfrak{F}}}\Big[\frac{\partial ^2{\mathfrak{P}}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},s)}{\partial\mathfrak{R}^2}\Big]+\frac{1}{s^{\mathfrak{F}}}\Big[\frac{\partial ^2{\mathfrak{P}}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},s)}{\partial\mathfrak{K}^2}\Big]+\frac{1}{s^{\mathfrak{F}}}\Big[\frac{\partial ^2{\mathfrak{P}}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},s)}{\partial\mathfrak{Z}^2}\Big] = 0. \end{split} \end{equation}$

(4.4)

The $k^{th}$ term's series is represented as

$\begin{equation} \begin{split} {\mathfrak{P}}({\mathfrak{R}},\mathfrak{K},\mathfrak{Z},s) = \frac{e^{\mathfrak{R}+\mathfrak{K}+\mathfrak{Z}}}{s}+\sum\limits_{r = 1}^{k}\frac{f_{r}({\mathfrak{R}},\mathfrak{K},\mathfrak{Z},s)}{s^{r\mathfrak{F}+1}}, \ \ r = 1,2,3,4\cdots.\\ \end{split} \end{equation}$

(4.5)

The residual function of Mohand is given by

$\begin{equation} \begin{split} \mathcal{M}_{{\mathfrak{t}}}Res({\mathfrak{R}},\mathfrak{K},\mathfrak{Z},s)& = {\mathfrak{P}}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},s)-\frac{e^{\mathfrak{R}+\mathfrak{K}+\mathfrak{Z}}}{s}+\frac{1}{s^{\mathfrak{F}}}\Big[\frac{\partial ^2{\mathfrak{P}}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},s)}{\partial\mathfrak{R}^2}\Big]+\frac{1}{s^{\mathfrak{F}}}\Big[\frac{\partial ^2{\mathfrak{P}}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},s)}{\partial\mathfrak{K}^2}\Big]\\&+\frac{1}{s^{\mathfrak{F}}}\Big[\frac{\partial ^2{\mathfrak{P}}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},s)}{\partial\mathfrak{Z}^2}\Big] = 0, \end{split} \end{equation}$

(4.6)

and the ${k}^{th}$ -MRFs as

$\begin{equation} \begin{split} \mathcal{M}_{{\mathfrak{t}}}Res_{k}({\mathfrak{R}},\mathfrak{K},\mathfrak{Z},s)& = {\mathfrak{P}}_{k}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},s)-\frac{e^{\mathfrak{R}+\mathfrak{K}+\mathfrak{Z}}}{s}+\frac{1}{s^{\mathfrak{F}}}\Big[\frac{\partial ^2{\mathfrak{P}}_{k}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},s)}{\partial\mathfrak{R}^2}\Big]+\frac{1}{s^{\mathfrak{F}}}\Big[\frac{\partial ^2{\mathfrak{P}}_{k}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},s)}{\partial\mathfrak{K}^2}\Big]\\&+\frac{1}{s^{\mathfrak{F}}}\Big[\frac{\partial ^2{\mathfrak{P}}_{k}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},s)}{\partial\mathfrak{Z}^2}\Big] = 0. \end{split} \end{equation}$

(4.7)

Now, we use these steps to find the values of $f_{r}({{\mathfrak{R}}}, \mathfrak{K}, \mathfrak{Z}, s)$ for $r = 1, 2, 3, ...$ : Take the $r^{th}$ -Mohand residual function Eq (4.7) for the $r^{th}$ -truncated series Eq (4.5), and then multiply the equation by $s^{r{\mathfrak{F}}+1}$ and solve $\lim_{s\rightarrow \infty}(s^{r{\mathfrak{F}}+1}){M}_{{\mathfrak{t}}}Res_{\mathfrak{P}, r}({\mathfrak{R}}, \mathfrak{K}, \mathfrak{Z}, s)) = 0$ for $r = 1, 2, 3, \cdots$ . Using this procedure, we obtain the following terms:

$\begin{equation} \begin{split} & f_{1}({\mathfrak{R}},\mathfrak{K},\mathfrak{Z},s) = -3e^{\mathfrak{R}+\mathfrak{K}+\mathfrak{Z}}, \end{split} \end{equation}$

(4.8)

$\begin{equation} \begin{split} &f_{2}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},s) = 9e^{\mathfrak{R}+\mathfrak{K}+\mathfrak{Z}}, \end{split} \end{equation}$

(4.9)

$\begin{equation} \begin{split} & f_{3}({\mathfrak{R}},\mathfrak{K},\mathfrak{Z},s) = -27e^{\mathfrak{R}+\mathfrak{K}+\mathfrak{Z}}, \end{split} \end{equation}$

(4.10)

and so on.

The values of Eqs (4.9) and (4.10) are inserted in Eq (4.5) to obtain the following result:

$\begin{equation} \begin{split} &{\mathfrak{P}}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},s) = \frac{e^{\mathfrak{R}+\mathfrak{K}+\mathfrak{Z}}}{s}-\frac{3e^{\mathfrak{R}+\mathfrak{K}+\mathfrak{Z}}}{s^{\mathfrak{F}+1}} +\frac{9e^{\mathfrak{R}+\mathfrak{K}+\mathfrak{Z}}}{s^{2 \mathfrak{F}+1}}-\frac{27e^{\mathfrak{R}+\mathfrak{K}+\mathfrak{Z}}}{s^{3 \mathfrak{F}+1}}+\cdots. \end{split} \end{equation}$

(4.11)

Using IMT, we obtain the final solution

$\begin{equation} \begin{split} &{\mathfrak{P}_{1}}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},\mathfrak{t}) = e^{\mathfrak{R}+\mathfrak{K}+\mathfrak{Z}}-\frac{3 \mathfrak{t}^\mathfrak{F} e^{\mathfrak{R}+\mathfrak{K}+\mathfrak{Z}}}{\Gamma (\mathfrak{F}+1)}+\frac{9 \mathfrak{t}^{2 \mathfrak{F}} e^{\mathfrak{R}+\mathfrak{K}+\mathfrak{Z}}}{\Gamma (2 \mathfrak{F}+1)}-\frac{27 \mathfrak{t}^{3 \mathfrak{F}} e^{\mathfrak{R}+\mathfrak{K}+\mathfrak{Z}}}{\Gamma (3 \mathfrak{F}+1)}. \end{split} \end{equation}$

(4.12)

$\bullet$ Implementation of MTIM

Consider the fractional PDE

$\begin{equation} \begin{split} &D_{\mathfrak{t}}^{\mathfrak{F}} {\mathfrak{P}}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},\mathfrak{t}) = -\frac{\partial ^2{\mathfrak{P}}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},\mathfrak{t})}{\partial\mathfrak{R}^2}-\frac{\partial ^2{\mathfrak{P}}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},\mathfrak{t})}{\partial\mathfrak{K}^2}-\frac{\partial ^2{\mathfrak{P}}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},\mathfrak{t})}{\partial\mathfrak{Z}^2},\\& \text { where }\ 0 < \mathfrak{F} \leq 1. \end{split} \end{equation}$

(4.13)

The initial condition is

$\begin{equation} \begin{split} &{\mathfrak{P}}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},0) = e^{\mathfrak{R}+\mathfrak{K}+\mathfrak{Z}}. \end{split} \end{equation}$

(4.14)

MT is used on Eq (4.13), giving

$\begin{equation} \begin{split} {M} [D_{\mathfrak{t}}^{\mathfrak{F}} {\mathfrak{P}}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},\mathfrak{t})]& = \frac{1}{{s}^{{\mathfrak{F}}}}\Big(\sum\limits_{k = 0}^{m-1}\frac{{\mathfrak{P}}^{(k)}({\mathfrak{R}},\mathfrak{K},\mathfrak{Z},0)}{{s}^{2-{\mathfrak{F}}+k}} +{M}\Big[-\frac{\partial ^2{\mathfrak{P}}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},\mathfrak{t})}{\partial\mathfrak{R}^2}-\frac{\partial ^2{\mathfrak{P}}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},\mathfrak{t})}{\partial\mathfrak{K}^2}\\&-\frac{\partial ^2{\mathfrak{P}}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},\mathfrak{t})}{\partial\mathfrak{Z}^2}\Big)\Big]\Big). \end{split} \end{equation}$

(4.15)

Applying IMT on Eq (4.15), we obtain

$\begin{equation} \begin{split} {\mathfrak{P}}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},\mathfrak{t})& = M^{-1}\Big[\frac{1}{{s}^{{\mathfrak{F}}}}\Big(\sum\limits_{k = 0}^{m-1}\frac{{\mathfrak{P}}^{(k)}({\mathfrak{R}},\mathfrak{K},\mathfrak{Z},0)}{{s}^{2-{\mathfrak{F}}+k}} +{M}\Big[-\frac{\partial ^2{\mathfrak{P}}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},\mathfrak{t})}{\partial\mathfrak{R}^2}-\frac{\partial ^2{\mathfrak{P}}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},\mathfrak{t})}{\partial\mathfrak{K}^2}\\&-\frac{\partial ^2{\mathfrak{P}}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},\mathfrak{t})}{\partial\mathfrak{Z}^2}\Big]\Big)\Big]. \end{split} \end{equation}$

(4.16)

Recursively applying the MT, we obtain

$\begin{equation*} \begin{split} {\mathfrak{P}}_0(\mathfrak{R},\mathfrak{K},\mathfrak{Z}, \mathfrak{t})& = {M}^{-1}\Big[\frac{1}{{s}^{{\mathfrak{F}}}}\Big(\sum\limits_{k = 0}^{m-1}\frac{{\mathfrak{P}}^{(k)}({\mathfrak{R}},\mathfrak{K},\mathfrak{Z},0)}{{s}^{2-{\mathfrak{F}}+k}}\Big)\Big] = {M}^{-1}\Big[\frac{{\mathfrak{P}}({\mathfrak{R}},\mathfrak{K},\mathfrak{Z},0)}{{s}^2}\Big] = e^{\mathfrak{R}+\mathfrak{K}+\mathfrak{Z}}. \end{split} \end{equation*}$

The RL integral is implemented on Eq (4.13), giving

$\begin{equation} \begin{split} &{\mathfrak{P}}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},\mathfrak{t}) = e^{\mathfrak{R}+\mathfrak{K}+\mathfrak{Z}} +{M}\Big[-\frac{\partial ^2{\mathfrak{P}}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},\mathfrak{t})}{\partial\mathfrak{R}^2}-\frac{\partial ^2{\mathfrak{P}}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},\mathfrak{t})}{\partial\mathfrak{K}^2}-\frac{\partial ^2{\mathfrak{P}}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},\mathfrak{t})}{\partial\mathfrak{Z}^2}\Big]. \end{split} \end{equation}$

(4.17)

By using MTIM approach, we obtain the following terms:

$\begin{equation} \begin{split} &{\mathfrak{P}}_{0}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},\mathfrak{t}) = e^{\mathfrak{R}+\mathfrak{K}+\mathfrak{Z}}, \end{split} \end{equation}$

(4.18)

$\begin{equation} \begin{split} &{\mathfrak{P}}_{1}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},\mathfrak{t}) = -\frac{3 \mathfrak{t}^\mathfrak{F} e^{\mathfrak{R}+\mathfrak{K}+\mathfrak{Z}}}{\Gamma (\mathfrak{F}+1)}, \end{split} \end{equation}$

(4.19)

$\begin{equation} \begin{split} &{\mathfrak{P}}_{2}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},\mathfrak{t}) = \frac{9 \mathfrak{t}^{2 \mathfrak{F}} e^{\mathfrak{R}+\mathfrak{K}+\mathfrak{Z}}}{\Gamma (2 \mathfrak{F}+1)}, \end{split} \end{equation}$

(4.20)

$\begin{equation} \begin{split} &{\mathfrak{P}}_{3}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},\mathfrak{t}) = -\frac{27 \mathfrak{t}^{3 \mathfrak{F}} e^{\mathfrak{R}+\mathfrak{K}+\mathfrak{Z}}}{\Gamma (3 \mathfrak{F}+1)}. \end{split} \end{equation}$

(4.21)

The final solution is represented as follows:

$\begin{equation} \begin{split} {\mathfrak{P}}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},\mathfrak{t}) = {\mathfrak{P}}_{0}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},\mathfrak{t}) +{\mathfrak{P}}_{1}(\mathfrak{R},\mathfrak{Z},\mathfrak{K},\mathfrak{t})+{\mathfrak{P}}_{2}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},\mathfrak{t}) +{\mathfrak{P}}_{3}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},\mathfrak{t})+\cdots, \end{split} \end{equation}$

(4.22)

$\begin{equation} \begin{split} &{\mathfrak{P}}(\mathfrak{R},\mathfrak{K},\mathfrak{Z},\mathfrak{t}) = e^{\mathfrak{R}+\mathfrak{K}+\mathfrak{Z}}-\frac{3 \mathfrak{t}^\mathfrak{F} e^{\mathfrak{R}+\mathfrak{K}+\mathfrak{Z}}}{\Gamma (\mathfrak{F}+1)}+\frac{9 \mathfrak{t}^{2 \mathfrak{F}} e^{\mathfrak{R}+\mathfrak{K}+\mathfrak{Z}}}{\Gamma (2 \mathfrak{F}+1)}-\frac{27 \mathfrak{t}^{3 \mathfrak{F}} e^{\mathfrak{R}+\mathfrak{K}+\mathfrak{Z}}}{\Gamma (3 \mathfrak{F}+1)}+\cdots. \end{split} \end{equation}$

(4.23)

Example 4.2. $\bullet$ Implementation of MRPSM

Consider the system of nonlinear PDEs

$\begin{equation} \begin{split} &D_{{{\mathfrak{t}}}}^{\mathfrak{F}} {\mathfrak{P}}_{1}({{\mathfrak{R}}}, {{\mathfrak{t}}})+\frac{\partial ^3{\mathfrak{P}}_{1}({{\mathfrak{R}}}, {{\mathfrak{t}}})}{\partial \mathfrak{R}^3}-3 {\mathfrak{P}}_{2}({{\mathfrak{R}}}, {{\mathfrak{t}}}) \frac{\partial {\mathfrak{P}}_{2}({{\mathfrak{R}}}, {{\mathfrak{t}}})}{\partial \mathfrak{R}}+6 {\mathfrak{P}}_{1}({{\mathfrak{R}}}, {{\mathfrak{t}}}) \frac{\partial {\mathfrak{P}}_{1}({{\mathfrak{R}}}, {{\mathfrak{t}}})}{\partial \mathfrak{R}} = 0,\\& D_{{{\mathfrak{t}}}}^{\mathfrak{F}} {\mathfrak{P}}_{2}({{\mathfrak{R}}}, {{\mathfrak{t}}})+\frac{\partial ^3{\mathfrak{P}}_{2}({{\mathfrak{R}}}, {{\mathfrak{t}}})}{\partial \mathfrak{R}^3}+3 {\mathfrak{P}}_{1}({{\mathfrak{R}}}, {{\mathfrak{t}}}) \frac{\partial {\mathfrak{P}}_{2}({{\mathfrak{R}}}, {{\mathfrak{t}}})}{\partial \mathfrak{R}} = 0\ \ \text { where }\ \ 0 < \mathfrak{F} \leq 1. \end{split} \end{equation}$

(4.24)

The IC's are given as

$\begin{equation} \begin{split} &{\mathfrak{P}}_{1}({{\mathfrak{R}}}, 0) = \frac{4 c^2 e^{c \mathfrak{R}}}{\left(e^{c \mathfrak{R}}+1\right)^2},\\ &{\mathfrak{P}}_{2}({{\mathfrak{R}}}, 0) = \frac{4 c^2 e^{c \mathfrak{R}}}{\left(e^{c \mathfrak{R}}+1\right)^2}. \end{split} \end{equation}$

(4.25)

Equation (4.24) is subjected to MT, and using Eq (4.25), we get the following result:

$\begin{equation} \begin{split} &{\mathfrak{P}}_{1}({{\mathfrak{R}}}, s)+\frac{\frac{4 c^2 e^{c \mathfrak{R}}}{\left(e^{c \mathfrak{R}}+1\right)^2}}{s}+\frac{1}{s^{\mathfrak{F}}}\Big[\frac{\partial ^3{\mathfrak{P}}_{1}({{\mathfrak{R}}}, s)}{\partial \mathfrak{R}^3}\Big]-\frac{3}{s^{\mathfrak{F}}}\mathcal{M}_{\mathfrak{t}}\Big[\mathcal{M}_{\mathfrak{t}}^{-1}{\mathfrak{P}}_{2}({{\mathfrak{R}}},s)\times \frac{\partial \mathcal{M}_{\mathfrak{t}}^{-1}{\mathfrak{P}}_{2}({{\mathfrak{R}}},s)}{\partial \mathfrak{R}}\Big]\\&+\frac{6}{s^{\mathfrak{F}}}\mathcal{M}_{\mathfrak{t}}\Big[\mathcal{M}_{\mathfrak{t}}^{-1}{\mathfrak{P}}_{1}({{\mathfrak{R}}},s)\times \frac{\partial \mathcal{M}_{\mathfrak{t}}^{-1}{\mathfrak{P}}_{1}({{\mathfrak{R}}},s)}{\partial \mathfrak{R}}\Big] = 0,\\ &{\mathfrak{P}}_{2}({{\mathfrak{R}}},s)-\frac{\frac{4 c^2 e^{c \mathfrak{R}}}{\left(e^{c \mathfrak{R}}+1\right)^2}}{s}+\frac{1}{s^{\mathfrak{F}}}\Big[\frac{\partial ^3{\mathfrak{P}}_{2}({{\mathfrak{R}}}, s)}{\partial \mathfrak{R}^3}\Big]+\frac{3}{s^{\mathfrak{F}}}\mathcal{M}_{\mathfrak{t}}\Big[\mathcal{M}_{\mathfrak{t}}^{-1}{\mathfrak{P}}_{1}({{\mathfrak{R}}},s)\times \frac{\partial \mathcal{M}_{\mathfrak{t}}^{-1}{\mathfrak{P}}_{2}({{\mathfrak{R}}},s)}{\partial \mathfrak{R}}\Big] = 0.\\ \end{split} \end{equation}$

(4.26)

The $k^{th}$ term's series is represented as

$\begin{equation} \begin{split} &{\mathfrak{P}}_{1}({{{\mathfrak{R}}}},s) = \frac{\frac{4 c^2 e^{c \mathfrak{R}}}{\left(e^{c \mathfrak{R}}+1\right)^2}}{s}+\sum\limits_{r = 1}^{k}\frac{f_{r}({{{\mathfrak{R}}}},s)}{s^{r\mathfrak{F}+1}},\\ &{\mathfrak{P}}_{2}({{{\mathfrak{R}}}},s) = \frac{\frac{4 c^2 e^{c \mathfrak{R}}}{\left(e^{c \mathfrak{R}}+1\right)^2}}{s}+\sum\limits_{r = 1}^{k}\frac{g_{r}({{{\mathfrak{R}}}},s)}{s^{r\mathfrak{F}+1}}, \ \ r = 1,2,3,4\cdots. \end{split} \end{equation}$

(4.27)

The residual function of Mohand is given by

$\begin{equation} \begin{split} {A}_{{{{\mathfrak{t}}}}}Res({{{\mathfrak{R}}}},s)& = {\mathfrak{P}}_{1}({{\mathfrak{R}}}, s)+\frac{\frac{4 c^2 e^{c \mathfrak{R}}}{\left(e^{c \mathfrak{R}}+1\right)^2}}{s}+\frac{1}{s^{\mathfrak{F}}}\Big[\frac{\partial ^3{\mathfrak{P}}_{1}({{\mathfrak{R}}}, s)}{\partial \mathfrak{R}^3}\Big]-\frac{3}{s^{\mathfrak{F}}}\mathcal{M}_{\mathfrak{t}}\Big[\mathcal{M}_{\mathfrak{t}}^{-1}{\mathfrak{P}}_{2}({{\mathfrak{R}}},s)\times \frac{\partial \mathcal{M}_{\mathfrak{t}}^{-1}{\mathfrak{P}}_{2}({{\mathfrak{R}}},s)}{\partial \mathfrak{R}}\Big]\\&+\frac{6}{s^{\mathfrak{F}}}\mathcal{M}_{\mathfrak{t}}\Big[\mathcal{M}_{\mathfrak{t}}^{-1}{\mathfrak{P}}_{1}({{\mathfrak{R}}},s)\times \frac{\partial \mathcal{M}_{\mathfrak{t}}^{-1}{\mathfrak{P}}_{1}({{\mathfrak{R}}},s)}{\partial \mathfrak{R}}\Big] = 0,\\ {A}_{{{{\mathfrak{t}}}}}Res({{{\mathfrak{R}}}},s)& = {\mathfrak{P}}_{2}({{\mathfrak{R}}},s)-\frac{\frac{4 c^2 e^{c \mathfrak{R}}}{\left(e^{c \mathfrak{R}}+1\right)^2}}{s}+\frac{1}{s^{\mathfrak{F}}}\Big[\frac{\partial ^3{\mathfrak{P}}_{2}({{\mathfrak{R}}}, s)}{\partial \mathfrak{R}^3}\Big]+\frac{3}{s^{\mathfrak{F}}}\mathcal{M}_{\mathfrak{t}}\Big[\mathcal{M}_{\mathfrak{t}}^{-1}{\mathfrak{P}}_{1}({{\mathfrak{R}}},s)\times \frac{\partial \mathcal{M}_{\mathfrak{t}}^{-1}{\mathfrak{P}}_{2}({{\mathfrak{R}}},s)}{\partial \mathfrak{R}}\Big] = 0,\\ \end{split} \end{equation}$

(4.28)

and the ${k}^{th}$ -MRFs as

$\begin{equation} \begin{split} {A}_{{{{\mathfrak{t}}}}}Res_k({{{\mathfrak{R}}}},s)& = {{\mathfrak{P}}_{1}}_k({{\mathfrak{R}}}, s)+\frac{\frac{4 c^2 e^{c \mathfrak{R}}}{\left(e^{c \mathfrak{R}}+1\right)^2}}{s}+\frac{1}{s^{\mathfrak{F}}}\Big[\frac{\partial ^3{{\mathfrak{P}}_{1}}_k({{\mathfrak{R}}}, s)}{\partial \mathfrak{R}^3}\Big]-\frac{3}{s^{\mathfrak{F}}}\mathcal{M}_{\mathfrak{t}}\Big[\mathcal{M}_{\mathfrak{t}}^{-1}{{\mathfrak{P}}_{2}}_k({{\mathfrak{R}}},s)\times \frac{\partial \mathcal{M}_{\mathfrak{t}}^{-1}{{\mathfrak{P}}_{2}}_k({{\mathfrak{R}}},s)}{\partial \mathfrak{R}}\Big]\\&+\frac{6}{s^{\mathfrak{F}}}\mathcal{M}_{\mathfrak{t}}\Big[\mathcal{M}_{\mathfrak{t}}^{-1}{{\mathfrak{P}}_{1}}_k({{\mathfrak{R}}},s)\times \frac{\partial \mathcal{M}_{\mathfrak{t}}^{-1}{{\mathfrak{P}}_{1}}_k({{\mathfrak{R}}},s)}{\partial \mathfrak{R}}\Big] = 0,\\ {A}_{{{{\mathfrak{t}}}}}Res_k({{{\mathfrak{R}}}},s)& = {{\mathfrak{P}}_{2}}_k({{\mathfrak{R}}},s)-\frac{\frac{4 c^2 e^{c \mathfrak{R}}}{\left(e^{c \mathfrak{R}}+1\right)^2}}{s}+\frac{1}{s^{\mathfrak{F}}}\Big[\frac{\partial ^3{{\mathfrak{P}}_{2}}_k({{\mathfrak{R}}}, s)}{\partial \mathfrak{R}^3}\Big]+\frac{3}{s^{\mathfrak{F}}}\mathcal{M}_{\mathfrak{t}}\Big[\mathcal{M}_{\mathfrak{t}}^{-1}{{\mathfrak{P}}_{1}}_k({{\mathfrak{R}}},s)\times \frac{\partial \mathcal{M}_{\mathfrak{t}}^{-1}{{\mathfrak{P}}_{2}}_k({{\mathfrak{R}}},s)}{\partial \mathfrak{R}}\Big] = 0. \end{split} \end{equation}$

(4.29)

Now, we use these steps to find the values of $f_{r}({{\mathfrak{R}}}, \mathfrak{K}, \mathfrak{Z}, s)$ and $g_{r}({{\mathfrak{R}}}, \mathfrak{K}, \mathfrak{Z}, s)$ for $r = 1, 2, 3, ...$ : Put the $r^{th}$ -Mohand residual function Eq (4.29) for the $r^{th}$ -truncated series Eq (4.27), and then multiply the equation by $s^{r{\mathfrak{F}}+1}$ , and solve ${\mathcal{M}}_{{{{\mathfrak{t}}}}}Res_{{\mathfrak{P}}_1, r}({{{\mathfrak{R}}}}, s) = 0$ and ${\mathcal{M}}_{{{{\mathfrak{t}}}}}Res_{{\mathfrak{P}}_2, r}({{{\mathfrak{R}}}}, s) = 0$ for $r = 1, 2, 3, \cdots$ . Using this procedure, we obtain

$\begin{equation} \begin{split} f_{1}({{{\mathfrak{R}}}},s) = \frac{4 c^5 e^{c {\mathfrak{R}}} \left(e^{c {\mathfrak{R}}}-1\right)}{\left(e^{c {\mathfrak{R}}}+1\right)^3},\qquad g_{1}({{{\mathfrak{R}}}},s) = \frac{4 c^5 e^{c {\mathfrak{R}}} \left(e^{c {\mathfrak{R}}}-1\right)}{\left(e^{c {\mathfrak{R}}}+1\right)^3}, \end{split} \end{equation}$

(4.30)

$\begin{equation} \begin{split} f_{2}({{{\mathfrak{R}}}},s) = \frac{4 c^8 e^{c {\mathfrak{R}}} \left(-4 e^{c {\mathfrak{R}}}+e^{2 c {\mathfrak{R}}}+1\right)}{\left(e^{c {\mathfrak{R}}}+1\right)^4},\qquad g_{2}({{{\mathfrak{R}}}},s) = \frac{4 c^8 e^{c {\mathfrak{R}}} \left(-4 e^{c {\mathfrak{R}}}+e^{2 c {\mathfrak{R}}}+1\right)}{\left(e^{c {\mathfrak{R}}}+1\right)^4}, \end{split} \end{equation}$

(4.31)

and so on.

The values of Eqs (4.30) and (4.31) are inserted into Eq (4.27) to obtain the following result:

$\begin{equation} \begin{split} &{\mathfrak{P}}_{1}({{\mathfrak{R}}},s) = \frac{4 c^2 e^{c \mathfrak{R}}}{s\left(e^{c \mathfrak{R}}+1\right)^2}+\frac{4 c^5 e^{c {\mathfrak{R}}} \left(e^{c {\mathfrak{R}}}-1\right)}{s^{\mathfrak{F}+1}\left(e^{c {\mathfrak{R}}}+1\right)^3}+\frac{4 c^8 e^{c {\mathfrak{R}}} \left(-4 e^{c {\mathfrak{R}}}+e^{2 c {\mathfrak{R}}}+1\right)}{s^{2 \mathfrak{F}+1}\left(e^{c {\mathfrak{R}}}+1\right)^4}+\cdots,\\ &{\mathfrak{P}}_{2}({{\mathfrak{R}}},s) = \frac{4 c^2 e^{c \mathfrak{R}}}{s\left(e^{c \mathfrak{R}}+1\right)^2}+\frac{4 c^5 e^{c {\mathfrak{R}}} \left(e^{c {\mathfrak{R}}}-1\right)}{s^{\mathfrak{F}+1}\left(e^{c {\mathfrak{R}}}+1\right)^3}+\frac{4 c^8 e^{c {\mathfrak{R}}} \left(-4 e^{c {\mathfrak{R}}}+e^{2 c {\mathfrak{R}}}+1\right)}{s^{2 \mathfrak{F}+1}\left(e^{c {\mathfrak{R}}}+1\right)^4}+\cdots.\\ \end{split} \end{equation}$

(4.32)

Using IMT, we obtain the final solution:

$\begin{equation} \begin{split} &{\mathfrak{P}}_{1}({{\mathfrak{R}}},{\mathfrak{t}}) = \frac{4 c^2 e^{c \mathfrak{R}}}{\left(e^{c \mathfrak{R}}+1\right)^2}+\frac{4 c^5\mathfrak{t}^{\mathfrak{F}} e^{c {\mathfrak{R}}} \left(e^{c {\mathfrak{R}}}-1\right)}{\Gamma(\mathfrak{F}+1)\left(e^{c {\mathfrak{R}}}+1\right)^3}+\frac{4 c^8\mathfrak{t}^{2\mathfrak{F}} e^{c {\mathfrak{R}}} \left(-4 e^{c {\mathfrak{R}}}+e^{2 c {\mathfrak{R}}}+1\right)}{\Gamma(2 \mathfrak{F}+1)\left(e^{c {\mathfrak{R}}}+1\right)^4}+\cdots,\\ &{\mathfrak{P}}_{2}({{\mathfrak{R}}},{\mathfrak{t}}) = \frac{4 c^2 e^{c \mathfrak{R}}}{\left(e^{c \mathfrak{R}}+1\right)^2}+\frac{4 c^5\mathfrak{t}^{\mathfrak{F}} e^{c {\mathfrak{R}}} \left(e^{c {\mathfrak{R}}}-1\right)}{\Gamma(\mathfrak{F}+1)\left(e^{c {\mathfrak{R}}}+1\right)^3}+\frac{4 c^8\mathfrak{t}^{2\mathfrak{F}} e^{c {\mathfrak{R}}} \left(-4 e^{c {\mathfrak{R}}}+e^{2 c {\mathfrak{R}}}+1\right)}{\Gamma(2 \mathfrak{F}+1)\left(e^{c {\mathfrak{R}}}+1\right)^4}+\cdots.\\ \end{split} \end{equation}$

(4.33)

$\bullet$ Implementation of MTIM

Consider the system of nonlinear PDEs

$\begin{equation} \begin{split} &D_{{{\mathfrak{t}}}}^{\mathfrak{F}} {\mathfrak{P}}_{1}({{\mathfrak{R}}}, {{\mathfrak{t}}}) = -\frac{\partial ^3{\mathfrak{P}}_{1}({{\mathfrak{R}}}, {{\mathfrak{t}}})}{\partial \mathfrak{R}^3}+3 {\mathfrak{P}}_{2}({{\mathfrak{R}}}, {{\mathfrak{t}}}) \frac{\partial {\mathfrak{P}}_{2}({{\mathfrak{R}}}, {{\mathfrak{t}}})}{\partial \mathfrak{R}}-6 {\mathfrak{P}}_{1}({{\mathfrak{R}}}, {{\mathfrak{t}}}) \frac{\partial {\mathfrak{P}}_{1}({{\mathfrak{R}}}, {{\mathfrak{t}}})}{\partial \mathfrak{R}},\\& D_{{{\mathfrak{t}}}}^{\mathfrak{F}} {\mathfrak{P}}_{2}({{\mathfrak{R}}}, {{\mathfrak{t}}}) = -\frac{\partial ^3{\mathfrak{P}}_{2}({{\mathfrak{R}}}, {{\mathfrak{t}}})}{\partial \mathfrak{R}^3}-3 {\mathfrak{P}}_{1}({{\mathfrak{R}}}, {{\mathfrak{t}}}) \frac{\partial {\mathfrak{P}}_{2}({{\mathfrak{R}}}, {{\mathfrak{t}}})}{\partial \mathfrak{R}},\ \ \text { where }\ \ 0 < \mathfrak{F} \leq 1. \end{split} \end{equation}$

(4.34)

The IC's are given as

$\begin{equation} \begin{split} &{\mathfrak{P}}_{1}({{\mathfrak{R}}}, 0) = \frac{4 c^2 e^{c \mathfrak{R}}}{\left(e^{c \mathfrak{R}}+1\right)^2},\qquad {\mathfrak{P}}_{2}({{\mathfrak{R}}}, 0) = \frac{4 c^2 e^{c \mathfrak{R}}}{\left(e^{c \mathfrak{R}}+1\right)^2}. \end{split} \end{equation}$

(4.35)

MT is used on Eq (4.34), giving

$\begin{equation} \begin{split} &{\mathcal{M}} [D_{{{\mathfrak{t}}}}^{\mathfrak{F}} {\mathfrak{P}}_{1}({{\mathfrak{R}}}, {{\mathfrak{t}}})] = \frac{1}{{s}^{{\mathfrak{F}}}}\Big(\sum\limits_{k = 0}^{m-1}\frac{{\mathfrak{P}}_{1}^{(k)}({{{\mathfrak{R}}}},0)}{{s}^{2-{\mathfrak{F}}+k}}+{\mathcal{M}} \Big[-\frac{\partial ^3{\mathfrak{P}}_{1}({{\mathfrak{R}}}, {{\mathfrak{t}}})}{\partial \mathfrak{R}^3}+3 {\mathfrak{P}}_{2}({{\mathfrak{R}}}, {{\mathfrak{t}}}) \frac{\partial {\mathfrak{P}}_{2}({{\mathfrak{R}}}, {{\mathfrak{t}}})}{\partial \mathfrak{R}}-6 {\mathfrak{P}}_{1}({{\mathfrak{R}}}, {{\mathfrak{t}}}) \frac{\partial {\mathfrak{P}}_{1}({{\mathfrak{R}}}, {{\mathfrak{t}}})}{\partial \mathfrak{R}}\Big]\Big),\\ &{\mathcal{M}} [D_{{{\mathfrak{t}}}}^{\mathfrak{F}} {\mathfrak{P}}_{2}({{\mathfrak{R}}}, {{\mathfrak{t}}})] = \frac{1}{{s}^{{\mathfrak{F}}}}\Big(\sum\limits_{k = 0}^{m-1}\frac{{\mathfrak{P}}_{2}^{(k)}({{{\mathfrak{R}}}},0)}{{s}^{2-{\mathfrak{F}}+k}}+{\mathcal{M}} \Big[-\frac{\partial ^3{\mathfrak{P}}_{2}({{\mathfrak{R}}}, {{\mathfrak{t}}})}{\partial \mathfrak{R}^3}-3 {\mathfrak{P}}_{1}({{\mathfrak{R}}}, {{\mathfrak{t}}}) \frac{\partial {\mathfrak{P}}_{2}({{\mathfrak{R}}}, {{\mathfrak{t}}})}{\partial \mathfrak{R}}\Big]\Big). \end{split} \end{equation}$

(4.36)

Applying IMT on Eq (4.36), we obtain

$\begin{equation} \begin{split} &{\mathfrak{P}}_{1}({{\mathfrak{R}}}, {{\mathfrak{t}}}) = {\mathcal{M}}^{-1}\Big[\frac{1}{{s}^{{\mathfrak{F}}}}\Big(\sum\limits_{k = 0}^{m-1}\frac{{\mathfrak{P}}_{1}^{(k)}({{{\mathfrak{R}}}},0)}{{s}^{2-{\mathfrak{F}}+k}}+{\mathcal{M}} \Big[-\frac{\partial ^3{\mathfrak{P}}_{1}({{\mathfrak{R}}}, {{\mathfrak{t}}})}{\partial \mathfrak{R}^3}+3 {\mathfrak{P}}_{2}({{\mathfrak{R}}}, {{\mathfrak{t}}}) \frac{\partial {\mathfrak{P}}_{2}({{\mathfrak{R}}}, {{\mathfrak{t}}})}{\partial \mathfrak{R}}-6 {\mathfrak{P}}_{1}({{\mathfrak{R}}}, {{\mathfrak{t}}}) \frac{\partial {\mathfrak{P}}_{1}({{\mathfrak{R}}}, {{\mathfrak{t}}})}{\partial \mathfrak{R}}\Big]\Big)\Big],\\ &{\mathfrak{P}}_{2}({{\mathfrak{R}}}, {{\mathfrak{t}}}) = {\mathcal{M}}^{-1}\Big[\frac{1}{{s}^{{\mathfrak{F}}}}\Big(\sum\limits_{k = 0}^{m-1}\frac{{\mathfrak{P}}_{2}^{(k)}({{{\mathfrak{R}}}},0)}{{s}^{2-{\mathfrak{F}}+k}}+{\mathcal{M}} \Big[-\frac{\partial ^3{\mathfrak{P}}_{2}({{\mathfrak{R}}}, {{\mathfrak{t}}})}{\partial \mathfrak{R}^3}-3 {\mathfrak{P}}_{1}({{\mathfrak{R}}}, {{\mathfrak{t}}}) \frac{\partial {\mathfrak{P}}_{2}({{\mathfrak{R}}}, {{\mathfrak{t}}})}{\partial \mathfrak{R}}\Big]\Big)\Big]. \end{split} \end{equation}$

(4.37)

Recursively applying the MT, we obtain

$\begin{equation*} \begin{split} {{\mathfrak{P}}_{1}}_0({{\mathfrak{R}}}, {{\mathfrak{t}}}) = &{\mathcal{M}}^{-1}\Big[\frac{1}{{s}^{{\mathfrak{F}}}}\Big(\sum\limits_{k = 0}^{m-1}\frac{{\mathfrak{P}}_{1}^{(k)}({{{\mathfrak{R}}}},0)}{{s}^{2-{\mathfrak{F}}+k}}\Big)\Big] = {\mathcal{M}}^{-1}\Big[\frac{{\mathfrak{P}}_{1}({{{\mathfrak{R}}}},0)}{{s}^2}\Big] = \frac{4 c^2 e^{c \mathfrak{R}}}{\left(e^{c \mathfrak{R}}+1\right)^2}, \end{split} \end{equation*}$

$\begin{equation*} \begin{split} {{\mathfrak{P}}_{2}}_0({{\mathfrak{R}}}, {{\mathfrak{t}}}) = &{\mathcal{M}}^{-1}\Big[\frac{1}{{s}^{{\mathfrak{F}}}}\Big(\sum\limits_{k = 0}^{m-1}\frac{{\mathfrak{P}}_{2}^{(k)}({{{\mathfrak{R}}}},0)}{{s}^{2-{\mathfrak{F}}+k}}\Big)\Big] = {\mathcal{M}}^{-1}\Big[\frac{{\mathfrak{P}}_{2}({{{\mathfrak{R}}}},0)}{{s}^2}\Big] = \frac{4 c^2 e^{c \mathfrak{R}}}{\left(e^{c \mathfrak{R}}+1\right)^2}. \end{split} \end{equation*}$

The RL integral is implemented on Eq (4.34), giving

$\begin{equation} \begin{split} &{\mathfrak{P}}_{1}({{\mathfrak{R}}},{{\mathfrak{t}}}) = \frac{4 c^2 e^{c \mathfrak{R}}}{\left(e^{c \mathfrak{R}}+1\right)^2}-{\mathcal{M}}\Big[-\frac{\partial ^3{\mathfrak{P}}_{1}({{\mathfrak{R}}}, {{\mathfrak{t}}})}{\partial \mathfrak{R}^3}+3 {\mathfrak{P}}_{2}({{\mathfrak{R}}}, {{\mathfrak{t}}}) \frac{\partial {\mathfrak{P}}_{2}({{\mathfrak{R}}}, {{\mathfrak{t}}})}{\partial \mathfrak{R}}-6 {\mathfrak{P}}_{1}({{\mathfrak{R}}}, {{\mathfrak{t}}}) \frac{\partial {\mathfrak{P}}_{1}({{\mathfrak{R}}}, {{\mathfrak{t}}})}{\partial \mathfrak{R}}\Big],\\ &{\mathfrak{P}}_{2}({{\mathfrak{R}}},{{\mathfrak{t}}}) = \frac{4 c^2 e^{c \mathfrak{R}}}{\left(e^{c \mathfrak{R}}+1\right)^2}-{\mathcal{M}}\Big[-\frac{\partial ^3{\mathfrak{P}}_{2}({{\mathfrak{R}}}, {{\mathfrak{t}}})}{\partial \mathfrak{R}^3}-3 {\mathfrak{P}}_{1}({{\mathfrak{R}}}, {{\mathfrak{t}}}) \frac{\partial {\mathfrak{P}}_{2}({{\mathfrak{R}}}, {{\mathfrak{t}}})}{\partial \mathfrak{R}}\Big]. \end{split} \end{equation}$

(4.38)

By using the MTIM approach, we obtain the following terms:

$\begin{equation} \begin{split} &{{\mathfrak{P}}_{1}}_{0}({{\mathfrak{R}}},{{\mathfrak{t}}}) = \frac{4 c^2 e^{c \mathfrak{R}}}{\left(e^{c \mathfrak{R}}+1\right)^2},\\ &{{\mathfrak{P}}_{1}}_{1}({{\mathfrak{R}}},{{\mathfrak{t}}}) = \frac{c^5 {{\mathfrak{t}}}^\mathfrak{F} \tanh \left(\frac{c {{\mathfrak{R}}}}{2}\right) \text{sech}^2\left(\frac{c {{\mathfrak{R}}}}{2}\right)}{\Gamma (\mathfrak{F}+1)},\\ &{{\mathfrak{P}}_{1}}_{2}({{\mathfrak{R}}},{{\mathfrak{t}}}) = -\frac{c^8 {{\mathfrak{t}}}^{2 \mathfrak{F}} \left(3 \text{sech}^2\left(\frac{c {{\mathfrak{R}}}}{2}\right)-2\right) \left(3 c^3 {{\mathfrak{t}}}^\mathfrak{F} \Gamma (2 \mathfrak{F}+1)^2 \tanh \left(\frac{c {{\mathfrak{R}}}}{2}\right) \text{sech}^2\left(\frac{c {{\mathfrak{R}}}}{2}\right)+\Gamma (\mathfrak{F}+1)^2 \Gamma (3 \mathfrak{F}+1)\right)}{\Gamma (\mathfrak{F}+1)^2 \Gamma (2 \mathfrak{F}+1) \Gamma (3 \mathfrak{F}+1) (\cosh (c {{\mathfrak{R}}})+1)}.\\ \end{split} \end{equation}$

(4.39)

$\begin{equation} \begin{split} &{{\mathfrak{P}}_{2}}_{0}({{\mathfrak{R}}},{{\mathfrak{t}}}) = \frac{4 c^2 e^{c \mathfrak{R}}}{\left(e^{c \mathfrak{R}}+1\right)^2},\\ &{{\mathfrak{P}}_{2}}_{1}({{\mathfrak{R}}},{{\mathfrak{t}}}) = \frac{c^5 {{\mathfrak{t}}}^\mathfrak{F} \tanh \left(\frac{c {{\mathfrak{R}}}}{2}\right) \text{sech}^2\left(\frac{c {{\mathfrak{R}}}}{2}\right)}{\Gamma (\mathfrak{F}+1)},\\ &{{\mathfrak{P}}_{2}}_{2}({{\mathfrak{R}}},{{\mathfrak{t}}}) = -\frac{c^8 {{\mathfrak{t}}}^{2 \mathfrak{F}} \left(3 \text{sech}^2\left(\frac{c {{\mathfrak{R}}}}{2}\right)-2\right) \left(3 c^3 {{\mathfrak{t}}}^\mathfrak{F} \Gamma (2 \mathfrak{F}+1)^2 \tanh \left(\frac{c {{\mathfrak{R}}}}{2}\right) \text{sech}^2\left(\frac{c {{\mathfrak{R}}}}{2}\right)+\Gamma (\mathfrak{F}+1)^2 \Gamma (3 \mathfrak{F}+1)\right)}{\Gamma (\mathfrak{F}+1)^2 \Gamma (2 \mathfrak{F}+1) \Gamma (3 \mathfrak{F}+1) (\cosh (c {{\mathfrak{R}}})+1)}.\\ \end{split} \end{equation}$

(4.40)

The final solution is represented as follows:

$\begin{equation} \begin{split} {\mathfrak{P}}_{1}({{\mathfrak{R}}},{{\mathfrak{t}}}) = {{\mathfrak{P}}_{1}}_{0}({{\mathfrak{R}}},{{\mathfrak{t}}})+{{\mathfrak{P}}_{1}}_{1}({{\mathfrak{R}}},{{\mathfrak{t}}})+{{\mathfrak{P}}_{1}}_{2}({{\mathfrak{R}}},{{\mathfrak{t}}})+\cdots, \end{split} \end{equation}$

(4.41)

$\begin{equation} \begin{split} {\mathfrak{P}}_{2}({{\mathfrak{R}}},{{\mathfrak{t}}}) = {{\mathfrak{P}}_{2}}_{0}({{\mathfrak{R}}},{{\mathfrak{t}}})+{{\mathfrak{P}}_{2}}_{1}({{\mathfrak{R}}},{{\mathfrak{t}}})+{{\mathfrak{P}}_{2}}_{2}({{\mathfrak{R}}},{{\mathfrak{t}}})+\cdots.\\ \end{split} \end{equation}$

(4.42)

$\begin{equation} \begin{split} &{\mathfrak{P}}_{1}({{\mathfrak{R}}},{{\mathfrak{t}}}) = \frac{4 c^2 e^{c \mathfrak{R}}}{\left(e^{c \mathfrak{R}}+1\right)^2}+\frac{c^5 {{\mathfrak{t}}}^\mathfrak{F} \tanh \left(\frac{c {{\mathfrak{R}}}}{2}\right) \text{sech}^2\left(\frac{c {{\mathfrak{R}}}}{2}\right)}{\Gamma (\mathfrak{F}+1)}\\&-\frac{c^8 {{\mathfrak{t}}}^{2 \mathfrak{F}} \left(3 \text{sech}^2\left(\frac{c {{\mathfrak{R}}}}{2}\right)-2\right) \left(3 c^3 {{\mathfrak{t}}}^\mathfrak{F} \Gamma (2 \mathfrak{F}+1)^2 \tanh \left(\frac{c {{\mathfrak{R}}}}{2}\right) \text{sech}^2\left(\frac{c {{\mathfrak{R}}}}{2}\right)+\Gamma (\mathfrak{F}+1)^2 \Gamma (3 \mathfrak{F}+1)\right)}{\Gamma (\mathfrak{F}+1)^2 \Gamma (2 \mathfrak{F}+1) \Gamma (3 \mathfrak{F}+1) (\cosh (c {{\mathfrak{R}}})+1)}+\cdots, \end{split} \end{equation}$

(4.43)

$\begin{equation} \begin{split} &{\mathfrak{P}}_{2}({{\mathfrak{R}}},{{\mathfrak{t}}}) = \frac{4 c^2 e^{c \mathfrak{R}}}{\left(e^{c \mathfrak{R}}+1\right)^2}+\frac{c^5 {{\mathfrak{t}}}^\mathfrak{F} \tanh \left(\frac{c {{\mathfrak{R}}}}{2}\right) \text{sech}^2\left(\frac{c {{\mathfrak{R}}}}{2}\right)}{\Gamma (\mathfrak{F}+1)}\\&-\frac{c^8 {{\mathfrak{t}}}^{2 \mathfrak{F}} \left(3 \text{sech}^2\left(\frac{c {{\mathfrak{R}}}}{2}\right)-2\right) \left(3 c^3 {{\mathfrak{t}}}^\mathfrak{F} \Gamma (2 \mathfrak{F}+1)^2 \tanh \left(\frac{c {{\mathfrak{R}}}}{2}\right) \text{sech}^2\left(\frac{c {{\mathfrak{R}}}}{2}\right)+\Gamma (\mathfrak{F}+1)^2 \Gamma (3 \mathfrak{F}+1)\right)}{\Gamma (\mathfrak{F}+1)^2 \Gamma (2 \mathfrak{F}+1) \Gamma (3 \mathfrak{F}+1) (\cosh (c {{\mathfrak{R}}})+1)}+\cdots.\\ \end{split} \end{equation}$

(4.44)

5. Results and discussion

The graphical and tabular results offer a comprehensive evaluation of the effectiveness and accuracy of the MTIM and MRPSM for solving FPDEs.

5.1. Graphical analysis

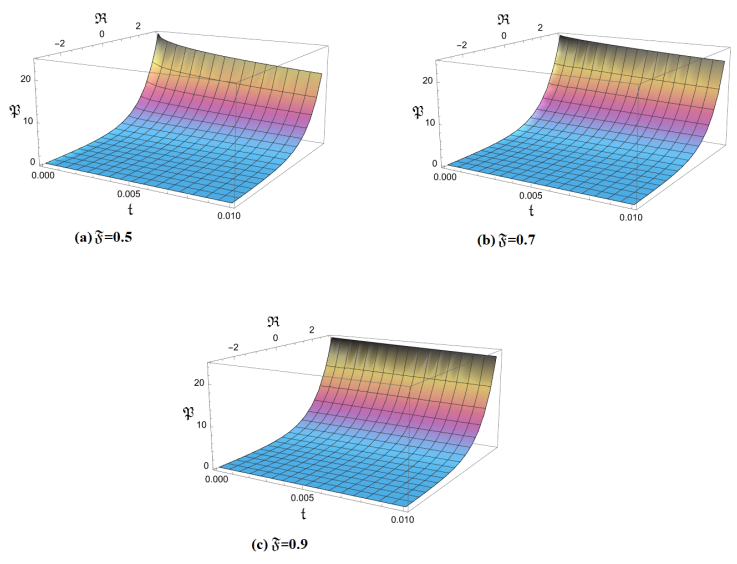
and demonstrate the influence of fractional order on the solutions of the equation $\mathfrak{P}(\mathfrak{R}, \mathfrak{K}, \mathfrak{Z}, \mathfrak{t})$ for specific values of the parameters $\mathfrak{K}$ , $\mathfrak{Z}$ , and $\mathfrak{t}$ . Both 3D and 2D plots highlight the effectiveness of MTIM and MRPSM in accurately capturing the behavior of the solution for varying fractional orders. Figures 3 and 4 provide a comparison between the exact solution and the solutions obtained via MTIM and MRPSM. The close agreement between these solutions demonstrates the reliability of the proposed methods for fractional PDEs. and offer further insights into the effect of fractional order on MRPSM solutions for two distinct cases, $\mathfrak{P}{1}$ and $\mathfrak{P}{2}$ . These plots indicate that MRPSM is highly sensitive to fractional-order variations, which enhances its adaptability for different problems. and depict the results for MTIM in comparison with MRPSM for $\mathfrak{P}{1}$ and $\mathfrak{P}{2}$ under different conditions. The strong correlation between the solutions obtained by the two methods further validates the applicability of both techniques. Figures 9 and 10 show a direct comparison of MRPSM and MTIM for the same fractional order, demonstrating their consistency across both 2D and 3D perspectives. The graphical results affirm that the methods provide nearly identical results, emphasizing their robustness.

Figure 1. Graphical comparison of the fractional-order effect on our proposed method's solutions of

$\mathfrak{P}(\mathfrak{R}, \mathfrak{K}, \mathfrak{Z}, \mathfrak{t})$ for

$\mathfrak{K} = \mathfrak{Z} = 0.1$ and

$\mathfrak{t} = 0.01$ in 3D.

DownLoad: Full-Size Img PowerPoint

Figure 2. Graphical comparison of the fractional-order effect on our proposed method's solutions of

$\mathfrak{P}(\mathfrak{R}, \mathfrak{K}, \mathfrak{Z}, \mathfrak{t})$ for

$\mathfrak{K} = \mathfrak{Z} = 0.1$ and

$\mathfrak{t} = 0.01$ in 2D.

DownLoad: Full-Size Img PowerPoint

Figure 3. Graphical depiction of the exact solution and our proposed method's solutions of

$\mathfrak{P}(\mathfrak{R}, \mathfrak{K}, \mathfrak{Z}, \mathfrak{t})$ for

$\mathfrak{K} = \mathfrak{Z} = 0.1$ and

$\mathfrak{t} = 0.01$ in 3D.

DownLoad: Full-Size Img PowerPoint

Figure 4. Graphical depiction of the exact solution and our proposed method's solutions of

$\mathfrak{P}(\mathfrak{R}, \mathfrak{K}, \mathfrak{Z}, \mathfrak{t})$ for

$\mathfrak{K} = \mathfrak{Z} = 0.1$ and

$\mathfrak{t} = 0.01$ in 2D.

DownLoad: Full-Size Img PowerPoint

Figure 5. Graphical comparison of the fractional-order effect on the MRPSM solution of

$\mathfrak{P}_{1}(\mathfrak{R}, \mathfrak{t})$ and

$\mathfrak{P}_{2}(\mathfrak{R}, \mathfrak{t})$ for

$\mathfrak{t} = 0.1$ in 3D.

DownLoad: Full-Size Img PowerPoint

Figure 6. Graphical comparison of the fractional-order effect on the MRPSM solution of

$\mathfrak{P}_{1}(\mathfrak{R}, \mathfrak{t})$ and

$\mathfrak{P}_{2}(\mathfrak{R}, \mathfrak{t})$ for

$\mathfrak{t} = 0.1$ in 2D.

DownLoad: Full-Size Img PowerPoint

Figure 7. Graphical comparison of the fractional-order effect on the MTIM solution of

$\mathfrak{P}_{1}(\mathfrak{R}, \mathfrak{t})$ and

$\mathfrak{P}_{2}(\mathfrak{R}, \mathfrak{t})$ for

$\mathfrak{t} = 0.1$ in 3D.

DownLoad: Full-Size Img PowerPoint

Figure 8. Graphical comparison of the fractional-order effect on the MTIM solution of

$\mathfrak{P}_{1}(\mathfrak{R}, \mathfrak{t})$ and

$\mathfrak{P}_{2}(\mathfrak{R}, \mathfrak{t})$ for

$\mathfrak{t} = 0.1$ in 2D.

DownLoad: Full-Size Img PowerPoint

Figure 9. Graphical comparison of the MRPSM and MTIM solutions for

$\mathfrak{t} = 0.1$ in 3D.

DownLoad: Full-Size Img PowerPoint

Figure 10. Graphical comparison of the MRPSM and MTIM solutions for

$\mathfrak{t} = 0.1$ in 2D.

DownLoad: Full-Size Img PowerPoint

5.2. Tabular results

presents an absolute error comparison for the solutions of example 1 using MTIM and MRPSM. The minimal error values indicate that both methods provide highly accurate solutions, making them practical for solving complex fractional PDEs. extends this error comparison to example 2, where both $\mathfrak{P}{1}$ and $\mathfrak{P}{2}$ are evaluated. Once again, the absolute errors are minimal, supporting the precision and reliability of MTIM and MRPSM for different cases. In conclusion, the graphical and tabular results strongly support the efficacy of the proposed methods. MTIM and MRPSM not only simplify the solution process for fractional PDEs, but also provide highly accurate and adaptable solutions. The methods demonstrate strong potential for application in various scientific and engineering fields requiring the analysis of fractional-order systems.

Table 1. Absolute error comparison for our proposed method's solutions for

$\mathfrak{P}(\mathfrak{R}, \mathfrak{K}, \mathfrak{Z}, \mathfrak{t})$ for

$\mathfrak{K} = \mathfrak{Z} = 0.1$ .

$\mathfrak{t}$	$\mathfrak{R}$	$MRPSM_{\mathfrak{F}=1}$	$MTIM_{\mathfrak{F}=1}$	$Exact$	$MRPSM\ Error_{\mathfrak{F}=1}$	$MTIM\ Error_{\mathfrak{F}=1}$
0.01	0.10	1.30996440544749	1.30996440544749	1.30996445073324	4.528574915063 $\times 10^{-8}$	4.528574915063 $\times 10^{-8}$
	0.35	1.68202759155083	1.68202759155083	1.68202764969888	5.814805303927 $\times 10^{-8}$	5.814805303927 $\times 10^{-8}$
	0.60	2.15976617912133	2.15976617912133	2.15976625378491	7.466357798691 $\times 10^{-8}$	7.466357798691 $\times 10^{-8}$
	0.85	2.77319466809436	2.77319466809436	2.77319476396429	9.586993199306 $\times 10^{-8}$	9.586993199306 $\times 10^{-8}$
0.03	0.10	1.23367443521972	1.23367443521972	1.23367805995674	3.624737017871 $\times 10^{-6}$	3.624737017871 $\times 10^{-6}$
	0.35	1.58406933074002	1.58406933074002	1.58407398499448	4.654254460056 $\times 10^{-6}$	4.654254460056 $\times 10^{-6}$
	0.60	2.03398528246572	2.03398528246572	2.03399125864675	5.976181022049 $\times 10^{-6}$	5.976181022049 $\times 10^{-6}$
	0.85	2.61168879985479	2.61168879985479	2.61169647342311	7.673568327426 $\times 10^{-6}$	7.673568327426 $\times 10^{-6}$
0.05	0.10	1.16180660244557	1.16180660244557	1.16183424272828	2.764028271173 $\times 10^{-5}$	2.764028271173 $\times 10^{-5}$
	0.35	1.49178920681574	1.49178920681574	1.49182469764127	3.549082552645 $\times 10^{-5}$	3.549082552645 $\times 10^{-5}$
	0.60	1.91549525789186	1.91549525789186	1.91554082901389	4.557112203484 $\times 10^{-5}$	4.557112203484 $\times 10^{-5}$
	0.85	2.45954459667798	2.45954459667798	2.45960311115694	5.851447895999 $\times 10^{-5}$	5.851447895999 $\times 10^{-5}$
0.07	0.10	1.09406933762259	1.09406933762259	1.09417428370521	1.049460826105 $\times 10^{-4}$	1.049460826105 $\times 10^{-4}$
	0.35	1.40481283712613	1.40481283712613	1.40494759056359	1.347534374536 $\times 10^{-4}$	1.347534374536 $\times 10^{-4}$
	0.60	1.80381538855917	1.80381538855917	1.80398841539785	1.730268386763 $\times 10^{-4}$	1.730268386763 $\times 10^{-4}$
	0.85	2.31614480592246	2.31614480592246	2.31636697678109	2.221708586298 $\times 10^{-4}$	2.221708586298 $\times 10^{-4}$
0.10	0.10	0.99957044701003	0.99957044701003	1.00000000000000	4.295529899697 $\times 10^{-4}$	4.295529899697 $\times 10^{-4}$
	0.35	1.28347385973080	1.28347385973080	1.28402541668774	5.515569569352 $\times 10^{-4}$	5.515569569352 $\times 10^{-4}$
	0.60	1.64801305754867	1.64801305754867	1.64872127070012	7.082131514557 $\times 10^{-4}$	7.082131514557 $\times 10^{-4}$
	0.85	2.11609065292577	2.11609065292577	2.11700001661267	9.093636869019 $\times 10^{-4}$	9.093636869019 $\times 10^{-4}$

| Show Table

DownLoad: CSV

Table 2. Absolute error comparison for our proposed method's solutions for

${{\mathfrak{P}}_{1}}({\mathfrak{R}}, {\mathfrak{t}})$ and

${{\mathfrak{P}}_{2}}({\mathfrak{R}}, {\mathfrak{t}})$ .

${\mathfrak{t}}$	${\mathfrak{R}}$	$MRPSM_{\mathfrak{F}=0.7}$	$MTIM_{\mathfrak{F}=0.7}$	$MRPSM_{\mathfrak{F}=1.0}$	$MTIM_{\mathfrak{F}=1.0}$	$\|MRPSM-MTIM\|_{\mathfrak{F}=0.7}$	$\|MRPSM-MTIM\|_{\mathfrak{F}=1.0}$
0.1	0.10	0.2499527853	0.2499526861	0.2499121337	0.2499121276	9.9242792095 $\times 10^{-8}$	6.0831994996 $\times 10^{-8}$
	0.35	0.2486293113	0.2486289768	0.2483568594	0.2483568389	3.3452402567 $\times 10^{-7}$	2.0505029479 $\times 10^{-8}$
	0.60	0.2454001942	0.2453996649	0.2449043498	0.2449043174	5.2929990035 $\times 10^{-7}$	3.2444037623 $\times 10^{-8}$
	0.85	0.2403639798	0.2403633177	0.2396597476	0.2396597070	6.6212486968 $\times 10^{-7}$	4.0585694638 $\times 10^{-8}$
0.3	0.10	0.2499229699	0.2499219730	0.2499903237	0.2499901595	9.9690462096 $\times 10^{-7}$	1.6424638585 $\times 10^{-7}$
	0.35	0.2490954767	0.2490921164	0.2488224297	0.2488218760	3.3603301558 $\times 10^{-6}$	5.5363579581 $\times 10^{-7}$
	0.60	0.2463478586	0.2463425417	0.2457429414	0.2457420654	5.3168749624 $\times 10^{-6}$	8.7598901432 $\times 10^{-7}$
	0.85	0.2417642150	0.2417575638	0.2408460059	0.2408449101	6.6511162011 $\times 10^{-6}$	1.0958137564 $\times 10^{-6}$
0.5	0.10	0.2497783238	0.2497754095	0.2499905839	0.2499898235	2.9143115888 $\times 10^{-6}$	7.6039993426 $\times 10^{-7}$
	0.35	0.2493515651	0.2493417416	0.2492122418	0.2492096786	9.8234564366 $\times 10^{-6}$	2.5631286842 $\times 10^{-6}$
	0.60	0.2469966017	0.2469810585	0.2465102198	0.2465061643	1.5543142236 $\times 10^{-5}$	4.0555046959 $\times 10^{-6}$
	0.85	0.2427855197	0.2427660760	0.2419673860	0.2419623128	1.9443610367 $\times 10^{-5}$	5.0732118353 $\times 10^{-6}$
0.7	0.10	0.2495611717	0.2495552642	0.2499129141	0.2499108276	5.9075153423 $\times 10^{-6}$	2.0865374195 $\times 10^{-6}$
	0.35	0.2494909149	0.2494710021	0.2495262957	0.2495192624	1.9912839737 $\times 10^{-5}$	7.0332251095 $\times 10^{-6}$
	0.60	0.2474879230	0.2474564159	0.2472061853	0.2471950570	3.1507046666 $\times 10^{-5}$	1.1128304885 $\times 10^{-5}$
	0.85	0.2436133438	0.2435739303	0.2430238880	0.2430099671	3.9413570944 $\times 10^{-5}$	1.3920893276 $\times 10^{-5}$
1.0	0.10	0.2491344789	0.2491219850	0.2496502911	0.2496442079	1.2493927289 $\times 10^{-5}$	6.0831994737 $\times 10^{-6}$
	0.35	0.2495497378	0.2495076238	0.2498553300	0.2498348249	4.2114079676 $\times 10^{-5}$	2.0505029473 $\times 10^{-5}$
	0.60	0.2480300251	0.2479633902	0.2481164215	0.2480839774	6.6634909492 $\times 10^{-5}$	3.2444037567 $\times 10^{-5}$
	0.85	0.2446212213	0.2445378648	0.2444869946	0.2444464089	8.3356582432 $\times 10^{-5}$	4.0585694683 $\times 10^{-5}$

| Show Table

DownLoad: CSV

6. Conclusions

In conclusion, this study has demonstrated the efficacy of MTIM and MRPSM in solving fractional-order PDEs and systems of PDEs. By employing the Caputo operator to define fractional derivatives, we have shown that these methods provide a powerful and flexible framework for tackling complex problems in applied mathematics. The results obtained through our examples confirm that MTIM and MRPSM not only simplify the solution process, but also improve the accuracy and reliability of the solutions. These methods hold significant potential for application in various scientific and engineering disciplines, particularly in situations where traditional approaches may fall short. Future work can extend these techniques to more complex and higher-dimensional problems, further establishing their utility in the field of fractional calculus and beyond.

Author contributions

A.S.A and H.Y Conceptualization, A.M.M; formal analysis, A.S.A. investigation, A.S.A. project administration, A.S.A. validation, visualization, H.Y. writing-review & editing; A.M.M; Data curation, H.Y. resources, A.M.M; validation, H.Y. software, H.Y. visualization, A.M.M; resources, H.Y. project administration, H.Y. writing-review & editing, H.Y. funding. All authors have read and agreed to the published version of the manuscript.

Funding

This work was supported by Princess Nourah bint Abdulrahman University Researchers Supporting Project number (PNURSP2024R183), Princess Nourah bint Abdulrahman University, Riyadh, Saudi Arabia. This work was supported by the Deanship of Scientific Research, Vice Presidency for Graduate Studies and Scientific Research, King Faisal University, Saudi Arabia (KFU242276).

Acknowledgments

Conflict of interest

The authors declare that they have no conflicts of interest.

References

[1]	S. R. Bhuia, A class of $C$ -normal weighted composition operators on Fock space $\mathcal{F}^2({\mathbb C})$ , J. Math. Anal. Appl., 508 (2022), 125896. https://doi.org/10.1016/j.jmaa.2021.125896 doi: 10.1016/j.jmaa.2021.125896
[2]	B. J. Carswell, B. D. MacCluer, A. Schuster, Composition operators on the Fock space, Acta. Sci. Math., 69 (2003), 871–887.
[3]	M. Chō, E. Ko, J. Lee, On $m$ -complex symmetric operators, Mediterr. J. Math., 13 (2016), 2025–2038. http://dx.doi.org/10.1007/s00009-015-0597-0 doi: 10.1007/s00009-015-0597-0
[4]	M. Chō, E. Ko, J. Lee, On $m$ -complex symmetric operators, Ⅱ, Mediterr. J. Math., 13 (2016), 3255–3264. http://dx.doi.org/10.1007/s00009-016-0683-y doi: 10.1007/s00009-016-0683-y
[5]	C. C. Cowen, E. A. Gallardo-Gutiérrez, A new class of operators and a description of adjoints of composition operators, J. Funct. Anal., 238 (2006), 447–462. https://doi.org/10.1016/j.jfa.2006.04.031 doi: 10.1016/j.jfa.2006.04.031
[6]	G. Exner, J. Jin, I. Jung, J. Lee, On $m$ -complex symmetric weighted shift operators on ${\mathbb C}^n$ , Linear Algebra Appl., 603 (2020), 130–153. https://doi.org/10.1016/j.laa.2020.05.030 doi: 10.1016/j.laa.2020.05.030
[7]	M. Fatehi, Complex symmetric weighted composition operators, Complex Var. Elliptic Equ., 64 (2019), 710–720. https://doi.org/10.1080/17476933.2018.1498087 doi: 10.1080/17476933.2018.1498087
[8]	F. Forelli, The isometries on $H^p$ , Canadian J. Math., 16 (1964), 721–728. https://doi.org/10.4153/CJM-1964-068-3 doi: 10.4153/CJM-1964-068-3
[9]	S. R. Garcia, M. Putinar, Complex symmetric operators and applications, Trans. Amer. Math. Soc., 358 (2006), 1285–1315. https://doi.org/10.2307/3845524 doi: 10.2307/3845524
[10]	S. R. Garcia, M. Putinar, Complex symmetric operators and applications, Ⅱ, Trans. Am. Math. Soc., 359 (2007), 3913–3931. https://doi.org/10.1090/S0002-9947-07-04213-4 doi: 10.1090/S0002-9947-07-04213-4
[11]	S. R. Garcia, W. Wogen, Complex symmetric partial isometries, J. Funct. Anal., 257 (2009), 1251–1260. https://doi.org/10.1016/j.jfa.2009.04.005 doi: 10.1016/j.jfa.2009.04.005
[12]	S. R. Garcia, W. Wogen, Some new classes of complex symmetric operators, Trans. Am. Math. Soc., 362 (2010), 6065–6077. https://doi.org/10.1090/s0002-9947-2010-05068-8 doi: 10.1090/s0002-9947-2010-05068-8
[13]	S. R. Garcia, E. Prodan, M. Putinar, Mathematical and physical aspects of complex symmetric operators, J. Phys. A-Math. Theor., 47 (2014), 353001. https://doi.org/10.1088/1751-8113/47/35/353001 doi: 10.1088/1751-8113/47/35/353001
[14]	A. Gupta, A. Malhotra, Complex symmetric weighted composition operators on the space $H_1^2({\mathbb D})$ , Complex Var. Elliptic Equ., 65 (2020), 1488–1500. https://doi.org/10.1080/17476933.2019.1664483 doi: 10.1080/17476933.2019.1664483
[15]	P. V. Hai, L. H. Khoi, Complex symmetry of weighted composition operators on the Fock space, J. Math. Anal. Appl., 433 (2016), 1757–1771. https://doi.org/10.1016/j.jmaa.2015.08.069 doi: 10.1016/j.jmaa.2015.08.069
[16]	J. W. Helton, Operators with a representation as multiplication by $x$ on a Sobolev space, Colloquia Math. Soc., Janos Bolyai 5, Hilbert space operators, Tihany, Hungary.
[17]	L. Hu, S. Li, R. Yang, 2-complex symmetric composition operators on $H^2$ , Axioms, 11 (2022), 358. https://doi.org/10.3390/axioms11080358 doi: 10.3390/axioms11080358
[18]	S. Jung, Y. Kim, E. Ko, J. Lee, Complex symmetric weighted composition operators on $H^2({\mathbb D})$ , J. Funct. Anal., 267 (2014), 323–351. https://doi.org/10.1016/j.jfa.2017.02.021 doi: 10.1016/j.jfa.2017.02.021
[19]	T. Le, Normal and isometric weighted composition operators on the Fock space, Bull. London Math. Soc., 46 (2014), 847–856. https://doi.org/10.1112/blms/bdu046 doi: 10.1112/blms/bdu046
[20]	R. Lim, L. Khoi, Complex symmetric weighted composition operators on $H_\gamma({\mathbb D})$ , J. Math. Anal. Appl., 464 (2018), 101–118.
[21]	S. Narayan, D. Sievewright, D. Thompson, Complex symmetric composition operators on $H^2$ , J. Math. Anal. Appl., 443 (2016), 625–630. https://doi.org/10.1016/j.jmaa.2016.05.046 doi: 10.1016/j.jmaa.2016.05.046
[22]	S. Narayan, D. Sievewright, M. Tjani, Complex symmetric composition operators on weighted Hardy spaces, Proc. Amer. Math. Soc., 148 (2020), 2117–2127. https://doi.org/10.1090/proc/14909 doi: 10.1090/proc/14909
[23]	D. Thompson, T. McClatchey, C. Holleman, Binormal, complex symmetric operators, Linear Multilinear A., 69 (2021), 1705–1715. https://doi.org/10.1080/03081087.2019.1635982 doi: 10.1080/03081087.2019.1635982
[24]	S. Ueki, Weighted composition operator on the Fock space, Proc. Amer. Math. Soc., 135 (2007), 1405–1410. https://doi.org/10.1090/s0002-9939-06-08605-9 doi: 10.1090/s0002-9939-06-08605-9
[25]	X. Yao, Complex symmetric composition operators on a Hilbert space of Dirichlet series, J. Math. Anal. Appl., 452 (2017), 1413–1419. https://doi.org/10.1016/j.jmaa.2017.03.076 doi: 10.1016/j.jmaa.2017.03.076
[26]	L. Zhao, Unitary weighted composition operators on the Fock space of ${\mathbb C}^N$ , Complex Anal. Oper. Th., 8 (2014), 581–590. https://doi.org/10.1007/s11785-013-0313-7 doi: 10.1007/s11785-013-0313-7
[27]	L. Zhao, A class of normal weighted composition operators on the Fock space of ${\mathbb C}^N$ , Acta Math. Sin., 31 (2015), 1789–1797. https://doi.org/10.1007/s10114-015-4758-7 doi: 10.1007/s10114-015-4758-7
[28]	L. Zhao, Invertible weighted composition operators on the Fock space of ${\mathbb C}^N$ , J. Funct. Space., 2015, 250358. http://dx.doi.org/10.1155/2015/250358 doi: 10.1155/2015/250358
[29]	L. Zhao, C. Pang, A class of weighted composition operators on the Fock space, J. Math. Res. Appl., 35 (2015), 303–310. http://dx.doi.org/10.1080/17476933.2019.1643332 doi: 10.1080/17476933.2019.1643332
[30]	K. H. Zhu, Analysis on Fock spaces, New York: Springer Press, 2012. Available from: http://www.springer.com/series/136

This article has been cited by:

Yousef Jawarneh, Humaira Yasmin, Ali M. Mahnashi, A new solitary wave solution of the fractional phenomena Bogoyavlenskii equation via Bäcklund transformation, 2024, 9, 2473-6988, 35308, 10.3934/math.20241678

Reader Comments

Your name:*

Email:*
© 2023 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)

通讯作者: 陈斌, bchen63@163.com

1.
沈阳化工大学材料科学与工程学院沈阳 110142

AIMS Mathematics

1.8 3.4

Metrics

Article views(1440) PDF downloads(73) Cited by(2)

Preview PDF

Download XML

Export Citation

Article outline

Show full outline

AIMS Mathematics

2-complex symmetric weighted composition operators on Fock space

Related Papers:

Abstract

1. Introduction

2. Mohand transformation

3. Mohand residual power series method

4. Uses of proposed methods

5. Results and discussion

5.1. Graphical analysis

5.2. Tabular results

6. Conclusions

Author contributions

Funding

Acknowledgments

Conflict of interest

References

This article has been cited by:

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Other Articles By Authors

Catalog

Abstract

1. Introduction

2. Mohand transformation

3. Mohand residual power series method

4. Uses of proposed methods

5. Results and discussion

5.1. Graphical analysis

5.2. Tabular results

6. Conclusions

Author contributions

Funding

Acknowledgments

Conflict of interest

References

AIMS Mathematics

2-complex symmetric weighted composition operators on Fock space

Related Papers:

Abstract

1. Introduction

2. Mohand transformation

3. Mohand residual power series method

4. Uses of proposed methods

5. Results and discussion

5.1. Graphical analysis

5.2. Tabular results

6. Conclusions

Author contributions

Funding

Acknowledgments

Conflict of interest

References

This article has been cited by:

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Other Articles By Authors

Related pages

Tools

Export File

Citation

Format

Content

Catalog

Abstract

1. Introduction

2. Mohand transformation

3. Mohand residual power series method

4. Uses of proposed methods

5. Results and discussion

5.1. Graphical analysis

5.2. Tabular results

6. Conclusions

Author contributions

Funding

Acknowledgments

Conflict of interest

References