Approximation properties of Stancu type Szász-Mirakjan operators

1.   Introduction

In recent years, approximation theory has attracted the attention of many mathematicians, especially in the field of mathematical analysis. In this context, many new positive and linear operators have been introduced and their approximate properties have been given. In this direction, Bernstein's main work on Bernstein polynomials has long maintained the primary position of approximation theory. More specifically, the Bernstein polynomial is defined as

for any $\sigma\in C[0, 1].$

In 1950, Szász ^[1] introduced the generalization of Bernstein polynomials to infinite intervals called the classical Szász-Mirakyan operators which are defined by

where $s_{n, k}(x) = e^{-nx}\cdot(nx)^{k}/k!.$

The operators $S_{n}$ have always been a research hotspot as can be seen in ^{[2,3,4,5,6,7,8]}.

In 1969, Stancu hoped to select nodes in a different way to achieve greater flexibility. In this way, he defined and studied the following linear and positive operators which are defined by ^[9]

where $x\in[0, 1], 0\leq\gamma\leq\delta$. When $\gamma = \delta = 0$, $B_{n}^{\gamma, \delta}$ become to the classical Bernstein polynomials.

Many scholars have shown great interest in the study of Stancu type operators ^[10,11,12].

Bézier curve with shape parameters is one of the important research fields of computer graphics and computer aided geometric design (CAGD). Because of its simple and stable calculation, Bézier curve is widely used in fuselage design, numerical solution of partial differential equations, networks, animation, robotics and other fields. The selection of shape parameters is important so Bézier curves and surfaces can be represented by their control grids.

Recently, Ye ^[13] presented the Bézier basis with shape parameter $\lambda\in[-1, 1]$. In this article, the authors constructed a new class of basis functions for single shape parameter curves and used these basis functions to provide a practical curve modeling algorithm. Later, Cai ^[14] introduced $\lambda$-Bernstein operators and studied some approximation properties of the operators. Finally, Cai gave some graphs and numerical examples to show the convergence of $\lambda$-Bernstein operators. They have also shown that in some cases the errors were smaller than the classical Bernstein operators. Many scholars have done research on this type of operators ^{[15,16,17,18,19,20]}.

Influenced by the construction of this type operators based on parameter $\lambda$, Qi ^[21] introduced the $\lambda$-Szász-Mirakjan operators as follows,

where $\lambda\in[-1, 1]$ and

$j = 1, 2, \cdots, \infty, x\in[0, \infty).$

In ^[21], the Korovkin type approximation theorem, the Voronovskaja-type asymptotic formula and the Grüss-Voronovskaja type theorem for the operators $S_{n, \lambda}$ were investigated. Then, Aslan ^[22,23] studied the Kantorovich type and Durrmeyer type of operators $S_{n, \lambda}$, respectively. In order to prove the accuracy and effectiveness of the discussed operators, Aslan provided a comparison of the convergence of the constructed operators for certain functions under certain parameters and provided some graphical explanations.

Based on the work of Qi and Aslan, we propose a new family of Stancu operators $S^{\gamma, \delta}_{n, \lambda}(\sigma, x)$ in the following way:

Obviously, when $\gamma = \delta = 0$ the operators $S^{\gamma, \delta}_{n, \lambda}$ reduce to the operators $S_{n, \lambda}$ defined by (1.2). When $\alpha = \beta = \lambda = 0$, the operators $S^{\gamma, \delta}_{n, \lambda}$ reduce to the operators $S_{n}$ defined by (1.1).

This article is organized in this way. First, the authors caculate the first to fourth moments of the operators. In the second section, by tools such as modulus of continuity and K-functional the approximation properties of the operators are discussed. The estimation of the Lipschitz function class by the operators is also studied. Later, the Voronvskaya type asymptotic expansion of the operators is established. Finally, we compare the convergence of these newly defined operators for certain functions with certain graphs.

2.   Some lemmas

Our results are based on the following lemmas.

Lemma 2.1. (^[21]) For $x\in[0, \infty), e_{i} = t^{i}, i = 0, 1, 2$, we have the following equalities:

Lemma 2.2. Let the operators $S^{\gamma, \delta}_{n, \lambda}$ be defined by (1.3), we have

Proof. By Lemma 2.1, we have

Using a completely similar derivation method we can get the expression of $S^{\gamma, \delta}_{n, \lambda}(e_{3}, x)$ and $S^{\gamma, \delta}_{n, \lambda}(e_{4}, x)$. Here, we omit it.

From Lemma 2.2 and simple calculation, we can get the following.

Lemma 2.3. For $x\in[0, \infty)$, we have

where $\xi_{n, \gamma, \delta, \lambda}(x)$ is defined as follows

Lemma 2.4. For $x\in[0, \infty)$, we have

Proof. Because of $S^{\gamma, \delta}_{n, \lambda}(1, x) = 1$, by Cauchy-Schwarz inequality and (2.2) we get

3.   Results

Let $C_{B}[0, \infty)$ be defined as the space of bounded and uniformly continuous functions $\sigma$ on $[0, \infty)$, endowed with the norm $\|\sigma\| = sup_{x\in[0, \infty)}|\sigma|$.

Theorem 3.1. For $\sigma\in C_{B}[0, \infty)$, $x\in [0, \infty)$, the following inequality holds

Proof. Since $S^{\gamma, \delta}_{n, \lambda}(1, x) = 1$, we get

Theorem 3.2. For $\sigma\in C_{B}[0, \infty)$, $x\in [0, \infty)$, the following equality holds

Proof. By Lemma 2.2, we get

The Korovkin theorem ^[24] is applied to obtain the conclusion.

Theorem 3.3. Let $\sigma\in C_{B}[0, \infty)$, $\tau > 0$ and

When $n$ sufficiently large we have

Proof. For $\nu > 0$ and $\rho > 0$, it is widely known that $\omega(\sigma, \nu\rho)\leq(\rho+1)\omega(\sigma, \nu)$. So, we get

The last inequality is obtained from (2.6). Let $\nu = \sqrt{\xi_{n, \gamma, \delta, \lambda}(x)}$, we get Theorem 3.3 immediately.

For $\tau > 0$ and $W^{2}[0, \infty) = \{g| g, g''\in C_{B}[0, \infty)\}$, the appropriate Peetre's K-functional is defined by

Let

where $\omega_{2}$ is the second order modulus of continuity of $\sigma\in C_{B}[0, \infty)$.

From ^[25], there exists an absolute constant $D > 0$ such that

Theorem 3.4. For $\sigma\in C_{B}[0, \infty)$ there exists an absolute constant $D > 0$ such that

Proof. We introduce the auxiliary operators

By Lemma 2.2, we get

Let $g\in W^{2}$. By Taylor's expansion, we get

Apply the operators $\widetilde{S}^{\gamma, \delta}_{n, \lambda}$ to the above equality and note that (3.5)–(3.7), we have

So,

By (3.5) and Theorem 3.1, we get

So,

Taking the infimum on the right hand side over all $g\in W^{2}$, we obtain

By the inequality of (3.3), we get Theorem 3.4 immediately.

Remark 3.1. When $\gamma = \delta = 0$, Theorem 3.4 is the form of the Theorem 3.2 of Qi ^[21].

Let $\phi(x) = \sqrt{x}$ and $\sigma\in C_{B}[0, \infty)$. The first order Ditzian-Totik modulus of smoothness and corresponding K-functional are given by

and

respectively. Here, $W_{\phi}[0, \infty) = \{g| g\in AC[0, \infty), \|\phi g'\| < \infty\}$ means that $g$ is differentiable and absolutely continuous on every compact subset of $[0, \infty)$. By ^[26], there exists a constant $E > 0$ such that

Theorem 3.5. For $\sigma\in C_{B}[0, \infty)$ there exists an absolute constant $E > 0$ such that

Proof. Applying the operators $S^{\gamma, \delta}_{n, \lambda}(\cdot, x)$ to the representation

we have

For any $x, t \in (0, \infty)$, we can get

By (2.6), we have

Thus,

Taking the infimum on the right hand side over all $g\in W_{\phi}(0, \infty)$, we can get

By (3.8) and the above inequality, we get (3.9) immediately. This completes the proof of Theorem 3.5.

Now we compute the rate of convergence of the operators $S^{\gamma, \delta}_{n, \lambda}(\sigma, x)$ for the Lipschitz class $Lip_{M}(\kappa) (0 < \kappa\leq1, M > 0)$. As usual, we say that a function $\sigma$ belongs to $Lip_{M}(\kappa)$ if the inequality

holds for all $t, x\in R$.

Theorem 3.6. For $\sigma\in Lip_{M}(\kappa)\bigcap C_{B}[0, \infty)$ and $x\in[0, \infty)$, we have

Proof. Let $e_{1} = \frac{2}{\kappa}, e_{2} = \frac{2}{2-\kappa}$. Then, $\frac{1}{e_{1}}+\frac{1}{e_{2}} = 1$. By the H$\ddot{o}$lder inequality, we get

Remark 3.2. When $\gamma = \delta = 0$, Theorem 3.6 is the form of the Theorem 3.4 of Qi ^[21].

Lastly, we will consider the Voronvskaya type asymptotic expansion of the operators $S^{\gamma, \delta}_{n, \lambda}(\sigma, x).$

Theorem 3.7. Let $\sigma', \sigma''\in C_{B}[0, \infty)$, we have

Proof. In view of Taylor's expansion formula, we have

where $\eta(t; x)$ ia a Peano of the rest term, $\eta(t; x)\in C[0, \infty)$ and $\lim_{t\rightarrow x}\eta(t; x) = 0.$

So,

By Cauchy-Schwarz inequality, we have

Noting $\eta^{2}(x; x) = 0$, $\eta^{2}(t; x)\in C[0, \infty)$ and Theorem 3.2, we get

By (2.3), (3.12) and (3.13), we get

Theorem 3.7 is obtained by (2.4), (2.5), (3.11) and (3.14).

4.   Graphical analysis

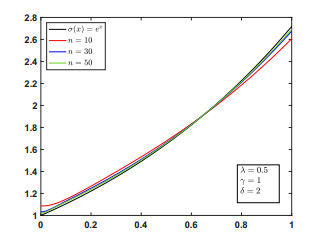
In this section, we show several graphics to present the convergence of operators (1.3) to certain functions with different values of $\gamma, \delta, n$ and $\lambda$.

In Figure 1, we choose the function $\sigma(x) = e^{x}$ (black), $\lambda = 0.9, \gamma = 1, \delta = 2$, $n = 10$ (red), $n = 30$ (blue) and $n = 50$ (green).

In Figure 2, we choose the function $\sigma(x) = cos(3\pi x)$ (black), $\lambda = 0.5, \gamma = 1, \delta = 2$, $n = 10$ (red), $n = 30$ (blue) and $n = 50$ (green).

In Figure 3, we choose the function $\sigma(x) = 3\pi x\cdot sin(3\pi x)$ (black), $\lambda = -0.9, \gamma = 2, \delta = 3$, $n = 10$ (red), $n = 50$ (blue) and $n = 90$ (green).

It is clear from Figures 1–3 that for the different values of $\lambda$ as the values of $n$ increases the convergence of operators (1.3) to the functions $\sigma(x)$ becomes better.

5.   Conclusions

In this paper, we introduce a class of Stancu type Szász Mirakjan operators and discuss their approximation properties using tools such as modulus of continuity, modulus of smoothness and K-functional. In addition, the estimation of Lipschitz function classes by the operators is also studied. Later, the Voronvskaya type asymptotic expansion of the operators is established. Finally, we give the comparison of the convergence of operators (1.3) to certain functions with some graphics.

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgments

This work is supported by the Natural Science Foundation of Fujian Province of China (Grant No. 2020J01783) and the Program for New Century Excellent Talents in Fujian Province University. We also thank Fujian Provincial Key Laboratory of Data-Intensive Computing, Fujian University Laboratory of Intelligent Computing and Information Processing and Fujian Provincial Big Data Research Institute of Intelligent Manufacturing of China. This work is also supported by the Discipline leader training programs of Yang-en University.

The authors would like to thank the referees for the helpful suggestions.

Conflict of interest

The authors declare no conflict of interest.