Time-dependent fractional second-grade fluid flow through a channel influenced by unsteady motion of a bottom plate

1.   Introduction

Fractional calculus plays a significant role in the solution of complex engineering problems. To tackle with solutions of different flow problems many researchers and scientists choose fractional model due to its important role in physical elaboration of flow problems. In contrast of ordinary differential equations (ODEs), the fractional model recovers the solution in more precise and accurate form and describes the solution of flow system at minute level. The operator used by Caputo and other scientists has involved kernel of singular form and has resulted in complex series solutions even for Newtonian fluid models. In order to overcome this complexity Fabrizio and Caputo ^[1] has introduced new operator of fractional form, the utilization of which was a simple task. Later, many researchers have used this concept for discussion of various fluid flow problems with the influence of various flow conditions and different geometries. A new fractional order derivative consisting of nonsingular kernel has introduced by Alshabanat et al. ^[2]. The authors of this study have used exponential as well as trigonometric functions in the flow problem. Hamid et al. ^[3] have studied numerically the unsteady fractional fluid flow upon a plate placed vertically subject to naturally convective thermal radiations. Singh et al. ^[4] have used the idea of fractional derivatives for determination of virus in computer system by considering epidemiological fractional model and have solved the modeled equations using the iterative method. Shah and Khan ^[5] have used the concept of Caputo-Fabrizio derivatives to discuss the heat transfer characteristics for oscillatory fluid motion past a vertical surface. In this study the exact solution has been determined for thermal and velocity profiles by using Laplace transformation. A comparative work has also been conducted in this study for integer and fractional orders where the authors have established that fractional parameter has augmented the flow of fluid. Hamid et al. ^[6] have evaluated a numerical solution efficiently, for MHD fractional model and have concluded that thermal profiles have been augmented with upsurge in radiation factor. Usman et al. ^[7] have analyzed the fractional model for Jeffery fluid past a vertical plate subject to unsteady diffusivity and conductivity. Other similar studies can be seen in Refs. ^[8,9,10].

The growing tendency in various applications at industrial level has appealed many researchers to analyze the mathematical models for non-Newtonian fluid. The important applications of these fluids at industrial level have enhanced this desire to a maximum level. The main applications of these fluids include spinning of metals, refinement of melted metals from non-metallic inclusion, shoes manufacturing (some shoes are normally filed with non-Newtonian fluid to protect feet from injury), metal extrusion, food, medicine industry etc. Many attempts have been made by different researchers to determine the exact solutions to different flow problems involving non-Newtonian fluid of second grade. Ali et al. ^[11] have determined the closed form solution for free convective unsteady fluid flow over oscillating vertical plate. The authors of this study have used Laplace transformation to determine the closed form solution of flow problem and have also discussed the influence of different substantial parameters upon velocity and temperature profiles. The closed form solution of Stokes flow problem for nanofluid has been determined by Taha et al. ^[12]. In this work the flow has induced by motion of lower plate and the partial differential equations have converted to dimensionless form by implementing Lie symmetry approach. For free convection unsteady fluid flow, the closed form solution has determined by Toki and Tokis ^[13] by employing Laplace transformation. Different cases such as impulsive flow and accelerating have also investigated in this study along with the effects of different flow parameters upon motion of fluid. Asif et al. ^[14] have determined the closed form solution for fluid flow through channel by considering different form of solution under the impact of various cases.

The flows bounded by side walls and a solid surface are the channel flows. Due to its important applications various researchers have carried out many investigations with main focus upon fluid flow characteristics. The influence of Dufour effects upon free convection fluid flow inside a vertical channel has been investigated by Jha and Ajibade ^[15]. Ingham ^[16] has determined transition flow for free convective fluid over a flat sheet in vertical form. Raptis and Singh ^[17] have discussed the MHD convective flow past a flat surface where the surface has taken to be accelerated in vertical direction. The exponential plate has used by Singh and Kumar ^[18] for fluid flow and have determined the exact solution for expressions of skin friction and velocity of fluid. The MHD fluid flow past a flat surface has deliberated by Khan et al. ^[19] along with the influence of side walls upon the flow of fluid. Haq et al. ^[20] discussed flow of MHD fluid over a penetrable sheet. The authors of this study have investigated the influence of side boundaries over the liquid flow by considering the limiting cases for second grade fluid. Fetecau ^[21] has investigated the analytical solution for flow of fluid in a pipe. The author has used the Steklov expansion theorem to determine the exact solution for modeled equations. Bandelli and Rajagopal ^[22] have inspected the second grade fluid flow in a finite and unidirectional domain. The Fourier and Laplace transformations have applied by the authors to determine the closed form solution. Fetecau et al. ^[23] have used the Fourier sine transformation for exact solution of time dependent second grade fluid past a sheet. Different cases imposed upon the bottom plate have considered by the authors by addressing the limiting cases under the influence of side boundaries. Fetecau and Zierep ^[24] have determined the analytical solutions for fluid flow upon a surface. In this studt a sudden jerk has given to the bottom plate for inducing motion into the fluid and Fourier transformation has used for finding exact solution.

From the above cited literature and other similar work, it has revealed that many investigations have been conducted for second grade fluid along with the determination of exact solutions by using different geometries. But no work has yet been conducted for exact solution of fractional second grade fluid flow through channel, which is the main novelty in the current work and is also a source of attraction for authors to conduct this investigation. In this study the authors have made an endeavor to determine the exact solution at the first time for second grade fractional fluid flowing in a channel by incorporating the combination of Laplace and Fourier transformations. The momentum equation is converted into dimensionless form by using set of suitable variables. The exact solution of resultant equations has been determined by employing Laplace and Fourier transformations ^[25,26]. After obtaining the general exact solutions for flow characteristics, three different cases at the surface of bottom plate are discussed; namely (i) Stokes first problem (ii) Accelerating flow (iii) Stokes second problem. The time fractional differential operator of Caputo-Fabrizio has employed to have constitutive equations of fractional order for second grade fluid. After recovering the exact solution for different cases we have then used Mathcad-15 for discussion of graphical view of the momentum equation.

2.   Problem description

Since the equation of motion for Newtonian as well as non-Newtonian fluid is described as ^[20]

In Eq (1) $T$ is the Cauchy stress tensor which can mathematically be expressed for second grade fluid as follows

In Eq (2) $\mathbf{I}$ is the identity tensor, $p$ is pressure, ${\alpha _1}, \, {\alpha _2}$ are normal stress moduli and ${A_1}, \, \, {A_2}$ are first two Rivlin-Ericken kinematic tensors and can be expressed mathematically as

In above equations $\mathbf{L}$ presents the gradient of velocity while $\left(* \right)$ is used for matrix transposition.

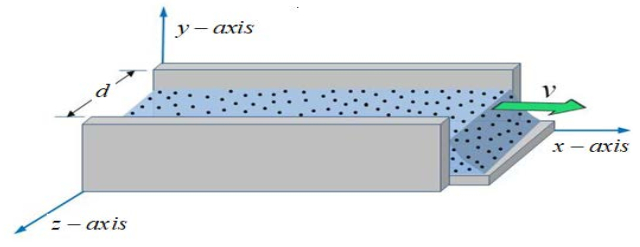
In this problem we have considered a time-dependent flow of fractional second grade fluid past an endless plate bounded by two side walls with a distance ' $d$ 'between them. These walls at the sides are normal to the plate at the bottom but are parallel to each other. The bottom plate supports the fluid flow because the plate along with fluid is at rest for $t = 0$ while with $t = \mathop 0\limits^ +$ the plate at the bottom gets into motion with time-dependent flow ${U_0}f\left(t \right)$ such that $f\left(0 \right) = 0$ . The flow of fluid is unidirectional which is mathematically described as $V = u\left({y, \, z, \, t} \right)i$ with $v = w = 0$ (see Figure 1).

The equation that governs the flow problem is mathematically expressed as ^[14,20]

From above we have

The related conditions at boundaries are described as ^[27]

In above equations $\rho$ is density, $\mu$ is dynamic viscosity, while $f\left(t \right) \ne 0$ for $t \gt 0$ and $f\left(0 \right) = 0$

For the purpose of converting the momentum equation and initial, boundary conditions into dimensionless form, the following set of variables is used ^[27]

By substituting Eq (8) in Eqs (5) and (6) we obtain the following set of equations ^[20,27]

The conditions defined at boundaries along with initial condition are

In above equations the symbol ${d_0}$ presents specified length, $\alpha = \frac{{{\alpha _1}}}{{\rho {d_0}}}$ is dimensionless parameter for second grade fluid and Re $= \frac{{{U_0}{d_0}}}{v}$ portrays Reynolds number.

The constitutive equations for fractional derivative in generalized form are

3.   Problem solution

Applying Laplace transform to Eqs (9) and (10) and employing Eq (12) we have

$s\bar u = \frac{{\partial {{\bar \tau }_{xy}}}}{{\partial y}} + \frac{{\partial {{\bar \tau }_{xz}}}}{{\partial z}}$ and ${\bar \tau _{xy}} = \frac{1}{ \mathrm{Re} }\frac{{\partial \bar u}}{{\partial y}} + \left({\frac{{\alpha s}}{{\left({1 - \beta } \right)s + \beta }}} \right)\frac{{\partial \bar u}}{{\partial y}}, \, \, {\bar \tau _{xz}} = \frac{1}{ \mathrm{Re} }\frac{{\partial \bar u}}{{\partial z}} + \left({\frac{{\alpha s}}{{\left({1 - \beta } \right)s + \beta }}} \right)\frac{{\partial \bar u}}{{\partial z}}$

After simplification of above equation we have

The conditions given in Eq (11) after using Laplace transform we have

Multiplying the term $\sqrt {\frac{2}{\pi }} \int\limits_0^\infty {\int\limits_0^L {\sin \left({y\zeta } \right).\sin \left({{\psi _n}z} \right)} } dydz$ with Eq (13) and employing Eq (14) with ${\psi _n} = \frac{{n\pi }}{L}$ we have

Rearrange Eq (15) to get

Using Laplace inversion in combination of convolution theorem we have after simplification

Following relations have been incorporated in Eq (17) which are constant terms and have been used for convenience. These terms are carried out till the end of solutions with slight changes in notation only.

Now in light of Fourier inversion formulae, Eq (17) has simplified to following form

In above equation $m = 2n - 1$ whereas

Substitute $L = 2h$ and transform the axis of coordinates by putting $z = h + {z^*}, \, \, \, {\gamma _m} = \frac{{\pi \left({2n - 1} \right)}}{{2h}}$ while $\, {\gamma _m} = {\psi _m}$ in Eq (19) which simplifies to

In Eq (21) the following relations have been incorporated

4.   Cases of interest

Next different cases will be considered keeping in mind the side walls of the flow system

4.1.   When the gap between the side walls is maximum i.e. $h \to \infty$

Whenever the gap between the walls of the system is maximized the flow will remain unaltered due to these side walls, in such situation Eqn. (21) simplifies to

In above equation, the following relations have been incorporated

4.2.   Stokes first problem when $f\left(t \right) = H\left(t \right)$

Replace $f\left(t \right)$ by $H\left(t \right)$ in Eq (21) where $H\left(t \right)$ is named as unit step function, we will have in this case

By removing the side walls we will have mathematically as $h \to \infty$ , in this situation Eq (24) reduces as

4.3.   For $f\left(t \right) = {t^a}$ (for accelerating plate)

By employing $f\left(t \right) = {t^a}$ in Eq (21) the following relation has obtained

Equation (26) depicts the solution of an accelerated case for fluid flow. Specifically if the bottom plate is moving with a constant acceleration then one can have $f\left(t \right) = t$ . With this value, Eq (21) simplifies to

For limiting case we have $h \to \infty$ , for which the flow field is expressed as

4.4.   For $f\left(t \right) = {e^{it\omega }}H\left(t \right)$

It is to be noticed that if the bottom plate is moving with some oscillation then the fraction fluid will have oscillational velocities denoted by ${u_c}$ and ${u_s}$ . These oscillating velocities correspond to cosine as well as sine oscillations and we have

Now if the effect of the side walls upon the motion of fractional fluid is ignored then as a limiting case we have $h \to \infty$ and in such a case we have from above equations

5.   Limiting case

It is obvious that by taking $'\beta \to 1$ our fractional second grade fluid model reduces to the solutions obtained by Haq et al. ^[20] that validates our solutions.

By using the property we have:

Using the above limiting case in all the fractional order solutions we see that our solution has reduced to published results as given in Ref ^[20] which verifies and validates our solutions as a limiting case.

6.   Discussion of results

This investigation theoretically describes the exact solution of an unsteady fractional fluid flow upon a bottom plate bounded by two side walls. The flow is induced by the time dependent motion of the bottom plate. Initially the flow equation along with boundary and initial conditions are considered which are then transformed to dimensionless notations using suitable set of variables. The Laplace as well as Fourier transformations have been employed to recover the exact solution of flow equation. The time fractional differential operator of Caputo-Fabrizio has been employed to have constitutive equations of fractional order for second grade fluid. After obtaining the general exact solutions for flow characteristics, three different cases at the surface of bottom plate have been discussed.

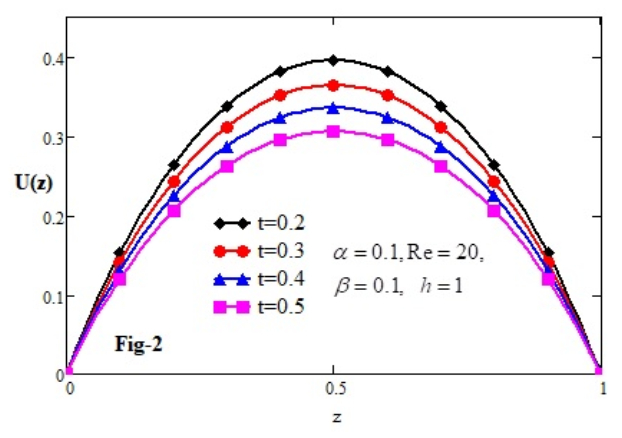
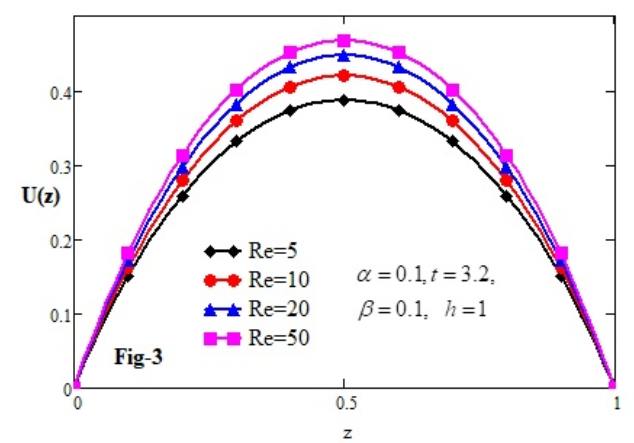
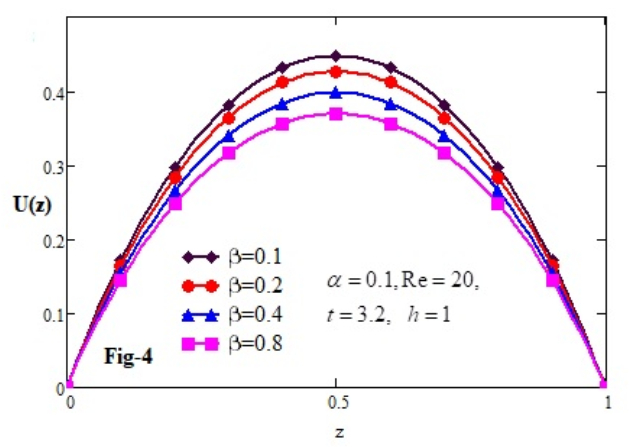
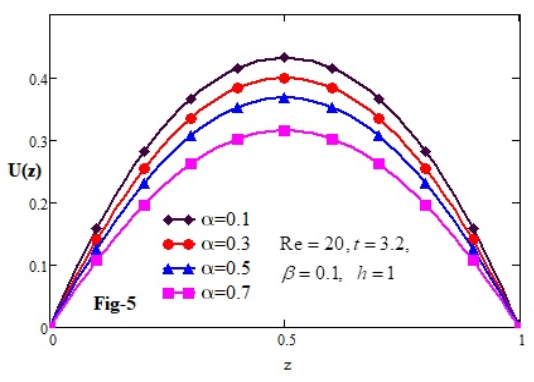
Figures 2–5 describe the flow profiles for Stokes first problem. It has noticed from Figure 2 that augmentation in the values of time variable $\left(t \right)$ results in decay in fractional fluid flow profiles for $\alpha = 0.1, \, \mathrm{Re} = 20, \, \beta = 0.1, \, h = 1.0$ . Figure 3 portrays the influence of Reynolds number $\left( \mathrm{Re} \right)$ upon flow profiles. Since it is the ratio of inertial to viscous forces so for augmentation in values of $\left( \mathrm{Re} \right)$ up to a specific range the behavior of fluid flow is laminar so flow profiles will augment in such case by keeping $\alpha = 0.1, \, t = 3.2, \, \beta = 0.1, \, h = 1.0$ as depicted in Figure 3. Form Figures 4 and 5 it has noticed that there is an inverse relationship between fractional parameter $\left(\beta \right)$ and second grade parameter $\left(\alpha \right)$ , because flow is a declining function of these parameters.

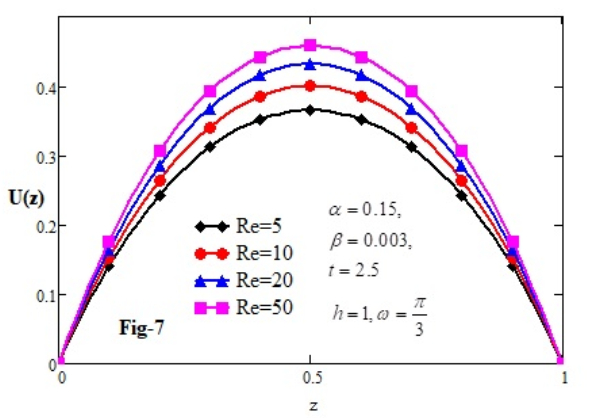
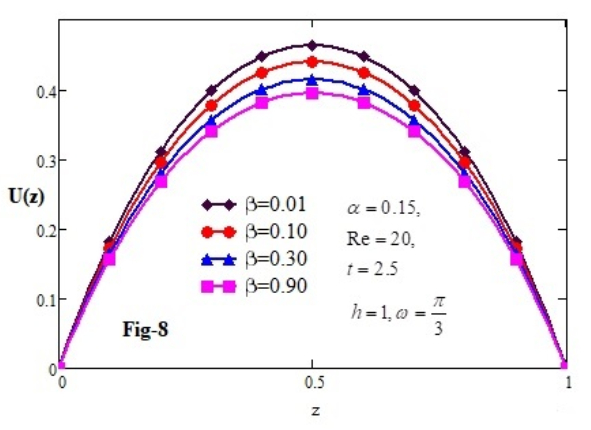
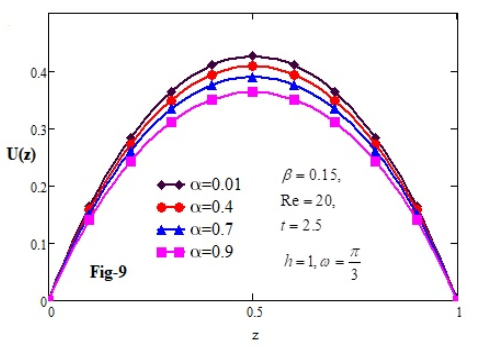
Figures 6–9 depict the flow characteristics for constantly accelerated flow of fractional fluid. It has observed from Figure 6 that by keeping $\alpha = 0.1, \, \, \beta = 0.1$ and $\mathrm{Re} = 10$ with augmentation in time variable $\left(t \right)$ the fractional fluid motion declines within the prescribed domain. Similar to Stokes first problem in response of augmentation in Reynolds number $\left( \mathrm{Re} \right)$ the flow characteristics jumps up by considering $\alpha = 0.15, \, \, \beta = 0.003, \, \, t = 2.5, \, \, h = 1.0, \, \, \omega = \pi /3$ as depicted in Figure 7. It has also concluded that with augmentation in fractional parameter $\left(\beta \right)$ and second grade parameter $\left(\alpha \right)$ there is a reduction in momentum of fractional fluid. So an inverse relationship between flow characteristics and these parameters exists as presented in Figures 8 and 9.

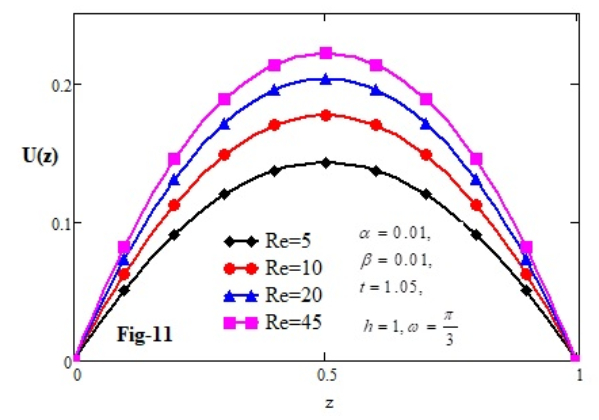
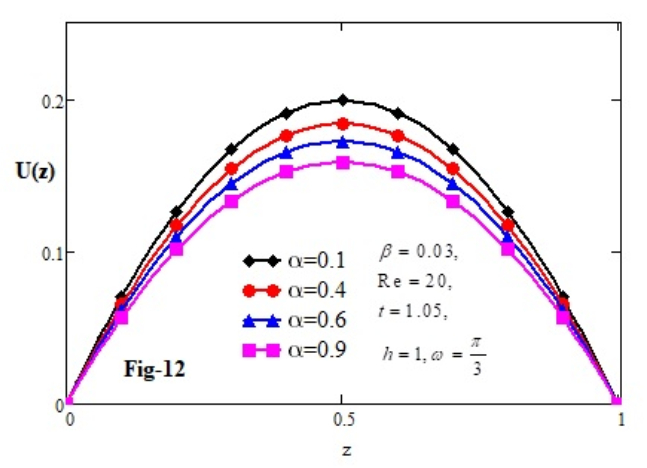
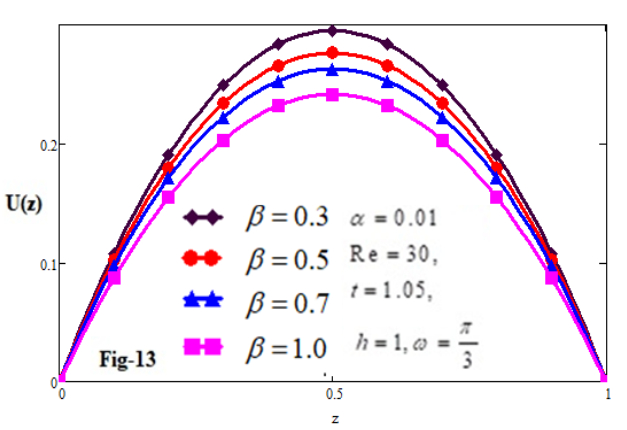
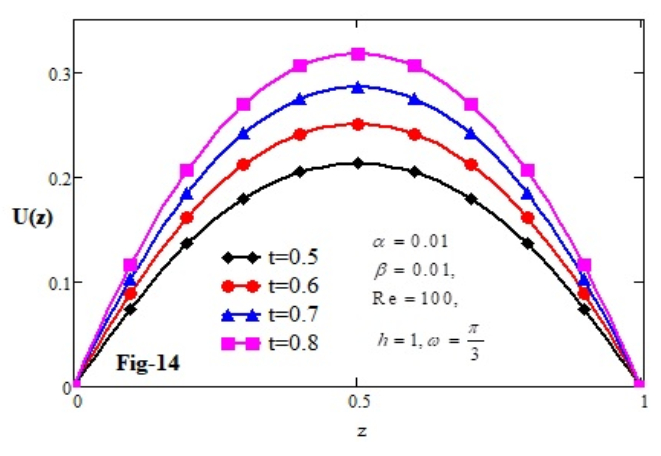
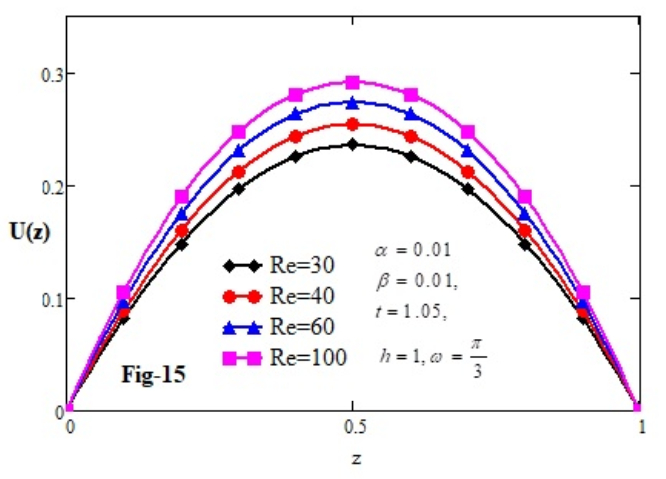
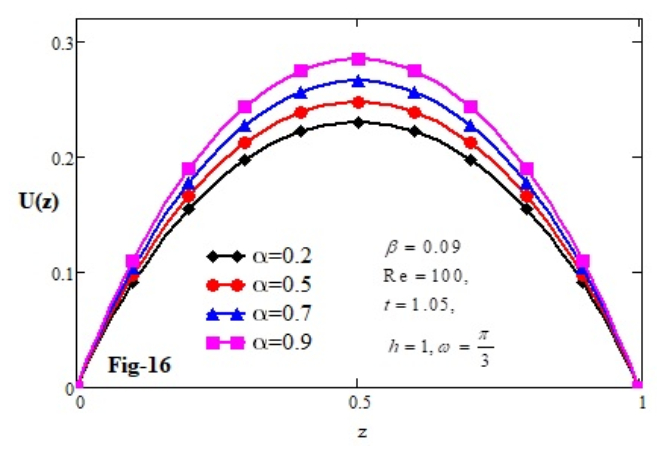
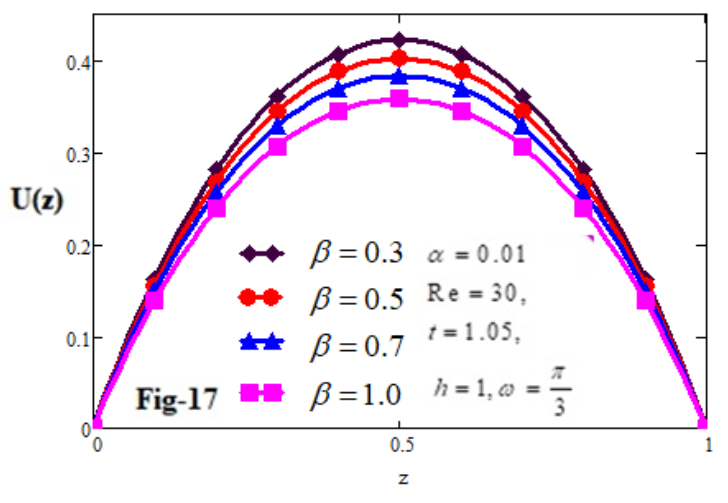
Figures 10–13 exhibit the behavior of flow characteristics in response of variations in different parameters for sine oscillation. Figures 10 and 11 depict that augmentation in time variable and Reynolds number results a corresponding growth in fraction second grade fluid motion for fixed values of the parameters $\alpha = 0.01, \, \, \beta = 0.01, \, \mathrm{Re} = 20, \, \, h = 1, \, \, \omega = \pi /3\,$ in case of variations in time, and $\alpha = 0.01, \, \, \beta = 0.01, \, t = 1.05, \, \, h = 1, \, \, \omega = \pi /3$ in case of variations in Reynolds number. On the other hand Figures 12 and 13 show that flow profiles of fractional fluid reduced with augmentation in the values of fractional parameter $\left(\beta \right)$ and second grade parameter $\left(\alpha \right)$ . Actually with augmenting values of these parameters the momentum boundary layer thickness reduced. The influence of different parameters upon flow profiles for cosine oscillations is presented in Figures 14–17. A similar behavior for fractional fluid motion is observed in response of variations in different flow parameters. Furthermore, it is of worth noting that Figures 13 and 17 are plotted to compare the fractional solutions to the classical solutions for $\left({\beta = 1} \right)$ which are reduced to the solutions obtained by Haq et al. ^[20] that validates our obtained solutions.

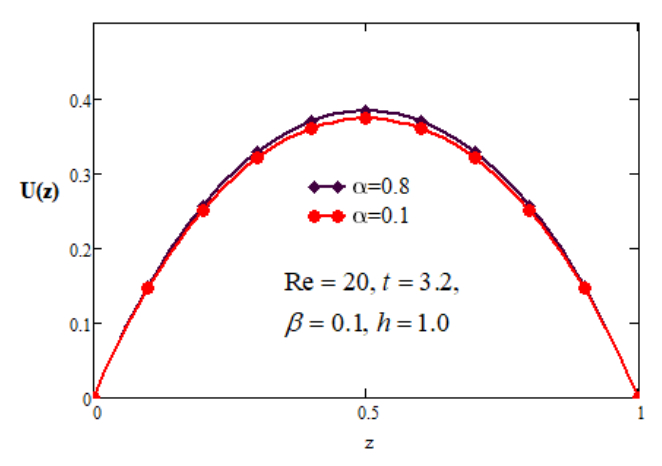
In Figure 18 a graphical comparison between $\alpha = 0.8, \, \, \alpha = \, 1.0$ has been carried out in current investigation by taking other parameter values as Re $= 20, \, \, t = 3.2, \, \, \beta = 0.1, \, \, h = 1.0$ .

7.   Conclusions

This investigation theoretically describes the exact solution of an unsteady fractional fluid flow upon a bottom plate bounded by two side walls. The flow is induced by the time dependent motion of the bottom plate. Initially the flow equation along with boundary and initial conditions are considered which are then transformed to dimensionless notations using suitable set of variables. The Laplace as well as Fourier transformations have been employed to recover the exact solution of flow equation. After obtaining the general exact solutions for flow characteristics, three different cases at the surface of bottom plate have been discussed with the help of graphical view. The following points are concluded after an insight of the problem:

● It has established in this investigation that augmentation in time variable declines the flow profiles for impulsive and constantly acceleration cases. On the other hand a growth in flow characteristics has been observed for higher values of time variable for both sine and cosine oscillations.

● Growth in Reynolds number results an augmentation in the flow characteristics for impulsive and constantly acceleration as well as for sine and cosine oscillations.

● It has also concluded that augmentation in fractional parameter and second grade parameter supports the flow profiles in all the three cases namely Stokes first problem, accelerating flow and Stokes second problem.

Nomenclature

Acknowledgment

The author (Z. Raizah) extends her appreciation to the Deanship of Scientific Research at King Khalid University, Abha, Saudi Arabia, for funding this work through the Research Group Project under Grant Number (RGP.1/334/43).

Conflict of interest

The authors have declared no conflict of interest.