1.
Introduction
Let A be an additive category. An idempotent morphism e2=e:A→A in A is said to be split if there are two morphisms p:A→B and q:B→A such that e=qp and pq=1B. The category A is said to be idempotent complete if every idempotent morphism splits. Note that A is idempotent complete if and only if every idempotent morphism has a kernel if and only if every idempotent morphism has a cokernel, see [1]. Every additive category A can be embedded fully faithfully into an idempotent complete category ˜A. Balmer and Schlichting [2] proved that the idempotent completion of a triangulated category is a triangulated category. Bühler showed that the idempotent completion of an exact category is an exact category. Liu and Sun [4] showed that the idempotent completion of a right triangulated category is again right triangulated.
Recently, suspended categories were introduced by Li in [3] as a simultaneous generalization of exact categories, triangulated categories and right triangulated categories. In this article, we will unify these conclusions stated above by showing that when A is a suspended category then the idempotent completion of A is also a suspended category.
2.
Preliminaries
We first recall some notions and facts on the idempotent completion of additive categories.
Definition 2.1. [2, Definition 1.2] Let A be an additive category. The idempotent completion of A is denoted by ˜A which be defined as follows. The objects of ˜A are pairs (A,p), where A is an object of A and p:A→A is an idempotent morphism. A morphism in ˜A from (A,p) to (B,q) is a morphism f:A→B such that qf=fp=f. For any object (A,p) in ˜A, the identity morphism 1(A,p)=p.
Remark 2.2. [1, Remark 6.3] Let A be an additive category and ˜A be an idempotent complete of A. The biproduct in ˜A is defined as
There exists a fully faithful additive functor ℓA:A→˜A defined as follows. For an object A in A, we have that ℓA(A)=(A,1A) and for a morphism f in A, we have that ℓA(f)=f. Since the functor ℓA is fully faithful, we can view A as a full subcategory of ˜A.
Proposition 2.3. [1, Proposition 6.10] Let A be an additive category and B be anidempotent complete category. For every additive functor F:A→B, there exists a functor ˜F:˜A→˜B and a natural isomorphism ϕ:F⇒˜FℓA.
Now we recall the notion of suspended categories from [3].
Let A be an additive category and X be a full subcategory of A. Recall that we say a morphism f:A→B in C is an X-monic if
is an epimorphism for all X∈X. Similarly, we say that f is a left X-approximation of A if f is an X-monic and B∈X. The subcategory X is said to be covariantly finite in A, if every object in A has a left X-approximation. The notions of left X-approximation and covariantly finite subcategories are also known as X-preenvelope and preenveloping subcategories, respectively.
Let A be an additive category with an additive endofunctor Σ:A→A and X⊆C be two full subcategories of A. A right Σ-sequence Af→Bg→Ch→ΣA in A is called a right C-sequence if C∈C, g is a weak cokernel of f (i.e. the induced sequence HomA(C,A)→HomA(B,A)→HomA(A,A) is exact) and h is a weak cokernel of g.
Dually, a left Σ-sequence ΣAf→Bg→Ch→A is called a left C-sequence if B∈C, f is a weak kernel of g and g is a weak kernel of h.
Definition 2.4. [3, Definition 3.1] Let A be an additive category with an additive endofunctor Σ:A→A and X⊆C be two full subcategories of A. A triple (A,R(C,Σ),X) is a right suspended category where R(C,Σ) is a class of right C-sequences (whose elements are also called right C-triangles) if R(C,Σ) is closed under isomorphisms and finite direct sums and the following conditions are satisfied:
(RS1) (a) For any A∈C, there exists a sequence Ai⟶X⟶U⟶Σ(A) in R(C,Σ) where i is an X-preenvelope such that for any morphism f:A⟶B in C, there exists a sequence
in R(C,Σ).
(b) For any morphism f:A⟶B in C, there exists a sequence
in R(C,Σ).
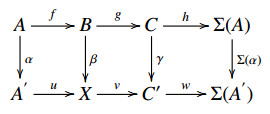
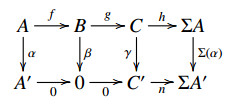
(RS2) For any commutative diagram of sequences in R(C,Σ)
with X∈X, if α factors through f, then γ factors through v.
(RS3) For each solid commutative diagram
with rows in R(C,Σ), the dotted morphism exists which makes the whole diagram commutative.
(RS4) If any three sequences
are in R(C,Σ) and f,g are X-monic, then there exists two morphisms α:D⟶F and β:F⟶E of C, such that the diagram below is commutative:
where the third column from the left is in R(C,Σ), with α is an X-monic.
Dually, we can define the notion of a left suspended category.
Now we give some examples of right suspended categories from [3].
Example 2.5. (1) If (A,Σ,Δ) is a right triangulated category, we take X=0,C=A and R(A,Σ)=Δ. Then the triple (A,R(A,Σ)=Δ,0) is a right suspended category. We know that any triangulated category can be viewed as a right triangulated category. Hence any triangulated category can be viewed as a right suspended category.
(2) Let (A,E) be an exact category and
Then (A,R(A,Σ=0),A) is a right suspended category.
(3) Let (A,E) be an exact category with enough injectives. We denote by I the full subcategory of all injectives objects in A. Then (A,R(A,Σ=0),I) is a right suspended category, where
We collect some useful lemmas which can be used in the sequel.
Lemma 2.6. Assume (A,R(C,Σ),X) be satisfies (RS1), (RS2), (RS3). If
are in R(C,Σ), then there exists an isomorphism γ:C⟶C′ which makes the following diagram commutative:
Proof. It can be proved in a similar way as in [3, Lemma 3.2]
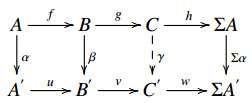
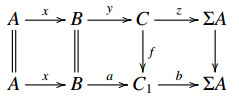
Lemma 2.7. Let (A,R(C,Σ),X) be a right suspended category.Given a commutative diagram
with rows in R(C,Σ).If p:A→A and q:B→B are idempotent morphisms, then there exists an idempotent morphism α:C→C such that the diagram
commutes.
Proof. The proof is very similar to [2, Lemma 1.13], we omit it.
3.
Idempotent completion of right suspended categories
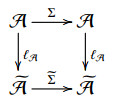
Let (A,R(C,Σ),X) be a right suspended category. Then the additive endofunctor Σ of A induces the endofunctor ˜Σ of idempotent completion ˜A given by ˜Σ(A,e)=(ΣA,Σe). Moreover, it is easy to see that there is a commutative diagram
Clearly, ℓA(C)⊆˜C, and ℓA(X)⊆˜X.
We define a right ˜Σ-sequence in ˜A,
to be a right ˜C-sequence in R(˜C,˜Σ) if there is a right ˜C-sequence in R(˜C,˜Σ)
such that Δ⊕Δ′ is isomorphic to a right C-sequence in R(C,Σ) or equivalently, it is a direct summand of a right C-sequence in R(C,Σ). It is easy to see that R(˜C,˜Σ) is closed under isomorphisms and finite direct sums. For convenience, we usually write ˜Σ as Σ.
Lemma 3.1. Let (A,R(C,Σ),X=0) be a right suspended category. A sequence
is a right C-sequence in R(C,Σ) if and only if both two sequences
are right C-sequences in R(C,Σ).
Proof. Since R(C,Σ) is closed under finite direct sums, it is enough to show the necessity. By axiom (RS1), there are two right C-sequences in R(C,Σ)
By axiom (RS3), there exists a commutative diagram
Thus, we have fy=a and bf=z. Similarly, one can find a morphism f′:C′→C′1 such that f′y′=a′ and and b′f′=z′. Hence, we have the following commutative diagram
By Lemma 2.6, we know that (f00f′) is an isomorphism. It follows that f and f′ are isomorphisms. It is easy to see that there exists a commutative diagram
where the second row lies in R(C,Σ). It follows that Ax→By→Cz→ΣA lies in R(C,Σ). Similarly, we can show that A′x′→B′y′→C′z′→ΣA′ lies in R(C,Σ).
Now we state and prove our main result in this article.
Theorem 3.2. Let Σ be an endofunctor when restricted to C,(A,R(C,Σ),X=0) be a right suspended category. Then the triple (˜A,R(˜C,˜Σ),˜X=0) is a right suspended category.
Proof. We will check the axioms of suspended categories.
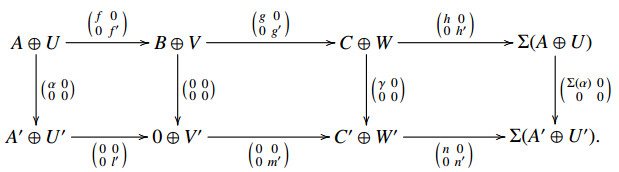
(RS1) (a) Let A be an arbitrary object in ˜C. Then there is A′ in ˜C such that A⊕A′∈C actually, if A=(N,e) take A′=(N,idN−e) we have A⊕A′≅ℓA(N)). Note that A⊕A′0⟶0⟶0⟶Σ(A⊕A′) is a right C-sequence in R(C,Σ). It is clear that 0 is an X-preenvelope. By the definition of right ˜C-sequences in ˜A, we obtain A0⟶0⟶0⟶Σ(A) with 0 is an ˜X-preenvelope.
For any morphism f:A→B in ˜C, there exists two objects A′,B′∈˜C such that A⊕A′,B⊕B′∈C. For the morphism A⊕A′(f000)→B⊕B′ in C, by axiom (RS1)(a), there exists a right C-sequence
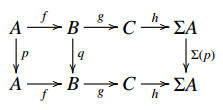
in R(C,Σ). By Lemma 2.7, there exists an idempotent morphism p=p2:N→N which makes the following diagram commutative:
Therefore, the sequence Af→Bpa1→(N,p)a2p→Σ(A) is in R(˜C,˜Σ).
(b) For each morphism f:A→B in ˜C, there are two objects A′,B′∈˜C such that A⊕A′,B⊕B′∈C. For the morphism A⊕A′(f000)→B⊕B′ in C, by axiom (RS1)(b), there is a right C-sequence in R(C,Σ)
which guarantees
is a right ˜C-sequence in R(˜C,˜Σ).
(RS2) For any two right ˜C-sequences
lies in R(˜C,˜Σ). For any commutative diagram
with α factors through f. Next we will prove γ=0, thus we are done.
By the definition of right ˜C-sequences, there are two right ˜C-sequences
lie in R(˜C,˜Σ). Taking the direct sum of right ˜C-sequences (3.2) and (3.4), we get a right ˜C-sequence
in R(˜C,˜Σ) such that (3.6) is isomorphic to a right C-sequence in R(C,Σ).
Similarly, taking the direct sum of right ˜C-sequences (3.3) and (3.5), we get a right ˜C-sequence
in R(˜C,˜Σ) such that (3.7) is isomorphic to a right C-sequence in R(C,Σ). Thus we have a commutative diagram in R(C,Σ)
Note that (α000) factors through (f00f′) since α factors through f, hence (γ000) factors through (000m′). In particular, we have
which implies γ=0.
(RS3) For any two right ˜C-sequences
in R(˜C,˜Σ), the diagram below with the leftmost square is commutative
Next we will prove that there exists a morphism γ:C⟶Z which makes the whole diagram commutative in ˜A. By the definition of right ˜C-sequences, there exists right C-sequence Δ′,Γ′ and morphisms i:Δ⟶Δ′,p:Δ′⟶Δ,j:Γ⟶Γ′,q:Γ′⟶Γ, such that pi=1Δ,qj=1Γ, which induce a morphism j∘(α,β)∘p:Δ′⟶Γ′ in A, since Δ′ and Γ′ are right C-sequence in R(C,Σ). According to axiom (RS3), we have a right C-sequence map u:Δ′⟶Γ′, which induces a right C-sequence morphism q∘u∘i:Δ⟶Γ extending (α,β) in R(˜C,˜Σ).
(RS4) For any three right ˜C-sequence Af⟶Bl⟶Di⟶Σ(A),Bg⟶Ch⟶Ej⟶Σ(B) and Agf⟶Ck⟶Em⟶Σ(A) are in R(˜C,˜Σ), with f,g are X-monics in ˜C. For the morphism f:A⟶B in ˜C, there exists A′,B′ in ˜C, such that A⊕A′,B⊕B′ in C, Clearly
and
are right ˜C-sequences in R(˜C,˜Σ). Take the direct sum of right triangles (3.8)–(3.10), we get the following right ˜C-sequence:
By the proof of (RS1), we know that any morphism in C can be embedded into a right C-sequence, since the morphism A⊕A′(f000)→B⊕B′ in C, therefore, it can be extended to a right C-sequence (3.1). By Lemma 2.6, (3.11) is isomorphic to (3.1) in R(C,Σ).
Similarly, the following right ˜C-sequence
is isomorphic to a right C-sequence in R(C,Σ). Since the morphism gf:A→C in ˜C, similar to above, the following right ˜C-sequence
is isomorphic to a right C-sequence in R(C,Σ).
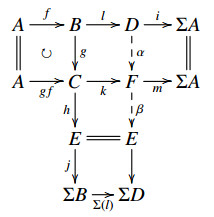
By axiom (RS4), we can get the following commutative diagram in A:
where the third column is a right C-sequence in R(C,Σ) and h1 is an X-monic.
We write
According to the above commutative diagram, we have
Hence
According to h2∘h1=0, we have
Thus we obtain
For the object F⊕C′⊕Σ(A′), there are morphisms u,v:F⊕C′⊕Σ(A′)⟶F⊕C′⊕Σ(A′) where
such that u and v are inverse of each other. Therefore we can get a commutative diagram as follows:
Note that
we obtain the right ˜C-sequence Da11→Fb11→EΣ(l)∘j→Σ(D) in R(˜C,˜Σ).
Therefore, we can get the following commutative diagram in ˜A:
where a11 is an ˜X-monic.
This completes the proof.
Remark 3.3. In Theorem 3.2, when A=C is a triangulated category, it is just Theorem 1.5 in [2]; when A=C is an exact category, it is just Proposition 6.13 in [1]; when A=C is a right triangulated category, it is just Theorem 2.14 in [4].
4.
Conclusions
In this article, we show that the idempotent completion of a right suspended category has a natural structure of right suspended category and dually this is true for a left suspended category.
Acknowledgments
The author would like to thank the anonymous reviewers for their comments and suggestions.
Conflict of interest
The author declares no conflict of interests.











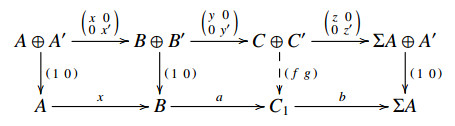
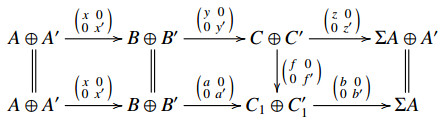

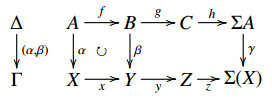

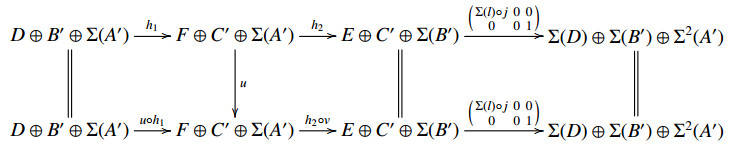





 DownLoad:
DownLoad: