Figure 1.
Structure of University English Online Teaching Mode.
In this paper, we prove some coincidence point theorems for weak C-contractions and K-contractions involving a new auxiliary function in a metric space endowed with a locally f-transitive binary relation. In this context, we generalize some relevant fixed point results in the literature. Further, we give an example to substantiate the utility of our results.
Citation: Faruk Sk, Asik Hossain, Qamrul Haq Khan. Relation-theoretic metrical coincidence theorems under weak C-contractions and K-contractions[J]. AIMS Mathematics, 2021, 6(12): 13072-13091. doi: 10.3934/math.2021756
| [1] | Wei Wu, Shicheng Luo, Hongying Wang . Design of an automatic landscape design system in smart cities based on vision computing. Mathematical Biosciences and Engineering, 2023, 20(9): 16383-16400. doi: 10.3934/mbe.2023731 |
| [2] | Meijuan Sun . A Vision sensing-based automatic evaluation method for teaching effect based on deep residual network. Mathematical Biosciences and Engineering, 2023, 20(4): 6358-6373. doi: 10.3934/mbe.2023275 |
| [3] | Boyi Zeng, Jun Zhao, Shantian Wen . A textual and visual features-jointly driven hybrid intelligent system for digital physical education teaching quality evaluation. Mathematical Biosciences and Engineering, 2023, 20(8): 13581-13601. doi: 10.3934/mbe.2023606 |
| [4] | Liguo Zhang, Liangyu Zhao, Yongtao Yan . A hybrid neural network-based intelligent body posture estimation system in sports scenes. Mathematical Biosciences and Engineering, 2024, 21(1): 1017-1037. doi: 10.3934/mbe.2024042 |
| [5] | Faten S. Alamri, Khalid Haseeb, Tanzila Saba, Jaime Lloret, Jose M. Jimenez . Multimedia IoT-surveillance optimization model using mobile-edge authentic computing. Mathematical Biosciences and Engineering, 2023, 20(11): 19174-19190. doi: 10.3934/mbe.2023847 |
| [6] | Yinhua Su . Visualization design of health detection products based on human-computer interaction experience in intelligent decision support systems. Mathematical Biosciences and Engineering, 2023, 20(9): 16725-16743. doi: 10.3934/mbe.2023745 |
| [7] | YoungSu Yun, Mitsuo Gen, Tserengotov Nomin Erdene . Applying GA-PSO-TLBO approach to engineering optimization problems. Mathematical Biosciences and Engineering, 2023, 20(1): 552-571. doi: 10.3934/mbe.2023025 |
| [8] | H. Y. Swathi, G. Shivakumar . Audio-visual multi-modality driven hybrid feature learning model for crowd analysis and classification. Mathematical Biosciences and Engineering, 2023, 20(7): 12529-12561. doi: 10.3934/mbe.2023558 |
| [9] | Ziyou Zhuang . Optimization of building model based on 5G virtual reality technology in computer vision software. Mathematical Biosciences and Engineering, 2021, 18(6): 7936-7954. doi: 10.3934/mbe.2021393 |
| [10] | Zhipeng Ding, Hongxia Yun, Enze Li . A multimedia knowledge discovery-based optimal scheduling approach considering visual behavior in smart education. Mathematical Biosciences and Engineering, 2023, 20(3): 5901-5916. doi: 10.3934/mbe.2023254 |
In this paper, we prove some coincidence point theorems for weak C-contractions and K-contractions involving a new auxiliary function in a metric space endowed with a locally f-transitive binary relation. In this context, we generalize some relevant fixed point results in the literature. Further, we give an example to substantiate the utility of our results.
With the continuous advancement of information technology and graphical technology, traditional teaching models have become difficult to cope with the rapidly developing teaching needs. How to enable students to learn and obtain information more vividly and concretely in a shorter period of time has become an important problem to be solved in multimedia teaching in universities. The multimedia system establishes a complete set of multimedia teaching systems by integrating sound, graphics and images. Teachers collect data after class, create slides and write teaching content. By accumulating a large amount of data before, good teaching effects can be achieved, and students can also more intuitively and vividly understand the teacher's teaching intentions and content through various media in the classroom. Avoiding a large amount of obscure and difficult to understand knowledge, making classroom teaching more vivid and specific. Therefore, designing a multimedia system can make the teaching and learning process more convenient in university classrooms, and can better improve the teaching system. The system controls the output of video and audio devices through multimedia terminal devices. First, the teacher creates and edits the teaching courseware, and then copies the created files onto the teaching computer through a disk. Transfer the generated video and audio files to the output device through computers and audio devices. In this way, the teaching content is transmitted to students through the teacher, simplifying the basic teaching process and enriching the teaching content, making the teacher's teaching content more vivid and specific.
The unsound course network system has also brought some serious problems, which make the online teaching effect unable to surpass the opposite teaching effect in a short time. Students can enter the classroom by scanning the QR code or link issued by the teacher, and the teacher can check the attendance through the sign in function attached to Tencent classroom. On the basis of students' preview, students should fully consider the actual situation of teachers, such as time, energy and enthusiasm; It can also encourage teachers to consciously form a teaching and research community, and share and negotiate with each other on the success or failure of online teaching. The teacher systematically explains key knowledge, such as vocabulary phrases, grammatical sentence patterns, writing frameworks, theme ideas, etc., and organizes students to brainstorm about the writing theme [1,2]. Online and offline hybrid courses, virtual simulation personalized courses and other high-quality courses, and implement a hybrid teaching mode [3]. The online English teaching mode based on video and image technology can expand teaching time and space, create a situation for autonomous learning and then lay a foundation for cultivating students' language ability. All regions actively respond to and participate in the reform, and accelerate the construction of online teaching platform and teacher training.
University English teachers can adopt more diversified online teaching modes when teaching writing, mobilize students' enthusiasm, turn dull into lively and interesting and let students really participate in the whole process of "teaching and learning", from "playing with mobile phones" to "learning with mobile phones", so as to obtain better learning results [4]. Use video image technology to design new online English teaching ideas and processes, pay attention to interactive and personalized training methods, make full use of video image technology to participate in teaching, stimulate students' interest in learning, enrich classroom content, focus students' attention, improve students' reading and answering ability, dig into students' potential learning ability, break through the key and difficult points of teaching, optimize the classroom structure and then show an efficient classroom [5]. Realize the human culture of online English learning website system in video image technology. The construction of websites and systems should reflect the humanistic spirit, achieve the balanced development of virtuality and authenticity, so that the interaction between people is no longer a simple "machine" and "machine" interaction, but through high-end technology to make people closer in spirit and spirit. The innovations in this paper are as follows:
● This paper constructs the functional structure diagram of the video image technology teacher module. The humanization of multimedia environment is proposed, and the network learning system provided for students should have strong stability; Create a humanistic environment that can attract students to participate. This kind of interaction can help students understand the learning situation of their peers, reflect on their own writing problems and get real and effective feedback from many aspects, which greatly stimulates students' enthusiasm for learning to write, promoting the in-depth promotion of English online teaching, deepening the content of textbooks, highlighting cultural differences in teaching.
● This paper analyzes the English online teaching process based on video and image technology. The design of online English teaching process structure fully considers the teacher's leading activities, students' participation activities, the organization of teaching content, the use of teaching media and other aspects as well as their interrelationships. Formative exercises are an important part of classroom teaching, so the design of teaching process structure can be used to describe, which means that teachers, students, content, media and exercises should be designed first and then organically combine all parts through the flow chart.
The overall structure of this paper consists of five parts. Section one describes the background and significance of online university english teaching. The second section mainly introduces the current situation of university English online teaching research and the research content of video image technology in university English online teaching. The third section describes the video image technology of online college english teaching mode and discusses the implementation scheme in online college english teaching. The fourth section is the experimental part of this paper. The fifth section is the summary of the full text.
With the rapid development of the Internet, university English audio-visual teaching is facing great challenges, but at the same time it enriches its teaching methods and contents. The network multimedia interactive teaching mode has become the mainstream teaching mode of university English audio-visual classroom teaching. As long as there is network support, online courses can enable students to study anytime and anywhere. Therefore, many scholars have made research on online college English teaching.
Meng F believes that online teaching separates teaching time and space from teaching behavior, and the content, rhythm and method of traditional face-to-face to ensure that under the condition of separation of time and space, teaching still plays a positive role in promoting learning [6]. Lin X, et al. There are many students in the college English classroom. Different students have different English foundations, and each student has different learning motivation and purpose, which leads to low efficiency in the overall classroom [7]. Li F, et al. show that online teaching is a new teaching form, which has many advantages and great particularity compared with traditional classroom, which determines that online teaching of university English must adopt different methods and strategies from traditional classroom [8]. Juan D, et al. By integrating Chinese traditional culture into the development and construction of online university English courses, it helps English learners to inherit Chinese traditional culture in their communication with the outside world, absorb and introduce excellent cultural ideas, and cultivate college students' feelings of home and country and social responsibility, so as to promote the innovation and development of Chinese traditional culture [9]. Li F, et al. showed that in order to better understand the differences between different platforms tested the functions of online teaching and live broadcast platforms according to the technical support and operational performance of the teaching platforms and teachers' operational ability, and selected the appropriate platforms to carry out teaching [10].
Horowitz K S put forward that university is an important stage of students' development. Whether students can get correct guidance at this stage will directly determine whether students can form correct values and become a useful talent for society in the future [11]. De Jager L, et al. put forward that online English teaching needs to make more efficient and rational use of the Internet, and strive to stimulate students' learning enthusiasm and initiative through virtual and multi-dimensional communication, ensure the effectiveness of teacher-student interaction, accurately record and evaluate the learning process and carry out timely and efficient teaching feedback [12]. Vdovina E, et al. Set up a teaching platform through Superstar Learning, import the list of students, select courseware or reference books from the resource library release recorded course videos as micro-courses, and set the opening hours, which is powerful and easy to implement [13]. Donitsa-Schmidt S, et al. shows that today, the content and form of moral education in English should also be reformed. How to continue to fulfill the mission of English moral education in the new situation is an important topic that English teachers should study deeply [14]. Syakur A, et al. Based on the characteristics of online teaching, taking university English courses as an example, relying on Tencent's classroom live broadcast platform, learning is used to effectively manage the classroom, supplemented by process evaluation, so as to ensure the high-quality and efficient operation and development of online college english teaching from three dimensions [15].
Online teaching separates teaching time and space from teaching behavior. The content, rhythm and method of traditional face-to-face University English teachers can adopt more diversified online teaching modes when teaching writing, mobilize students' enthusiasm, turn dull into lively and interesting and let students really, from "playing with mobile phones" to "learning with mobile phones", so as to obtain better learning results learning when time and space are separated. This paper applies video image processing technology to university English online teaching and learning, which can provide rich feedback information for specific tasks to be completed at each stage, provide scientific tools for As we all know, the university English classroom. Different students have different English foundations, and each student has different learning motivation and purpose, which leads to low classroom efficiency as a whole. University English online teaching has made some achievements, but many classroom teaching models still focus on teachers' speaking and students' listening, but also needs to cultivate students' broader vision, so that students can understand the diversity of human culture, and have a deeper understanding of human history and values.
In the video image technology environment, using schema theory, the knowledge of individual implicit teaching strategies is expressed by means of visual representation to form explicit knowledge products that can directly affect the interaction process and results, thus promoting the dissemination and innovation of knowledge. The real-time interactive information includes text, voice, image, etc. English teachers should have the awareness of moral education, be able to extract appropriate moral education themes and materials from social life, and then choose appropriate ways to combine them with English teaching. The teachers will give guidance on the spot, and at the same time use the video image technology professional software to analyze the students' technical parameters, so as to provide guidance and suggestions for students' training, these will greatly improve the English teaching effect.
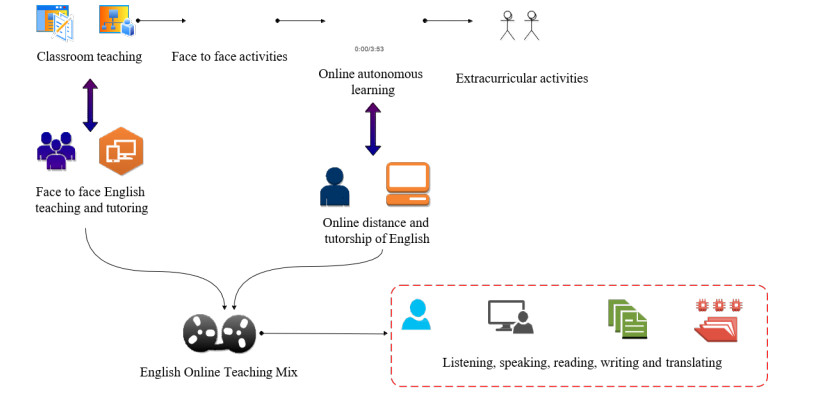
The online teaching mode should be supported by modern information technology, especially network technology, so that different students have different English foundations, and each student has different learning motivation and purpose, which leads to low classroom efficiency as a whole [16]. University English online teaching has made some achievements, but many classroom teaching models still focus on teachers' speaking and students' listening. We have designed a practical university English online teaching model structure in the framework of mixed learning model, as shown in Figure 1.
The online teaching mode of university English writing mainly relies on Tencent classroom live broadcast platform, Welearn accompanying classroom and class QQ group. The curriculum design includes three parts: preparation before class, live lecture and feedback after class.
1) Preparation stage before class: Students are always the ultimate goal of all teaching. We should fully understand students' personal factors and external environment, and provide students with a mixed learning atmosphere suitable for their development. Students preview and read relevant materials independently, and discuss the tasks and thinking questions assigned by teachers in the study group in advance. If there is a topic to discuss, you can make a mind map on your mobile phone or computer in advance, and then share it in class to provide support for improve the classroom teaching effect.
2) Live lecture stage: During the live lecture, students enter the classroom by scanning the QR code or link issued by the teacher, and the teacher can check the attendance through the sign-in function that comes with Tencent classroom. Based on students' preview, students should fully consider the actual situation of teachers, such as time, energy and enthusiasm; It can also encourage teachers to consciously form a community of teaching and research, and share and consult with each other on the success or failure of online teaching. Teachers systematically explain key knowledge such as vocabulary phrases, grammatical sentence patterns, writing framework, theme ideas, etc., and organize students to brainstorm on writing topics. Students actively participate in the discussion in the speech area, express their own views and comment on the views of other students, and finally form their own writing ideas, so that they have a fixed outline when writing after class, and only need to organize the language to complete a complete composition.
3) Feedback stage after class: Teachers organize students to peer-evaluate each other in QQ learning group, teachers release evaluation criteria in advance and students judge classmates' compositions one-on-one according to the criteria. The humanity of multimedia environment. The network learning system provided for students should have strong stability; Create a humanistic environment that can attract students' participation. This kind of interaction can help students understand their peers' learning situation, reflect on their own writing problems and get real and effective feedback from many aspects, which greatly stimulates students' enthusiasm for learning writing. Finally, students can also review the live content through the review function of Tencent classroom, so that students who can't participate in live learning due to network problems or personal reasons can achieve full coverage.
There are still many problems in university English online teaching at this stage, online and offline hybrid courses, virtual simulation personalized courses and other high-quality courses and implement a hybrid teaching mode [17]. The online English teaching mode based on video and image technology can expand teaching time and space, create a situation for autonomous learning and then lay a foundation for cultivating students' language ability. All regions actively respond to and participate in the reform, and accelerate teacher training students' learning. This should be considered in depth when allocating resources on campus with video and image technology. When allocating funds, we should give them a certain preference. Only with sufficient funds, can online classroom construction be free of worries [18]. You can query, add, delete or modify the specified teacher's class. The system will list the qualified class information table according to the entered query criteria. Then the administrator can add, delete or modify the teacher's class information. If it is not successful, you can re operate. The functional structure of the teacher module is shown in Figure 2.
At the same time, it should also be equipped with an excellent network technology team on campus. Technical personnel are the guarantee of online classroom construction, operation and management, and relevant staff can be supplemented by off-campus recruitment. Video technology has outstanding advantages in English online classroom, It shows that students are always the ultimate goal of all teaching. We should fully understand students' personal factors and external environment, and provide students with a mixed learning atmosphere suitable for their development. Students preview and read relevant materials independently, and discuss the tasks and thinking problems assigned by teachers in the study group in advance. If there is a topic to discuss, you can make a mind map on your mobile phone or computer in advance mobilize students' participation and interaction, speed up students' understanding and application of knowledge and more effectively improve students' sense of knowledge acquisition [19]. According to the functional requirements of video image technology, this paper describes the English online teaching architecture of video image technology, which mainly includes four levels: content database, student data, content organization and content display. The database of content database contains four tables, namely, content database for listening, content database for speaking, content database for reading and content database for writing. The table structure of online English teaching content library is shown in Table 1.
| Field Name | Data type | Describe |
| ID | Automatic numbering | Content number |
| Type | Text | Types of exercises |
| Rank | Text | Grade of exercises |
| Scope | Text | Exercise module |
DownLoad:
CSV
The student data layer is to input the basic information of each student and the quantity limit of the Teachers systematically explain key knowledge such as vocabulary phrases, grammatical sentence patterns, writing framework, theme ideas, etc., and organize students to brainstorm on writing topics. Students actively participate in the discussion in the speech area, express their own views. On-line English teaching content organization layer is to use video image technology to organize a set of learning content for students according to the input conditions of students' data layer. In this respect, we can consider giving full play to the advantages of students of related majors on campus, which can not only build online classrooms, but also provide jobs for students of related majors on campus, killing two birds with one stone. Video technology multiplies the general form is:
| h(x)=w0x0+w1x1+…+wmxm | (3.1) |
It is expressed as:
| h(x)=wTx(2) | (3.2) |
When the value is greater than the set threshold, it is considered as a positive category, and when the value is less than the threshold, it is considered as a negative category, namely:
| g(z)=11+e−wx | (3.3) |
For each sample, the posterior probability is:
| p(y∣x,w)=p(y=1∣xi) | (3.4) |
Then the maximum likelihood function of the sample is the posterior probability product of each sample, namely:
| L(w)=p(yi=1∣xi) | (3.5) |
Logarithmic likelihood function:
| I(w)=logp(yi=1∣xi) | (3.6) |
| I(w)i=logp(yi=1∣xi)vi+log | (3.7) |
It is expanded and solved, and the derivative of w is obtained.
| ∂1(w)∂w=m∑i=1xi | (3.8) |
Let the derivative be 0, we can see that w cannot be solved, so we need to optimize the algorithm to solve w.
Online teaching through video and image technology is composed of teachers, students, teaching content, teaching media and other elements. According to the theory of system science, forming a more perfect organizational structure. In order to achieve the best effect of teaching. The flow chart of online English teaching based on video image technology is shown in Figure 3. Because the structure of English online teaching process is the form of the relationship and connection among teachers. Use video image technology to design new online English teaching ideas and processes, pay attention to interactive and personalized training methods, make full use of video image technology to participate in teaching, stimulate students' interest in learning, enrich classroom content, focus students' attention, improve students' reading and answering ability, dig into students' potential learning ability, break through students are always the ultimate goal of all teaching. We should fully understand students' personal factors and external environment, and provide students with a mixed learning atmosphere suitable for their development. Students preview and read relevant materials independently, and discuss the tasks and thinking questions assigned by teachers in the study group in advance. If there is a topic to discuss, you can make a mind map on your mobile phone or computer in advance, and then share it in class to provide support for improve the classroom teaching effect during the live broadcast, optimize the classroom structure and then show an efficient classroom, so the design of teaching process structure can be used to describe it, which means that teachers, students, content, media and exercises are designed first, and then all parts are organically combined through flow charts. The information entropy of data set D is:
| H=−y∑k=1pklog2 | (3.9) |
where H represents the information entropy of dataset D, y represents the number of categories in dataset. The information gain of a feature in the data set is the difference between the information entropy of the data set D and the empirical condition entropy of D under the given conditions of the feature:
| H(D,a)=H−v∑v=1H(DV) | (3.10) |
The calculation attribute splitting formula is:
| H(D,a)=H(D,a)H(D) | (3.11) |
| H(D,a)ratio =H(D,a)H(D)max | (3.12) |
The formula for calculating the Gini index of dataset D is:
| Gini(D)=1−y∑k=1 | (3.13) |
where y represents the number of categories of dataset D.
Gini index is calculated for characteristic a in training data set, and its formula is as follows:
| Gini =v∑v=1Gini(Dv) | (3.14) |
where v represents the number of feature a values. Find the feature that minimizes the error, which is the optimal split point. The square error formula is:
| ∑xi∈Rmyi−f(xi) | (3.15) |
At the same time, the online English teaching video through video image technology is conducted without interference to students, and students' real teaching materials are directly obtained.
According to the purpose of learning English online in college, there are four situations: liking English (A), needing English communication (B), improving comprehensive ability and quality (C) and being competitive in work or going abroad (D). According to the degree of interest in English, it can be seen from Figure 4 that students still agree with the importance of English as a skill in their future development, but their interest in English learning is not strong enough. According to the learning attitude, it can be divided into three situations: often actively seeking various opportunities to learn English (a), occasionally actively seeking opportunities to learn English (b), and never actively learning or speaking English even in class (c). After analyzing the data, more than 72% of students actively seek opportunities to learn English occasionally. As can be seen from Figure 5, students are not active enough in learning English and most students actively seek opportunities occasionally.
The basic situation of online English teaching learners used in this experiment is that their English level is intermediate, their learning purpose is to work and their occupations are financial trade. The initial parameters of the experiment are set as follows: a set of learning contents consists of 20 units, each unit consists of three parts: listening, speaking and reading. The requirements of each part are shown in Table 2. Let the initial population size, that is, the total number of learning contents generated initially, be, and both the crossover probability and the mutation probability are generated randomly. According to the offline English learning time, Almost no learning, just learning in class. It can be seen from Figure 6 this part of students do not have a fixed time to learn English, and they almost stop learning English when their lessons are tight. Some students think that learning English has a lot to do with their mood.
| Module | Exercise Type | Quantity |
| Aural comprehension | Teacher's explanation | 2 |
| Choice question | 11 | |
| Completion | 6 | |
| Spoken language | Teacher's explanation | 2 |
| Oral Practice | 11 | |
| Read | Teacher's explanation | 2 |
| Fill in the blank | 12 |
DownLoad:
CSV
According to English learning styles, there are four situations: reading English magazines, going to English clubs, writing English emails and watching English movies. As can be seen from Figure 7, more than 72% of the students learn English by watching English movies. Few students choose English corners, clubs, mail, letters and other ways to learn English, mainly by memorizing words, doing exercises and reading papers. Most of the existing online English learning predictions for students are based on online assessment, and then randomly grade students according to the assessment results, and then arrange courses for students to learn. Some English online learning students do not even predict, so all students learn the same course. Although online English learning has made a certain grade division for students, it is also randomly divided by the evaluation results. According to the above clustering results, we can get the score ranges of English listening, speaking, reading and courses corresponding to different grades. The specific results are shown in Table 3.
| Preparatory level | Entry level | Grade qualified | Mastery level | Proficiency level | |
| Llisten to | [0, 46] | [47,68] | [68,76] | [76,80] | [80,84] |
| Say | [0, 43] | [43,68] | [68,78] | [78,80] | [80,85] |
| Read | [0, 56] | [43,63] | [63,70] | [70,83] | [83,86] |
DownLoad:
CSV
In this experiment, the ways of online English teaching and training and improving oral English ability are studied, and the following four ways are obtained: group discussion, film dubbing, role-playing and deskmate discussion. As can be seen from Figure 8, 48.32% of students think that group discussion can practice oral English, followed by film dubbing (25.71%) and role-playing (19.53%), and finally table discussion (13.55%). From the data, it can be reflected that each student has chosen the way that suits him according to his own situation.
Through the research on the application and analysis of video image technology in university English online teaching, this chapter has carried out the simulation experiment content. University English teachers can adopt more diversified online teaching modes when teaching writing, mobilize students' enthusiasm, turn dull into lively and interesting, and let students really participate in the whole process of "teaching and learning", from "playing with mobile phones" to "learning with mobile phones", so as to obtain better learning results. Realize the human culture of online English learning website system in video image technology carefully select the materials that meet the traditional Chinese culture, and use video image technology to make online course resources, such as making micro course courseware, PPT courseware, small videos, cultural stories, reference materials, etc. The construction of websites and systems should reflect the humanistic spirit, achieve the balanced development of virtuality and authenticity, so that the interaction between people is no longer a simple "machine" and "machine" interaction, but through high-end technology to make people closer in spirit and spirit. Use video image technology to design new online English teaching ideas and processes, pay attention to interactive and personalized training methods, make full use of video image technology to participate in teaching, stimulate students' interest in learning, enrich classroom content, focus students' attention, improve students' reading and answering ability, dig into students' potential learning ability, break through the key and difficult points of teaching, optimize the classroom structure, and then show an efficient classroom.
Video image technology provides useful help for students to learn fragmentary chemistry. Realize the human culture of online English learning website system in video image technology. The construction of websites and systems should reflect the humanistic spirit, achieve the balanced development of virtuality and authenticity, so that the interaction between people is no longer a simple "machine" and "machine" interaction, but through high-end technology to make people closer in spirit and spirit. Teachers can push the online course resources of video and image technology to students, and students need to complete the corresponding topics or tasks while watching; Teachers can also observe students' learning feedback in the background and get students' English feelings and English homework related to traditional Chinese culture.
With the support of modern information and network technology, online teaching has become an important supplement to the traditional teaching forms in universities, and it has achieved initial results. University English teachers should actively carry out moral education in combination with social hot events while completing the subject teaching tasks. The real-time interactive information includes text, voice, image, etc. English teachers should have the awareness of moral education, be able to extract appropriate moral education themes and materials from social life, and then choose appropriate ways to combine them with English teaching. The research shows that 48.32% of students think that group discussion can practice oral English, followed by film dubbing (25.71%) and role playing (19.53%), and table discussion (13.55%). From the data, it can be reflected that each student has chosen the way that suits him according to his own situation. During the online teaching of English subject through video image technology, students should be given moral education. English teachers should give priority to guiding education, choose the right time and accurate content, so that students can experience and feel noble emotions and great spirits while paying attention to the society, build a platform for them to form good moral qualities, and let students gradually form good moral qualities The teachers will give guidance on the spot, and at the same time use the video image technology professional software to analyze the students' technical parameters, so as to provide guidance and suggestions for students' training, these will greatly improve the English teaching effect transformation of English classroom teaching in higher vocational colleges.
The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.
The authors declare there is no conflict of interest.
| [1] |
S. Banach, Sur les operations dans les ensembles abstraints er leur application aux equations intgrales, Fund. Math., 3 (1922), 133–181. doi: 10.4064/fm-3-1-133-181
|
| [2] |
A. Ali, H. Işık, H. Aydi, E. Ameer, J. R. Lee, M. Arshad, On multivalued SU-type θ-contractions and related applications, Open Math., 18 (2020), 386–399. doi: 10.1515/math-2020-0139
|
| [3] |
A. Ali, F. Uddin, M. Arshad, M. Rashid, Hybrid fixed point results via generalized dynamic process for F-HRS type contractions with application, Phys. A. Stat. Mech. Appl., 538 (2020), 122669. doi: 10.1016/j.physa.2019.122669
|
| [4] | A. Ali, M. Alansari, F. Uddin, M. Arshad, A. Asif, G. A. Basendwah, Set-valued SU-type fixed point theorems via Gauge function with applications, J. Math., (2021), 6612448. |
| [5] | R. Kannan, Some results on fixed points, Bull. Calcutta Math. Soc., 60 (1968), 71–76. |
| [6] | S. K. Chatterjea, Fixed-point theorems, Comptes Rendus de l'Académie Bulgare des Sci., 25 (1972), 727–730. |
| [7] | B. S. Choudhury, Unique fixed point theorem for weak C-contractive mappings, Kathmandu Univ. J. Sci., Eng. Technol., 5 (2009), 6–13. |
| [8] |
A. Razani, V. Parvaneh, Some fixed point theorems for weakly T-Chatterjea and weakly T-Kannan-contractive mappings in complete metric spaces, Russ. Math. (Izv. VUZ), 57 (2013), 38–45. doi: 10.3103/S1066369X13030055
|
| [9] |
A. Alam, M. Imdad, Relation-theoretic contraction principle, J. Fixed Point Theory Appl., 17 (2015), 693–702. doi: 10.1007/s11784-015-0247-y
|
| [10] | M. U. Ali, Y. Guo, F. Uddin, H. Aydi, K. Javed, Z. Ma, On partial metric spaces and related fixed point results with applications, J. Funct. Spaces, (2020), 6671828. |
| [11] | P. Gopi, K. Deepak, Fixed point theorems in relational metric spaces with an application to boundary value problems, J. Part. Differ. Equ., 34 (2021), 83–93. |
| [12] | F. Uddin, C. Park, K. Javed, M. Arshad, J. R. Lee, Orthogonal m-metric spaces and an application to solve integral equations, Adv. Differ. Equ., (2021), 159. |
| [13] |
A. Alam, M. Imdad, Nonlinear contractions in metric spaces under locally T-transitive binary relations, Fixed Point Theory, 19 (2018), 13–24. doi: 10.24193/fpt-ro.2018.1.02
|
| [14] |
H. A. Hammad, M. De la Sen, A solution of Fredholm integral equation by using the cyclic ηqs-rational contractive mappings technique in b-metric-like spaces, Symmetry, 11 (2019), 1184. doi: 10.3390/sym11091184
|
| [15] | H. A. Hammad, M. De la Sen, Solution of nonlinear integral equation via fixed point of cyclic αψL-rational contraction mappings in metric-like spaces, Bull. Braz. Math. Soc. New Ser., 51 (2020), 81–105. |
| [16] | H. A. Hammad, M. De la Sen, A coupled fixed point technique for solving coupled systems of functional and nonlinear integral equations, Mathematics, 7 (2019), 634. |
| [17] |
G. Jungck, Commuting maps and fixed points, Amer. Math. Mon., 83 (1976), 261–263. doi: 10.1080/00029890.1976.11994093
|
| [18] | G. Jungck, Common fixed points for noncontinuous non self maps on non-metric space, Far. East. J. Math. Sci., 4 (1996), 199–215. |
| [19] | S. Lipschutz, Schaum's outlines of theory and problems of set theory and related topics, McGraw-Hill, New York, 1964. |
| [20] | R. D. Maddux, Relation algebras, studies in logic and the foundations of mathematics, Elsevier, Amsertdam, 2006. |
| [21] | V. Flaˇska, J. Jeˇzek, T. Kepka, J. Kortelainen, Transitive closures of binary relations I, Acta Univ. Carolin. Math. Phys., 48 (2007), 55–69. |
| [22] |
H. L. Skala, Trellis theory, Algebr. Univ., 1 (1971), 218–233. doi: 10.1007/BF02944982
|
| [23] |
A. Stouti, A. Maaden, Fixed point and common fixed point theorems in pseudo-ordered sets, Proyecciones, 32 (2013), 409–418. doi: 10.4067/S0716-09172013000400008
|
| [24] | B. Samet, M. Turinici, Fixed point theorems on a metric space endowed with an arbitrary binary relation and applications, Commun. Math. Anal., 13 (2012), 82–97. |
| [25] | B. Kolman, R. C. Busby, S. Ross, Discrete mathematical structures, 3 Eds, PHI Pvt. Ltd., New Delhi, 2000. |
| [26] | A. Alam, M. Imdad, Relation-theoretic metrical coincidence theorems, Filomat, 31 (2015), 693–702. |
| [27] | S. Sessa, On a weak commutativity conditon of mappings in fixed point consideration, Publ. Inst. Math. Soc., 32 (1982), 149–153. |
| [28] |
G. Jungck, Compatible mappings and common fixed points, Int. J. Math. Sci., 9 (1986), 771–779. doi: 10.1155/S0161171286000935
|
| [29] | A. F. Roldán-López-de-Hierro, E. Karapinar, M. de la sen, Coincidence point theorems in quasi-metric spaces without assuming the mixed monotone property and consequences in G-metric spaces, Fixed Point Theory Appl., (2014), 184. |
| [30] |
J. Harjani, B. López, K. Sadarangani, Fixed point theorems for weakly C-contractive mappings in ordered metric spaces, Comput. Math. Appl., 61 (2011), 790–796. doi: 10.1016/j.camwa.2010.12.027
|
| 1. | Yong Liu, 2023, English Teaching Ability Evaluation Algorithm based on K-Means Data Algorithm, 979-8-3503-0082-6, 1, 10.1109/NMITCON58196.2023.10275848 |
Faruk Sk, Asik Hossain, Qamrul Haq Khan. Relation-theoretic metrical coincidence theorems under weak C-contractions and K-contractions[J]. AIMS Mathematics, 2021, 6(12): 13072-13091. doi: 10.3934/math.2021756
| Field Name | Data type | Describe |
| ID | Automatic numbering | Content number |
| Type | Text | Types of exercises |
| Rank | Text | Grade of exercises |
| Scope | Text | Exercise module |
DownLoad:
CSV
| Module | Exercise Type | Quantity |
| Aural comprehension | Teacher's explanation | 2 |
| Choice question | 11 | |
| Completion | 6 | |
| Spoken language | Teacher's explanation | 2 |
| Oral Practice | 11 | |
| Read | Teacher's explanation | 2 |
| Fill in the blank | 12 |
DownLoad:
CSV
| Preparatory level | Entry level | Grade qualified | Mastery level | Proficiency level | |
| Llisten to | [0, 46] | [47,68] | [68,76] | [76,80] | [80,84] |
| Say | [0, 43] | [43,68] | [68,78] | [78,80] | [80,85] |
| Read | [0, 56] | [43,63] | [63,70] | [70,83] | [83,86] |
DownLoad:
CSV
| Field Name | Data type | Describe |
| ID | Automatic numbering | Content number |
| Type | Text | Types of exercises |
| Rank | Text | Grade of exercises |
| Scope | Text | Exercise module |
| Module | Exercise Type | Quantity |
| Aural comprehension | Teacher's explanation | 2 |
| Choice question | 11 | |
| Completion | 6 | |
| Spoken language | Teacher's explanation | 2 |
| Oral Practice | 11 | |
| Read | Teacher's explanation | 2 |
| Fill in the blank | 12 |
| Preparatory level | Entry level | Grade qualified | Mastery level | Proficiency level | |
| Llisten to | [0, 46] | [47,68] | [68,76] | [76,80] | [80,84] |
| Say | [0, 43] | [43,68] | [68,78] | [78,80] | [80,85] |
| Read | [0, 56] | [43,63] | [63,70] | [70,83] | [83,86] |
