Theoretical and numerical stability results for a viscoelastic swelling porous-elastic system with past history

1.   Introduction

Over the few last decades, a great number of scientists and mathematicians have got interested in the theory of elastic materials with voids (porous materials), see Figure 1. This theory is considered to be a simple extension of the classical theory of elasticity. See in this regard the concept of granular materials with voids introduced by Goodman and Cowin ^[1] and the idea of Nuziato and Cowin ^[2] related to the nonlinear theory of elastic materials with voids. The importance of materials with microstructure has been demonstrated by the very large number of published work and the various applications in the fields of petroleum industry, material science, biomedical, etc. Scientists investigated the coupling of macroscopic and microscopic structures in the porous material and its strength. Moreover, the stability has been studied by adding some dissipation mechanisms at the microscopic and/or macroscopic level. We start with the pioneer contribution of Quintanilla ^[3] in 2003, where he considered the following problem

where $u, \phi$, and $\rho_0, \kappa > 0$ are the longitudinal displacement, the volume fraction, the mass density, and the equilibrated inertia respectively; and $\mu, \alpha, \beta, \tau, \xi$ are positive constitutive constants which satisfy $\mu \xi > \beta^2$. He showed that the damping in the porous equation $(-\tau\phi_{t})$ is not strong enough to establish an exponential decay. However, a slow decay was obtained. Casas and Quintanilla ^[4] looked into a thermo-porous system of the form

where $\theta$ is the temperature difference and the other parameters are as defined above. Under the same conditions, the authors showed that the presence of the macro-temperature and the porous dissipations acting simultaneously are able to stabilize the system exponentially. In addition, the same authors, in ^[5], considered (1.2), with $\tau = 0$, and proved that heat effect alone is not strong enough to obtain an exponential decay. In this case, a slow decay was established. But, adding a micro-temperature to the system (1.1) with $\tau = 0$ leads to an exponential decay. Managa and Quintanilla ^[6] studied diverse one-dimensional porous systems and established many slow and exponential decay results. The summary of their result is that the exponential decay can only be obtained if two dissipations from the macroscopic and microscopic equations are combined. Otherwise, only the slow decay can be obtained. In ^[7], Pamplona et al. studied the problem

and showed that combining the strong damping in the elastic equation with the macro-temperature effect is not enough to obtain an exponential decay. However, for regular enough solutions, the decay is polynomial. Managa and Quintanilla ^[8] discussed several systems with quasi-static micro-voids and proved many slow and exponential decay theorems. Rivera and Quintanilla ^[9] improved the slow-decay results obtained for some systems in ^[8] and proved several polynomial results. They also showed that the rate of the polynomial decay can be improved for more regular solutions. Soufyane ^[10] used a viscoelastic damping in the porous equation together with a macro-temperature effect and established an exponential (respect. polynomial) decay for relaxation functions of exponential (respect. polynomial) decay. A similar result was also obtained by Soufyane et al. ^[11,12], for system (1.2) supplemented with the following boundary conditions:

where $g_{1}$ and $g_{2}$ are relaxation functions of general type. They obtained a general decay result, from which the usual exponential and polynomial decay rates are particular cases. Pamplona et al. ^[13] treated a one-dimensional porous elastic problem with history and proved, in the absence of the porous or the elastic dissipation, the lack of exponential decay. Messaoudi and Fareh ^[14,15] considered the following viscoelastic porous system

together with initial and boundary conditions. Here, $\rho_1, \rho_2, \rho_3, k, \kappa, \alpha$ are positive constants and $g : [0, +\infty) \rightarrow (0, +\infty)$ is a non-increasing differentiable function satisfying

where $\gamma(t)$ is a positive nonincreasing differentiable function. They established general decay results for the case of equal and non-equal speed of wave propagations. Also, Messaoudi and Fareh ^[16] considered the following

together with initial and boundary conditions. They used the multiplier method and established an expoential decay result. See also a similar result by Han and Xu ^[17], where they established the well posedness and proved an exponential decay result using a very lengthy and detailed spectral theory approach. For the Cauchy problems, Said-Houari and Messaoudi ^[18] looked into the following

together with initial data. Here $\rho, \mu, \alpha, J, a$ are strictly positive constants such that $\mu a > b^{2}$ and $b,$ $\beta$ and $m$ are different from zero. They showed that the decay rate of the system is very slow and of regularity-loss type. Apalara ^[19] considered

together with initial and boundary conditions where the relaxation function satisfied $g'(t) \leq -\eta (t)g(t)$ and established a general decay result under the assumption of equal-speed wave propagations. After that, Feng and Yin ^[20] extended the result of ^[19] to the case of non-equal wave speeds. Magana and Quintanilla ^[6] considered

together with initial and boundary conditions and proved that the viscoelasticity damping is not strong enough to bring about exponential stability. However, they showed that the presence of both porous-viscosity and viscoelasticity stabilizes the system exponentially. Some other forms of damping were also considered in the literature. See ^[21] for thermal damping, ^[5] for thermal damping with microtemperatures, ^[4] for porous-elasticity with thermal damping, ^[22] for porous dissipation with microtemperatures. For more recent stability results related to porous problems, we refer the reader to ^[23,24] and the references therein.

Swelling (also called expansive) soils have been characterized under porous media theory. They contain clay minerals that attract and absorb water, which may lead to increase pressure. In architectural and civil engineering, swelling soils are considered to be sources of problems and harms. If the pressure of the soil is higher compared to the main structure, it could result in heaving. The more the initial dry density of a soil, the more its potential to swell due to capillary action that accompanies absorption of underground water or shrinkage due to dryness as a result of changes in weather condition. Swelling soils causes serious engineering problems. Estimates indicate that about 20–25% of land area in the United State is covered with such problematic soils with the accompanied economic loss of 5.5 to 7 billions USD in 2003 ^[25]. Hence, it is crucial to study the ways to annihilate or at least minimize such damages. Reader is referred to ^{[26,27,28,29,30,31,32]} for other details concerning swelling soil. As established by Ieșan ^[33] and simplified by Quintanilla ^[34], the basic field equations for the linear theory of swelling porous elastic soils are mathematically given by

where the constituents $z$ and $u$ represent the displacement of the fluid and the elastic solid material, respectively. The positive constant coefficients $\rho_z$ and $\rho_u$ are the densities of each constituent. The functions $(P_1, G_1, F_1)$ represent the partial tension, internal body forces, and eternal forces acting on the displacement, respectively. Similar definition holds for $(P_2, G_2, F_2)$ but acting on the elastic solid. In addition, the constitutive equations of partial tensions are given by

where $a_1, a_3$ are positive constants and $a_2 \neq 0$ is a real number. The matrix $A$ is positive definite in the sense that $a_1 a_3 \geq a_2^2$.

Quintanilla ^[34] investigated (1.9) by taking

where $\xi$ is a positive coefficient, with initial and homogeneous Dirichlet boundary conditions and obtained an exponential stability result. Similarly, Wang and Guo ^[35] considered (1.9) with initial and some mixed boundary conditions, taking

where $\gamma(x)$ is an internal viscous damping function with a positive mean. They used the spectral method to establish an exponential stability result. Ramos et al. ^[36] looked into the following swelling porous elastic soils

and established an exponential decay rate provided that the wave speeds of the system are equal. Recently, Apalara ^[37] considered the following

where the relaxation function satisfies the condition

and established a general decay result. For more results in porous elasticity system, porous-thermo-elasticity systems, Timoshenko system and other systems, we refer the reader to see ^{[38,39,40,41,42]}.

Motivated by all the above works and the other works of some viscoelastic problems with infinite memory ^{[43,44,45,46,47,48,49,50,51,52]}, we consider the following problem

where the solution is $(z, u)$ such that $u$ and $u$ represent the displacement of the fluid and the elastic solid material. The positive constant coefficients $\rho_u$ and $\rho_z$ are the densities of each constituent. The coefficients $a_1, a_2$ and $a_3$ are positive constants satisfying specific conditions.

Our main objectives

We consider Problem (1.14) and intend to establish a four fold objective:

● extend many earlier works such as the ones in ^[37] from finite memory to infinite memory.

● prove a general decay estimate for the solution of Problem (1.14) with a wider class of relaxation functions; that is

where $\xi$ and $\Psi$ are two functions that satisfy some conditions to be specified later.

● get a better decay rate without imposing some assumptions on the boundedness of initial data considered in many papers in the literature such as the ones assumed in ^[48,53,54].

● perform some numerical tests to illustrate our theoretical results.

We establish our result by using the multiplier method and some convexity properties. In fact, the result in the present paper is essential to engineers and architects in order to plan and to ensure safe construction.

The rest of this paper is organized as follows. In section 2, we present some assumptions and material needed for our work. Some technical lemmas are presented and proved in section 3. We state and prove our main decay result and provide some conclusions in section 4. The numerical illustrations are presented in section 5.

2.   Assumptions

In this section, we state some assumptions needed in the proof of our results. Throughout this paper, $c$ is used to denote a generic positive constant. For the relaxation function $g$, we assume the following:

$(A)$ $g : [0, + \infty) \rightarrow (0, +\infty)$ is a $C^1$ nonincreasing function satisfying

In addition, there exists a $C^1$ function $\Psi:\mathbb{R}_+ \to \mathbb{R}_+$ which is linear or it is strictly increasing and strictly convex $C^2$ function on $(0, r]$ for some $r > 0$ with $\Psi(0) = \Psi^{\prime}(0) = 0$, $\lim_{s\rightarrow + \infty} \Psi^{\prime}(s) = +\infty$, $s\mapsto s \Psi^{\prime}(s)$ and $s \mapsto s \left(\Psi^{\prime}\right)^{-1}(s)$ are convex on $(0, r]$. Moreover, there exists a positive nonincreasing differentiable function $\xi$ such that

Remark 2.1. ^[55] If $\Psi$ is a strictly increasing, strictly convex $C^2$ function over $(0, r]$ and satisfying $\Psi(0) = \Psi'(0) = 0$, then it has an extension $\overline{\Psi}$, that is also strictly increasing and strictly convex $C^2$ over $(0, \infty)$. For example, if $\Psi(r) = a, \Psi'(r) = b, \Psi''(r) = c$, and for $t > r$, $\overline{\Psi}$ can be defined by

For simplicity, in the rest of this paper, we use $\Psi$ instead of $\overline{\Psi}$

Remark 2.2. Since $\Psi$ is strictly convex on $(0, r]$ and $\Psi(0) = 0,$ then

3.   Technical lemmas

In this section, we establish some essential lemmas needed for the proof of our stability result.

Lemma 3.1. The energy functional $E,$ defined by

where

and satisfies

Proof. By multiplying the first two equations of (1.14) by $z_t$ and $u_{t}$, respectively, and then, integrating by parts over $(0, 1),$ we end up with (3.3).

Lemma 3.2. (^[55]) For all $u \in H^1_0([0, 1])$,

for any $0 < \alpha < 1$,

Lemma 3.3. There exists a positive constant $M_1$ such that

where $h_0(t) = \int_{0}^{\infty}g(t+s)\left(1+\vert\vert u_{0x}(s)\vert\vert^{2} \right) ds$.

Proof.

where $M_1 = \max\bigg\{2, \bigg(\frac{8 a_2^2}{a_1} E(0) \bigg) \bigg\}.$

Lemma 3.4. The functional

satisfies, for any $\varepsilon_1 > 0$ and some constant $a_0$,

where $a_0 = a_3-\frac{a_2^2}{a_1}-\int_{0}^{\infty}g(s)ds.$

Proof. Direct computations using integration by parts gives

Applying Young's inequality, we get for $\varepsilon_1 > 0$,

and for $\varepsilon_2 > 0$,

Combining (3.9)–(3.11), we end up with

Using assumption ${\rm(A)}$, taking $\varepsilon_2 = a_0,$ where $a_0 = a_3-\frac{a_2^2}{a_1}-\int_{0}^{\infty}g(s)ds$, we obtain (3.8).

Lemma 3.5. Assume that ${\rm(A)}$ holds. Then, the functional

satisfies, for any $\delta_2, \delta_3 > 0,$

Proof. Differentiating $F_2$, taking into account (1.14), and using integrating by parts, we obtain

Using Young's inequality and Lemma 3.2, for any $\delta_1 > 0$, we obtain

Similarly, we can get for any $\delta_2 > 0$

Using Young's inequality and performing similar calculations as in (3.15), we obtain for any $\delta_3 > 0$

Combining all the above estimates, we get

By taking $\delta_2 = \frac{\rho_u c_0}{2}$, we end up with the desired inequality (3.13).

Lemma 3.6. Assume that ${\rm(A)}$ holds. Then the functional

satisfies

Proof. Direct computations give

Using Young's inequality,

Similarly, we can get for any $\eta_1 > 0$,

Exploiting Young's inequality again, we obtain

Recalling that $\int_0^\infty g(s) ds = \ell$ and exploiting Young's and Cauchy-Schwarz inequalities and using $(a+b)^2 \leq (1+ \eta) a^2 + (1+ \frac{1}{\eta})b^2, \ \forall \eta > 0$, we obtain

By taking $\eta_2 = \frac{1-\ell}{\ell}$, and aiming to lemma 3.2, we obtain

Estimate (3.18) follows by combining all the above estimates.

Lemma 3.7. The functional

satisfies, for any $\varepsilon_3 > 0$,

Proof. It is straightforward to see that

Using Young's inequality and the fact that $a_1 a_3 > a_2^2$, we end up with (3.22).

Lemma 3.8. (^[55]) Under the assumption $(A)$, the functional

satisfies the estimate

where $r(t) = \int_t^{+\infty} g(s) ds$.

Proof. The proof can be achieved by following the same calculations in ^[40].

Lemma 3.9. The functional $\mathcal{L}$ defined by

satisfies, for suitable choice of $\mu, \mu_1, \mu_2, \mu_3, \mu_4$ and for all $t\geq 0$,

and

Proof. By taking the derivative of the functional $\mathcal{L}$ and using the above estimates, we get

Firstly, we select $\mu_4$ large enough such that

Next, we take $\mu_3$ large enough such that

and then, we choose $\mu_1$ so large that

After fixing $\mu_1$, we select $\mu_2$ large enough such that

Now, for any fixed $\mu_1, \mu_2, \mu_3, \mu_4 > 0$, we pick $\delta_3 < \frac{\beta_2}{2 \mu_2 a_2^2}$, $\delta_1 < \frac{\beta_3}{4 \mu_2}$, $\epsilon_3 < \frac{\beta_3}{4 \mu_4}$, $\epsilon_1 < \frac{\beta_1}{2 \mu_1}$ and $\eta_1 < \frac{\beta_4}{4\mu_3}$, so that the following estimates are satisfied

Since $\frac{\alpha g^2(s)}{\alpha g(s)-g'(s)} < g(s)$, using the Lebesgue dominated convergence theorem, we can get

Hence, there exists some $0 < \alpha^* < 1$, such that if $\alpha < \alpha^*$, then

By putting $\alpha = \frac{1}{2 \mu}$ and choosing $\mu$ sufficiently large such that

which gives

Hence, we conclude that (3.26) holds. Moreover, we can choose $\mu$ even larger (if needed) so that (3.27) is satisfied, which means that, for some constants $\alpha_1, \alpha_2 > 0$,

Lemma 3.10. Assume that $(A)$ holds. Then, the energy functional satisfies, for all $t\in \mathbb{R}^+$, the following estimate

where $h_1(t) = \bigg(1+\int_{0}^{t} h_0(s)ds\bigg)$ and $h_0$ is defined in Lemma (3.3).

Proof. Let $\mathcal{F}(t) = \mathcal{L}(t)+F_{5}(t)$, then using (3.26) and (3.23), we obtain for all $t\in \mathbb{R}^+$,

where $\lambda$ is some positive constant. Therefore,

Therefore, (3.39) is established with $\tilde{m} = \max \bigg\{\frac{F(0)}{\lambda}, \frac{M_1}{2\lambda}\bigg\}$.

Corollary 3.11. There exists $0 < q_0 < 1$ such that, for all $t \ge 0$, we have the following estimate:

where $\Psi$ is defined in Remark (2.1),

and

Proof. Using (3.1) and (3.39), we have

Thanks to (3.39), then for all $t \ge 0$ and for $0 < q_0 < \min\bigg\{1, \big(\frac{a_1}{8 a_2^2\tilde{m}}\big)\bigg\}$, we have

Then, the rest of the proof of (3.42) is straightforward as the one in ^[56].

4.   A decay result

In this section, we state and prove a new general decay result for our problem (1.14). We introduce the following functions:

Further, we introduce the class $S$ of functions $\chi: \mathbb{R}_+ \rightarrow \mathbb{R}_+^{*}$ satisfying for fixed $c_1, c_2 > 0$ (should be selected carefully in (4.18)):

and

where $d > 0$, $c$ is a generic positive constant which may change from line to line, $h_0$ and $q$ are defined in (3.6) and (3.44) and

Remark 4.1. According to the properties of $\Psi$ introduced in $(A)$, $\Psi_2$ is convex increasing and defines a bijection from $\mathbb{R}_+$ to $\mathbb{R}_+$, $\Psi_1$ is decreasing defines a bijection from $(0, 1]$ to $\mathbb{R}_+$, and $\Psi_3$ and $\Psi_4$ are convex and increasing functions on $(0, r]$. Then the set $S$ is not empty because it contains $\chi(s) = \varepsilon \Psi_5(s)$ for any $0 < \varepsilon \leq 1$ small enough. Indeed, (4.3) is satisfied (since (1.15) and (4.5)). On the other hand, we have $q(t) h_0(t)$ is nonincreasing, $0 < \Psi_5 \leq 1$, and $\Psi'$ and $\Psi_4$ are increasing, then (4.4) is satisfied if

which holds, for $0 < \varepsilon \leq 1$ small enough, since $\lim_{t \rightarrow + \infty} \Psi'(t) = + \infty$. But with the choice $\chi = \varepsilon \Psi_5$, (4.6) (below) does not lead to any stability estimate. The idea is to choose $\chi$ satisfy (4.3) and (4.4) such that (4.6) gives the best possible decay rate for $E$.

Theorem 4.2. Assume that $(A)$ holds, then there exists a strictly positive constant $C$ such that, for any $\chi$ satisfying (4.3) and (4.4), the solution of (1.14) satisfies, for all $t \geq 0$,

Proof. We start by combining (3.3), (3.6), (3.26), and (3.42), then, for some $m > 0$ and for any $t\geq 0$, we have

Without loss of generality, one can assume that $E(0) > 0$. For $\varepsilon_{0} < r$, let the functional $\mathcal{F}$ defined by

which satisfies $\mathcal{F} \sim E$. By noting that $\Psi^{\prime\prime}\geq0,$ $q'\leq0$, $E'\leq0$ and using (4.7), we get

Let $\Psi^{*}$ be the convex conjugate of $\Psi$ in the sense of Young (see ^[57]), then

and $\Psi^{*}$ satisfies the following generalized Young inequality

So, with $A = \Psi^{\prime}\left(\varepsilon_{0}\frac{E(t)q(t)}{E(0)}\right)$ and $B = \Psi^{-1}\left(\frac{q(t) \mu(t)}{\xi(t)}\right)$ and using (3.3) and (4.8)–(4.10), we arrive at

So, multiplying (4.11) by $\xi(t)$ and using (3.43) and the fact that $\varepsilon_{0}\frac{E(t)q(t)}{E(0)} < r$ gives

Consequently, recalling the definition of $\Psi_2$ and choosing $\varepsilon_{0}$ so that $k = (\frac{m E(0)}{\varepsilon_{0}} -c) > 0$, we obtain, for all $t \in \mathbb{R}_+$,

where $\mathcal{F}_{1} = \xi \mathcal{F}+c E \sim E$ and satisfies for some $\alpha_{1}, \alpha_{2} > 0.$

Since $\Psi^{\prime}_{2}(t) = \Psi^{\prime}(t)+t \Psi^{\prime \prime}(t),$ then, using the strict convexity of $\Psi$ on $(0, r],$ we find that $\Psi_{2}^{\prime}(t), \Psi_{2}(t) > 0$ on $(0, r].$

Let $d > 0$. Using the general Young's inequality (4.10) on the last term in (4.13) with $A = \Psi^{\prime}\left(\varepsilon_{0}\frac{E(t)q(t)}{E(0)}\right)$ and $B = [\frac{c}{d}h_0 (t)]$, we have

Now, combining (4.13) and (4.15) and choosing $d$ small enough so that $k_1 = (k-d) > 0$, we arrive at

Using the equivalent property in (4.14) and the nonincreasing of $\Psi_2$, we have, for some $d_{0} = \frac{\alpha_1}{E(0)} > 0$,

Letting $\mathcal{F}_{2}(t): = d_{0} \mathcal{F}_{1}(t)q(t)$ and recalling $q'\leq0$, then we arrive at,

Then, (4.17) becomes for some constant $c_1 = d_0 k_1 > 0$ and $c_2 = d_0 d > 0,$

Since $d_{0}q(t)$ is is nonincreasing. Using the equivalent property $\mathcal{F}_{1}\sim E$ implies that there exists $b_0 > 0$ such that $\mathcal{F}_{2}(t)\geq b_{0} E(t)q(t)$. Let $t\in \mathbb{R}_+$ and $\chi(t)$ satisfying (4.3) and (4.4).

If

then, we have

If

then, for any $0\leq s \leq t$, we get

since, $q(t) E(t)$ is nonincreasing function. Therefore, for any $0\leq s \leq t$, we have

Using (2.4), $0 < \chi\leq 1$ and the fact that $\Psi_2$ is convex, we get, for any $0 < \epsilon_1 \leq 1$,

Recalling the definition of $\Psi_2$, that is $\Psi_2(t) = t\Psi'(t)$, we obtain

Now, using (4.23) and the fact that $\Psi^{\prime}$ is increasing, for any $0 \leq s \leq t$, we arrive at

Therefore, we have

Now, we let

where $\epsilon_1$ small enough so that $\mathcal{F}_{3}(0)\leq 1$. Then, (4.24) becomes, for any $0 \leq s \leq t$,

Further, we get

Since $\chi' \leq 0$ and using (4.18), then for any $0 \leq s \leq t$, $0 < \epsilon_1 \leq 1$, we obtain

Then, using (4.29), we get

From the definition of $\Psi_1$ and $\Psi_5$, we have

hence,

Now, we have

Then, according to (4.4), we get

Then (4.28) gives

Thus from (4.35) and the definition of $\Psi_1$ and $\Psi_2$ in (1.15) and (4.2), we obtain

Integrating (4.36) over $[0, t]$, we get

Since $\Psi_1$ is decreasing, $\mathcal{F}_{3}(0)\leq 1$ and $\Psi_1(1) = 0$, then

Recalling that $\mathcal{F}_{3}(t) = \epsilon_1 \chi(t)\mathcal{F}_{2}(t)-\epsilon_1 \Psi_5(t)$, we have

Similarly, recall that $\mathcal{F}_{2}(t): = d_{0} \mathcal{F}_{1}(t)q(t)$, then

Since $\mathcal{F}_{1}\sim E$, then for some $b > 0$, we have $E(t)\leq b \mathcal{F}_{1}$; which gives

From (4.20) and (4.41), we obtain the following estimate

where $c_3 = \max\{\frac{2}{b_0}, \frac{b(1+\epsilon_1)}{d_0\epsilon_1}\}.$

Example 1: Let $g(t) = \frac{a}{(1+t)^\nu}$, where $\nu > 1$ and $0 < a < \nu-1$ so that $(A)$ is satisfied. In this case $\xi(t) = \nu a^{\frac{-1}{\nu}}$ and $\Psi(t) = t^{\frac{\nu+1}{\nu}}$. Then, there exist positive constants $a_i (i = 0, ..., 3)$ depending only on $a, \nu$ such that

We will discuss two cases:

Case 1: if

where $0 < r < \nu-1$ and $m_0, m_1 > 0$, then we have, for some positive constants $a_i (i = 4, ..., 7)$ depending only on $a, \nu, m_0, m_1, r$, the following:

We notice that condition (4.4) is satisfied if

where $a_8 > 0$ depending on $a, \nu, c_1$ and $c_2$. Choosing $\chi(t)$ as the following

with $0 < \lambda \leq 1$, so that (4.3) is valid. Moreover, using (4.45) and (4.46), we see that (4.48) is satisfied if $0 < \lambda \leq 1$ is small enough, and then, (4.4) is satisfied. Hence (4.6) and (4.47) imply that, for any $t \in \mathbb{R}_+$

Thus, the estimate (4.50) gives $\lim_{t\rightarrow +\infty} E(t) = 0$.

Case 2: if $m_0 \leq 1+\vert \vert u_{0x}\vert \vert^2 \leq m_1$. That is $r = 0$ in (4.44) (as it was assumed in ^[48,53,54], then (4.50) holds with $r = 0$.

5.   Numerical results

In this section, some numerical experiments are performed to illustrate the energy decay results in Theorem 4.2. For this purpose, we use a finite element scheme in space, where for the time discretization, we use the Crank-Nicolson method, which is a second-order method in time that has the property to be unconditionally stable. The spatial interval $(0, L) = (0, 1)$ is subdivided into $100$ subintervals, where the temporal interval $(0, T)$ is subdivided into $N$ with a time step $\Delta t = T/N$. The solution at the time step $n+1$ is given by

where

and the matrices $\mathbb{M}$ and $\mathbb{R}$ are the mass matrix and the stiffness matrix respectively.

We run our code for $N$ time steps ($N = T/\Delta t$) using the following initial conditions:

For the numerical experiments, we choose the functional $g$ as in the Example 1 in the previous section

where $\nu > 1$ and $0 < a < \nu-1$.

Based on the Example 1, we perform three tests in order to the energy satisfies

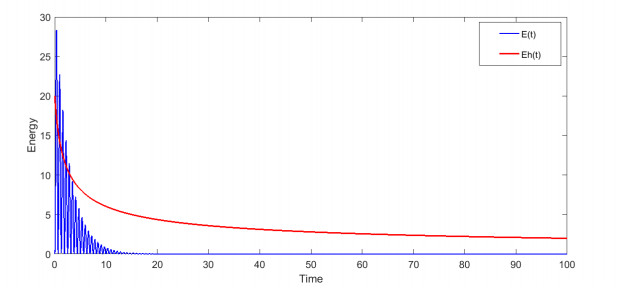
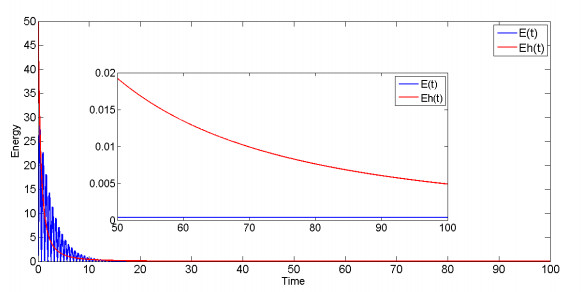
where $E_h$ given by (4.50) and the tests done as follows:

● Test 1: For the first numerical test, we choose the following entries:

and

where

● Test 2: For the second test, we choose the following entries:

and

where

● Test 3: Then, we consider the last case of (4.50) by choosing the following quantities:

and

where

● Test 4: Finally, we consider the case whene $r = 0$ and keeping the same quantities of Test 3:

and

where

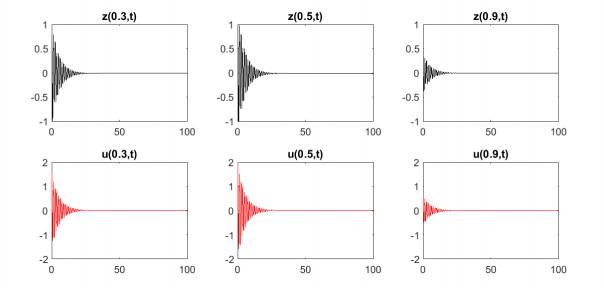
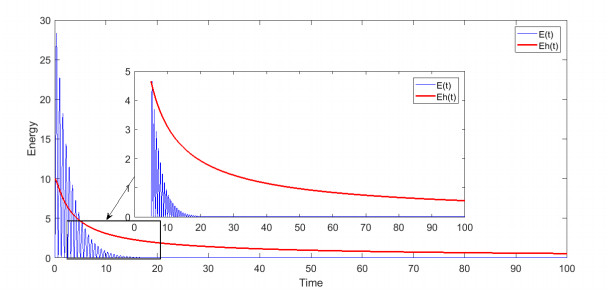
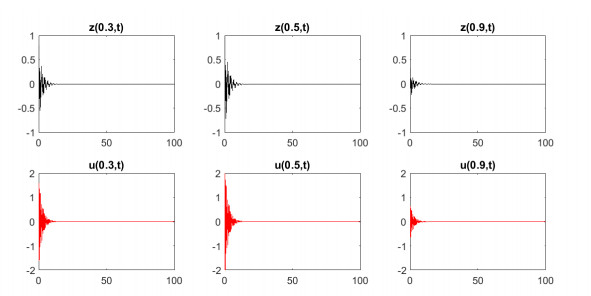
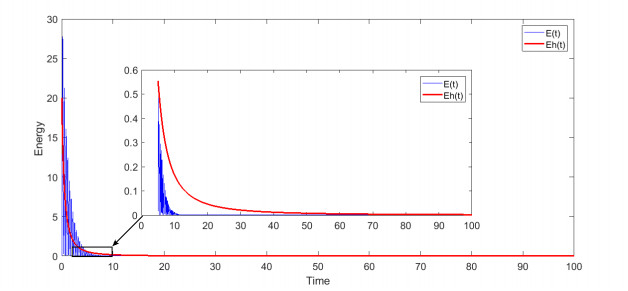
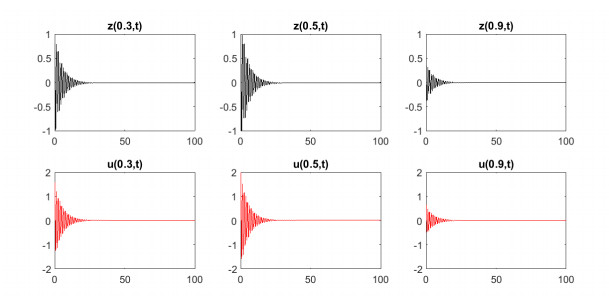
In Figure 2, we show the cross section cuts of the numerical solution $(z, u)$ at $x = 0.3$, $x = 0.5$ and at $x = 0.9$ for Test 1. Figure 3 presents the evolution in time of the system energy $E(t)$ compared with $E_h(t)$ for Test 1, we made a zoom on the first part to show the difference between the curves. Next, by following the same process, we present the rest of numerical results in Figures 4 and 5 for Test 2, in Figures 6 and 7 for Test 3 and in Figures 8 and 9 for Test 4.

The computational simulations show the decay behaviour for the solutions and the energy of the system (1.14), we examined four tests based on Example 1 (in both cases $r\neq 0$ and $r = 0$). As a conclusion, we observed that the energy decay uniformly for all tests and satisfies (4.50) in Example 1, and moreover independently of the waves speeds of the system.

6.   Conclusions

In this work, we considered a viscoelastic swelling porous elastic system with an infinite memory term. We proved a general decay result with a large class of the relaxation functions associated with the memory term. The proof is based on the multiplier method and some properties of convex functions and without imposing some assumptions on the boundedness of the history data considered in many earlier results in the literature. We tested our decay results numerically through several numerical tests and we found the energy decay uniformly for all tests and satisfies our decay theory. Our result generalizes some earlier works on swelling porous media in the literature and it is significant to engineers and architects as it might help to attenuate the harmful effects of swelling soils swiftly.

Acknowledgements

The authors thank KFUPM and University of Sharjah for their continuous support. The authors also thank the referee for her/his very careful reading and valuable comments. This work is funded by KFUPM, Grant No. SB201005.

Conflict of interest

The authors declare that there is no conflict of interest regarding the publication of this paper.