Stabilization of a viscoelastic wave equation with boundary damping and variable exponents: Theoretical and numerical study

1.   Introduction

The importance of partial differential equations in comprehending and explaining physical interpretation of problems that arise in numerous fields and engineering motivates many researchers to analyze and investigate the existence and stability of their solutions. Hyperbolic partial differential equations are the most interesting kind of partial differential equations, since they are utilized to simulate a wide and important collection of phenomena, such as aerodynamic flows, fluid and contaminant flows through porous media, atmospheric flows, and so on. Of the higher order hyperbolic equations, the wave equation is the most obvious. Klein-Gordon, Telegraph, sine-Gordon, Van der Pol, dissipative nonlinear wave and others are well-known hyperbolic equations that are important in the fields of wave propagation ^[1], random walk theory ^[2], signal analysis ^[3], relativistic quantum mechanics, dislocations in crystals and field theory ^[4], quantum field theory, solid-state physics, nonlinear optics ^[5], mathematical physics ^[6], solitons and condensed matter physics ^[7], interaction of solitons in collision-less plasma ^[8], fluxions propagation in Josephson junctions between two superconductors ^[9], motion of a rigid pendulum coupled to a stretched wire ^[10], material sciences ^[11] and non-uniform transmission lines ^[12] are some of the topics covered. For more related results, we refer to ^[13,14,15]. There is a vast range of publications for numerical solutions of hyperbolic partial differential equations, such as the one in ^{[16,17,18,19,20,21]}. In recent years, great efforts have been devoted to study problems with nonlinear dampings and source terms, and several existence, decay and blow up results have been established. Georgiev and Todorova ^[22] considered the following nonlinear problem

where the damping term $h(u_t) = \vert u_t \vert^{m-2} u_t$ and the source term $F(u) = \vert u \vert^{q-2} u$ are localized on the domain and established global existence when $q\le m$ and a blow up result when $q > m$. This work was improved by Levine and Serrin ^[23] to the case of negative energy and $m > 1$. For problems with boundary damping and source terms, we mention the work of Vitillaro ^[24] who considered the following problem

where the damping term $h(u_t) = \vert u_t \vert^{m-2} u_t$ and the source term $F(u) = \vert u \vert^{q-2} u$ are localized on a part of the boundary. The author established local existence and global existence of the solutions under some suitable conditions on the initial data and the exponents. In the presence of the viscoelastic term, Cavalcanti et al. ^[25] discussed the following problem

In this work, a global existence result for strong and weak solutions was established and some uniform decay rates were proved under some assumptions on $g$ and $h$. Al-Gharabli et al. ^[26] established general and optimal decay result for the same problem (1.3) considered in ^[25] where the relaxation $g$ satisfies more general conditions than the one in ^[25]. For more results in this direction, we refer to ^{[27,28,29,30,31]}. In particular, Liu and Yu ^[31] investigated the following problem

where the damping term $h(u_t) = \vert u_t \vert^{m-2} u_t$ and the source term $F(u) = \vert u \vert^{q-2} u$ are localized on a part of the boundary, and established several decay and blow up results under some suitable conditions on the initial data, the relaxation function and the exponents. Notice here that both the damping and source terms in ^[31] are localized on a part of the boundary, although, they are of constant nonlinearity. Moreover, the relaxation function $g$ satisfies the condition

where $\xi$ is a positive differentiable function. In fact, Liu and Yu ^[31] used the Multiplier method for stability and the potential well technique to prove the existence of the global solution. Moreover, the authors established a general decay when $m \geq 2$ and an exponential decay $m = 2$.

Many new real-world problems, such as electro-rheological fluid flows, fluids with temperature-dependent viscosity, filtration processes through porous media, image processing, hemorheological fluids, and others, came as a result of advances in science and technology, such as those problems which required modeling with non-standard mathematical functional spaces. The Lebesgue and Sobolev spaces with variable exponents ^{[32,33,34,35]} have shown to be very important and user-friendly tools to tackle such models. PDEs with variable exponents have recently attracted a lot of attention from researchers and academics. However, the majority of the findings for hyperbolic issues with variable exponents dealt with blow-up and non-global existence. On the stability of nonlinear damped wave equations with variable exponent nonlinearities, we only have a few results. It is worth mentioning the work of Messaoudi et al. ^[36], who explored the stability of the following equation

where $m(\cdot)\geq r(\cdot)\geq 2.$ The authors in their work showed that the solution energy decays exponentially if $m \equiv 2$ and when $m_{2} = {\rm{esssup}}_{x\in\Omega}m(x) > 2$, they obtained a polynomial decay at the rate of $t^{2/(m_{2}-2)}$. Also, Ghegal et al. ^[37] established a stability result similar to that of ^[36] for the equation

and proved under appropriate conditions on $m(\cdot), \; q(\cdot),$ and the initial data, a global existence result. Messaoudi et al. ^[38] recently looked at the following problem

and used the well-depth approach to verify global existence and provide explicit and general decay results under a very general relaxation function assumption.

In our present work, we are concerned with the following problem

on a bounded domain $\Omega \subseteq \mathbb{R}^n$ with a smooth boundary $\partial\Omega = \Gamma_0 \cup \Gamma_1$ where $\Gamma_{0}$ and $\Gamma_{1}$ are closed and disjoint and meas.($\Gamma_{0}) > 0$. The vector $n$ is the unit outer normal to $\partial \Omega$. The function $g$ is a relaxation function and $u_0$ and $u_1$ are given data. The functions $m(\cdot)$ and $q(\cdot)$ are the variable exponents. System 1.6 describes the spread of strain waves in a viscoelastic configuration. We first prove a global existence result for the solutions of problem (1.6) by using the potential well theory. Then we establish explicit and general decay results of problem (1.6) for a larger class of relaxation functions (see Assumption $A1$ below). To back up our theoretical decay results, we provide two numerical tests. Our decay results extend and improve some earlier results such as the one of Cavalcanti et al. ^[25], Al-Gharabli et al. ^[26], Liu and Yu ^[31] and the one of Messaoudi et al. ^[39]. In our work, we apply the energy approach (Multiplier Method), combined with various differential and integral inequalities equipped with the Lebesgue and Sobolev spaces with variable exponents. The multiplier method relies mostly on the construction of an appropriate Lyapunov functional $\mathcal {L}$ equivalent to the energy of the solution $E$. By equivalence $\mathcal {L}\sim E$, we mean

for two positive constants $\alpha_1\ {\rm{and}}\ \alpha_2$. To prove the exponential stability, we show that $\mathcal {L}$ satisfies

for some $c_{1} > 0.$ A simple integration of (1.8) over $(0, t)$ together with (1.7) gives the desired exponential stability result. In the case of a general decay result, we prove that $\mathcal {L}$ satisfies a differential inequality that combines the relaxation function and the other terms coming from the nonlinearites. Then we use some properties of the convex functions and other mathematical arguments to obtain general decay estimates depending on the relaxation function and the nature of the variable exponent nonlinearity. In fact, the Multiplier Method proved to be efficient in tackling such problems with dissipative terms either on the domain or in a part of the boundary. In the present paper, some properties of the convex functions are exploited. We also use the well-depth method to establish the global existence of the solutions. We show that the methods and tools used in this paper are sufficient to handle our problem and are less complicated than other methods which guide us to our target.

Related results to our problem

● Cavalcanti et al. ^[25] and Al-Gharabli et al. ^[26] investigated the same problem. However, in ^[25] and ^[26], the nonlinear damping term is $h(u_t)$ which satisfies some specific conditions. In our case $h(u_t) = \vert u_t \vert^{m(\cdot)-2} u_t$ where $m(\cdot)$ is a function of $x$ where $x$ is in a part of the boundary which makes our problem more complicated especially in the numerical computations. Additionally, in ^[25] the class of the relaxation function is a special case of the one in our paper. The decay results in both ^[25] and ^[26] were without numerical tests and without nonlinear source term.

● Liu and Yu ^[31] investigated the same problem where the exponents $m$ and $q$ are of constant nonlinearity and the relaxation function $g$ satisfies the condition $g'(t) \leq -\xi(t) g(t)$. In our paper, we extend the work of Liu and Yu ^[31] in which the exponents $m(\cdot)$ and $q(\cdot)$ are functions of $x$ where $x$ is in a part of the boundary. Moreover, we use a wider class of relaxation functions; that is $g'(t) \leq -\xi(t) H\left(g(t)\right)$ so that the class of the relaxation function in ^[31] is a special case. In addition, we provide some numerical experiments to illustrate our decay theories.

● Messaoudi et al. ^[38] investigated a similar problem. However, the nonlinear damping terms are in the domain. In our case the nonlinear damping and source terms are localized in the boundary, which makes the computations are more difficult. Also, ^[38] did not provide numerical computation.

The remainder of this work is arranged in the following manner: In Section 2, we write some of the assumptions and materials that are needed for our work. In Section 3, we establish and prove the global existence result. In Section 4, we present our main decay result as well as some examples. Section 5 presents and proves some technical lemmas. In Section 6, we prove the main decay results. Finally, in Section 7, we show numerical simulations to support our theoretical findings.

2.   Preliminaries

In this section, we present some background information on the Lebesgue and Sobolev spaces with variable exponents (see ^[40,41]) as well as some assumptions for the main result proofs. We will use the letter $c$ to denote a generic positive constant.

Definition 2.1.

1. The space $H^1_{\Gamma_0}(\Omega) = \{u\in H^1(\Omega):u|_{\Gamma_0} = 0\}$ is a Hilbert space endowed with the equivalent norm $\|\nabla u\|^2_2$.

2. Let $\beta:\Gamma_1\rightarrow [1, \infty]$ be a measurable function, where $\Omega$ is a domain of $\mathbb{R}^n$, then:

$a.$ the Lebesgue space with a variable exponent $\beta(\cdot)$ is defined by

where $\varrho_{\beta(\cdot)}(v) = \int_{\Omega}\frac{1}{\beta(x)}|v(x)|^{\beta(x)}dx$ is a modular.

$b.$ the variable-exponent Sobolev space $W^{1, \beta(\cdot)}(\Gamma_1)$ is:

3. $W_{0}^{1, \beta(\cdot)}(\Gamma_1)$ is the closure of $C_{0}^{\infty}(\Gamma_1)$ in $W^{1, \beta(\cdot)}(\Gamma_1)$.

Remark 2.2. ^[42]

1. $L^{\beta(\cdot)}(\Gamma_1)$ is a Banach space equipped with the following norm

2. $W^{1, \beta(\cdot)}(\Gamma_1)$ is a Banach space with respect to the norm

We define

Lemma 2.3. ^[42] If $\beta:\Gamma_1\rightarrow [1, \infty)$ is a measurable functionwith $\beta_2 < \infty,$ then $C_{0}^{\infty}(\Gamma_1)$ is dense in $L^{\beta(\cdot)}(\Gamma_1)$.

Lemma 2.4. ^[42] If $1 < \beta_1\leq \beta(x)\leq \beta_2 < \infty$ holds, then

for any $w\in L^{\beta(\cdot)}(\Gamma_1).$

Lemma 2.5 (Hölder's Inequality). ^[42] Let $\alpha, \beta, \gamma \geq 1$ be measurable functions defined on $\Omega$ such that

If $f\in L^{\alpha(\cdot)}(\Omega)$ and $g\in L^{\beta(\cdot)}(\Omega)$, then $fg\in L^{\gamma(\cdot)}(\Omega)$ and

Lemma 2.6. ^[42] [Poincaré's Inequality]Let $\Omega$ be a bounded domain of $\mathbb{R}^n$ and $p(\cdot)$ satisfies $(2.4),$ then, there exists $c_{\rho}$, such that

Lemma 2.7. ^[42] [Embedding Property] Let $\Omega$ be a bounded domain in $\mathbb{R}^n$ with a smoothboundary $\partial \Omega.$ Assume that $p, k\in C(\overline{\Omega})$ such that

and $k(x) < p^*(x)$ in $\overline{\Omega}$ with

then we have continuous and compact embedding $W^{1, p(.)}(\Omega)\hookrightarrow L^{k(.)}(\Omega).$ So, thereexists $c_e > 0$ such that

Assumptions

The following assumptions are essential in the proofs of the main results in this work.

$(A1)$ The relaxation function $g:\mathbb{R^+}\to \mathbb{R^+}$ is a $C^1$ nonincreasing function satisfying

and there exists a $C^1$ function $\Psi :(0, \infty)\to (0, \infty)$ which is linear or it is strictly increasing and strictly convex $C^2$ function on $(0, r]$ for some $0 < r \le g(0),$ with $\Psi(0) = \Psi^{\prime}(0) = 0$, $\lim_{s\rightarrow + \infty} \Psi^{\prime}(s) = +\infty$, $s\mapsto s \Psi^{\prime}(s)$ and $s \mapsto s \left(\Psi^{\prime}\right)^{-1}(s)$ are convex on $(0, r]$ and there exists a a $C^1$ nonincreasing function $\vartheta$ such that

$(A2)$ $m: \overline{\Gamma_1} \rightarrow [1, \infty)$ is a continuous function such that

and $1 < m_1 < m(x) \leq m_2,$ where

$(A3)$ $q: \overline{\Omega} \rightarrow [1, \infty)$ is a continuous function such that $2 < q_1 < q(x) < q_2,$ where

$(A4)$ The variable exponents $m$ and $q$ are given continuous functions on $\overline{\Gamma_1}$ satisfying the log-Hölder continuity condition:

where $c > 0$ and $0 < \delta < 1$.

Remark 2.8. ^[43] Using $(A1)$, one can prove that, for any $t \in[0, t_0]$,

and, hence,

Moreover, we can define $\bar \Psi$, for any $t > r$, by

where $\bar \Psi:[0, +\infty)\longrightarrow[0, +\infty)$, is a strictly convex and strictly increasing $\boldsymbol{C}^2$ function on $(0, \infty)$, is an extension of $\Psi$ and $\Psi$ is defined in $(A1)$.

We introduce the "modified energy" associated to our problem

where for $v\in L^{2}_{loc}(\mathbb{R}^+; L^2(\Omega)),$

A direct differentiation, using (2.6), leads to

Lemma 2.9. ^[43] Under the assumptions in $(A1)$, we have, for any $t \ge t_0,$

3.   Existence

The local existence theorem is stated in this section, and its proof can be demonstrated by combining the arguments of ^[44,45,46]. We also state and show a global existence result on the initial data under smallness conditions on $(u_0, u_1)$.

Theorem 3.1 (Local Existence). Given $(u_0, u_1)\in H^1_{\Gamma_0}(\Omega)\times L^2(\Omega)$ andassume that $(A1)- (A4)$ hold. Then, there exists $T > 0$, suchthat problem (1.6) has a weak solution

We will now go over the following functionals:

and

Clearly, we have

Lemma 3.2. Suppose that $(A1)-(A4)$ hold and $(u_0, u_1)\in H^1_{\Gamma_0}(\Omega)\times L^2(\Omega)$, such that

then

Proof. Sine $I$ is continuous and $I(u_0) > 0,$ then there exists $T_m < T$ such that

which gives

Now,

Using Youngs and Poincaré inequalities and the trace theorem, we get $\forall t\in [0, T_m],$

where

Therefore,

Notice that (3.7) shows that $u \in L^{q(\cdot)}(\Gamma_1 \times (0, T))$.

Proposition 3.3. Suppose that $(A1)-(A4)$ hold. Let $(u_0, u_1)\in H^1_{\Gamma_0}(\Omega)\times L^2(\Omega)$ be given, satisfying (3.4). Then the solution of (1.6) is global andbounded.

Proof. It suffices to show that $\|\nabla u\|_2^2+\|u_t\|_2^2$ is bounded independently of $t$. To achieve this, we use (2.7), (3.2) and (3.5) to get

Since $I(t)$ and $(g\circ \nabla u)(t)$ are positive, Therefore

where $C$ is a positive constant, which depends only on $q_1$ and $\ell$ and the proof is completed.

Remark 3.4. Using (3.6), we have

4.   Decay results

In this section, we state our decay result and provide some examples to illustrate our theorems.

Theorem 4.1 (The case: ${\bf{m_1\geq 2}}$). Assume that $(A1)-(A4)$ and (3.4) hold. Let$(u_0, u_1)\in H^1_{\Gamma_0}(\Omega)\times L^2(\Omega).$ Then, there exist positive constants ${\lambda}_1$, ${\lambda}_2$, ${\lambda}_3, \, {\lambda}_4$ such that

and

where $\Psi_0(s) = \int_{t}^{r}\frac{1}{s\Psi^{\prime}(s)}ds$ and $r = g(t_0)$.

Theorem 4.2 (The case: ${\bf{1 < m_1 < 2}}$). Assume that $(A1)-(A4)$ and (3.4) hold. Let $(u_0, u_1)\in H^1_{\Gamma_0}(\Omega)\times L^2(\Omega).$ Then, there exist positive constants $\beta_1$, $\beta_2, \, \beta_3$ and $t_1 > t_0$ such that

and, if $\Psi$ is nonlinear, we have

where $\Psi_1(\tau) = \tau^{\frac{1}{m_1-1}} \Psi'(\tau).$

Example 1 (The case: ${\bf{m_1\geq 2}}$). ● We consider $g(t) = ae^{-\sigma t}$, $t \geq 0$, where $a, \sigma > 0$ and $a$ is chosen in such a way that $(A1)$ is hold, then

So, (4.1) gives, for $d_1, d_2 > 0$

● Let $g(t) = ae^{-(1+t)^\nu}$, for $t\geq 0$, $0 < \nu < 1$ and $a$ is chosen so that condition $(A1)$ is satisfied. Then

Hence, (4.1) implies, for some $C > 0$,

● For $\nu > 1$, let

and $a$ is chosen so that hypothesis $(A1)$ remains valid. Then

where $\rho$ is a fixed constant, $p = \frac{1+\nu}{\nu}$ which satisfies $1 < p < 2$. Therefore, by estimate (4.2), we have

Example 2 (The case: ${\bf{1 < m_1 < 2 }}$). ● Consider $g(t) = \alpha e^{-\sigma (1+t)^{\nu}}, \quad t \geq 0, \quad 0 < \nu < 1, \quad \alpha, \sigma > 0,$ and $\alpha$ is chosen so that $(A1)$ holds, then $g'(t) = -\sigma\Psi(g(t))$ with $\vartheta(t) = \nu (1+t)^{\nu-1}$ and $\Psi(s) = s.$ We next infer that the solution of (1.6) satisfies the following energy estimate under the conditions of Theorem 4.2

● Let

and $\alpha$ is chosen such that hypothesis $(A1)$ remains valid. Then

where $\sigma$ is a fixed constant. Then, we conclude for $t$ large enough and some constant $C > 0$ that the solution of (1.6) satisfies the following energy estimate under the conditions of Theorem 4.2

where $\lambda = \frac{(m_1-1)(m_1+\nu-2)}{m_1+\nu-1} > 0$.

The proofs of Theorem 4.1 and Theorem 4.2 will be done through several Lemmas.

5.   Technical lemmas

We establish various lemmas for our proofs in this section.

Lemma 5.1 (^[47]). Assume that $(A1)$ holds. Then for any $0 < {\varepsilon} < 1$, we have

where

Lemma 5.2. Assume that $(A1)-(A4)$ and (3.4) hold, the functional

satisfies the estimates:

Proof. By differentiating $F_1$ and using (1.6), we get

(5.1) and Young's inequality, give, for any $\delta_0 > 0$,

The use of Young's inequality with $\lambda(x) = \frac{m(x)}{m(x)-1}$ and $\lambda^{\prime}(x) = m(x)$, leads to

where

Combining (2.1), (2.6), (2.7) and (3.9), we get

where

From (5.6) and (5.7), we have

Combining all the above results, choosing $\delta_0 = \frac{\ell}{2}$ and $\delta = \frac{\ell}{4 c_{0}}$ and using Poincaré's inequality and the trace theorem completes the proof of (5.2).

To prove (5.3), we apply Young's and Poincaré's inequalities and the trace theorem to obtain

where

By selecting $\eta = \frac{\ell}{8 c^2_\rho}$, (5.9) becomes

Next, for any $\delta$ we have, by the case $m(x) \ge 2,$

As a result of combining the estimates above, we arrive at

By choosing $\delta = \frac{\ell}{8c_0}$, then $c_{\delta}(x)$ is bounded and hence (5.3) is obtained.

Lemma 5.3. Assume that $(A1)-(A4)$ and (3.4) hold. Then for any $\delta > 0$, the functional

satisfies the estimates:

and for $1 < m_1 < 2$, we have

where the constant $c_m > 0$ depends on $m_1, m_2$ and $\ell$, and$h_{\varepsilon}$ is defined earlier in Lemma (5.1).

Proof. Direct differentiation of $F_2$ and using (1.6) leads to

Using Young's inequality and Lemma 5.1, we get

From Lemma (5.1) and Young's inequality, we get

Now, for almost every $x\in \Omega$, we get

Next, for almost every $x\in \Omega,$ we obtain

Using Young's, Hölder's, Poincaré's inequalities and Lemma 5.1, we have

where

Further, we have

Therefore,

where $c_1 = \left[c_e^{m_2}\left(\frac{2q_1}{\ell (q_1-2)}E(0)\right)^{\frac{m_2-2}{2}}+c_e^{m_1}\left(\frac{2q_1}{\ell (q_1-2)}E(0)\right)^{\frac{m_1-2}{2}}\right].$

To estimate the last term in (5.15), we use Young's inequality and Lemma 5.1, to obtain

The first term in (5.23) can be estimated as follows:

where

Collecting all the above estimates with (5.15), we see that (5.13) is archived.

To prove (5.14), we start by re-estimating the fifth term in (5.15) as follows:

Hence, (5.14) is established.

Lemma 5.4. ^[43] Assume that $(A1)$ and $(A3)$ hold, then the functional

satisfies the estimate:

where $f(t) = \int_{t}^{\infty}g(s)ds$.

Lemma 5.5. Given $t_0 > 0.$ Assume that $(A1)-(A4)$ and (3.4) holdand $m_1 \geq 2$. Then, the functional $\mathcal{L}$ defined by

satisfies, for fixed $N, \, {\varepsilon}_1, \, {\varepsilon}_2 > 0$,

and for any $\, t \geq t_0$,

Proof. The equivalence $\mathcal{L} \sim E$ can be proved straightforward. For the proof of (5.28), we start combining (2.6), (2.7), (5.2) and (5.13) and recalling $g'(t): = {\varepsilon} g(t)-h_{\varepsilon}(t)$, to get:

Now, set $g_0 = \int_{0}^{t_0}g(s)ds$ and select $\delta$ small enough so that

Once $\delta$ is fixed, then ${c_\delta}(x)$ is bounded and the choice of ${\varepsilon}_1 = \frac{3}{8}g_0 {\varepsilon}_2$ yields

By taking ${\varepsilon}_2 = \frac{1}{8 c_1 \delta (1-\ell)^{m_1-1}},$ we get

Then (5.29) becomes

From $\frac{{\varepsilon} g^2(s)}{{\varepsilon} g(s)-g'(s)} < g(s)$ and using the Lebesgue Dominated Convergence Theorem, we conclude that

So, there exists $0 < {\varepsilon}_0 < 1$ such that if ${\varepsilon} < {\varepsilon}_0$, then

Now, choosing $N$ large enough so that $\mathcal{L} \sim E$ and

For ${\varepsilon} = \frac{1}{4N}$, we have

This gives

A Combination of (5.31)-(5.32), leads to (5.28).

Lemma 5.6. Given $t_0 > 0$. Assume that $(A1)-(A4)$ and (3.4) holdand $1 < m_1 < 2$. Then, the functional $\mathcal{L}$ defined by

satisfies, for fixed $N, \, {\varepsilon}_1, \, {\varepsilon}_2 > 0$,

and for any $\, t \geq t_0$,

Proof. Estimate (5.34) can be established by using the same above arguments with some changes only on

Lemma 5.7. Assume that $(A1)-(A4)$ and (3.4) hold, then for $m_1\geq 2$, then

Proof. Combining Lemmas 5.4 and 5.5 and choosing $\varepsilon_1$ small enough, we see that the functional $L_1$ defined by

is nonnegative and satisfies, for some $c_0 > 0$ and for any $t \geq t_0$,

An integration over $(t_0, t)$, leads

Using the continuity of $E$, we obtain

Lemma 5.8. Assume that $(A1)-(A4)$ and (3.4) hold, then for $1 < m_1 < 2$, we have

Furthermore,

Proof. Combing Lemmas 5.4 and 5.6 and selecting $\varepsilon_1$ small enough, we conclude that the functional $L_2$ defined by

satisfies, for some $c_0, \; c > 0$ and for any $t \geq t_0$,

Now, multiplying (5.38) by $E^\alpha(t), \; \alpha = \frac{2-m_1}{m_1-1}$, and using Young's inequality, we arrive at

Choosing ${\varepsilon}$ small enough and using the fact $E'\leq 0$, then (5.39) becomes:

where $L_3(t) = E^\alpha(t)L_2(t)+cE(t)$.

Integrating over $(t_0, t)$, we get

Therefore, we get

Using Hölder's inequality, we get

This completes the proof.

6.   Proofs of the decay theorems

In this section, we prove Theorem 4.1 and Theorem 4.2.

6.1.   Proof of Theorem 4.1

Case 1: $\Psi$ is linear. Using (2.3), (2.6), and (5.28), then for any $t\geq t_0$, we have

Letting $\vartheta \mathcal{L}+cE\sim E$ and integrating over $(t_0, t)$, we get for some $C, \lambda > 0,$

Case 2: $\Psi$ is nonlinear. We start defining the following functional

where $\gamma > 0$ should be carefully selected. Using (3.9) and (5.35), we get

Therefore, we can select $\gamma$ small enough so that

We also define the following

Since $\bar \Psi$ is strictly convex and $\bar \Psi(0) = 0$, we have

Combining the above with (2.3), Jensen's inequality and (6.2), we obtain, for any $t > t_0$,

Then, for any $t \geq t_0$, we have

Combining (2.6), (5.28), (6.4) and using Lemma 2.9, we get, for any $t\geq t_0$,

where $\mathcal{F}: = \mathcal{L}+cE$. For ${\varepsilon}_0 < r$, we define

Then, using the facts that $E'\leq0$, $\Psi' > 0$ and $\Psi'' > 0$, estimate (6.6) becomes

Recall that $\bar \Psi$ is convex on $(0, \infty)$ and let $\bar \Psi^*$ be the convex conjugate of $\bar \Psi$ in the sense of Young ^[48] such that

and satisfies the following generalized Young inequality

Then a combination of (2.7), (6.7)) and (6.9) with applying the generalized Young inequality over $(0, \infty)$ with $A = \bar \Psi'\left(\frac{{\varepsilon}_0 E(t)}{E(0)}\right)$ and $B = \bar \Psi^{-1}\left(\frac{\gamma\theta(t)}{\vartheta(t)}\right),$

Take ${\varepsilon}_0$ small enough, if needed, to obtain, for some positive constant $\beta_1$,

Multiplying both sides of the last inequality by $\vartheta(t)$ and using ${\varepsilon}_0\frac{E(t)}{E(0)} < r$ and inequality 6.3, we get

Hence by setting $\mathcal{F}_2 = \vartheta \mathcal{F}_1+cE$, we obtain, for two constants $\alpha_1, \alpha_2 > 0,$

and

Now, let

then we deduce from $(A2)$ that $\Psi_2, \Psi_2^{\prime} > 0$ on $(0, 1]$, and from (6.10) and (6.11) that $\Lambda\sim E$ and

Integration over $(t_0, t)$, we get

Hence,

where $\Psi_0 = \int_{t}^{r}\frac{1}{s\Psi'(s)}ds$ and $\lambda_2 > 0.$

6.2.   Proof of Theorem 4.2

Case 1: $\Psi$ is linear. Combining (2.3), (2.6) and (5.34), then for some $\gamma_1 > 0$, we have

Letting $\mathcal{L}_1: = \vartheta \mathcal{L}+cE\sim E$, multiplying both sides of the above estimate by $E^k$, with $k = \frac{2-m_1}{m_1-1}$ and applying Young's inequality, we obtain

Set $\mathcal{L}_2: = E^k \mathcal{L}_1+cE \sim E$, take $\epsilon$ small enough and use the fact $E'\leq 0$ we get, for some $\gamma_2, \gamma_3 > 0,$

Now, we integrate over $(t_0, t)$ and use $\mathcal{L} \sim E,$ to get,

Case 2: $\Psi$ is nonlinear. We define the following functional

Thanks to (5.37), we can pick $\gamma_0$ small enough so that $\eta_1(t) < 1$. Then, for any $t\ge t_0,$ we have

which gives

Using (2.6), (5.34), (6.14) and Lemma 2.9, then for any $t\geq t_0$, we get

Thus, (6.15) becomes

where $\mathcal{F}: = \mathcal{L}+cE\sim E$.

For $0 < {\varepsilon}_1 < r$, we shall define

Using (2.7), (6.16), the assumption $(A1)$, and the generalized Young inequality, then for any $t > t_0,$ we have

where $\gamma_5 > 0.$ Multiplying the last inequality by $\vartheta(t)$ and using (6.3), then for any $t > t_0,$ we obtain

By setting $\mathcal{F}_2: = \vartheta \mathcal{F}_1+cE$, we get, for any $t > t_0,$

Multiplying the above inequality by $E^n$, $\left(n = \frac{2-m_1}{m_1-1}\right),$ and using Young's inequality, then for some $\gamma_6$, we have

Let $\mathcal{F}_3 = E^n \mathcal{F}_2+cE$ and choose ${\varepsilon}$ small enough, then for a constant $\gamma_6 > 0$, we get

We deduce from $\lim_{t\to \infty}{\frac{1}{(t-t_0)^{2-m_1}}} = 0$, that, there exists $t_1 > t_0$ such that $\frac{1}{(t-t_0)^{2-m_1}} < 1$ for any $t\ge t_1$, which implies

Integrating (6.19) over $(t_1, t)$ yields

Since $\Psi'' > 0$ and $E' \leq 0$, it follows that the map

is non-increasing. Therefore, we get

Multiplying (6.21) by $\bigg(\frac{{\varepsilon}_1}{(t-t_0)^{2-m_1}}\bigg)^{n+1}$ and setting $\Psi_1(\tau): = \tau^{n+1}\Psi^{\prime}(\tau)$, which is strictly increasing, we obtain, for $\lambda_1, \lambda_2 > 0,$

This completes the proof.

7.   Numerical tests

We give numerical simulations in this section to support our theoretical results in Theorems 4.1 and 4.2. We use the conservative Lax-Wendroff strategy presented in ^[49] to demonstrate the decay of two tests. To discretize the system $(1.1)$, we use a second-order finite difference method (FDM) in time and space for the space-time domain $\Omega\times (0, T) = [0, 1]\times (0, 25)$. The mixed boundary conditions in the system $(1.1)$ could be viewed as a Dirichlet boundary condition on one hand and a Neuman boundary condition on the other. Let for instance, $u_0(x) = x$ and $u_1(x) = 1-x.$ Then, the condition

will apply to the following two tests:

● TEST 1: In the first test, we set $m(x) = q(x) = 2$. We use the boundary condition at $x = 1$ (the term $u_1$ will be vanish at $x = 1$, while the right-hand side condition will have a nonzero starting value).

● TEST 2: In the second numerical test, we examine the case $m(x) \neq 2$ and $q(x) \neq 2$ for all $x\in[0, 1]$. We use the boundary at $x = 0$ (the term $u_1$ term will not vanish at $x = 0$ and the right hand side condition will be canceled). For this, we use the functions $m(x) = q(x) = 2+\dfrac{1}{1+x}$ for all $x\in [0, 1]$.

To check that the implemented method and the run code are numerically stable, we use $\Delta{t} < 0.5\Delta x$, satisfying the stability condition according to the Courant-Friedrichs-Lewy (CFL) inequality, where $\Delta t = 0.0025$ represents the time step and $\Delta x = 0.01$ the spatial step. The spatial interval $[0, 1]$ is subdivided into $100$ subintervals, whereas the temporal interval $[0, T] = [0, 25]$ is deduced from the stability condition above. We run our code for $10,000$ time steps using the following initial conditions:

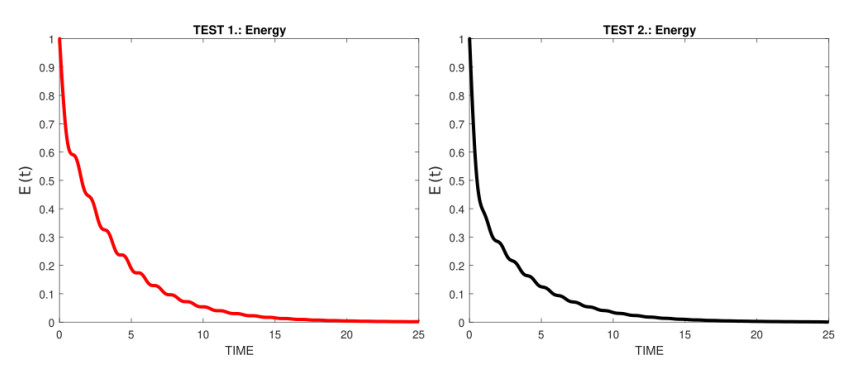
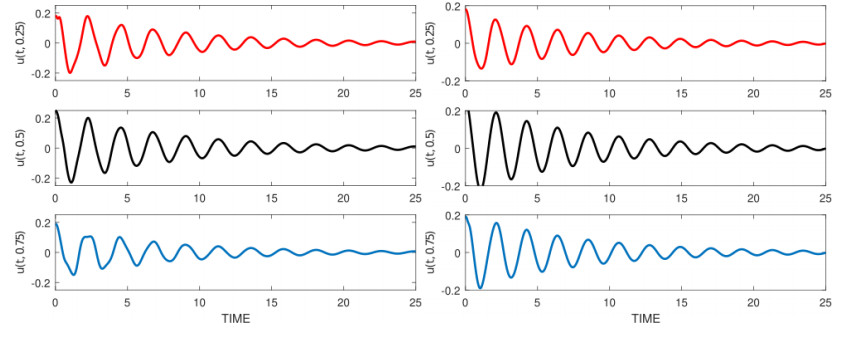
In Tests 1 and 2, we demonstrated the decay under the initial and boundary conditions. The plots in Figure 1 show the temporal wave evolution in cross sections. The three cross sections are taken at $x = 0.75, 1.5, 2.25$ (see Figure 1. The corresponding energies given by the "modified" equation $(3.1)$ are presented in Figure 2. The damping behavior is well seen in both tests. The result shown in Figure 2 is equally important. As a result, the similarity decrease for the energy decay rates obtained in Test 1 and Test 2 can be clearly observed. We normalized the output by dividing the maximums value in order to compare the asymptotic convergence of the energy.

Finally, it should be stressed that our intention focuses is to show the energy decay represented in Figure 2. However, we remarked that there are some similarities in the energy decay behavior. Both functions have at least a polynomial decay. This is due to the initial conditions used for the problem. We believe that, for other choices of the initial solution, we could obtain a clear difference between the outputs of the energy function.

8.   Conclusions

In this work, we considered a viscoelastic wave equation with boundary damping and variable exponents. We first proved the existence of global solutions and then we established optimal and general decay estimates depending on the behavior of the relaxation function and the nature of the variable exponent nonlinearity. We finally end our paper with some numerical illustrations. Working with variable exponents in the boundary is totally different from the earlier results and of much challenging. We compared our results with other related results and showed that our results improved and extended some earlier results in the literature.

Acknowledgment

The authors appreciate the continued assistance of King Fahd University of Petroleum and Minerals (KFUPM) and the University of Sharjah. The authors also thank the referees for their very careful reading and valuable comments. This work is funded by KFUPM, Grant No. #SB201012.

Conflict of interest

The authors declare that there is no conflict of interest regarding the publication of this paper.