1.
Introduction
Throughout this paper, we denote the set of m×n complex matrices by Cm×n. And denote the identity matrix of order n by In, the range space, the null space, the conjugate transpose and the rank of the matrix A∈Cm×n by R(A), N(A), A∗ and r(A), respectively. The index of A∈Cn×n, denoted by Ind(A), is the smallest nonnegative integer k such that r(Ak)=r(Ak+1). The subset of Cn×n with index k will be denoted by Cn×nk. PL,M stands for the projector (idempotent) on the subspace L along the subspace M. For A∈Cn×n, PA and PA∗ represent the orthogonal projections onto R(A) and R(A∗) respectively, i.e. PA=AA† and PA∗=A†A.
For the readers' convenience, we will first recall the definitions of some generalized inverses. For A∈Cm×n, the Moore-Penrose inverse A† of A is the unique matrix X∈Cn×m satisfying the following four Penrose equations [4,18]:
A matrix X∈Cn×m satisfying (2) is called an outer inverse of A and is denoted by A(2). For A∈Cm×n, a matrix X∈Cn×m satisfying XAX=X, R(X)=T and N(X)=S, is denoted by A(2)T,S [6], where T is a subspace of Cn and S is a subspace of Cm. If A(2)T,S exists then it is unique.
The Drazin inverse of A∈Cn×nk, denoted by AD, is the unique matrix X∈Cn×n satisfying the following three equations [4,9]:
In particular, if Ind(A)=1, then the Drazin inverse of A is called the group inverse of A and is denoted by A#. The core-inverse of A∈Cn×n, denoted by A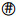 is defined in [2] as the unique matrix X∈Cn×n satisfying AX=PA and R(X)⊆R(A). A
is defined in [2] as the unique matrix X∈Cn×n satisfying AX=PA and R(X)⊆R(A). A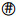 exists if and only if Ind(A)=1 [2,20]. The core-EP inverse of A∈Cn×nk, denoted by A
exists if and only if Ind(A)=1 [2,20]. The core-EP inverse of A∈Cn×nk, denoted by A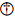 is defined in [19] as the unique matrix X∈Cn×n satisfying XAX=X and R(X)=R(X∗)=R(Ak) [11,19,21,30]. The BT-inverse of A∈Cn×n, denoted by A⋄, which is defined in [1] written by (APA)† [1,13]. The DMP-inverse of A∈Cn×nk, written by AD,† is defined in [14] as the unique matrix X∈Cn×n satisfying XAX=X,XA=ADA and AkX=AkA†. Moreover, it was proved that AD,†=ADAA†. Also, the dual DMP-inverse of A was introduced in [14], namely A†,D=A†AAD [14,31]. The CMP-inverse of A∈Cn×nk, written by AC,† is defined in [15] as the unique matrix X∈Cn×n satisfying XAX=X,AX=AADAA† and XA=A†AADA. Moreover, it was proved that AC,†=A†AADAA† [15,24]. The (B,C)-inverse of A∈Cm×n, denoted by A(B,C) [8], is the unique matrix X∈Cn×m satisfying XAB=B,CAX=C, N(X)=N(C) and R(X)=R(B), where B, C∈Cn×m [3,8].
is defined in [19] as the unique matrix X∈Cn×n satisfying XAX=X and R(X)=R(X∗)=R(Ak) [11,19,21,30]. The BT-inverse of A∈Cn×n, denoted by A⋄, which is defined in [1] written by (APA)† [1,13]. The DMP-inverse of A∈Cn×nk, written by AD,† is defined in [14] as the unique matrix X∈Cn×n satisfying XAX=X,XA=ADA and AkX=AkA†. Moreover, it was proved that AD,†=ADAA†. Also, the dual DMP-inverse of A was introduced in [14], namely A†,D=A†AAD [14,31]. The CMP-inverse of A∈Cn×nk, written by AC,† is defined in [15] as the unique matrix X∈Cn×n satisfying XAX=X,AX=AADAA† and XA=A†AADA. Moreover, it was proved that AC,†=A†AADAA† [15,24]. The (B,C)-inverse of A∈Cm×n, denoted by A(B,C) [8], is the unique matrix X∈Cn×m satisfying XAB=B,CAX=C, N(X)=N(C) and R(X)=R(B), where B, C∈Cn×m [3,8].
The weak group inverse of A∈Cn×nk was defined for the first time by Wang and Chen [22]:
Definition 1.1 [22] Let A∈Cn×nk. The weak group inverse AⓌ of A is the unique matrix X∈Cn×n satisfying
The weak group matrix was defined for the first time by Wang and Liu [23]:
Definition 1.2 [23] Let A∈Cn×nk. We say a weak group matrix if A∈CWGn, in which
Recently, the study of the weak group inverse and the generalized weak group inverse has received a lot of attention (see e.g. [16,17,26,27,28]).
In this paper, we discuss some new characterizations and properties of the weak group inverse and the weak group matrix. First, we characterize the weak group inverse of a square matrix based on its range space and null space. Several different characterizations of the weak group inverse are presented by projection and the Bott-Duffin inverse. We also give the limit representations for the weak group inverse. Then we study the relationships between AⓌ and other generalized inverses such as A#, 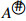 , A†,
, A†,  , AD,AD,†. And some characterizations of the weak group matrix are obtained.
, AD,AD,†. And some characterizations of the weak group matrix are obtained.
The research is arranged as follows. In Section 2, some indispensable matrix classes and lemmas are given. In Section 3, some characterizations of AⓌ are presented. In Section 4, we discuss the relationships between AⓌ and other generalized inverses by core-EP decomposition. Finally, we study the characterizations of the weak group matrix.
2.
Preliminaries
For convenience, some matrix classes will be given as follows.
These symbols CCMn,CPn,COPn,CEPn,CUn and CHn will stand for the subsets of Cn×n consisting of core matrices, projectors (idempotent matrices), orthogonal projectors (Hermitian idempotent matrices), EP-matrices (Range-Hermitian matrices), unitary matrices and Hermitian matrices, respectively, i.e.,
In order to study the characterizations and properties of the weak group inverse and the weak group matrix, we need to recall the core-EP decomposition of A, which plays an important role in this paper.
According to Theorem 2.2 in [21], every matrix A∈Cn×nk can be represented in the form:
where T∈Cp×p is nonsingular with p:=r(T)=r(Ak), N is nilpotent of index k, and U∈Cn×n is unitary. The representation of A given in (2.1) satisfies Ind(A1)≤1, Ak2=0, and A∗1A2=A2A1=0 [21, Theorem 2.1]. Moreover, it is unique [21, Theorem 2.4] and is called the core-EP decomposition of A.
Lemma 2.1 Let A∈Cn×nk be as in (2.1). Then
In which case, we have
Proof. The proof is easy.
In [7,10,13,21,29], some generalized inverses such as A†,AD,  , A⋄,AD,†,A†,D,AC,† can be represented by the core-EP decomposition of A. We list these results in the lemmas below.
, A⋄,AD,†,A†,D,AC,† can be represented by the core-EP decomposition of A. We list these results in the lemmas below.
Lemma 2.2 [7,10,13,21,29] Let A∈Cn×nk be as in (2.1). Then
The notation ˜T and Δ are often used as follows.
Lemma 2.3 [10,21,22] Let A∈Cn×nk be as in (2.1). Then
Lemma 2.4 [25] Let A∈Cm×n, X∈Cn×l and Y∈Cl×m. Then the following conditions are equivalent:
(a) limλ→0X(λIl+YAX)−1Y exists;
(b) r(XYAXY)=r(XY);
(c) A(2)R(XY),N(XY) exists,
in which case,
Lemma 2.5 [16] Let A∈Cn×nk. Then
Lemma 2.6 [17] Let A∈Cn×nk. Then the following statements hold:
(a) AAⓌ=PR(Ak),N((Ak)∗A);
(b) AⓌA=PR(Ak),N((Ak)∗A2).
3.
Some characterizations of the weak group inverse
Using the results of Lemma 2.5, we have R(AⓌ)=R(Ak) and N(AⓌ)=N((Ak)∗A). Now, we will give several different characterizations of the weak group inverse for a matrix A.
Theorem 3.1 Let A∈Cn×nk and X∈Cn×n. Then the following conditions are equivalent:
(a) X=AⓌ;
(b) R(X)=R(Ak),N(X)=N((Ak)∗A) and AX2=X;
(c) R(X)=R(Ak),N(X)=N((Ak)∗A) and XAk+1=Ak;
(d) R(X)=R(Ak) and AX=  A;
A;
(e) R(X)=R(Ak) and A2X=PAkA;
(f) R(X)=R(Ak) and (Ak)∗A2X=(Ak)∗A.
Proof. (a)⇒(b). The assertion follows directly from Lemma 2.5 and the definition of AⓌ.
(b)⇒(c). From (2.1), we can calculate that
where ˜T=k−1∑j=0TjSNk−1−j.
Let X=U(X1X2X3X4)U∗, from R(X)=R(Ak) and AX2=X, we have
where X2∈Cp×(n−p),p=r(Ak).
Hence, XAk+1=U(T−1X200)U∗⋅U(Tk+1T˜T00)U∗=U(Tk˜T00)U∗=Ak.
(c)⇒(a). By R(X)=R(Ak), we have X=AkL for some L∈Cn×n. Then we get that XAX=X since XAk+1=Ak. Hence, X=A(2)R(Ak),N((Ak)∗A)=AⓌ by Lemma 2.5.
(a)⇒(d). It can be obtained directly from Lemma 2.5 and the definition of AⓌ.
(d)⇒(e). Notice that A =PAk, which is obvious.
=PAk, which is obvious.
(e)⇒(f). From the condition, it follows that (Ak)∗A2X=(Ak)∗PAkA=(Ak)∗A.
(f)⇒(a). From (2.1) and R(X)=R(Ak), we can let X=U(X1X200)U∗, where X1∈Cp×p and X2∈Cp×(n−p),p=r(Ak). And by (Ak)∗A2X=(Ak)∗A, we have X1=T−1,X2=T−2S.
Hence, X=U(T−1T−2S00)U∗=AⓌ.
In the following theorem, we show the other characterizations of weak group inverse by AⓌAAⓌ=AⓌ.
Theorem 3.2 Let A∈Cn×nk and X∈Cn×n. Then the following conditions are equivalent:
(a) X=AⓌ;
(b) XAX=X,R(X)=R(Ak) and N(X)=N((Ak)∗A);
(c) XAX=X,R(XA)=R(Ak) and AX= A;
A;
(d) XAX=X,R(X)=R(Ak) and A∗A2X∈CHn.
Proof. (a)⇒(b). We can obtain the assertion from Lemma 2.5.
(b)⇒(c). By XAX=X and R(X)=R(Ak), we can obtain that R(AX)=AR(X)=AR(Ak)=R(Ak+1)=R(Ak)=R( A) and N(AX)=N(X)=N((Ak)∗A)=N(
A) and N(AX)=N(X)=N((Ak)∗A)=N( A). Hence AX =
A). Hence AX =  A, since AX,
A, since AX,  A∈CPn.
A∈CPn.
From R(X)=R(Ak) we get Ak=PXAk. Hence
Thus, we have R(Ak)=R(XAk+1)⊆R(XA)⊆R(X)=R(Ak), we get R(XA)=R(Ak).
(c)⇒(d). Notice that A =PAk∈CHn, then A∗A2X=A∗(A
=PAk∈CHn, then A∗A2X=A∗(A )A∈CHn. From XAX=X and R(XA)=R(Ak), we obtain R(X)=R(Ak).
)A∈CHn. From XAX=X and R(XA)=R(Ak), we obtain R(X)=R(Ak).
(d)⇒(a). Using the core-EP decomposition of A∈Cn×nk (2.1), we partition X as follows:
where X1∈Cp×p, X2∈Cp×(n−p), X3∈C(n−p)×p, X4∈C(n−p)×(n−p) and p=r(Ak).
By R(X)=R(Ak), we get that X=U(X1X200)U∗ and r(X1,X2)=p, the matrix (X1,X2) is full row rank. Thus, we have X1T=Ip by XAX=X. Hence X1=T−1. Again note that A∗A2X∈CHn, moreover
we have S∗T=(T∗T2X2)∗ and X2=T−2S. Hence X=U(T−1T−2S00)U∗=AⓌ.
From Theorem 3.2, by R(AⓌ)=R(Ak) we know that AⓌ also satisfies the condition (7) in the definition of Drazin inverse. In the following theorem, we show some characterizations of AⓌ by AⓌAk+1=Ak.
Theorem 3.3 Let A∈Cn×nk and X∈Cn×n. Then the following conditions are equivalent:
(a) X=AⓌ;
(b) XAk+1=Ak,AX2=X and A2X=PAkA;
(c) XAk+1=Ak,AX=  A and r(X)=r(Ak).
A and r(X)=r(Ak).
Proof. (a)⇒(b). By (2.13) and simple calculation, it is obvious.
(b)⇒(c). Since AX2=X, by induction it then follows that X=AX2=AXX=A(AX2)X=A2X3=⋯=AkXk+1. Thus, by Ak=U(Tk˜T00)U∗, where ˜T=∑k−1j=0TjSNk−1−j, we can let X=U(X1X200)U∗, where X1∈Cp×p, X2∈Cp×(n−p) and p=r(Ak).
By conditions XAk+1=Ak and A2X=PAkA, we get
Thus AX=  and r(X)=r(Ak).
and r(X)=r(Ak).
(c)⇒(a). From XAk+1=Ak and r(X)=r(Ak), we have R(X)=R(Ak). XAX=X and R(XA)=R(Ak). Thus, by (c) of Theorem 3.2, we obtain that X=AⓌ.
Remark 3.4 Notice that the condition XAk+1=Ak in items (b) and (c) of Theorem 3.3 can be replaced by XAl+1=Al(l≥k). Also the condition AX2=X in items (b) of Theorem 3.3 can be replaced by X=AtXt+1(t≥1).
By Lemma 2.6, it is clear that AX=PR(Ak),N((Ak)∗A) and XA=PR(Ak),N((Ak)∗A2) when X=AⓌ. However, the conditions AX=PR(Ak),N((Ak)∗A) and XA=PR(Ak),N((Ak)∗A2) can not deduce that X=AⓌ. We will present the following example to illustrate that.
Example 3.5 Let
It is easy to check that k=Ind(A)=2, AX=PR(Ak),N((Ak)∗A) and XA=PR(Ak),N((Ak)∗A2). However, X≠AⓌ.
But we have the following result.
Theorem 3.6 Let A∈Cn×nk and X∈Cn×n. Then X=AⓌ if and only if X satisfies the following:
Proof. If X=AⓌ, we can obtain the assertion directly by Lemma 2.6 and Theorem 3.3.
Conversely, by conditions R(XA)=R(Ak) and r(X)=r(Ak), we get R(X)=R(Ak) and XAX=X. Thus, N(X)=N(AX)=N((Ak)∗A). Therefore, X=AⓌ by Lemma 2.5.
The definition of AⓌ has been introduced from an algebraic approach. In [17], Dijana Mosic and Daochang Zhang characterized AⓌ by AX=PR(Ak),N((Ak)∗A),R(X)⊆R(Ak). In the following, we characterize AⓌ by the condition XA=PR(Ak),N((Ak)∗A2).
Theorem 3.7 Let A∈Cn×nk. Then AⓌ is the unique matrix X that satisfies:
Proof. AⓌ satisfies the two equations in (3.1) by Lemma 2.5 and Lemma 2.6. It remains to prove the uniqueness.
Suppose that X1,X2 satisfy (3.1). Then X1A=X2A, N(X1)⊇N((Ak)∗A) and N(X2)⊇N((Ak)∗A), we first show that N((Ak)∗)∩R(A∗Ak)={0}. For any η∈N((Ak)∗)∩R(A∗Ak), we get (Ak)∗η=0, η=A∗Akξ for some ξ∈Cn. Since Ind(A)=k, then Akξ=Ak+1ξ0 for some ξ0∈Cn. Since 0=(Ak)∗η=(Ak+1)∗Ak+1ξ0, we get Ak+1ξ0=0, that is η=A∗Akξ=A∗Ak+1ξ0=0. Hence, N((Ak)∗)∩R(A∗Ak)={0}.
From (X1−X2)A=0, we get R(X∗1−X∗2)⊆N(A∗)⊆N((Ak)∗). From N(X1)⊇N((Ak)∗A) and N(X2)⊇N((Ak)∗A), we get R(X∗1−X∗2)⊆R(A∗Ak), that is R(X∗1−X∗2)⊆N((Ak)∗)∩R(A∗Ak)={0}. Hence, X∗1=X∗2 and X1=X2.
Bott-Duffin defined the B-D inverse of A∈Cn×n by A(−1)(L)=PL(APL+PL⊥)−1=PL(APL+I−PL)−1 when APL+PL⊥ is nonsingular (see [5]). In [12], A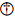 is expressed by the B-D inverse. In the following, we use a special B-D inverse of A2 to express the weak group inverse of A.
is expressed by the B-D inverse. In the following, we use a special B-D inverse of A2 to express the weak group inverse of A.
Theorem 3.8 Let A∈Cn×nk. Then
Proof. By (2.1), we have Ak=U(Tk˜T00)U∗, where ˜T=∑k−1j=0TjSNk−1−j. Then PAk=Ak(Ak)+=U(I000)U∗. Thus
By direct calculation, we can get AⓌ=(PAkA2PAk)+A.
Example 3.9 (see [16], Example 3.1) Let A=(55432144432133332122221011110−10000−1−2).
Since Ind(A)=2, by Theorem 2.1 of [16] the error-free weak group inverse of A is
On the other hand,
After simplification, it follows that A(2)(R(A2))A=AⓌ and (PA2A2PA2)+A=AⓌ.
In Theorem 2.2 of [16] the authors proved that AⓌ=(A+P)−1(I−Q)=(A−P)−1(I−Q) by using P=I−AⓌA and Q=I−AAⓌ. Now we generalize this equation by other ways.
Theorem 3.10 Let A∈Cn×nk, a≠0, P=I−AⓌA and Q=I−AAⓌ. Then the matrices A+aP and A+aQ are invertible. In addition, the following identities hold:
(a) AⓌ=(A+aP)−1(I−Q);
(b) AⓌ=(I−P)(A+aQ)−1.
Proof. (a) Firstly, we show that A+aP is invertible by (2.1) and (2.13).
Let α=U(α1α2)∈Cn, where α1∈Cp, α∈N(A+aP), then
Thus, α2=0 and α1=0 as a≠0, N is nilpotent and T is nonsingular.
By (A+aP)AⓌ=[A+a(I−AⓌA)]AⓌ=AAⓌ=I−Q, we get AⓌ=(A+aP)−1(I−Q).
(b) It is similar to the proof of (a).
Remark 3.11 In the part (a) of Theorem 3.10, let a=±1, we have Theorem 2.2 of [16].
Example 3.12 Let A,AⓌ be the same as in example 3.9, P=I−AⓌA and Q=I−AAⓌ. Then
By direct calculation, we have (A+12P)−1(I−Q)=AⓌ and (I−P)(A−13Q)−1=AⓌ.
In the following theorem we present a connection between (B,C)-inverse and weak group inverse, it shows that a weak group inverse of a matrix A∈Cn×nk is its (Ak,(Ak)∗A)-inverse.
Theorem 3.13 Let A∈Cn×nk. Then AⓌ=A(Ak,(Ak)∗A).
Proof. From the properties of weak group inverse (Lemma 2.5, Lemma 2.6 and Theorem 3.3), it follows that
Hence AⓌ=A(Ak,(Ak)∗A).
In Theorem 3.1 of [16], the limit representation for the weak group inverse is derived using the limit representation of MP-inverse. In the following, the weak group inverse can also be characterized by Lemma 2.4.
Theorem 3.14 Let A∈Cn×nk. Then
(a) AⓌ=limλ→0Ak[λIn+(Ak)∗Ak+2]−1(Ak)∗A;
(b) AⓌ=limλ→0Ak(Ak)∗A[λIn+Ak+1(Ak)∗A]−1;
(c) AⓌ=limλ→0[λIn+Ak(Ak)∗A2]−1Ak(Ak)∗A;
(d) AⓌ=limλ→0Ak(Ak)∗[λIn+Ak+2(Ak)∗]−1A.
Proof. In the proof of the theorem, the results of Lemma 2.4 and Lemma 2.5 are used frequently.
(a). Let X=Ak,Y=(Ak)∗A. We have
(b). Let X=Ak(Ak)∗A,Y=In. Then
(c). Let X=In,Y=Ak(Ak)∗A. Then
(d). Let X=Ak(Ak)∗, Y=A. Then
Example 3.15 Let A,AⓌ be the same as in example 3.9, M=A2[λI6+(A2)∗A4]−1(A2)∗A=(mij)6×6, where
By direct calculation, we get
4.
Some properties of weak group inverse
In this section, we first give some properties of weak group inverse by core-EP decomposition.
Theorem 4.1 Let A∈Cn×nk be as in (2.1). Then the following statements hold:
(a) AⓌ=0⟺A is nilpotent;
(b) AⓌ=A⟺A3=A;
(c) AⓌ=A∗⟺T∈CUp and A∈CEPn;
(d) AⓌ=PA⟺A∈COPn.
(e) AⓌ=PA∗⟺A∈COPn.
Proof. Using the core-EP decomposition of A and (2.13).
where Δ=[TT∗+S(In−p−N†N)S∗]−1.
It is well-known that A†=A# if and only if A is EP matrix. By the core-EP decomposition we will give the conditions which ensure that AⓌ=X, where X is one of A#, 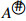 , A†,
, A†,  , AD,A⋄,AD,†,AC,† and A†,D.
, AD,A⋄,AD,†,AC,† and A†,D.
Theorem 4.2 Let A∈Cn×nk. Then the following statements hold:
(a) AⓌ=A#⟺A∈CCMn;
(b) AⓌ= 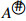 ⟺A∈CEPn;
⟺A∈CEPn;
(c) AⓌ=A†⟺A∈CEPn.
Proof. The proof is based on the core-EP decomposition of A.
(a). From (2.2) and (2.13), we have
(b). From (2.2) and (2.13), we get that
(c). From (2.3) and (2.13), we have
where Δ=[TT∗+S(In−p−N†N)S∗]−1.
Theorem 4.3 Let A∈Cn×nk be as in (2.1). Then the following statements hold:
(a) AⓌ=  ⟺S=0;
⟺S=0;
(b) AⓌ=AD⟺SN=0;
(c) AⓌ=A⋄⟺S=0 and N2=0;
(d) AⓌ=AD,†⟺SN2=0 and S=SNN†;
(e) AⓌ=A†,D⟺S=SN†N and SN=0;
(f) AⓌ=AC,†⟺S=SN†N,SN2=0 and S=SNN†.
Proof. The core-EP decomposition of A is still our main tool.
(a). From (2.5) and (2.13), the result is obvious.
(b). See [10, Lemma 4.2, Theorem 4.3].
(c). By (2.3), (2.11) and (2.13), we have
where Δ2=[T−1(T−1)∗+T−2S(T−2S)∗]−1.
(d). By (2.7) and (2.13), we get that
where ˜T=k−1∑j=0TjSNk−1−j.
(e). By (2.8) and (2.13), we have
where Δ=[TT∗+S(In−p−N†N)S∗]−1 and ˜T=k−1∑j=0TjSNk−1−j.
(f). By (2.9) and (2.13), we get that
where Δ and ˜T are the same as in (e).
5.
Different characterizations of weak group matrix
In [23], Wang and Liu introduced the weak group matrix defined by the commutability: AAⓌ=AⓌA. In this section we shall give different characterizations of weak group matrix by using the core-EP decomposition.
For convenience, we introduce a necessary lemma.
Lemma 5.1 [23] Let A∈Cn×nk be as in (2.1). Then the following statements are equivalent:
(a) A∈CWGn;
(b) SN=0;
(c) (A2)Ⓦ=(AⓌ)2;
(d)  A commutes with
A commutes with  A2;
A2;
(e) r(A∗Ak,(A∗)2Ak)=r(Ak).
Remark 5.2 For (e) of Lemma 5.1, we give a short proof by using the properties of the weak group inverse.
Since r(A∗Ak)=r((A∗)2Ak)=r(Ak), we get that r(A∗Ak,(A∗)2Ak)=r(Ak) can be equivalently expressed as R(A∗Ak)=R((A∗)2Ak), that is: N((Ak)∗A)=N((Ak)∗A2).
Hence, AAⓌ=AⓌA if and only if r(A∗Ak,(A∗)2Ak)=r(Ak) by Lemma 2.6.
Theorem 5.3 Let A∈Cn×nk. Then the following conditions are equivalent:
(a) A∈CWGn;
(b) ( )tA= (
)tA= ( )t+1A2(t≥1);
)t+1A2(t≥1);
(c) ( )tA commutes with (
)tA commutes with ( )tA2(t≥1).
)tA2(t≥1).
Proof. By the core-EP decomposition of A, we have
Then, it is easy to prove these conclusions.
Remark 5.4 Let t=1 in (c) of Theorem 5.3, we get (d) of Lemma 5.1.
Theorem 5.5 Let A∈Cn×nk. Then the following conditions are equivalent:
(a) A∈CWGn;
(b) AkA†=Ak ;
;
(c) Ak = AkAD,†;
= AkAD,†;
(d)  Ak=AkAⓌ;
Ak=AkAⓌ;
(e) AAD=  A;
A;
(f) AAD=AAⓌ;
(g)  A=AD,†A.
A=AD,†A.
Proof. (a)⟺(c). By (2.5) and (2.7), we get that
where ˜T=k−1∑j=0TjSNk−1−j.
(a)⟺(f). By (2.4) and (2.13), we have
where ˜T=k−1∑j=0TjSNk−1−j.
The rest of the proof is similar.
6.
Conclusions
Our goal is to provide some new characterizations and properties of the weak group inverse and the weak group matrix by range space, null space, projection and the Bott-Duffin inverse. We also study the relationships between the weak group inverse and other generalized inverses such as A#, 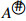 , A†,AD,AD,†.
, A†,AD,AD,†.
We believe that research about the weak group inverse will be very popular in the next years. Some further investigations are proposed as follows:
1. Considering the weak group inverse of finite potent endomorphisms.
2. The applications of the weak group inverse in linear equations and matrix equations.
Acknowledgements
The authors are thankful to two anonymous referees for their careful reading, detailed corrections and pertinent suggestions on the first version of the paper, which enhanced the presentation of the results distinctly.
Funding
This research is supported by the Natural Science Foundation of China under Grants 11961076; the Science Foundation of Hubei Province Education Department (B2018148); the Youth Science Foundation of Hubei Normal University (HS2020QN031) and Thousands of Young and Middle-aged Key Teachers Training Programme in Guangxi Colleges and Universities.
Conflict of interest
All authors read and approved the final manuscript. The authors declare no conflict of interest.









 is defined in [
is defined in [ is defined in [
is defined in [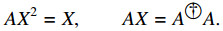
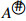 ,
,  ,
, 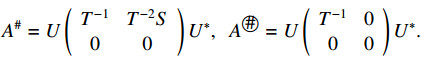
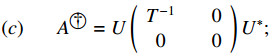

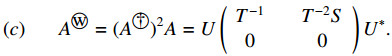
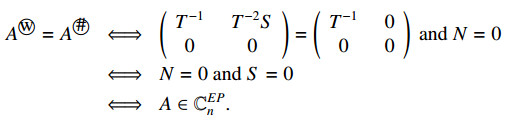

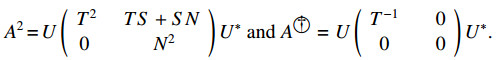
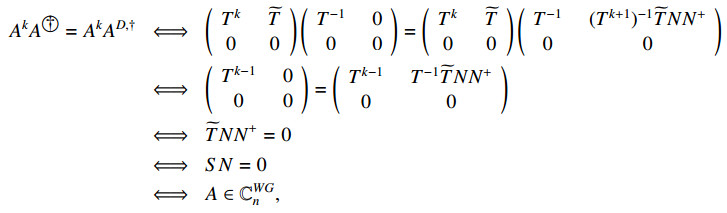




 DownLoad:
DownLoad: