In this paper, we investigate the existence, uniqueness and stability of coupled impulsive fractional integro-differential equations with Riemann-Liouville derivatives. To prove the existence and uniqueness results for afore mentioned system, we use the techniques of Kransnoselskiios type fixed point theorem. Furthermore, different kinds of Ulam stabilities are discussed along with examples, to demonstrate the validity of main results.
1.
Introduction
Fractional order differential equations are the generalizations of the classical integer order differential equations. The idea about the fractional order derivative was introduced at the end of the sixteenth century (1695) when Leibniz used the notation dndσn for nth order derivative. By writing a letter to him, L'Hospital asked the question: what would be the result if n=12? Leibniz answered in such words, "An apparent Paradox, from which one day useful consequences will be drawn", and this question became the foundation of fractional calculus. Fractional calculus has become a speedily developing area and its applications can be found in diverse fields ranging from physical sciences, porous media, electrochemistry, economics, electromagnetics, medicine and engineering to biological sciences. Progressively, fractional differential equations play a very important role in fields such as thermodynamics, statistical physics, nonlinear oscillation of earthquakes, viscoelasticity, defence, optics, control, signal processing, electrical circuits, astronomy etc. There are some outstanding articles which provide the main theoretical tools for the qualitative analysis of this research field, and at the same time, shows the interconnection as well as the distinction between integral models, classical and fractional differential equations, see [14,16,18,19,22,25,26,28,30]
Impulsive fractional differential equations are used to describe both physical, social sciences and many dynamical systems such as evolution processes pharmacotherapy. There are two types of impulsive fractional differential equations the first one is instantaneous impulsive fractional differential equations while the other one is non-instantaneous impulsive fractional differential equations. In last few decades, the theory of impulsive fractional differential equations are well utilized in medicine, mechanical engineering, ecology, biology and astronomy etc. There are some remarkable monographs [3,6,8,15,20,23,33,34], considering fractional differential equations with impulses.
The most preferable research area in the field of fractional differential equations (FDE′s), which received great attention from the researchers is the theory regarding the existence of solutions. Many researchers developed some interesting results about the existence of solutions of different boundary value problems (BVPs) using different fixed point theorems. For details we refer the reader to [2,7,9,10,11,13,27]. Most of the time, it is quite intricate to find the exact solutions of nonlinear differential equations, in such a situation different approximation techniques are introduced. The difference between exact and approximate solutions is nowadays dealt with using Hyers-Ulam (HU) type stabilities, which were first introduced in 1940 by Ulam [29] and then answered by Hyers in the following year in the context of Banach spaces. Many researchers investigated HU type stabilities for different problems with different approaches [12,17,31,35,36,37,39,40].
Zada and Dayyan [38], investigated the existence, uniqueness and Ulam's type stability for the implicit fractional differential equation with instantaneous impulses and Riemann-Liouville fractional integral boundary conditions having the following form
where I=[0,T], and cDα0,σ is a generalization of classical Caputo derivative of order α with lower bound at 0, ϕ1:I×R×R→R is a continuous function. Furthermore, u(σ+j) and u(σ+j) represent the right-sided and left-sided limits respectively at σ=σj for j=1,2,…,q−1.
Ali et al. [4], studied a coupled system for the existence and uniqueness of solution using Riemann-Liouville derivative
where σ∈J=[0,T], T>0, α,β∈(1,2], and β1,β2,γ1,γ2≠1. Dα, Dβ are the Riemann-Liouville fractional derivatives and ϕ1,ϕ2:[0,1]×R×R→R are continuous functions.
Wang et al. [32], presented stability of the following coupled system of implicit fractional integro-differential equations having anti-periodic boundary conditions:
where 1<α,β≤2, 0≤r1,r2≤2, γ1,γ2>0, and J=[0,T], T>0. ϕ1,ϕ2,f,g:J×R×R→R are continuous functions.
Motivated by the above work, we focus our attention on the following coupled impulsive fractional integro-differential equations with Riemann-Liouville derivatives of the form:
where 1<α,β≤2, ϕ1,ϕ2:[0,T]×R×R→R being continuous functions and
where u(σ+j),v(σ+k) and u(σ−j),v(σ−k) are the right limits and left limits respectively, Ej,E∗j,Ek,E∗k:R→R are continuous functions, and Dα,Iα are the α-order Riemann-Liouville fractional derivative and integral operators respectively.
The remaining article is arranged as follows: In Section 2, we present some basic definitions, theorems, and lemmas that will be used in our main results. In Section 3, we use suitable cases for the existence and uniqueness of solution for the proposed system (1.1) using Kransnoselskii's type fixed point theorem. In Section 4, we discuss different kinds of stabilities in the sense of Ulam under certain conditions. In Section 5, an example is given to support the main results.
2.
Auxiliary results
In this section, we present some basics notations, definitions, and results that are used in the whole article.
Let T>0, ω=[0,T]. The Banach space of all continuous functions from ω into R is denoted by C(ω,R) with the norm
and the product of these spaces is also a Banach space with the norm
The piecewise continuous functions with 1<α,β≤2 are denoted as follows:
with the norms
respectively. Their product ϑ=ϑ1×ϑ2 is also a Banach space with the norm ‖(u,v)‖ϑ=‖u‖ϑ1+‖v‖ϑ2.
Definition 2.1. [1] The Riemann-Liouville fractional integral of order α>0 for a function u:R+→R is defined as
where Γ(⋅) is the Euler gamma function defined by Γ(α)=∫∞0e−σσα−1dσ,α>0.
Definition 2.2. For a function u:R+→R, the Riemann-Liouville derivative of fractional order α>0, p=[α]+1, is defined as
provided that integral on the right side exists. [α] denotes the integer part of the real number α. For more properties, the reader may refer to [1].
Lemma 2.1. [1] Let u be any function, and let α>0, then the Riemann-Liouville fractional derivative for the Homogeneous differential equation
has a solution
and for non-homogeneous differential equation
has a solution
where p=[α]+1 and ci,i=1,2,…,p, are real constants.
Theorem 2.1. (Altman [5]) Let Λ≠0 be a convex and closed subset of Banach space ϑ. Consider two operators ℑ1,ℑ2 such that
(1) ℑ1(u,v)+ℑ2(u,v)∈Λ;
(2) ℑ1 is a contractive operator;
(3) ℑ2 is a compact and continuous operator.
Then there exists (u,v)∈Λ such that ℑ1(u,v)+ℑ2(u,v)=(u,v)∈ϑ.
2.1. Ulam's Stabilities and Remarks
The following definitions and remarks are taken from [21,24].
Definition 2.3. The given system (1.1) is HU stable if there exists Nα,β=max{Nα,Nβ}>0 such that, for κ=max{κα,,κβ}>0 and for every solution (ξ,ζ)∈ϑ of the inequality
there exists a solution (u,v)∈ϑ with
Definition 2.4. The given system (1.1) is generalized HU stable if there exists N′∈C(R+,R+) with N′(0)=0 such that, for any approximate solution (ξ,ζ)∈ϑ of inequality (2.1), there exists a solution (u,v)∈ϑ of (1.1) satisfying
Definition 2.5. The given system (1.1) is HUR stable with respect to ψα,β=max{ψα,ψβ} with ψα,β∈C(ω,R) if there exists a constant Nψα,ψβ=max{Nψα,Nψβ}>0 such that, for any κ=max{κα,,κβ}>0 and for any approximate solution (ξ,ζ)∈ϑ of the inequality
there exists a solution (u,v)∈ϑ with
Definition 2.6. The given system (1.1) is generalized HUR stable with respect to ψα,β=max{ψα,ψβ} with ψα,β∈C(ω,R) if there exists a constant Nψα,ψβ=max{Nψα,Nψβ}>0 such that, for any approximate solution (ξ,ζ)∈ϑ of inequality (2.2), there exists a solution (u,v)∈ϑ of (1.1) satisfying
Remark 2.1. Let (ξ,ζ)∈ϑ be a solution of inequalities (2.1) if there exist functions Kϕ1,Lϕ2∈C(ω,R) depending on ξ,ζ respectively such that
(1) |Kϕ1(σ)|≤κα,|Lϕ2(σ)|≤κβ,σ∈ω;
(2)
3.
Existence and uniqueness
In this section, we discuss the existence and uniqueness of solution of the proposed system (1.1).
Theorem 3.1. Let α,β∈(1,2] and ϕ1 be any linear and continuous function. The fractional impulsive differential equation
has a solution
Proof. Consider
For σ∈[0,σ1], Lemma 2.1 gives
Again, for σ∈(σ1,σ2], Lemma 2.1 gives
Hence it follows that
Using
we obtain
Substituting the values of b1, b2 in (3.5), we get
Similarly, for σ∈(σj,σj+1],
Finally, after applying conditions ν1Dα−2u(σ)|σ=0=u1, and μ1u(σ)|σ=T+ν2Iα−1u(σ)|σ=T=u2 to (3.6) and finding the values of a1 and a2, we obtain Eq (2.2).
Corollary 1. In view of Theorem 3.1, our coupled system (1.1) has the following solution:
and
Now, for transformation of the given system (1.1) into a fixed point problem, let the operators ℑ1,ℑ2:ϑ→ϑ be define as follows:
and
For additional analysis, the following hypothesis needs to hold:
(H1) ● For σ∈ω there exist bounded functions o,τ,υ∈ϑ such that
with o1=supσ∈ωo(σ), τ1=supσ∈ωτ(σ), and υ1=supσ∈ωυ(σ)<1.
● Similarly, for σ∈ω there exist bounded functions o∗,τ∗,υ∗∈ϑ such that
with o2=supσ∈ωo∗(σ), τ2=supσ∈ωτ∗(σ), and υ2=supσ∈ωυ∗(σ)<1.
(H2) Ej,E∗j:R→R are continuous and there exist constants GE,GE∗,G′E,G′E∗,ˆGE,ˆGE∗,ˆG′E,ˆG′E∗>0 such that, for any (u,v)∈ϑ,
where z=1,2,…,p.
(H3) ● For all x1,x2,x∗1,x∗2∈R and for each σ∈ω, there exist constants Lϕ1>0, 0<L∗ϕ1<1 such that
● Similarly, for all x1,x2,x∗1,x∗2∈R and for each σ∈ω, there exist constants Lϕ2>0, 0<L∗ϕ2<1 such that
(H4) Ez,E∗z:R→R are continuous and there exist constants LE,LE∗,L∗E,L∗E∗ such that, for any (u,v),(u∗,v∗)∈ϑ,
Here we use Kransnoselskii's fixed point theorem to show that the operator ℑ1+ℑ2 has at least one fixed point. Therefore, we choose a closed ball
where
Theorem 3.2. If hypotheses (H1)–(H4) are hold, then the given system (1.1) has at least one solution.
Proof. 1) For any (u,v)∈ϑr, we have
From (3.9), we get
This implies that
Similarly, we can obtain
where
Also, we have
Now by (H1)
Now, taking supσ∈ω on both sides, we get
Now taking supσ∈ω of (3.15) and using (3.16) in (3.15), we get
Similarly,
where
Putting (3.13), (3.14), (3.17) and (3.18) in (3.11), we get
Hence, ‖ℑ1(u,v)+ℑ2(u,v)‖ϑ∈ϑr.
2) Next, for any σ∈ω, (u,v),(ξ,ζ)∈ϑ
Here L=max{LE,LE∗,L∗E,L∗E∗},
and
Therefore, ℑ1 is a contractive operator.
3) Now, for the continuity and compactness of ℑ2, we make a sequence Ts=(us,vs) in ϑr such that (us,vs)→(u,v) for s→∞ in ϑr. Thus, we have
This implies ‖ℑ2(us,vs)−ℑ2(u,v)‖ϑ→0 as s→∞, therefore ℑ2 is continuous.
Next, we show that ℑ2 is uniformly bounded on ϑr. From (3.17) and (3.18), we have
Thus, ℑ2 is uniformly bounded on ϑr.
For equicontinuity, suppose η1,η2∈ω with η1<η2, and for any (u,v)∈ϑr⊂ϑ where ϑr is clearly bounded, we have
This implies that
In the same way, we have
Hence
Thus, ℑ2 is equicontinuous. So ℑ2 is relatively compact on ϑr. Hence, by the Arzelˊa–Ascoli Theorem, ℑ2 is compact on ϑr. Thus all the condition of Theorem 2.1 are satisfied. So the given system (1.1) has at least one solution.
Theorem 3.3. Let hypotheses (H3), (H4) be satisfied with
then the given system (1.1) has unique solution.
Proof. First we define an operator φ=(φ1,φ2):ϑ→ϑ, i.e., φ(u,v)(σ)=(φ1(u,v),φ2(u,v))(σ), where
and
In view of Theorem 3.2, we have
Taking supσ∈ω, we get
where
Similarly,
where
Hence
This implies that the operator φ is a contraction. Therefore, (1.1) has a unique solution.
4.
Ulam's stability analysis
In this section, we study different kinds of stabilities, like HU, generalized HU, HUR, and generalized HUR stability of the proposed system.
Theorem 4.1. If assumptions (H3), (H4) and inequality (3.19) are satisfied and
then the unique solution of the coupled system (1.1) is HU stable and consequently generalized HU stable.
Proof. Let (ξ,ζ)∈ϑ is a solution of inequality (2.1), and let (u,v)∈ϑ be the unique solution of the coupled system given by
By Remark 2.1 we have
By Corollary 1, the solution of problem (4.2) is
and
We consider
As in Theorem 3.3, we get
and
From (4.5) and (4.6), we have
and
respectively. Let
Then the last two inequalities can be written in a matrix form as follows:
where
From system (4.7) we have
which implies that
If κ=max{κα,κβ} and Nα,β=P2F+P1P4F+P2P3F+P4F, then
Thus system (1.1) is HU stable. Also, if
with N′(0)=0, then the given system (1.1) is generalized HU stable.
For the next result, we assume the following:
(H5) Let there exists two nondecreasing functions wα,wβ∈C(ω,R+) such that
Theorem 4.2. If assumptions (boldsymbol)–(H5) and inequality (3.19) are satisfied and
then the unique solution of the given system (1.1) is HUR stable and accordingly generalized HUR stable.
Proof. With the help of Definitions 2.5 and 2.6, we can achieve our result doing the same steps as in Theorem 4.1.
5.
Example
Here we present a specific example, as follows.
Example 5.1. Let
From system (5.1), we see that α=65, β=54, μ1=μ2=−50, ν1=ν3=1, ν2=ν4=185, T=e, σ1=32, and u1,u2,v1,v2∈R.
Set
Now, for all u,u∗,v,v∗∈R, and σ∈[0,e], we obtain
and
These satisfy condition (H3) with Lϕ1=L∗ϕ1=180e90, Lϕ2=L∗ϕ2=195.
Set
Then we have
These satisfy condition (H4) with LE=L∗E=LE∗=L∗E∗=170.
From Theorem 3.3, we use the inequality and get
hence (5.1) has a unique solution, so (5.1) has a solution (u,v)∈ϑ. The solution of (5.1) is given by
and
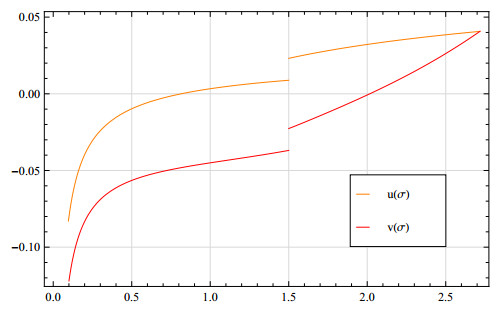
(i) If we take ϕ1(σ,Iαu(σ),Iβv(σ))=180eσ+90, ϕ2(σ,Iαu(σ),Iβv(σ))=σcos(σ)−sin(σ)95−195, E1(u(32))=E∗1(u(32))=E1(v(32))=E∗1(v(32))=170, and u(σ)=v(σ)=σ then with the constant values u1=v1=115, u2=v2=2, the graph of the solution is shown in Figure 1.
(ii) If we take ϕ1(σ,Iαu(σ),Iβv(σ))=σ+180eσ+90, ϕ2(σ,Iαu(σ),Iβv(σ))=σ2+195−σ95, E1(u(32))=E∗1(u(32))=E1(v(32))=E∗1(v(32))=170, and u(σ)=v(σ)=σ then with the constant values u1=v1=−115, u2=v2=−2, the graph of the solution is shown in Figure 2.
From Theorem 4.1, we use the inequality and get
thus, the given system (5.1) is HU stable and also generalized HU stable. Likewise, we can justify the condition of Theorems 3.2 and 4.2.
6.
Conclusion
In this article, we used the Kransnoselskii's fixed point theorem and acquired the necessary cases for the existence and uniqueness of solution for the given fractional integro-differential Eqs (1.1). Furthermore, under specific assumptions and conditions, we proved different kinds of Ulam's stability of system (1.1). The concept of Ulam's stability is very important because it gives a relationship between approximate and exact solutions, so our results may be very helpful in approximation theory and numerical analysis. The mentioned stability is rarely investigated for impulsive fractional integro-differential equations. Finally, we illustrated the main results by giving a suitable example.
Acknowledgments
This research was supported by the Natural Science Foundation of Jiangxi Province (Grant Nos. 20192BAB201011, 20192BCBL23030 and 20192ACBL21053) and the National Natural Science Foundation of China (Grant No. 11861053).
Conflict of interest
The authors declare that they have no conflict of interest.










 DownLoad:
DownLoad: