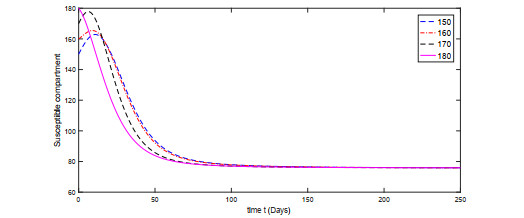
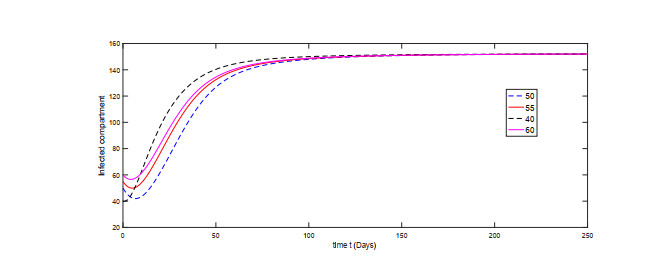
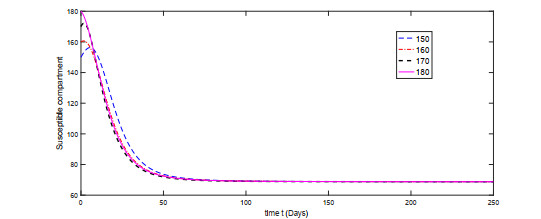
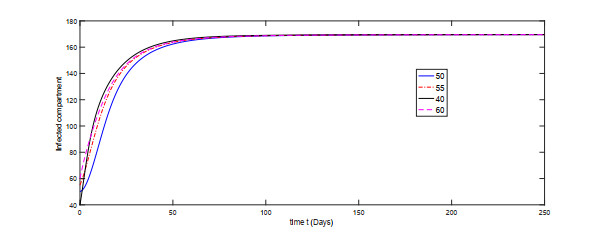
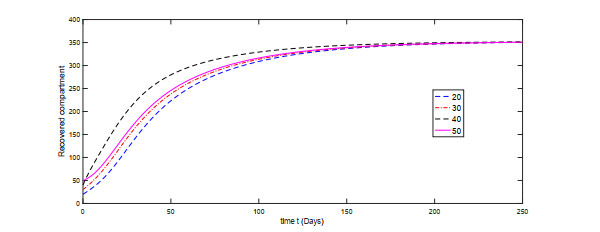
In this manuscript, we examine the SIR model under convex incidence rate. We first formulate the famous SIR model under the aforesaid incidence rate. Further, we develop some sufficient analysis to examine the dynamical behavior of the model under consideration. We compute the basic reproductive number R0. Also we study the global attractivity results via using Dulac function theory. Further, we also provide some information about the stability of the endemic and disease free equilibria for the considered model. In addition, we use nonstandard finite difference scheme to perform numerical simulation of the considered model via using Matlab. We provide different numerical plots for two different values of contact rate and taking various initial values for compartments involved in the considered model.
List of abbreviations
1.
Introduction
The uncertainty and complexity of decision-making information have an impact on decision-making results. To describe decision-making information more accurately and truly, Zadeh [10] introduced fuzzy set theory. One of the most successful and promising adaptations of the Zadeh notion is the intuitionistic fuzzy set (IFS) [1] theory. Colak et al. [2] exhibited the criteria and sub-criteria for performance assessment of Blockchain Technology (BT) in supply chain management. The weights of main and sub-criteria have been attained through HF-AHP method, and a categorized MCDM problem that comprises of 5 main and 17 sub-criteria has been established and the alternative segments have been analyzed. A decision model for selecting the finest blockchain platform has been exhibited by Farshidi et al. [3]. The stated model has been assessed with three case studies of real problems. Cognitive function methods based on FST and Decision-Making Trail and Evaluating Laboratory (DEMATEL), MCDM methodology has been introduced by Karaşan et al. [4].
For instance, the depositor wishes an agreement to choose the most efficient enterprise according to avert asset risk than secure the highest payback, yet the organization desires in imitation of assessing its automation degree before improving it. Usually, whole its things to do, need conformity and remain evaluated along respect in conformity with several attributes, as are additionally acknowledged as MADM issues yet are entirely frequent between our daily life. MADM is a condition confronted when persons together brand an excellent from the replacements with deference to a usual attribute. MADM difficulties inhabit vital situations in the arena of culture decision making. For sample, the depositor desires to choose the optimum business to avoid venture danger and gain the supreme reappearance, and the innovativeness. MADM problems have conventional countless considerations in the historical few decades. The MCDM techniques are represented in Figure 1.
Lin et al. [5] presented reviewed blockchain-based theories connected with ICT-based technology. Tang et al. [7] presented assessment statistics and the ranking results of public blockchains.
A generic class of intuitionistic and Pythagorean sets is known as q-rung Orthopair fuzzy sets which was suggested by Yager [8]. Orthopair fuzzy subsets are such that the degree of support for membership in the fuzzy set and the degree of support against membership are indicated by pairs of values from the unit interval in the membership grades. We noticed that the q-rung Orthopair fuzzy sets where the qth power of the support for and qth power of the support against are sum together and bonded by one. We observe that when q rises, the range of acceptable Orthopair expands, giving the user greater latitude in expressing their opinion regarding membership grade. The key properties and features of the blockchain were provided by Yaqoob et al. [9]. We go over the top benefits of implementing blockchain technology as well as potential for the healthcare sector. Zhang et al. [11] suggested a cyber security paradigm with human-in-the-loop intelligence based on the limitations and challenges. Zhou et al. [12] presented a new PF-VIKOR method considering the DM's choice for risk, a new way to estimate the distance that is presented in detail, and a difficulty with choosing the best blockchain technology solution.
Senapati et al. [13] developed the Fermatean fuzzy TOPSIS method to address the problem of multiple criteria decision-making. The approach for the MCDM issue considering new operators under the Fermatean fuzzy condition was provided by Senapati et al. [14]. The Fermatean fuzzy weighted product model was presented by Senapati et al. [15] to address the multi-criteria decision-making issue. Type-2 fuzzy sets were presented by Own [16], as well as the relationship between type-2 fuzzy sets and intuitionistic fuzzy sets. Mondal et al. [17] developed the notion of the tangent similarity measure of intuitionistic fuzzy sets.
Deng et al. [18] introduced the matters in the outranking relative secret into three diverse areas by a clustering technique. Wang et al. [19] presented uniqueness of the essential fixings for the regret philosophy of the regret-rejoice function and hesitant fuzzy environments. Deng et al. [20] introduced the renovate the multi-scale assessment data into an interval fuzzy number by applying a linguistic term set. Zhan et al. [21] introduced the Triple interactive executive with hesitant fuzzy data. Gai et al. [22] presented dependable capitals to facilitate the attainment of cluster consensus. An approach based on large-scale group decision making (LSGDM) and online reviews is proposed by Feixia Ji et al. [23] to assess user satisfaction with shared living. Wu et al. [24] presented an optimization model built to minimize the total adjustment cost of reaching consensus by determining the personalized feedback adoption coefficient based on individuals' consensus levels. This method reflects both the subjective assessments of the constant standards and the unique risk perspectives about innovation.
Different variations of the Fermatean fuzzy set were introduced by Akram and other scholars, we summarized these as follows. Akram et al. [25] introduced the Fermatean fuzzy soft expert knowledge. Akram et al. [26] introduction complex Fermatean fuzzy N-soft sets. Akram et al [27] introduced the objective functions that should be maximized. Akram et al. [28] developed a novel MCGDM analysis by fusing soft expert sets with m-polar fuzzy sets, he also looked into their structural characteristics, as well as the subset, complement, intersection, union, and OR and AND operators. Alcantud et al. [29] proposed a novel theory for the aggregation of N-soft sets, initial scores of alternatives, determined by multi-agent, multinary evaluations. Akram et al. [30] presented 2TLCq-RPF aggregation operators, including 2TLCq-RPF weighted averaging/geometric operators, sophisticated aggregation operators based on the Hamacher operator key characteristics of the new operators, and multi-attribute decision making strategy for problems posed in the 2TLCq-RPFS setting. The algebraic and Einstein operations are extended by the Hamacher operation. The technique based on the Combined Compromise Solution (COCOSO) with Criteria Importance was developed by Akram et al. [31] by combining 2-tuple linguistic Fermatean fuzzy (2TLFF) numbers and the Hamacher operation. Issues with multiple attribute group decision-making (MAGDM) in a 2TLFF context can be managed by Criteria Importance Through inter-criteria correlation (CRITIC). Different extensions of the fuzzy set and their interrelationship have been described in Figure 2.
In accordance with the available literature and magnificent structure of innovation, we define the standard criteria to comprehensively grade the blockchain skill beneficiaries' performance concerning the reopen allowance for chief circles. We define the Fermatean fuzzy Schweizer-Sklar Operators, which can inarticulacy and hesitations in verdicts due to deficient postulation and sharing related, to gain the criterion weights with FF statistics. We use the Fermatean fuzzy Schweizer-Sklar Operators, determining the inclusive authority ranking for each pretender, and active the entrants in dropping direction sighted the DM's threat need regarding a blockchain by decisive the reduction subject of the affronted.
The standards and techniques for evaluating blockchain capabilities, phases, crops, and suppliers are briefly evaluated. This establishes the framework for developing the standards for selecting the best blockchain knowledge provider for primary sets of financial organizations to link blockchain expertise throughout the process of cross-border transmittal.
The goals and motivations of this paper are:
(1) To endorse the notion of FFS.
(2) Investigate the structure of FFSSTWA operators.
(3) Suggest an SSTT operation for FF components.
(4) To suggest an innovative technique for MADM founded on the planned operators. Congruently, the offerings of this paper are:
i. We presented an intimate of data aggregation operators by joining the FFS and SST operators founded on SSTT functioning rules.
ii. We provide a universal background of the proposed technique to MADM complications.
iii. Offered process to innovativeness statistics and assessment.
The break of this paper is systematized as follows: Section 2, discussed FFN and operational laws. Section 3 remembrances approximately plain designs and provides the SSTT operational rules established on FFS. Section 4 proposes a series of FFN aggregation operators. Section 5 presents an innovative method to MADM. Section 6 delivers a mathematical example to authenticate the rationality and qualities of the proposed viewpoint. Section 7, we discuss in the conclusion. Finally, interdependency of the different sections of the paper has been exhibited in Figure 9.
2.
Preliminaries
We recall some basic concepts of Fuzzy set in this section, to understand the paper. Zadeh introduced the fuzzy set [10]. We recall this as follows.
Definition 2.1. [10] Let us consider that Φ≠H and by a fuzzy set γ={⟨y,ηγ(y)⟩:y∈H},ηγ(y) is a mapping from H to [0,1] characterize membership task of an component y in H.
FFN and operational laws
Fermatean Fuzzy Numbers (FFN) and their operational laws, score and accuracy functions were defined by Senapati et al. [14], we recall it's as follows.
Definition 2.1.1. [14] The fixed setE and the FFN F is defined in F={⟨ςF(y),χF(y)⟩:y∈E}, anywhere ςF(y) and χF(y) characterize the MED and NOMED, and ςF(y)∈[0,1], χF(y)∈[0,1] and 0⩽ςF(y)3+χF(y)3⩽1. The grade of indeterminacy is defined as πF(y)=3√(ςF(y)3+χF(y)3−ςF(y)3χF(y)3). The FFN is indicated as F=⟨ςF,χF⟩.
Operational laws of FFNs are described as follows:
Definition 2.1.2. [14] Let a1={ς1,χ1} and a2={ς2,χ2} be two FFNs, λ>0, then.
Definition 2.1.3. [14] Let ϖ={υ,δ},be the FFNs, then the score is ϖ={υ3−δ3}.
Definition 2.1.4. [14] Letϖ={υ,δ},be the FFNs, then the accuracy is ϖ={υ3+δ3}.
3.
Schweizer-Sklar t-norm and t-conorm
The descriptions of Schweizer-Sklar t-norm and t-conorm are offered as follows:
where Γ<0,f,e∈[0,1]. Additionally, when Γ→0, we have
T(f,e)=fe and T(f,e)=f+e−fe.
They are the statistical t-norm and statistical t-conorm.
FFN operational laws founded on Schweizer-Sklar Operators
Definition 3.1.1. Let a1=[η1,κ1] and a2=[η2,κ2] be two FFNs based on SST operator and Γ>0, then,
4.
FFN aggregation operators
In this section, FFSSTWA, FFSSTOWA, FFSSTHWA, FFSSTWG, FFSSTOWG and FFSSTHWG operators are defined and graphically represented their interrelationship, see Figure 3.
4.1. FFSSTWA operator
Definition 4.1.1. The collection of FFNs are cj=[η,κ] and the weight vector is e=(e1,e2,...,en)T with ej∈[0,1] and n∑j=1ej=1. Then FFSST(c1,c2,...,cn)=n⊕j=1ejcj
is assumed to be a FFSSTWA operator.
Theorem 4.1.2. The collection of FFNs gj={[η,ϕ]} and the weight vector is χ=(χ1,χ2,...,χn)T with χj∈[0,1] and n∑j=1χj=1. Then it will be a FFSSTWA operator and
Proof. By induction on n, we will continue. If n is 1 then
Let the statement is true for n=k
Now for n=k+1
The following theorems' proofs are simple; therefore, we skip them.
Theorem 4.1.3. (Idempotency): If ⌢Y=[κ,ς] for all h=1,2,3,...,m, then
Theorem 4.1.4. (Commutativity): If (d′1,d′2,...,d′n) is any permutation of (d1,d2,...,dn), then FFSSTWA (d′1,d′2,...,d′n)= FFSSTWA (d1,d2,...,dn).
Theorem 4.1.5. (Boundedness): If Y−=min(d1,d2,...,dn),Y+=max(d1,d2,...,dn), then Y−⩽FFSSTWA(d1,d2,...,dn)⩽Y+.
4.2. FFSSTOWA operator
Definition 4.2.1. The gathering of FFNs are ck=[η,ϕ] and the weight vector is h=(h1,h2,...,hm)T with hk∈[0,1] and m∑k=1hk=1.The
is said to be FFSSTOWA operator.
The proof of the following theorems is straightforward, and we omit it.
Theorem 4.2.2. The collection of FFNs are fk={[η,ϕ]} and the weight vector is ϑ=(ϑ1,ϑ2,...,ϑn)T with ϑj∈[0,1] and n∑k=1ϑk=1. Then it will be a FFSSTOWA operator and
The proof of the following theorems is straightforward, and we omit it.
Theorem 4.2.3. (Idempotency): If ⌢Y=[κ,ς] for all r=1,2,3,...,m, then FFSSTOWA(Y,Y,Y,...,Y)=Y.
Theorem 4.2.4. (Commutativity): If (d′1,d′2,...,d′n) is any permutation of (d1,d2,...,dn), then FFSSTOWA (d′1,d′2,...,d′n)=FFSSTOWA (d1,d2,...,dn)
Theorem 4.2.5. (Boundedness): If Y−=min(e1,e2,...,en),Y+=max(e1,e2,...,en), then Y−⩽FFSSTOWA(e1,e2,...,en)⩽Y+.
4.3. FFSSTHWA operator
Definition 4.3.1. The congregation of FFNs are cj=[η,φ] and the weight vector is g=(g1,g2,...,gn)T with gj∈[0,1] and n∑j=1gj=1. Then FFSST(c1,c2,...,cn)=n⊕j=1gjcj
will be a FFSSTHWA operator.
The proof of the following theorems is straightforward, and we omit it.
Theorem 4.3.2. The gathering of FFNs are gj={[η,ϕ]} and the weight vector is u=(u1,u2,...,un)T with uj∈[0,1] and n∑j=1uj=1. Then it is held FFSSTHWA operator and
Theorem 4.3.3. (Idempotency): If ⌢O=[κ,ς] for all k=1,2,3,...,n, then FFSSTHWA (O,O,O,...,O)=O.
Theorem 4.3.4. (Commutativity): If (e′1,e′2,...,e′m) is any permutation of (e1,e2,...,em), then FFSSTHWA (e′1,e′2,...,e′m)=FFSSTHWA (e1,e2,...,em).
Theorem 4.3.5. (Boundedness): If Y−=min(d1,d2,...,dm),Y+=max(d1,d2,...,dm), then Y−⩽ FFSSTHWA (d1,d2,...,dm)⩽Y+.
4.4. FFSSTWG operator
Definition 4.4.1. The congregation of FFNs are ck=[η,ϕ] and the weight vector is f=(f1,f2,...,fm)T with fk∈[0,1] and m∑k=1fk=1. Then FFSST(c1,c2,...,cm)=m⊕k=1cfkk
is said FFSSTWG operator.
Theorem 4.4.2. The collection of FFNs are dj={[η,ϕ]} and the weight vector is e=(e1,e2,...,em)T with ej∈[0,1] and m∑j=1ej=1. Then it is held FFSSTWG operator and
Proof. By induction on m, we will continue. If m is 1 then
Let the statement is true for m=k
Now for m=k+1
The proof of the following theorems is straightforward, and we omit it.
Theorem 4.4.3 (Idempotency): If ⌢Y=[κ,ς] for all h=1,2,3,...,m, then
FFSSTWG(Y,Y,Y,...,Y)=Y.
Theorem 4.4.4. (Commutativity): If (d′1,d′2,...,d′n) is some permutation of (d1,d2,...,dn), then FFSSTWG (d′1,d′2,...,d′n)=FFSSTWG (d1,d2,...,dn)
Theorem 4.4.5. (Boundedness): If Y−=min(d1,d2,...,dn),Y+=max(d1,d2,...,dn), then Y−⩽FFSSTWG(d1,d2,...,dn)⩽Y+.
4.5. FFSSTOWG operator
Definition 4.5.1. The collection of FFNs are cj=[η,κ] and the weight vector is e=(e1,e2,...,en)T with ej∈[0,1] and n∑j=1ej=1. Then FFSST (c1,c2,...,cn)=n⊕j=1cejj is said FFSSTOWG operator.
The proof of the following theorems is straightforward, and we omit it.
Theorem 4.5.2. The gathering of FFNs are dj={[η,ϕ]} and the weight vector is e=(e1,e2,...,em)T with ej∈[0,1] and m∑j=1ej=1. Then it is held FFSSTOWG operator and
Theorem 4.5.3. (Idempotency): If ⌢Y=[κ,ς] for all h=1,2,3,...,n, then FFSSTOWG(Y,Y,Y,...,Y)=Y.
Theorem 4.5.4. (Commutativity): If (R′1,R′2,...,R′n) is any permutation of (R1,R2,...,Rn), then FFSSTOWG (R′1,R′2,...,R′n)=FFSSTOWG (R1,R2,...,Rn).
Theorem 4.5.5. (Boundedness): If Y−=min(L1,L2,...,Ln),Y+=max(L1,L2,...,Ln), then Y−⩽FFSSTOWG(L1,L2,...,Ln)⩽Y+.
4.6. FFSSTHWG operator
Definition 4.6.1. The gathering of FFNs are cj=[η,ϕ] and the weight vector is e=(e1,e2,...,en)T with ej∈[0,1] and n∑j=1ej=1. Then FFSST(c1,c2,...,cn)=n⊗j=1cejj
is said FFSSTHWG operator.
The proof of the following theorems is straightforward, and we omit it.
Theorem 4.6.2. The collection of FFNs are dj={[η,ϕ]} and the weight vector is e=(e1,e2,...,en)T with ej∈[0,1] and n∑j=1ej=1. Then it is held FFSSTHWG operator and
Theorem 4.6.3. (Idempotency): If ⌢Y=[κ,ς] for all h=1,2,3,...,n, then
FFSSTHWG(Y,Y,Y,...,Y)=Y.
Theorem 4.6.4. (Commutativity): If (d′1,d′2,...,d′n) is any permutation of (d1,d2,...,dn), then. FFSSTHWG(d′1,d′2,...,d′n)=FFSSTHWG(d1,d2,...,dn).
Theorem 4.6.5. (Boundedness): If Y−=min(d1,d2,...,dn),Y+=max(d1,d2,...,dn), then Y−⩽FFSSTHWG(d1,d2,...,dn)⩽Y+.
5.
FF-AHP for innovativeness procedure selection
In this section we discuss the challenges of creative service collecting for major tiers or fiscal organizations by utilising a novel combination method that joins AHP with SST using FF data. To evaluate the work and the individual aspects of the innovation, a system of hierarchical evaluation criteria will be set up, able to balance strong presentation with obvious risks. The AHP is then applied to the FF scenery to quickly address the DMs' queries regarding the pairwise assessment of the relevant criteria, and to categorise the reliable criterion weights of the innovativeness dealers. The SST scheme is then added to the FF scenario to raise the suppliers' overall quality ratings while seeing the DMs' risk distaste concerning innovation. The position effects will quickly assess both the criteria's impartial values as well as the DMs' individual risk assurance through the blockchain. As a result, the proposed FF-AHP-SST technique address more effectively the problem of innovativeness worker collecting by considering the material topographies of innovativeness as well as the logical and emotional performance of DMs. Further graphically stepwise algorithm of proposed aggregation operators has been represented in Figure 4.
5.1. Description of the innovativeness group problem
Innovativeness assortment, as a distinguishing MCDM problems, is done by gathering the decision mediums. Let A={A1,A2,...,An} signify sure dormant blockchain ability workers PS={PS1,PS2,...,PSn} denote weighty evaluative criteria transported by frequent specialists DM={DM1,DM2,...,DMn}, and the conforming vigorous of experts is signified by Δ=(Δ1,Δ2,...,Δn)T satisfying the situations n∑j=1Δj=1. Furthermore, the vigorous of the evaluative criteria is characterized by W=(W1,W2,⋯,Wn)T, consultation the ensuing environments: n∑j=1Wj=1. Each constituent in the decision medium means the appropriate aspirant's evaluative valuation on a persuaded principle from the DM.
5.2. MCDM system on FFNs
Case 1.
Step 1: Designate the FF decision matrix.
Step 2: Designate the FFSSTHWA operator and ξ=(ξ1,ξ2,...,ξn).
Step 3: Designate the FFSSTHWA operator and ξ=(ξ1,ξ2,...,ξn).
Step 4: Find the level.
Case 2.
Step 1: Describe the FF decision matrix.
Step 2: Describe the FFSSTHWG operator and ξ=(ξ1,ξ2,...,ξn).
Step 3: Describe the FFSSTHWG operator and ξ=(ξ1,ξ2,...,ξn).
Step 4: Find the rank.
5.3. FF-AHP case history
TE-FOOD is a community permissioned nourishment traceability system that enables all supply chain participants blockchain-based farm-to-table and customer trace of the food's data. The Food Chain (TE-FOOD's blockchain) is a community permissioned blockchain that lets supply chain performers and patrons uphold chief bulges to disperse traceability data. Customers of TE-FOOD use the flexibility to improve comprehensive perspectives into the stock restraint of food manufacture. TFD is symbolic of TE-ERC FOOD, which is classically abandoned on the wraithlike phase.
The situation, to provide visibility in the food stream shackle by guiding the products through the entire spring chain (farm, computer or abattoir, supplier, shop) and offering clients, supply chain businesses, and management activities with tools to study the history and perfection of food. The TE-FOOD aims to enhance customer confidence and communication, acquire better supply chain data to restore operating effectiveness, abide by spread guidelines, protect their products from counterfeiting, and carry out earlier invention memories.
The TE-Food system consists of diverse arenas:
Understanding tools it includes safety closures, tag stickers, 1D/2D barcodes, RFID ear stickers, and tag labels. Traceability management includes a mobile B2B (Business-to-Business) app, a web app, a dominating system, outer interfaces, and journalistic tools. Skill and client tools include B2C (Business-to-Consumer), new yield legacy mobile apps, web apps, and trade side nutrition ancient numerical signage tools. Solutions offered by national livestock organisations: It includes plans for managing and implementing cattle.
Gears for farm organisation: These tools are based on the category-specific (Inoculation, nourishing, composts, vegetable defence harvests, etc.). A Scam organisation arrangement, nourishment complaint device paraphernalia, and meat excellence graphic examination scheme are some of the equipment used in food care. The plan uses several documentation resources, including paper-based marker labels with QR codes, flexible terminations with QR codes, and malleable credentialing labels with RFID, to categorise the watched bodily fluids (crops, chairs, etc.)
It fulfils many customers' needs for traceability, including movable apps used by B2B and clients to access double documentation capitals and appeal/arrival statistics. For users who do not want to use a mobile app to see the creation history, there is a web app. Exposed border for supply chain businesses that now use practise software to wrest origination's information's-FOOD propositions dualistic implementation representations, distant or official, and IoT API for food industries that permit to organisation statistics conservative from the strategies.
TE-FOOD being focused on food production yields only solutions for the agricultural sector. Only the trackability solution provides multiple B2B (Business-to-Business), B2C (Business-to-Consumer), and B2A (Business-to-Authorities) capabilities, supporting businesses, customers, and institutions.
Customer trust is shaped since they can trace the origin of the food creation while accounting for all the distributions the creation received.
Good trackability and sensors allow for the early isolation of contaminated food production before it spreads to stores, thereby reducing the number of foodborne illnesses.
Controlling entities have realistic perspectives on the food industry that support the application of food care regulations.
One of the TE-FOOD restrictions is the TFD symbolising the relatively slow deal/second (15 TPS) rate of delicate net earnings. Additionally, TE-FOOD must demonstrate an unintentional and direct rivalry with other rival companies like Ambrosius, WABI, MOD, and WTC. Additionally, TE-FOOD has a significant customer base in Vietnam and Republic of Hungary, and it is important to establish contacts in the world-wide market.
5.4. Numerical application of FF SST technique
The operation and growth of innovativeness are devoted from data building in the data stage. Innovative information building points to recover the organization break even with the complete trade wrapped up and information expertise. Activities can recoup the competence of their operations and organization through information director, planning by automatic and brainy progressed equipment and computer program device and administrations. Further by establishing a work organize checking framework database on all sorts of information, organization plans, to come about the commitment of modern innovativeness organization. In public, it is fundamental to make an automation even for calculation as it is one most essential tool for any organisation in this modern era. In uncertainty, DMs must provide their evaluations for the innovativeness with devotion to set up a right direction; in this way, the estimation movement can be seen as an agent MADM risky. Let a corporation is scheduled to support its four companies to expand their automation structure equally. The corporation will request four experts to make material for assessment of the four companies. PSi(i=1,2,3,4) with reverence to four qualities GBIUi(i=1,2,3,4). As the availability of data and the feasibility of the review, four attributes are ultimately chosen: GBIU1(IT attention degree), GBIU2 (IT reserve attention degree), GBIU3 (IT influence degree), and GBIU4 (amount of speculation for automation). The weight vector of four experts is w=(0.2,0.3,0.4,0.1)T.
Attractive into clarifying the effort of real-world states and the suitability of data, the ultimate decision maker requires DMs to utilize Fermatean fuzzy basics to direct their appraisal data. So, the decision matrices measured by DMs are observable in Tables 1 and 2.
The FF decision matrix Table 2 is as below.
The assessment originates that the overweight of the four are wholly reported confidential after four cross units. By performing the four steps algorithm, the final ranking fallouts of FFSSTWA operators of Cases 1 and 2 has been denoted in Figures 5 and 6 individually.
Case 1.
Step 1: Define the FF decision matrix. The FF decision matrix Table 1 is as below.
Step 2: Describe the FFSSTWA operator and ξ=(0.23,0.24,0.25,0.28).
FFSSTWA operator Table 3.
Step 3: Designate the FFSSTWA operator and ξ=(0.23,0.24,0.25,0.28).
FFSSTWA operator Table 4.
Step 4: Determine the score values.
Case 2
Step 1: The FF decision matrix. The FF decision matrix Table 5 is as below.
FF decision matrix. The FF decision matrix Table 6 is as below.
Step 2: Designate the FFSSTWG operator and ξ=(0.23,0.24,0.25,0.28).
FFSSTWG operator Table 7.
Step 3: The FFSSTWG operator and ξ=(0.23,0.24,0.25,0.28).
FFSSTWG operator Table 8.
Step 4: Determine the score value.
6.
Comparison technique with existing method
Sketches are used to compare the optional plan to another plan that is inferior based on IFSs [1] and FFWG statistics [14] to support and conventionally optimistic feasibility. Other strategies are odd applications of our technique strategy, which was developed on FFN to the same description circumstance. Ranking result of the optimal solution of the existing technique has been represented in Figures 7 and 8.
6.1. IFN with existing technique
Step 1: IF decision matrix Table 9 in is as below.
Step 2: Describe the IFWA operator and the weight (0.1,0.2,0.3,0.4). In Table 10.
Step 3: The score value is
Step 4: Given the ranking Z4>Z3>Z2>Z1 and hence Z4is the best.
6.2. FF number with existing technique
Step 1: The FF decision matrix is given in Table 11.
Step 2: The FFWG operator and the weight vector is(0.1,0.2,0.3,0.4). FFWG operator Table 12.
Step 3: The score function is.
Step 4: Find the ranking Z4>Z3>Z2>Z1 and henceZ4is the best.
When there are interrelationships including any various criteria: The varied methods can hold this formal, see Table 13.
6.3. Merits of the proposed method
We highlighted some merits of the suggested approach, as follows.
1) The approach proposed in this paper is related with the group decision-making technique grounded on imitation and FFS proposed in (Senapati et al. 2019), and the ranking result by the mixture of imitation and FFS isη2>η4>η3>η1. It is observed that the best structure found by the process proposed in this study is the similar as that gained by the group decision-making system in (Senapati et al. 2019), that is η2, but the command of each structure is still changed. Since the risk favourites of dissimilar decision-makers are booked into explanation in the aggregation technique used in this paper. The consequence of indecision on decision-making consequences is counted from the viewpoint of decision-makers, so the decision-making consequence is more in streak with the real decision-making condition.
2) It is more sensible and applied to use the technique suggested in this paper to regulate the weight of decision-makers than the lengthy FFS technique used in (Senapati, T and Yager, R. R. (2020)). As The masses of decision-makers resolute through the FFs technique is the similar for dissimilar qualities, and folks using the method outlined in this paper are different for different qualities. In actual decision-making, the different qualities may include changed arenas, and correspondingly decision maker is clearly only decent at some fields but not altogether in all fields. It is more sensible that decision makers have diverse weights under different attributes. In adding, the existing study consequences use resemblance or nearness to control the weight of decision makers, but the resemblance is not essentially high underneath great closeness. Consequently, this paper interminably uses comparison and nearness in the technique of critical the weight of decision makers to enhanced variety filled procedure of decision data, so that the persevering decision creator commonalities are closer to the real weights.
3) The technique suggested in this paper has persistent distinguishability compared to the extended FFS technique in works (Senapati, T., and Yager, R. R. (2020)), which can help us to control the unique ideal explanation for various decision-makers' safety preferences. To the contrary, the extended FFS approach may produce close complete evaluation values for each scheme, weakening the distinguishability of this method and making it challenging to distinguish the schemes.
4) There is a little doubt that the two strategies' focal points and methods differ (Own C-M 2009). In contrast to the strategy suggested by the former, which generates the Fermatean fuzzy number and is used to the MADM problematic with solitary one decision maker, the approach offered in this study is appropriate to the MAGDM problem with FFN whose quality weight information is completely unknown. The prior way only needs the decision maker to assess the favourite relationship between schemes after pairwise judgement, as opposed to the current method, which analyses each scheme in relation to each attribute.
5) The same planned DM approach is used with a smaller MAGDM subject to instruct students in a way that produces the best results. The effective and true MAGDM method allows us to apply the same strategy to a minor unruly and obtain the same outcome as with the MAGDM challenging.
6) The complex alternative evaluation must be comparable to the undecomposed difficulty's unique assessment when the primary MAGDM issue is reduced to a minor issue. To strengthen the alternative, we employ techniques like those utilised in the MAGDM problem on small subjects.
7) The FFSSTWA operator must be satisfied to resonant a proficient and accurate MAGDM approach.
8) We can assume that the position leans are equal based on the outputs of the optional operators and previous laborious procedures. The MCDM method is a complete and innovative solution for commerce with indecision under DM conditions and is dependent on the Schweizer-Sklar aggregation operations. In practical situations, using Schweizer-Sklar norm-dependent aggregate operators in a different ranking value are more flexible and cost-effective.
9) Operations research's branch known as multiple-criteria decision-making openly assesses numerous, contradictory factors when making decisions (as well as in environments including business, government, and medical). Inconsistent criteria are commonly present while analysing possibilities: cost or price is typically one of the fundamental criteria, and a quality measure is frequently another, readily at conflict with the cost. Cost, comfort, safety, and fuel efficiency may be some of the primary factors we take into consideration when buying a car. It is unusual for the economical car to also be the greatest contented and safest one. The goal of portfolio management is to maximize returns while minimizing risks, yet the companies with the highest potential returns often have the most risk.
6.4. Comparing method with existing new methods
In this subsection, the proposed method compares with a different method.
1) This method is innovative and good assessment for Schweizer-Sklar t-norm and t-conorm operational comments for FFNS.
2) Further, the presented FFNS method founded on Schweizer-Sklar considers the concordance set beside the different set, but the existing FFS technique does not expressly consider the different set when two choices have the same performance.
3) The proposed aggregation operators are superior to the existing aggregation operators. As FFSSTWA is more informative and more flexible.
4) An extended MCGDM technique for FFNS heightened the sturdiness of the application by inspection the alternative superiority, impartiality and subservience dealings completed other changes, as well as the graphical explanation of weedy and robust outranking relatives that energy elsewhere the construction of preceding replicas.
5) This feature climaxes the superiority of the original FFNS practise completed the former classical.
Different existing techniques, see Table 14.
6.5. Effectiveness of the method
The anxiety is by what means to evaluate the former multi-criteria efficiency mark for a suitable decision scenery because the proposed FFN context, which is an effectiveness or scoring degree-based model for MCDA, selects choice with the maximum helpfulness degree, as compared to the existing replicas, which are concession degree replicas and select a selection that is contiguous to the ideal explanation, see Table 15.
6.6. Limitation
In this subsection limitation of FFs based on two sets has been exhibited (see Table 16).
7.
Conclusions
The primary aim of this research is to present a unique MADM technique and tie it to a real innovation in information and evaluation issues. To achieve this, we focused primarily on three components: The data face way, the data aggregation technique, and the operational rules in the data aggregation process. Initially, we proposed the concept of FFS, which is a practical and significant data presentation strategy for controlling uncertainty. The proposed FFS has the advantage of giving DMs more autonomy. To provide an additional flexibility to the circumstance, the Schweizer-Sklar t-norm and t-conorm operational defined for FFNS. A series of operators, with the combination FFNS and SSTT operational rules are presented, namely the FFSSTWA operator, FFSSTOWA operator, FFSSTHWA operator, FFSSTWG operator, FFSSTOWG operator, and FFSSTHWG operator, in control to hold the interrelationship during qualities. We worked with the SST operator to collectively assess data with FFNs. The interrelationship between different attributes might be considered using the provided operator. Then, an innovated method to MADM problems based on the suggested operators is pre-summit. The FFSSTWA and FFSSTWG operators are utilised to fix the challenge in the appropriate ways. To conclude, we coupled the method to an innovativeness automation equal appraisal tricky. To demonstrate that the offered operators provide a reducible approach to the MCDM problem, a comparison with several existing operators was made.
In the future, we will offer the proposed techniques to other FFN methods and aggregation operators, such as the Muirhead mean operator and presence worth exploring power average operator. Additionally, our suggested strategy can be related to additional issues including dealer collecting challenges, speculative topics.
Interdependency of the different sections of the paper has been exhibited in Figure 9.
Conflict of interest
The authors declare no conflict of interest









 DownLoad:
DownLoad: