Averaging methods for piecewise-smooth ordinary differential equations

1.   Introduction

Averaging method has many applications and it is well-developed for ordinary and partial differential equations ^[4,8], for impulsive and differential inclusions ^[7], and for more general types of differential equations as well ^[6,5]. Non-smooth dynamical systems also possess many applications ^[2]. The goal of this paper is to extend the tool set available for analyzing periodic piecewise-smooth differential equations by generalizing the averaging method. Several intrinsic problems surfaced while trying to generalize the results to the piecewise-smooth setting. We focus on the cases of transversal and sliding solutions of the piecewise-smooth systems. In these cases, we discuss the problems and try to shed some light on their nature and causes by considering concrete examples. Several conditions are introduced which allow us to prove results analogous to the averaging theorem for smooth systems.

Section 2 is a brief introduction to the theory of averaging for smooth systems and contains an averaging theorem whose statement serves as a prototype for statements of our generalizations for piecewise-smooth systems. Section 3 introduces piecewise-smooth systems and our main definitions. Section 4 provides examples that motivated us for these definitions. Section 5 presents some auxiliary results concerning the discontinuity boundary and its intersection with a solution that are used in the next sections. Sections 6 and 7 deal with the proofs of our main results. The final Section 8 summarizes our results with a possible outline for further study.

Throughout the paper, we shall denote $u\cdot v$ the inner product of vectors $u, v\in\mathbb{R}^n$ and $|u|$ the Euclidean norm of $u\in\mathbb{R}^n$. Furthermore, the distance of sets $A, B\subset\mathbb{R}^n$ is defined as ${\rm dist}(A, B) = \inf\{|a-b|\mid a\in A, \, b\in B\}$.

2.   Averaging for smooth systems

Let $\Omega \subset \mathbb{R}^n$ be a domain and $a \in \Omega$. We consider the initial value problem

defined for $x \in \Omega$, $\epsilon \in [0, 1]$ and $t \in \mathbb{R}$ where $f(x, t, \epsilon)$ is a continuously differentiable periodic function in the time variable $t$ with the period $T$.

Definition 2.1. Let $f$ be the right-hand side of initial value problem (2.1). Denote

The averaged system associated with (2.1) is the system

and the guiding system associated with (2.1) is the system

Initial value problem (2.1) gives naturally a rise to the initial value problem for the averaged system (2.2) with $z(0) = a$, and for the guiding system (2.3) with $w(0) = a$. The guiding system is only a rescaled averaged system.

The following theorem from [8,Theorem 2.8.1] is the key result we need and it will be used throughout the whole paper.

Theorem 2.2. Consider initial value problem (2.1). Suppose that $f$ is Lipschitz continuous and $w: [0, L] \to \Omega$ is solution of the associated guiding system. Then there exist positive constants $\epsilon_0$, $C$ such that for all $\epsilon \in (0, \epsilon_0]$ there exists a unique solution $x_{\epsilon}:[0, \frac{L}{\epsilon}] \to \Omega$ such that

for $0 \leq t \leq \frac{L}{\epsilon}$.

To simplify some of the statements in the rest of the paper, we collect some of the common assumptions on the right-hand side $f$ in the following definition.

Definition 2.3. We say, that a function $f: \Omega \times \mathbb{R} \times [0, 1] \to \mathbb{R}^n$ is $T$-PCDLB if it is $T$-periodic in the second argument, continuously differentiable, Lipschitz continuous and bounded.

The following lemma is an extension of the averaging theorem for smooth systems which will be useful in the next sections.

Lemma 2.4. Let $f$ be a $T$-PCDLB function, $w: [L_1, L_2] \to \Omega$ be a solution of the guiding system $\dot w = \bar f(w)$ with $w(L_1) = a$ and $A \geq 0$ be a constant. Let $\epsilon \in (0, 1]$. Consider the local solution of initial value problem $\dot x = \epsilon f(x, t, \epsilon)$, $x(\frac{L_1}{\epsilon}+A) = b_\epsilon$ for $b_\epsilon\in\Omega$ such that $| a - b_\epsilon | \leq D \epsilon$ for some constant $D > 0$.

Then there exist $\epsilon_0, C > 0$ and a unique solution $x_\epsilon: [\frac{L_1}{\epsilon}+A, \frac{L_2}{\epsilon}] \to \Omega$ of $\dot x = \epsilon f(x, t, \epsilon)$, $x(\frac{L_1}{\epsilon}+A) = b_\epsilon$ for all $\epsilon \in (0, \epsilon_0]$ such that for all $t \in [\frac{L_1}{\epsilon}+A, \frac{L_2}{\epsilon}]$ it holds

Proof. Let us at first assume the existence of $x_\epsilon$ for sufficiently small $\epsilon > 0$ and prove estimate (2.4). Let $A_\epsilon$ be such that $A_\epsilon \geq A$, $| A_\epsilon - A | \leq T$, $\frac{L_1}{\epsilon} + A_\epsilon = k_\epsilon T$ for some $k_\epsilon \in \mathbb{Z}$. The existence of such $A_\epsilon$ for all $\epsilon$ is obvious. Let $\epsilon_{0} > 0$ be so small that $\frac{L_1}{\epsilon}+A_\epsilon\leq \frac{L_2}{\epsilon}$ for all $\epsilon\in(0, \epsilon_0]$. Let $M$ be a bound of $|f|$.

First, we prove inequality (2.4) for $t \in [\frac{L_1}{\epsilon} +A, \frac{L_1}{\epsilon} +A_\epsilon]$. Using the assumption $| a-b_\epsilon | \leq D \epsilon$ and the Lipschitz continuity of solutions $x_{\epsilon}$ and $w$, we obtain

Next, we prove inequality (2.4) for $t \in [\frac{L_1}{\epsilon} +A_\epsilon, \frac{L_2}{\epsilon}]$. Since the solution $w$ is defined on interval $[L_1, L_2]$, we will use the averaging theorem for smooth systems for function $\widetilde w(\tau) = w(\tau + L_1)$. Hence there exists some $C > 0$ such that for all $\epsilon > 0$ sufficiently small, there is a solution $y_\epsilon:[0, \frac{L_2-L_1}{\epsilon}] \to \Omega$ of the initial value problem $\dot y = \epsilon f(y, t, \epsilon)$, $y(0) = a$, and for all $t \in [0, \frac{L_2-L_{1}}{\epsilon}]$ it holds

Using $y_\epsilon$ we can define a function $u_\epsilon:[\frac{L_1}{\epsilon}+A_\epsilon, \frac{L_2}{\epsilon}+A_\epsilon] \to \Omega$ by $u_\epsilon(t) = y_\epsilon(t-(\frac{L_1}{\epsilon}+A_\epsilon))$. The function $u_\epsilon$ satisfies the equation

since $\frac{L_1}{\epsilon} + A_\epsilon = k_\epsilon T$ and $f$ is $T$-periodic in the second argument.

Now, let $t \in [\frac{L_1}{\epsilon} +A_\epsilon, \frac{L_2}{\epsilon}]$. Using (2.5) we estimate

The functions $u_{\epsilon}$, $x_{\epsilon}$ satisfy the same equation (2.1) (however, with possibly different initial values), hence it holds

Using the Lipschitz continuity of $f$ and the Gronwall lemma, we obtain

for some constant $C > 0$. The estimates (2.4), (2.6) for $t = \frac{L_1}{\epsilon}+A_\epsilon$ imply

Thus the proof of the estimate (2.4) for $t \in [\frac{L_1}{\epsilon} +A_\epsilon, \frac{L_2}{\epsilon}]$ is finished.

Now we prove the existence and uniqueness. The uniqueness and the local existence of $x_\epsilon$ are consequences of the Lipschitz continuity. The only issue for global existence of $x_\epsilon$ would be if $x_\epsilon$ approaches the boundary $\partial \Omega$.

Denote $d = {\rm dist}(\partial \Omega, w([L_{1}, L_2]))$. Let $\epsilon_0 > 0$ be such small that $C \epsilon_{0} \leq \frac{d}{2}$. Then we have

for any $\epsilon \in(0, \epsilon_0]$ and $t \in [\frac{L_1}{\epsilon}+A, \frac{L_2}{\epsilon}]$. Consequently,

which means that ${\rm dist}(\partial \Omega, x_\epsilon(t)) \geq \frac{d}{2}$ for all $t \in [\frac{L_1}{\epsilon}+A, \frac{L_2}{\epsilon}]$. Hence, $x_\epsilon$ exists globally on interval $[\frac{L_1}{\epsilon}+A, \frac{L_2}{\epsilon}]$.

3.   Definition of a piecewise-smooth system

We start with a definition of a discontinuity boundary.

Definition 3.1. We say that a function $G: \Omega \to \mathbb{R}$ is a boundary function if $G$ is continuously differentiable and $\nabla G(x) \not = 0$ for every $x \in \Omega$ such that $G(x) = 0$.

For a boundary function $G$, let us define

The set $\Omega_0$ is a $C^1$-manifold and is called a discontinuity boundary or a discontinuity surface. The notation introduced above will be used throughout our paper.

Definition 3.2. Let $\Omega \subset \mathbb{R}^n$ be an open set, let $f^-, f^+: \Omega \times \mathbb{R} \times [0, 1] \to \mathbb{R}^n$ and $G: \Omega \to \mathbb{R}$ be functions. We say that the tuple $(\Omega, G, f^-, f^+)$ is a piecewise-smooth system if $f^-$ and $f^+$ are continuously differentiable and $G$ is a boundary function. We say that the tuple $(\Omega, G, f^-, f^+)$ is a well-behaved piecewise-smooth system if $f^-$ and $f^+$ are $T$-PCDLB and $G$ is a boundary function.

A shorthand $\dot x = f^{\pm}(x, t, \epsilon)$ will be often used instead of the tuple $(\Omega, G, f^-, f^+)$ while talking about a piecewise-smooth system, implicitly including a set $\Omega$ and a boundary function $G$.

Definition 3.3. An absolutely continuous function $x:[a, b] \to \Omega$ is called a solution of the piecewise-smooth system $(\Omega, G, f^-, f^+)$ if for almost all $t \in [a, b]$,

Furthermore, we say that a solution of the piecewise-smooth system is a solution of an initial value problem $\dot x = \epsilon f^{\pm}(x, t, \epsilon)$, $x(a) = x_a \in \Omega$ if $x(a) = x_a$.

The formalism that we just introduced is called the Filippov regularization of a piecewise-smooth system (see ^[1,2]).

Next we define two properties for which we will prove the averaging theorem.

Definition 3.4. We say that a well-behaved piecewise-smooth system $(\Omega, G, f^-, f^+)$ is uniformly transversal at the point $x_0 \in \Omega_0$ if there exists a positive constant $m$ such that

for all $t \in \mathbb{R}$ and $\epsilon \in [0, 1]$.

The uniform transversality at $x_0$ also implies that $\bar{f^\pm}(x_0) \cdot \nabla G(x_0) \geq m$.

Definition 3.5. We say that a well-behaved piecewise-smooth system $(\Omega, G, f^-, f^+)$ is uniformly sliding, if there exists a positive constant $m$ such that

for all $x \in \Omega_0$, $t \in \mathbb{R}$ and $\epsilon \in [0, 1]$.

The condition of uniform sliding itself is not sufficient to prove an averaging theorem. We need to reformulate the guiding system in a following way. Suppose that the condition of uniform sliding is true for every $x \in \Omega_0$. This implies, that any solution sliding at $t_0$ has to stay on $\Omega_0$ up to some $t_1 > t_0$. Hence the derivative of the solution is tangent to the manifold $\Omega_0$ or, equivalently, it is orthogonal to $\nabla G(x)$. A short calculation shows that for a given $(x, t, \epsilon)$ such that the piecewise smooth system is sliding at $(x, t, \epsilon)$, there is a unique element of ${\rm conv}(\{f^-(x, t, \epsilon), f^+(x, t, \epsilon) \})$, which is orthogonal to $\nabla G(x)$. We define function $g: \Omega_0 \times \mathbb{R} \times [0, 1] \to \mathbb{R}^n$,

where

The function $g$ can be used to define an induced differential equation on some neighborhood of $(x, t, \epsilon)$ in the set $\Omega_0 \times \mathbb{R} \times [0, 1]$. The smoothness of $g$ depends on the smoothness of $f^-$, $f^+$ and $\nabla G$; see ^[1] for details. We can apply averaging to this function to get

which serves as a right-hand side of the guiding system $\dot u = \bar g(u)$ for the equation induced on the discontinuity surface.

Definition 3.6. Let $(\Omega, G, f^-, f^+)$ be a well-behaved piecewise-smooth system that is uniformly sliding. Let $\bar g$ be defined as in (3.3). The sliding adjusted guiding system associated with the initial value problem $\dot x = \epsilon f^{\pm}(x, t, \epsilon)$, $x(0) = a \in \Omega_-$ is given by

An absolutely continuous function $w: [0, L] \to \Omega$ is a solution of the sliding adjusted guiding system, if it satisfies the above equality almost everywhere.

Suppose that we are given an initial value problem for a piecewise-smooth system $(\Omega, G, \epsilon f^-, \epsilon f^+)$,

such that both $f^-$ and $f^+$ are $T$-periodic. Our goal is to generalize the averaging theorem, Theorem 2.2, to this setting. Akin to Definition 2.1, we can define

and also define a guiding system associated with (3.4) as

Given a solution $w:[0, T] \to \Omega$ of the guiding system (3.5), it is clear that if $({\rm Im}~ w) \cap \Omega_0 = \emptyset$, we can use the result for smooth systems by restricting ourselves to a neighborhood of ${\rm Im}~ w$ that entirely lies inside $\Omega_-$ or inside $\Omega_+$.

That is the reason why we will be concerned only with solutions of the guiding system (3.5), which intersect $\Omega_0$. Furthermore, we will assume that $w(0) = a \in \Omega_-$. It is easy to see that if $a \in \Omega_+$, it suffices to exchange $G$ for $-G$ and all the results hold. We are not going to explicitly consider $a \in \Omega_0$, but such an initial condition is a special case that can usually be easily inferred from presented results.

4.   Some useful examples

Let us start with an example, which shows that we cannot simply adapt the averaging theorem by adding the word `piecewise' to the statement. More specifically, we construct a well-behaved piecewise-smooth system and a transversal solution of the associated initial value problem and we show that the result of the averaging theorem does not hold.

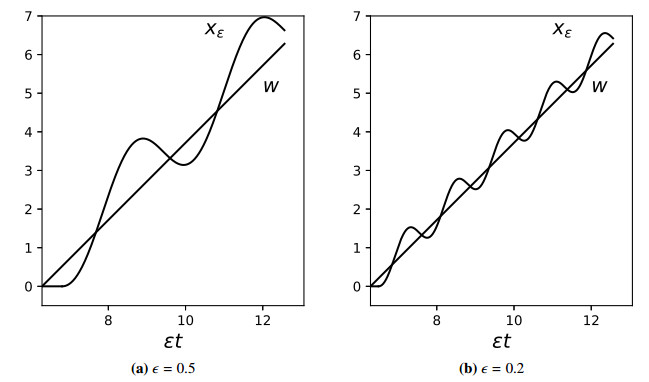
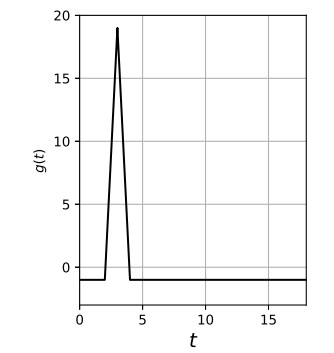
Example 4.1. Let us define $g$ as a periodic function with period $18$, which is defined on the interval $[0, 18]$ by the following formula (see Figure 1):

We can calculate that

We will use the standard mollifier as defined in [3,p.629],

For each $\delta > 0$, we set

and we define

By [3,p.630,Theorem 6(ⅰ)], $g^{\delta} \in C^{\infty} (\mathbb{R})$. Let $0 < \delta < 2$ be fixed such that

and $\int^{18}_0 g^{\delta} (t) \, \mathrm{d} t > 0,$ which can be done due to [3,p.630,Theorem 6(ⅲ)]. We can immediately see that

We will show that $g^{\delta}$ has exactly two zeros in $[0, 18]$. The derivative of $g^\delta$ is

where $h$ is the periodic continuation of

This implies that $(g^{\delta})^{\prime}(t) > 0$ for $t \in (2-\delta, 3)$ and $(g^{\delta})^{\prime}(t) < 0$ for $t \in (3, 4+\delta)$. Hence, by (4.1), $g^\delta$ has on $[0, 18]$ just two zeros: $r_1 \in (2, 3)$, $r_2 \in (3, 4)$.

Consider $\dot x = \epsilon f^{\pm} (x, t)$, $x \in \mathbb{R}$, $t \in \mathbb{R}$ with a boundary function $G(x) = x$ and

Such a system is well-behaved. The corresponding averaged version of these functions is

and the guiding system is $\dot w = I$. Consider the initial value problem $w(0) = -C$ whose solution is $w: [0, L] \to \mathbb{R}$, $w(t) = -C +It$ where $C \in \mathbb{R}^+$ and $L > \frac{C}{I}$.

We will show that although the averaged system has a transversal solution for all choices of $C$ and $L$ satisfying the conditions above, the original system has no solution that begins in $\mathbb{R}^-$ and then crosses over to $\mathbb{R}^+$.

Suppose that $x_\epsilon(t)$ is such a solution, meaning that $x_\epsilon(0) < 0$ and there is a $t^*$ such that $x_\epsilon(t^*) = 0$ (that is, it intersects the boundary $\Omega_0 = \{0\}$).

Let $t_0 = \sup \{ t \mid \forall s\in[0, t): x_\epsilon(s) < 0 \}$. The definition of $t_0$ and existence of $t^*$ imply that $x_\epsilon(t_0) = 0$. Since for all $s < t_0$ we have $x_\epsilon(s) < 0$, for each $m \in \mathbb{N}$ there is $t_m \in (t_0-\frac{1}{m}, t_0)$ such that $\epsilon g^{\delta}(t_m) = \dot x_\epsilon(t_m) > 0$. This implies that $t_0 \in [r_1+18l, r_2+18l]$ for some $l \in \mathbb{Z}$. Consequently, for all $t \in [t_0, r_2+18l]$ it holds that $\epsilon f^-(t) \geq 0$, $\epsilon f^+(t) < 0$ implying that $x_\epsilon(t) = 0$.

For all $t \in (r_2+18l, (18-r_2)+18l)$ it holds that $\epsilon f^-(t) < 0$, $\epsilon f^+(t) < 0$ which means that $\dot x_\epsilon(t) < 0$. Thus, $x_\epsilon(t) < 0$ and also $x_\epsilon((18-r_2)+18l) < 0$.

For all $t \in [(18-r_2)+18l, r_1+18(l+1)]$, $\epsilon f^-(t) < 0$ which implies

Since

there is $t_1 \in (r_1+18(l+1), r_2+18(l+1))$ such that $x_\epsilon(t_1) = 0$ and $t_1 = \sup \{ t \mid \forall s\in (r_2+18l, t): x_\epsilon(s) < 0 \}$.

The same analysis as for $t_0$ can be repeated for $t_1$, etc. The solution can be extended up to infinity, but does not cross the boundary. However, the solution of the guiding system crosses the boundary without a problem. The problem is that the guiding system does not reflect the behavior of the original equation at the discontinuity boundary.

Now, we provide an example of an initial value problem for a well-behaved piecewise-smooth system. The solution of the associated guiding system is transversal, while there is a solution of the original system for which the estimate from the averaging theorem holds and this solution is not transversal.

Example 4.2. Let $G(x) = x$ be the boundary function and consider a system $\dot x = \epsilon f^{\pm} (x, t)$, $x \in \mathbb{R}$, $t \in \mathbb{R}$ with

and an initial condition $x(0) = -2 \pi$.

The guiding system is $\dot w = 1$, which is transversal at $x = 0$ and we can immediately write the solution of the guiding system as

which means that

The solution of the original system up to the intersection with the discontinuity, that is for $t \in [0, \frac{2 \pi}{\epsilon}]$, is given by

The solution $x_\epsilon$ can cross $\Omega_0$ transversally at $t = \frac{2 \pi}{\epsilon}$ only if $f^+(0, \frac{2 \pi}{\epsilon}) > 0$. That implies

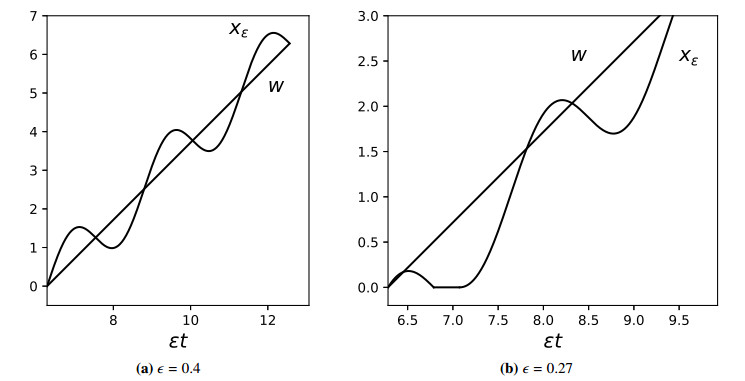
Let $\epsilon_k > 0$ be such that $\frac{1}{\epsilon_k} \in (-\frac{1}{6} + k, \frac{1}{6} + k)$ for some $k\in\mathbb{Z}$ (see Figure 2). For such $\epsilon_k$, the solution $x_{\epsilon_k}$ makes the first contact with the boundary at $t = \frac{2 \pi}{\epsilon_k} \in (-\frac{\pi}{3}+2k \pi, \frac{\pi}{3}+2k\pi)$. However, since $f^+(t) \leq 0$ up to $\frac{\pi}{3}+2k\pi$, we can say that the solution "sticks" to $x = 0$ up to $t = \frac{\pi}{3} +2k \pi$.

By solving the system $\dot x = {\epsilon_k} f^+(x, t)$, $x(\frac{\pi}{3}+2 k \pi) = 0$ we obtain a continuation of the solution $x_{\epsilon_k}$, namely

where $C_k = 2 \epsilon_k \sin (\frac{\pi}{3}+2 k \pi) - \epsilon_k (\frac{\pi}{3}+2 k \pi)$.

Such a solution does not make a contact with the boundary again. To see that, it only suffices to look at the values of $t-2 \sin t - \frac{\pi}{3} + 2 \sin(\frac{\pi}{3})$ for $t > \frac{\pi}{3}$.

Now, we show that condition (2.4) is satisfied. For $t \in [\frac{2\pi}{\epsilon_k}, 2k\pi+\frac{\pi}{3}]$,

For $t > 2k\pi+\frac{\pi}{3}$,

because

The analysis for other values of $\epsilon$ is a bit more tricky. The solution crosses $\Omega_0$ transversally, but it can make a contact with the boundary again. If it makes the second contact with the boundary, it will continue with the same motion as we just analyzed (see Figure 3).

However, the difference in values of $w_\epsilon$ and $x_\epsilon$ will be less than $C |\epsilon|$ for some $C > 0$. That means that the averaging estimate still holds on an interval $[0, T]$ for any $T$.

Note that the condition that the solution of the guiding system $w$ is transversal translates to a condition that the system $\dot w = \bar f^{\pm}(w)$ is transversal at $x_0$. However, that does not imply that the original system $\dot x = \epsilon f(x, t, \epsilon)$ is transversal at $x_0$ for all $t \in \mathbb{R}, \epsilon \in [0, 1]$. This means that the behavior of the original system near $x_0 \in \Omega_0$ is not reflected by the guiding system and can be more complex. For this reason, we introduce the notion of uniform transversality of the system $\dot x = \epsilon f(x, t, \epsilon)$ at $x_0$ (uniform in $t$ and $\epsilon$).

Now consider the guiding system $\dot w = \bar f^{\pm}(w)$ associated with $\dot x = \epsilon f^{\pm}(x, t, \epsilon)$. The right-hand side of the differential equation induced by this guiding system on the discontinuity boundary is given by

where $\beta$ is given by

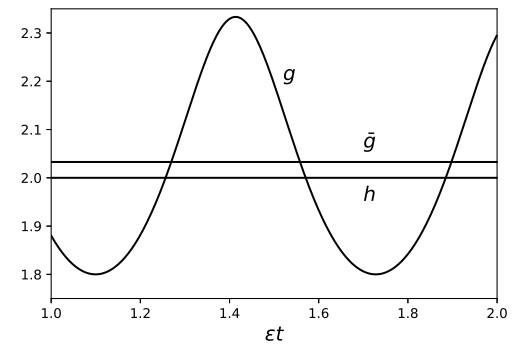
The sliding solution of the guiding system $\dot w = \bar f^{\pm}(w)$ is governed by $h$, but $\bar g$ of (3.1) is the true right-hand side of the guiding system for the sliding motion on $\Omega_0$.

A question that naturally arise is: What is the relationship between $\bar g$ and $h$? We could hope that these two functions are equal. However, this is not the case in general. The function $\bar g$ is a result of first inducing an equation on $\Omega_0$ and then averaging. For $h$, those operations are reversed. That is, we first average and induce an equation on $\Omega_0$ afterwards. Their relationship is not that simple.

The following example shows a specific system, where $\bar g$ and $h$ are not equal.

Example 4.3. Let the discontinuity surface be defined by $G: \mathbb{R}^2 \to \mathbb{R}$, $G(x) = x_1$ and consider the system $\dot x = \epsilon f^{\pm} (x, t)$, $x \in \mathbb{R}^2$, $t \in \mathbb{R}$ with

along with the initial condition $x(0) = (-1, -1)$.

The differential equation induced on $\Omega_0 = \{0\}\times \mathbb{R}$ is $\dot{x} = \epsilon g(x, t)$, where $g$ depends only on $t$, and is given by

The function $\alpha$ is defined by the equality

By solving for $\alpha$ and substituting into the previous equality, we get

By averaging over the period, we obtain

The guiding system for this example is given by the right-hand side

which induces a differential equation on $\Omega_0$, $\dot{x} = \epsilon h(x)$, where

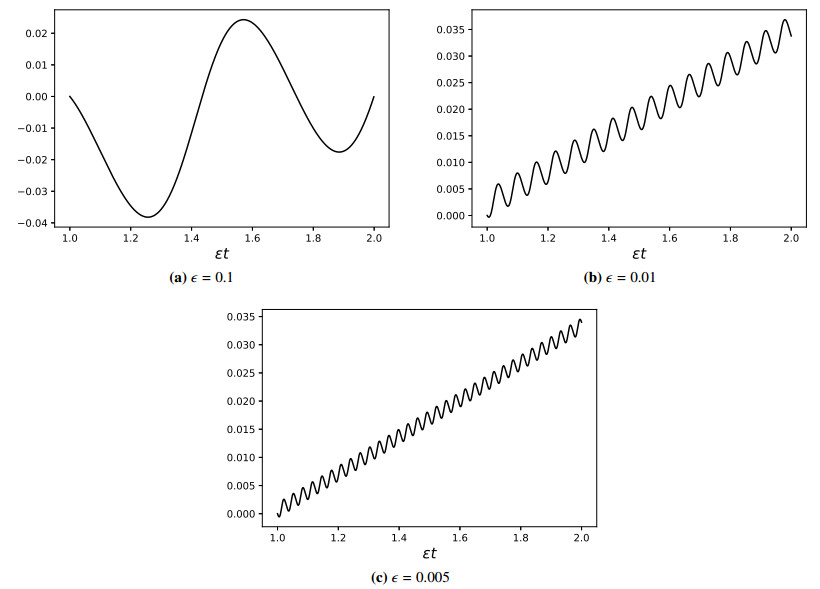
Let us compare the solution of the original initial value problem $x_\epsilon$ and $w_\epsilon$ obtained from the guiding system for $\tau \in [0, 2]$ (that is, $t \in [0, \frac{2}{\epsilon}]$).

For $t \in [0, \frac{1}{\epsilon}]$ we have

and the solutions make contact with the boundary at $t = \frac{1}{\epsilon}$ in $x = (0, 0)$. After that time, both solutions slide along the discontinuity boundary $\Omega_0$. The motion of $x_\epsilon$ is given by the differential equation $\dot x_\epsilon = \epsilon g(x_\epsilon, t)$, while the motion of $w_\epsilon$ is given by $\dot w_\epsilon = \epsilon h(w_\epsilon) = \epsilon(0, 2)$ (see Figure 4).

To analyze the behavior of $x_\epsilon$ on the boundary for sufficiently small $\epsilon$, we can employ the averaging theorem for smooth systems. In the following, let us drop the first coordinate (which is constant and equal to $0$).

The guiding system for the equation on the boundary has the right-hand side equal to $\bar g_0$. Using Lemma 2.4 (an extension of the averaging theorem), there is $\epsilon_0 > 0$, $C > 0$ such that for all $\epsilon \in (0, \epsilon_0]$ and $t \in [\frac{1}{\epsilon}, \frac{2}{\epsilon}]$,

In particular, at $t = \frac{2}{\epsilon}$ we have

On the other side,

which means that it cannot be true that there exists a constant $\widetilde C > 0$ such that for all $t \in [\frac{1}{\epsilon}, \frac{2}{\epsilon}]$ and all $\epsilon > 0$ sufficiently small,

Indeed, otherwise one would get a contradiction from

(see Figure 5).

5.   Analysis of the discontinuity boundary $\Omega_0$

For the purpose of this section, we denote $B_r(a) = \{x\in\mathbb{R}^n\mid |x-a| < r\}$. The next theorem shows that the function $G$ can be thought of as a distance function on some neighborhood of a point $x_0 \in \Omega_0$.

Theorem 5.1. Let $\Omega \subset \mathbb{R}^n$ be an open set and $G: \Omega \to \mathbb{R}$ a continuously differentiable function. Suppose that $x_0 \in \Omega_0 = \{ x \in \Omega\mid G(x) = 0 \}$ and that $\nabla G(x_0) \neq 0$. Then there exist positive numbers $d, \alpha, \beta \in \mathbb{R}$, such that $\overline{B_d(x_0)} \subset \Omega$, and for each $x \in \overline{B_d(x_0)}$,

Proof. Since the functions $(x, y) \mapsto \nabla G(x) \cdot \nabla G(y)$ defined on $\Omega \times \Omega$ and $x \mapsto | \nabla G(x) |$ defined on $\Omega$ are continuous and $\nabla G(x_0) \cdot \nabla G(x_0) > 0$, $| \nabla G(x_0) | > 0$, there are positive constants $d$, $a$, $A$, $b$, $B$ such that $\overline{B_{2d}(x_0)} \subset \Omega$ and for all $x, y \in \overline{B_{2d}(x_0)}$,

Suppose that $x \in \overline{B_d(x_0)}$ and $z \in \Omega_0$ is such that $| x-z | = {\rm dist}(x, \Omega_0)$. Since $x_0\in\Omega_0$ and $| x- x_0 | \leq d$, it follows that $| x-z | \leq d$. Hence $| x_0 - z | \leq 2d$, i.e., $z \in \overline{B_{2d}(x_0)}$. Consequently, $\nabla G(z) \neq 0$. It follows from the geometry that $x-z = k \nabla G(z)$ where $k = {\rm sgn}~G(x) \, \frac{| x-z |}{| \nabla G(z) |} = {\rm sgn}~G(x)\, \frac{{\rm dist}(x, \Omega_0)}{| \nabla G(z) |}$. Hence, it holds

Using the inequalities (5.1), we arrive at

and the statement easily follows.

In the next lemma, we consider a system with $C^1$-smooth right-hand side but included in $\Omega = \Omega_+\cup\Omega_0\cup\Omega_-$. So, we can estimate the time and position of the contact with $\Omega_0$.

Lemma 5.2. Let $f$ be $T$-PCDLB and $G$ be a boundary function. Let $w:[0, L] \to \Omega$ be a solution of the initial value problem $\dot w = \bar f(w)$, $w(0) = a \in \Omega_-$ and $x_\epsilon :[0, \frac{L}{\epsilon}] \to \Omega$ be a solution to $\dot x = \epsilon f(x, t, \epsilon)$, $x(0) = a$. Assume that $w$ intersects $\Omega_0$ in exactly one point $x_0$ at $\tau_0 \in (0, L)$ and $\bar f(x_0) \cdot \nabla G(x_0) > 0$.

Then there exist positive constants $\epsilon_0, A, B$ such that for all $\epsilon \in (0, \epsilon_0]$, the solution $x_\epsilon$ intersects $\Omega_0$ in some point and if we define $t_\epsilon$ to be the smallest $t$ such that $x_\epsilon(t) \in \Omega_0$, then

Proof. Let $M$ be a bound of $|f|$. Due to the smooth averaging, Lemma 2.4 can be used to show that there exist $C > 0$ and $\epsilon_0 > 0$ sufficiently small such that for all $\epsilon \in (0, \epsilon_0]$ and $t \in [0, \frac{L}{\epsilon}]$, it holds

In the rest of the proof, $\epsilon_0$ may decrease.

Since $w(L)\in\Omega_+$, we have $G(w(L)) > 0$. Then the estimate (5.2) and the continuity of $G$ imply that $G(x_\epsilon(\frac{L}{\epsilon})) > 0$ for all $\epsilon \in (0, \epsilon_0]$. Since $G(x_\epsilon(0)) = G(a) < 0$, the solution $x_\epsilon$ crosses $\Omega_0$ in some point.

Due to the continuity of mapping $x \mapsto \bar f(x) \cdot \nabla G(x)$ and Theorem 5.1, there exist positive constants $d$, $m$, $\alpha$, $\beta$ such that $\overline{B_d(x_0)} \subset \Omega$, and for all $x \in \overline{B_d(x_0)}$, it holds

Let $A$ be a constant such that $A > \frac{C}{m \alpha}$ where $C$ is from (5.2). Let $\epsilon_0$ be such that $\tau_0 -\epsilon_0 A > 0$, $\tau_0 +\epsilon_0 A < L$ and $MA \epsilon_0 \leq d$. If we choose $\epsilon\in(0, \epsilon_0]$, then for all $\tau \in [\tau_0 - A \epsilon, \tau_0 + A \epsilon]\subset [0, L]$, it holds

i.e., $w(\tau) \in \overline{B_d(x_0)}$. Since $w(\tau_0 + A \epsilon)\in\Omega_+$, we have $G(w(\tau_0 + A\epsilon)) > 0$ and we obtain

due to the first inequality of (5.3). Now, we can use the other inequality of (5.3) to get

Observe that the set $w([\tau_0+A \epsilon_{0}, L])$ is compact and lies in $\Omega_+$. Therefore, ${\rm dist}(w([\tau_0+A \epsilon_{0}, L], \Omega_0) > 0$. Consequently, for some $\epsilon_0 > 0$ and all $\epsilon \in (0, \epsilon_0]$, we obtain

The estimate (5.2) implies that for any $\epsilon\in(0, \epsilon_0]$,

Due to our choice of $A$, we deduce that $x_\epsilon(t) \in \Omega_+$ for all $t \in [\frac{\tau_0 + A\epsilon}{\epsilon}, \frac{L}{\epsilon}]$, $\epsilon\in(0, \epsilon_0]$.

Using similar arguments, we can show that

for all $\epsilon\in(0, \epsilon_0]$ for some (possibly smaller than before) $\epsilon_0 > 0$. Hence, there exists $t_\epsilon \in (\frac{\tau_0 - A\epsilon}{\epsilon}, \frac{\tau_0 + A\epsilon}{\epsilon})$ such that $x_{\epsilon}(t_{\epsilon})\in\Omega_0$. Furthermore, the following estimate is valid:

6.   Uniformly transversal systems

In this section, we prove an averaging theorem for uniformly transversal systems. First we prove an auxiliary result.

Lemma 6.1. Let $(\Omega, G, f^-, f^+)$ be a well-behaved piecewise-smooth system that is uniformly transversal at $x_0 \in \Omega_0$. Let $w : [0, L] \to \Omega$ be a solution of the guiding system $\dot w = \bar{f^{\pm}}(w), w(0) = a \in \Omega_-$ which intersects the boundary $\Omega_0$ in exactly one point $w(\tau_0) = x_0$ at $\tau_0 \in (0, L)$.

Suppose that there is $\epsilon_1 \in (0, 1]$ such that for all $\epsilon \in (0, \epsilon_1]$ there is a unique solution $x_{\epsilon} : [0, \frac{L}{\epsilon}] \to \Omega$ of the problem $\dot x_{\epsilon} = \epsilon f^{\pm}(x_{\epsilon}, t, \epsilon)$, $x_{\epsilon}(0) = a$ intersecting the boundary $\Omega_0$ at exactly one point in time.

Then there are $\epsilon_0 \in (0, \epsilon_1]$ and $C > 0$ such that for all $\epsilon \in (0, \epsilon_0]$ and all $t \in [0, \frac{L}{\epsilon}]$,

Proof. We know that $w$ is a solution of $\dot w(\tau) = \bar f^-(w(\tau))$ for $\tau \in [0, \tau_0]$, satisfying $w(0) = a$. Since the right-hand side $\bar f^-$ is defined on the whole $\Omega$, there is a solution $w^-:[0, L^-] \to \Omega$ of the same problem for some $L^- > \tau_0$. This solution is equal to $w$ on $[0, \tau_0]$. The uniform transversality at $x_0$ implies that $\dot w^-(\tau_{0}) \neq 0$. Hence the solution $w^-$ intersects $\Omega_0$ exactly once if $L^{-}$ is sufficiently close to $\tau_0$.

In what follows, the constants $\epsilon_0$ and $C$ may vary from step to step. By Theorem 2.2, for every $\epsilon\in(0, \epsilon_0]$, there is a unique solution $x^-_\epsilon: [0, \frac{L^-}{\epsilon}] \to \Omega$ of the initial value problem $\dot x = \epsilon f^-(x, t, \epsilon)$, $x(0) = a$ such that

for all $t \in [0, \frac{L^-}{\epsilon}]$. The assumption of uniform transversality at $x_0$ and Lemma 5.2 imply that there are positive constants $A$, $B$ such that for all $\epsilon \in (0, \epsilon_1]$, the solution $x^-_\epsilon$ intersects $\Omega_0$ and it holds

where $t_\epsilon$ is the earliest time of intersection. Our assumption on $f^-$ implies that $t_{\epsilon}$ is the time of the only intersection. Clearly, the solution $x^-_\epsilon$ is equal to $x_\epsilon$ on $[0, t_{\epsilon}]$ and $x_\epsilon^{-}(t)\in \Omega_+$ for $t\in(t_{\epsilon}, L]$.

Since the functions $f^\pm$ are bounded, the solutions $x_{\epsilon}$, $w$ are Lipschitz continuous. For $t \in [\frac{\tau_0}{\epsilon}-A, \frac{\tau_0}{\epsilon}+A]$, we estimate

Since $x^-_\epsilon(\frac{\tau_0}{\epsilon}-A) = x_\epsilon(\frac{\tau_0}{\epsilon}-A)$ and $w^-(\tau_0-\epsilon A) = w(\tau_0-\epsilon A)$, using Lipschitz continuity of $x_\epsilon$, $w$ and inequality (6.2), we arrive at (6.1) for $t\in[\frac{\tau_0}{\epsilon}-A, \frac{\tau_0}{\epsilon}+A]$.

Now, let $t \in [\frac{\tau_0}{\epsilon}+A, \frac{L}{\epsilon}]$. For all such $t$ we have that $w(t), x_\epsilon(t) \in \Omega_+$. Hence $x_{\epsilon}$ is a solution of equation $\dot x = \epsilon f^+(x, t, \epsilon)$ and $w$ is a solution of the corresponding guiding system. Lemma 2.4 with $L_1 = \tau_0$, $a = w(\tau_0)$ and $b_\epsilon = x_\epsilon(\frac{\tau_0}{\epsilon}+A)$ implies the existence of $\epsilon_0, C > 0$ such that for all $\epsilon \in (0, \epsilon_0]$, it holds

for all $t \in [\frac{\tau_0}{\epsilon}+A, \frac{L}{\epsilon}]$. This estimate concludes the proof.

Theorem 6.2. Let $(\Omega, G, f^-, f^+)$ be a well-behaved piecewise-smooth system that is uniformly transversal at $x_0 \in \Omega_0$. Let $w : [0, L] \to \Omega$ be a solution of the guiding system $\dot w = \bar{f^{\pm}}(w)$, $w(0) = a \in \Omega_-$ which intersects the boundary $\Omega_0$ in exactly one point $w(\tau_0) = x_0$ at time $\tau_0 \in (0, L)$.

Then there are $\epsilon_0 > 0$ and $C > 0$ such that for all $\epsilon \in (0, \epsilon_0]$ there exists a unique solution $x_\epsilon$ of the problem $\dot x_\epsilon = \epsilon f^{\pm}(x, t, \epsilon)$, $x_\epsilon(0) = a$, intersecting $\Omega_0$ exactly once, the intersection is transversal, and for all $t \in [0, \frac{L}{\epsilon}]$, it holds

Proof. The main idea of the proof is to use the averaging theorem, Theorem 2.2, for smooth systems in $\Omega_-$ and $\Omega_+$ separately and to construct a solution of the piecewise-smooth system $\dot x = \epsilon f^{\pm}(x, t, \epsilon)$ that crosses $\Omega_0$ only once.

The proof is divided into two parts. Lemma 6.1 shows that, given a transversal solution of $\dot x = \epsilon f^{\pm}(x, t, \epsilon)$, the averaging estimate (6.4) holds for all sufficiently small $\epsilon > 0$.

Now, it only remains to show that there exists a unique transversal solution for all sufficiently small $\epsilon$. The uniform transversality at $x_0$ implies the existence of $d, m > 0$ such that $\overline{B_{2d}(x_0)} \subset \Omega$ and

for all $x \in \overline{B_{2d}(x_0)}$, $t \in \mathbb{R}$, $\epsilon \in [0, 1]$.

Let $M$ be a bound of both $| f^+ |$ and $| f^- |$. Analogously to the proof of Lemma 6.1, there is $L^- > \tau_0$ and a solution $w^-:[0, L^-] \to \Omega$ of $\dot w = \bar f^-(w)$, $w(0) = a$ that intersects $\Omega_0$ exactly once and $w^-(\tau) = w(\tau)$ for $\tau \in [0, \tau_0]$. Due to the averaging theorem for smooth systems, there is $\epsilon_1 \in (0, 1]$ and $C > 0$ such that for any $\epsilon \in (0, \epsilon_0]$, there is a unique solution $x^-_\epsilon: [0, \frac{L^-}{\epsilon}] \to \Omega$ of the initial value problem $\dot x = \epsilon f^-(x, t, \epsilon)$, $x(0) = a$ such that it holds

for all $t \in [0, \frac{L^-}{\epsilon}]$. In the following again, the constants $\epsilon_0$ and $C$ may vary from step to step. By Lemma 5.2, there are constants $A$, $B$ such that for all $\epsilon \in (0, \epsilon_0]$ the solution $x_\epsilon^-$ intersects $\Omega_0$ and it holds

where $t_\epsilon$ is the time of the first contact. Let $\epsilon_0$ be so small that the following inequalities are true

The first inequality of (6.5) implies

for all $\epsilon \in (0, \epsilon_0]$.

Now, let $\epsilon \in (0, \epsilon_0]$ be fixed. Denote $x^+_\epsilon(t): [t_\epsilon, s] \to \Omega$ the unique local solution of the initial value problem $\dot x = \epsilon f^+(x, t, \epsilon)$, $x(t_\epsilon) = x^-(t_\epsilon)$ for some $s > t_{\epsilon}$. This solution can be extended until it approaches $\partial \Omega$. For $t \in [t_\epsilon, s]$, we estimate

Denote $\Delta = \min\{ \frac{d}{2M}, L- \tau_0 \}$. From the third inequality of (6.5) we deduce that

Assume that $s \leq \frac{\tau_0+\Delta}{\epsilon}$. The inequality $t_\epsilon \geq \frac{\tau_0-\epsilon A}{\epsilon}$ together with (6.5) imply

Combining this estimate with (6.6), we get

for any $t \in [t_\epsilon, s]$. This means that the solution $x^+_\epsilon$ is separated from the boundary $\partial\Omega$ on $[t_\epsilon, s]$ for arbitrary $s \leq \frac{\tau_0+\Delta}{\epsilon}$. Hence, it exists on $[t_\epsilon, \frac{\tau_0+\Delta}{\epsilon}]$. Moreover, since $x^+_\epsilon(t) \in \overline{B_{2d}(x_0)}$ for all $t \in [t_\epsilon, \frac{\tau_0+\Delta}{\epsilon}]$, it holds

for all $t\in[t_\epsilon, \frac{\tau_0+\Delta}{\epsilon}]$, and so $x^+_\epsilon(t) \in \Omega_+$.

For $\epsilon \in [0, \epsilon_0]$, define $x_\epsilon: [0, \frac{\tau_0+\Delta}{\epsilon}] \to \Omega$ as follows:

This is a solution of the initial value problem $\dot x = f^\pm(x, t, \epsilon)$, $x(0) = a$, which intersects $\Omega_0$ exactly once and the intersection is transversal. Lemma 6.1 implies validity of estimate (6.4) for $\epsilon \in (0, \epsilon_0]$ and $t \in [0, \frac{\tau_0+\Delta}{\epsilon}]$.

If $\tau_0 + \Delta = L$ the proof is done. Thus assume that $\tau_0 + \Delta < L$. Let

Since $\dot w(\tau) = \bar f^+(w(\tau))$ for $\tau \in (\tau_0, L)$, we can use Lemma 2.4 with $L_1 = \tau_0 + \Delta$, $a = w(L_1)$, $b_\epsilon = x_\epsilon(\frac{\tau_0+\Delta}{\epsilon})$ and $A = 0$. Hence, there is a unique solution $x^{++}_\epsilon: [\frac{\tau_0+\Delta}{\epsilon}, \frac{L}{\epsilon}] \to \Omega$ such that

for all $t \in [\frac{\tau_0+\Delta}{\epsilon}, \frac{L}{\epsilon}]$ and $\epsilon \in (0, \epsilon_0]$. Observe that $x^{++}_\epsilon(t) \in \Omega_+$ for all $t \in [\frac{\tau_0+\Delta}{\epsilon}, \frac{L}{\epsilon}]$ and $\epsilon \in (0, \frac{h}{C}]$.

Finally for $\epsilon \in [0, \epsilon_0]$, we define the desired solution $x_\epsilon: [0, \frac{L}{\epsilon}] \to \Omega$ as

and estimate (6.4) is valid.

The uniqueness of the solution $x_\epsilon$ follows from the smoothness of $f^-$, $f^{+}$ and the transversality.

7.   Uniformly sliding systems

Notice that $\nabla G$ is used in the definition of the function $g$. To ensure that the function $g$ is Lipschitz continuous, which is needed to use the averaging theorem for smooth systems, we will need to impose some further conditions on $\nabla G$. One can see that we deal with a differential equation defined on $(n-1)$-dimensional surface. We will show that it can be analyzed using the techniques available for differential equations defined on open subsets of $\mathbb{R}^n$ by extending the equation to an open set in $\Omega$.

Lemma 7.1. Let $(\Omega, G, f^-, f^+)$ be a well-behaved piecewise-smooth system that is uniformly sliding and let $g$ be defined by (3.1). Let $\mathcal{M}$ be a compact subset of $\Omega_0$ and let $\nabla G$ be continuously differentiable and Lipschitz continuous on some open neighborhood $V$ of $\mathcal{M}$. Then there exists an open set $U \subset V$ such that $\mathcal{M} \subset U$, $g$ can be defined by the formula (3.1) on $U \times \mathbb{R} \times [0, 1]$, and the function $g: U \times \mathbb{R} \times [0, 1] \to \mathbb{R}^n$ is $T$-PCDLB.

Proof. We define a function $\phi: \Omega \to \mathbb{R}$ as

The uniform sliding implies that $\phi(x) \geq 2m$ for all $x \in \Omega_0$, where $m$ is a positive constant from the definition of uniformly sliding systems. For every $x \in \mathcal{M}$, there is a radius $d > 0$ such that $\overline{B_{d}(x)} \subset V$ and $\phi(y) \geq m$ for all $y \in B_{d}(x)$. Since the compact set $\mathcal{M}$ is covered by these balls, there exists a finite open subcover of $\mathcal{M}$. Let $U$ be the union of the balls from the finite open subcover of $\mathcal{M}$. It is clear that $\overline{U} \subset V$ and $\phi(x) \geq m$ for all $x \in U$.

This implies that for all $x \in U$, $t \in \mathbb{R}$ and $\epsilon \in [0, 1]$,

Hence, the function $g$ can be extended to $\overline{U}$ and one can prove that it has the desired properties.

We are now ready to formulate and prove an averaging theorem for sliding solutions of piecewise-smooth systems.

Theorem 7.2. Let $(\Omega, G, f^-, f^+)$ be a well-behaved piecewise-smooth system that is uniformly sliding. Let $w: [0, L] \to \Omega$ be a solution of the sliding adjusted guiding system

associated with the initial value problem $\dot x = \epsilon f^{\pm}(x, t, \epsilon)$, $x(0) = a \in \Omega_-$, where $g$ is given by (3.1). Let $\tau_0$ be such that $0 < \tau_0 < L$, $w(\tau) \in \Omega_-$ for $\tau \in [0, \tau_0)$ and $w(\tau) \in \Omega_0$ for $\tau \in [\tau_0, L]$. Furthermore, let there be an open set $V$ such that $w([\tau_0, L]) \subset V \subset \Omega$ and $\nabla G$ is continuously differentiable and Lipschitz continuous on $V$.

Then there is a constant $\epsilon_0 > 0$ such that for all $\epsilon \in (0, \epsilon_0]$, there is a unique solution $x_\epsilon$ of the problem $\dot x = \epsilon f^{\pm}(x, t, \epsilon)$, $x(0) = a \in \Omega_-$ defined on $[0, \frac{L}{\epsilon}]$ and sliding from time $t_\epsilon$. In addition, there are constants $C, A > 0$ such that $| t_\epsilon - \frac{\tau_0}{\epsilon} | \leq A$ and

for all $t \in [0, \frac{L}{\epsilon}]$.

Proof. The uniform sliding condition implies that

Hence there is a solution $w^-$ of $\dot w = \bar f^-(w)$, $w(0) = a$ defined on $[0, L^-]$ for some $L^- > \tau_0$ such that $w^{-}(\tau) \in \Omega_+$ for all $\tau \in (\tau_0, L^-]$.

The averaging theorem for smooth systems implies that there exist $\epsilon_0, C > 0$ such that for all $\epsilon \in (0, \epsilon_0]$, there is a unique solution $x^-_\epsilon$ of $\dot x = \epsilon f^-(x, t, \epsilon)$, $x(0) = a$ defined on $[0, \frac{L^-}{\epsilon}]$ and it holds

for every $t\in[0, \frac{L^-}{\epsilon}]$. Lemma 5.2 implies that there are constants $A$, $B$ such that for all $\epsilon \in (0, \epsilon_0]$, the solution $x^-_\epsilon$ crosses $\Omega_0$ and we have

where $t_\epsilon$ is the time of the first contact of $x^-_\epsilon$ with $\Omega_0$.

For $\tau \in [\tau_0, L]$, we define $w^0(\tau) = w(\tau)$. Thus the function $w^0$ is the solution of the initial value problem $\dot w = \bar g(w)$, $w(\tau_0) = x_0$.

Due to Lemma 7.1, there is an open set $U\subset V$ such that g is $T$-PCDLB on $U$. The uniform sliding condition implies that the solution $x_\epsilon^{-}$ can be uniquely extended as a solution $x_{\epsilon}^{0}$ of the initial value problem $\dot x = \epsilon g(x, t, \epsilon)$, $x(t_\epsilon) = x^-_\epsilon(t_\epsilon)$. Trajectories of solutions of this system lie in $\Omega_0$ and the local solution $x_{\epsilon}^{0}$ can be extended at least as long as the solution does not approach the boundary of $U$. Suppose that $x_{\epsilon}^{0}$ can be defined at least up to some $s > t_\epsilon$.

Assume that $s \leq \frac{\tau_0}{\epsilon}+A$. For every $t \in [t_\epsilon, s]$, we estimate

Using estimate (7.1) and the Lipschitz continuity of solutions $x^0_\epsilon$, $x^-_\epsilon$ and $w^-$, $w^0$, we arrive at

for $t_\epsilon \leq t \leq s \leq \frac{\tau_0}{\epsilon}+A$. Observe that $C$ does not depend on $s$, if $s \leq \frac{\tau_0}{\epsilon}+A$ and $x^0_\epsilon$ is separated from $\partial U$ for $\epsilon_{0}$ sufficiently small. Since $s$ was arbitrary, the solution $x^0_{\epsilon}$ can be defined up to $\frac{\tau_0}{\epsilon}+A$.

At this point, we can use Lemma 2.4 on the initial value problem $\dot x = \epsilon g(x, t, \epsilon)$, $x(\frac{\tau_0}{\epsilon}+A) = x^0_{\epsilon}(\frac{\tau_0}{\epsilon}+A)$ with $w^0:[\tau_0, L] \to \Omega$ and $L_1 = \tau_0$, $a = w^0(\tau_0)$, $L_2 = L$. The assumptions of Lemma 2.4 are satisfied since

Hence for all $\epsilon\in(0, \epsilon_{0}]$, the solution $x^0_{\epsilon}$ can be extended to the interval $[\frac{\tau_0}{\epsilon}, \frac{L}{\epsilon}]$, and it holds

for all $t \in [\frac{\tau_0}{\epsilon}+A, \frac{L}{\epsilon}]$. It is clear that the function

is the desired unique solution.

8.   Conclusion

We have contributed to the study of piecewise-smooth differential equations by extending the results of averaging methods for periodic systems to the piecewise-smooth setting. Our main contribution is the analysis of how the boundary behavior influences averaging, especially in case of sliding. We have also proposed some sufficient conditions that allowed us to prove results on averaging in case of transversal crossing and sliding. Moreover, we demonstrated how to achieve results for solutions of piecewise-smooth systems by dividing them into solutions of smooth systems and patching together results from the smooth setting. Future opportunities for study include weakening of the assumptions in the transversal and sliding case and extension of the results to situations, in which $f^+ \cdot \nabla G$ or $f^- \cdot \nabla G$ vanish, which includes grazing solutions. Moreover, it would be interesting to develop an averaging method for differential equations with impacts ^[2].

Acknowledgments

We thank the referee for valuable comments. The paper was supported in part by the Slovak Research and Development Agency under the contract No. APVV-18-0308 and by the Slovak Grant Agency VEGA No. 2/0153/16 and No. 1/0078/17.

Conflict of interest

All authors declare no conflicts of interest in this paper.