Global well-posedness of 2D incompressible Navier–Stokes–Darcy flow in a type of generalized time-dependent porosity media

1.   Introduction

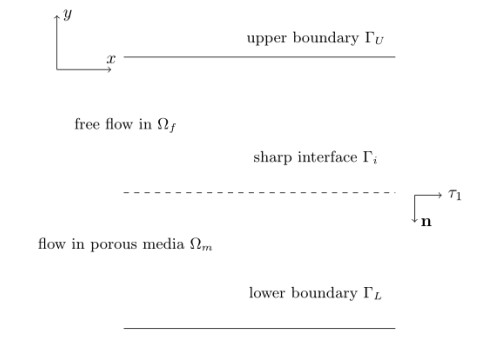
We begin by providing a comprehensive explanation of the simplified Navier-Stokes-Darcy system within the open strip domain $\Omega \subset \mathbb{R}^2$, as illustrated in Figure 1. The incompressible free fluid flows within the confined conduit region $\Omega_f \subset \mathbb{R}^2$, which is interconnected with a time-dependent porous medium region $\Omega_m \subset \mathbb{R}^2$. These two regions are separated by an interface denoted as $\Gamma_i$, where the two types of fluid flow interact. The boundaries $\Gamma_U = \partial \Omega_f \setminus \Gamma_i$ and $\Gamma_L = \partial \Omega_m \setminus \Gamma_i$ represent the upper and lower boundaries, respectively.

The porous medium flow in the conduit $\Omega_{m}$ is governed by the continuity equation and Darcy's law, as stated in ^{[1,2,3,4,5,6]}:

where $p = p(\mathbf{x}, t)$ denotes the pressure, $\mu_2$ is the constant viscosity of the fluid, and the real constant symmetric matrix $K = k \mathbb{I}$ represents the permeability of the porous medium. Here, $k$ is a positive constant satisfying $0 < \lambda \leq k \leq \Lambda$, where $\lambda$ and $\Lambda$ are known constants, and $\mathbb{I}$ is the identity matrix. Additionally, $\phi = \phi(p)$ denotes the pressure-dependent porosity. Combining the two equations in (1.1), we obtain the heat equation for the porous medium flow:

The flow in the conduit $\Omega_{f}$ is described by the Navier–Stokes equations ^[7]:

where $\mathbf{u} = \mathbf{u}(\mathbf{x}, t)$ and $p_f = p_f(\mathbf{x}, t)$ denote the velocity and pressure of the free flow, respectively, and $\mu_1$ is the constant viscosity. The stress tensor is given by $\mathbb{T}(\mathbf{u}, p_f) = 2 \mu_1 D(\mathbf{u}) - p_f \mathbb{I}$, where $D(\mathbf{u}) = \frac{1}{2}(\nabla \mathbf{u} + \nabla \mathbf{u}^T)$ represents the rate of strain tensor. The Lions condition in $(1.4)_{1}$ (see ^[8,9]) describes the balance of forces in the normal direction, while the Beavers–Joseph–Saffman–Jones interface condition in $(1.4)_{2}$ (see ^[10,11]) explains the relationship between shear stress and tangential velocity. Furthermore, we require the continuity of velocity at the interface as follows:

where $\mathbf{n}$ denotes the unit normal vector to $\Omega_{f}$, $\mathbf{\tau}$ is the tangential unit vector to $\Gamma_{i}$, and the constant $G$ relates to the trace of the matrix $K$ and experimental data used in the numerical analysis. Additionally, we impose a no-slip condition at the top boundary $\Gamma_{U}$ and an impermeable condition at the bottom boundary $\Gamma_{L}$:

The initial conditions are as follows:

Due to the extensive application of the Navier–Stokes–Darcy equation in modeling porous media coupled with free fluid flow in industrial contexts, its mathematical analysis has garnered significant attention from scholars, particularly in the field of numerical analysis. This research has yielded a wealth of results ^{[12,13,14,15,16,17,18,19,20,21]} for the case of steady porosity. When the system incorporates temperature variability, results for the Stokes–Darcy–Boussinesq equation can be found in the literature ^[22,23]. Recently, a series of numerical results ^{[11,24,25,26,27]} have been obtained for the Cahn–Hilliard–Navier–Stokes–Darcy equation, which addresses a two-phase miscibility phenomenon at the interface.

Although there is an abundance of numerical analysis on the time-dependent Navier-Stokes-Darcy equation, results on fundamental theories, particularly the well-posedness of strong solutions, remain limited. The strong coupling of the interface involving convection phenomena is evidently more complex than that in a single fluid (see ^[28,29,30]), making high-order estimates more challenging to obtain.

A comprehensive review of the selection of interface conditions for this system of equations is provided by M. McCurdy et al. ^[31]. This review indicates that the relationship between shear stress and tangential velocity in $(1.4)_{1}$ can be replaced by the Beavers–Joseph (BJ) condition ^[32] or the Beavers–Joseph–Jones (BJJ) condition ^[33] on $\Gamma_i$. Additionally, the balance of forces in the normal direction of the interface in $(1.4)_{2}$ can be replaced by Rankine–Hugoniot interface conditions:

Here, we highlight several results and corresponding references related to the mathematical analysis of the coupled Navier–Stokes and Darcy equations. For the flow system involving non-deformable porous media with constant porosity $\phi(p) \equiv C$ and a permeability tensor $K$ that is either a constant matrix or satisfies ellipticity conditions, typical mathematical analyses include those by Layton ^[18] and Discacciati ^[19]. The analysis of underground mixed-phase displacement problems has been addressed by H. Alt and S. Luckhaus ^[34], as well as P. Fabrie and M. Langlais ^[35], P. Fabrie and T. Gallouët ^[36], and F. Marpeau and M. Saad ^[37]. P. Liu and W. Liu ^[38] obtained global well-posedness results for the strong solution of the porous medium fluid coupled with free flow in the two-dimensional case with the BJSJ–Lions interface condition. Subsequently, M. Cui et al. ^[39] achieved global well-posedness results for the strong solution of the porous media fluid coupled with free flow system under BJSJ–Rankine–Hugoniot interface conditions with periodic boundary conditions, and demonstrated the attenuation of the solution. L. Tan et al. ^[40] extended this result to the three-dimensional case with flat domains, removing the assumption of periodic boundary conditions.

The paper is structured as follows: in Section 2, we provide the necessary definitions, hypotheses, main theorems, and preparatory lemmas. Section 3 introduces the a priori estimates and their proofs. Finally, Section 4 presents the proofs of the main theorems.

2.   Preliminaries and main results

For the convenience of notation in this article, we make the following conventions:

$1)$ The function $B(p)\in L^{\infty}(0, T;L^{1}(\Omega_{m}))$ satisfying:

$2)$ For any $T\in (0, +\infty]$, we first define a function space $X(0, T)$ as

then $(\mathbf{u}, p) \in X(0, T)$ is called the strong solution of (1.2)–(1.6), if it satisfies systems (1.2) and $(1.3)$, and a.e. in $\Omega \times (0, T)$, and fulfills the conditions (1.4)–(1.6).

$3)$ Let $\|\cdot\|_{p, f}\triangleq \|\cdot\|_{L^{p}(\Omega_{f})}$, $\|\cdot\|_{p, m}\triangleq \|\cdot\|_{L^{p}(\Omega_{m})}$, $\|\cdot\|_{p, i}\triangleq \|\cdot\|_{L^{p}(\Gamma_{i})}$, for any $p$, $1\leq p\leq +\infty.$

Assumption 2.1. Suppose that $\phi \in C^4(\mathbb{R})$, and

where $\epsilon_{0}$ is small enough depends only on $\lambda$, $\mu_{2}$, $\Omega_{m}$, $\|\mathbf{u}_{0}\|_{H^{2}(\Omega_{f})}^{2}$, $\| \nabla p_{0}\|_{H^{1}(\Omega_{m})}^{2}$ and $\|B(p_{0})\|_{L^{1}(\Omega_{m})}$.

Assumption 2.2. At least one of the following two equations is true:

2.1.   Main results

We are now ready to present the main results of this paper.

Theorem 2.1 (Global existence). For $\mathbf{u}_0 \in H^2(\Omega_f)$, $B(p_{0})\in L^1(\Omega_m),$ $\nabla p_0 \in H^1(\Omega_m)$, if Assumptions 1 and 2 hold, then there exists a sufficiently small constant $\epsilon_{0}$, depending only on $\mu_{1}$, $\mu_{2}$, $\lambda$, $\Omega_{f}$, and $\Omega_{m}$, such that if

the coupled time-dependent Navier–Stokes–Darcy flow system (1.2)–(1.6) has a unique global strong solution $(\mathbf{u}, p)$ in $X(0, +\infty)$.

A few remarks are in order:

Remark 1. This paper studies two cases of $\phi(p)$: both linear and nonlinear dependencies. For the linear dependency, as addressed in ^{[41,42,43,44]}, we consider

where $\bar{C}_1$ and $\bar{C}_2$ are constants. As is well known, if (2.5) holds, the Eq (1.2) is equivalent to

which simplifies to a typical parabolic equation. According to ^{[41,42,43,44]}, research on this model has thus far been limited to global existence results for weak solutions.

The nonlinear relationship, as described in ^[45], is given by

where $\phi_{r}$ is the critical value of porosity when the pressure $p$ reaches the pressure limit $p_{r}$, and $C_{R}$ is a constant representing the deformability of the rock components, if (2.7) holds, the Eq (1.2) is equivalent to

It appears that this is the first exploration of the theoretical results for the incompressible Navier–Stokes flow coupled with the time-dependent Darcy flow (2.8), particularly concerning the global well-posedness of strong solutions. It is noteworthy that the constraint (2.2) is applicable to both (2.6) and (2.8). When the condition $n \geq 2$ is satisfied, $\phi^{(n)} = 0$ becomes sufficiently small. Consequently, it is reasonable to disregard the term involving $\left\|\frac{\phi^{(n)}}{(\phi')^m}\right\|_{\infty, m}$ due to its insignificance, thereby ensuring that our estimation process effectively encompasses this scenario.

Remark 2. Note that the Assumption 2.2 is consistent with the system that concerns the physical significance of the rock compressibility ratio $C_{R}$, as described in (2.7). Thus, we have

if and only if $C_{R} \ll \epsilon_{0}$, which is consistent with the slight compressibility of actual underground rocks.

3.   A priori estimates

As is well known, the global strong solution to nonlinear partial differential equations can be obtained by combining local solutions with global a priori estimates. The local solution can be derived through higher-order regularity estimates, which are omitted here. Instead, we present the necessary a priori estimates crucial for establishing the global well-posedness of the coupled problems (1.2)–(1.6).

Let $(\mathbf{u}, p)$ be the strong solution to (1.2)–(1.6) satisfying $\mathbf{u}_0 \in H^2(\Omega_f)$, $B(p_{0})\in L^1(\Omega_m),$ and $\nabla p_0 \in H^1(\Omega_m)$. The basic energy estimate is stated in the following lemma.

Lemma 3.1. Under the conditions of Theorem 2.1, it holds that

Proof. Multiplying $(1.3)_{1}$ by $\mathbf{u}$ and integrating the resulting equation with respect to $\mathbf{x}$ over $\Omega_{f}$, we obtain

where we have used the interface-boundary conditions and the vector decomposition of free flow velocity at the interface $\Gamma_{i}$:

Simultaneously, we multiply (1.2) by $p$ and integrate the resulting equation with respect to $\mathbf{x}$ over $\Omega_{m}$. Using (2.1), we obtain

Adding (3.2) and (3.4), and then integrating the resulting equation over $(0, t)$ gives:

The proof of Lemma 3.1 is complete. □

The second-order estimate is obtained under the a priori assumptions. We have the following proposition.

Proposition.

There exist positive constants $\epsilon_{0}$ depending only on $\lambda$, $\mu_{2}$, and $\Omega_{m}$, such that if $(\mathbf{u}, p)$ is a smooth solution of (1.2)–(1.6) on $\mathbb{R}^{2} \times (0, T]$ satisfying:

the following estimates hold:

and

provided

where $M = \max\{1, \; C\epsilon_{0}\}$, and $C$ depends only on $\mu_{1}, \; \mu_{2}, \; \lambda, \; G, \; \Omega_{f}$, and$\; \Omega_{m}.$

Proposition 3.1 is a direct consequence of the following lemmas, from Lemma 3.2 to Lemma 3.5.

Lemma 3.2. Under the conditions of Proposition 3.1, it holds that

where $C$ depends only on $\mu_{1}, \; \mu_{2}, \; \lambda, \; G, \; \Omega_{f}, \; \Omega_{m}$.

Proof. To estimate the second derivative term of the fluid velocity with respect to space, one must consider the fact that

Next, we focus on $\|\mathbf{u}_{yy}\|_{2, f}$. From the Eq $(1.3)_{1}$, we know

It is straightforward to derive, using Hölder's inequality, Young's inequality, and the Gagliardo–Nirenberg inequality ^[46], that

where $\varepsilon$ is sufficiently small. For $\|\nabla p_{f}\|_{2, f}$, taking the divergence of $(1.3)_{1}$ and using (1.2), we can derive

due to $(1.4)_{1}$, we have

According to the standard results (Lemma 2.5, ^[39]) for the Dirichlet–Neumann problem, and using Young's inequality with $\varepsilon$ (where $\varepsilon$ is sufficiently small), the Trace theorem, and Poincaré's inequality ^[47], we have

Combining (3.11), (3.12), and (3.13), we obtain

Next, we will estimate it sequentially.

Step 1. ($L^{2}$ estimate of $D(\mathbf{u})$)

Multiply $(1.3)_{1}$ by $\mathbf{u}_{t}$ and integrate the resulting equation with respect to $\mathbf{x}$ over $\Omega_{f}$. Similarly, multiply (1.2) by $p_{t}$ and integrate the resulting equation with respect to $\mathbf{x}$ over $\Omega_{m}$. Adding the two resulting equations and applying Green's formula yields

Now, integrating (3.17) over $(0, t)$ and applying (3.19), Young's inequality, and Grönwall's inequality, we obtain

where we have used the following estimates (3.19), derived from Hölder's inequality, Korn's inequality, Young's inequality, the Gagliardo–Nirenberg inequality, (3.1), and (3.16):

and the following estimation obtained by Green's formula and Lemma 3.1:

Step 2. ($L^{2}$ estimate of $D(\mathbf{u}_{x})$).

Differentiate $(1.3)_{1}$ with respect to $x$, multiply by $\mathbf{u}_{x}$, and then integrate the resulting equation with respect to $\mathbf{x}$ over $\Omega_{f}$. Similarly, differentiate (1.2) with respect to $x$, multiply by $p_{x}$, and integrate the resulting equation with respect to $\mathbf{x}$ over $\Omega_{m}$. Adding the two equations, we obtain

Thus, applying Hölder's inequality, Young's inequality, the Gagliardo–Nirenberg inequality, and Korn's inequality ^[48], we derive that

We know from (2.2), (3.6), Hölder's inequality, Young's inequality, and the Gagliardo–Nirenberg inequality that

where $\epsilon_{0}$ is sufficiently small. We then substitute the estimates from (3.21) and (3.22) into (3.20) to

summing it up to (3.17), and integral the resulting equation over $(0, t)$, using Grönwall's inequality and (3.18), we obtain

Therefore, the proof of Lemma 3.2 is complete. □

Lemma 3.3. Under the conditions of Proposition 3.1, it holds that

where $C$ depends only on $\mu_{1}, \; \mu_{2}, \; \lambda, \; G, \; \Omega_{f}, \; \Omega_{m}$.

Proof. We have, given that $\phi(p) \in C^4(\mathbb{R})$ and (3.6):

Differentiating $(1.3)_{1}$ with respect to $t$, multiplying by $\mathbf{u}_{t}$, and integrating the result with respect to $\mathbf{x}$ over $\Omega_{f}$, and differentiating (1.2) with respect to $t$, then multiplying by $p_{t}$, and integrating with respect to $\mathbf{x}$ over $\Omega_{m}$, we obtain:

We deduce from Hölder's inequality, Young's inequality, Gagliardo–Nirenberg inequality, and Korn's inequality ^[48] that:

Based on Young's inequality, we obtain

For $\|\sqrt{|\phi'|} p_{t}\|_{4, m}^{2}$, applying Hölder's inequality, Young's inequality, Gagliardo–Nirenberg inequality ^[46], and Korn's inequality ^[48], we have:

From $\nabla^2 p = \{p_{xx}, p_{xy}, p_{yy}\}$, we can derive, using (1.2) and (3.25), that

Therefore, recalling (3.1) and (3.9), we obtain

Then, by integrating (3.26) over $(0, t)$ and utilizing (3.31), (3.27), and Grönwall's inequality,

The proof of Lemma 3.3 is complete. □

Lemma 3.4. Under the conditions of Proposition 3.1, it holds that

where $C$ depends only on $\mu_{1}, \; \mu_{2}, \; \lambda, \; G, \; \Omega_{f}, \; \Omega_{m}$.

Proof. Step 1. ($L^{2}$ estimate of $D(\mathbf{u}_{x})$)

Differentiating $(1.3)_{1}$ with respect to $x$, multiplying by $\mathbf{u}_{tx}$, and then integrating the resulting equation with respect to $\mathbf{x}$ over $\Omega_{f}$, we apply Hölder's inequality, Young's inequality, the extension theorem ^[7], Gagliardo–Nirenberg inequality, and Korn's inequality ^[48] to obtain

Next, integrating (3.34) over $(0, t)$, and using (3.1), (3.9) and (3.24), along with Grönwall's inequality, we obtain

By substituting (3.1) and (3.9) into (3.16), for any $t \in [0, T]$, we obtain:

Step 2. ($L^{2}$ estimate of $\nabla p_{x}$)

Differentiating (1.2) with respect to $x$, multiplying by $p_{tx}$, and then integrating the resulting equation with respect to $\mathbf{x}$ over $\Omega_{m}$, with Hölder's inequality, Gagliardo–Nirenberg inequality, Young's inequality and the extension theorem ^[7] to obtain:

with (3.9), (3.24) and (3.29), we have

Then, integrating (3.37) over $(0, t)$, and utilizing (3.9), (3.35), the extension theorem ^[7], Young's inequality, and Grönwall's inequality, we obtain:

From (3.30), (3.32), and (3.38), we have

Thus, the proof of Lemma 3.4 is complete. □

We are now in a position to prove (3.7). If (2.3) in Assumption 2.2 holds, then $\|\phi'(p)\|_{\infty, m} \leq C$ naturally follows, and so does $\|p\|_{\infty, m} \leq C$. If (2.4) holds, we employ truncation techniques to ensure that the fluid domain in the porous medium region $\Omega_{m}$ satisfies:

Applying L'H\^{o}pital's rule, we have

Thus, by (2.4), it follows that

Using the Gagliardo–Nirenberg inequality, we obtain

Thus, the proof of (3.7) is complete, and Proposition 3.1 is established by synthesizing (3.9), (3.24), and (3.33).

Lemma 3.5. Under the conditions of Proposition 3.1, it holds that

where $C$ depends only on $\mu_{1}, \; \mu_{2}, \; \lambda, \; G, \; \Omega_{f}, \; \Omega_{m}$.

Proof. Differentiate $(1.3)_{1}$ twice with respect to $x$, multiply both sides of the resulting equation by $\mathbf{u}_{xx}$, and integrate over $\Omega_{f}$ with respect to $\mathbf{x}$. Similarly, differentiate (1.2) twice with respect to $x$, multiply both sides of the resulting equation by $p_{xx}$, and integrate over $\Omega_{m}$ with respect to $\mathbf{x}$. Then, summing the two resulting equations yields:

Applying Hölder's inequality, Young's inequality, the Gagliardo–Nirenberg inequality, and Korn's inequality ^[48], we obtain:

Using Hölder's inequality, Young's inequality, and the Gagliardo–Nirenberg inequality, we can derive that

Then, integrating (3.41) over $(0, t)$, and using (3.1), (3.9), (3.24), (3.33), (3.42), and (3.43), we obtain:

Thus, we can successfully complete the proof of Lemma 3.5. □

It is time to obtain the high-order estimates. We have a refined version of (3.29) given by:

3.1.   Higher-order estimation

Let $\sigma(t) = \min\{1, t\}$. Then, we obtain the following higher-order estimates.

Lemma 3.6. It holds that

where $C$ depends only on $\mu_{1}, \; \mu_{2}, \; \lambda, \; G, \; \Omega_{f}, \; \Omega_{m}, \; \|\mathbf{u}_{0}\|_{H^{2}(\Omega_{f})}, \; \|p_{0}\|_{H^{2}(\Omega_{m})}$.

Proof. By considering $\|\nabla^{3}\mathbf{u}\|_{2, m} = \{\mathbf{u}_{xxx}, \mathbf{u}_{xxy}, \mathbf{u}_{xyy}, \mathbf{u}_{yyy}\}$, we have

To estimate $\|\nabla\mathbf{u}_{yy}\|_{2, f}$, we differentiate $(1.3)_{1}$ to obtain

It follows from Hölder's inequality, Young's inequality, and the Gagliardo–Nirenberg inequality that

For $\|\nabla^{2} p_{f}\|_{2, f}$, using results from the Dirichlet-Neumann problem (Lemma 2.5, ^[39]), along with Young's inequality, the Trace theorem, and Poincaré's inequality ^[47], we have

Combining (3.46)–(3.48), we derive that

Next, we will estimate $\|D(\mathbf{u}_{t})\|_{2, f}$, $\| \mathbf{u}_{xyy}\|_{2, f}$, and $\|D(\mathbf{u}_{xx})\|_{2, f}$ step by step.

Step 1. ($L^{2}$ estimate of $D(\mathbf{u}_{t})$)

Differentiating $(1.3)_{1}$ with respect to $t$, multiplying the resulting equation by $\mathbf{u}_{tt}$, and integrating the equation with respect to $\mathbf{x}$ over $\Omega_{f}$, simultaneously, differentiating (1.2) with respect to $t$, multiplying the resulting equation by $p_{tt}$, and integrating the equation with respect to $\mathbf{x}$ over $\Omega_{m}$, and summing up the two resulting equations, we get

We have the following inequalities: Hölder's inequality, Young's inequality, Gagliardo–Nirenberg inequality, and Korn's inequality ^[48]:

and we obtain, using Hölder's inequality, Young's inequality, Gagliardo–Nirenberg inequality, (2.2), and (3.44):

Multiplying (3.50) by $\sigma(s)$ and integrating the result over $(0, t)$, we obtain, using Young's inequality, Hölder's inequality, Gagliardo–Nirenberg inequality, Grönwall's inequality, and (3.51)–(3.54), that

where we have used the extension theorem ^[7] to estimate the interface term as follows:

where $\varepsilon$ and $\eta$ are sufficiently small.

Step 2. ($L^{2}$ estimate of $\mathbf{u}_{xyy}$)

We differentiate $(1.3)_{1}$ with respect to $x$ to obtain:

We know from Young's inequality, the Gagliardo–Nirenberg inequality, and Korn's inequality ^[48] that

For $\|\nabla p_{fx}\|_{2, f}$, by taking the partial derivative of $(1.3)_{1}$ with respect to $x$ and then applying the divergence operator, and using the incompressibility condition $(1.2)$, we obtain

Due to $(1.4)_{1}$, we have the following boundary conditions:

Similarly to the method used to obtain (3.13), and based on the typical results (Lemma 2.5, ^[39]) for the Dirichlet–Neumann problem, as well as the Trace theorem and Poincaré's inequality ^[47], we have

By applying the gradient to (1.2) and using (2.2), we obtain

where we have utilized Hölder's inequality, Young's inequality, Gagliardo–Nirenberg inequality, and (3.44). Thus, combining (3.55), (3.47), (3.59), and (3.60), we conclude that

In this case, the term $\|D(\mathbf{u}_{xx})\|_{2, f}^{4}$ is not sufficient to close the estimate. We should proceed to the next step for now.

Step 3. ($L^{2}$ estimate of $D(\mathbf{u}_{xx})$)

Differentiating $(1.3)_{1}$ twice with respect to $x$, multiplying the resulting equation by $\mathbf{u}_{txx}$, and integrating over $\Omega_{f}$ with respect to $\mathbf{x}$, and simultaneously differentiating (1.2) twice with respect to $x$, multiplying the resulting equation by $p_{txx}$, and integrating over $\Omega_{m}$ with respect to $\mathbf{x}$, we obtain:

We employ Hölder's inequality, Young's inequality, Gagliardo–Nirenberg inequality, Korn's inequality ^[48], and (3.61) to obtain:

Similarly, with the help of Hölder's inequality, Young's inequality, Gagliardo–Nirenberg inequality ^[46], (2.2), and (3.65)–(3.68), we obtain

The proofs for (3.65)–(3.68) are as follows. By applying the Gagliardo–Nirenberg inequality, we have

Using the Gagliardo–Nirenberg inequality, Young's inequality, Hölder's inequality, along with (2.2) and (3.44), we obtain

Using Young's inequality and Hölder's inequality, we obtain

By applying the Gagliardo–Nirenberg inequality, Young's inequality, and Hölder's inequality to (1.2), we obtain

Next, by multiplying (3.62) by $\sigma(s)$ and integrating with respect to $t$ over $(0, t)$, and applying (3.51), (3.64), and Grönwall's inequality, we obtain

where we have also utilized the result derived using the extension theorem ^[7], given as follows:

where $\varepsilon$ and $\eta$ are small enough.

Therefore, with (3.8), (3.53), (3.61), (3.69), and Korn's inequality ^[48], we obtain

so combine it with (3.49), we have:

Knowing from (1.2), (3.8), (3.24), (3.30), (3.53), and (3.44), we can derive, with the aid of the Gagliardo–Nirenberg inequality, Young's inequality, and Hölder's inequality, that

Therefore, the proof of Lemma 3.6 is complete. □

We can establish Lemma 3.7 before deriving the fourth-order estimates.

Lemma 3.7. It holds that

Proof. Step 1. ($L^{2}$ estimate of $\mathbf{u}_{tx}$)

Apply $\partial_{t}\partial_{x}$ to $(1.3)_{1}$, then multiply the resulting equation by $\sigma(t)\mathbf{u}_{tx}$ and integrate over $\Omega_{f}$. Similarly, apply $\partial_{t}\partial_{x}$ to $(1.2)$, then multiply the resulting equation by $\sigma(t) p_{tx}$ and integrate over $\Omega_{m}$. Summing the two resulting equations, we obtain the following result using Hölder's inequality, Gagliardo–Nirenberg inequality, and Young's inequality:

where we have utilized (3.74) and (3.75), which were derived using Hölder's inequality, Gagliardo–Nirenberg inequality, and Young's inequality.

where $\varepsilon$ and $\eta$ are small enough. Based on (3.8) and (3.53), we can obtain that

Thus, integrating (3.73) over $(0, t)$, and using (3.8), (3.74), (3.75), and Grönwall's inequality, we obtain

Additionally, based on equations (3.74) and (3.76), the following results can be derived:

Step 2. ($L^{2}$ estimate of $\mathbf{u}_{xxx}$)

By differentiating $(1.3)_{1}$ three times with respect to $x$, multiplying both sides of the resulting equation by $\sigma(t) \mathbf{u}_{xxx}$, and integrating over $\Omega_{f}$ with respect to $\mathbf{x}$, and similarly, by differentiating (1.2) three times with respect to $x$, multiplying both sides of the resulting equation by $\sigma(t) p_{xxx}$, and integrating over $\Omega_{m}$ with respect to $\mathbf{x}$, we obtain:

Applying Hölder's inequality, Young's inequality, the Gagliardo–Nirenberg inequality, and Korn's inequality ^[48], we obtain:

And we obtain with (2.2):

It can be derived with Gagliardo–Nirenberg inequality that

similarly, we obtain

By applying the Gagliardo–Nirenberg inequality and Young's inequality, we obtain

Then, integrating (3.78) over the interval $(0, t)$ and utilizing (3.8), (3.75)–(3.77), (3.79), (3.80), as well as Grönwall's inequality, we obtain:

Thus, the proof of Lemma 3.7 is complete. □

Next, it is time to get the fourth-order estimates.

Lemma 3.8. It holds that

Proof. It follows from the fact that

Clearly, by applying Hölder's inequality, Young's inequality, the Gagliardo–Nirenberg inequality ^[46], Poincaré's inequality ^[47], Sobolev inequality, and Korn's inequality ^[48] to (3.11), we obtain:

For $\|\nabla^{3} p_{f}\|_{2, f}$, by leveraging the typical results (Lemma 2.5, ^[39]) of the Dirichlet–Neumann problem, (3.47), Young's inequality, the Trace theorem, and Poincaré's inequality ^[47], we have:

Combining (3.85), (3.86) and (3.87), we have

Next, we will estimate $\|D(\mathbf{u}_{xxx})\|_{2, f}$, $\|\nabla^{2}\mathbf{u}_{t}\|_{2, f}$, $\| \nabla \mathbf{u}_{xyy}\|_{2, f}$, and $\|\mathbf{u}_{xxyy}\|_{2, f}$ step by step.

Step 1. ($L^{2}$ estimate of $D(\mathbf{u}_{xxx})$)

By differentiating $(1.3)_{1}$ three times with respect to $x$, multiplying the resulting equation by $\mathbf{u}_{txxx}$, and integrating with respect to $\mathbf{x}$ over $\Omega_{f}$, we obtain:

We employ Hölder's inequality, Young's inequality, the Gagliardo–Nirenberg inequality, and Korn's inequality ^[48] to obtain:

Similarly, by differentiating (1.2) three times with respect to $x$, multiplying both sides of the resulting equation by $p_{txxx}$, and integrating with respect to $\mathbf{x}$ over $\Omega_{m}$, we obtain, using Hölder's inequality:

Then, for $J_{1}$, using (3.81)–(3.83), along with Hölder's inequality, Young's inequality, and the Gagliardo–Nirenberg inequality, we obtain:

where we have used the fact

In this case, by summing (3.89) and (3.91), multiplying the resulting equation by $\sigma(s)^{2}$, and integrating with respect to time over $(0, t)$, and utilizing (3.90), (3.92), (3.8), (3.45), and (3.72), we find that:

In fact, we have applied the extension theorem ^[7] once more, as done in the previous steps, as follows:

where $\varepsilon$ and $\eta$ are small enough.

Step 2. ($L^{2}$ estimate of $\nabla^{2}\mathbf{u}_{t}$)

For $\|\nabla^{2}\mathbf{u}_{t}\|_{2, f}^{2}$, according to $\nabla^{2}\mathbf{u}_{t} = \{ \mathbf{u}_{txx}, \mathbf{u}_{txy}, \mathbf{u}_{tyy}\} = \{ \nabla \mathbf{u}_{tx}, \mathbf{u}_{tyy}\},$ we have $\|\nabla^{2}\mathbf{u}_{t}\|_{2, f}^{2} \leq C(\|\nabla \mathbf{u}_{tx}\|_{2, f}^{2} +\| \mathbf{u}_{tyy}\|_{2, f}^{2}).$ Taking the derivative of $(1.3)_{1}$ with respect to $t$ yields:

and

Thus, we have

For $\| D(\mathbf{u}_{tx})\|_{2, f}^{2}$, we apply $\partial_{t}\partial_{x}$ to $(1.3)_{1}$ and multiply by $\mathbf{u}_{ttx}$, then integrate the resulting equation by parts with respect to $\mathbf{x}$. Similarly, applying $\partial_{t}\partial_{x}$ to (1.2) and multiplying by $p_{tx}$, we integrate this equation by parts with respect to $\mathbf{x}$. Summing the results of these two integrations, and then multiplying by $\sigma(s)^{2}$ and integrating the resulting equation with respect to time over $(0, t)$, using (3.75) and (3.77), we obtain:

Thus, applying Hölder's inequality, Young's inequality, and the Gagliardo–Nirenberg inequality ^[46], we have:

and using (2.2), we derive that

Thus, we can derive

where we have used the following estimates

where $\varepsilon$ and $\eta$ are small enough.

To complete this step, we need to estimate $\|\mathbf{u}_{tt}\|_{2, f}^{2}$. We apply $\partial_{t}^{2}$ to $(1.3)_{1}$ and multiply by $\sigma(t)^{2} \mathbf{u}_{tt}$, then integrate the resulting equation with respect to $\mathbf{x}$ over $\Omega_{f}$. Similarly, we apply $\partial_{t}^{2}$ to (1.2) and multiply by $\sigma(t)^{2} p_{tt}$, then integrate the resulting equation with respect to $\mathbf{x}$ over $\Omega_{m}$. Finally, summing these results yields:

We obtain from (3.95), Gagliardo–Nirenberg inequality, Hölder's inequality, Young's inequality, and Korn's inequality ^[48] that

Applying the Gagliardo–Nirenberg inequality, Hölder's inequality, Young's inequality, and (2.2), we obtain:

For $\|\sqrt{|\phi'|} p_{t}\|_{6, m}$, using the Gagliardo–Nirenberg inequality, (3.8), (3.45), and (3.72), we can derive:

For $\| p_{t}\|_{\infty, m}^{2}$, we have with (3.8), (3.45), (3.72), and (3.75) that

Therefore, by integrating (3.100) with respect to $t$ and applying Grönwall's inequality, with the assistance of (3.45) and (3.72), we obtain:

Thus, we can substitute (3.8), (3.45), (3.99), and (3.103) into (3.95) to obtain:

Step 3. ($L^{2}$ estimate of $\nabla\mathbf{u}_{xyy}$)

For $\| \nabla \mathbf{u}_{xyy}\|_{2, f}^{2}$, by differentiating $(1.3)_{1}$ with respect to $x$ and applying the gradient operator $\nabla$ to the resulting equation, and utilizing Korn's inequality ^[48], we obtain:

From (3.87), using the typical results (Lemma 2.5, ^[39]) of the Dirichlet-Neumann problem, along with (3.47), Young's inequality, the Trace theorem, and Poincaré's inequality ^[47], we have:

so we have with (3.45), (3.72), (3.94), and (3.99) that

For $\|\mathbf{u}_{xxyy}\|_{2, m}^{2}$, by applying $\partial_{x}^{2}$ to $(1.3)_{1}$ and using (3.45) and (3.72), we obtain:

where we have used the following result:

Thus, we apply (3.107) into (3.94) to obtain

Using (3.108) and (3.109), it can be derived that:

Applying $\partial_{x}^{2}$ to $(1.3)_{1}$, with (3.45), (3.72), (3.99), and (3.109) gives

And with (3.106), (3.107), and (3.111), we have

From (1.2), we know $\nabla ^{4}p = \{ p_{xxxx}, \; p_{xxxy}, \; \; p_{xxyy}, \; p_{xyyy}\}, \; \; \; \nabla^{2} p_{t} = \{ p_{txx}, \; p_{txy}, \; \; p_{tyy}\}.$ Using the Gagliardo–Nirenberg inequality, Young's inequality, (3.45), and (3.99), we can derive

Thus, with (3.45) (3.88), (3.99), (3.103), (3.104), (3.109), and (3.111)–(3.113), the proof of Lemma 3.8 is complete. □

4.   Global well-posedness

According to Lemmas 3.1–3.5, the local existence and uniqueness of the solution to the systems (1.2)–(1.6) on some interval $0 < T_{*} \leq T$ is established. The focus of this study is now to verify the continuity of the strong solution in order to achieve global well-posedness.

In fact, since $\mathbf{u}_{0} \in H^{2}(\Omega_{f})$ and $p_{0} \in H^{2}(\Omega_{m})$, there exists $T_{1} \in (0, T_{*}]$ such that (3.6) holds for $T = T_{1}$. Next, we set:

For $T^{*} \geq T_{1} \geq 0$, applying Proposition 3.1, Lemmas 3.6–3.8 implies that for any bounded time $T$, i.e., $0 < \tau < T \leq T_{*}$, we have:

Therefore, it can be derived

since

Now, we set

By Proposition 3.1 and (3.6), with the Bootstrap principle ^[7] we know $T = T^{*}$ holds. Additionally, (4.2) implies that:

which means there exists $T^{**} > T^{*}$ such that (3.6) holds for $T = T^{**}$. This contradicts the definition of $T^{*}$ in (4.1), thus $T^{*} = \infty$.

5.   Conclusions

In this study, we address the global well-posedness of a coupled Navier–Stokes–Darcy model in two-dimensional Euclidean space, incorporating the Beavers–Joseph–Saffman–Jones interface boundary condition. We establish the existence of global strong solutions for both linear and nonlinear cases where porosity depends on pressure. For the linear case, with a porosity function $\phi(p) = \bar{C}_{1}p+\bar{C}_{2}$, the Darcy equation simplifies to a standard parabolic equation, aligning with existing research on weak solutions. In the nonlinear case, characterized by $\phi(p) = \phi_{r}\exp\{C_{R}(p-p_{r})\}$, we introduce a novel analysis of the time-dependent Darcy flow, demonstrating that our results are applicable even under complex interface conditions. The constraints considered ensure that our estimations are robust, highlighting the importance of these coupled systems for understanding underground fluid flow and providing a rigorous foundation for future research in porous media modeling and simulation. This research contributes to a deeper understanding of fluid flow phenomena in complex geological formations, offering valuable insights for both theoretical developments and practical applications in hydrogeology and related disciplines.

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgments

We wish to acknowledge the support of the National Natural Science Foundation of China (Grant No. 11931013), and the Natural Science Foundation of Shaanxi Province (Grant No. 2023-JC-QN-0073).

Conflict of interest

The authors declare there are no conflicts of interest.