1.
Introduction
With the rapid development of social networks, the spread of information becomes rapid and extensive, including the spread of rumors. The spread of rumors can cause significant economic losses and even bad political influence[1]. Research on spread of rumors has a long tradition. For decades, one of the most popular ideas has been the analysis of the dynamics of rumor propagation [2,3,4,5,6,7]. Moreover, the main purpose of most studies on rumor propagation has focused on the study of immunization strategies against rumors [8,9,10,11,12,13].
One of the main theoretical and conceptual frameworks used to model the spread of rumors is to model rumors as infectious diseases. This has been widely adopted in the field of spread of rumors research. The first serious discussion and analysis of rumor propagation appeared in the 1960s by Daley and Kendall, which is called DK model[14,15]. In this model, people in rumor spreading networks are divided into three states: Susceptible (S) are those who are not having been exposed to rumor; Infected (I) are those who are being exposed to rumor and believed to spread it. Removed(R) are those who have no interest to spread rumor. In 2001, Zanette et al.[16,17] studied the propagation rule of rumor in small-world network based on SIR model, and proved the existence of critical threshold for rumor propagation for the first time. Moreno et al.[18] in 2004 studied the propagation behavior of rumors based on SIR model on scale-free complex networks. This research approach was gradually extended to model the evolution of SIR. A number of authors [19,20] have considered the effects of influence of media on rumor spreading. Several previous studies [21,22,23] have also explored the relationship between rumor propagation and hesitation mechanisms. There is a large number of published studies [24,25] that describe the communication model of competitive information in multiplex social networks. Liu et al.[26,27] conducted a series of trials in which he mixed comments and rumor spreading in the network.
In terms of information dissemination modeling, it seems to be a common treatment to use information dissemination among friends as the main dissemination channel. As far as we know, few studies have focused on modeling information dissemination through non-friends. In fact, users in social networks not only rely on their friends to spread information, but also receive information by non-friend. In view of this situation, this paper discusses the rumor propagation model under two kinds of propagation modes (friend propagation and marketing account propagation). In some social networks, such as TikTok and other popular APPs, users can not only receive information posted by friends, but also receive a large number of non-friends' information, mainly marketing account information. In fact, the marketing account is a professional account for forwarding and commenting on hot issues in the society or a certain circle. These accounts are well-informed and have a large number of followers, so its information is more likely to be received by others, although it may not be his followers. Therefore, rumors once forwarded by marketing accounts will quickly ferment in the network. Based on the above, a main research problem of this paper is to establish a suitable rumor propagation model and control strategy.
The main contributions of this paper are as follows:
1. First, the rumor propagation model under the coexistence of two communication modes was established, and steady-state analysis was carried out.
2. Second, considering the negative losses caused by rumors and the cost of controlling rumor, the optimal control strategy to reduce rumor propagation density by suppressing individual accounts and marketing accounts is discussed.
3. Third, this paper compares the correlation between the spread rate of the rumor spread of friends accounts and marketing accounts when the network average degree is different.
4. Fourth, through the sensitivity analysis of parameters, the influences of different parameters including initial value on rumor propagation is analyzed and compared.
5. Finally, the superiority over the optimal control strategy is proved by simulation experiment.
The subsequent materials are organized in this fashion. In Section 2, we establish the Susceptible-Infected-Marketing-Removed (SIMR) model based on the coexistence of friend propagation and marketing account propagation, and conduct a steady state analysis. In Section 3, we perform theoretical analysis on the optimal control problem and a dynamic strategy for rumor immunity. In Section 4, the stability of SIMR model is verified by simulation experiments, and the influence of parameters and initial value on rumor propagation is discussed, and the influence of optimal control strategies for suppressing individual and marketing accounts on rumor propagation with different average degree is discussed. A brief conclusion is drawn in Section 5.
2.
Materials and methods
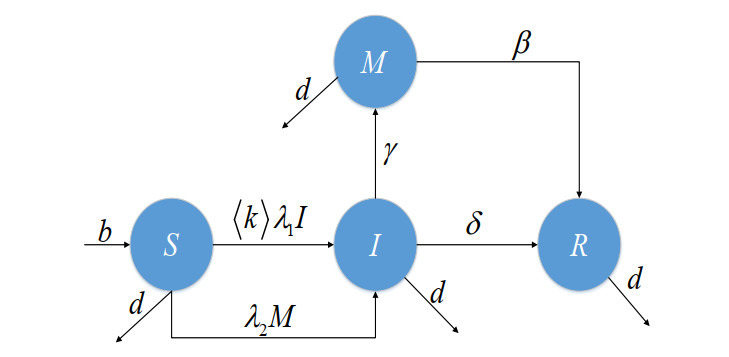
Inspired by the classic SIR model of infectious diseases, in order to describe the spread of rumors in a social network with a public marketing account (which can push information to a non-friend account), we propose the Susceptible-Infected-Marketing-Removed (SIMR) model. We divide the people in the network into four states: Susceptible (people who have not been exposed to rumors); Infected (people who believe in rumors and spread them through friends); Marketing (people who believe in rumors and push them through non-friends); Removed (people who have no interest to spread rumors). S(t),I(t),M(t),R(t) are the relative density of susceptible, infected, marketing, removed nodes at time t, respectively. (1−S(t)−I(t)−M(t)−R(t)) denotes the density of the empty nodes that can make the new users transplant into the online social networks with a certain constant rate b. For this purpose, we consider the following ordinary differential equations.
Where ⟨k⟩=∑nk=1kP(k) implies the average degree in complex network and P(k) stands for a connective distribution function. As shown in Figure 1, the rumor spreading rules of the model can be summarized as follows:
1. We consider the non-closed nature of social networks. Assume that the rate of joining network and exiting network is b and d, respectively.
2. When a susceptible node contacts infected nodes, the susceptible node turns into an infected node with rate λ1. When the susceptible node contacts the marketing accounts, the susceptible node becomes the infected node with rate λ2.
3. Infected nodes are converted to marketing nodes at a certain rate γ to spread messages.
4. An infected node becomes removed node with the rate δ when contacts removed nodes or loses interest in spreading rumors.
5. A marketing account becomes removed node with the rate β when loses interest in spreading rumors.
The vector E(t)=(S(t),I(t),M(t),R(t)) represents the relative density of state in the network at time t. The system (2.1) can be written as
Taking I(t)=M(t)=0 in system (2.1), the rumor-free equilibrium point is E0=(b/(b+d),0,0,0).
2.1. Basic reproduction number
In general, the basic reproduction number R0 means the average number of infections in a purely susceptible population[28]. Let χ=(I,M,R,S)T, then the system (2.1) can be written as χ′=Ψ(χ)−Φ(χ), where
The Jacobian matrices of Ψ and Φ evaluated at the rumor-free equilibrium χ0=(I0,M0,R0,S0)=(0,0,0,b/(b+d)) are given by
where
Then
Therefore, the basic reproduction number R0 defined by the spectral radius FV−1 [29] is
For the analytical and numerical computation of R0 in general structured population models see e.g., [30,31].
2.2. Equilibrium points
The rumor-free equilibrium point of SIMR model and the basic reproduction number of the model have been calculated. When the system reaches the rumor-free equilibrium point E0=(b/(b+d),0,0,0), the rumor disappears. And when rumor persistently spreads, it means the system has reached the rumor-prevailing equilibrium point E1=(S1,I1,M1,R1). The rumor-prevailing equilibrium should satisfy
Then the rumor-prevailing equilibrium E1 has the following form
Since
Thus, the positive rumor-prevailing equilibrium point E1=(S1,I1,M1,R1) exists if R0>1, which satisfies (2.9). In another word, when the system (2.1) reaches stability, the rumor will disappear if R0<1. On the contrary, when R0>1, rumor continues to spread in the system.
2.3. Stability of the equilibrium solution
Theorem 2.1. If R0<1, the rumor-free equilibrium point E0 of system (2.1) is locally asymptotically stable.
Proof. We can find the Jacobian matrix J(E0), which is a 4×4 matrix, is in the following form
Where J′12,J′22 are obtained by the elementary transformation,
We can easily conclude that J(E0) is an upper triangular matrix, and its four eigenvalues are −b−d,−β−d,−d,b(λ1(β+d)⟨k⟩+λ2γ)(b+d)(β+d)−(δ+d+γ) respectively. Obviously the first three eigenvalues are all negative. And then the last eigenvalue J′22<0 if and only if R0<1, since
Hence, the rumor-free equilibrium E0 is locally asymptotically stable if R0<1 by Routh-Hurwitz criterion[32], completing the proof.
So E0 is locally asymptotically stable. If R0>1, the matrix J(E0) has a positive eigenvalue, so E0 is unstable. In the following, we consider the globally asymptotically stability of the rumor-free equilibrium E0, which is the important content of the stability analysis.
Lemma 2.1. Since there are S(0),I(0),M(0),R(0)≥0, E=(S,I,M,R) is a solution of the system (2.1) and N=S+I+M+R, then the solutions of the model are uniformly bounded.
Proof. From the equations of system (2.1), we can easily observed that
It is easy to see that
It follows from the first equation of system (2.1) as
It is easy to see that
In the following, we proof that I(t),M(t)≥0 for all t>0. Assume there exists t∗1,t∗2 such that I(t∗1),M(t∗2) is negative, and t1=sup{t>0:I(t)>0,M(t)>0,∈[0,t]}. Thus, t1>0. There will be three cases as follows:
1) We have t1 such that I(t1)=M(t1)=0.
2) We have t1 such that I(t1)>0,M(t1)=0.
3) We have t1 such that I(t1)=0,M(t1)>0.
In 1), it easy to obtain I(t)=M(t)=0 for all t>t1.
In 2), for all t∈[0,t1), I(t)>0,M(t)>0 holds. By the third equation of system (2.1), we obtain
Thereby, there exists a sufficiently small positive constant ε such that for any t∈(t1−ε,t1), with M(t)<0 holds. This is in contradiction with case 2), then M(t)≥0 for all t>0. It is easy to obtain that the case 3) also contradicts the conditions known, then I(t)≥0 for all t>0. In similar fashion it can be shown that R(t)≥0 for all t>0.
So all solutions of the model are confined in the region {(S(t),I(t),M(t),R(t))∈R4+∪{0}:N(t)=bb+d}. Therefore, Lemma 2.1 has been proved. Therefore, the positive invariant set of the system (2.1) is Ω, where
Here, we use the method developed by Castillo-Chavez et al.[33]. we list two conditions that if met, also guarantee the global asymptotic stability of the rumor-free state. We rewrite the model system (2.1) as
where x∈Rm denotes (its components) the number of uninfected individuals including susceptible, removed, et al. and H∈Rn denotes (its components) the number of infected individuals including infected, et al. u0=(x∗,0) denotes the rumor-free equilibrium of this system.
Lemma 2.2. [33] If the equilibrium point u0=(x∗,0) of (2.1) is locally asymptotically stable when R0<1, the fixed point U0=(x∗,0) is a globally asymptotic stable equilibrium of (2.1) provided that R0<1 and that assumptions (H1) and(H2) are satisfied.
(H1) For dxdt=F(x,0), x∗ is globally asymptotically stable,
(H2) G(x,H)=AH−ˆG(x,H), ˆG(x,H)≥0 for (x,H)∈Ω, where A=DHG(x∗,0) is a M-matrix (the off diagonal elements of A are nonnegative) and Ω is the region where the model is biological sense.
Theorem 2.2. If R0<1, then the rumor-free equilibrium E0=(S0,I0,M0,R0) of system (2.1) is globally asymptotically stable.
Proof. Let x=(S,R),H=(I,M), u0=(S0,R0,I0,M0)=(x∗,0), where x∗=(bb+d,0), then
When S(t) is equal to bb+d=S0, R(t) is equal to 0, we can obtain F(x,0)=0. As t→+∞, there are S(t)→bb+d, R(t)→0. Hence x∗=(S0,0) is globally asymptotically stable.Thus the conditions (H1) is satisfied. And G(x,H)=AH−ˆG(x,H), where
According to the Lemma 2.1, it can be obtained ˆG(x,H)≥0, and A is a M-matrix; the conditions (H2) is satisfied, and by Lemma 2.2, the rumor-free equilibrium E0 is globally asymptotically stable if R0<1.
This means that when R0<1, there are no more rumors in the network after the system is stabilized, i.e., the rumors disappear.
Theorem 2.3. Let R0>1, the rumor-prevailing equilibrium E1=(S1,I1,M1,R1) (2.10) of system (2.1) is asymptotically stable.
Proof. The Jacobian matrix of the model at E1(S1,I1,M1,R1) is given by
The characteristics equation of J(E1) is
where
Therefore, according to The Routh-Hurwitz criterion, when R0>1, the system (2.1) is asymptotically stable.When R0>1, the system stabilizes at the rumor-prevailing equilibrium point, i.e., rumors in the network will continue to spread.
3.
The optimal control modeling of the SIMR problem
In this section, based on the SIMR model, there are two different ways of spreading rumors: infection between friends and marketing account push. Under the premise of considering the cost of rumor control, this paper proposes the optimal control strategy for controlling the spread of rumors by controlling individual and marketing accounts. Consider Θ(t)=(θ1(t),θ2(t)),0≤t≤T as the control variable of the SIMR problem, where θ1(t),θ2(t) represents the control variable for the individual account and marketing account, respectively. The upper limit of this strategy is determined as shown in the following remarks:
Remark 3.1. Suppose the feasible region of Θ(t),0≤Θ≤¯Θ,t∈(0,T], where ¯Θ are the upper bounds of Θ. The upper bound ¯Θ is determined the budgeted costs of immunization rumors.
So we assume the SIMR strategy is
where L[0,T]2 represents the set of Lebesgue integrable functions defined on [0,T] [34]. In this paper, under the premise of controlling the cost of rumor, the expected cost effectiveness caused by rumor can be minimized. Assume that the loss caused by rumor in the network is J1, and the cost of rumor control in the network is J2. The following remark can be obtained.
Remark 3.2. Assuming that the unit loss caused by spreading rumors in individual accounts and marketing accounts per unit time is a constant c1 and c2, respectively. Then the total loss caused by the spreading of rumor in the time horizon [0,T] can be expressed as
Remark 3.3. Assuming that the unit cost of controlling individual accounts and marketing accounts per unit time is a constant c3 and c4, respectively. Then the total cost in the time horizon [0,T] can be expressed as
In summary, the expected cost effectiveness of rumor spread is
Based on the above description, the rumor immunity problem of the SIMR model is established as the following optimal control problem
In order to solve the optimal control problem, we adopted the optimal principle of Pontryagin [35] and define the Lagrangian and Hamilton function of the optimal control problem as follows:
where μ1,μ2,μ3,μ4 are the adjoint functions. We obtain the necessary conditions for optimal control of SIMR problems as follows.
Theorem 3.1. Suppose Θ(t)={θ1(t),θ2(t)} is an optimal control of the SIMR problem (3.4), E(t)=(S(t),I(t),M(t),R(t)) is the solution to the associated rumor spreading model (3.5). Then there exists an adjoint function μ(t)=(μ1(t),μ2(t),μ3(t),μ4(t)) such that the following equations hold.
We obtain the optimal control ˜Θ=(~θ1,~θ2) as follow:
where g1(θ1)=c3−λ1IS⟨k⟩μ2+μ4λ1IS⟨k⟩,g2(θ2)=c4−λ2MSμ2+μ4λ2MS.
Proof. According the Minimum principle of Pontryagin, there exists μ=(μ1,μ2,μ3,μ4) such that
By the optimal conditions, we have
According to the Pontryagin Minimum Principle, the optimal solution of the objective function in the time horizon [0,T] is Θ(t)=argmin˜θ∈ΘH(E(t),¯Θ,μ(t)).
4.
Numerical simulation and discussion
In this section, we introduce the synthetic scale-free network. The degree distribution of scale-free network follows the power law property with P(k)∼ak−3, n=500, and the constant a satisfis ∑nk=1P(k)=1. By a simple calculation, we can conclude that the average degree of the scale-free network structure ⟨k⟩=∑nk=1kP(k)=1.367. The initial conditions are given by S(0)=0.7, I(0)=0.2, M(0)=0.05, R(0)=0. To verify the accuracy of the model, we have conducted numerical computation using the Runge-Kutta method and MATLAB by setting parameter value in the system[36,37].
Example 1: Stabilities of the equilibrium points.
In this part, we will verify the impact on R0 on the stability of system (2.1). When the parameter reference value is the first row of Table 2, we calculate the basic reproduction number R0=0.41<1. When the parameter reference value is the second row in Table 2, we calculate the basic reproduction number R0=1.43>1. Figures 2(a), (b) indicate the stability of individual ratios when R0<1 and R0>1, respectively. Figure 2(c) shows that the stability of nodes density when R0<1 (R0=0.41 and R0=0.89).
According to Theorem 2.2, system (2.1) is globally asymptotically stable in E0. According to Theorem 2.3, system (2.1) is asymptotically stable in E1. Moreover, in the same case, we take R0<1 as an example, when R0 is larger, the transmission rate of infected individuals tending to 0 is slower. The density of the infected individuals and R0 has a positive linear relationship, and the greater the value of the R0, the greater the value of the density of the infected individuals. Finally, Figure 2 reveals the relative volatility of the early systems of rumor. As time goes by, the system tends to be stable, so controlling the rumors in the early stages often leads in better results.
Example 2: The effect of initial value on system stability.
Figures 3 and 4 indicate the influence of different initial S(0) and I(0) on rumor propagation results. The initial value is that the density of individual spreaders I(0) ranges from 0.05 to 0.45 and the density of susceptible S(0) ranges from 0.9 to 0.5, M(0)=0.05,R(0)=0. It can be seen that although the initial value of the system is different, the system (2.1) stabilized at the same value. In Figure 3, the infected individual is stable at 0, and in Figure 4 the system is stable at the rumor-prevailing equilibrium solution E1(S1,I1,M1,R1).
Figures 3 and 4 reveal that when the basic reproduction number R0 of the rumor system is fixed, the initial value (S(0),I(0),M(0),R(0)) of the individual in the network will not affect the stability of the rumor. Because the basic number of reproduction means the speed of rumor propagation, it is the decisive factor in the continued spread of rumors.
Example 3: The effect of parameters on rumor diffusion.
Figures 5–7 indicate the effect of the parameter λ1,λ2,γ,δ,β on rumor diffusion. Parameter λ1,λ2 respectively represents the conversion rate from susceptible individuals to infected individuals through the influence of friends and marketing accounts. Obviously, it can be seen from the Figures 5(a), (b) that the higher the value of the parameter, the higher the density of infection, and ultimately the higher the rate of infected individuals in the network.
Parameter γ represents the rate that the infected individual converted into a marketing account. It can be seen by Figures 6(a), (b) that both R0<1 or R0>1, the change of γ has little effect on rumors in the network.
Parameter δ and β respectively represent the rate that individual accounts and marketing accounts lose interest in rumors and thus become removed nodes. It can be seen from Figures 7(a), (b) that the rate of rumor spreading individuals decreases with the increase of parameters. In particular, when the parameter is small, the rate of rumor spreading individuals decreases significantly with the increase of parameter.
In conclusion, infected individuals increased with λ1 and λ2, and decreased with γ, δ, β. As can be seen from Figure 2, the relationship between the density of infected individuals and basic reproduction number R0 is a positive correlation. Then in the Example 4, the parameter sensitivity analysis on R0 is verified.
Example 4: Sensitivity analysis of parameter on R0.
In this part, we focus on the effect of parameters on R0. As can be seen by (2.8) and Figure 5, the density of infected individual increases when λ1 and λ2 increases. Figure 8 refers to the impact of λ1 and λ2 on the basic reproduction number R0 in the network of rumor spreading when the average degree ⟨k⟩ is different.
As can be seen from Figure 8, the larger the ⟨k⟩, the greater the number of friends in the network node, and the easier it is for rumors to spread among friends. This means that when the connection between nodes in the network is relatively small, the spread of rumors is more affected by marketing account push. On the contray, when the average degree of the networks is greater, rumors are easier to spread through friends. This is why marketing accounts encourage ordinary users to focus on their accounts.
Figure 9 shows that δ has the most significant effect on R0. Moreover, when δ is less than 0.4, the value of R0 decreases significantly as δ increase, and similarly, when β is less than 0.2, the value of R0 decreases significantly as β increases. Therefore, it is a very effective way to control rumors by enhancing the immune rate of friends accounts and marketing accounts.
Example 5: The effects of the optimal control strategies.
It can be concluded from Figure 9 that the spread of rumors can be effectively controlled by increasing the rate of rumor-infected individuals and marketing accounts converting to removed nodes. In this part, we discuss the control strategy of controlling rumors by suppressing individual accounts and marketing accounts. We simulated the effect of the rumor control strategy on three synthetic BA scale-free networks. The scale-free network with an average degree ⟨k⟩=2.04,4.06,7.92 were selected. The degree distribution of scale-free network is shown in Figure 10.
Figure 11 shows the comparison results of optimal control strategy and uniform control strategy under three networks with different average degree ⟨k⟩=2.04,4.06,7.92 when c1=100,c2=100, c3=10 and c4=10. The red line represents the value of J(Θpoc) and the black line represents the minimum value of J(Θp,q),p=0,0.1,...,1,q=0,0.1,...,1. It can be seen that the red function value is lower than the black function value. It is can conclude that Θpoc is superior to all the uniform control in three network in terms of the cost of rumor control.
Controlling cost changes in individual accounts and marketing accounts will have an impact on control. When ⟨k⟩=4.06, the changes of costs c3 and c4 are shown in Table 3, the corresponding value of J(minΘp,q) and J(Θpoc) are shown in Figure 12. In Figure 12, regardless of c3 and c4, the cost of optimal control is lower than the minimum cost of uniform control. And when c3=c4>40, the minimum value of uniform control remains unchanged, because when the control cost is too high, even using low-intensity control will greatly increase the total cost. Therefore, the minimum cost of unified control strategy is Θ0,0. On the contrary, the optimal control can dynamically adjust the control strategy according to the different cost of control.
Figure 13 shows that under optimal control, the density of rumor propagation nodes, including individual accounts and marketing accounts, decreases by varying degrees. As can be seen from Figure 13, the optimal control strategy of rumors significantly reduces the density of infected individuals, so as to achieve a satisfactory control effect. Combined with Figures 11–13, the optimal control strategy is an ideal strategy to control the density of infected individuals. On the other hand, the optimal control strategy is also the best choice from the perspective of economic benefits. This also provides a reference value for controlling rumors.
5.
Conclusions
In this paper, we have proposed a model of information propagation based on two kinds of propagation types. In the network, the state of the user was divided into S,I,M,R. Different from the traditional SIR Information communication model, it increased the marketing state, and could push information to non-friends. First, we calculated the basic reproduction number R0 by the method of the next generation matrix. In addition, we discussed the existence of the rumor-free equilibrium point and rumor-prevailing equilibrium point, and proved the globally asymptotically stability of the rumor-free equilibrium point when R0<1, and the asymptotically stability of rumor-prevailing equilibrium point when R0>1. More importantly, we proposed an optimal control strategy for rumors. Finally, the correctness of the above theory was verified by numerical simulation. Firstly, we verified the stability of the model and discussed the impact of initial value on the stability of the rumor. Secondly, we used sensitivity analysis to discuss the impact of parameters on R0 and draw two conclusions. On the one hand, the influence of λ1 on R0 increased with the increase of the average degree of the network. On the other hand, the rate δ of I to R was the most significant effect on R0. The rate β of I to R was the second effect of R0. Finally, based on the above conclusions, we proposed the optimal control strategy of the two kinds of immunity and verified the superiority of the optimal control strategy, and provided the reference for the control of rumors.
Acknowledgements
This work is supported by the National Natural Science Foundation of China under Grant Nos. 61807028, 61772449 and 61802332. The authors are grateful to valuable comments and suggestions of the reviewers.
Conflict of interest
The authors declare no potential conflict of interests.









 DownLoad:
DownLoad:














