Partially doubly strictly diagonally dominant matrices with applications

1.   Introduction

A matrix $A = [a_{ij}]\in \mathbb{C}^{n\times n}$ is called a strictly diagonally dominant (SDD) matrix if

for all $i\in N: = \{1, \ldots, n\}$, where $r_i(A) = \sum\limits_{j\in N\setminus\{i\}} |a_{ij}|$.

The concept of SDD originated from the well-known Lévy-Desplanques Theorem ^[1], which states that if condition (1.1) holds, then $A$ is nonsingular, i.e., SDD matrices are nonsingular. It is well known that the class of SDD matrices has wide applications in many fields of scientific computing, such as the Schur complement problem ^[2,3], eigenvalue localizations ^{[4,5,6,7,8,9,10]}, convergence analysis of the parallel-in-time iterative method ^[11], estimating the infinity norm for the inverse of $H$-matrices ^{[12,13,14,15]}, error bound for linear complementarity problems ^[16,17], structure tensors ^[18,19], etc.

Some well-known matrices have been presented and studied ^[4,7,20] by breaking the diagonal dominance condition (1.1). For instance, by allowing at most one row to be non-SDD, Ostrowski introduced the class of Ostrowski matrices ^[20] (also known as doubly strictly diagonally dominant (DSDD) matrices). Here, a matrix $A = [a_{ij}]\in \mathbb{C}^{n\times n}$ is an Ostrowski matrix ^[20] if for all $j\neq i$,

In addition, based on the partition-based approach that allows more than one row to be non-SDD, a well-known class of matrices called $S$-SDD matrices has been proposed and studied ^[7,21].

Definition 1.1. ^[7,21] Let $A = [a_{ij}]\in \mathbb{C}^{n\times n}$ and $S$ be a nonempty proper subset of $N$. Then, a matrix $A$ is called an $S$-SDD matrix if

where $r_i^S(A) = \sum\limits_{j\in S\setminus \{i\}}|a_{ij}|$.

Besides DSDD matrices and $S$-SDD matrices, there are many generalizations of SDD matrices, such as Nekrasov matrices ^[22,23], $DZ$-type matrices ^[24], CKV-type matrices ^[25] and so on.

Observe from Definition 1.1 that $S$-SDD matrices only consider the effect of "interaction" between $i\in S$ and $j\in \overline{S}$ on the nonsingular. However, other "interaction, " such as the "constraint condition" between $i\in S$ ($i\in \overline{S}$) and $j\in S$ ($j\in \overline{S}$) with $i\neq j$, might also affect the non-singularity of the matrix. Naturally, an interesting question arises: When we consider this "constraint condition, " can we get the non-singularity of the matrix? To answer this question, in this paper, we introduce a new class of nonsingular matrices arising from this "constraint condition" and show several benefits from this new class of matrices, which we will call partially doubly strictly diagonally dominant matrices.

This paper is organized as follows. In Section 2, we present a new class of matrices called PDSDD matrices and prove that it is a subclass of nonsingular $H$-matrices, which is similar to, but different from, the class of $S$-SDD matrices. Section 3 gives a new eigenvalue localization set for matrices and presents an infinity norm bound for the inverse of PDSDD matrices. It is proved that the obtained bound is better than the well-known Varah's bound for SDD matrices. Based on this infinity norm bound, we also obtain a new pseudospectra localization for matrices and apply it to measure the distance to instability. Finally, we give concluding remarks in Section 4.

2.   Partially doubly strictly diagonally dominant matrices

We start with some preliminaries and definitions. Let $\mathbb{Z}^{n\times n}$ be the set of all matrices $A = [a_{ij}]\in \mathbb{R}^{n\times n}$ with $a_{ij}\leq 0$ and $i\neq j$. A matrix $A\in\mathbb{Z}^{n\times n}$ is called a nonsingular $M$-matrix if its inverse is nonnegative, i.e., $A^{-1}\geq 0$ ^[26]. A matrix $A = [a_{ij}]\in \mathbb{C}^{n\times n}$ is called a nonsingular $H$-matrix ^[26] if its comparison matrix $\mathcal{M}(A) = [m_{ij}]\in \mathbb{R}^{n\times n}$ defined by

is a nonsingular $M$-matrix. Let $|N|$ be the cardinality of set $N$.

In the following, we define a new class of matrices called PDSDD matrices.

Definition 2.1. Let $S$ be a subset of $N$ and $\overline{S}$ be the complement of $S$. Given a matrix $A = [a_{ij}]\in \mathbb{C}^{n\times n}$, for each subset $\Delta \in \{S, \overline{S}\}$, denote $\Delta_{-}: = \{i\in \Delta|a_{ii}\leq r_i(A)\}$ and $\Delta_{+}: = \{i\in \Delta|a_{ii} > r_i(A)\}$. Then, a matrix $A$ is called a partially doubly strictly diagonally dominant (PDSDD) matrix if for each $\Delta \in \{S, \overline{S}\}$ either $|\Delta_{-}| = 0$ or $|\Delta_{-}| = 1$, and for $i\in \Delta_{-}$,

where $r_i^\Delta(A) = \sum\limits_{j\in \Delta\setminus \{i\}}|a_{ij}|$.

Note that the class of DSDD matrices allows at most one row to be non-SDD, whereas the class of matrices defined in Definition 2.1 allows at most one row to be non-SDD in each subset $\Delta\in \{S, \overline{S}\}$. For this reason, we call it the class of PDSDD matrices.

The following theorem provides that the class of PDSDD matrices is a subclass of nonsingular $H$-matrices.

Theorem 2.1. Every PDSDD matrix is a nonsingular $H$-matrix.

Proof. According to the well-known result that SDD matrices are nonsingular $H$-matrices, it is sufficient to consider the case that $A$ has one or two non-SDD rows. Assume, on the contrary, that $A$ is a singular matrix, and then there exists a nonzero eigenvector ${\bf x} = [x_1, x_2, \ldots, x_n]^T$ corresponding to 0 eigenvalue such that

Let $|x_p|: = \max\limits_{i\in N}\{|x_i|\}$. Then, $|x_p| > 0$, and $p\in \Delta\cup \overline{\Delta}$, where $\Delta\in \{S, \overline{S}\}$. Without loss of generality, we assume that $\Delta = S$. Next, we need only consider the case $p\in S$, and another case $p\in \overline{S}$ can be proved similarly. For $p\in S$, it follows from Definition 2.1 that $p\in S_{-}\cup S_{+}$, where $S_{-}: = \{i\in S|a_{ii}\leq r_i(A)\}$ and $S_{+}: = \{i\in S|a_{ii} > r_i(A)\}$.

If $p\in S_{-}$, then by Definition 2.1 we have

and for all $j\in S_{+}$,

Note that $|S_{-}| = 1$. Then, $S\setminus \{p\} = S_+$. Let $|x_q|: = \max\limits_{k\in S\setminus \{p\}}\{|x_k|\}$. Considering the $p$-th equality of (2.2), we have

Taking the modulus in the above equation and using the triangle inequality yields

which implies that

If $|x_{q}| = 0$, then $|a_{pp}|-r_p^{\overline{S}}(A)\leq0$ as $|x_p| > 0$, which contradicts (2.3). If $|x_{q}|\neq0$, then from the $q$-th equality of (2.2), we obtain

i.e.,

Multiplying (2.6) with (2.5) and dividing by $|x_p||x_{q}| > 0$, we have

which contradicts (2.4).

If $p\in S_{+}$, then $|a_{pp}| > r_p(A)$. Considering the $p$-th equality of (2.2) and using the triangle inequality, we obtain

i.e., $|a_{pp}|\leq r_p(A)$, which contradicts $|a_{pp}| > r_p(A)$. Hence, we can conclude that 0 is not an eigenvalue of $A$, that is, $A$ is a nonsingular matrix.

We next prove that $A$ is a nonsingular $H$-matrix. For any $\varepsilon\geq 0$, let

Note that $b_{ii} = |a_{ii}|+\varepsilon$, $b_{ij} = -|a_{ij}|$, and $B_\varepsilon\in \mathbb{Z}^{n\times n}$. Then, $\Delta_{-}(B_\varepsilon)\subseteq \Delta_{-}(A)$, and $\Delta_{+}(A)\subseteq\Delta_{+}(B_\varepsilon)$. If $A$ is an SDD matrix, then $\Delta_{-}(A) = \emptyset$, and thus $\Delta_{-}(B_\varepsilon) = \emptyset$, which implies that $B_\varepsilon$ is an SDD matrix. If $A$ is not an SDD matrix, then $|\Delta_{-}(A)| = 1$ for some $\Delta\in \{S, \overline{S}\}$. For this case, $|\Delta_{-}(B_\varepsilon)| = 0$ or $|\Delta_{-}(B_\varepsilon)| = 1$. If $|\Delta_{-}(B_\varepsilon)| = 0$ for each $\Delta\in \{S, \overline{S}\}$, then $B_\varepsilon$ is also an SDD matrix. If $|\Delta_{-}(B_\varepsilon)| = 1$ for some $\Delta\in \{S, \overline{S}\}$, then $\Delta_{-}(B_\varepsilon) = \Delta_{-}(A)$ and $\Delta_{+}(B_\varepsilon) = \Delta_{+}(A)$. Hence, for $i\in \Delta_{-}(B_\varepsilon)$,

and for all $j\in \Delta_{+}(B_\varepsilon)$,

Therefore, $B_\varepsilon$ is a PDSDD matrix and thus nonsingular for each $\varepsilon\geq 0$. This implies that $\mathcal{M}(A)$ is a nonsingular $M$-matrix (see the condition ($D_{15}$) of Theorem 2.3 in [26, Chapter 6]). Therefore, $A$ is a nonsingular $H$-matrix. The proof is complete.

Proposition 2.1. Every DSDD matrix is a PDSDD matrix.

Proof. If $A$ is an SDD matrix, then $A$ is a PDSDD matrix. If $A$ is not an SDD matrix, then by the assumptions it holds that there exists $i_0\in N$ such that $|a_{i_0i_0}|\leq r_{i_0}(A)$ and

Taking $S = N$, we have $r_i^{\overline{S}}(A) = 0$ and $r_i^{S}(A) = r_i(A)$ for all $i\in N$. This implies that for all $j\in S\setminus \{i_0\}$,

Hence, by Definition 2.1, we conclude that $A$ is a PDSDD matrix. The proof is complete.

Next, we give an example to show that neither PDSDD matrices nor $S$-SDD matrices are included in each other.

Example 2.1. Consider the following matrices:

By calculation, we know that $A$ is a PDSDD matrix for $S = \{1, 3\}$, but it is not an $S$-SDD matrix for any nonempty proper subset $S$ of $N$ and thus not a DSDD matrix. Meanwhile, $B$ is an $S$-SDD matrix for $S = \{1, 2, 3\}$, but it is not a PDSDD matrix, because $B$ has three non-SDD rows.

According to Theorem 2.1, Proposition 2.1 and Example 2.1, the relations among DSDD matrices, PDSDD matrices, $S$-SDD matrices and $H$-matrices can be depicted as follows:

and

3.   Some possible applications

3.1.   A new eigenvalue localization set for matrices

It is well known that the non-singularity of matrices can generate the equivalent eigenvalue inclusion set in the complex plane ^[4,5,7,9,24]. By the non-singularity of PDSDD matrices, we in this section give a new eigenvalue localization set for matrices. Before that, an equivalent condition for the definition of PDSDD matrices is given, which can be proved immediately from Definition 2.1.

Lemma 3.1. Let $A = [a_{ij}]\in \mathbb{C}^{n\times n}$ and $\{S, \overline{S}\}$ be a partition of the set $N$. A matrix $A$ is called a PDSDD matrix if and only if $S^\star$ is not empty unless $S$ is empty, and $\overline{S}^\star$ is not empty unless $\overline{S}$ is empty, where

and

with $r_i^S(A) = \sum\limits_{j\in S\setminus \{i\}}|a_{ij}|$.

By Lemma 3.1, we can obtain the following theorem.

Theorem 3.1. Let $A = [a_{ij}] \in \mathbb{C}^{n\times n}$ and $S$ be any subset of $N$. Then,

where $\sigma(A)$ is the set of all the eigenvalues of $A$,

and

with

and

Proof. Without loss of generality, we assume that $S$ is a nonempty subset of $N$. For the case of $S = \emptyset$, we have $\Theta^{S}(A) = \theta^{\overline{S}}(A)$, and the conclusion can be proved similarly. Suppose, on the contrary, that there exists an eigenvalue $\lambda$ of $A$ such that $\lambda\notin\Theta^{S}(A)$, that is, $\lambda\notin\theta^{S}(A)$ and $\lambda\notin\theta^{\overline{S}}(A)$. For $\lambda\notin\theta^{S}(A)$, there exists an index $i\in S$ such that $\lambda\notin \Gamma_i^{\overline{S}}(A)$, and for all $j\in S\setminus \{i\}$, $\lambda\notin \Gamma_j(A)$ and $\lambda\notin \widehat{V}_{ij}^{\overline{S}}(A)$, that is, $|\lambda-a_{ii}| > r_i^{\overline{S}}(A)$, $|\lambda-a_{jj}| > r_j(A)$, and

Similarly, for $\lambda\notin\theta^{\overline{S}}(A)$, there exists an index $i\in \overline{S}$ such that $\lambda\notin \Gamma_i^{S}(A)$, and for all $j\in \overline{S}\setminus \{i\}$, $\lambda\notin \Gamma_j(A)$ and $\lambda\notin \widehat{V}_{ij}^{S}(A)$, that is, $|\lambda-a_{ii}| > r_i^{S}(A)$, $|\lambda-a_{jj}| > r_j(A)$, and

These imply that $S^{\star}(\lambda I-A)$ and $\overline{S}^{\star}(\lambda I-A)$ are not empty. It follows from Lemma 3.1 that $\lambda I-A$ is a PDSDD matrix. Then, by Theorem 2.1, $\lambda I-A$ is nonsingular, which contradicts that $\lambda$ is an eigenvalue of $A$. Hence, $\lambda\in\Theta^{S}(A)$. This completes the proof.

Remark 3.1. Take the intersection over all possible subsets $S$ of $N$, and we can get a satisfactory eigenvalue localization although it has more computation costs:

To compare our set $\Theta(A)$ with the Geršgorin disks $\Gamma(A)$ in ^[8], Brauer's ovals of Cassini $\mathcal{K}(A)$ in ^[27] and the Cvetković-Kostić-Varga eigenvalue localization set $\mathcal{C}(A)$ in ^[7], }let us recall the definitions of $\Gamma(A)$, $\mathcal{K}(A)$ and $\mathcal{C}(A)$ as follows.

Theorem 3.2. ^[8] Let $A = [a_{ij}]\in \mathbb{C}^{n \times n}$ and $\sigma(A)$ be the set of all eigenvalues of $A$. Then,

where $\Gamma_i(A) = \{ z \in \mathbb{C}:|{a_{ii}} - z| \le {r_i}(A) \}$.

Theorem 3.3. ^[27] Let $A = [a_{ij}]\in \mathbb{C}^{n \times n}$ and $\sigma(A)$ be the set of all eigenvalues of $A$. Then,

where $\mathcal{K}_{ij}(A) = \{ z \in \mathbb{C}:|{a_{ii}} - z||{a_{jj}} - z| \le {r_i}(A){r_j}(A)\}$.

Theorem 3.4. ^[7] Let $S$ be any nonempty proper subset of $N$, and $n\geq 2$. Then, for any $A = [a_{ij}] \in \mathbb{C}^{n\times n}$, all the eigenvalues of $A$ belong to set

and hence

where $\Gamma_i^S(A)$ is given by Theorem 3.1, and

Remark 3.2. Observe that the class of SDD matrices is a subclass of DSDD matrices, which is a subclass of of PDSDD matrices. Hence, the corresponding set $\Theta(A)$ will contain Brauer's ovals of Cassini $\mathcal{K}(A)$, which contains the Geršgorin disks $\Gamma(A)$, that is,

In addition, because PDSDD class and $S$-SDD class do not contain each other, it follows that the relation between $\Theta(A)$ and $\mathcal{C}(A)$ is

3.2.   An infinity norm bound for the inverse of PDSDD matrices

In this section, we consider the infinity norm bounds for the inverse of PDSDD matrices, since it might be used for the convergence analysis of matrix splitting and matrix multi-splitting iterative methods for solving large sparse systems of linear equations, linear complementarity problems and pseudospectra localizations.

Theorem 3.5. Let $A = [a_{ij}] \in \mathbb{C}^{n\times n}$ be a PDSDD matrix. Then,

where $S^\star$ and $\overline{S}^\star$ are defined by Lemma 3.1,

with

Proof. According to a well-known fact (see ^[10,30]),

for some ${\bf x}^{*} = [x_1^{*}, x_2^{*}, \ldots, x_n^{*}]^{T}$ such that $||{\bf x}||_{\infty} = 1$. Denote $|x_p^{*}| = ||{\bf x}||_{\infty} = 1$. It follows that

Consider the $p$-th row of $Ax^*$, and we have

Since $A$ is a PDSDD matrix, it follows from Lemma 3.1 that $S^\star$ is not empty unless $S$ is empty, and $\overline{S}^\star$ is not empty unless $\overline{S}$ is empty. We only consider the case both $S$ and $\overline{S}$ are not empty, and the case of $S = \emptyset$ or $\overline{S} = \emptyset$ can be proved similarly.

The first case. Suppose that either $S$ or $\overline{S}$ is a singleton set. We assume that $S = \{k\}$. Then, $\overline{S} = N\setminus\{k\}$, and from Lemma 3.1 it holds that $S^\star$ is not empty and $\overline{S}^\star$ is not empty, that is,

For each $i_0\in \overline{S}^\star$, $|a_{i_0i_0}| > r_{i_0}^{S}(A)$, and for all $j\in \overline{S}\setminus \{i_0\}$,

Note that $p\in S\cup \overline{S}$. If $p\in S = \{k\}$, then

and

Hence,

If $p\in \overline{S}$, then $p = i_0$ or $p\neq i_0$, where $i_0\in \overline{S}^\star$. If $p = i_0$, then let $|x_{q}^{*}| = \max\limits_{j\in \overline{S}\setminus\{p\}}\{|x_j^{*}|\}$. By (3.2), it follows that

and taking absolute values on both sides and using the triangle inequality, we get

which implies that

Consider the $q$-th row of $A{\bf x}^{*}$, and we have

It follows that

i.e.,

Then, from (3.5) and (3.6), we get that

which implies that

If $p\neq i_0$, then $|a_{pp}| > r_p(A)$. Similarly to the proof of (3.4), we obtain

Hence,

Since $i_0$ is arbitrary in $\overline{S}^\star$, it follows that

By (3.4) and (3.8), it holds that

If $\overline{S}$ is a singleton set, then similar to the above case, we obtain

The second case. Suppose that both $S$ and $\overline{S}$ are singleton sets. By Lemma 3.1, it follows that $S^\star$ is not empty, and $\overline{S}^\star$ is not empty. Then, similar to the proof of the first case, we have

and

Now, the conclusion follows from the above two cases.

In ^[15], an elegant upper bound for the inverse of SDD matrices is presented.

Theorem 3.6. ^[15] Let $A = [a_{ij}] \in \mathbb{C}^{n\times n}$ be an SDD matrix. Then,

This bound is usually called Varah's bound and plays a critical role in numerical algebra ^{[11,16,28,29]}. As discussed before, an SDD matrix is a PDSDD matrix as well. Thus, Theorem 3.5 can be applied to SDD matrices. In the following, we show that bound (3.1) of Theorem 3.5 works better than Varah's bound of Theorem 3.6.

Theorem 3.7. Let $A = [a_{ij}] \in \mathbb{C}^{n\times n}$ be an SDD matrix and $S$ be any subset of $N$. Then,

where

and $\varphi_i^{S}(A)$ is given by Theorem 3.5.

Proof. Since $A$ is an SDD matrix, it follows from Lemma 3.1 that $S^\star = S$ for any subset $S$ of $N$. Hence, by Theorem 3.5, it follows that

We next prove that $\varphi^{S}(A)\leq \max\limits_{i\in N}\frac{1}{|a_{ii}|-r_i(A)}$. Define $|a_{i_0i_0}|-r_{i_0}(A): = \min\limits_{i\in N}\{|a_{ii}|-r_{i}(A)\}$. Obviously, for each $i\in N$,

Note that for each $i\in S$,

and

If $|a_{ii}|-r_i(A)\geq|a_{jj}|-r_j(A)$, then

which implies that

If $|a_{ii}|-r_i(A) < |a_{jj}|-r_j(A)$, then

which implies that

By (3.9), (3.10) and (3.11), it follows that for each $i\in S$,

Similarly, for each $i\in \overline{S}$, we can see that

This completes the proof.

Remark 3.3. For SDD matrices, consider the intersection over all possible subsets $S$ of $N$, and a tighter upper bound for $||A^{-1}||_{\infty}$ can be obtained from Theorem 3.7:

Besides SDD matrices, for other subclasses of $H$-matrices, such as DSDD matrices, $S$-SDD matrices and Nekrasov matrices, various infinity norm bounds for their inverse have also been derived. For details, see ^{[12,23,28,29,30,31,32,33]} and references therein. A numerical example is given to illustrate the advantage of the proposed bound in Theorem 3.6.

Example 3.1. Consider the matrix in Example 2.1:

By computation, we know that $A$ is a PDSDD matrix for $S = \{1, 3\}$ but neither SDD nor a Nekrasov matrix. Moreover, it is easy to verify that there is no nonempty proper subset $S$ of $N$ such that $A$ is an $S$-SDD matrix, and so it is not a DSDD matrix. Therefore, neither of the existing bounds for SDD, DSDD, $S$-SDD, and Nekrasov matrices can be used to estimate $||A^{-1}||_\infty$. However, by our bound (3.1), we have

The exact value of the infinity norm of the inverse of $A$ is $||A^{-1}||_\infty = 1.$

3.3.   A new pseudospectra localization and its application

For a given $\varepsilon > 0$, denoted by

the $\varepsilon$-pseudospectrum of a matrix $A$ consists of all eigenvalues of matrices ^[30], which is equivalent to

where the convention is $||A^{-1}||^{-1} = 0$ if $A$ is singular ^[30]. This implies that the infinity norm bounds of the inverse of a given matrix could be used to generate new pseudospectra localizations. For details, see ^[13,25,30]. So, in this section, we shall give a new pseudospectra localization using the obtained bound in Section 3.2. Before that, a useful lemma is given that will be used later.

Lemma 3.2. Let $A$ be an arbitrary matrix and $S$ be any subset of $N$. Then,

where

and

with the convention $||A^{-1}||_{\infty}^{-1}$ if $A$ is singular, and $\mu_{ij}^{S}(A) = 0$ if $\; |a_{jj}|-r_j^{S}(A)+|a_{ji}|+r_i^{S}(A) = 0$; otherwise,

Proof. For any given subset $S$ of $N$, if $A$ is a PDSDD matrix, then it follows from Lemma 3.1 and Theorem 3.5 that

If $A$ is not a PDSDD matrix, then it follows from Lemma 3.1 that at least one of the following conditions holds: ($i$) $|a_{ii}|\leq r_i^{\overline{S}}(A)$ for all $i\in S$; ($ii$) $|a_{ii}| > r_i^{\overline{S}}(A)$ for some $i\in S$; but for some $j\in S\setminus \{i\}$, $|a_{jj}|\leq r_j(A)$ or

($iii$) $|a_{ii}|\leq r_i^{S}(A)$ for all $i\in \overline{S}$; ($iv$) $|a_{ii}| > r_i^{S}(A)$ for some $i\in \overline{S}$; but for some $j\in \overline{S}\setminus \{i\}$, $|a_{jj}|\leq r_j(A)$ or

This implies that $\mu(A)\leq 0\leq ||A^{-1}||_{\infty}^{-1}$. The proof is complete.

Now, a new pseudospectra localization for matrices is given based on Lemma 3.2.

Theorem 3.8. ($\varepsilon$-pseudo PDSDD set) Let $A = [a_{ij}] \in \mathbb{C}^{n\times n}$ and $S$ be any subset of $N$. Then,

where

and

with

and

Proof. From Lemma 3.2 and (3.12), we immediately get

where $\mu(A-zI)$ is defined as in Lemma 3.2. Note that $r_i^S(A-zI) = r_i^S(A)$ and $r_i^{\overline{S}}(A-zI) = r_i^{\overline{S}}(A)$. Therefore, for any $\lambda\in \Lambda_\varepsilon(A)$, it follows from (3.13) that

where $f(A-\lambda I)$ and $g(A-\lambda I)$ are given by Lemma 3.2.

Case Ⅰ. If $f(A-\lambda I)\leq \varepsilon$, then for all $i\in S$, $|a_{ii}-\lambda|\leq r_i^{\overline{S}}(A)+\varepsilon$ or $|a_{ii}-\lambda| > r_i^{\overline{S}}(A)+\varepsilon$ for some $i\in S$; but for some $j\in S\setminus \{i\}$, $|a_{jj}-\lambda|\leq r_j(A)+\varepsilon$ or $\mu_{ij}^{S}(A-\lambda I)\leq\varepsilon$, i.e.,

If $|a_{jj}-\lambda|-r_j^{S}(A) > 0$, then it follows from (3.14) that

If $|a_{jj}-\lambda|-r_j^{S}(A)\leq0$, then $|a_{jj}-\lambda|\leq r_j^{S}(A)+\varepsilon\leq r_j(A)+\varepsilon$. These imply that $\lambda\in\theta^{S}(A, \varepsilon)$.

Case Ⅱ. If $g(A-\lambda I)\leq \varepsilon$, then for all $i\in \overline{S}$, $|a_{ii}-\lambda|\leq r_i^S(A)+\varepsilon$ or $|a_{ii}-\lambda| > r_i^{S}(A)+\varepsilon$ for some $i\in \overline{S}$; but for some $j\in \overline{S}\setminus \{i\}$, $|a_{jj}-\lambda|\leq r_j(A)+\varepsilon$ or $\mu_{ij}^{\overline{S}}(A-\lambda I)\leq\varepsilon$, i.e.,

If $|a_{jj}-\lambda|-r_j^{\overline{S}}(A) > 0$, then it follows from (3.15) that

If $|a_{jj}-\lambda|-r_j^{\overline{S}}(A)\leq0$, then $|a_{jj}-\lambda|\leq r_j^{\overline{S}}(A)+\varepsilon\leq r_j(A)+\varepsilon$. These imply that $\lambda\in\theta^{\overline{S}}(A, \varepsilon)$.

From Case Ⅰ and Case Ⅱ, the conclusion follows.

As an application, using Theorem 3.8, we next give a lower bound for distance to instability. Denote by $Red(A)\in \mathbb{R}^{n\times n}$ the real matrix associated with a given matrix $A = [a_{ij}]\in \mathbb{C}^{n\times n}$ in the following way:

Theorem 3.9. Consider $A = [a_{ij}]\in \mathbb{C}^{n\times n}$, such that $Red(A)$ is a PDSDD matrix with all diagonal elements negative. Then, $\mu(\mathit{\text{Red}}(A)) > 0$ and

where $\mathbb{C}^{-}$ is the open left half plane of $\mathbb{C}$, $\mu(\mathit{\text{Red}}(A))$ is defined as in Lemma 3.2, and $\Lambda_\varepsilon(A)$ denotes the infinity norm $\varepsilon$-pseudospectrum of $A$.

Proof. Since $Red(A)$ is a PDSDD matrix, it follows from Lemma 3.2 that $\mu(\text{Red}(A)) > 0$. To prove (3.16), for $0 < \varepsilon < \mu(Red(A))$, it suffices to show that $Re(z) < 0$ for each $z\in \Theta^{S}(A, \varepsilon)$. It follows from Theorem 3.8 that $z\in \theta^{S}(A, \varepsilon)$ or $z\in \theta^{\overline{S}}(A, \varepsilon)$. We only consider the case of $z\in \theta^{S}(A, \varepsilon)$, and the case of $z\in \theta^{\overline{S}}(A, \varepsilon)$ can be proved similarly. Since $z\in \theta^{S}(A, \varepsilon)$, it follows that for all $i\in S$, either ($i$) $|a_{ii}-z|\leq r_i^{\overline{S}}(A)+\varepsilon$ or ($ii$) $|a_{ii}-z| > r_i^{\overline{S}}(A)+\varepsilon$ for some $i\in S$; but for some $j\in S\setminus \{i\}$, $|a_{jj}-z|\leq r_j(A)+\varepsilon$ or

Note that

where

and

with $\mu_{ij}^{S}(Red(A))$ defined as in Lemma 3.2.

For case ($i$), i.e., $|a_{ii}-z|\leq r_i^{\overline{S}}(A)+\varepsilon$ for all $i\in S$, we have

which implies that $Re(z) < 0$.

For case ($ii$), i.e., $|z-a_{ii}| > r_i^{\overline{S}}(A)+\varepsilon$ for some $i\in S$, if $|a_{jj}-z|\leq r_j(A)+\varepsilon$, then

which implies that $Re(z) < 0$. If (3.17) holds, then

{If $|z-a_{ii}| < |Re(a_{ii})|$, then $Re(z)-Re(a_{ii})\leq|z-a_{ii}| < |Re(a_{ii})|$, which leads to $Re(z) < 0$. Otherwise, since}

it follows that

which together with (3.18) yields that

and thus $Re(z) < 0$. This completes the proof.

The following example shows that the bound $\mu(Red(A))$ in Theorem 3.9 is better than those of ^[13] and ^[30] in some cases.

Example 3.2. Consider the matrix $A\in \mathbb{R}^{10\times 10}$ in ^[13], where

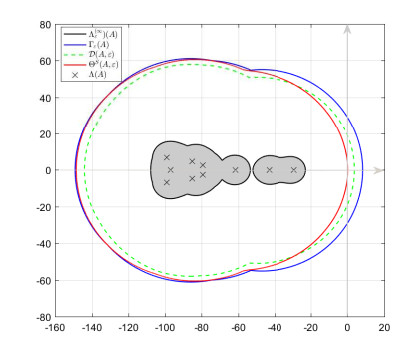
It follows from ^[13] that $A$ is a DZ-type matrix, and $\varepsilon = \mu(Red(A)) = 0.66$. On the other hand, it is easy to verify that $A$ is also a PDSDD matrix for $S = \{1, 2, 3, 4, 9\}$. Hence, from Theorem 3.9, we can get a new lower bound for distance to instability $\varepsilon = \mu(Red(A)) = 4.17$ and plot the corresponding pseudospectrum as shown in Figure 1, where $\Gamma _\varepsilon(A)$, $\mathcal{D}(A, \varepsilon)$, $\Theta^{S}(A, \varepsilon)$, the pseudospectrum ${\Lambda _\varepsilon }(A)$ and the eigenvalues of $A$ are represented by a blue solid boundary, a green dotted boundary, a red solid boundary, a gray area, and a black "$\times$, " respectively.

As can be seen from Figure 1, the sets ${\Gamma _\varepsilon }(A)$ of ^[30] and $\mathcal{D}(A, \varepsilon)$ of ^[13] propagate far into the right half-plane of $\mathbb{C}$, but the localization set $\Theta^{S}(A, \varepsilon)$ touches the $y$-axis. This implies that we cannot use ${\Gamma _\varepsilon }(A)$ and $\mathcal{D}(A, \varepsilon)$ to determine the stability of $A$. However, using the localization set $\Theta^{S}(A, \varepsilon)$, we can determine that $A$ is a stable matrix.

4.   Conclusions

This paper proposes a new class of nonsingular $H$-matrices called PDSDD matrices, which is similar to but different from $S$-SDD matrices. By its non-singularity, a new eigenvalue localization set for matrices is presented, which improves some existing results in ^[8] and ^[27]. Furthermore, an infinity norm bound for the inverse of PDSDD matrices is obtained, which improves the well-known Varah's bound for strictly diagonally dominant matrices. Meanwhile, utilizing the proposed infinity norm bound, a new pseudospectra localization for matrices is given, and a lower bound for distance to instability is provided as well. In addition, applying the proposed infinity norm bound to explore the error bounds for linear complementarity problems of PDSDD matrices is also an interesting problem. It is worth studying in the future.

Acknowledgments

The authors would like to thank the editor and the anonymous referees for their valuable suggestions and comments. This work was partly supported by the National Natural Science Foundations of China (61962059 and 31600299), the Young Science and Technology Nova Program of Shaanxi Province (2022KJXX-01), the Science and Technology Project of Yan'an (2022SLGYGG-007), the Scientific Research Program Funded by Yunnan Provincial Education Department (2022J0949).

Conflict of interest

The authors declare there are no conflicts of interest.