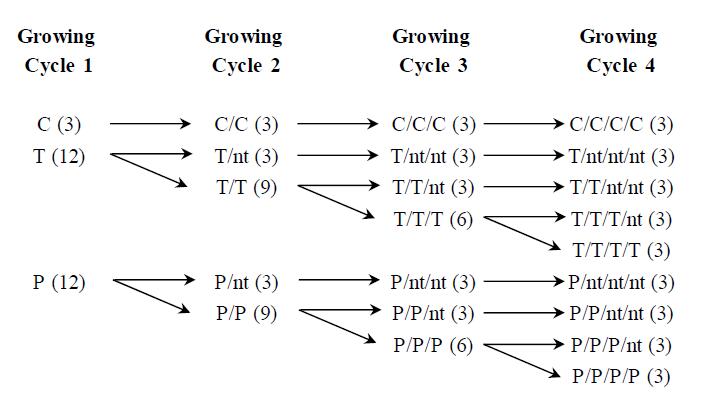
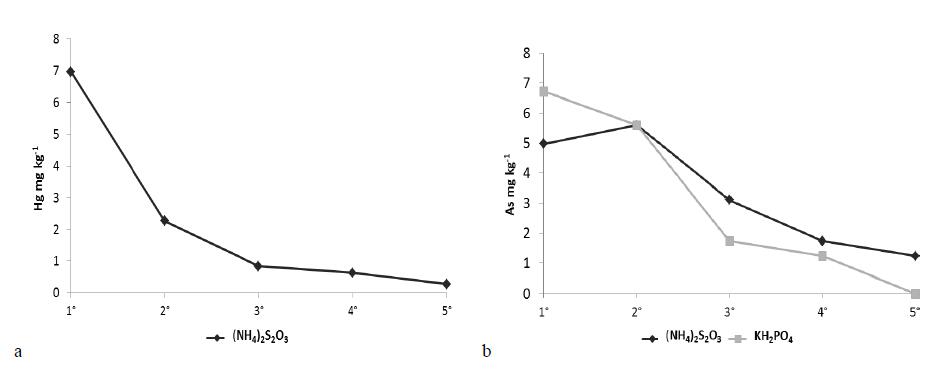
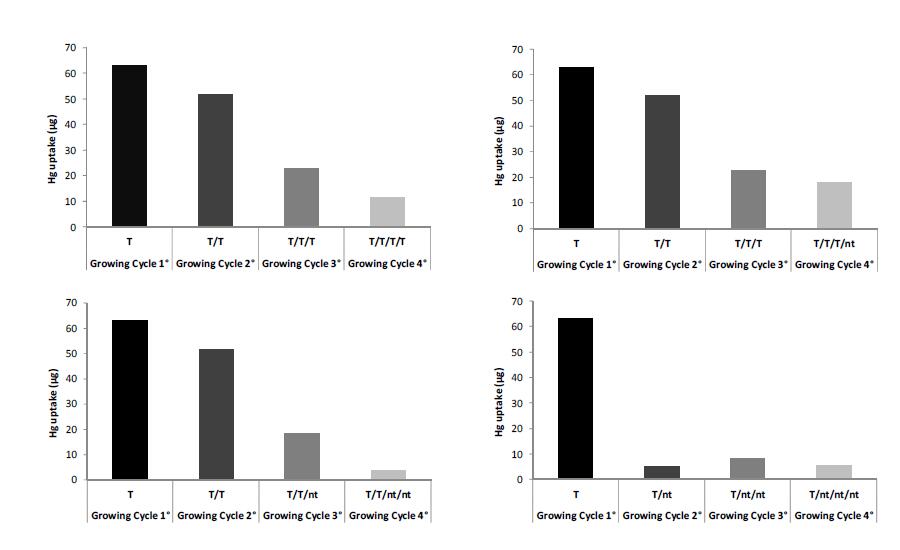
Mercury is widely distributed throughout the environment. In many contaminated soils other contaminants are present along with mercury; of these, arsenic is one of the most frequently found metals. In the presence of mixed contamination of this kind, remediation technologies must overcome many difficulties due to the different chemical characteristics of the various contaminants. In this study, repeated assisted phytoextraction cycles with Brassica juncea, were conducted on a laboratory scale to evaluate the removal efficiency of mercury and arsenic from a multi-contaminated industrial soil. The possibility of using only one additive, ammonium thiosulphate, to remove mercury and arsenic from co-contaminated soil simultaneously was also investigated. The thiosulfate addition greatly promoted the plant uptake of both contaminants, with an efficiency comparable to that of phosphate specifically used to mobilize specifically arsenic. Repeated additions of mobilizing agents increased metal availability in soil, promoted plant uptake and consequently increased the removal of contaminants in the studied soil.
Repeated treatments with thiosulfate increased the concentration of mercury and arsenic in the Brassica juncea aerial part, but due to toxic effects of mercury that reduce biomass production, the total accumulation of both metals in plants tended to decrease at each subsequent re-growth.
The use of a single additive to remove both contaminants simultaneously offers several new advantages to phytoextraction technology in terms of reducing cost and time.
1.
Introduction
The fundamental work of Hansen and Spies [4] modeled a two-layer beam with a structural damping due to the interfacial slip through the following system
where φ=φ(x,t) is the transverse displacement, ψ=ψ(x,t) is the rotation angle, w=w(x,t) is proportional to the amount of slip along the interface, 3w−ψ denotes the effective rotation angle. The physical quantities ρ,Iρ,G,D,β and γ are respectively: the density, mass moment of inertia, shear stiffness, flexural rigidity, adhesive damping and adhesive stiffness. Equation (1.1)3 describes the dynamics of the slip. For β=0, system (1.1) describes the coupled laminated beams without structural damping at the interface. In the recent result [1], Apalara considered the thermoelastic-laminated beam system without structural damping, namely
where (x,t)∈(0,1)×(0,+∞), θ=θ(x,t) is the difference temperature. The positive quantities γ,β,k,λ are adhesive stiffness, adhesive damping, heat capacity and the diffusivity respectively. The author proved that (1.2) is exponential stable provided
When β>0, the adhesion at the interface supplies a restoring force proportion to the interfacial slip. But this is not enough to stabilize system (1.1), see for instance [2]. To achieve exponential or general stabilization of system (1.1), many authors in literature have used additional damping. In this direction, Gang et al. [9] studied the following memory-type laminated beam system
and established a general decay result for more regular solutions and Gρ≠DIρ. Mustafa [15] also considered the structural damped laminated beam system (1.4) and established a general decay result provided Gρ=DIρ. Feng et al. [8] investigated the following laminated beam system
and established the well-posedness, smooth global attractor of finite fractal dimension as well as existence of generalized exponential attractors. See also, recent results by Enyi et al. [20]. We refer the reader to [5,6,7,11,13,14,17,18] and the references cited therein for more related results.
In this present paper, we consider a thermoelastic laminated beam problem with a viscoelastic damping
under initial conditions
and boundary conditions
In the system (1.6), the integral represents the viscoelastic damping, and g is the relaxation function satisfying some suitable assumptions specified in the next section. According to the Boltzmann Principle, the viscoelastic damping (see [21] for details) is represented by a memory term in the form of convolution. It acts as a damper to reduce the internal/external forces like the beam's weight, heavy loads, wind, etc., that cause undesirable vibrations.
In most of the above works, the authors have established their decay result by including the structural damping along with other dampings. So, the natural question that comes to mind.
Is it possible to obtain general/optimal decay result (decay rates that agrees with that of g) to the thermoelastic laminated beam system (1.6)–(1.8), in the absence of the structural damping.
The novelty of this article is to answer this question in a consenting way, by using the ideas developed in [10] to establish general and optimal decay results for Problem 1.6. Moreover, we establish a weaker decay result in the case of a non-equal wave of speed propagation. To the best of our knowledge, there is no stability result for the latter in the literature.
The rest of work is organized as follows: In Section 2, we recall some preliminaries and assumptions on the memory term. In Section 3, we state and prove the main stability result for the case equal-speed and in the case of non-equal-speed of propagation. We also give some examples to illustrate our findings. Finally, in Section 4, we give the proofs of the lemmas used our main results.
2.
Preliminaries
In this section, we recall some useful materials and conditions. Through out this paper, C is a positive constant that may change through lines, ⟨.,.⟩ and ‖.‖2 denote respectively the inner product and the norm in L2(0,1). We assume the relaxation function g obeys the assumptions:
(G1). g:[0,+∞)⟶(0,+∞) is a non-inecreasing C1− function such that
(G2). There exist a C1 function H:[0,+∞)→(0,+∞) which is linear or is strictly convex C2 function on (0,ϵ0), ϵ0≤g(0), with H(0)=H′(0)=0 and a positive nonincreasing differentiable function ξ:[0,+∞)→(0,+∞), such that
Remark 2.1. As in [10], we note here that, if H is a strictly increasing convex C2− function on (0,r], with H(0)=H′(0)=0, then H has an extension ˉH, which is strictly increasing and strictly convex C2-function on (0,+∞). For example, ˉH can be defined by
Let
The existence and regularity result of problem (1.6) is the following
Theorem 2.1. Let (w0,3s0−ψ0,s0,θ0)∈H1∗(0,1)×ˉH1∗(0,1)×ˉH1∗(0,1)×H1∗(0,1) and (w1,3s1−ψ1,s1)∈L2(0,1)×L2(0,1)×L2(0,1) be given. Suppose (G1) and (G2) hold. Then problem (1.6) has a unique global weak solution (w,3s−ψ,s,θ) which satisfies
Furthermore, if (w0,(3s0−ψ0),s0,θ0)∈H2∗(0,1)×ˉH2∗(0,1)×ˉH2∗(0,1)×H2(0,1)∩H1∗(0,1) and (w1,(3s1−ψ1),s1)∈H1∗(0,1)×ˉH1∗(0,1)×ˉH1∗(0,1), then the solution of (1.6) satisfies
The proof of Theorem 2.1 can be established using the Galerkin approximation method as in [16]. Throughout this paper, we denote by ⋄ the binary operator, defined by
We also define h(t) and Cα as follow
The following lemmas will be applied repeatedly throughout this paper
Lemma 2.1. For any function f∈L2loc([0,+∞),L2(0,1)), we have
Lemma 2.2. Let v∈H1∗(0,1) or ˉH1∗(0,1), we have
where Cp>0 is the poincaré constant.
Lemma 2.3. Let (w,3s−ψ,s,θ) be the solution of (1.6). Then, for any 0<α<1 we have
Proof. Using Cauchy-Schwarz inequality, we have
Lemma 2.4. [12] Let F be a convex function on the close interval [a,b], f,j:Ω→[a,b] be integrable functions on Ω, such that j(x)≥0 and ∫Ωj(x)dx=α1>0. Then, we have the following Jensen inequality
In particular if F(y)=y1p, y≥0, p>1, then
Lemma 2.5. The energy functional E(t) of the system (1.6)-(1.8) defined by
satisfies
Proof. Multiplying (1.6)1, (1.6)2, (1.6)3 and (1.6)4, respectively, by wt, (3st−ψt), st and θ, integrating over (0,1), and using integration by parts and the boundary conditions (1.7), we arrive at
and
Adding the equations (2.13)–(2.16), taking into account (G1) and (G2), we obtain (2.12) for regular solutions. The result remains valid for weak solutions by a density argument. This implies the energy functional is non-increasing and
3.
Stability results
This section is subdivided into two. In the first subsection, we prove the stability result for equal-wave-speed of propagation, whereas in the second subsection, we focus on the stability result for non-equal-wave-speed of propagation.
3.1. Equal-wave-speed of propagation
Our aim, in this subsection, is to prove an explicit, general and optimal decay rate of solutions for system (1.6)–(1.8). To achieve this, we define a Lyapunov functional
where N, Nj, j=1,2,3,4,5,6 are positive constants to be specified later and
where
The following lemma is very important in the proof of our stability result.
Lemma 3.1. Suppose Gρ=DIρ. Under suitable choice of t0,N, Nj, j=1,2,3,4,5,6, the Lyapunov functional L satisfies, along the solution of (1.6)−(1.8), the estimate
and the equivalence relation
holds for some β>0, α1, α2>0.
Proof. By virtue of assumption (3.1) and using h(t)=αg(t)−g′(t), it follows from Lemmas 2.5, 4.1-4.6 (see the Appendix for detailed derivations) that, for all t≥t0>0,
Now, we choose
and select N1 large enough such that
Next, we choose N6 large so that
Also, we select N2 large enough so that
After fixing N1,N2,N6, and letting ϵ3=μ12N3, we then select ϵ1,ϵ2, and δ4 very small such that
and select N3 large enough so that
Now, we note that αg2(s)h(s)=αg2(s)αg(s)−g′(s)<g(s); thus the dominated convergence theorem gives
Therefore, we can choose some 0<α0<1 such that for all 0<α≤α0,
Finally, we select N so large enough and take α=1N So that
Combination of (3.6) - (3.13) yields the estimate (3.2). The equivalent relation (3.3) can be obtain easily by using Young's, Cauchy-Schwarz, and Poincaré's inequalities.
Now, we state and prove our stability result for this subsection.
Theorem 3.1. Assume Gρ=DIρ and (G1) and (G2) hold. Then, there exist positive constants a1 and a2 such that the energy solution (2.11) satisfies
and H1 is a strictly decreasing and strictly convex function on (0,r], with limt→0H1(t)=+∞.
Proof. Using the fact that g and ξ are positive, non-increasing and continuous, and H is positive and continuous, we have that for all t∈[0,t0]
Thus for some constants a,b>0, we obtain
Therefore, for any t∈[0,t0], we get
and
From (2.12) and (3.15), it follows that
From (3.2) and (3.17), we have
Thus, we get
where L1=L+CE∼E by virtue of (3.3). To finish our proof, we distinct two cases:
Case 1: H(t) is linear. In this case, we multiply (3.18) by ξ(t), keeping in mind (2.12) and (G2), to get
Therefore
Since ξ is non-increasing and L1∼E, we have
Thus, from (3.20), we get for some positive constant α
Integrating (3.22) over (t0,t) and recalling (3.21), we obtain
Case 2: H(t) is nonlinear. In this case, we consider the functional L(t)=L(t)+I7(t). From (3.2) and Lemma 4.7 (see the Appendix), we obtain
where d>0 is a positive constant. Therefore,
Hence, we get
Using (3.24), we define p(t) by
where 0<η<1 so that
Moreover, we can assume p(t)>0 for all t≥t0; otherwise using (3.18), we obtain an exponential decay rate. We also define q(t) by
Then q(t)≤−CE′(t), ∀t≥t0. Now, we have that H is strictly convex on (0,r] (where r=g(t0)) and H(0)=0. Thus,
Using (3.26), condition (G2), (3.25), and Jensen's inequality, we get
where ˉH is the convex extention of H on (0,+∞) (see remark 2.1). From (3.27), we have
Therefore, (3.18) yields
For r0<r, we define L3(t) by
since L1∼E. From (3.28) and using the fact that
we obtain for all t≥t0
Let us consider the convex conjugate of ˉH denoted by ˉH∗ in the sense of Young (see [3] page 61-64). Thus,
and ˉH∗ satisfies the generalized Young inequality
Let A=ˉH′(r0E(t)E(0)) and B=ˉH−1(μz(t)ξ(t)), It follows from (2.12) and (3.29)-(3.31) that
Next, we multiply (3.32) by ξ(t) and recall that r0E(t)E(0)<r and
we arrive at
Let L4(t)=ξ(t)L3(t)+CE(t). Since L3∼E, it follows that
for some b0,b1>0. Thus (3.33) gives
We select r0<r small enough so that βE(0)−Cr0>0, we get
for some constant m>0 and H2(τ)=τH′(r0τ). We note here that
thus the strict convexity of H on (0,r], yields H2(τ)>0,H′2(τ)>0 on (0,r]. Let
From (3.34) and (3.35), we obtain
and
Integrating (3.37) over (t0,t), we arrive at
This implies
Using the properties of H, we see easily that H1 is strictly decreasing function on (0,r] and
Hence, (3.14) follows from (3.36) and (3.39). This completes the proof.
Remark 3.1. The stability result in (3.1) is general and optimal in the sense that it agrees with the decay rate of g, see [10], Remark 2.3.
Corollary 3.2. Suppose Gρ=DIρ, and (G1), and (G2) hold. Let the function H in (G2) be defined by
then the solution energy (2.11) satisfies
for some positive constants a2,a1 and C.
3.2. Nonequal-wave-speed of propagation
In this subsection, we establish another stability result in the case non-equal speeds of wave propagation. To achieve this, we consider a stronger solution of (1.6). Let (w,3s−ψ,s,θ) be the strong solution of problem (1.6)–(1.8), then differentiation of 1.6 with respect to t gives
where (x,t)∈(0,1)×(0,+∞) and (3s−ψ)xx(x,0)=(3s0−ψ0)xx. The modified energy functional associated to (3.42) is defined by
Lemma 3.2. Let (w,3s−ψ,s,θ) be the strong solution of problem (1.6)-(1.8). Then, the energy functional (3.43) satisfies, for all t≥0
and
Proof. The proof of (3.44) follows the same steps as in the proof of Lemma 2.5. From (3.44), it is obvious that
So, using Cauchy-Schwarz inequality, we obtain
This implies
Integrating (3.47) over (0,t) yields
Hence, (3.45) follows.
Remark 3.2. Using Young's inequality, we observe from (3.44) and (3.45) that
for some fixed positive constant c1. Similarly, we obtain
As in the case of equal-wave-speed of propagation, we define a Lyapunov functional
where ˜N, ~Nj, j=1,2,3,4,5,6, are positive constants to be specified later and
Lemma 3.3. Suppose Gρ≠DIρ. Then, under suitable choice of ˜N, ~Nj, j=1,2,3,4,5,6, the Lyapunov functional ˜L satisfies, along the solution of (1.6), the estimate
for some positive constants ˜β and c1.
Proof. Following the proof of Lemma 3.1, we end up with (3.52).
Lemma 3.4. Suppose assumptions (G1) and (G2) hold and the function H in (G2) is linear. Let (w,3s−ψ,s,θ) be the strong solution of problem (1.6)-(1.8). Then,
where c1 is a fixed positive constant.
Proof. Using (3.50) and the fact that ξ is decreasing, we have
Our stability result of this subsection is
Theorem 3.3. Assume (G1) and (G2) hold and Gρ≠DIρ. Then, there exist positive constants a1,a2 and t2>t0 such that the energy solution (2.11) satisfies
Proof. Case 1: H is linear. Multiplying (3.52) by ξ(t) and using (G1), we get
Using the fact that ξ non-increasing, we obtain
for some fixed positive constant c2. This implies
Integrating (3.56) over (t0,t), using the fact that E is non-increasing and the inequality (3.45), we arrive at
Thus, we have
Case II: H is nonlinear. First, we observe from (3.52) that
From (2.12), (3.16) and (3.50), we have for any t≥t0
where c2 is a fixed positive constant. Substituting (3.60) into (3.59), we obtain for any t≥t0
where c_3 is a fixed positive constant. Now, we define the functional \Phi by
Using (2.11), (2.12), (3.43) and (3.45), we easily get
This last inequality allows us to choose 0 < \sigma < 1 such that
Hence forth, we assume \Phi(t) > 0 , otherwise, we get immediately from (3.61)
Next, we define the functional \mu by
and observe that
where c_4 is a fixed positive constant. The fact that H is strictly convex and H(0) = 0 implies
Using assumption (G1) , (3.67), Jensen’s inequality and (3.64), we get for any t > t_0
where
and \bar{H} is the C^2- strictly increasing and convex extension of H on (0, +\infty). This implies
Thus, the inequality (3.61) becomes
Let \tilde{L}_1(t): = \tilde{L}(t)+C\left(E(t)+ E_1(t) \right). Then (3.70) becomes
For 0 < r_1 < r, we define the functional \tilde{L}_2 by
From (3.71) and the fact that
we obtain, for all t > t_0,
Let \bar{H}^{\ast} be the convex conjugate of \bar{H} as in (3.30) and let
Then, (3.30), (3.31) and (3.73) yield, for all t > t_0,
By selecting r_1 small enough so that (\tilde{\beta}E(0)-Cr_1) > 0 , we arrive at
for some positive constant \tilde{\beta}_2.
Now, multiplying (3.75) by \xi(t) and recalling that r_1\frac{E(t)}{E(0)} < r , we arrive at
Since \frac{r_1}{t-t_0}\longrightarrow 0 as t\longrightarrow \infty, there exists t_2 > t_0 such that \frac{r_1}{t-t_0} < r_1 , whenever t > t_2 . Using this fact and observing that H' strictly increasing, and E and \xi are non-decreasing, we get
Using (3.77), it follows from (3.76) that
where \tilde{L}_3 = (\xi\tilde{L}_2+CE+ CE_1) and c_5 > 0 is a constant. Using the non-increasing property of \xi, we have
Using the fact that E is non-increasing and H'' > 0 we conclude that the map
is non-increasing. Therefore, integrating (3.79) over (t_2, t) yields
Next, we multiply both sides of (3.80) by \frac{1}{t-t_0} , for t > t_2, we get
Since H' is strictly increasing, then H_2(\tau) = \tau H'(\tau) is a strictly increasing function. It follows from (3.81) that
for some positive constants a_1 and a_2. This completes the proof.
3.3. Examples
(1). Let g(t) = ae^{-bt}, \ t\geq 0, \ \ a, \ b > 0 are constants and a is chosen such that ( G_1) holds. Then
Therefore, from (3.14), the energy function (2.11) satisfies
Also, for H_2(\tau) = \tau , it follows from (3.55) that, there exists t_2 > 0 such that the energy function (2.11) satisfies
for some positive constant C.
(2). Let g(t) = ae^{-(1+t)^{b}}, \ t\geq 0, \ \ a > 0, \ 0 < b < 1 are constants and a is chosen such that ( G_1) holds. Then,
where \xi(t) = b(1+t)^{b-1} and H(t) = t. Thus, we get from (3.14) that
Likewise, for H_2(t) = t , then estimate (3.55) implies there exists t_2 > 0 such that the energy function (2.11) satisfies
for some positive constant C.
(3). Let g(t) = \frac{a}{(1+t)^{b}}, \ t\geq 0, \ \ a > 0, \ b > 1 are constants and a is chosen in such a way that ( G_1) holds. We have
where
Hence, we deduce from (3.41) that
Furthermore, for H_2(t) = qt^q , estimate (3.55) implies there exists t_2 > 0 such that the energy function (2.11) satisfies
for some positive constant C.
4.
Appendix
In this section, we prove the functionals L_i, i = 1\cdots8, used in the proof of our stability results.
Lemma 4.1. The functional I_1(t) satisfies, along the solution of (1.6)-(1.8), for all t\geq t_0 > 0 and for any \epsilon_1, \epsilon_2 > 0 , the estimate
where g_0 = \int_0^{t_0}g(\tau)d\tau\leq \int_0^{t}g(\tau)d\tau.
Proof. Differentiating I_1(t) , using (1.6)_2 and integrating by part, we have
where D(t) = \left(D-\int_0^t g(\tau)d\tau\right). Now, we estimate the terms on the right hand-side of (4.2). Exploiting Young's and Poincaré's inequalities, Lemmas 2.1-2.6 and performing similar computations as in (2.8), we have for any \epsilon_1 > 0 ,
and
Also, for \delta_1 > 0, we have
For the last term, we have
Combination of (4.2)-(4.6) lead to
Since g(0) > 0 and g is continuous. Thus for any t\geq t_0 > 0, we get
We select \delta_1 = \dfrac{I_{\rho}g_0}{2} to get (4.1).
Lemma 4.2. The functional I_2(t) satisfies, along the solution of (1.6)-(1.8) and for any \delta_4 > 0 , the estimate
Proof. Differentiation of I_2(t) , using (1.6)_1 and (1.6)_3 and integration by part, leads to
Applying Cauchy-Schwarz and Young's inequalities and (2.5), we get for any \delta_4 > 0,
This completes the proof.
Lemma 4.3. The functional I_3(t) satisfies, along the solution of (1.6)-(1.8) and for any \epsilon_3 > 0, the estimate
Proof. Differentiation of I_3 , using (1.6)_3 , (1.6)_4 and integration by parts, yields
Using Cauchy-Schwarz, Young's and Poincaré's inequalities together with Lemmas 2.1-2.6, we have
We choose \delta_2 = \dfrac{5I_{\rho}\delta}{2} to get (4.10).
Lemma 4.4. The functional I_4(t) satisfies, along the solution of (1.6)-(1.8) and for any \epsilon_4 > 0, the estimate
Proof. Using (1.6)_1 and integration by parts, we have
We note that w_x = -(\psi-w_x)-(3s-\psi)+3s to arrive at
It follows from Young's and Poincaré's inequalities that
This completes the proof.
Lemma 4.5. The functional I_5(t) satisfies, along the solution of (1.6)-(1.8) and for any 0 < \alpha < 1 , the estimate
Proof. Differentiating I_5 , using (1.6)_2 , we arrive at
Applying Lemmas 2.1-2.6, Cauchy-Schwarz, Young's and Poincaré's inequalities, we obtain any \delta_3 > 0
We select \delta_3 = \dfrac{l_0}{4} and obtain the desired result.
Lemma 4.6. The functional I_6(t) satisfies, along the solution of (1.6)-(1.8) and for any for any \epsilon_1 , the estimate
Proof. Differentiating I_6(t) , using (1.6)_1 and (1.6)_3 and integration by parts, we obtain
Young's and Poincaré's inequalities give
Substituting (4.16) into (4.15), we obtain (4.14). This completes the proof.
Lemma 4.7. The functional I_7(t) satisfies, along the solution of (1.6)-(1.8), the estimate
Proof. Differentiate I_7(t) and use the fact that J'(t) = -g(t) to get
Using Cauchy-Schwarz and (G1), we have
Thus, we get
Since J is decreasing (J'(t) = -g(t)\leq 0) , so J(t)\leq J(0) = D-l_0 . Hence, we arrive at
The next lemma is used only in the proof of the stability result for nonequal-wave-speed of propagation.
Lemma 4.8. Let (w, 3s-\psi, s, \theta) be the strong solution of problem (1.6). Then, for any positive numbers \sigma_1, \sigma_2, \sigma_3 , the functional I_8(t) satisfies
Proof. Differentiation of I_8 , using integration by part and the boundary condition give
We note that w_x = -(\psi-w_x)-(3s-\psi)+3s and from (1.6)_4, \ \lambda\theta_{xx} = k\theta_t +\delta s_{xt} . So, (4.22) becomes
Using Young's and Poincaré's inequalities, we have for any positive numbers \sigma_1, \sigma_2, \sigma_3 ,
Substituting (4.24) into (4.23), we obtain (4.21).
5.
Conclusions
In this paper, we have established a general and optimal stability estimates for a thermoelastic Laminated system, where the heat conduction is given by Fourier's Law and memory as the only source of damping. Our results are established under weaker conditions on the memory and physical parameters. From our results, we saw that the decay rate is faster provided the wave speeds of the first two equations of the system are equal (see (1.3)). A similar result was established recently in [19] when the heat conduction is given by Maxwell-Cattaneo's Law. An interesting case is when the kernel memory term is couple with the first or third equations in system (1.6). Our expectation is that the stability in both cases will depend on the speed of wave propagation.
Acknowledgments
The authors appreciate the continuous support of University of Hafr Al Batin, KFUPM and University of Sharjah. The first and second authors are supported by University of Hafr Al Batin under project #G-106-2020 . The third author is sponsored by KFUPM under project #S B181018.
Conflict of interest
The authors declare no conflict of interest














 DownLoad:
DownLoad: