Analysis of small oscillations of a pendulum partially filled with a viscoelastic fluid

1.   Introduction

Over the past few years, there has been a growing interest in the dynamic characteristics of viscoelastic fluids driven by the requirements of hydrodynamic engineering specifically in ensuring the safety of fluid transportation in tankers, aerospace and shipbuilding industries. This interest stems not only from practical needs but also from the mathematical analysis of the associated problem.

The investigation of a system comprising a rigid body containing an ideal or viscous liquid using functional analysis methods has been the focus of several studies which can be found in fundamental monographs ^[1,2,3]. Furthermore, certain cases of an ordinary viscous fluid (homogeneous or heterogeneous) partially or fully filling a fixed container have been studied and discussed in ^[4,5,6,7].

To date, researchers have developed various analytical and numerical approaches to investigate the behavior of different structures interacting with a viscoelastic fluid ^[8]. Simultaneously, the study of hydrodynamic problems related to small motions and normal oscillations of viscoelastic fluids in immovable containers has extensively utilized methods based on functional analysis and the theory of operators in abstract Hilbert spaces (e.g., ^{[9,10,11,12,13,14,15]}). Recently, the specific case of a pendulum fully filled with a viscoelastic fluid was examined in ^[16]. Furthermore, the case of two nonmixing fluids, with the lower one being viscoelastic and the upper one inviscid was investigated in ^[15]. On the other hand, piezoelectric materials are introduced to examine mechanical behaviors on the stability of pendulum in presence of an electric field. To describe this effect, simultaneous coupling equations with tensor parameters associated to mechanical behaviors and the electric field are used ^[17,18] (and references therein).

The objective of this study is to expand upon previous works ^[2,16,19] by investigating the more challenging case of a pendulum that is partially filled with a viscoelastic fluid. The investigation of a pendulum containing a viscoelastic fluid topped with a barotropic gas will be reserved for another work.

Once the linearized equations of system motion (pendulum-liquid) are derived, presupposing the liquid inside the cavity to be following the simpler Oldroyd's model for viscoelastic fluids we reformulate these equations as a variational problem and then as an operatorial problem involving bounded linear operators on an appropriate Hilbert space.

Finally, through the examination of the eigenvalues of the linear operator that describes the dynamics of the coupled system we demonstrate that under the aforementioned hypotheses the given equilibrium is stable, as all eigenvalues have a non-negative real part. Moreover, if the viscosity coefficient reaches a certain threshold, the spectrum exhibits three branches of eigenvalues with potential cluster points at $0$, $\beta$, and $\infty$. Among these eigenvalues, $\lambda = 0$ possesses infinite multiplicity, while $\lambda = \beta$ is not an eigenvalue. These three branches of eigenvalues correspond to frequencies associated with various types of waves.

It is argued that the presence of viscoelastic forces gives rise to novel physical effects that are not typically observed in an ordinary viscous incompressible fluid ^[2,19]. These effects are associated with the emergence of a distinct essential spectrum. Specifically, the existence of internal dissipative waves, whose decay rates are influenced by the viscoelastic parameter $\beta$ of the fluid is observed.

2.   The simpler Oldroyd model for a viscoelastic fluid

The studied system involves a viscoelastic fluid in which the tensor of viscous stresses $\sigma'$ and the doubled tensor of deformation velocities $\tau$ satisfy a specific differential equation ^[2]

Setting

with

where $\mu$ is the viscosity coefficient of the solvent, $\gamma$ is the inverse of the relaxation time and $k_0$ and $k_1$ are the total viscosity and elastic modulus of the viscoelastic fluid, respectively.

In the following, we set

It is supposed ^[2] that if the tensor of deformation velocity is equal to zero at the instant $t = 0$ then the same condition holds for the tensor of viscous stresses. Under this hypothesis the differential equation (2.1) can be replaced by an integral relation

Indeed, if $\Sigma'(p)$ and $\mathcal{T}(p)$ denote the Laplace transforms of $\sigma'$ and $\tau$, respectevely, we have

From the equation (2.5), noting that $\frac{1}{\gamma+p}\mathcal{T}(p)$ is a product of transformed we deduce

3.   Position of the problem

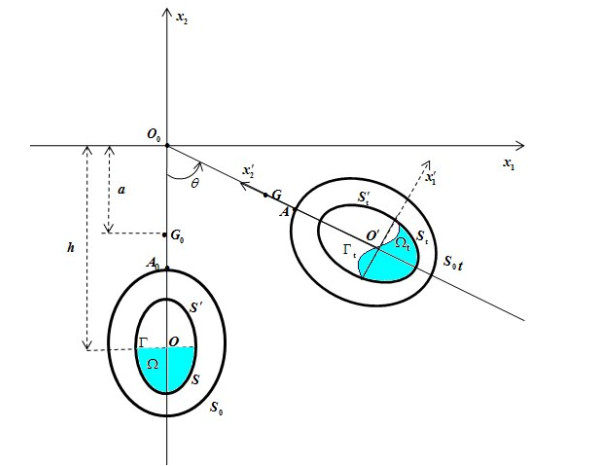
The pendulum under consideration consists of a rigid rod, denoted as $O_0A$ which is firmly attached at a point $A$ to a rigid body known as $O_0$. The rod is constrained to rotate around a fixed point $O_0$ referred to as the suspension point.

The body ($S_0$) is assumed to be two-dimensional and symmetric with respect to the axis that contains $O_0A$ and with center of mass $G$ on the rod $O_0A$ ($O_0G = a$). Within this body ($S_0$) there exists a symmetric cavity that is partially filled with a homogeneous incompressible viscoelastic fluid. The constitutive equation of the fluid is described by the simpler Oldroyd model ^[2].

In the lowest equilibrium position, $\Omega$, $S$, $\Gamma$ are respectively the domain occupied by the fluid, the wetted wall of the cavity and the horizontal free line [Figure 1]. $\Omega_t$, $S_t$, $\Gamma_t$ are their positions at the instant $t$, respectively. We call $h$ the distance of $O_0$ to $\Gamma$, $\theta$ the small angle of rotation of the pendulum.

We use the fixed orthogonal axes $O_0x_1x_2$ $(O_0x_2)$ directed vertically upwards and orthogonal axes $O'x'_1x'_2$ fixed to the pendulum, with $(O'x'_1)$ carrying out the position of $\Gamma$ at the instant $t$ are used [Figure 1]. The acceleration $\vec{g}$ of the gravity field is such that $\vec{g} = -g\vec{x}_2$.

The small oscillations of the system "pendulum - viscoelastic fluid" in linear theory are going to be studied.

4.   Equations of motion of the viscoelastic fluid

Let $\vec{u}(x_1, x_2, t)$ be the small displacement of a fluid particle $M$ with respect to the fixed axes $O_0x_1x_2$ and $\overrightarrow{U}(x_1, x_2, t)$ its displacement with respect to the mobile axes $O'x'_1x'_2$. Then,

4.1.   Navier Stokes equations

If $P(x_1, x_2, t)$ is the pressure then

If we denote by

the components of the tensor $\tau$, we have for the tensor $\sigma'$

Then,

The equation (4.2) takes the form, since $\Delta \vec{u} = \Delta \vec{U}$:

If it is supposed that the pressure is equal to zero above the free line, the pressure in the equilibrium position is

Introducing the dynamic pressure $p = P-P_{\text{st}}$, we obtain

and the equation (4.3) can be written

4.2.   Dynamic conditions on the free line $\Gamma_t$

We must add the dynamic conditions on the free line $\Gamma_t$ whose equation is

or

We have

or

where $\varepsilon_{ij} (i, j = 1, 2)$ is the rate of deformation tensor.

Or, in linear theory

and finally

The equations (4.10), (4.11), (4.4), (4.17), (4.18) are the equations of motion of the fluid.

4.3.   A new form of the precedent equations

Let $\vec{{\widetilde{U}}}$ a sufficiently smooth function defined in $\Omega$ and verifying $\text{div} \, \vec{{\widetilde{U}}} = 0$ and $\vec{{\widetilde{U}}}_{|S} = 0$. We have

The Green's formula and (4.11) (4.4) give

The vectorial Laplacian formula ^[1], and (4.4), (4.17) give

Therefore, we obtain

and using the dynamic condition (4.18)

The assumption is going to be made that $\vec{U}$ and $\vec{\widetilde{U}}$ belong to the space

equipped with the scalar product

(it is well-known that the associated norm is equivalent in $J_{0, S}^{1}(\Omega)$ to the classical norm of $\chi^{1}(\Omega)$ ^[1]).

We introduce the space

equipped with the scalar product (of $\mathscr{L}^2(\Omega)$) :

It is well-known ^[1] that the embedding from $\mathcal{V} \subset \chi$ is continuous, dense and compact. We denote by $A$ the unbounded operator of $\chi$ associated to the pair $\left(\mathcal{V}, \chi \right)$ and to the scalar product of $\left(\cdot, \cdot \right)_\mathcal{V}$.

4.4.   A few operators

In this section, a few operators are introduced.

4.4.1.   Definition and properties of the operator $L$

We set

$L$ being a compact operator from $\chi$ into $\mathbb{C}$.

We have

The adjoint $L^\ast$ is compact from $\mathbb{C}$ into $\chi$.

4.4.2.   Definition and properties of the operator $K$

Using a trace theorem in $\chi^1(\Omega)$, we can set

where $K$ is not negative, selfadjoint, bounded operator from $\mathcal{V}$ into $\mathcal{V}$.

Let us prove that $K$ is compact.

Indeed, let $\left\lbrace\vec{U^p} \right\rbrace$ a weakly convergent sequence in $\mathcal{V}$. By a trace theorem, the sequence $\left\lbrace U_{n|\Gamma}^p \right\rbrace$ is strongly convergent in $\widetilde{L}^2(\Gamma) = \left\lbrace f \in L^2(\Gamma), \; \; \int_\Gamma \, f \, \mathrm{d} \Gamma = 0 \right\rbrace$ and we have

consequently ^[20], $K$ is compact.

4.4.3.   Definition and properties of the operator $K_1$

Let

$K_1$ being compact from $\mathcal{V}$ into $\mathbb{C}$.

Then,

where the adjoint $K_1^\ast$ is compact from $\mathbb{C}$ into $\mathcal{V}$.

Consequently, the variational equation (4.23) can be written

for all $\vec{\widetilde{U}} \in \mathcal{V}$ and then by density for all $\vec{\widetilde{U}} \in \chi$.

4.5.   Operatorial equation

It is well-known ^[21] that the equation (4.34) is equivalent to the operatorial equation

where $\nu = \mu \, \rho^{-1}$.

An equation with bounded coefficients is obtained by setting

and by applying the operator $A^{-1/2}$. The equation can be expressed in operator form as:

5.   Equation of the moment of momentum for the system pendulum-fluid

Let $m_p$ and $J_p$ be the mass and the moment of inertia about $O_0$ of the body $S_0+O_0A$. We have the equation

At first, we have

where $J_\ell$ is the moment of inertia about $O_0$ of the liquid solidified in the equilibrium position.

We have

Finally, we write

But, we have

We can write

so that we have

where $b$ is the ordinate of the center of inertia of the liquid in the equilibrium position with respect to the axes $O'x'_1x'_2$ and $m_\ell$ the mass of the liquid.

Finally, setting

we obtain the equation

or

or

The equations (4.37) and (5.11) are the operatorial equations of the motion of the system pendulum - fluid.

6.   Operatorial equations of the small oscillations and first results on the spectrum

6.1.   Operatorial equations with constant coefficients

Setting $J_1 = \frac{J}{\rho}$ and $m_0 = \frac{m\, c}{\rho}$, the equations (4.37), (5.11) become

Using the definition of $\widehat{I}_0$, we have

Integrating by parts, we obtain

and, setting

we have

On the other hand, derivating gives us

Finally, three equations with constant coefficients are obtained for $\overrightarrow{W}$, $\theta$, $\overrightarrow{W}_1$ (5.11), (6.6) and (6.7), where

6.2.   Normal oscillations

The solutions of the precedent equations (5.11), (6.6) and (6.7) are sought depending on the time $t$ by the law $e^{-\lambda \, t}, \, \, \lambda \in \mathbb{C}$.

We obtain

6.3.   The first results about the spectrum

6.3.1.   Preliminary remarks

It should be noted that the spectrum $\sigma(A)$ of linear self-adjoint operator $A$ in Hilbert space $H$ is divided into two parts, the discrete and the essential spectrum ^[1]:

${\bf{a)}}$ The discrete spectrum, denoted $\sigma_d(A)$ contains the isolated eigenvalues with finite multiplicity.

${\bf{b)}}$ The essential spectrum, denoted $\sigma_e(A)$ contains the set of points of $\sigma_(A)$ which are not isolated eigenvalues with finite multiplicity. Then, $\sigma_e(A)$ is formed by the eigenvalues with infinite multiplicity, the accumulation points of eigenvalues and "continuous spectrum".

We conclude that:

From a physical perspective, we observe that in the oscillations of a liquid that partially fills a container the normal oscillations can be categorized into two distinct classes surface waves and internal waves:

${\bf{c)}}$ The surface waves are caused by the presence of the free surface of the liquid and the gravitation, capillar, and centrifugal forces (and others...) acting on the system.

${\bf{d)}}$ The internal waves whose modes have the following property: For them, the free surface of the fluid is almost unperturbed during the process of oscillations and the proper movements take place mainly inside the region fluid.

Let us recall that in spectral analysis we seek the properties of normal oscillations and their corresponding waves (surface or internal). Then, we seek $\lambda \in \mathbb{C}$ solution of the spectral problem i.e., $\lambda \in \sigma(A)$ and the characteristics of the corresponding associated waves.

6.3.2.   $\lambda=0$ is an eigenvalue with infinite multiplicity

Setting $\lambda = 0$ and eliminating $\theta$ and $\overrightarrow{W}_1$, we obtain

and consequently

or

Using the Schwarz inequality, we have

and the right-hand side is positive if the pendulum is preponderant.

Therefore,

or if $\gamma_{\Gamma}$ is the normal trace of $\chi^1(\Omega)$ on $\Gamma$ :

i.e

From $U_{n|\Gamma} = 0$, we deduce $K_1\vec{U} = 0$ or $\theta = 0$. Finally, we have

The eigenspace of $\lambda = 0$ is the subspace of $\chi \oplus \mathbb{C}$ defined by $\overrightarrow{W} \in {\bf{Ker}}\, \gamma_{\Gamma} A^{-1/2}$, $\theta = 0$.

6.3.3.   $\lambda=\gamma$ is not an eigenvalue

If $\lambda = \gamma$ then we have $\overrightarrow{W} = 0$ and $\overrightarrow{W}_1 = 0$. Thus

We conclude that all proper oscillations of the considered hydrodynamic system cannot be represented with fading decrements $e^{(-\gamma \, t)}$. On the other hand, we can divide by the number ($\lambda-\gamma \neq 0$). Then, the expression $I_0(\lambda) = \frac{\lambda-\beta}{\lambda-\gamma}$ is true.

6.3.4.   Two operatorial equations for studying the spectrum

Consequently, for studying the spectrum, we can use the equation (6.10) and we obtain only two equations for $\overrightarrow{W}$, $\theta$.

Setting

we have

The case $\overrightarrow{W} = \overrightarrow{0}$ or $\vec{U} = \overrightarrow{0}$ is dismissed, i.e the case where the liquid is solidified in the equilibrium position. Then, we have the classical pendulum whose pulsation $\omega$ is given by $\omega^2 = \frac{m_0\, g}{J_1}$.

Eliminating $\theta$, we have the unique equation for $\overrightarrow{W}$ :

6.3.5.   Existence and the symmetry of the spectrum

Dividing the equation (4.22) by $-\nu \, \lambda \, I_0(\lambda)$, we obtain

The terms between the brackets are selfadjoint and are compact, except $I_\chi$.

Therefore, we have a Fredholm pencil in the domain

regular in this domain since $\lambda = \gamma$ is not an eigenvalue ^[1].

Consequently, the spectrum of the problem exists; it is formed by isolate points and its possible points of accumulation can be only $0 \, , \, \beta \, , \, \pm \, i\, \sqrt {\frac{m_0\, g}{J_1}} \, \, \text{and} \, \, \infty \, .$

On the other hand, if the pencil is selfadjoint, the spectrum is symmetrical with respect to the real axis.

6.3.6.   The spectrum is located in the right-half plane

1) Stability of equilibrium : Discarding the eigenvalue $\lambda = 0$, we divide by $\lambda$ the equation (6.22) and (6.23).

We obtain

with

and

We have

Since

we have

so that, since $J_1 > J_\ell \rho^{-1}$, $\left(\mathcal{Q} Z, Z\right)$ is definite positive quadratic form of $\Vert \vec{U} \Vert_\chi$ and $\left| \theta \right|$, then equal to zero only for $Z = 0$.

Now, we have, in the same manner

so that, since $m_0-\int_{\Gamma} \, x_1^2 \, \mathrm{d} \Gamma > 0$, we have

From the equation (6.26), we deduce

Taking the real parts, we obtain

or

so

and the equilibrium of the system is stable in linear approximation.

2) Location of the eigenvalues :

From (6.34), we deduce $\Re I_0(\lambda) > 0$ or

Setting, $\lambda = x+iy$, we obtain

The eigenvalues are in the not dotted part of the plane $(\lambda)$.

6.3.7.   $\lambda=\beta$ is not an eigenvalue

If $\lambda = \beta$ is an eigenvalue then there exists $\overrightarrow{W} \neq 0$ such that

This equality can be written

Using the Cauchy's inequality for the second term, we have

If the pendulum is preponderant

the left-hand side is equal to zero only for $\vec{U} = 0$ then for $\overrightarrow{W} = 0$.

Therefore $\lambda = \beta$ is not an eigenvalue and we can note that all proper oscillations of the considered hydrodynamic system can not be represented with fading decrements $e^{(-\beta \, t)}$. On the other hand, we can divide by ($\lambda-\beta \neq 0$) then $\left(I_0(\lambda)\right)^{-1} = \frac{\lambda-\gamma}{\lambda-\beta}$ exist and we can replace the pencil $\mathscr{L} (\lambda)$ by the pencil ${\rm{Ł}} _0 (\lambda) = \frac{-1}{\nu \, I_0(\lambda)} \, \mathscr{L} (\lambda)$ (see section 7.2).

7.   There exists a branch of positive real eigenvalues having the infinity as point of accumulation

7.1.   An operator pencil

We consider the pencil $\mathscr{L} (\lambda)$ defined by :

Setting

we obtain

$\widehat{\mathscr{L}}(\lambda')$ is a self adjoint operatorial function that is holomorphic in the vicinity of $\lambda' = 0$.

We have

Let us prove that ${{\bf{Ker}}} \widehat{\mathscr{L}}(0) = 0$.

We have

$\widehat{\mathscr{L}}(0) \overrightarrow{W} = 0$ only for $\vec{U} = 0$ or $\overrightarrow{W} = 0$.

Consequently ^[1], for each $\varepsilon > 0$ sufficiently small in $\left] 0, \varepsilon\right[$ there exists an infinity of positive real eigenvalues $\lambda'_{n}$ having zero as point of accumulation. For our problem, there exists an infinity of positive real eigenvalues $\lambda_{n} = \lambda'^{-1}_{n}$ having the infinity as point of accumulation. The corresponding eigenelements form a Riesz basis in a subspace of $\chi$ having a finite defect.

7.2.   Asymptotic formula

Dividing $\mathscr{L}(\lambda)$ by $-\nu \, \lambda \, I_0(\lambda)$, we obtain the pencil

After calculations, we have

and for the last term

so that we can write

But, we have the following theorem ^[1] :

Let the pencil

with $T$ compact, $Q$ selfadjoint, positive definite, compact, $B(\lambda)$ analytic outside the circle $\left| \lambda \right|\leq R$ and turning into zero at infinity.

We have

Here, the conditions are satisfied and we have

8.   There exists a branch of positive real eigenvalues having zero as point of accumulation

Let us consider still the pencil $\mathscr{L} (\lambda)$.

$\mathscr{L} (\lambda)$ is a self adjoint operatorial function, holomorphic in the vicinity of $\lambda = 0$.

We have

On the other hand, $\mathscr{L}(0)$ admits $\lambda = 0$ as eigenvalue with infinite multiplicity because we have

Therefore, for all $\varepsilon > 0$ sufficiently small there exists in $\left] 0, \varepsilon\right[$ an infinity of positive real eigenvalues $\lambda_{n}^0$ having zero as point of accumulation. The corresponding eigenelements and an orthonormal basis of ${\bf{Ker}} \, \gamma_{\Gamma} A^{-1/2}$ form a Riesz basis in a subspace of $\chi$ having a finite defect.

9.   If the viscosity coefficient is sufficiently large, there exists a branch of real eigenvalues having $\lambda =\beta$ as point of accumulation

Setting

we have

Let

For each $\psi$, this operator is compact.

We have

with $A^{-1}$ and $F'(\beta^2)$ being bounded operators, $\widetilde{\mathscr{L}}'(0)$ is strongly negative if $\nu$ is sufficiently large.

On the other hand, $\lambda" = 0$ cannot be an eigenvalue of $\widetilde{\mathscr{L}}(\lambda")$ since $\beta$ is not an eigenvalue of $\mathscr{L}(\lambda)$. Therefore, if the viscosity coefficient is sufficiently large and if the pendulum is preponderant, for each $\varepsilon > 0$ sufficiently small, there is in $\left] 0, \varepsilon\right[$ an infinity of positive real eigenvalues $\lambda''_n$ having zero as point of accumulation. So for our problem, in $\left] \beta, \beta+\varepsilon\right[$ there is an infinity of real eigenvalues $\lambda^{\beta}_n = \lambda''_{n}+\beta$ having $\lambda = \beta$ as accumulation point. The eigenelements form a Riesz basis in a subspace of $\chi$ having a finite defect.

10.   Physical meaning of the results

This study builds upon previous works ^[2,16,19] that investigated the normal oscillations of a pendulum with a cavity partially or fully filled with fluid. Specifically, in ^[2,19] the model of a cavity partially filled with an ordinary viscous fluid was examined. In ^[16], a special case was explored where the cavity is completely filled with a viscoelastic fluid. It was demonstrated that the presence of viscoelastic forces leads to a more complex structure of normal oscillation waves notably resulting in the emergence of internal dissipative waves within the fluid region.

Let us state some important results and explain their physical meaning.

${\bf{1)}}$ If the pendulum is preponderant, it is proved that the lowest equilibrium position of the considered hydrodynamic system is stable in linear approximation.

${\bf{2)}}$ For a large viscosity of the viscoelastic fluid, the spectrum consisting of three branches of real eigenvalues $\left({\lambda_n^0}\right)_n$, $\left({\lambda_n^\infty}\right)_n$ and $\left({\lambda_n^\beta}\right)_n$ with limiting points $0, \infty$ and $\beta$, and corresponding to various kinds of normal oscillation waves. If we take $\theta = 0$, we have the same results for normal oscillations of a viscoelastic fluid in an open container ^[2] (section 11.3) and ^[11].

${\bf{3)}}$ It is proved that the new branch $\left({\lambda_n^\beta}\right)$ of finite multiplicity eigenvalues, that corresponds on the viscoelastic parameter $\beta = \frac{\kappa_0}{\kappa_1}$ of the fluid satisfied : ${\lambda_n^\beta} \rightarrow \beta$ $\left(n \rightarrow +\infty \right)$. This result confirms the fundamental influence of the viscoelastic forces on the structure of the spectrum of the considered hydrodynamic system. Physically, wave motions corresponding to these eigenvalues are predominantly internal in nature and arise exclusively from the action of viscoelastic forces. We remark that this effect disappears in the case of an ordinary viscous fluid ^[2,19], where the elastic parameter $\alpha = 0$, then $\beta = \gamma$ and $I_0(\lambda) = 1$. This gives that the viscoelastic forces in fluids have a significant impact onto the asymptotic distribution of the eigenvalues related to the internal waves inside the region fluid.

${\bf{4)}}$ For the limit points $\lambda = \infty$, we have the branch $\left({\lambda_n^\infty}\right)_n$ of finite multiplicity eigenvalues that corresponds to the internal dissipative waves in the viscoelastic fluid and just as in the case of an ordinary viscous fluid ^[2,19]. For these waves, the fading decrements can be as large as possible.

${\bf{5)}}$ For the limit points $\lambda = 0$, we have the branch $\left({\lambda_n^0}\right)_n$ of finite multiplicity eigenvalues that corresponds to the surface waves with decrements of the oscillation fading as small as possible. Their appearance is caused by the presence of the free surface of the fluid and by the influence of the gravitation field. This type of wave motions in fluids disappears (in particular $\lambda = 0$ is not an eigenvalue) in ^[16] where the cavity is completely filled by a viscoelastic fluid.

${\bf{6)}}$ When the effects of elastic forces inside the viscoelastic fluid are absent the elastic parameter $\alpha = 0$, $\beta = \gamma$ and $I_0(\lambda) = 1$. Therefore, the problem (4.24) is reduced to the study of a classical Askerov, Krein, Laptev pencil for studying small motions of viscous fluid in arbitrary open container. The small motions of the system depend on the viscosity coefficient of the fluid, and we have the well known results for a pendulum partially filled with an ordinary viscous liquid ^[2,19].

${\bf{i)}}$ For a large viscosity coefficient there is a set of real eigenvalues ${\lambda_n^+} \rightarrow \infty$ corresponding to arbitrary strongly damped aperiodic motions (internal dissipative waves) and a set of real eigenvalues ${\lambda_n^-} \rightarrow 0$, corresponding to arbitrary weakly damped aperiodic motions (surface waves).

${\bf{ii)}}$ For a small viscosity coefficient, there is a finite number of complex eigenvalues corresponding to damped "oscillatory" motions.

11.   Concluding remarks

In conclusion, we can confirm that this problem is new both in its hydrodynamic statement and as a problem in mathematical physics. It is shown that the visco-elastic forces are the cause of new physical effects that are not characteristic for an ordinary viscous incompressible fluid filling completely or partially a certain moving vessels. We believe that problems of this kind will capture the attention of other researchers. These are two important directions in which we believe that the studies should directed in the future.

${\bf{1)}}$ For pendulum, piezoelectric materials can be used to examine mechanical behaviors on the stability of pendulum with viscoelastic fluid in presence of an electric field.

${\bf{2)}}$ For fluid inside the cavity, relaxation property can be introduced, and dynamical characteristics of pendulum can be investigated in relation with this new physical fluid property.

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgements

The authors are grateful to the referees and the editorial board for some useful comments that improved the presentation of the manuscript.

Conflict of interest

The authors do not have any competing interests in the manuscript.