1. Introduction
The World Health Organization boasts that over the last 15 years, malaria incidence has decreased by 37% and deaths from malaria declined by 60% globally [1]. While this is undoubtedly marked progress, it is important to acknowledge that headway is not uniform across regions or countries, with some nations, particularly in Sub-Saharan Africa and some areas of Southeast Asia, lagging behind in malaria improvements [1]. Furthermore, malaria represents an infectious disease keenly tied to environmental conditions with mosquitoes as the disease vector [2,3]; thus, changes in environmental conditions can greatly alter the density and activity of mosquito populations and therefore malaria rates (e.g., [2]). As human alterations to the natural environment are only increasing in scale and intensity over time [3,4], we cannot assume that progress in addressing this disease will continue with manifest success. Instead, research needs to continue to investigate how human activities may be altering ecosystem conditions in ways that enhance mosquito habitats and transmission of malaria.
Although a number of forms of environmental degradation or ecosystem alteration could affect mosquito populations, deforestation is an increasingly studied factor in several locales across Asia, Sub-Saharan Africa, and, most prominently, Latin America (e.g., [5,6,7,8]). While a number of case studies identify links between forest loss and malaria or mosquito prevalence [5,6,7,8,9], whether relationship exists across areas or regions remains insufficiently examined. We attempt to contribute to this line of inquiry by imparting a cross-national analysis of less-developed, non-desert nations to examine the potential association deforestation and malaria prevalence across countries.
Prior examinations of deforestation and malaria tend to focus on the epidemiological aspects of ecosystem change and mosquito habitat proliferation [5,6,8,9,10]. While these studies provide key evidence on the direct mechanisms that cause deforestation to lead to increased levels of larvae, parasite concentrations, mosquito populations, or actual malaria rates [5,6,8,9,10], they fail also to consider fully the wider socio-economic context in which forest loss occurs. Our study contributes to this endeavor by simultaneously investigating the causes and disease consequences of deforestation in developing nations. By illuminating some of the key anthropogenic forces behind deforestation, our study contributes to broader understanding on how environmental degradation affects global trends in infectious disease.
2. Background
2.1. Malaria: characteristics and global trends
In 2015, around 450,000 people died from malaria worldwide [1]. The burden of this disease spreads across around 90 countries, with 88% of malaria cases concentrated in the Sub-Saharan African region [1,11]. Over the last several decades, partially due to major initiatives and programs, such as the WHO's "Roll Back Malaria, Roll in Development", there have been major reductions in malaria deaths and incidence [1,11]. However, this disease is still a leading threat to health and premature death in several countries [1]. Poverty represents one of the greatest risks for malaria cross-nationally [12]; undoubtedly, socioeconomic development fundamentally relates to the global distribution of morbidity and mortality for many health issues (e.g., [13,14,15,16,17,18]).
Caused by a parasite in the genus Plasmodium, four of the species, P. falciparum, P. vivax, P. malariae and P. ovale, lead to malaria in humans [19]. These parasites rely on two hosts throughout theirlifecycle: a mosquito and a human (or another animal) [19]. Only around 30-40 of the 400 species of Anophelesmosquito can act as vectors for the parasite, and only the bite of a female Anopheles mosquito spreads the parasite from one person to another [19,20]. The different types of malaria cause different variations of the disease in humans, with some forms being milder than other strains [19,20]. P. falciparum is the most prevalent species in Africa and causes the most deaths worldwide [1,11].
Early diagnosis of malaria is crucial and essential for management of the disease [1,13]. The WHO recognizes microscopy and rapid diagnostic tests as the current most common and effective ways of diagnosing the disease [1,11]. However, the quality of this method can be inadequate due to lack of sensitivity and insufficient expertise of personnel required to analyze results properly [1,11,21]. Especially in poor nations and rural areas, access to quality healthcare is limited [13,12,21]. Currently, there are many drugs available to combat the infectious parasite, but some are not very effective [1,22,23]. The most effective and popular drug, artemisinin combination treatments (ACTs), contain an active ingredient that is a derivative of artemisinin from the sweet wormwood tree [11,23]. Other drug treatments, including quinine and chloroquine were also popular in past decades; however, the parasite has developed resistance to these drug treatments [1,23]. In addition, recent studies already document increased tolerance to ACTs in many regions, leading to concerns over the potentials of widespread resistance [22].
As previously emphasized, patterns in malaria prevalence and international development are closely linked, as rates are highest in the poorest nations and, conversely, eradication of malaria took place decades ago in affluent nations [13,24,12,23]. Many studies in the areas of global health and development emphasize that impoverished people lack knowledge of disease prevention techniques, have little access to modern 'Western' medicine, and face limited access to appropriate preventative strategies, such as screened windows or insecticide-treated bed nets [13,24,21]. In addition, many poor households lack adequate access to water or sanitation, increasing potential mosquito habitats (e.g., [12,21,24,25,26]).
Education in particular signifies a key determinant of malaria incidence. For example, Dike et al. [27] find that formal education teaches basic skills that remove cultural ideologies leading to misconceptions that affect proper malaria management. In particular, people of higher education were more likely to identify mosquitoes as the malaria disease vector and to use insecticide-treated bed nets [27]. Numerous other studies of malaria report similar findings (e.g., [21,26,28]), suggesting that education also enhances people's awareness of Western medicine techniques and propensity to seek proper treatment. The potential importance of education in predicting cross-national malaria rates fits with other comparative global health assessments, including examinations of HIV, life expectancy, and infant and child mortality, which find that participation in schooling greatly improves health outcomes across nations (e.g., [14,15,16,17,18]).
While social and economic factors are important in shaping cross-national disease trends, environmental factors are also especially relevant with mosquitoes as the vector [2]. Thus, prevalence patterns are not only shaped by education, healthcare access, and poverty, but also the degree to which environmental conditions exist or are created that can sustain or flourish mosquito habitats [2,3]. Location in tropical or sub-tropical zones alone is argued to account for a significant amount of vulnerability to malaria, as the parasite quickly becomes weak and eliminated below 16 degrees Celsius
[29]. Additionally, the role of other environmental factors, such as deforestation, represents an emerging area of inquiry (e.g., [7,8]). As deforestation results from human activities, this represents a potential underlying anthropogenic cause of the malaria burden in many developing countries.
2.2. Links between forest loss and malaria
Today over 3 billion people worldwide remain at risk of acquiring malaria [13], and many of these vulnerable people tend to live in areas where environmental factors facilitate Plasmodium growth and prevalence. Mosquitoes fundamentally depend upon stagnant water and warm temperatures for their breeding and life cycle, explaining why malaria largely remains a tropical disease. Deforestation represents an additional environmental factor that can influence malaria [2,6,8,9,30,31]. The goal of this section is to draw on case study and epidemiological evidence from distinct studies to establish a rationale for examining the relationship between deforestation and malaria prevalence at the cross-national level.
Deforestation can impact malaria prevalence by several mechanisms, including increasing the amount of sunlight and standing water in some areas [2,6,9,32]. Although it is important to emphasize that different sub-types of Anopheles mosquitoes prefer shadier versus more sunlit habitats, or may be more or less sensitive to land use changes in general, typically, increasing standing water and sunlight is favorable for most species
[2,6,9,30,32]. Deforestation potentially contributes to these processes in multiple ways. For example, primary growth forests tend to be heavily shaded with thick debris on the ground, which absorbs water and often leaves any standing water acidic [32]. After clearing non-steep terrain, the land usually become flatter and more likely to pool water, which is typically less acidic and more conducive to Anopheles larvae development [32]. Not only does ponding more readily increase the availability of breeding grounds for the malaria vector, but increases in sunlight resulting from deforestation also promotes more ideal breeding grounds by warming temperatures [2,6,7,8,9]. When agriculture replaces forested areas, the plants can still provide the bushy cover needed for some species of Anopheles mosquito or stages of larvae development. Increases in mosquito reproduction potentially impact rates of malaria transmission to nearby populations [2,6,9].
Another possible link between forest loss and malaria concerns biodiversity (e.g., [33,34]). Disease ecologists find that higher levels of biodiversity generally take on a protective role for the human population, in a so-called "dilution effect." If there is a wider variety of species available, the proportion that are able to transmit the vector are reduced and thus transmission of diseases occurs less frequently [33,34]. One of the primary effects of deforestation is a loss in biodiversity, as a single, typically nonnative, crop often replaces a huge variety of vegetation and animal life [33]. Establishing a connection between forest loss and malaria is not straightforward; there are many confounding factors or factors specific to certain regions and locales. Thus, comparative assessments that examine trends in forest loss and malaria across regions can help to establish whether we can generalize the findings from epidemiological case studies to other areas endemic to the Plasmodium parasite.
Several studies conducted in sites in Asia indeed establish a link between malaria prevalence and forest loss. For example, studies conducted in China and Myanmar determine that the pupation rate of Anopheles minimus, a malaria vector, was highest in samples collected from deforested areas ( [7,8]). Similarly, a study carried out over the course of a year in Vietnam found that there was a statistically significant relationship between percentage of forest cover loss and prevalence of multiple strains of malaria [10]. In Sub-Saharan Africa, one study set in the highlands of Kenya in a case-control format found that living on land without trees led to increased risk for malaria contraction [5].
South America, particularly the Amazon region, represents perhaps the most popular site to examine the links between environmental degradation and malaria. For example, studies conducted in the Peruvian Amazon, which has experienced deforestation due to small-scale farming, discovered that
Anopheles darlingi had a statistically significant preference for living and breeding in areas that had experienced a loss of forest cover [6,9]. The observed connection between malaria rates and deforestation persisted even when considering changes in population density [9]. A study that sampled from over 800 water sources throughout the Amazon found that Anopheles darlingi sites were more likely to be located in areas that had experienced alterations in land cover, such as reductions in forest and the establishment of new croplands [6].
These studies demonstrate that multiple researchers across different regions and variety of Anopheles mosquito species identify a link between deforestation and malaria rates or mosquito populations. In fact, Yasuoka and Levins [35] conduct a content analysis of case studies centered on changes in ecology and malaria. They find around 60 examples from a variety of areas, including Thailand, Nepal, India, China, Guyana, Uganda, and others, that demonstrate a link between deforestation and land-use changes and growth in mosquito populations or malaria incidence [35].
It is important to emphasize that deforestation is not a natural phenomenon, but rather results predominantly from human activities [4]. Expanding agricultural areas represents an underlying cause of deforestation in the majority of these studies (e.g., [6,9,30]). Population growth within developing nations can lead to increased pressure for food production, but also many developing nations are encouraged to expand agricultural exports as a means for development (e.g., [36]). Aside from food demands, rural population pressures overall are likely to spur forest loss [4]. Many developing nations where malaria is most prevalent continue to have high rates of fertility and rural population growth [14]. Research also identifies access to timber for building or for use as fuelwood as key causes of deforestation in developing nations (e.g., [37,38]). Thus, rural population dynamics and pressures to increase food production are likely to be key anthropogenic causes of deforestation. In our analysis, we engage an innovative statistical method, structural equation modeling, in order to examine not only the potential role of deforestation in explaining cross-national variation in malaria prevalence, but also to account for the underlying causes of forest loss.
3. Materials and Methods
3.1. Sample
The sample is restricted to malaria-endemic nations. Malaria-endemic nations are nations that have a constant and measurable incidence of malaria and are located in natural areas of transmission [1]. Countries that register malaria cases resulting from imported cases are not included in the analyses. Malaria-endemic nations overwhelmingly have GDP per capita estimates in the lower three quartiles of the income classification of countries [1], thus largely representing a sample of less-developed nations. We exclude desert nations as deforestation is only relevant and measurable in countries with significant forest stock. Our sample includes 67 non-desert, less-developed nations for which data are reported for the key variables in the analysis, including the deforestation rate and malaria prevalence. For a complete list of the countries included in the analyses, see Table 1.
Table 1. Countries included in the Analyses (N = 67).
| Angola | SSA | Gambia, The | SSA | Pakistan | AS |
| Bangladesh | AS | Ghana | SSA | Papua New Guinea | PAC |
| Belize | LA | Guatemala | LA | Peru | LA |
| Benin | SSA | Guinea | SSA | Philippines | AS |
| Bhutan | AS | Guinea-Bissau | SSA | Rwanda | SSA |
| Bolivia | LA | Guyana | LA | Sao Tome & Principe | SSA |
| Burkina Faso | SSA | Haiti | LA | Senegal | SSA |
| Burundi | SSA | Honduras | LA | Sierra Leone | SSA |
| Cabo Verde | SSA | India | AS | Solomon Islands | PAC |
| Cambodia | AS | Indonesia | AS | South Africa | SSA |
| Cameroon | SSA | Kenya | SSA | Sudan | SSA |
| Central African Rep. | SSA | Lao PDR | AS | Swaziland | SSA |
| Chad | SSA | Liberia | SSA | Tajikistan | AS |
| China | AS | Madagascar | SSA | Tanzania | SSA |
| Colombia | LA | Malawi | SSA | Timor-Leste | AS |
| Comoros | SSA | Mali | SSA | Togo | SSA |
| Congo, Dem. Rep. | SSA | Mauritania | SSA | Uganda | SSA |
| Congo, Rep. | SSA | Mozambique | SSA | Vanuatu | PAC |
| Cote d'Ivoire | SSA | Namibia | SSA | Vietnam | AS |
| Dominican Rep. | LA | Nepal | AS | Zambia | SSA |
| Ecuador | LA | Nicaragua | LA | Zimbabwe | SSA |
| El Salvador | LA | Niger | SSA | | |
| Ethiopia | SSA | Nigeria | SSA | | |
| Notes: AS—Asia, LA—Latin America, PAC—Pacific Islands, SSA—Sub-Saharan Africa |
3.2. Analytic strategy
To examine the association between deforestation and malaria prevalence, as well as the underlying predictors of deforestation, we utilize structural equation models (SEMs). SEM is particularly useful in this context based on its efficient ability to model direct and indirect effects (e.g., [39]). While most comparative analyses utilize direct effects approaches, this strategy prevents modeling the mediating and interrelationships outlined above, such as the potential pathway involving rural population growth, deforestation, and malaria. Structural equation modeling also allows us to utilize latent or composite constructs of multi-dimensional concepts, such as health resources, and circumvent issues of multicollinearity, which often occur with cross-national data [40].
Another key aspect of SEMs involves the estimation of fit statistics that enable the researcher to judge the fit of the model as a whole to the data provided and compare equally plausible models [39]. An additional feature of SEM is its utilization of maximum likelihood (ML) missing value routine that calculates pathway coefficients based on all available data points; when cases are missing information on select variables, those cases are dropped from those pathway estimations, but retained for others when the data are available [40]. Thus, SEM allows us to maximize our sample of nations by retaining cases that might be missing data on one or two control variables included in the models [40].
In SEM, we simultaneously estimate a system of linear equations that correspond to our hypotheses about the correlations in our observed data [39]. For empirical identification, it is important that the models estimate normally [39]. We utilize SEM software in AMOS and Mplus; in both statistical packages, the path diagram displayed in Figures 1 and 2 all estimate normally. The assumptions of SEM include multivariate normality, completely random missing data, sufficiently large sample, and the correct model specification [40]. To protect against the negative consequences of multivariate non-normality we also ran the analyses using the robust ML estimator (MLR) implemented in Mplus [41]. These estimates with the MLR estimator are robust to non-normality [41]. The results were consistent with those achieved with the ML procedure. Although data should be completely missing at random, the use of the maximum likelihood (ML) estimator also provides consistent estimates under the assumption of missing at random, which is an easier condition to satisfy [42]. Additionally, we fail to see any pattern to the missing data to suggest that the data are not missing at random. Third, the positive asymptotic properties of the ML estimation procedure (consistency and efficiency) are known when the sample size is relatively large. As our sample is relatively small (N = 67), we re-ran our analyses with bootstrap standard errors as well as a robust ML (MLR) procedure and obtained estimates and model fit statistics for Figures 1 & 2 that are consistent with the ML estimator. Model specification errors occur withthe omission of relevant variables. If this occurs, the errors and the exogenous variables in the model correlate, leading to biased estimates [40]. To avoid this, we draw on prior research to select a wide range of variables. Our review of the literature engages perspectives on development, health, and the environment into our structural model. We test all theoretically informed paths as shown in Figures 1 and 2 below.
3.3. Variables included in the analysis
The key dependent variable in the analysis is the malaria prevalence rate. We created the malaria prevalence variable using data on the estimated number of malaria cases from the World Health Organization [1] and total population level from the World Bank [43] for the year 2013. We weighted the number of malaria cases for each nation by its total population, and then multiplied by 100,000 to form the prevalence rate.
As the malaria parasite requires particular climate conditions, latitude represents an important exogenous, environmental condition to consider. We transformed the latitude scores into absolute values to capture distance from the equator [43].
We examine deforestation as a prominent form of environmental degradation that is likely to be directly associated with increasing malaria prevalence. The natural deforestation rate represents an annual percent change score, calculated using FAO estimates of natural forest area, from 2012 to 2013 [43]. These data come from the Global Forest Resource Assessment (GFRA) and represent pointestimates for natural forest stock measured in thousand square hectares for 2012 and 2013. The natural forest area measure includes land area that is more than 0.5 hectares which contains trees higher than 5 meters and a canopy cover of more than 10%. We multiply the change score by -1 to capture rate of forest loss.
We consider both agriculture and rural population growth as key drivers of forest loss. We therefore include a measure of rural population growth, which accounts for the annual percent change in the rural population for the year 2012 [43]. We measure specialization in agriculture using data on the percent of the economy that comes from agricultural production, or agriculture as a percent of GDP
[43].
To assess the influence of economic development, we include
GDP per capita. This represents the total annual output of a country's economy divided by its population, measured in current international dollars for the year 2012 [43]. More specifically, GDP per capita is the total market value of all final goods and services produced in a country in a given year, equal to total consumer, investment, and government spending, divided by the mid-year population. It is converted into current international dollars using Purchasing Power Parity (PPP) rates, providing a standard measure allowing for comparisons of real price levels between countries [43].
Public health conditions are important non-economic factors that are likely to influence malaria rates in less-developed nations. We therefore measure public health conditions using a latent construct comprised of the following indicators: number of physicians, the fertility rate, access to clean water, and secondary schooling for the year 2012. Physicians, secondary schooling, access to clean water and the fertility rate represent some of the most prominent predictors of malaria rates, as well as other health outcomes in developing nations (e.g., [14,15,16]). The variable medical doctors measures the number of physicians in a nation per 100,000 people. The fertility rate is an estimate of the number of children an average woman would have if current age-specific fertility rates remained constant during her reproductive years. Percent access to clean water refers to the percentage of the population using an improved drinking water source. Improved drinking water sources include piped water located inside the user's dwelling, plot, or yard, and other improved drinking water sources, such as public taps or standpipes, tube wells or boreholes, protected dug wells, protected springs, and rainwater collection. Secondary school enrollment represents a gross enrollment ratio, which calculates the ratio of total enrollment, regardless of age, to the population of the age group that officially corresponds to secondary level education. We acquired all of these measures from the World Bank [43].
Additionally, we include a regional indicator for Sub-Saharan Africa, as many Sub-Saharan African nations have an especially high level of malaria and the highest concentrations of P. falciparum, which is one of the severest strains of the parasite. We measure this as a dummy variable, where countries coded with a '1' indicate location in Sub-Saharan Africa and those with a '0' indicate that a nation is located in a different region of the world [43].
4. Results
Table 2 displays the bivariate correlation matrix and univariate statistics for all of the variables used in the analyses. The magnitude of the bivariate correlation coefficients in Table 2 demonstrates that many of the predictor variables in the sample are highly correlated, such as the indicators for public health conditions variables. This further warrants the use of the SEM analytical technique given its superior handling of inter-correlated independent variables through the creation of latent constructs and direct and indirect pathways that circumvents the tendency to bias coefficient estimates [39].
Table 2. Correlation matrix and univariate statistics
| 1. | 2. | 3. | 4. | 5. | 6. | 7. | 8. | 9. | 10. | 11. |
| 1. Malaria Prevalence | 1.00 | | | | | | | | | | |
| 2. Latitude | −0.365 | 1.00 | | | | | | | | | |
| 3. GDP per capita | −0.591 | 0.175 | 1.00 | | | | | | | | |
| 4. Sub-Saharan Africa | 0.652 | −0.274 | −0.563 | 1.00 | | | | | | | |
| 5. Secondary Schooling | −0.582 | 0.157 | 0.777 | −0.650 | 1.00 | | | | | | |
| 6. Medical Doctors | −0.531 | 0.367 | 0.698 | −0.658 | 0.664 | 1.00 | | | | | |
| 7. Clean Water | −0.409 | 0.195 | 0.592 | −0.621 | 0.656 | 0.457 | 1.00 | | | | |
| 8. Fertility Rate | 0.754 | −0.304 | −0.757 | 0.730 | −0.793 | −0.650 | −0.658 | 1.00 | | | |
| 9. Agriculture % GDP | 0.608 | −0.220 | −0.753 | 0.445 | −0.672 | −0.506 | −0.606 | 0.605 | 1.00 | | |
| 10. Rural Pop Growth | 0.465 | −0.196 | −0.726 | 0.533 | −0.633 | −0.563 | −0.487 | 0.738 | 0.530 | 1.00 | |
| 11. Deforest-ation Rate | 0.391 | −0.158 | 0.490 | 0.174 | −0.267 | −0.140 | −0.177 | 0.354 | 0.346 | 0.316 | 1.00 |
| Mean | 12433 | 13.49 | 4148.2 | 0.582 | 55.86 | 0.401 | 76.38 | 4.08 | 23.94 | 1.23 | 0.526 |
| Standard Deviation | 13996 | 8.40 | 3243.8 | 0.497 | 22.26 | 0.533 | 15.04 | 1.41 | 12.92 | 1.28 | 1.64 |
A preliminary step in the empirical assessment of our complete SEM was to validate empirically whether public health conditions represent latent factors that can be appropriately estimated secondary school enrollments, trained medical doctors, access to clean water, and the average fertility rate. To test this, we initially construct a confirmatory factor analysis (CFA) of the measures and analyze the overall and component measures of fit. By empirical standards, we find evidence at both the component and overall model levels to support our predictions that these indicators can be used to measure public health conditions. This fits with our substantive interpretations of prior health and development research discussed earlier.
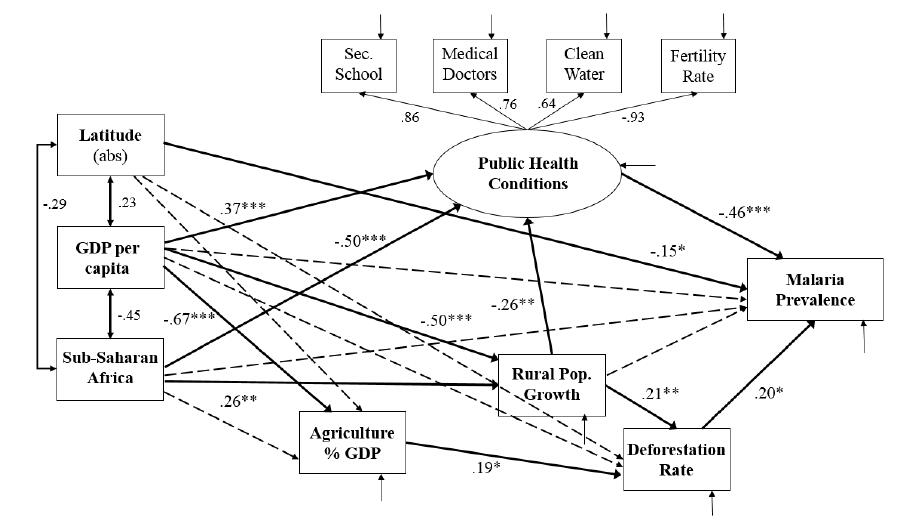
Figures 1 and 2 present the SEM results of malaria prevalence. We test all theoretically and substantively informed paths in the path diagram displayed in Figure 1, and then eliminate all non-significant relationships to present the most parsimonious model in Figure 2. Before turning to a discussion of results, we note the model fit statistics indicate an excellent fit of both models to the data. Specifically, for Figure 1, in accordance with empirical standards the chi-square test statistic is non-significant (χ2 = 33.15 with df= 31); the values of the Incremental Fit Index (0.980), Tucker-Lewis Index (0.965), and the Confirmatory Fit Index (0.979) all exceed 0.90; and the root mean squared error of approximation (RMSEA) value (0.046) is below the suggested threshold of .10 for smaller samples [39].
The results from the trimmed model (where non-significant paths are eliminated) presented in Figure 2 are also well within range of the empirical standards for SEMs. The chi-square test statistic is non-significant (χ2 = 45.08 with df= 37); the values of the Incremental Fit Index (0.968), Tucker-Lewis Index (0.966), and the Confirmatory Fit Index (0.981) all exceed 0.90; and the root mean squared error of approximation (RMSEA) value (0.048) is below the appropriate threshold.
It is common when using SEMs to eliminate non-significant paths for the sake of parsimony. When looking across the saturated model in Figure 1 and the trimmed model in Figure 2, there are a few substantial differences in the path coefficients. The only changes are a small reduction in the magnitudes for effects on malaria prevalence and deforestation in the more saturated model. As the substantive findings are essentially consistent across the two models, and as the fit statistics are acceptable in the second model as well as the first, we prefer to focus our discussion of the results on the findings presented in Figure 2. In the path diagrams, standardized regression coefficients are reported and flagged for statistical significance. We also include Table 3, which displays the standardized regression coefficients in addition to the unstandardized regression coefficients and standard errors for the model in Figure 2. However, we keep our interpretation of the results focused on Figure 2, as the path diagram facilitates accessible interpretations.
Table 3. Regression results for SEM depicted in Figure 2 predicting malaria prevalence.
| Standardized
Regression
Coefficient | Unstandardized
Regression
Coefficient | Standard
Error |
| Latitude → Malaria Prevalence | −0.179* | −294.49 | 144.13 |
| Public Health Conditions → Malaria Prevalence | −0.658*** | −483.02 | 76.51 |
| Deforestation Rate → Malaria Prevalence | 0.243* | 784.28 | 217.01 |
| Rural Pop Growth → Deforestation Rate | 0.280** | 0.334 | 0.095 |
| Agriculture % GDP → Deforestation Rate | 0.273** | 0.087 | 0.002 |
| Rural Pop Growth → Public Health Conditions | −0.257** | −3.78 | 1.18 |
| GDP per capita → Public Health Conditions | 0.368*** | 0.002 | 0.000 |
| GDP per capita → Rural Pop Growth | −0.508*** | −0.001 | 0.000 |
| GDP per capita → Agriculutre % GDP | −0.604*** | −0.003 | 0.000 |
| Sub-Saharan Africa → Public Health Conditions | −0.501*** | 18.99 | 2.93 |
| Sub-Saharan Africa → Rurual Pop Growth | 0.270** | 0.698 | 0.263 |
| Public Health Conditions → Secondary Schooling | 0.858 | - | - |
| Public Health Conditions → Medical Doctors | 0.756*** | 0.021 | 0.003 |
| Public Health Conditions → Clean Water | 0.640*** | 0.511 | 0.088 |
| Public Health Conditions → Fertility Rate | −0.928*** | −0.070 | 0.007 |
| Notes: ***p < 0.001, **p < 0.01, *p < 0.05 (two-tailed tests); standardized coefficients flagged for statistical significance; unstandardized coefficients reported in in italics; standard errors reported in parentheses. |
The results presented in Figure 2 suggest that deforestation is associated with increased prevalence of malaria in developing nations, which is consistent with our predictions (0.24). In addition, we find that economic specialization in agriculture (0.27) and rural population growth (0.28) are positively associated with the deforestation rate. Thus, not only do we find empirical evidence for links between deforestation and malaria across nations, but also that population growth in rural areas and increased emphasis on agriculture contribute to deforestation.
In addition, we find that public health conditions have a significant, negative association with malaria rates in less-developed nations. In particular, secondary school enrollments, medical doctors, access to clean water, and the average fertility rate together represent a set of public health conditions that are robustly associated with declines in malaria prevalence
(−0.66). In addition to public health conditions, latitude also explains cross-national variation in malaria prevalence, where nations located further from the equator tend to have lower rates of prevalence (−0.18).
Our results also suggest that GDP per capita or level of economic development and location in Sub-Saharan Africa are important underlying factors contributing to variations in the malaria burden across developing nations in indirect ways. It is important to emphasize that the results across Figures 1 and 2 demonstrate that each of these variables has an indirect association to malaria prevalence. Specifically, more economically developed nations tend to have improved public health conditions (0.37), which then reduce the malaria burden. In addition, more affluent nations tend to have significantly lower levels of rural population growth (−0.51) and increased economic specialization in agricultural production (0.70), which in turn are associated with deforestation rates.
We also find in Figure 2 that Sub-Saharan African nations tend to have considerably higher rates of rural population growth than other nations (0.27), and that Sub-Saharan African nations also have much weaker public health conditions in comparison to other nations (−0.50). Our results also suggest that nations experiencing high levels of rural population growth are more likely to have poorer public health conditions (−0.26). This fits with prior research identifying that rural populations face especially poor access to and quality of a variety of health and social resources, including health facilities and education [21].
Overall, our results suggest that deforestation is an important factor in explaining cross-national variation in malaria prevalence. We also find that anthropogenic forces related to specialization in agriculture and rural population pressures are associated with heightened levels of deforestation, thereby suggesting that human activities contribute to some level of malaria prevalence. In considering the relative weight of impact of latitude versus deforestation in the model, we find in our analyses that forest loss is more relevant in explaining cross-national variability in malaria rates than being located close to a tropical zone. Thus, human-induced environmental changes may be more significant in predicting malaria prevalence than the truly "natural
" or inherent environmental conditions.
4. Conclusions & limitations
Nearly 130 million hectares of forest—an area almost equivalent in size to South Africa - have been lost since 1990, according to a recent FAO report [4]. Not only does deforestation contribute to profound impacts on climate change, biodiversity loss, and changing weather patterns, but this study builds on a body of evidence that deforestation also influences malaria transmission. While isolated studies have begun to identify links between forest loss and mosquito proliferation or malaria prevalence in certain locales (e.g., [5,6,7,8]) this study adds to the research on this phenomena by finding an association between deforestation and malaria prevalence at the cross-national level.
In addition, we extend our analysis to also to consider some of the most prominent causes of forest loss in developing nations. Our findings suggest that rural population growth and economic specialization in agriculture are significant in increasing levels of forest loss in many developing nations. Therefore, not only are there potential links between environmental change and malaria, but we consider the anthropogenic underpinnings of this form of degradation. Rural population pressures not only affect forests, but public health conditions in developing nations as well. Perhaps in some areas, growing rural populations who tend to live closer to the natural habitats of mosquitoes may experience further compounding risks due to their lack of health resources and proximity to deforested areas.
One limitation of our study is sample size. Sample size is inherently restricted as it is only appropriate to include malaria endemic, non-desert nations in the analyses. Given those parameters, a sample of nearly 70 nations is actually robust, and our model adheres to the model fit and other parameters appropriate for SEMs. We also re-ran our analyses with bootstrap standard errors as well as a robust ML (MLR) procedure and obtained estimates and model fit statistics for Figures 1 & 2 that were consistent with the ML estimator. Although the use of cross-national data represents a key contribution of this research, there are notable limitations with this approach. Cross-national analyses cannot account for the particular sub-national ecological factors that may affect malaria prevalence, such as soil conditions, level of rainfall, or temperature variations, as well as the particular vector susceptibilities and preferences that vary by region or sub-species of mosquito. These represent topics more appropriate for the epidemiological field studies we draw on, and instead we prefer to focus on determining if comparative analysis uncovers broad patterns linking deforestation to malaria across nations. In so doing, we acknowledge that we cannot assume that deforestation will cause increased malaria rates in every nation, region, or area, but rather emphasize that there appears to be a pattern across countries between deforestation and malaria prevalence, where the nations with higher rates of deforestation also tend to have higher rates of malaria, net of other factors. Our results help to expand the generalizability of case studies exploring forest loss and mosquito proliferation across different sites (e.g., [5,6,7,8,9,10]). Certainly, given this complex topic, we hope to encourage further research examining how forest loss influences trends in malaria and other mosquito-borne diseases in and across diverse settings.
Our study makes an interdisciplinary contribution by bringing together ideas and insights from across fields of global development and sociology, global health, epidemiology, environmental science, and human ecology. We consider many of the social, demographic, economic, and environmental predictors found to be important in predicting both malaria prevalence and deforestation from across these disciplines. The use of structural equation models (SEMs) allows us to test the interconnections and complex pathways among these different types of indicators, and serves to illuminate how international inequalities frame the proximate predictors of malaria rates in developing nations, such as forest loss and public health conditions.
Importantly, we find there is no direct association between GDP per capita and Sub-Saharan Africa and malaria prevalence. Although many studies make direct links between rising affluence and malaria management, our results suggest that economic growth will be most effective if channeled to improving public health conditions or addressing rural population growth. Similarly, the indirect associations involving Sub-Saharan Africa imply that there is nothing inherent about this region that explain disproportionately high malaria rates; rather our results suggest that Sub-Saharan African nations suffer from a lack of public health services and extreme levels of rural population growth. Our results also find links between GDP per capita and economic specialization in agriculture, where poorer nations in our sample are much more likely to generate a larger share of their economic revenues from food production, which in turn are associated with increased deforestation rates and malaria prevalence. Although we do not focus on exports specifically, it is important to keep in mind that a notable amount of food produced in poor nations is destined for markets in affluent nations [44]. Developing nations are often encouraged by supra national organizations and core governments to specialize in agriculture as a means to development [36]; perhaps these policy recommendations should be interpreted with caution given the potentially contaminant influences on forests and malaria rates.
Although there have been major improvements in malaria prevention, diagnosis, and treatment in many nations over the last several decades [1], malaria remains a leading cause of death and threat to health in many regions and countries across the Global South [1,12]. Some patterns in climate change, deforestation, and other human-induced changes to the natural environment could alter and amplify malaria transmission (e.g., [31]). In addition to the influence of environmental changes, resistance to both insecticides and antimalarial medications is increasing [22,23]. As human alterations to the natural environment are only intensifying, understanding and mitigating the underlying anthropogenic causes of malaria transmission deserves vigilant attention.
Conflict of Interest
The authors declare there is no conflict of interest.









 DownLoad:
DownLoad: