Filter design for continuous-discrete Takagi-Sugeno fuzzy system with finite frequency specifications

1.   Introduction

In the last few decades, many researchers have investigated two-dimensional (2-D) systems including continuous-continuous, discrete-discrete and continuous-discrete settings, as these systems have great applications in engineering fields such as process control, multi-dimensional digital filtering and image processing; see, for instance, ^[1] and reference therein. Recently, due to the continuous-discrete systems having an advantage of describing vehicle platoon model ^[2], linear repetitive processes ^[3], iterative learning control systems ^[4] and others, the study of continuous-discrete systems has been a major topic. Now, the main research is about stability problem ^[5] and feedback control ^[6].

In practice, many systems have complex characteristics and nonlinearities, which can not be fully described by the linear system models ^[7,8,9]. Utilizing the Takagi-Sugeno (T-S) fuzzy model ^[10] to approximate the nonlinear systems via fuzzy sets and fuzzy reasoning is an efficient approach. It is well known that the T-S fuzzy model has the favourable ability to approximate any nonlinear system ^[11], which provides sufficient tools and techniques for researchers to design complex nonlinear systems ^[12]. To this day, some designers have tried to investigate the continuous-discrete nonlinear systems via using fuzzy-model-based control methods ^[13]. Now, the T-S fuzzy model has been an efficient tool to solve nonlinear problems by adopting the mature linear system theories.

On the other hand, the practical systems are often disturbed by the noise signals; therefore, the filtering issues for nonlinear systems have been studied extensively by the T-S fuzzy model approach ^[14]. In order to describe systems more accurately, filtering problem is considered to estimate the system state by using the known information under the noisy disturbance. One commonly studied scheme for 2-D systems is $H_{\infty}$ filtering ^[15,16].

Among most of the existing research results on the filtering problem, frequency of the disturbances is usually assumed to occupy the entire frequency domain, which actually brings over-design compared to the finite frequency (FF) case since the useful information of the FF range of disturbances are not fully utilized. In some practical engineering applications, the disturbance signals are intrinsically dominated within FF ranges, which could be known in advance ^[17]. It is more reasonable and useful to design controllers or filters according to the frequency characteristics of disturbances. The generalized Kalman-Yakubovich-Popov lemma proposed in ^[18] presented an efficient way in inspecting the FF specifications of the controlled system, which can be directly utilized to derive certain linear matrix inequality (LMI) conditions so as to design appropriate filters. The 2-D generalized Kalman-Yakubovich-Popov lemmas proposed in ^[19,20] are useful tools when dealing with the problems of $H_{\infty}$ control, $H_{\infty}$ filtering and fault detection observer/filter design for linear 2-D systems in FF domains, which have been studied in ^[21,22].

It should be noted that the system properties formalized as frequency domain inequalities are inapplicable to nonlinear systems. Due to this reason, it cannot describe the system representations accurately through the method of frequency-domain analysis. Stimulated by the concept of time domain interpretations of the frequency domain inequalities ^[23], the authors of ^[24] dealt with the problem of filtering design for nonlinear systems in the T-S fuzzy model by introducing the FF $H_\infty$ index. Based on the above theories, the filter design method and FF static output feedback $H_\infty$ controller design method have been proposed for Roesser-type 2-D discrete systems in the T-S fuzzy model in ^[25,26], respectively. The most striking characteristic of continuous-discrete systems lies in that there are a differential equation and a difference equation with respect to the continuous and discrete variables, respectively ^[27]. Therefore, the results proposed for discrete and continuous 2-D systems are unable to be used for the addressed systems directly in this paper. To the authors' best knowledge, there has been no results on FF $H_{\infty}$ filtering for nonlinear continuous-discrete systems. Thus, it is necessary to develop the FF filtering theory specially for continuous-discrete T-S fuzzy systems. Inspired by these works, the authors aim to design the $H_{\infty}$ filter for continuous-discrete T-S fuzzy systems in this paper.

This paper aims to design a filter for the continuous-discrete system in the T-S fuzzy model with FF disturbances. The main contributions of this work are summarized as follows:

1) Motivated by the time-domain interpretations of the frequency domain inequalities ^[23], definition of the FF $H_{\infty}$ performance for continuous-discrete systems is proposed, which contains the frequency information of the disturbance input and generalizes the standard $H_{\infty}$ performance.

2) Based on the Parseval's theorem for continuous-discrete systems, the FF $H_{\infty}$ performance analysis results have been obtained, which generalizes the FF bounded real lemma for linear systems ^[20].

3) Sufficient conditions for the existence of the desired filter and a systematic method for the filter design are developed to ensure the asymptotic stability and FF $H_{\infty}$ performance of the filtering error system. By setting the matrix and decision variables dependent of the membership function, the proposed results are applicable to linear systems, which means that the prosed results are very general.

The paper proceeds as follows. The preliminaries and problem formulation are presented in Section 2. Main results, including FF $H_{\infty}$ performance analysis and filter design are given in Section 3. An illustrative example demonstrates the theoretical results potency in Section 4. Finally, some conclusions are summarized in Section 5.

2.   Preliminaries and problem formulation

Notations: ${\mathbb{R} }$, ${\mathbb{C} }$ and ${\mathbb{H} _n}$ stand for the sets of all real numbers, complex numbers and Hermitian $n\times n$ dimensional matrices, respectively. $A^{-1}$, $A^T$ and ${A^*}$ denote the inverse, the transpose and the complex conjugate transpose of matrix $A$, respectively.

and ${\rm tr}(A)$ stand for the Hermitian and the trace of square matrix $A$, respectively. The notation $A > 0$ (${A \ge 0}$) means that $A$ is positive definite (positive semi-definite). $N_A$ denotes a matrix whose columns form a basis of the null-space of matrix $A$. The symbol "$\star$" represents the term originated by conjugate symmetry in a matrix. The $L_2$ norm of a 2-D signal $w(t, k)$ is given by

$w(t, k)$ is said to belong to $L_2{\lbrace [0, \infty), \lfloor {{0, \infty}})\rbrace }$ if $\|w\|_2 < \infty$, where $\lfloor {{0, \infty } }) : = \{0, 1, 2, \cdots \}$.

2.1.   System description

Consider the continuous-discrete T-S fuzzy system with its $i$-th rule as follows:

Rule $i$: IF ${\theta}_1(t, k)$ is $M_{i1}$, $\cdots$, and ${\theta}_L (t, k)$ is $M_{iL}$, THEN

with

where $i\in\underline{N} = \lbrace 1, 2, \cdots, N\rbrace$, $t\in[0, \infty)$ and $k\in\lfloor 0, \infty)$; $x^h(\cdot, \cdot)\in {\mathbb{R}}^{n_h}$ and $x^v(\cdot, \cdot)\in {\mathbb{R}}^{n_v}$ are state vectors that vary in the horizontal and the vertical directions, respectively; $x({\cdot}, {\cdot})$ denotes the whole state in ${\mathbb{R}^n}$ with $n = {n_h} + {n_v}$; $w({\cdot}, {\cdot})\in {\mathbb{R}}^{n_w}$, $y(\cdot, \cdot)\in {\mathbb{R}}^{n_y}$ and $z(\cdot, \cdot)\in {\mathbb{R}}^{n_z}$ are the disturbance input, the measured output and the signal to be estimated, respectively. ${\theta}_p(t, k)$, $p = 1, 2, .., L$, are the premise variables; $M_{ip}$ is the fuzzy set; $L$ is the number of premise variables; $N$ is the number of IF-THEN rules; $A_i$, $B_i$, $C_i$, $D_i$, $L_i$ and $E_i$ are known matrices of appropriate dimensions. The energy of the disturbance $w(t, k)$ is assumed to be dominated in a known rectangular FF region introduced later.

Via using the inference product, the singleton fuzzifer and the center-average defuzzifer, (2.1) can be described by

with

where $M_{ip}({\theta}_p(t, k))$ is the degree of the membership function of ${\theta}_p(t, k)$ in $M_{ip}$. Assume that ${M_{ip}({\theta}_p(t, k))}\geq 0$ for all $i\in {\underline N}$ and $p\in {\underline L} = \{1, 2, \cdots, L\}$, then for all $t \geqslant 0$ and $k \in {\lfloor {{0, \infty } }) }$, the normalized membership function ${\mu}_{i}(t, k)$ satisfies

The boundary conditions (BCs) associated with (2.1) are

where $h_0\in {\mathbb{R}}^{n_h}$ and $v_0\in {\mathbb{R}}^{n_v}$ are given vectors, $z_1$ is a positive scalar and $z_2$ is a positive integer.

2.2.   Fuzzy filter

In order to estimate the signal $z(t, k)$, a fuzzy Roesser-type filter is designed as follows:

Filter rule $i$: IF ${\theta}_1(t, k)$ is $M_{i1}$, $\cdots$, and ${\theta}_L(t, k)$ is $M_{iL},$ THEN

with $x_F^h(0, k) = 0$, $x_F^v(t, 0) = 0$ for $t\geq 0$ and $k\in \lfloor {0, \infty})$,

where $x_F^h(\cdot, \cdot)\in {\mathbb{R}}^{n_h}$ and $x_F^v(\cdot, \cdot)\in {\mathbb{R}}^{n_v}$ are the horizontal and the vertical filter states, respectively; $z_F(\cdot, \cdot)\in {\mathbb{R}}^{n_z}$ is an estimation of $z(\cdot, \cdot)$; $A_{F, i}$, $B_{F, i}$, $C_{F, i}$ and $D_{F, i}$ $({i}\in \underline{N})$ are appropriately dimensioned filter matrices to be determined.

The defuzzified output of system (2.4) is obtained as

where

Defining

and augmenting the filter states with system (2.2), the following filtering error system (FES) can then be obtained

where

2.3.   Problem statement

In practice, effects of the noises are usually dominated in FF ranges. Based on the practical situation, denoting the Fourier transform of $w(t, k)$ as $W({\rm j} w_h, e^{{\rm j} w_v})$ with ${\rm j}$ being the imaginary unit, a rectangular FF domain ${R}$ for the noise signal $w(t, k)$ is given as follows:

where $w_{h1}, \; w_{h2}\in \mathbb{R}$ and $w_{v1}, \; w_{v2}\in (-\pi, \pi]$ are the lower and upper bounds of frequency variables $w_h$ and $w_v$ respectively.

Definition 2.1. For the FF domain ${R}$ and a given positive scalar $\gamma$, the FES (2.7) is said to have an FF $H_{\infty}$ index $\gamma$ if the following two conditions are satisfied:

(i) When $w(t, k) \equiv 0$, the FES (2.7) is AS;

(ii) Under the zero BCs, the inequality

holds for all solutions of (2.7) with

such that

where

The FF filtering problem to be addressed in this paper is to design a fuzzy filter in the form of (2.4), such that the FES (2.7) is AS and has an FF $H_\infty$ performance level $\gamma$. To get the main results, we still need the following lemma.

Lemma 2.1. (^[28]) If the 2-D complex vector functions $x({t, k})$ and $y({t, k})$ belong to ${L_2} \{ { [ {0, \infty }), \lfloor {0, \infty })} \}$, then the corresponding Laplace-Z transforms $X({s, z})$ and $Y({s, z})$ exist and the following relation holds, provided that $x(t, k)$ and $y(t, k)$ have the same dimensions

3.   Main results

3.1.   FF performance analysis

In the following, one sufficient condition is presented for the FES (2.7) having an FF $H_\infty$ performance.

Theorem 3.1. Assume that the FES (2.7) is asymptotically stable (AS). For the FF domain ${R}$ and the scalar $\gamma > 0$, the FES (2.7) has an FF $H_\infty$ performance $\gamma$ if there exist symmetric matrices

and

such that $\hat P^h > 0$, $\hat Q^v > 0$ and

with

Proof. Multiplying the inequality (3.1) by $[{\hat x}^T (t, k) \; \; w^T(t, k)]$ from the left and by its transpose from the right with

we have

with

Taking integration for $t$ from zero to $\infty$ and summation for integer $k$ from zero to $\infty$, in view of the zero BCs, we have

where

Clearly, $S^v$ is Hermitian, and it follows from condition (2.10) that $S^h < 0$. Due to the zero BCs, it is true that

and combining with constraint (2.11) infers that $S^v < 0$. Applying Lemma 2.1 to $S^h$, we have

which directly guarantees that $S^h$ is also Hermitian, where $\hat X^h({\rm j}w_h, e^{{\rm j}w_v})$ denotes the 2-D Laplace-Z transform of $\hat x^h(t, k)$. Additionally, since $\hat P^h > 0$ and $\hat Q^v > 0$, $-{\rm tr}({\rm He}(\hat P^h S^h)) -{\rm tr}(\hat Q^v S^v)$ is also positive, then it follows from (3.3) that

which means that FES (2.7) has an FF $H_\infty$ performance $\gamma$ according to Definition 2.1. This completes the proof. □

To further achieve the main result, the following lemma is needed to guarantee the asymptotic stability of FES (2.7).

Lemma 3.1. ^[28] The FES (2.7) with zero input is AS if positive definite matrices $\bar P^h \in {\mathbb{R}}^{2n_h \times 2n_h}$ and $\bar P^v \in {\mathbb{R}}^{2n_v \times 2n_v}$ exist such that

holds, where

and

Lemma 3.1 gives a sufficient condition ensuring the considered system to be AS. To facilitate the filter design, it is necessary to decouple the product terms between the matrix variables $\hat P$, $\hat Q$ and the system matrices $\hat A(\mu)$, $\hat B(\mu)$ in (3.1). Thus, we need to present an alternative of Theorem 3.1.

Theorem 3.2. For the FF domain $R$ and the scalar $\gamma > 0$, the FES (2.7) has an FF $H_\infty$ performance $\gamma$ if there exist matrices $\hat G(\mu)$, $\hat F(\mu)$, $\bar G(\mu)$, $\bar F(\mu)\in {\mathbb R}^{2n\times 2n}$ and $\hat H(\mu)\in {\mathbb R}^{n_w\times 2n}$, symmetric matrices

and

such that $\hat P^h > 0$, $\hat Q^v > 0$, $\bar P^h > 0$, $\bar P^v > 0$ and

where

and the other notations are the same as defined in Theorem 3.1 and Lemma 3.1.

Proof. Define

where

and

Then, we have

with

By Schur complement lemma, $\Psi (\mu) < 0$ is equivalent to inequality (3.5), then by taking

pre- and post-multiplying both sides of $\Psi (\mu) < 0$ by $N_{U(\mu)}^T$ and $N_{U(\mu)}$, respectively, and noting that $N_{U(\mu)}$ is column full rank, it is known that inequality (3.1) does hold.

In addition, we set

where $\bar{Q}_{11}$, $\bar{Q}_{12}$ and $\bar{Q}_{22}$ are defined in Lemma 3.1. The constraint condition (3.6) is exactly

Similarly, taking

pre- and post-multiplying both sides of $\bar \Psi (\mu) < 0$ by $N_{\bar U(\mu)}^T$ and $N_{\bar U(\mu)}$, respectively, and noting that $N_{\bar U(\mu)}$ is column full rank, it follows that

From Lemma 3.1, we know that the FES (2.7) with $w(\cdot, \cdot)\equiv 0$ is AS. The proof is completed by resorting to Theorem 3.1 and Definition 2.1. □

Remark 3.1. The slack matrices $\hat G(\mu)$, $\hat F(\mu)$, $\bar G(\mu)$, $\bar F(\mu)$ and $\hat H(\mu)$ are introduced to decouple the products between the Lyapunov matrices $\hat P$, $\bar P^h$, $\bar P^v$, $\hat Q$ and the system matrices, which aids in the filter design while also bringing certain conservatism.

3.2.   Fuzzy filter design

The following result can be derived via specifying the structure of the slack matrices in Theorem 3.2 and coverting the filter design problem to a group of LMI constraints, which are numerically tractable.

Theorem 3.3. For the FF domain ${R}$ and the scalar $\gamma > 0$, the FES (2.7) has an FF $H_\infty$ performance $\gamma$ if there exist matrices

and symmetric matrices

such that

hold for all $i, j\in {\underline N}$, where

Moreover, the coefficients of filter (2.4) are designed as

Proof. Parameterize the slack matrices $\hat G(\mu)$, $\hat F(\mu)$, $\bar G(\mu)$, $\bar F(\mu)$ and $\hat H(\mu)$ as

with $\Pi$ given in (2.7). Define matrix variables $\hat P$, $\bar P^h$, $\bar P^v$ and $\hat Q$ as follows:

i.e.,

In addition, we can obtain the following equalities

Due to ${\Pi}^T\Pi = I$, taking

and putting (3.8), (3.9), (3.13)–(3.15) into (3.5) and (3.6), respectively, we have

with

Define

with $l = 1, \; 2$. Pre- and post-multiplying both sides of (3.16) by ${\rm{diag}} \{ {{\Pi ^ T }, {\Pi ^ T }, I, I} \}$ and ${\rm{diag}} \{ {{\Pi }, {\Pi }, I, I} \}$, respectively, (3.16) is equivalent to condition (3.10). Similarly, pre- and post-multiplying both sides of (3.17) by ${\rm{diag}} \{ {{\Pi ^ T }, {\Pi ^ T }} \}$ and ${\rm{diag}}\{{{\Pi}, {\Pi}}\}$, respectively, (3.17) is equivalent to condition (3.11). It is noted that (3.11) guarantees $-V-V^T < 0$, which means that $V$ is nonsingular and the filter coefficients could be calculated by (3.12). This concludes the proof. □

From the above result, it is known that by solving the convex optimization problem,

a sub-optimal filter with filter gains designed in (3.12) could be achieved with FF $H_\infty$ performance $\gamma^{**}$, which is the minimum value of $\gamma$.

Remark 3.2. The conditions ${\bar P}^h > 0$, ${\bar P}^v > 0$, ${\hat P}^h > 0$ and ${\hat Q}^v > 0$ in Theorem 3.2 are implied by (3.8) and (3.9) in Theorem 3.3, utilizing the equivalent variable transformation.

4.   Simulation example

The filter design method will be applied to a practical process of gas absorption, which is represented by the following nonlinear differential equation

where $\varphi (p, q)$ is the variable function; $a_0$, $a_1$, $a_2$ and $b$ are real coefficients; $w(p, q)$ is the disturbance input; the BCs are

Define

where $T$ is the difference step. Next, the following continuous-discrete model can be obtained

with BCs

and

Take the membership functions

in consideration of two IF-THEN rules. System (4.2) could be further approximated by the following continuous-discrete T-S fuzzy system

IF ${\sin ^2}({x^v}(t, k))$ is about zero, THEN

IF ${\sin ^2}({x^v}(t, k))$ is about one, THEN

where

Letting ${a_0} = -2.4$, ${a_1} = -0.4$, ${a_2} = -5.5$, $b = - 0.1$ and $T = 0.2$, the other matrix parameters are also taken as follows for the purpose of discussion: ${E_1} = 1.5$, ${E_2} = 1$,

The aim is to design a fuzzy continuous-discrete filter in the form of (2.5) to guarantee the asymptotic stability and an FF $H_\infty$ performance of the FES (2.7). The FF domain of the disturbance input signal is taken from interval $[0.5, 13.06] \times [0, 1.57]$. Via solving the sub-optimal problem (3.18), the sub-optimal FF $H_\infty$ performance level $\gamma^{**} = 1.9112$ is obtained and the corresponding solution to the LMIs is available, which is partially presented for space consideration

By (3.12), the obtained matrix parameters of the FF $H_\infty$ filter are designed as follows: $D_{F, 1} = -0.1157$, $D_{F, 2} = 1.0978$,

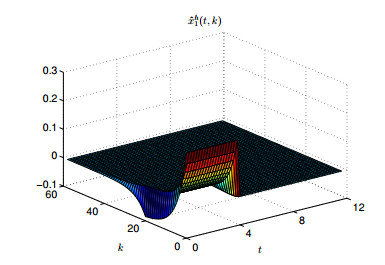
In the following, simulation results are provided. First, Figures 1–5 show the trajectories of $\hat x_1^h(t, k)$, $\hat x_2^h(t, k)$, $\hat x_1^v(t, k)$, $\hat x_2^v(t, k)$ and $\hat z(t, k)$ of the FES without disturbance input, respectively, where the BCs are

Figures 1–4 further show that the FES is AS. Next, take the disturbance input as follows:

where $u(t)$ is the unit step function. Applying the Fourier transformation, the FF domain of the disturbance input signal (4.5) is $[0.5, 13.06] \times [0, 1.57]$. Under the zero BCs, it can be calculated that ${\| \hat z\|}_2/{{\| w\|}_2}\doteq 1.5338$ with the disturbance input (4.5). Thus, the constraint (2.9) is satisfied, which means that the FES satisfies a prescribed FF $H_\infty$ performance $\gamma^{**} = 1.9112$.

Finally, to investigate the relationship between the size of the FF domain and the conservativeness of the proposed result, the obtained sub-optimal values of $\gamma$ based on Theorem 3.3 for different FF sets of disturbances are displayed in Table 1. From Table 1, we find that, for a fixed continuous component interval, $\gamma^{**}$ becomes larger as the discrete one becomes wider; while for a fixed discrete component interval, if the continuous part is wider, then the obtained $\gamma^{**}$ is larger. That is, a larger FF domain leads to a more conservative filtering performance.

5.   Conclusions

The filter design problem has been concerned for the continuous-discrete T-S fuzzy systems in the Roesser model with FF disturbances. The systematic method has been proposed for the filter design, with which the FES was AS and had an FF $H_\infty$ performance. A simulation case further showed validity of the results discussed above. Applying the similar techniques to FF fault detection problems and extending the proposed technique to the 2-D T-S fuzzy systems with delays are important and challenging research topics that deserve more future efforts.

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgments

This work was supported in part by the National Natural Science Foundation of China under Grant (No. 62373191), in part by the China Postdoctoral Science Foundation funded project under Grant (No. 2020M671293), in part by the Jiangsu Planned Projects for Postdoctoral Research Fund under Grant (No. 2020Z125), and in part by the Fundamental Research Funds for the Central Universities under Grant (No. 2242023K40010).

Conflict of interest

All authors declare that there are no conflicts of interest in this paper.