Solvability of the Sylvester equation $AX-XB = C$ under left semi-tensor product

1.   Introduction and Preliminaries

Let $G$ be a finite, simple and connected graph. A family of subetaaphs $H_1, H_2, \dots, H_t$ is called an edge-covering if every edge from $E(G)$ belongs to at least one of the subetaaphs $H_i$, $i = 1, 2, \dots, t$. When $H_i, i = 1, 2, \dots, t$ is isomorphic to a given graph $H$, then graph $G$ admits an $H$-covering. $G$ is called an $(\alpha, d)$-$H$-antimagic if there exists a total labeling $\phi:V(G)\cup E(G) \to \{1, 2, \dots, v+e\}$ with the $H$-weights,

forming an arithmetic progression $\alpha, \alpha+d, \alpha+2d, \dots, \alpha+(t -1)d$, where $\alpha > 0$ and $d\ge 0$ are two integers and $t$ is the number of all subetaaphs of $G$ isomorphic to $H$. Moreover, $G$ is said to be super $(\alpha, d)$-$H$-antimagic if $\phi(V(G)) = \{ 1, 2, 3, \dots, |V(G)|\}$.

The $H$-supermagic graph was first introduced by Gutiérrez and Lladó in ^[3]. Some other results can be seen from ^{[4,7,8,9,10,11]}. An $(a, d)$-$H$-antimagic labeling was introduced by Inayah et al. ^[5]. The further results on antimagic labeling are discussed in ^[2,6,16]. In ^[12], authors discussed the supermagic and super $(\alpha, 1)$-$C_4$-antimagic labeling of book graph and its disjoint union. The super $(a, d)$-$C_3$-antimagicness of a corona graph for differences $d\in\{0, 1, \dots, 5\}$ is discussed in ^[1]. M. A. Umar ^[14] study the existence of the super cycle-antimagic labeling of ladder graphs for differences $d\in\{0, 1, \dots, 15\}$. M.A.Umar et al. ^[13] gives the super $(\alpha, d)$-$C_4$-antimagic labeling of book graphs for differences $d = 1, 2, \dots, 13$.

In this research manuscript, we investigated the existence of super $(\alpha, 1)$-$C_4$-antimagic labeling of stacked book graphs $SB_{(p, q)}$ that can be thought of as generalization of a book graph and super $(\alpha, 1)$-$C_{4(r+1)}$-antimagic labeling of its r subdivided graph $SB_{(p, q)}(r)$.

2.   Cycle-antimagic labeling of stacked book graphs

A Cartesian product of two graphs $G_1$ and $G_2$, denoted by $G_1 \Box G_2$, is the graph with vertex set $V(G_1) \Box V(G_2)$, where two vertices $(u, u')$ and $(v, v')$ are adjacent if and only if $u = v$ and $u'v' \in E(G_2)$ or $u' = v'$ and $uv \in E(G_1)$.

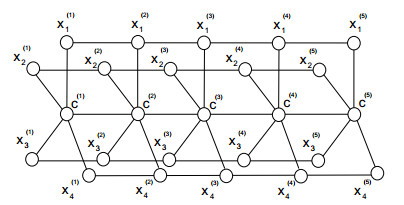
A stacked book graph denoted by $SB_{(p, q)}$ is defined as the cartesian product of a star graph $S_p$ on $p+1$ vertices with a path $P_q$ on $q$ vertices. i.e., $SB_{(p, q)}\cong S_{p+1}\Box P_q$, where the symbol $\Box$ used to denote the cartesian product of two graphs. The stacked book graph $SB_{(p, q)}$ contains $q(p+1)$ vertices and $q(2p+1)-(p+1)$ edges.

The vertex set $V(SB_{(p, q)})$ have the elements $\{c^{(j)}, x_i^{(j)}: 1 \leq i \leq p, 1 \leq j \leq q\}$ and the edge set $E(SB_{(p, q)})$ have the elements

A typical picture of stacked book graph $SB_{(p, q)}$ is given in Figure 1:

Clearly stacked book graph $SB_{(p, q)}$ admits $C_4$-covering. It will be worth noting for $p = 1$, the stacked book graph $SB_{(1, q)}$ is a ladder graph $P_2\Box P_q$, for $p = 2$, the stacked book graph $SB_{(2, q)}$ is a grid graph $P_2\Box P_q$ and for $q = 2$, the stacked book graph $SB_{(p, 2)}$ is a book graph $P_p\Box P_2$. Ming-Ju Lee et al. describe the super $(\alpha, 1)$-cycle-antimagic labeling of grid graph $P_p\Box P_q$ in ^[15]. M. A. Umar et al. ^[12] give the supermagic and super $(\alpha, 1)$-$C_4$-antimagic labeling of book graph and its disjoint union while ^[13] describes the super $(\alpha, d)$-$C_4$-antimagic labeling of book graphs for differences $d = 1, 2, \dots, 13$. Therefore we consider $p, q\geq3$ in this paper.

Let $C_{4}^{i, j}$ be the $(i, j)^{\text{th}}$ cycle for $1\leq i\leq p, 1\leq j\leq q-1$ in $SB_{(p, q)}$. Each $(i, j)^{\text{th}}$-cycle $C_{4}^{i, j}$ in $SB_{(p, q)}$ has the vertex set $\{c^{(j)}, c^{(j+1)}, x_i^{(j)}, x_i^{(j+1)}\}$ and the edge set $\{c^{(j)}c^{(j+1)}, x_i^{(j)}x_i^{(j+1)}, c^{(j)}x_i^{(j)}, c^{(j+1)}x_i^{(j+1)}\}$.

The corresponding $C_{4}^{i, j}$-weight under a total labeling $\phi$ would be:

For our convenience, throughout this paper by $i = \overline{1, p}$, we mean $i = 1, 2, \dots, p$ and vice versa.

Theorem 1. Let $p, q \geq 3$ be positive integers and $S_p$ be a star on $p+1$ vertices. Then stacked book graph $SB_{(p, q)}$ admits a super $(\alpha, 1)$-$C_4$-antimagic labeling.

Proof. The total labeling $\phi_0$ have the form:

Evidently,

and therefore,

Equations (2.1) and (2.2) gives:

For convenience, define $wt_{\phi_0}(\text{partial}) = 8q(p+1)+2+\lceil\frac{q}{2}\rceil$.

Therefore the $C_{4}^{i, j}$-weights are:

which makes the total labeling $\phi_0$ a super $(\alpha, 1)$-$C_4$-antimagic labeling and the proof is complete.

3.   Cycle-antimagic labeling of $r$-subdivided stacked book graph

Let $G$ be a graph and $r\geq1$ be a positive integer. By $G(r)$, we define $r$-subdivided graph of $G$ constructed by inserting $r$ new vertices into every edge of $G$.

In this way, $SB_{(p, q)}(r)$ is the $r$-subdivided graph of stacked book graph with the vertex set $\{c^{(j)}, x_i^{(j)}, u_{r}^{(i, j)}:1\leq i \leq p, 1\leq j\leq q\} \cup \{\epsilon_r^{(j)}, \delta_r^{(i, j)}:1\leq i \leq p, 1\leq j \leq q-1 \}$ and the edge set

where $u_{r}^{(i, j)}, \ \epsilon_r^{(j)}, \ \delta_{r}^{(i, j)}$ are $r$ new vertices inserted into the edges $c^{(j)}x_i^{(j)}, \ c^{(j)}c^{(j+1)}$ and $x_i^{(j)}x_i^{(j+1)}$ respectively. Clearly $r$-subdivided stacked book graph $SB_{(p, q)}(r)$ admits $C_{4(r+1)}$-covering.

Let $C_{4(r+1)}^{i, j}$ be the $(i, j)^{\text{th}}$-cycle for $1\leq i\leq p, 1\leq j\leq q-1$ in $SB_{(p, q)}(r)$. Each $(i, j)^{\text{th}}$-cycle $C_{4(r+1)}^{i, j}$ in $SB_{(p, q)}(r)$ has the vertex set

and the edge set $\{u_{k}^{(i, j)}u_{k+1}^{(i, j)}, u_{k}^{(i, j+1)}u_{k+1}^{(i, j+1)}, \epsilon_{k}^{(j)}\epsilon_{k+1}^{(j)}, \delta_{k}^{(i, j)}\delta_{k+1}^{(i, j)}:1\leq k\leq r-1 \}\cup$

$C_{4(r+1)}^{i, j}$-weight under a total labeling $\phi$ would be:

where

Theorem 2. Let $p, q \geq 3$ and $r\geq1$ be positive integers and $SB_{(p, q)}(r)$ be $r$-subdivided stacked book graph then $SB_{(p, q)}(r)$ admits a super $(\beta, 1)$-$C_{4(r+1)}$-antimagic labeling.

Proof. The total labeling $\phi$ have the form:

Using expressions (3.2), (3.3) and (3.4), we have:

where $\text{SPartial}_3 = r(r+1)(q-1)(2p+3)+q(2pr^2+5pr+2p+2r+1)+r$

One can observe here that the $\text{Partial}_1+2\text{Partial}_2+\text{SPartial}_3$ are independent of $i$ and $j$. Equation (3.8) clearly shows that $wt_{\phi}(C_{4(r+1)}^{i, j})$ only depends on $i$ and $j$. Equation (3.8) with equation (2.4) proves that $SB_{(p, q)}(r)$ admits a super $(\beta, 1)$-$C_{4(r+1)}$-antimagic labeling which completes the proof.

4.   Conclusion

In this manuscript, we prove results related to super $(\alpha, 1)$-$C_4$-antimagic labeling of stacked book graphs $SB_{(p, q)}$ and super $(\alpha, 1)$-$C_{4(r+1)}$-antimagic labeling of its r subdivided graph $SB_{(p, q)}(r)$. One can extend these results for other differences $d$ and for disjoint union of stacked book graphs. One can also prove results about applications of graph labeling in data science and communication networks.

Acknowledgments

The study was supported by the Key Industrial Technology Development Project of Chongqing Development and Reform Commission, China (Grant No. 2018148208), Key Technological Innovation and Application Development Project of Chongqing, China (Grant No. cstc2019jscx-fxydX0094), Innovation and Entrepreneurship Demonstration Team of Yingcai Program of Chongqing, China (Grant No. CQYC201903167), Science and Technology Innovation Project of Yongchuan District (Ycstc,2020cc0501).

The research was supported by the National Natural Science Foundation of China (Grant Nos. 11971142, 11871202, 61673169, 11701176, 11626101, 11601485).

The authors are grateful to the anonymous reviewers of this journal who helped to improve the paper.

Conflict of interests

The authors declare that there is no conflict of interests.