More complex dynamics in a discrete prey-predator model with the Allee effect in prey

1.   Introduction

Prey-predator interaction among populations is a kind of universal phenomena, which may lead to a stable coexistence and population cycles. Some population problems, such as the persistence, the stability of steady states, the bifurcation, etc. have been research focal points. How to deal with these problems is the main question. An effective method is to adopt reasonable assumptions and establish appropriate mathematical models to analyze such problems. So, mathematical modeling is a tool to deal with these problems, as it can give some insight into the corresponding model dynamics. Among the mathematical models, the Lotka-Volterra model is a famous prey-predator one, and it was independently constructed by Lotka ^[1] and Volterra ^[2], respectively. These mathematical models have two different kinds of types: continuous-time models and discrete-time models. Over the last several decades, an increasing number of studies have investigated discrete-time prey-predator models. There are two reasons for this. One reason is that discrete models are sometimes more appropriate as a tool to describe the evolution rule for the population than continuous models when the population size is small or when the generation of the population does not overlap. The other reason is that some discrete models have more diverse dynamical behaviors than the corresponding continuous models. For example, it is well known that the single-species discrete-time logistic model has very complex dynamical behavior, ranging from period-doubling bifurcation to chaotic behavior ^[3], whereas the solutions of the corresponding continuous model are monotonic.

To date, the investigations of bifurcation problems for discrete prey-predator systems have already received much attention, especially after Smith ^[4] considered the Lotka–Volterra type discrete-time prey-predator model. For this, refer to ^{[5,6,7,8,9,10,11]}.

Focusing on the deeper research, people have started to consider an important ecological mechanism that describes realistic interactions among species in the ecosystem. For the readers' convenience, we present some existing works ^{[12,13,14,15,16]}. Li et al. considered the interactions of time delay and spatial diffusion to induce the periodic oscillation of a vegetation system ^[12]. Chowdhury et al. deduced canard cycles, relaxation oscillations and pattern formation for a slow-fast ratio-dependent predator-prey system ^[13]. Sun et al. investigated the impacts of climate change on vegetation patterns ^[14]. Chowdhury et al. studied eco-evolutionary cyclic dominance among predators, prey and parasites ^[15]. The dynamic behavior of a plant-water model with spatial diffusion has been analyzed by Sun et al. ^[16]. But one must remember that this work was first considered by the well known ecologist W. C. Allee and later named the Allee effect ^{[17,18,19,20,21,22]}. The Allee effect, classified into two kinds of types, i.e., the strong Allee effect and weak Allee effect, states that a population that is low in number is affected by a positive relationship between the population growth rate and its density. In the process of the population growing, a critical density, called the Allee threshold, will occur and measure the extinction of the population. The Allee effect is used to study the extinction scenario of the population. When the average growth rate of the population at low density is negative, one says that there is a strong Allee effect whereas a weak Allee effect corresponds to when the average growth rate of the population at zero density is positive. For more investigations of the Allee threshold under the strong/weak Allee effect, see also, for example, ^{[17,18,19,20,21,22,23,24]} and the references cited therein. In fact, most of the research is on the codim 1 bifurcation problem of such systems. Therefore, our main aim in this paper is to consider the codim 2 bifurcation problem of a discrete prey-predator model with the Allee effect in prey.

Recall that Khan ^[25] considered the local stability and Neimark-Sacker bifurcation of the following discrete-time prey-predator model

where $X_t$ and $Y_t$ denote the numbers of prey and predator respectively, and the parameters $a$ and $\beta$ are positive real numbers.

A number of changes in the model (1.1) have been presented by researchers to include Holling-type functional responses and density-dependent prey growth. Another way of modification of the Lotka–Volterra model can be considered by introducing the Allee effect which can more realistically model the prey–predator interaction.

In ^[26], the author considered the modification of the model (1.1) by introducing the Allee effect to the prey population as follows

where $\frac{X_t}{X_t+m}$ is the term for the Allee effect, $m > 0$ can be defined as the Allee effect coefficient ^[12], and the parameters $a$ and $\beta$ are the growth rates of the prey and predator, respectively.

Considering the biological meanings of system (1.2), its nonnegative fixed points were derived in ^[26], and they are $E_1 = (0, 0)$, $E_2 = (\frac{a-1}{a}, 0)$ if $a > 1$ and $E_* = (\beta, \dfrac{(\beta+m)(a(1-\beta)-1)}{\beta})$ if $0 < \beta < 1-\frac{1}{a}.$

Regarding the stability analysis of $E_1,$ $E_2$ and $E_*,$ some results have been obtained in ^[26]. For the readers'convenience, we present them in Appendix A.

On the one hand, however, based on those results presented in Appendix A, which are mainly for the type and the stability of the positive fixed point $E_*,$ we find that there are some errors, which can be shown as follows.

Counterexample 1.1. Consider system (1.2) with the parameters

Obviously, $0 < \beta < 1-\frac{1}{a},$ which means that $E_*$ exists. Again, $\frac{1}{1-2\beta-m} < a < \frac{3\beta+5m}{3\beta^2+m\beta+m-\beta},$ which means that ($iii$.2) in Appendix A holds. According to ($iii$.2), $E_*$ should be a source. But, by some computations, one finds that

which shows that the fixed point $E_*$ is a saddle, not a source.

Counterexample 1.2. Consider system (1.2) with the parameters

Evidently, $0 < \beta < 1-\frac{1}{a},$ which means that $E_*$ exists. $a > \frac{1}{1-2\beta-m}$ is also true. According to the condition ($iii$.3) in Appendix A, $E_*$ should be a saddle. But one can easily derive the following:

which indicates that $E_*$ is an unstable focus, not a saddle.

These counterexamples show that the stability of $E_*$ is worthy of further studies.

On the other hand, the author of ^[26] only studied codim 1 Neimark-Sacker bifurcation in $E_*.$ A question naturally arises: are there other bifurcations in $E_*,$ especially, 1:2 resonance bifurcation? Additionally, are there bifurcations that occur in the fixed points $E_1$ and $E_2$?

These problems have not yet been considered in any known studies. This has motivated us to investigate these problems in this paper. We not only give precise conditions for the stability of $E_*$ in the whole parametric space in which it exists, which serves to correct those known errors, but, also, and what is more important, we consider the complex bifurcation problems of (1.2), mainly focusing on its codim 2 resonance 1:2 bifurcation.

The rest of the paper is organized as follows. In Section 2, we give the complete stability results for $E_*$ for the entire parametric space in which it exists. In Section 3, we respectively choose $a$ and $\beta$ as bifurcation parameters to discuss its bifurcation problems at the fixed points $E_1$ and $E_2$, including transcritical bifurcation and period-doubling bifurcation. For the fixed point $E_*,$ we not only consider its codim 1 period-doubling bifurcation, but also the 1:2 resonance bifurcation of codim 2 while varying the two parameters $a$ and $m.$ In Section 4, we present some numerical simulations to illustrate our theoretical analysis results. Finally, we draw some conclusions and discussions in Section 5.

2.   Stability of fixed point $E_*$

In this section, we revisit the stability of the fixed point $E_*$ of system (1.2). In order to study the stability and local bifurcation of fixed points of a general 2D system, the definitions of the topological types of a fixed point and a lemma for determining the stability of a fixed point are essential, and they are presented in Appendix B. The main results in this section are as follows.

Theorem 2.1. When $0 < \beta < 1-\frac{1}{a},$ the unique positive fixed point $E_*$ exists. Its dynamical properties are completely formulated in Table 1, where $R_1 = \dfrac{3\beta^2+(m-1)\beta+m}{5m+3\beta}$ and $R_2 = 1-2\beta-m.$

Proof. The characteristic equation of $J(E_*)$ is

where $B = \frac{-2\beta-3m+am+a\beta^2}{m+\beta},$ $C = \frac{a\beta-2a\beta^2-a\beta m+m}{m+\beta}.$

Now, we will analyze the stability of the unique positive fixed point $E_*.$ Notice that $F(1) > 0$ always holds when $0 < \beta < 1-\frac{1}{a}.$ Obviously,

and

If $1 < a \le 2$, then $(0, 1-\frac{1}{a}) \subset (0, \frac{1}{2})$; if $2 < a$, then $(0, \frac{1}{2}) \subset (0, 1-\frac{1}{a})$. Now for $a > 2,$ we separate $\beta\in(0, 1-\frac{1}{a})$ into two cases: $\beta$ $\in(0, \frac{1}{2})$ and $\beta$ $\in[\frac{1}{2}, 1-\frac{1}{a}).$

Case Ⅰ: $\frac{1}{2}\leqslant\beta < 1-\frac{1}{a}.$

Then, $\frac{4-9\beta}{5} < 0 < m$. So, it follows from (2.1) that $R_1 > R_2,$ and $1-2\beta-m < 0 < \frac{1}{a}.$ So $C < 1.$ Notice the following condition:

Hence, one can further divide $\beta$ $\in[\frac{1}{2}, 1-\frac{1}{a})$ into two subcases $\beta$ $\in[\frac{1}{2}, \frac{2}{3})$ and $\beta$ $\in[\frac{2}{3}, 1-\frac{1}{a})$ if possible.

Subcase Ⅰ: $\frac{1}{2}\leqslant\beta < \frac{2}{3}.$ Then the following derivations hold true.

1) $0 < \frac{1}{a} < R_1.$ It follows from (2.3) that $F(-1) < 0.$ According to Lemma 5.2(ⅰ.3), the eigenvalues are $|\lambda_1| < 1$ and $|\lambda_2| > 1.$ So, Definition 5.1(3) tells us that $E_*$ is a saddle.

2) $\frac{1}{a} = R_1.$ One can see from (2.3) that $F(-1) = 0$ and $C\neq1.$ Lemma 5.2(ⅰ.2) yields that the eigenvalues are $\lambda_1 = -1$ and $\lambda_2\neq1.$ By Definition 5.1(4), $E_*$ is non-hyperbolic.

3) $R_1 < \frac{1}{a} < 1-\beta.$ Then from (2.3) we know that $F(-1) > 0$ and $C < 1.$ According to Lemma 8.2(ⅰ.1), the eigenvalues satisfy that $|\lambda_{1, 2}| < 1.$ From Definition 5.1(1), $E_*$ is a sink.

Subcase Ⅱ: $\frac{2}{3}\leqslant\beta < 1-\frac{1}{a}.$ Then $0 < \dfrac{1}{a} < 1- \beta < R_1.$ Using (2.3), Lemma 5.2(ⅰ.3) and Definition 5.1(3) in the same way, it is easy to derive that $E_*$ is a saddle.

Case Ⅱ: $0 < \beta < \frac{1}{2}$.

Then, we consider two subcases: Ⅰ: $0 < \beta < \frac{4}{9}$ and Ⅱ: $\frac{4}{9}\leqslant\beta < \frac{1}{2}.$ First consider Subcase Ⅰ: $0 < \beta < \frac{4}{9}.$ We divide $m\in(0, +\infty)$ into three cases:

Correspondingly, $R_1 < ( = , > )R_2.$ For the first case that $0 < m < \frac{4-9\beta}{5}, R_1 < R_2.$ Then consider $\frac{1}{a}\in(0, 1-\beta)$ for the following five cases:

1) $0 < \frac{1}{a} < R_1 \Longrightarrow F(-1) < 0\Longrightarrow |\lambda_1| < 1, |\lambda_2| > 1\Longrightarrow$ $E_*$ is a saddle;

2) $\frac{1}{a} = R_1 \Longrightarrow F(-1) = 0, C\neq1\Longrightarrow\lambda_1 = -1, \lambda_2\neq-1\Longrightarrow$ $E_*$ is non-hyperbolic (flip bifurcatin may occur);

3) $R_1 < \frac{1}{a} < R_2\Longrightarrow F(-1) > 0, C > 1\Longrightarrow |\lambda_{1, 2}| > 1\Longrightarrow$ $E_*$ is a source;

4) $\frac{1}{a} = R_2\Longrightarrow C = 1, F(-1) > 0\Longrightarrow |\lambda_{1, 2}| = 1, \lambda_1 = \overline{\lambda_2}\Longrightarrow$ $E_*$ is non-hyperbolic (Neimark-Sacker bifurcation possibly occurs);

5) $R_2 < \frac{1}{a} < 1-\beta\Longrightarrow C < 1, F(-1) > 0\Longrightarrow|\lambda_{1, 2}| < 1\Longrightarrow$ $E_*$ is a sink.

For the second case of $m = \frac{4-9\beta}{5} (R_1 = R_2)$ and the third case that $m > \frac{4-9\beta}{5}(R_1 > R_2)$, $\frac{1}{a}\in(0, 1-\beta)$ can be similarly divided. For simplification, these cases can be shown in the following formats.

Next consider Subcase Ⅱ: $\frac{4}{9}\leqslant\beta < \frac{1}{2}.$ Then one can get that $R_1 > R_2.$ Similar to the analysis for Subcase Ⅰ, one can derive the following:

this may be reduced to the following:

This case is the same as the case that $\frac{1}{2}\leqslant\beta\leqslant \frac{2}{3}$. So, these two cases can be combined into one case. Thus, we have summarized all of the results in Table 1.

3.   Bifurcation analysis

In this section, we respectively consider the local bifurcation problems of system (1.2) at the fixed points $E_1$, $E_2$ and $E_*,$ including codimension one (codim 1) bifurcation and codimension two (codim 2) bifurcation.

3.1.   Codimension one bifurcation

3.1.1.   Bifurcation at the trivial fixed point $E_1$(0, 0)

For the bifurcation at the fixed point $E_1(0, 0),$ one has the following result.

Theorem 3.1. As the parameter $a$ passes through the critical value $a_0 = 1,$ system (1.2) undergoes a transcritical bifurcation at the fixed point $E_1(0, 0).$

Proof. Given a small perturbation $a^*$ of the parameter $a$ around $a_0$, namely, $a^* = a-a_0$, with $0 < |a^*|\ll 1$, system (1.2) is changed into the following form:

Set $a_{t+1}^* = a_t^* = a^*;$ then, (3.1) becomes

Applying Taylor expansion to (3.2) at ($x_t, y_t, a_t^*$) = (0, 0, 0) yields

where $\rho_1 = \sqrt{x_t^2+y_t^2+(a_t^*)^2}$,

Assume the following for the center manifold

where $\rho_2 = \sqrt {x_t^2+{a_t^*}^2};$ then, from

comparing the corresponding coefficients of terms with the same order as in the above center manifold equation, it is easy to derive that

So, system (3.3), restricted to the center manifold, is given by

Therefore, the following results are derived:

According to (21.1.43)–(21.1.46) in [27, pp 507], all conditions hold for a transcritical bifurcation to occur; hence, system (1.2) undergoes a transcritical bifurcation at the fixed point $E_1$.

3.1.2.   Bifurcation at the boundary fixed point $E_2=(\frac{a-1}{a}, 0)$

The fixed point $E_2$ always exists when $a > 1$. One can see from ^[26] that $E_2$ is a non-hyperbolic fixed point when $a = 3$ or $\beta = \frac{a-1}{a}$. As soon as the parameter $a$ or $\beta$ goes through the corresponding critical values, the dimensional numbers for the stable manifold and the unstable manifold of the fixed point $E_2$ vary. So, a bifurcation probably occurs. Now, the considered parameter values are divided into the following three cases:

Case Ⅰ: $a = 3, \beta \ne \frac{2}{3}$;

Case Ⅱ: $a \ne 3, \beta = \frac{a-1}{a}$;

Case Ⅲ: $a = 3, \beta = \frac{2}{3}$.

First, we consider Case Ⅰ: $a = 3, \beta \ne\frac{2}{3}$, namely, the parameters $(a, \beta) \in \Omega_1 = \{(a, \beta) \in R^2_{+}| \beta \ne \frac{2}{3}, a > 1 \}$. Then, the following result is obtained.

Theorem 3.2. Assume the parameters $(a, \beta) \in \Omega_1 = \{(a, \beta) \in R^2_{+}| \beta \ne \frac{2}{3}, a > 1 \}$, and let $a_0 = 3;$ then, system (1.2) undergoes a period-doubling bifurcation at $E_2$ when the parameter $a$ goes through the critical value $a_0$.

Proof. Let $l_t = X_t-\frac{a-1}{a}$ and $m_t = Y_t-0$, which transforms $E_2 = (\frac{a-1}{a}, 0)$ to the origin $O(0, 0)$ and system (1.2) into the following:

Give a small perturbation $a^*$ of the parameter $a$ around $a_0,$ namely, $a^* = a-a_0$, with $0 < |a^*|\ll 1;$ then, system (3.4) is changed into the following:

Set $a_{t+1}^* = a_t^* = a^*$ to change (3.5) into the following:

Applying a Taylor expansion to (3.6) at ($l_t, m_t, a_t^*$) = (0, 0, 0) yields

where $\rho_1 = \sqrt{l_t^2+m_t^2+(a_t^*)^2}$,

It is easy to derive the three eigenvalues of the matrix $A = \left({\begin{array}{*{20}{c}} -1 & \frac{-4}{3(3m+2)} & 0 \\ 0 & \frac{2}{3\beta} & 0\\ 0 & 0 & 1 \end{array}} \right)$ to be $\lambda_1 = -1$, $\lambda_2 = \frac{2}{3\beta}$ and $\lambda_3 = 1$ with the corresponding eigenvectors $\xi_1 = \left({\begin{array}{*{20}{c}} 1 \\ 0\\ 0 \end{array}} \right),$ $\xi_2 = \left({\begin{array}{*{20}{c}} \frac{3\beta}{2+3\beta} \\ \frac{-3(3m+2)}{4}\\ 0 \end{array}} \right)$ and $\xi_3 = \left({\begin{array}{*{20}{c}} 0\\ 0\\ 1 \end{array}} \right)$. $\beta \ne\frac{2}{3}$ implies that $|\lambda_2| \ne 1$.

Set $T = (\xi_1, \; \xi_2, \; \xi_3),$ namely,

The transformation $\left({\begin{array}{*{20}{c}} l_t\\ m_t\\ a_t^* \end{array}} \right) = T \left({\begin{array}{*{20}{c}} u_t\\ v_t\\ \sigma_t \end{array}} \right)$ changes system (3.7) into the following:

where $\rho_2 = \sqrt {u_t^2+v_t^2+\sigma_t^2},$

Assume the following for the center manifold ^[28]:

where $\rho_3 = \sqrt {u_t^2+\sigma_t^2};$ then, according to the following relations:

and by comparing the corresponding coefficients of terms with the same order as in the above center manifold equation, one derives that

So, system (3.8) restricted to the center manifold is given by

Thus, one has

Therefore, the following results are derived:

which, according to (21.2.17)–(21.2.22) in [27, pp 516], satisfy all of the conditions for a period-doubling bifurcation to occur. So, system (1.2) undergoes a period-doubling bifurcation at $E_2$. Again,

So, the period-two orbit bifurcated from $E_2$ lies to the right of $a_0 = 3$.

Of course, one can also compute the following two quantities, which respectively, are the transversal condition and non-degenerate condition for judging the occurrence and direction of a period-doubling bifurcation (see ^[27,28]):

It is easy to get that $\alpha_1 = -1$ and $\alpha_2 = 9$. Because $\alpha_1 \ne0$ and $\alpha_2 > 0$, a period-doubling bifurcation occurs in system (1.2) at $E_2$ and the period-two orbit bifurcating from $E_2$ is stable. The proof is complete.

Next, we study Case Ⅱ: $a \ne 3$, $\beta = \frac{a-1}{a}$. We can see that $|\lambda_1|\ne1$ and $\lambda_2 = -1$ when $a \ne 3$ and $\beta = \frac{a-1}{a}$. Thus, the following result can be derived.

Theorem 3.3. Assume the parameters $(a, \beta) \in \Omega_2 = \{(a, \beta) \in R^2_{+}| a \in (1, 3)\bigcup(3, +\infty)\},$ and set $\beta_0 = \frac{a-1}{a}$. If the parameter $\beta$ goes through the critical value $\beta_0$, then system (1.2) at $E_2$ undergoes a transcritical bifurcation.

Proof. Shifting $E_2 = (\frac{a-1}{a}, 0)$ to the origin $O(0, 0),$ applying a small perturbation $\beta^*$ of the parameter $\beta$ with $0 < |\beta^*|\ll 1$ and letting $\beta_{t+1}^*$ = $\beta_t^*$ = $\beta^*,$ system (3.4) is changed into the following form:

Applying a Taylor expansion to (3.9) at $(l_t, m_t, \beta_t^*) = (0, 0, 0)$ produces

where $\rho_4 = \sqrt{l_t^2+m_t^2+{\beta_t^*}^2},$

It is not difficult to derive the three eigenvalues of the matrix $A = \left({\begin{array}{*{20}{c}} 2-a & \frac{-(a-1)^2}{a(ma+a-1)} & 0 \\ 0 & 1 & 0\\ 0 & 0 & 1 \end{array}} \right)$ to be $\lambda_1 = 2-a$ and $\lambda_{2, 3} = 1,$ with the corresponding eigenvectors $\xi_1 = \left({\begin{array}{*{20}{c}} 1 \\ 0\\ 0 \end{array}} \right),$ $\xi_2 = \left({\begin{array}{*{20}{c}} \frac{1}{1-a} \\ \frac{a(ma+a-1)}{(a-1)^2}\\ 0 \end{array}} \right)$ and $\xi_3 = \left({\begin{array}{*{20}{c}} 0\\ 0\\ 1 \end{array}} \right)$. The condition $a \in (1, 3)\bigcup(3, +\infty)$ shows that $|\lambda_1| \ne 1$.

Take $T = \left({\begin{array}{*{20}{c}} 1 & \frac{1}{1-a} & 0\\ 0 & \frac{a(ma+a-1)}{(a-1)^2} & 0\\ 0 & 0 & 1 \end{array}} \right); \text{ then, }\; T^{-1} = \left({\begin{array}{*{20}{c}} 1 & \frac{(a-1)}{a(ma+a-1)} & 0\\ 0 & \frac{(a-1)^2}{a(ma+a-1)} & 0\\ 0 & 0 & 1 \end{array}} \right).$

Using the transformation

system (3.9) is changed into the following:

where $\rho_5 = \sqrt {u_t^2+v_t^2+\sigma_t^2},$

Assume the following for this center manifold:

where $\rho_6 = \sqrt {v_t^2+\sigma_t^2}$, which must satisfy

Comparing the corresponding coefficients of terms to the same type in the above equations produces

That is to say,

Thus, one has

Hence, the following equations may be obtained:

According to (21.1.43)–(21.1.46) in [27, pp 507], all conditions are true for a transcritical bifurcation to occur; hence, system (1.2) undergoes a transcritical bifurcation at the fixed point $E_2$.

Finally, for the bifurcation of system (1.2) at $E_2$ in Case Ⅲ: $a = 3, \beta = \frac{2}{3}$, which implies that $\lambda_1 = -1$ and $\lambda_2 = 1,$ a fold-flip bifurcation may occur there. This is left as our future work.

Remark. For the fold-flip bifurcation, refer also to ^[29,30].

3.1.3.   Bifurcation analysis at the positive fixed point $E_*=(x^*, y^*$)

From Theorem 2.1 and Table 1, it is clear that $F(1) > 0,$ $F(-1) = 0$ and $B\ne2$ when $0 < \beta < \frac{2}{3},$ $m\notin\{\frac{\beta(1-3\beta)}{\beta+1}, \frac{\beta(1-3\beta)}{\beta-3}, \frac{4}{5}-\frac{9}{5}\beta\}$ and

At this time, the eigenvalues are $\lambda_1 = -1$ and $\lambda_2 = \dfrac{6\beta^2+4\beta m-m-3\beta}{3\beta^2+\beta m+m-\beta},$ with $|\lambda_2|\ne1$. Moreover, when the parameter $a$ goes through the critical value $a_0$, the dimensional numbers for the stable manifold and the unstable manifold of the fixed point $E_*$ vary. Hence, a bifurcation is likely to occur. In what follows, we consider the bifurcation problem of system (1.2) in this case.

Notice that the parameters $a,$ $\beta$ and $m$ belong to the set

Take $l_t = X_t-x^* = X_t-\beta$ and $m_t = Y_t-y^* = Y_t-\dfrac{(\beta+m)(a(1-\beta)-1)}{\beta};$ then, system (1.2) is changed into the following form:

Take a small perturbation $a^*$ of the parameter $a$ around $a_0$, i.e., $a^* = a-a_0$, with $0 < |a^*|\ll 1;$ then, system (3.14) becomes as follows:

Supposing that $a_{t+1}^* = a_t^* = a^*$, system (3.15) can be regarded as follows:

Applying a Taylor expansion to (3.16) at $(l_t, m_t, a_t^*) = (0, 0, 0)$ yields the following:

where $r_1 = \sqrt{l_t^2+m_t^2+{a_t^*}^2},$

$a_{200} = \frac{1}{3\beta^2+m\beta+m-\beta}(\frac{2m^2(3\beta-2)}{\beta(m+\beta)}-(3\beta+5m)), a_{110} = -\dfrac{\beta^2+2m\beta}{(m+\beta)^2},$

$a_{101} = -\frac{\beta^2+m}{\beta+m}, a_{020} = a_{011} = a_{002} = 0,$

$a_{300} = \frac{-2m^2(3\beta-2)}{\beta(m+\beta)^2(3\beta^2+m\beta+m-\beta)}, a_{210} = -\frac{m^2}{(m+\beta)^3}, a_{201} = -\frac{\beta^3+2m\beta^2+m^2}{\beta(\beta+m)^2},$

$a_{120} = a_{111} = a_{102} = a_{030} = a_{021} = a_{012} = a_{003} = 0,$

$b_{200} = 0, b_{110} = \frac{1}{\beta}, b_{101} = -\frac{(m+\beta)(\beta-1)}{\beta^2}, b_{020} = b_{011} = b_{002} = b_{300} = 0,$

$b_{210} = b_{201} = b_{120} = b_{111} = b_{102} = b_{030} = b_{021} = b_{012} = b_{003} = 0.$

It is easy to see that the three eigenvalues of the matrix $A = \left({\begin{array}{*{20}{c}} \dfrac{2m\beta-3m-\beta}{(3\beta^2+m\beta+m-\beta)} & \frac{-\beta^2}{m+\beta} & 0\\ \dfrac{-2(m+\beta)^2(3\beta-2)}{\beta^2(3\beta^2+m\beta+m-\beta)} & 1 & 0\\ 0 & 0 & 1 \end{array}} \right)$ are $\lambda_1 = -1$, $\lambda_2 = \dfrac{6\beta^2+4\beta m-m-3\beta}{3\beta^2+\beta m+m-\beta}$ and $\lambda_3 = 1,$ with the corresponding eigenvectors $\xi_1 = \left({\begin{array}{*{20}{c}} \frac{\beta^2(3\beta^2+m\beta+m-\beta)}{(m+\beta)^2(3\beta-2)} \\ 1\\ 0 \end{array}} \right)$, $\xi_2 = \left({\begin{array}{*{20}{c}} -\frac{\beta^2}{2(m+\beta)} \\ 1\\ 0 \end{array}} \right)$ and $\xi_3 = \left({\begin{array}{*{20}{c}} 0 \\ 0\\ 1 \end{array}} \right)$.

Notice that $m\ne \frac{4}{5}-\frac{9}{5}\beta$. So $|\lambda_2| \ne 1$.

then

Next, we consider the following translation:

Then system (3.17) is translated as follows:

where $r_2 = \sqrt {u_t^2+v_t^2+\sigma_t^2}$,

Assume for the center manifold that $v_t = m_{20}u_t^2+m_{11}u_t\sigma_t+m_{02}\sigma_t^2+o(r_3^2),$ where $r_3 = \sqrt {u_t^2+\sigma_t^2}$. It is easy to derive the following:

Hence, system (3.18) restricted to the center manifold is given by $u_{t+1} = : f_3(u_t, \sigma_t) = -u_t+a_{11}u_t\sigma_t+a_{20}u_t^2+a_{30}u_t^3+a_{21}u_t^2\sigma_t +a_{12}u_t\sigma_t^2+o(r_3^3),$ where

Next we calculate the following quantities to judge the occurrence of a period-doubling bifurcation. One can derive the following:

Notice that $\beta_1 = \dfrac{2(3\beta^2+m\beta-3m-\beta)(3\beta^2+m\beta+m-\beta)}{\beta(m+\beta)(9\beta+5m-4)}.$ Under the condition that $3\beta^2+m\beta-3m-\beta\neq0$ and $3\beta^2+m\beta+m-\beta\neq0,$ one can see that $\beta_1\neq0.$ At the same time,

or, equivalently,

So, according to [27, (21.2.17)–(21.2.22), pp 516], all conditions hold for a period-doubling bifurcation to occur. Accordingly, system (1.2) undergoes a period-doubling bifurcation at $E_*.$ Of course, the transversal condition and non-degenerate condition for judging the occurrence and direction of a period-doubling bifurcation are also given by the following two quantities:

It is easy to derive that $\gamma_1 = a_{11}\neq0$, so system (1.2) undergoes a period-doubling bifurcation at $E_*.$ Again,

In order to determine whether the period-doubling orbits that bifurcate from $E_*$ are stable, let

If $K > 0$ $(<0),$ the period-doubling orbits that bifurcate from $E_*$ are stable (unstable). Based on the above analysis, we have the following consequences for the period-doubling bifurcation of system (1.2) at $E_*$.

Theorem 3.4. Let the symbols $a_0,$ $\Theta$ and $K$ respectively be defined as in (3.12), (3.13) and (3.20). Assume the parameter vector $(a, \beta, m) \in \Theta$. Then system (1.2) undergoes a period-doubling bifurcation at $E_*$ when the parameter $a$ passes through the critical value $a_0.$ If $K > 0$ $(K < 0),$ the period-doubling bifurcation is supercritical (subcritical), as well as stable (unstable).

3.2.   Codimension two bifurcation

When $a = 3$ and $\beta = \frac{2}{3},$ the eigenvalues of system (1.2) at $E_2$ are $\lambda_1 = -\lambda_2 = 1.$ Then a fold-flip bifurcation probably occurs. Even a limit cycle of a closed invariant curve or a more complicated invariant set exists nearby. We will further study this case in the future.

In this subsection, we mainly consider codimension two bifurcation of system (1.2) at $E_*$ in the parameter space $\{(a, \beta, m)\lvert 0 < \beta < \frac{4}{9}, m > 0, a > 0\}$ when the two parameters $a$ and $m$ vary. When $m = m_0: = \frac{4}{5}-\frac{9}{5}\beta,$ $a = a_0: = \frac{5}{1-\beta}$ and $\lambda_{1, 2} = -1,$ then a 1:2 strong resonance bifurcation may occur there (see Section 9.5.3 in ^[30], Section 3.2 in ^[31] and Section 3 in ^[32,33]). The following result may be derived.

Theorem 3.5. Consider the parameter vector $(a, \beta, m)\in$ $\{ (a, \beta, m) \in R_+^3 \lvert 0 < \beta < \frac{4}{9}, \beta\neq\frac{2175}{637}-\frac{\sqrt{40978029}}{1911}\ and\ \beta\neq \frac{\sqrt{38187398689}}{39358}-\frac{17511}{3578} \}$. If the parameters $(a, m)$ vary in the neighborhood of $(a_0, m_0),$ then system (1.2) undergoes a codimension two bifurcation with 1:2 resonance at the fixed point $E_*.$ Moreover, around $E_*$ system (1.2) admits a pitchfork bifurcation and a non-degenerate Neimark-Sacker bifurcation.

Proof. In order to transfer the fixed point $E_*$ to the origin point $(0, 0),$ let $l_t = x_t-\beta$ and $m_t = y_t-\dfrac{(\beta+m)(a(1-\beta)-1)}{\beta};$ then, system (1.2) becomes

where $r_3 = \sqrt {l_t^2+m_t^2},$

$G_5(l_t, m_t) = (\dfrac{m^2[a(\beta-1)+1]}{\beta(m+\beta)^2}-a)l_t^2-\dfrac{\beta^2+2m\beta}{(m+\beta)^2}l_tm_t,$

$G_6(l_t, m_t) = \frac{1}{\beta}l_tm_t.$

Denote

where $\alpha$ = $(a, m).$

Then

The eigenvector and the generalized eigenvector of $A_0$ corresponding to the eigenvalue of –1 are respectively $q_0$ = $\left({\begin{array}{*{20}{c}} 1\\ 8(\beta-1)/(5\beta ^2) \end{array}} \right)$ and $q_1$ = $\left({\begin{array}{*{20}{c}} 1\\ 12(\beta-1)/(5\beta^2) \end{array}} \right)$. At the same time, the eigenvector and the generalized eigenvector of $A_0^T$ corresponding to the eigenvalue of –1 are $p_1$ = $\left({\begin{array}{*{20}{c}} -2\\ 5\beta^2/(4(\beta-1)) \end{array}} \right)$ and $p_0$ = $\left({\begin{array}{*{20}{c}} 3\\ 5\beta^2/(-4(\beta-1)) \end{array}} \right)$, respectively. These four vectors satisfy the following equalities:

where $\langle.\rangle$ stands for the standard scalar product in $\mathbb{R}^2$.

Make an invertible linear transformation:

and denote $x = \left({\begin{array}{*{20}{c}} l_{t} \\ m_{t} \end{array}} \right);$ then, the new coordinates $(u_n, v_n)$ can be computed explicitly as follows:

In the coordinates $(u_n, v_n),$ system (3.21) takes the following form:

where $r_4 = \sqrt {u_n^2+v_n^2+|\alpha|^2},$

also,

and

Clearly, $a(\alpha_0) = b(\alpha_0) = c(\alpha_0) = d(\alpha_0) = 0.$ Denoting the matrix

with the nonsingular linear coordinate transformation

system (3.24) can be written as

where $\varepsilon(\alpha) = d(\alpha)+b(\alpha)c(\alpha)-a(\alpha)d(\alpha),$ $\sigma(\alpha) = a(\alpha)+d(\alpha),$

Set

then $\beta_1(\alpha_0)$ = $\beta_2(\alpha_0) = 0.$ System (3.27) can be rewritten in the following form:

Expand $G$ and $H$ into Taylor series with respect to $x_n$ and $y_n$ to get

where $r_5 = \sqrt{x_n^2+y_n^2},$ $g_{20} = a_{20}(1+b(\alpha))-a_{11}a(\alpha)+\frac{a^2(\alpha)a_{02}}{1+b(\alpha)},$ $g_{11} = a_{11}-\frac{2a(\alpha) a_{02}}{1+b(\alpha)},$ $g_{02} = \frac{a_{02}}{1+b(\alpha)},$ $h_{20} = (1+b(\alpha))^2b_{20}+(a_{20}-b_{11})(1+b(\alpha))a(\alpha)+(b_{02}-a_{11})a^2(\alpha)+\frac{a^3(\alpha)a_{02}}{1+b(\alpha)},$ $h_{11} = a_{11}a(\alpha)-\frac{a^2(\alpha)a_{02}}{1+b(\alpha)}-2a(\alpha)b_{02}+b_{11}(1+b(\alpha)),$ $h_{02} = b_{02}+\frac{a(\alpha)a_{02}}{1+b(\alpha)}$ and $g_{jk} = h_{jk} = 0$ for all nonnegative integers $j, k$ and $j+k = 3.$

Given the transformation

with $\phi_{03} = \psi_{03},$ $\phi_{20} = \frac{1}{2}g_{20}+\frac{1}{4}h_{20},$ $\phi_{11} = \frac{1}{2}g_{20}+\frac{1}{2}+g_{11}+\frac{1}{2}h_{20}+\frac{1}{4}h_{11},$ $\phi_{02} = \frac{1}{4}g_{11}+\frac{1}{2}g_{02}+\frac{1}{8}h_{20}+\frac{1}{4}h_{11}+\frac{1}{4}h_{02},$ $\psi_{20} = \frac{1}{2}h_{20},$ $\psi_{11} = \frac{1}{2}h_{20}+\frac{1}{2}h_{11},$ $\psi_{02} = \frac{1}{4}h_{11}+\frac{1}{2}h_{02},$ $\phi_{30} = \phi_{21} = \phi_{12} = 0$ and $\psi_{30} = \psi_{21} = \psi_{12} = 0,$ we can obtain the following 1:2 resonance normal form:

where $r_6 = \sqrt{u_{n}^2+v_n^2},$

Thus $C(\beta_1(\alpha_0), \beta_2(\alpha_0)) = \frac{(19679\beta^2+192621\beta-13778)(-1911\beta^2+13050\beta-836)}{104\beta^2(1-\beta)^4},$ $D(\beta_1(\alpha_0), \beta_2(\alpha_0)) = \frac{630616082\beta^4+19939696470\beta^3+49520266820\beta^2-5731104734\beta+1730000030820}{(52\beta(1-\beta)^2)^2}.$ By Theorem 9.3 in ^[29], for all sufficiently small $|\beta|,$ the second iteration of (3.32) can be represented in the following form:

where $r_7 = \sqrt{u^2+v^2}$ and $\varphi_\beta^1(u, v)$ is the time-one flow of a planar system, which is smoothly equivalent to

where

and

Assume that $C_1(\beta(\alpha_0))\neq0$ and $D_1(\beta(\alpha_0))\neq0.$ These conditions can be expressed in terms of the normal form coefficients:

Notice that $C(\beta_1(\alpha_0), \beta_2(\alpha_0))\neq0$ is equivalent to

and

Under the condition that $0 < \beta < \frac{4}{9},$ $19679\beta^2+192621\beta-13778\neq0 \leftrightarrow \beta \ne \beta_1^* = :\frac{\sqrt{38187398689}}{39358}-\frac{17511}{3578}$ and $-1911\beta^2+13050\beta-836\neq0 \leftrightarrow \beta \ne \beta_2^* = :\frac{2175}{637}-\frac{\sqrt{40978029}}{1911})$.

Assume the following:

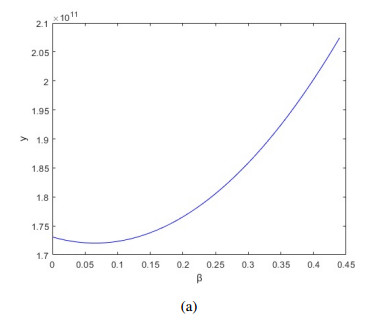
Then, (3.36) holding is equivalent to $y\neq0.$ It follows from the following Figure 1 that $y > 0$ for $0 < \beta < \frac{4}{9},$ which means that $D(\beta_1(\alpha_0), \beta_2(\alpha_0))+3C(\beta_1(\alpha_0), \beta_2(\alpha_0)) > 0.$ Therefore, when $\beta\neq\beta_1^*$ and $\beta\neq \beta_2^*,$ the non-degeneracy conditions $C_1(\beta_1(\alpha_0), \beta_2(\alpha_0))\neq0$ and $D_1(\beta_1(\alpha_0), \beta_2(\alpha_0))\neq0$ are satisfied; so, the fixed point $E_*$ of system (1.2) is a 1:2 resonance bifurcation point.

By scaling the variables, parameters and time in (3.34), we obtain the following system:

where $s = sign C(\beta_1(\alpha_0), \beta_2(\alpha_0)) = \pm1.$ If $s = 1,$ then, for the approximating system (3.37), the following holds:

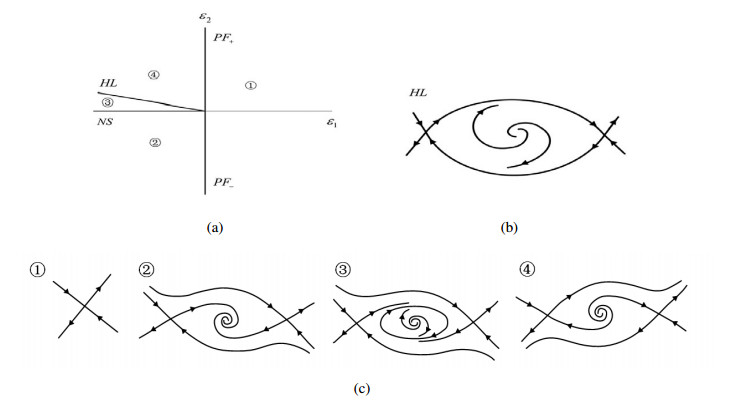
(ⅰ) there is a pitchfork bifurcation which occurs on the curve

$PF = \{(\varepsilon_1, \varepsilon_2):\varepsilon_1 = 0\};$

(ⅱ) there is a non-degenerate Neimark-Sacker bifurcation which occurs on the curve

$NS = \{(\varepsilon_1, \varepsilon_2):\varepsilon_2 = 0, \varepsilon_1 < 0\};$

(ⅲ) there is a heteroclinic bifurcation which occurs on

$HL = \{(\varepsilon_1, \varepsilon_2):\varepsilon_2 = -\frac{1}{5}\varepsilon_1+o(\varepsilon_1), \varepsilon_1 < 0\}.$

The bifurcation diagram for (3.37) for $s = 1$ is presented in Figure 2(a). Because of the $Z_2$-symmetry, the heteroclinic orbits connecting the two saddles appear simultaneously, forming a heteroclinic cycle upon crossing the curve HL (see Figure 2(b)). Moreover, the corresponding phase portraits of the contents of Figure 2(a) are showed in Figure 2(c). For the details, refer to ^[30].

Remark. If system (1.2) undergoes a 1:2 resonance bifurcation near the positive fixed point $E_*$, system (1.2) is strongly unstable. The predator and the prey will increase rapidly and breakout suddenly. For this, refer also to ^[26]}.

4.   Numerical simulation

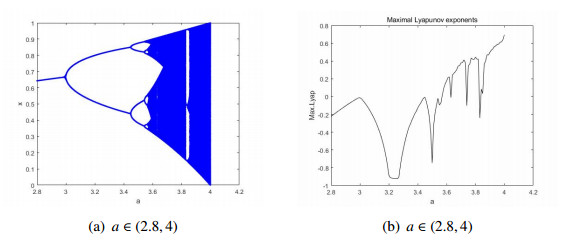
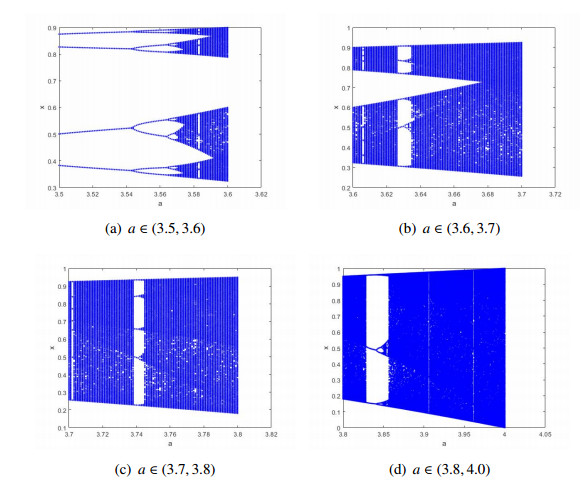
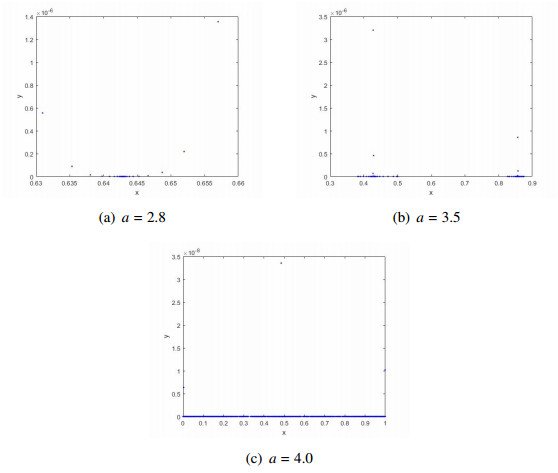
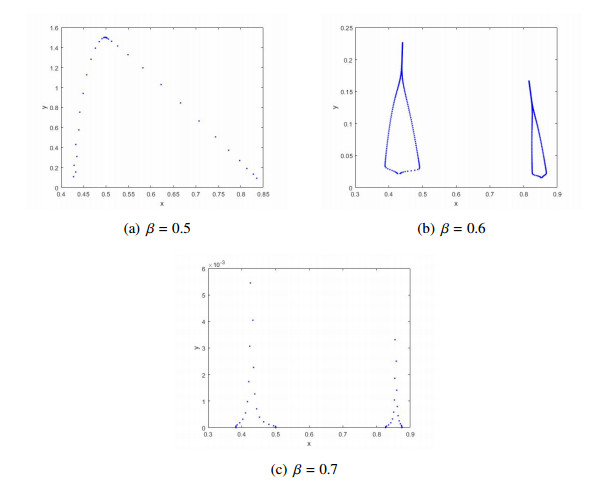
In this section, to illustrate our theoretical results and reveal some new dynamical behaviors in system (1.2), we present the bifurcation diagrams, phase portraits and maximal Lyapunov exponents that were derived for specific parameter values by using Matlab software. First, for the fixed point $E_2(\frac{a-1}{a}, 0)$, we chose the parameters to be $a\in(2.8, 4)$, $m = 0.5$ and $\beta = 1.6,$ and the initial values as $(x_0, y_0) = (0.1, 0.1)$. Because the bifurcation diagram for the $(a, x)$-plane is similar to that for the $(a, y)$-plane, we will only show the former. Figure 3(a) shows that the fixed point $E_2$ is stable for $a < 3,$ and that the period-doubling bifurcation occurs at $a = 3$ (the multipliers are $\lambda_1 = -1$ and $|\lambda_2|\neq1).$ Meanwhile $E_2$ becomes unstable when $a > 3$, which is in accordance with the result in Theorem 3.2. Moreover, a chaotic set emerges with the increase of the value of the parameter $a.$ Figure 3(b) shows the spectrum of the maximum Lyapunov exponents with respect to the parameter $a\in(2.8, 4)$ when $m = 0.5$ and $\beta = 1.6;$ it also shows that the maximal Lyapunov exponents are positive, that is to say, chaos may occur. Figure 4(a) shows that periods of 2, 4, 8, etc., will occur, which means that chaos will occur (period-doubling bifurcation to chaos). However, chaos is a ubiquitous nonlinear phenomenon that leads to undesirablity. So controlling chaos to make dynamic behavior predictable is essential. How can one control chaos? The main methods of chaos control are state feedback, pole-placement and hybrid control. For this, refer to ^[34,35]. From Figure 4(b), (c), we observe that, when the value of the growth rate $a$ of prey is close to 3.63 or 3.74, the population density distribution is sparse. Meanwhile, the prey population may become extinct when the parameter $a$ increases to 4 according to Figure 4(d). The phase portrait is given in Figure $5.$ From Figure 5(a), (b), the predator population is gradually decreasing with a small number representing two species coexistence. And, when the parameter $a = 2.8$ or $a = 3.5$, the prey population is near 0.643 or 0.42 (0.85) respectively. In Figure 5(c), the predator population is decreasing to extinction and there is only intraspecific competition for prey. The density of the prey population ranges from $0$ to $1$. The excessive growth rate of the prey first reduces the density of the predator population until extinction; it then causes them to compete among themselves until they reach balance. We set the parameters as $m = 0.5$ and $a = 3.5$ and varied the value of the parameter $b$ to get Figures 6. One finds that the prey population and predator population coexist at $\beta = 0.5,$ and that the two species are periodic and coexist on two closed orbits at $\beta = 0.6$. Then the predator population decreases and the prey population gradually becomes stable.

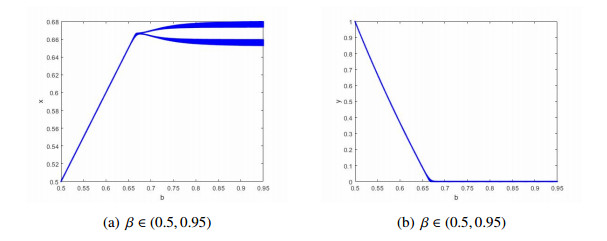
Next, one can see that two eigenvalues of system (1.2) are $\lambda_1 = 1$ and $\lambda_2 = -1$ when $a = 3$ and $\beta = \frac{2}{3}.$ We call the fixed point $E_2(\frac{2}{3}, 0)$ a fold-flip bifurcation point. The bifurcation diagram for system (1.2) is plotted in Figure 7. As we can see, when the parameter $a = 3$ and the parameter $\beta$ varies from 0.5 to 0.95, the prey population keeps increasing until it is split into two parts at the parameter $\beta = \frac{2}{3}$; meanwhile, the predator population gradually decreases to extinction. For the codim 2 bifurcation diagram and corresponding details, refer also to ^[29].

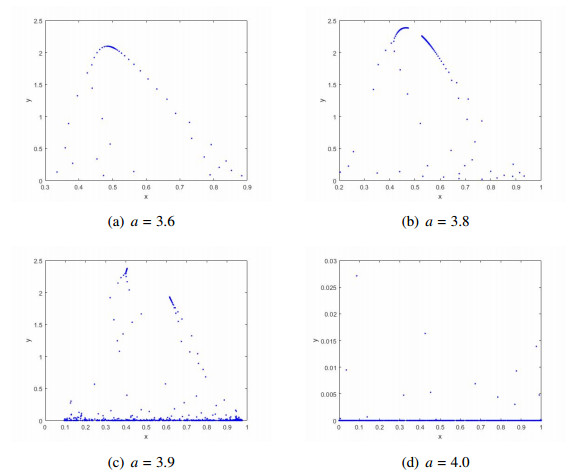
Finally, we applied the parameters $a\in(3.6, 4.15), m = 0.8$ and $\beta = 0.5$ and the initial values $(x_0, y_0) = (0.2, 3.1)$ for the fixed point $E_*$. We constructed Figure 8(a) and determined the existence of period-doubling bifurcation when $a = a_0 = 3.79.$ Figure 8(b) represents the maximum Lyapunov exponents under the conditions of $a\in(3.6, 4.15),$ $m = 0.8$ and $\beta = 0.5;$ it indicates that chaos probably occurs in the system when the parameter $a$ is beyond 4.15. According to the result in Theorem 3.4, one has $K = -2.6398 < 0;$ thus, the period-doubling bifurcation is subcritical and the system is unstable. From Figure 9, the phase portraits demonstrate that the prey population and predator population can coexist at $a = 3.6, \ 3.8.$ When the parameter $a$ increases continuously, the predator population will decrease to extinction. Thus, the period-doubling orbit that is bifurcated from $E_*$ is unstable, which will break the population balance.

5.   Discussion and conclusions

In this paper, we revisit a discrete-time prey-predator model with the Allee effect in prey. Besides correcting the errors for the local stability at the fixed point $E_*,$ we have mainly studied the bifurcation problems of the fixed points $E_1(0, 0),$ $E_2(\frac{a-1}{a}, 0)$ and $E_*(\beta, \frac{(\beta+m)(a(1-\beta)-1)}{\beta})$ of system (1.2) to find some complex bifurcation results, which are ecologically important. Especially, for the fixed point $E_*,$ we observed the existence of the codimension one period-doubling bifurcation and Neimark-Sacker bifurcation and codimension two 1:2 strong resonance bifurcation. The 1:2 strong resonance bifurcation observed to occur at the fixed point $E_*$ implies that the discrete system may undergo pitchfork bifurcation, Neimark-Sacker bifurcation and heteroclinic bifurcation near $E_*,$ and this will lead to a potential dramatic variation of the system dynamics. The conditions for these bifurcations to occur have been given, and interesting dynamical properties have also been obtained through numerical simulations. Our results clearly demonstrate the dynamics of the system: as the parameter $\beta$ increases, the two species change from the coexistence on two closed orbits to gradual extinction of the predator population; at the same time, the value of the parameter $a$ controls the prey and predator population. Moreover, by taking appropriate value of Allee effect, 1:2 strong resonance bifurcation at fixed point $E_*$ will occur, which indicates that the two species will increase rapidly and breakout suddenly ^[29]. However, the fold-flip bifurcation at $E_2$ and chaos control that have not been solved in this paper are still interesting problems, and they will be considered in our future work.

Use of AI tools declaration

The authors declare that they have not used artificial intelligence tools in the creation of this article.

Acknowledgments

This work was partly supported by the National Natural Science Foundation of China (61473340), Distinguished Professor Foundation of Qianjiang Scholars in Zhejiang Province (F703108L02) and Natural Science Foundation of Zhejiang University of Science and Technology (F701108G14).

Conflict of interest

The authors declare that they have no known competing financial interests or personal relationships that could have appeared to influence the work reported in this paper.

Appendix A.   Known results for the stability of fixed points

For the fixed point $E_1(0, 0)$, the following statements are true.

$(i.1)$ $E_1(0, 0)$ is a sink if $a < 1$;

$(i.2)$ $E_1(0, 0)$ is a saddle if $a > 1$;

$(i.3)$ $E_1(0, 0)$ is non-hyperbolic if $a = 1$.

Assume that $a > 1.$ For the fixed point $E_2(\frac{a-1}{a}, 0)$, the following statements are true.

$(ii.1)$ $E_2(\frac{a-1}{a}, 0)$ is a sink if $1 < a < min \{\frac{1}{1-\beta}, 3\}$ and $0 < \beta < 1$;

$(ii.2)$ $E_2(\frac{a-1}{a}, 0)$ is a saddle if $(\frac{1}{1-\beta} < a < 3$ and $0 < \beta < \frac{2}{3})$ or $(3 < a < \frac{1}{1-\beta}$ and $\frac{2}{3} < \beta < 1)$;

$(ii.3)$ $E_2(\frac{a-1}{a}, 0)$ is a source if $a > max\{3, \frac{1}{1-\beta}\}$ and $0 < \beta < 1$;

$(ii.4)$ $E_2(\frac{a-1}{a}, 0)$ is non-hyperbolic if $a = 3$ or $(a = \frac{1}{1-\beta}, 0 < \beta < 1$ and $\beta\neq \frac{2}{3})$.

Assume that $a > \frac{1}{1-\beta}$ and $\beta < 1;$ then, for the unique positive fixed point $E_*$, the following statements are true.

$(iii.1)$ $E_*$ is a sink if the following condition holds:

$(iii.2)$ $E_*$ is a source if the following condition holds:

$(iii.3)$ $E_*$ is a saddle if the following condition holds:

$(iii.4)$ $E_*$ is a non-hyperbolic if the following condition holds:

$(iii.5)$ $E_*$ is a non-hyperbolic with the conjugate complex roots if the following condition holds:

Appendix B.   Key definition and lemma

Definition 5.1. Let $E(x, y)$ be a fixed piont of the system (1.2) with multipliers $\lambda_1$ and $\lambda_2$.

$1)$ If $|\lambda_1| < 1$ and $|\lambda_2| < 1$, a fixed point $E(x, y)$ is called a sink; thus, a sink is locally asymptotically stable.

$2)$ If $|\lambda_1| > 1$ and $|\lambda_2| > 1$, a fixed point $E(x, y)$ is called a source; thus, a source is locally asymptotically unstable.

$3)$ If $|\lambda_1| < 1$ and $|\lambda_2| > 1$ $($or $|\lambda_1| > 1$ and $|\lambda_2| < 1$$)$, a fixed point $E(x, y)$ is called a saddle.

$4)$ If either $|\lambda_1| = 1$ or $|\lambda_2| = 1$, a fixed point $E(x, y)$ is called non-hyperbolic.

Lemma 5.2. Let $F(\lambda) = \lambda^2+B\lambda+C$, where $B$ and $C$ are two real constants. Suppose that $\lambda_1$ and $\lambda_2$ are two roots of $F(\lambda) = 0$. Then the following statements hold.

$(i)$ If $F(1) > 0,$ then

$(i.1)$ $|\lambda_1| < 1$ and $|\lambda_2| < 1$ if and only if $F(-1) > 0$ and $C < 1$;

$(i.2)$ $\lambda_1 = -1$ and $\lambda_2\ne-1$ if and only if $F(-1) = 0$ and $B\ne2$;

$(i.3)$ $|\lambda_1| < 1$ and $|\lambda_2| > 1$ if and only if $F(-1) < 0$;

$(i.4)$ $|\lambda_1| > 1$ and $|\lambda_2| > 1$ if and only if $F(-1) > 0$ and $C > 1$;

$(i.5)$ $\lambda_1$ and $\lambda_2$ are a pair of conjugate complex roots and $|\lambda_1| = |\lambda_2| = 1$ if and only if $-2 < B < 2$ and $C = 1;$

$(i.6)$ $\lambda_1 = \lambda_2 = -1$ if and only if $F(-1) = 0$ and $B = 2$.

$(ii)$ If $F(1) = 0,$ namely, $1$ is one root of $F(\lambda) = 0$, then the other root $\lambda$ satisfies that $|\lambda| = (<, > )1$ if and only if $|C| = (<, > )1.$

$(iii)$ If $F(1) < 0,$ then $F(\lambda) = 0$ has one root lying in $(1, \infty)$. Moreover,

$(iii.1)$ the other root $\lambda$ satisfies that $\lambda < ( = )-1$ if and only if $F(-1) < ( = )0$;

$(iii.2)$ the other root $-1 < \lambda < 1$ if and only if $F(-1) > 0$.