1.
Introduction
Seasonal influenza is an acute respiratory infection caused by influenza viruses. Worldwide, these annual epidemics are estimated to result in about 3 to 5 million cases of severe illness, and about 290,000 to 650,000 respiratory deaths [1]. This infection can have an endemic, epidemic or pandemic behavior.
There were, three major flu pandemics during the 20th century, the so-called Spanish flu (H1N1) in 1918 was the most devastating pandemic. It has been estimated that the Spanish flu claimed around 40–50 million deaths (as much as 3% of the total population), and it also infected 20–40% of the whole population. In 1957–1958, the Asian flu or bird flu pandemic (H2N2) caused more than two million deaths [2]. Unlike the Spanish flu, this time the infection-causing virus was detected earlier due to the advancement of science and technology. A vaccine was made available but with limited supply. After a decade (in 1968), a flu pandemic (H3N2) that originated again in Hong Kong hit mankind. That flu pandemic also claimed one million lives. In 2009, the H1N1 swine flu is one of the more publicized pandemics that attracted the attention of all scientists and health professionals in the world and made them very much concerned. However, the pandemic did not result in great casualties like before. As of July 2010, only about 18,000 related deaths had been reported [2]. Besides the 4 influenza pandemics since 1918, annual seasonal influenza epidemics have spread among nations on smaller scales. There are many methods of preventing the spread of infectious disease, one of them is vaccination. Vaccination is the administration of agent-specific, but relatively harmless, antigenic components that in vaccinated individuals can induce protective immunity against the corresponding infectious agent [3].
Influenza causes serious public-health problems around the world, therefore, we need to understand transmission mechanisms and control strategies. Mathematical models also provided insight into the severity of past influenza epidemics. Some models were used to investigate the three most devastating historical pandemics of influenza in the 20th century [4,5,6]. There are a lot of pathogens with several circulating strains.
An important factor when analyzing the dynamics of a disease is the way in which it is transmitted from an infected individual to a healthy one. The incidence rate of a disease is defined as the number of susceptible individuals that become infected per unit of time. It measures the number of new cases of a disease in a period of time. There are different types of incidence functions that have been used in literature in order to model the force of infection of a disease. For example, Rahman and Zou [2] used the bilinear incidence rate βSI. However, there are more realistic incidence rates than the bilinear incidence rate, For instance, Capasso and his co-workers observed in the seventies [7] that the incidence rate may increase more slowly as I increases, so they proposed a saturated incidence rate βIS1+ζI.
Baba and Hincal [8] studied an epidemic model consisting of three strains of influenza (I1, I2, and I3) where we have vaccine for strain 1 (V1) only, and force of infection βSI1+ζS for strain 2. Baba et al. [9] studied an epidemic model consisting of two strains of influenza (I1 and I2) where force of infection βSI21+ζI22 for strain 2. As models with more general incidence functions are considered, the dynamics of the system become more complicated. Models with incidence functions of the form g(I)h(S) have been studied, such as [10]. In the most general case, the transmission of the disease may be given by a non-factorable function of S and I.
In this paper, our purpose is to study model considered in [2] modifying the force of infection in the compartments I1 and I2, by extending the incidence function to a more general form F(S,I), which is based on the incidence rate studied in [11]. Our main aim is to mathematically analyze the effect of the vaccine for strain 1, the general incidence rate of strain 1 (F1(S,I1)), and the general incidence rate of strain 2 (F2(S,I2)) on the dynamics of the model (2.2).
This paper is organized as follows. In section 2.1, we formulate the model. In section 3.1, we investigate the disease dynamics described by the model. In section 3.2, we calculate the basic reproduction number. In section 3.3, we establish the existence of equilibrium points. In section 3.4, we study the stability of the model. In section 3.5, provides some numeric simulations to illustrate our main theoretical results. The paper ends with some remarks.
2.
Materials and method
2.1. The model
Rahman and Zou [2] proposed a two-strain model with a single vaccination, namely.
where λ=r+μ, α1=γ1+v1+μ, α2=γ2+v2+μ. The compartments are S(t), V1(t), I1(t), I2(t) and R(t) which denote the population of susceptible, vaccine of strain 1, infective with respect to strain 1, infective with respect to strain 2 and removed individuals at time t, respectively. We assume that all the parameters are positive constants that can be interpreted as follows:
● Λ is the birth rate.
● μ is the death rate.
● r is the rate of vaccination with strain 1.
● k is the transmission coefficient of vaccinated individuals to strain 2.
● β1 is the transmission coefficient of susceptible individuals to strain 1.
● β2 is the transmission coefficient of susceptible individuals to strain 2.
● 1γ1 is the average infection period of strain 1.
● 1γ2 is the average infection period of strain 2.
● v1 is the infection-induced death rate of strain 1.
● v2 is the infection-induced death rate of strain 2.
The modification of the model (2.1) is given by the following system:
Whose state space is R5+={(S,V1,I1,I2,R):S≥0,V1≥0,I1≥0,I2≥0,R≥0} and subject to the initial conditions S(0)=S0≥0, V1(0)=V10≥0, I1(0)=I10≥0, I2(0)=I20≥0 and R(0)=R0≥0.
We make the following hypotheses on Fi, i=1,2.:
H1) Fi(S,Ii)=Iifi(S,Ii) with Fi, fi∈C2(R2+→R+) and F(0,Ii)=F(S,0)=0 for all S,Ii≥0.
H2) ∂fi∂S(S,Ii)>0 and ∂fi∂Ii(S,Ii)≤0 for all S,Ii≥0.
H3) limIi→0+Fi(S,Ii)Ii exists and is positive for all S>0.
The first of this hypotheses is a basic requirement for any biologically feasible incidence rate, since the disease cannot spread when the number of susceptible or infected individuals is zero.
As for (H2), the condition ∂fi∂S(S,Ii)>0 ensures the monotonicity of fi(S,Ii) on S, while ∂fi∂Ii(S,I)≤0 suggests that Fi(S,Ii)Ii is non-increasing with respect to Ii. In the case when fi monotonically increases with respect to both variables and is concave with respect to Ii, the hypothesis (H2) naturally holds. Concave incidence functions have been used to represent the saturation effect in the transmission rate when the number of infected is very high and exposure to the disease is virtually certain.
(H3) is needed only to ensure that the basic reproduction number is well defined. Some examples of incidence functions studied in the literature that satisfy (H1)–(H3) are as follows:
(C1) F(S, I) = βSI [2].
(C2) F(S, I) = βSI1+ζS, where ζ≥0 describes the psychological effect of general public towards the infective [8].
(C3) F(S, I) = βSI1+ζI2, where ζ≥0 measures the psychological or inhibitory effect of the population [9].
A more thorough list can be found in [11]. It should be noted that model (2.2) extends as well as generalizes many special cases.
3.
Results
3.1. Disease dynamics described by the model
Lemma 1. Under the initial value (S0,V10,I10,I20,R0)∈R5+ the system (2.2) has a unique positive and bounded solution in R5+ for t>0. All solutions ultimately enter and remain in the following bounded and positively invariant region
Proof. The right hand side of system (2.2) is continuous and satisfies the Lipschitz condition in R5+. Then the system (2.2) has a unique solution (S(t,V1(t),I1(t),I2(t),R(t)) in [0,tm) for some tm>0. Adding all equations in (2.2), the total population N=S+V1+I1+I2+R satisfies:
The comparison theorem implies that limt→∞supN(t)≤Λμ. Hence N(t) is bounded and so are all components S(t), V1(t), I1(t), I2(t) and R(t). This in turn shows that the solution exists globally, i.e. for all t≥0. Consequently, the solutions S(t), V1(t), I1(t), I2(t), R(t) of (2.2) are ultimately bounded in the positively invariant region Ω.
Let (S(t), V1(t), I1(t), I2(t), R(t)) be a solution of system (2.2) with positive initial conditions. Assume by contradiction that there exists t>0 such that S(t)≤0, V1(t)≤0, I1(t)≤0, I2(t)≤0 or R(t)≤0. By continuity of solutions, this implies that there is a minimal t0>0 such that S(t0), V1(t0), I1(t0), I2(t0) or R(t0) is zero.
If S(t0)=0, then ˙S=Λ>0 at t0, so S is increasing in a neighbourhood (t0−ϵ, t0+ϵ) of t0. Thus S(t0−ϵ2)<S(t0)=0, and since S(0)>0 and S(t0−ϵ2)<0, there exists a t1∈(0, t0−ϵ2) with S(t1)=0. But t1<t0, which contradicts the minimality of t0, then S(t0)>0.
If V1(t0)=0, then ˙V1=rS(t0)>0 at t0. So V1 is increasing in a neighbourhood (t0−ϵ, t0+ϵ) of t0. Thus V1(t0−ϵ2)<V1(t0)=0, and since V1(0)>0 and V1(t0−ϵ2)<0, there exists a t1∈(0, t0−ϵ/2) with V1(t1)=0. But t1<t0, which contradicts the minimality of t0, then V1(t0)>0.
If I1(t0)=0, then ˙I1=0 at t0. On the other hand, any solution with I1(0)=0 satisfies I(t)=0 for all t>0. Since I1(0)>0 and I1(t0)=0, this contradicts the uniqueness of solutions. Similar contradictions are obtained if we assume that I2(t0)=0 or R(t0)=0. Thus we conclude that the solutions of (2.2) are positive for all t>0.
Since the equation for ˙R is actually decoupled from the rest in Eq (2.2), we only need to consider dynamics of the following four-dimensional sub-system:
3.2. Basic reproduction number
The basic reproduction number is a dimensionless quantity denoted by R0. It is defined as the expected number of secondary infection cases caused by a single typical infective case during its entire period of infectivity in a wholly susceptible population. Then, referring to the method of [12].
Then
where E0=(S0,V01,0,0)=(Λλ,rΛμλ,0,0). The matrix F is non-negative and is responsible for new infections (transmission matrix), while the V is invertible and is referred to as the transition matrix for the model (3.1). It follows that,
where σi=∂Fi(S0,0)∂Ii, for i=1,2. Thus, the basic reproduction number can be calculated as
where ρ(A) denotes the spectral radius of a matrix A. Let
Then
Therefore R1,R2≤R0.
3.3. Existence of equilibrium solutions
The four possible equilibrium points for the system (3.1) are: Disease-free equilibrium, single-strain (I1)-infection, single-strain (I2)-infection and endemic equilibrium. The system (3.1) has disease-free equilibrium E0=(Λλ,rΛμλ,0,0) for all parameter values. We will now prove the existence of the other equilibrium points. First we will show some lemmas.
Lemma 2. For i = 1, 2.
Also:
Proof. By H1)
Then
By H2) ∂fi(S,Ii)∂Ii≤0, then:
Lemma 3. For model (3.1), the closed set Ω1={(S,V1,I1,I2)∈Ω|S≤S0 and V1≤V01} is a positively invariant set.
Proof. As Ω is a positively invariant set for model (3.1), it will be enough to show that if S=S0, then ˙S≤0 and if S≤S0 and V1=V01, then ˙V1≤0.
If S=S0, then
If S≤S0 and V1=V01, Then
Lemma 4. For i = 1, 2.
Proof. By (H1)
Then
Remark 1. By (H2) given a and b, if S≤a and Ii≥b, then fi(S,Ii)≤fi(a,b), i=1,2.
Theorem 1. (1) The model (3.1) admits a unique single-strain (I1)-infection equilibrium E1=(ˉS,¯V1,¯I1,0) if and only if R1>1.
(2) The model (3.1) admits a unique single-strain (I2)-infection equilibrium E2=(˜S,~V1,0,~I2) if and only if R2>1.
Proof. (1) If I2=0 and R1>1, we consider the system
By (3.3) and (3.4)
Substituting in (3.2).
Note that ˉS≥0 if and only if ¯I1≤Λα1. ¯I1 being determined by the positive roots of the equation.
Then
And
Therefore G(¯I1)>0 by I1 sufficiently small. Also
Then Eq (3.5) has a positive root. Also if E1 exists then
Note that ˉS<S0. Then by Lemma 2 and remark 1
Then R1>1.
Next, we shall show that ¯I1 is unique. From (3.4), it follows that
Using (H2) and Lemma 2, we have that −α1λ∂F1(ˉS,¯I1)∂S<0 and ¯I1∂f1(ˉS,¯I1)∂I1≤0. Furthermore, it can be found that
Which implies that G(¯I1) strictly decreases at any of the zero points of (3.5). Let us suppose that (3.5) has more than one positive root. Without loss of generality, we choose the one, denoted by ¯I1∗, that is the nearest to ¯I1. Because of the continuity of G(¯I1), we must have G′(¯I1∗)≥0, which results in a contradiction with the strictly decreasing property of G(¯I1) at all the zero points.
(2) If I1=0 and R2>1, we consider the system
By (3.7) and (3.8)
Substituting in (3.6).
Note that ˜S≥0 if and only if ~I2≤Λα2. ~I2 being determined by the positive roots of the equation.
Then
And
Therefore H(~I2)>0 by ~I2 sufficiently small. Also
Then Eq (3.9) has a positive root. Also if E2 exists then
Note that by H1 we have −F2(˜S,~I2)<0, then Λ−λ˜S>0, therefore ˜S<S0 and ~V1<V01. Then by Lemma 2 and remark 1
Then R2>1.
Next, we shall show that ~I2 is unique. From (3.8), it follows that
Furthermore, it can be found that
If −α2rμ−α2μ2+kΛr≤0, then H′(~I2)<0 which implies that H(~I2) strictly decreases at any of the zero points of (3.9). Let us suppose that (3.9) has more than one positive root. Without loss of generality, we choose the one, denoted by ~I2∗, that is the nearest to ~I2. Because of the continuity of H(~I2), we must have H′(~I2∗)≥0, which results in a contradiction with the strictly decreasing property of H(~I2) at all the zero points.
If −α2rμ−α2μ2+kΛr>0. Next, we show that ~I2∉[0,−rα2−α2μ+√rα2(rα2+α2μ+kΛ)α2k). Note that
Then
If ~I2∈[0,−rα2−α2μ+√rα2(rα2+α2μ+kΛ)α2k), then ˜S′(~I2)>0, therefore ˜S≥S0, which results in a contradiction, since ˜S<S0.
Thus ~I2∈[−rα2−α2μ+√rα2(rα2+α2μ+kΛ)α2k,Λα2], which implies that H(~I2) strictly decreases at any of the zero points of (3.9). Let us suppose that (3.9) has more than one positive root in [−rα2−α2μ+√rα2(rα2+α2μ+kΛ)α2k,Λα2]. Without loss of generality, we choose the one, denoted by ~I2∗, that is the nearest to ~I2. Note that H′(~I2∗)<0 and H′(~I2)<0. Because of the continuity of H(~I2), we must have H′(~I2∗)≥0, which results in a contradiction.
The model (3.1) can have endemic infection equilibrium E3=(S∗,V∗1,I∗1,I∗2). To find E3, we consider the system
By (3.11), (3.12) and (3.13)
Substituting in (3.10).
Note that S∗≥0 if and only if I∗1≤Λ−α2I∗2α1 and I∗2≤Λ−α1I∗1α2. ¯I2 being determined by the positive roots of the equation.
I∗1 being determined by the positive roots of the equation.
3.4. Stability of equilibrium
In this section we will study the local and global stability of the equilibrium points.
Theorem 2. The disease-free equilibrium E0=(Λλ,rΛμλ,0,0) is unstable if R0>1 while it is locally asymptotically stable if R0<1.
Proof. The Jacobian matrix of the model we get is the following one
Then Eq (3.14) at the disease-free equilibrium E0 is
Thus the eigenvalues of the above Eq (3.15) are
From (3.16), if R0<1, then λ3,λ4<0 and we obtain that the disease-free equilibrium E0 of Model (3.1) is locally asymptotically stable. If R0>1, then the disease-free equilibrium loses its stability.
Theorem 3. Let ¯R2=1α2∂F2(ˉS,0)∂I2+k¯V1α2. The equilibrium E1 is unstable if ¯R2>1 while it is locally asymptotically stable if ¯R2<1.
Proof. Then Eq (3.14) at the equilibrium E1 is
where
The last equality regarding A33, is because Eq (3.4) implies that f1(ˉS,¯I1)−α1=0. The corresponding characteristic polynomial is
Then an eigenvalue is A44 and the remaining ones satisfy
where
Note that
Then a1, a0>0 and
Applying the Routh–Hurwitz criterion, we see that all roots of x3+a2x2+a1x+a0 have negative real parts. If ¯R2>1, then A44>0 therefore E1 is unstable and if ¯R2<1, then A44<0 therefore E1 is stable.
Remark 2. ˉS≤S0 and ¯V1≤V01, then ¯R2≤R2, therefore if R2<1 then ¯R2<1.
Theorem 4. Let ~R1=1α1∂F1(˜S,0)∂I1. If ∂F2(˜S,~I2)∂I2≤0 the equilibrium E2 is unstable if ~R1>1 while it is locally asymptotically stable if ~R1<1.
Proof. Then Eq (3.14) at the equilibrium E1 is
Where
The last equality regarding B44, is because Eq (3.8) implies that k~V1−α2=−f2(˜S,~I2). The corresponding characteristic polynomial is
Then (3.18) has an eigenvalue equal to B33 and the remaining ones satisfy
where
Note that
And
Then b1, b0>0. Also
Applying the Routh–Hurwitz criterion, we see that all roots of x3+b2x2+b1x+b0 have negative real parts. If ~R1>1, then B33>0 therefore E2 is unstable and if ~R1<1, then B33<0 therefore E2 is stable.
Remark 3. ˜S≤S0, then ~R1≤R1, therefore if R1<1 then ~R1<1.
Remark 4. If ∂F2(˜S,~I2)∂I2>0, then bi>0 i=0,1,2.
Remark 5. The Theorem 4 is valid for ∂F2(˜S,~I2)∂I2>0 if b2b1−b0>0.
Theorem 5. If ¯R2>1 and ~R1>1 then system (3.1) is uniformly persistent.
Proof. The result follows from an application of Theorem 4.6 in [13], with X1=int(R4+) and X2=bd(R4+) this choice is in accordance with the conditions stated in the theorem. Now, note that by of Lemma 1 there exists a compact set Ω in which all solution of system (3.1) initiated in R4+ ultimately enter and remain forever after. The condition (C4.2) is easily verified for this set Ω1. On other hand, we denote the omega limit set of the solution x(t,x0) of system (3.1) starting in x0∈R4+ by w(x0). Note that w(x0) is bounded (Lemma 1), we need to determine the following set:
From the system equations (3.1) it follows that all solutions starting in bd(R4+) but not on the I1 axis or on the I2 axis leave bd(R4+). This implies that
Furthermore, we see that Ω2={E0,E1,E2}, then ⋃3i=1{Ei} is a covering of Ω2, which is isolated (since Ei (i=1,2,3) is a saddle point) and acyclic. Finally we need to prove that Ei (i = 1, 2, 3) is a weak repeller for X1 to end the prove.
By definition Ei is a weak repeller for X1 if for every solution (S(t),V1(t),I1(t),I2(t)) starting in (S0,V10,I10,I20)∈X1
We will first show that E0 is a weak repeller for X1. Since ¯R2>1 and ~R1>1, then R2=1α2(f2(S0,0)+kV0)>1 and R1=1α1(f1(S0,0))>1, therefore f2(S0,0)+kV0−α2>0 and f1(S0,0)−α1>0. Because of the continuity of f2(S,I2)+kV1−α2 and f1(S,I1)−α1, there exists a sufficiently small constant η2>0, such that f1(S0−η2,η2)−α1>0 and f2(S0−η2,η2)+k(V01−η2)−α1>0.
Now, we suppose that E0 is not a weak repeller for X1, i.e., there exists a solution (S(t),V1(t),I1(t),I2(t)) starting in (S0,V10,I10,I20)∈X1 such that
Then exists T1>0 such that for every η1>0
Let η1=η2, then for t≥T1.
and
By comparison principle, we have
Note that f1(S0−η2,η2)−α1>0, f2(S0−η2,η2)+k(V01−η2)−α1>0, I1(T1)>0 and I2(T1)>0, which implies that limt→∞I1=limt→∞I2=∞, this gives a contradiction. Then E0 is a weak repeller for X1.
Similarly it is shown that E1 and E2 are weak repeller for X1. Then we conclude that system (3.1) is uniformly persistent.
Further, it is proved in [14] uniform persistence implies the existence of an interior equilibrium point. Therefore, we have established the following.
Theorem 6. The model (3.1) admits a endemic equilibrium E3=(S∗,V∗1,I∗1,I∗2) if ¯R2>1 and ~R1>1.
Theorem 7. If c1c2−c3>0 and c1c2c3−c23−c21c4>0, where
Then E3 is locally asymptotically stable.
Proof. Then Eq (3.14) at the equilibrium E3 is
Where
The corresponding characteristic polynomial is
Note that c1>0,
then c2>0, If C14≥0 then c3>0 and c4>0, while if C14<0 we have that
and let
then
Then c3>0 and c4>0. If c1c2−c3>0 and c1c2c3−c23−c21c4>0 by Routh–Hurwitz criterion, we see that all roots of x4+c1x3+c2x2+c3x+c4 have negative real parts, then E3 is locally asymptotically stable.
3.4.1. Global stability of equilibria
In this section, we study the global properties of the equilibria. We use Lyapunov function to show the global stabilities. Such Lyapunov functions all take advantage of the properties of the function.
which is positive in R+ except at x=1, where it vanishes.
Theorem 8. The DFE E0 is globally asymptotically stable if,
Proof. Consider the Lyapunov function
Since I1,I2>0, then V(S,V1,I1,I2)≥0 and V(S,V1,I1,I2) attains zero at I1=I2=0.
Now, we need to show ˙V≤0.
For S≤S0 and V1≤V01
Furthermore, dVdt=0 if and only if I1=I2=0, so the largest invariant set contained in {(S,V1,I1,I2)∈Ω1|dVdt=0} is the hyperplane I1=I2=0, By LaSalle's invariant principle, this implies that all solution in Ω1 approach the hyperplane I1=I2=0 as t→∞. Also, All solution of (3.1) contained in such plane satisfy ˙S=Λ−λS, ˙V1=rS−μV1, which implies that S→Λλ and V1→rΛμλ as t→∞, that is, all of these solution approach E0. Therefore we conclude that E0 is globally asymptotically stable in Ω1.
Now we will show that every solution (S(t),V1(t),I1(t),I2(t))∈R4+, where t→∞ (S(t),V1(t),I1(t),I2(t))∈Ω1, let (S(t),V1(t),I1(t),I2(t))∈R4+. Then
By the comparison principle limt→∞supS(t)≤Λλ=S0. Then S(t)≤S0 for t sufficiently large.
Also if S(t)≤S0.
By the comparison principle limt→∞supV(t)≤rS0μ=V01. Therefore E0 is globally asymptotically stable.
From now on, we assume that
H4) For i=1,2. fi(S,Ii)=Sgi(S,Ii).
Lemma 5. Let a>0 be a constant, for i=1,2 if ∂Fi(S,Ii)∂Ii≥0 for all Ii, then
Proof. Note that
If a≥Ii, then
If a≤Ii, then
Therefore
Theorem 9. Suppose that ∂F1(S,I1)∂I1≥0 for all I1, then E1 is globally asymptotically stable if,
Proof. Consider the Lyapunov function
Since I2>0, then V(S,V1,I1,I2)≥0 and V(S,V1,I1,I2) attains zero at I2=0. Now, we need to show ˙V≤0.
For S≤S0 and V1≤V01
Furthermore, dVdt=0 if and only if I2=0. Suppose that (S(t),V1(t),I1(t),I2(t)) is a solution of (3.1) contained entirely in the set M={(S(t),V1(t),I1(t),I2(t))∈Ω1|˙V=0}. Then, ˙I2=0 and, from the above inequalities, we have I2=0. Thus, the largest positively invariant set contained in M is the plane I2=0. By LaSalle’s invariance principle, this implies that all solutions in approach the plane I2=0 as t→∞. On the other hand, solutions of (3.1) contained in such plane satisfy
Now we will show that S(t)→ˉS, V1(t)→¯V1 and I1(t)→¯I1 Consider the Lyapunov function
Note that 1−F1(ˉS,¯I1)F1(χ,¯I1)=¯I1(f1(χ,¯I1)−f1(ˉS,¯I1))F1(χ,¯I1), by H2) f1(S,¯I1)−f1(ˉS,¯I1)≥0 if S≥ˉS and f1(S,¯I1)−f1(ˉS,¯I1)≤0 if S≤ˉS, then ∫SˉS(1−F1(ˉS,¯I1)F1(χ,¯I1))dχ≥0 for all S. Therefore, V(S,V1,I1)≥0 and V(S,V1,I1) attains zero at S(t)=ˉS, and I1(t)=¯I1.
Now, we need to show ˙V≤0.
Note that
and
The last inequality is due to the Lemma 5 and the relation of the geometric and arithmetic means, then ˙V≤0. Furthermore, dVdt=0 if and only if S=ˉS and I1=¯I1, which implies that S→ˉS, I1→¯I1 and I2→0 as t→∞. By LaSalle's invariant principle, this implies that all solutions in Ω1 approach the plane S=ˉS, I1=¯I1 and I2=0 as t→∞. Also, All solutions of (3.1) contained in such plane satisfy ˙V1=rˉS−μV1, which implies that V1→rˉSμ=¯V1 as t→∞, that is, all of these solution approach E1. Therefore we conclude that E1 is globally asymptotically stable in Ω1.
Corollary 1. If 2−F1(ˉS,¯I1)F1(S,¯I1)+F1(S,I1)F1(S,¯I1)−I1¯I1−¯I1f1(S,I1)F1(ˉS,¯I1)≤0 and R2<1 then E1 is globally asymptotically stable.
Theorem 10. Suppose that ∂F2(S,I2)∂I2≥0 for all I2, then E2 is globally asymptotically stable if,
Proof. Consider the Lyapunov function
Since I1>0, then V(S,V1,I1,I2)≥0 and V(S,V1,I1,I2) attains zero at I1=0. Now, we need to show ˙V≤0.
For S≤S0
Furthermore, dVdt=0 if and only if I1=0. Suppose that (S(t),V1(t),I1(t),I2(t)) is a solution of (3.1) contained entirely in the set M={(S(t),V1(t),I1(t),I2(t))∈Ω1|˙V=0}. Then, ˙I1=0 and, from the above inequalities, we have I1=0. Thus, the largest positively invariant set contained in M is the plane I1=0. By LaSalle’s invariance principle, this implies that all solutions in approach the plane I1=0 as t→∞. On the other hand, solutions of (3.1) contained in such plane satisfy.
Now we will show that S(t)→˜S, V1(t)→~V1 and I1(t)→~I1 Consider the Lyapunov function
Now, we need to show ˙V≤0.
Note that
The last inequality is due to the Lemma 5 and the relation of the geometric and arithmetic means, then ˙V≤0. Furthermore, ˙V=0 if and only if S=˜S, I2=~I2 and V1=~V1. Therefore E2 is globally asymptotically stable.
Remark 6. Note that if ∂g2(S,I2)∂S≥0, then
Corollary 2. If 2−F2(˜S,~I2)F2(S,~I2)+F2(S,I2)F2(S,~I2)−I2~I2−~I2f2(S,I2)F2(˜S,~I2)≤0, R1<1 and 2−F2(˜S,~I2)F2(S,~I2)+SF2(˜S,~I2)˜SF2(S,~I2)−V1~V1−S~V1˜SV1≤0 then E2 is globally asymptotically stable.
Theorem 11. E3 is globally asymptotically stable if
Proof. Assume E3 exists. Consider the Lyapunov function
Where g(x) = x -1 -ln(x) . Then V (S, V_1, I_1, I_2) \geq 0 and V (S, V_1, I_1, I_2) attains zero at E_3 .
Now, we need to show \dot{V}\leq 0 .
By the relation of geometric and arithmetic means, we conclude \dot{V}\leq 0 , with equality holding only at the equilibrium E_3 . Therefore E_3 is globally asymptotically stable.
3.5. Numerical simulations
In this section, we present some numerical simulations of the solutions for system (3.1) to verify the results obtained in section 3.3 and give examples to illustrate theorems in section 3.4. In system (3.1), we set:
In this case
Parameters and units are arbitrary and have been used for illustration purposes only. Anyway, when considering a realistic scenario such values could be derived from statistical data.
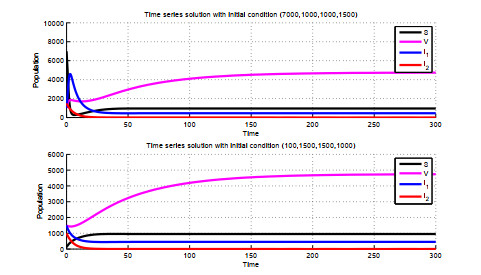
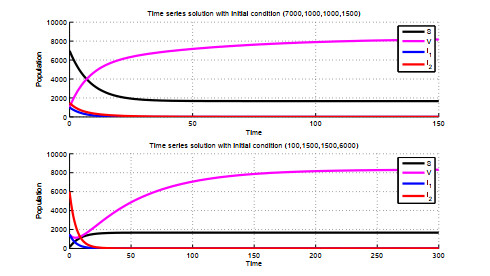
• Example 6.1. In system (3.1), we set \beta_1 = 0.00003 , r = 0.1 , \beta_2 = 0.0002 , \zeta_1 = 0.7 and \zeta_2 = 0.9 . Then S^0\approx 1667 , V^0\approx 8333 , \mathcal{R}_1\approx0.2632 \mathcal{R}_2\approx0.7947 . By Theorem 8, we see that the disease-free equilibrium E_0 is globally asymptotically stable. Numerical simulation illustrates our result (see Figure 1).
• Example 6.2. In system (3.1), we set \beta_1 = 0.0002 , r = 0.1 , \beta_2 = 0.0002 , \zeta_1 = 0 and \zeta_2 = 0.9 . Then \bar{S}\approx 950 , \bar{V_1}\approx 4750 , \bar{I_1}\approx 453 , \mathcal{R}_1\approx1.7544 , \mathcal{R}_2\approx0.7947 . By Theorem 9, we see that the E_1 is globally asymptotically stable. Numerical simulation illustrates our result (see Figure 2).
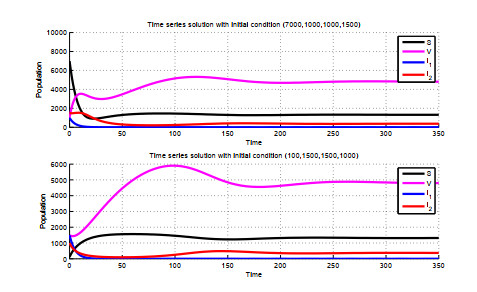
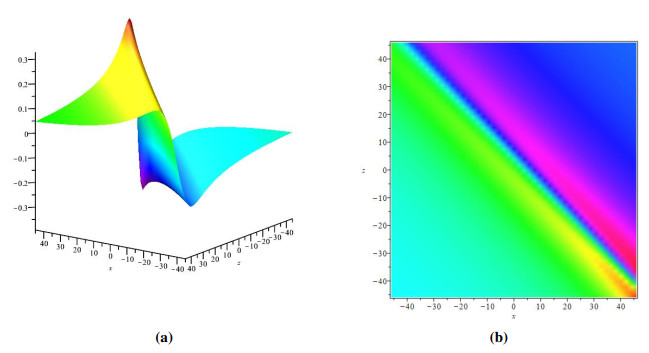
• Example 6.3. In system (3.1), we set \beta_1 = 0.00003 , r = 0.1 , \beta_2 = 0.0002 , \zeta_1 = 0.7 and \zeta_2 = 0.001 . Then \tilde{S}\approx 1317 , \tilde{V_1}\approx 4814 , \tilde{I_2}\approx 368 , \mathcal{R}_1\approx 0.2632 , \mathcal{R}_2\approx 1.3889 and 2-\frac{F_2(\tilde{S}, \tilde{I_2})}{F_2(S, \tilde{I_2})}+\frac{S F_2(\tilde{S}, \tilde{I_2})}{\tilde{S} F_2(S, \tilde{I_2})}-\frac{V_1}{\tilde{V_1}}-\frac{S\tilde{V_1}}{\tilde{S}V_1}\leq 0 (see Figure 3). By corollary 2, we see that the E_2 is globally asymptotically stable. Numerical simulation illustrates our result (see Figure 4).
• Example 6.4. In system (3.1), we set \beta_1 = 0.0002 , r = 0.01 , \beta_2 = 0.0002 , \zeta_1 = 0.0001 and \zeta_2 = 0.0001 . Then \mathcal{R}_1\approx 7.0175 , \mathcal{R}_2\approx 4.1270 , \tilde{S}\approx 1134 , \bar{S}\approx 5310 , \bar{V_1} \approx 2655 , \bar{R_2}\approx 3.555 and \tilde{R_1}\approx 1.194 . Then by Theorem 6, E_3 = (S^*, V_1^*, I_1^*, I_2^*) exists ( S^*\approx 1133 , V_1^*\approx 320 , I_1^* \approx 44 , I_2^* \approx 774 ), Also c_1\approx 0.2501 c_2\approx 0.0171 c_3\approx 3.4759\times 10^{-04} c_4\approx 3.4759\times 3.9242 10^{-06} , c_1 c_2-c_3^2\approx 0.0043 and c_1c_2c_3-c_3^2-c_1^2c_4\approx 1.1218e\times 10^{-06} by Theorem 7, E_3 is locally asymptotically stable. Also E_3 satisfies F_1(S^*, I_1^*)\left(2 - \frac{S^*}{S}- \frac{S g_1(S, I_1)}{S^* g_1(S^*, I_1^*)} \right)+F_2(S^*, I_2^*) \left(2-\frac{S^*}{S}-\frac{S g_2(S, I_2)}{S^* g_2(S^*, I_2^*)}\right)+\mu S^* \left(2- \frac{S^*}{S}-\frac{S}{S^*} \right) +I_1 \left(S^* g_1(S, I_1)-\alpha_1 \right)+I_2 \left({S}^* g_2(S, I_2)+ k {V_1}^*-\alpha_2 \right) < 0 . By Theorem 11, we see that the E_3 is globally asymptotically stable. Numerical simulation illustrates our result (see Figure 5).
4.
Conclusions and discussions
In this paper, we studied a system of ordinary differential equations to model the disease dynamics of two strains of influenza with only one vaccination for strain 1 being implemented, and general incidence rate for strain 1 and strain 2. We obtained four equilibrium points:
● E_0 disease-free equilibrium, I_1 and I_2 are both zero.
● E_1 single-strain-infection equilibria, I_2 are zero.
● E_2 single-strain-infection-equilibria, I_1 are zero.
● E_3 double-strain-infection equilibrium, I_1 and I_2 are both positive.
We have investigated the topics of existence and non-existence of equilibrium points and their stabilities. We also used the next-generation matrix method to obtain two threshold quantities \mathcal{R}_1 and \mathcal{R}_2 , called the basic reproduction ratios for strain 1 and 2 respectively. It was shown that the global stability of each of the equilibrium points depends on the magnitude of these threshold quantities. More precisely, we have proved the following:
● If \mathcal{R}_0 < 1 the disease free equilibrium E_0 is globally asymptotically stable and if \mathcal{R}_0 > 1 , then E_0 is unstable.
● If \mathcal{R}_1 > 1 the model (3.1) admits a unique single-strain-infection-equilibria E_1 . Also if \mathcal{R}_2 < 1 then E_1 is globally asymptotically stable and if \bar{\mathcal{R}_2} > 1 , then E_1 is unstable.
● If \mathcal{R}_2 > 1 the model (3.1) admits a unique single-strain-infection equilibria E_2 . Also if \mathcal{R}_1 < 1 and 2-\frac{F_2(\tilde{S}, \tilde{I_2})}{F_2(S, \tilde{I_2})}+\frac{S F_2(\tilde{S}, \tilde{I_2})}{\tilde{S} F_2(S, \tilde{I_2})}-\frac{V_1}{\tilde{V_1}}-\frac{S\tilde{V_1}}{\tilde{S}V_1} < 0 , then E_2 is globally asymptotically stable and if \tilde{\mathcal{R}_1} > 1 , then E_2 is unstable.
● If \bar{\mathcal{R}_2} > 1 and \tilde{\mathcal{R}_1} > 1 the model (3.1) admits a double strain infection equilibrium E_3 . Also if F_1(S^*, I_1^*)\left(2 - \frac{S^*}{S}- \frac{S g_1(S, I_1)}{S^* g_1(S^*, I_1^*)} \right)+F_2(S^*, I_2^*) \left(2-\frac{S^*}{S}-\frac{S g_2(S, I_2)}{S^* g_2(S^*, I_2^*)}\right) +r S^*\left(3-\frac{S^*}{S}-\frac{V_1}{V_1^*}-\frac{S V_1^*}{S^* V_1}\right)+\mu S^* \left(2- \frac{S^*}{S}-\frac{S}{S^*} \right) +I_1 \left(S^* g_1(S, I_1)-\alpha_1 \right)+I_2 \left({S}^* \right. \left.g_2(S, I_2)+k {V_1}^*-\alpha_2 \right) < 0 . Then E_3 is globally asymptotically stable.
In order to discuss the meaning of our mathematical results, let us rewrite the two key indirect parameters \mathcal{R}_1 and \mathcal{R}_2 in terms of the rate of vaccination ( r ), the incidence rate of strain 1 ( F_1(S, I_1) ) and the incidence rate of strain 2 ( F_2(S, I_2) ) as shown below:
Also, the derivative of \mathcal{R}_2 with respect to r is,
Note that \mathcal{R}_1(r) is decreasing and \mathcal{R}_2(r) depends on \frac{\partial f_2\left(\frac{\Lambda}{\mu}, 0\right)}{\partial S} . Now we will analyse some cases of incidence rate.
(C1) F_i(S, I) = \beta_i SI_i , then \frac{\partial f_2\left(\frac{\Lambda}{\mu}, 0\right)}{\partial S} = \beta_i .
(C2) F_i(S, I) = \frac{\beta_i SI_i} {1 + \zeta_i S} , then \frac{\partial f_2\left(\frac{\Lambda}{\mu}, 0\right)}{\partial S} = \frac{\beta_i} {1 + \zeta_i \left(\frac{\Lambda}{r+\mu} \right)} .
(C3) F_i(S, I) = \frac{\beta_i SI_i} {1 + \zeta_i I_i^2} , then \frac{\partial f_2\left(\frac{\Lambda}{\mu}, 0\right)}{\partial S} = \beta_i .
Note that for (C1) and (C3), \mathcal{R}_2(r) is increasing if \beta_i < k , \mathcal{R}_2(r) is decreasing if \beta_i > k and \mathcal{R}_2(r) is constant if \beta_i = k . For (C2), \mathcal{R}_2(r) is increasing if \beta_i\leq k ( \zeta\neq 0 ). If \beta_i > k \mathcal{R}_2(r) is increasing if \frac{\zeta_i k \Lambda}{\beta_i-k}-\mu < r and decreasing if \frac{\zeta_i k \Lambda}{\beta_i-k}-\mu > r .
Furthermore, if the force of infection of strain 1 is (C2), then \mathcal{R}_1 = \frac{\beta_1}{\alpha_1(1 + \zeta_1 S^0)} , note that \mathcal{R}_1 is decreasing in \zeta_1 . If the force of infection of strain 2 is (C2), then \mathcal{R}_2 = \frac{\beta_2 \Lambda}{\alpha_2(\lambda+\zeta_2 \Lambda)}+\frac{k r \Lambda}{\alpha_2 \mu \lambda} , note that \mathcal{R}_2 is decreasing in \zeta_2 .
With the above information and the results in section 3.4, we conclude that the vaccination is always beneficial for controlling strain 1, its impact on strain 2 depends on the force of infection of strain 2. For example, if the force of infection of strain 2 is (C2), the impact of vaccination depends on values of \beta_2 , k and \zeta_2 . If \zeta_2 = 0 and \beta_2 > k it plays a positive role and if \zeta_2 = 0 and \beta_2 < k , it has a negative impact in controlling strain 2. This is reasonable because larger k (than \beta_2 ) means that vaccinated individuals are more likely to be infected by strain 2 than those who are not vaccinated, and thus, is helpful to strain 2. Smaller k (than \beta_2 ) implies the opposite. If \zeta_2 \neq 0 and \beta_2 \leq k , it plays a negative role and if \zeta_2 \neq 0 and \beta_2 > k , not necessarily has a positivity impact in controlling strain 2. This is reasonable because larger k (than \beta_2 ) means that vaccinated individuals are more likely to be infected by strain 2 than those who are not vaccinated, but if \zeta_2 is large it means that the population is taking precautions to avoid the infection of strain 2. Also, we conclude that \zeta_1 (of the force of infection (C2)) is always beneficial for controlling strain 1 and \zeta_2 (of the force of infection (C2)) is always beneficial for controlling strain 2, it means that it is very important that people are taking precautions not to get infected.
Acknowledgments
This work was supported by Sistema Nacional de Investigadores (15284) and Conacyt-Becas.
Conflict of interest
No conflict of interest.









 DownLoad:
DownLoad: