Global stability of a tridiagonal competition model with seasonal succession

1.   Introduction

The far-reaching consequences of ecological interactions in the dynamics of biological communities remain an intriguing subject. The interaction between two species can basically be of three different kinds: competition, mutualism and predator-prey. Competition and predator-prey are, perhaps, the most well-known types of interactions. They embody the natural dispute for resources in ecology. Competition is characterized by a decrease of the growth rate as the density of the other species increases, which has been extensively studied (see ^[1,2,3,4]). One special competition example occurs in the water column of an ocean or on a steep mountain side or on island groups, where each species dominates a species zone (depth, altitude or different island) but is obliged to interact with other species in the narrow overlap of their zones of dominance. Taking three species as an example, one can think of a hierarchy of species $x_1$, $x_2$ and $x_3$, where $x_i$ is the density or biomass of the $i$-th species. In this hierarchy, $x_1$ only competes with $x_2$, $x_3$ only with $x_2$, and $x_2$ competes with $x_1$ and $x_3$. The concrete form is as follows,

where $r_i$ and $a_{ij}$ are all positive real numbers. Such system is called tridiagonal competitive system and there have also been a number of theoretical works (see ^[5,6,7,8]).

As we know, interactive species often live in a fluctuating environment. Due to seasonal or daily variations, species experience a periodic dynamical environment such as temperature, rainfall, humidity, wind, and other resources. A typical example here is the phytoplankton and zooplankton of the temperate lakes, where the species grow during the warmer months and die off or form resting stages in winter. The phenomenon is described as seasonal succession. It has been a fascinating subject for ecologists and mathematicians to explore the dynamics of periodic models by means of seasonal succession numerically and analytically. Recently, Klausmeier ^[9] proposed a novel approach, called successional state dynamics (SSD), to modeling seasonally forced food webs. The SSD approach treats succession as a series of state transitions driven by both the internal dynamics of species interactions and external forcing, and can uncover unexpected phenomena such as multiple stable annual trajectories and year-to-year irregularity in successional trajectories (chaos). Steiner et al. ^[10] later validated the utility of the SSD approach as a framework for predicting the effects of altered seasonality on the structure and dynamics of multitrophic communities by using controlled laboratory experiments.

However, there are few analytic results on these ecological models with seasonal succession. One of the major reasons is that the vector fields of these models are discontinuous and periodic in time $t$. Hsu and Zhao ^[11] first studied the global dynamics of a Lotka-Volterra two-species competition model with seasonal succession and obtained a complete classification for the global dynamics and the effects of season succession on the competition outcomes via the theory of monotone dynamical systems. Recently, Niu et al. ^[12] were concerned with an $n$-dimensional Lotka-Volterra competition model with seasonal succession and obtained the existence of a carrying simplex. Based on this, they reconsidered the two-dimensional model proposed by Hsu and Zhao ^[11] and simplified their approach to acquire the complete classification of global dynamics. In ^[13], Xie and Niu analyzed a three-dimensional Lotka-Volterra cooperation model with seasonal succession and derived a completed dynamics of global coexistence and extinction, which extends previous results with respect to three-dimensional cooperation models. There are other works on seasonal succession, such as ^{[14,15,16,17,18]} and references therein.

Yet, to our knowledge, there are few works on the global stability for high dimensional competitive systems with seasonal succession due to such obstacles as the estimates for the Floquet multipliers, the existence and uniqueness of the positive periodic solutions, etc. Therefore, it is interesting for us to introduce the seasonal succession into the three-speices tridiagonal competition model and study the global stability for such a periodically forced system. Motivated by the modelling methods in Klausmeier ^[9], we propose a tridiagonal three-species competition model with seasonal succession as follows:

where $m\in \mathbb{Z_+}$, $\varphi \in [0, 1]$ and $\omega$, $\lambda_i$, $r_i$, $a_{ij}$ $(i, j = 1, 2, 3)$ are all positive constants.

In particular, if $\varphi = 0$, then the system (1.2) become the linear system

While, if $\varphi = 1$, system (1.2) turns out to be the tridiagonal competitive system (1.1).

Obviously, system (1.2) is a time periodic system in a season alternate environment. Overall period is $\omega$, and $\varphi$ stands for the switching proportion of a period between two subsystems (1.1) and (1.3). Biologically, $\varphi$ is used to describe the proportion of the period in the good season in which the species follow system (1.1), while $(1-\varphi)$ represents the proportion of the period in the bad season in which the species die exponentially according to system (1.3).

In addition, when we write

where $i, j = 1, 2, 3$, system (1.2) can be expressed as a three-dimensional time $\omega$-periodic tridiagonal competitive system with discontinuous $\omega$-periodic coefficients,

From system (1.2), it can easily be seen that system (1.2) admits a unique nonnegative global solution $x(t, x^0)$ on $[0, +\infty)$ for any $x^0 \in \mathbb{R}^3_+$. Since the system is $\omega$-periodic, it suffices to consider the Poincar$\rm \acute{e}$ map $S$ on $\mathbb{R}^3_+$, that is,

Let us first define a linear map $L$ by

We also let $\{Q_t\}_{t\geq 0}$ represent the solution flow associated with the tridiagonal competitive system (1.1). Then, $Q_t(x^0)$ is the unique global solution of the system (1.1) on $[0, +\infty).$ Thus, we have

We only need to focus on the dynamics of the discrete-time system $\{S^n\}_{n\geq 0}$.

The purpose of this paper is to investigate the global stability for system (1.2). Firstly, the Floquet multipliers of all non-negative periodic solutions, including trivial periodic solution, semi-trivial periodic solutions and the positive periodic solutions, are estimated via the stability analysis of equilibria (see Lemmas 3.1–3.8). To obtain the existence and uniqueness for the positive periodic solution, we provide the index theory of the fixed points for the Poincaré map $S$ (see Lemma 4.1). Compared to the proof of the existence and uniqueness for the positive periodic solution in Xie and Niu ^[13] and [Lemmas 4.1 and 4.2], our approach is much more general which avoids Brouwer fixed point theorem and the connecting orbits theorem and can be applied to more mappings, such as the the Poincaré maps associated with competition and cooperation models with seasonal succession. Together with the existence of carrying simplex, we obtain that system (1.2) has a unique positive periodic solution under appropriate conditions, and moreover the positive periodic solution is globally asymptotically stable in Int$\mathbb{R}^3_+$ (see Theorem 4.4). In addition, sufficient conditions for two-species coexistence, two-species extinction and global extinction are given (see Theorems 4.5–4.9). Some numerical examples are provided to illustrate our theoretical results (see Figures 1–5).

The paper is organized as follows. In Section 2, we introduce some notations, relevant definitions and preliminaries. Section 3 is devoted to analyzing the local dynamics of all nonnegative periodic solutions of system (1.2). In Section 4, the global dynamics of coexistence and extinction for three competing species in terms of system (1.2) are obtained. In Section 5, we present some numerical simulations to illustrate our analytic results. The paper ends with a discussion in Section 6.

2.   Notations and preliminaries

In this section, we first introduce some definitions and describe some results which are essential tools for the later sections.

Define $\mathbb{Z}_+$ to be the set of nonnegative integers. Let $\mathbb{R}^3_+ = \{x\in \mathbb{R}^3:x_i\geq 0, \forall \ i\in \Lambda\}$ and Int$\mathbb{R}^3_+ = \{x\in \mathbb{R}^3:x_i > 0, \forall \ i\in \Lambda\}$, where $\Lambda: = \{1, 2, 3\}$. Let $\emptyset \neq L \subset \Lambda$ and $\bar{L} = \Lambda \setminus L$ be its complementary set in $\Lambda$. We define the sets $H_L = \{x \in \mathbb{R}^3: x_j = 0 \ \ {\rm for} \ \ j\in \bar{L} \}$, $H_L^+ = \mathbb{R}^3_+ \cap H_L$, and Int$H_L^+ = \{x\in H_L^+: x_i > 0 \ \ {\rm for \ all}\ \ i\in L \}$. For two vectors $x, y \in \mathbb{R}^3$, we write $x\leq y$ if $x_i\leq y_i$ for all $i \in \Lambda$, and $x \ll y$ if $x_i < y_i$ for all $i \in \Lambda$. If $x \leq y$ but $x \neq y$, we write $x < y$. If $x, y \in \mathbb{R}^3$ and $x\leq y$, let $[x, y] = \{z \in \mathbb{R}^3 : x\leq z\leq y \}$ be a closed order interval, and if $x\ll y$, let $[[x, y]] = \{z\in \mathbb{R}^3:x\ll z \ll y\}$ be a open order interval. For an $n\times n$ matrix $A$, we write $A\geq 0$ iff $A$ is a nonnegative matrix (i.e., all the entries are nonnegative) and $A\gg 0$ iff $A$ is a positive matrix (i.e., all the entries are positive).

Let $X\subset \mathbb{R}^3_+$ and $S:X \rightarrow X$ be the Poincar$\acute{\rm e}$ (period) map. The orbit of a state $x$ for $S$ is $\gamma(x) = \{S^n(x)$, $n\in \mathbb{Z}_+ \}$. A fixed point $x$ of $S$ is a point $x\in X$ such that $S(x) = x$. A point $y\in X$ is called a $k$-periodic point of $S$ if there exists some positive integer $k > 1$, such that $S^k(y) = y$ and $S^m(y)\neq y$ for every positive integer $m < k$. The $k$-periodic orbit of the $k$-periodic point $y$, $\gamma(y) = \{y$, $S(y)$, $S^2(y)$, $...$, $S^{k-1}(y)\}$, is often called a periodic orbit for short. The $\omega$-limit set of $x$ is defined by $\omega(x) = \{y\in \mathbb{R}_+^3: S^{n_k}x\rightarrow y \ (k\rightarrow \infty)\ {\rm for \ some \ sequence} \ n_k\rightarrow +\infty \ {\rm in} \ \mathbb{Z}_+\}$. A set $V\subset X$ is positively invariant under $S$ if $SV\subset V$, and invariant if $SV = V$. Note that if the orbit $\gamma(x)$ of $x$ has compact closure, $\omega(x)$ is nonempty, compact and invariant. If $S$ is a differentiable map, we write $DS(x)$ as the Jacobian matrix of $S$ at the point $x$, and $r(DS(x))$ stands for the spectral radius of $DS(x)$.

A continuous map $S:\mathbb{R}_+^3 \rightarrow \mathbb{R}_+^3$ is said to be monotone if $S(x)\leq S(y)$ whenever $x\leq y$ with $x, y\in \mathbb{R}_+^3$; $S$ is called strictly monotone if $x < y$, then $S(x) < S(y)$; strongly monotone if $x < y$, then $S(x)\ll S(y)$.

A carrying simplex for the periodic map $S$ is a subset $\Sigma\subset \mathbb{R}_+^n\backslash \{0\}$ with the following properties ^[26]:

$\rm(P1)$ $\Sigma$ is compact, invariant and unordered;

$\rm(P2)$ $\Sigma$ is homeomorphic via radial projection to the $(n-1)$-dim standard probability simplex $\Delta^{n-1}: = \{x\in \mathbb{R}_+^n |$ $\Sigma_ix_i = 1\}$;

$\rm(P3)$ $\forall\ x\in \mathbb{R}_+^n\backslash \{0\}$, there exists some $y\in \Sigma$ such that $\lim\limits_{n\rightarrow \infty}|S^nx-S^ny| = 0$.

Lemma 2.1. ([11, Lemma 2.1]) Let $x(t, x_0)$ be the unique solution of the following system

where $\lambda$, $r$, $a$ are all positive constants. Then the following two statements are valid:

(ⅰ) If $r\varphi-\lambda(1-\varphi)\leq 0$, then $\lim\limits_{t\rightarrow \infty}x(t, x_0) = 0$ for all $x_0\in \mathbb{R}_+$;

(ⅱ) If $r\varphi-\lambda(1-\varphi) > 0$, then system (2.1) admits a unique positive $\omega$-periodic solution $x^*(t)$, and $\lim\limits_{t\rightarrow \infty}\big(x(t, x_0)-x^*(t)\big) = 0$ for all $x_0\in \mathbb{R}_+\backslash \{0\}$.

Lemma 2.2. (Boundness) The Poincar$\rm \acute{e}$ map $S$ associated with system (1.2) is bounded in $\mathbb{R}_+^3$. Moreover, every forward orbit of $S$ is precompact in $\mathbb{R}_+^3$.

Proof. Firstly, we rewrite system ( 1.1 ) as

where

Let $b = \max\big\{ \frac{r_1}{a_{11}}+1, \frac{r_2}{a_{22} }+1, \frac{r_3}{a_{33}}+1 \big\}$, we have $B: = (b, b, b)\gg 0$. For any positive number $l\geq 1$, whenever $x: = (x_1, x_2, x_3) \in [0, lB]$ satisfies $x_1 = lb$, then

Similarly, for any $x \in [0, lB]$ satisfies $x_2 = lb$, then

while, for any $x \in [0, lB]$ satisfies $x_3 = lb$, we also have

Thus, $[0, lB]$ is positive invariant for system ( 1.1 ).

For any $x^0\in \mathbb{R}_+^3$, there exists some positive number $l_0\geq 1$ such that $0\leq x^0 \leq l_0B$. The positive invariance of $[0, l_0B]$ implies that $0\leq x(t, x^0)\leq l_0B$ for all $t\geq 0$ where $x(t, x^0) = \big(x_1(t), x_2(t), x_3(t)\big)$ stands for the solution of system (1.1) with initial value $x^0$ in $\mathbb{R}_+^3$. Note that $\{Q_t\}_{t\geq 0}$ is the solution flow of system (1.1), it follows that $0\leq Q_t(x^0)\leq l_0B$ for any $t\geq 0$. By the expression $(*)$ of $L(x_1, x_2, x_3)$, we have $Lx^0\leq x^0$, and then, $0\leq Q_t(Lx^0)\leq l_0B$ for any $t\geq 0$. This implies that $0 \leq Q_{\varphi\omega}(Lx^0) = S(x^0) \leq l_0B$, that is, $S$ is bounded. Based on this, we easily see that every forward orbit of $S$ is precompact. The proof is completed.

Lemma 2.3. (Monotonicity) If $x, y \in \mathbb{R}_+^3$ and $S(x) < S(y)$, then $x < y$. In particular, if $x, y \in {\rm{Int}} \mathbb{R}_+^3$ and $S(x) < S(y)$, then $x\ll y$.

Proof. For $x, y \in \mathbb{R}_+^3$, if $S(x) < S(y)$, then $Q_{\varphi\omega}(Lx) < Q_{\varphi\omega}(Ly)$. Define $u(t): = Q_{\varphi\omega-t}(Lx)$ and $v(t): = Q_{\varphi\omega-t}(Ly)$. It is clear that $u(t)$ and $v(t)$ are two solutions of a three dimensional cooperative system for $t \in [0, \varphi\omega]$. Since $u(0) = Q_{\varphi\omega}(Lx) < Q_{\varphi\omega}(Ly) = v(0)$, it follows from the comparison theorem of cooperative systems that $Lx = u(\varphi\omega) < v(\varphi\omega) = Ly$. By the expression of the linear map $L$, we have $x < y$. For the strong monotonicity of $S$, see Smith [24, Chapter 3].

Remark 2.1. By the expression of system (1.4), $H_L, H_L^+$ and ${\rm{Int}}$$H_L^+$ are positively invariant under the map $S$. So we can rewrite the Poincar$\rm \acute{e}$ map $S$ as:

where

Moreover, $G_i(x)$ are continuous functions with $G_i(x)\geq 0$ for $x\in \mathbb{R}_+^3, i = 1, 2, 3$.

3.   Local dynamics

In this section, we will analyze the local stabilities of all nonnegative fixed points of $S$ in $\mathbb{R}_+^3$. Clearly, $O: = (0, 0, 0)$ is a trivial fixed point of $S$. Let $h_i: = (r_i\varphi-\lambda_i(1-\varphi))\omega, i = 1, 2, 3.$ By Lemma 2.1, if $h_i > 0$ $(i = 1, 2, 3)$, then system (1.2) has three axial fixed points, say $R_1: = (x_1^*, 0, 0)$, $R_2: = (0, x_2^*, 0)$ and $R_3: = (0, 0, x_3^*)$. By the second and fourth equations of system (1.2), it is not difficult to see that there admits a unique interior fixed point in coordinate planar $\{x_2 = 0\}$, say $E_2 = (\hat{x}_1, 0, \hat{x}_3)$. Noticing that Hsu and Zhao [11, Theorems 2.3 and 2.4], system (1.2) has interior fixed points in coordinate planar $\{x_1 = 0\}$ and $\{x_3 = 0\}$ under certain conditions. If exist, we write them as $E_3 = (\bar{x}_1, \bar{x}_2, 0)$ and $E_1 = (0, \check{x}_2, \check{x}_3)$, respectively.

Lemma 3.1. (Stability of the fixed point $O$)

(ⅰ) If $h_i < 0$ $(i = 1, 2, 3)$, then $O$ is an asymptotically stable fixed point of $S$.

(ⅱ) If one of $h_1, h_2$ and $h_3$ is greater than $0$, then $O$ is an unstable fixed point of $S$. In particular, if $h_i > 0$ $(i = 1, 2, 3)$, then $O$ is a hyperbolic repeller.

Proof. Let $F(x) = (F_1, F_2, F_3)^T$, where

Then, $DF(x) =$

For simplicity, we denote $u(t, x): = Q_t(x)$ and $V(t, x): = D_xu(t, x) = D_xQ_t(x)$. Then $S(x) = Q_{\varphi\omega}(Lx) = u(\varphi\omega, Lx)$. Thus,

Note that $V(t, x)$ satisfies

Taking $x = O$, we have $u(t, LO) = (0, 0, 0)$, which implies that $DF(O) = {\rm diag}\{r_1, r_2, r_3\}$. Then,

and hence, one has

Consequently, the matrix $DS(O)$ has three positive eigenvalues $\mu_1, \mu_2$ and $\mu_3$ given by

Then, the conclusion is immediate.

By Lemma 3.1, we assume that $h_i > 0$ $(i = 1, 2, 3)$ in following Lemmas 3.2–3.8.

Lemma 3.2. (Stability of the axial fixed point $R_1$)

(ⅰ) If $\frac{h_2}{a_{21}} > \frac{h_{1}}{a_{11}}$, then $R_1$ is a saddle point with one-dimensional stable manifold.

(ⅱ) If $\frac{h_2}{a_{21}} < \frac{h_{1}}{a_{11}}$, then $R_1$ is a saddle point with two-dimensional stable manifold.

Proof. Let $u(t, LR_1): = (u_1^*(t), 0, 0)$. By the proof of Lemma 3.1, it is not difficult to see that

Then,

Note that $u_1^*(t)$ satisfies the equation $\frac{du_1^*(t)}{dt} = u_1^*(t)\big(r_1-a_{11}u_1^*(t)\big)$, it follows that

and then,

where $*$ stands for unknown algebraic expression. Therefore, the matrix $DS(R_1)$ has three positive eigenvalues $\mu_1, \mu_2$ and $\mu_3$ given by

Therefore, the conclusion is immediate.

Similarly, we have the Lemmas 3.3 and 3.4.

Lemma 3.3. (Stability of the axial fixed point $R_2$)

(ⅰ) If $\frac{h_{2}}{a_{22}} > \max\{\frac{h_1}{a_{12}}, \frac{h_3}{a_{32}}\}$, then $R_2$ is an asymptotically stable fixed point of $S$.

(ⅱ) If $\frac{h_{2}}{a_{22}} < \min\{\frac{h_1}{a_{12}}, \frac{h_3}{a_{32}}\}$, then $R_2$ is a saddle point with one-dimensional stable manifold.

(ⅲ) If $\frac{h_1}{a_{12}} < \frac{h_{2}}{a_{22}} < \frac{h_3}{a_{32}}$ or $\frac{h_1}{a_{12}} > \frac{h_{2}}{a_{22}} > \frac{h_3}{a_{32}}$, then $R_2$ is a saddle point with two-dimensional stable manifold.

Lemma 3.4. (Stability of the axial fixed point $R_3$)

(ⅰ) If $\frac{h_2}{a_{23}} > \frac{h_{3}}{a_{33}}$, then $R_3$ is a saddle point with one-dimensional stable manifold.

(ⅱ) If $\frac{h_2}{a_{23}} < \frac{h_{3}}{a_{33}}$, then $R_3$ is a saddle point with two-dimensional stable manifold.

Lemma 3.5. (Stability of the planar fixed point $E_3$) If $\frac{h_2}{a_{21}} > (<)\frac{h_1}{a_{11}}, \frac{h_1}{a_{12}} > (<)\frac{h_2}{a_{22}}$, then there admits a unique interior fixed point, say $E_3: = (\bar{x}_1, \bar{x}_2, 0)$, for $S$ in the coordinate plane $\{x_3 = 0\}$. Moreover,

${\rm(i)}$ If $\frac{h_2}{a_{21}} > \frac{h_1}{a_{11}}, \frac{h_1}{a_{12}} > \frac{h_2}{a_{22}}$ and $\frac{h_3}{a_{32}} < \frac{h_1a_{21}-h_2a_{11}}{a_{12}a_{21}-a_{11}a_{22}}$, then $E_{3}$ is an asymptotically stable fixed point of $S$.

${\rm(ii)}$ If $\frac{h_2}{a_{21}} < \frac{h_1}{a_{11}}, \frac{h_1}{a_{12}} < \frac{h_2}{a_{22}}$ and $\frac{h_3}{a_{32}} < \frac{h_1a_{21}-h_2a_{11}}{a_{12}a_{21}-a_{11}a_{22}}$, then $E_{3}$ is a saddle point with two-dimensional stable manifold.

${\rm(iii)}$ If $\frac{h_2}{a_{21}} < \frac{h_1}{a_{11}}, \frac{h_1}{a_{12}} < \frac{h_2}{a_{22}}$ and $\frac{h_3}{a_{32}} > \frac{h_1a_{21}-h_2a_{11}}{a_{12}a_{21}-a_{11}a_{22}}$, then $E_{3}$ is a saddle point with one-dimensional stable manifold.

Proof. By Remark 2.1, we can see that all coordinate axes and planes are positively invariant under the map $S$. Theorem 3.6 in ^[12] implies that if $\frac{h_2}{a_{21}} > (<)\frac{h_1}{a_{11}}, \frac{h_1}{a_{12}} > (<)\frac{h_2}{a_{22}}$, then system (1.2) admits a unique interior fixed point in the coordinate planar $\{x_3 = 0\}$, say $E_{3}: = (\bar{x}_1, \bar{x}_2, 0)$. Let $F(x)$, $u(t, x)$ and $V(t, x)$ be denoted as in the proof of Lemma 3.1. We also define $u(t, LE_{3}): = (\bar{u}_1(t), \bar{u}_2(t), 0)$. Then,

For convenience, we write $DF(u(t, LE_{3})): = \left (\begin{matrix} A_1 & B_1 \\ 0 & r_3-a_{32}\bar{u}_2(t) \\ \end{matrix} \right)$, where

Since $DS(x) = V(\varphi\omega, Lx)\cdot D(Lx)$, it follows that $DS(E_{3})$ has a positive eigenvalue $\mu_3$ given by

Note that $\bar{u}_1(t)$ and $\bar{u}_2(t)$ satisfy the following equations

Integrating the equation (3.4) for $t$ from $0$ to $\varphi\omega$, one has

Then,

On the other hand, the other two eigenvalues $\mu_1$ and $\mu_2$ are determined by $A_1$. Together with Lemma 2.4 in ^[11], the conclusion is immediate.

Similarly, we also have the following Lemmas 3.6 and 3.7.

Lemma 3.6. (Stability of the planar fixed point $E_{2}$) There admits a unique interior fixed point, say $E_{2}: = (\hat{x}_1, 0, \hat{x}_3)$, for $S$ in the coordinate plane $\{x_2 = 0\}$. Moreover,

${\rm(i)}$ If $h_2 < \frac{h_1a_{21}}{a_{11}}+\frac{h_3a_{23}}{a_{33}}$, then $E_{2}$ is an asymptotically stable fixed point of $S$.

${\rm(ii)}$ If $h_2 > \frac{h_1a_{21}}{a_{11}}+\frac{h_3a_{23}}{a_{33}}$, then $E_{2}$ is a saddle point with two-dimensional stable manifold.

Lemma 3.7. (Stability of the planar fixed point $E_{1}$) If $\frac{h_2}{a_{23}} > (<)\frac{h_{3}}{a_{33}}, \frac{h_3}{a_{32}} > (<)\frac{h_{2}}{a_{22}}$, then there admits a unique interior fixed point, say $E_{1}: = (0, \check{x}_2, \check{x}_3)$, for $S$ in the coordinate plane $\{x_1 = 0\}$. Moreover,

${\rm(i)}$ If $\frac{h_2}{a_{23}} > \frac{h_{3}}{a_{33}}, \frac{h_3}{a_{32}} > \frac{h_{2}}{a_{22}}$ and $\frac{h_1}{a_{12}} < \frac{a_{23}h_3-a_{33}h_2}{a_{23}a_{32}-a_{22}a_{33}}$, then $E_{1}$ is an asymptotically stable fixed point of $S$.

${\rm(ii)}$ If $\frac{h_2}{a_{23}} < \frac{h_{3}}{a_{33}}, \frac{h_3}{a_{32}} < \frac{h_{2}}{a_{22}}$ and $\frac{h_1}{a_{12}} < \frac{a_{23}h_3-a_{33}h_2}{a_{23}a_{32}-a_{22}a_{33}}$, then $E_{1}$ is a saddle point with two-dimensional stable manifold.

${\rm(iii)}$ If $\frac{h_2}{a_{23}} < \frac{h_{3}}{a_{33}}, \frac{h_3}{a_{32}} < \frac{h_{2}}{a_{22}}$ and $\frac{h_1}{a_{12}} > \frac{a_{23}h_3-a_{33}h_2}{a_{23}a_{32}-a_{22}a_{33}}$, then $E_{1}$ is a saddle point with one-dimensional stable manifold.

Assume that $S$ has a positive fixed point, say $P: = (\tilde{x}_1, \tilde{x}_2, \tilde{x}_3)$, we will discuss the dynamics of $P$ in the following. For simplicity, we write

Lemma 3.8. (Stability of the positive fixed point $P$)

${\rm(i)}$ If $\det \bar{A} < 0$, then $P$ is an asymptotically stable fixed point of $S$.

${\rm(ii)}$ If $\det \bar{A} > 0$, then $P$ is an unstable fixed point of $S$.

Proof. By the expression of $L$, it follows that

Define $Q_t(LP): = \big(\tilde{u}_1(t), \tilde{u}_2(t), \tilde{u}_3(t)\big) = \tilde{u}(t)$, and $V(t, x): = D_x \tilde{u}(t).$ Note that

the matrix function $V(t) = V(t, LP)$ satisfies

Let $w(t) = P(t)V(t)$, where $P(t) = {\rm diag} \{\frac{1}{\tilde{u}_1(t)}, \frac{1}{\tilde{u}_2(t)}, \frac{1}{\tilde{u}_3(t)} \}.$ Then, one has

where

Let $W(t)$ be the monodromy matrix of the above Eq (3.5), then $W(t)$ satisfies

and hence $W(t) = P(t)V(t)P^{-1}(0)$. Thus $V(t) = P^{-1}(t)W(t)P(0)$. In view of

and

we have

Then,

and hence, $DS(P)\sim W(\varphi\omega)$, that is, $r(DS(P)) = r(W(\varphi\omega))$.

Let

then

where

Note that $Z(\varphi\omega)\sim W(\varphi\omega)$ implies that $DS(P)\sim Z(\varphi\omega)$, then,

Since the matrix $\tilde{A}(t)$ is cooperative and irreducible, it follows that $Z(t) > Z(0)$ for each $t > 0$, and then $Z(t)$ is a positive matrix (see [25, Theorem B.3]). By Perron-Frobenius theorem, $\rho_3: = r(Z(\varphi\omega))$ is a simple eigenvalue of $Z(\varphi\omega)$ with a positive eigenvector $e = (e_1, e_2, e_3)^T$. If $\rho_1$ and $\rho_2$ represent the other two eigenvalues of $Z(\varphi\omega)$, then $|\rho_i| < \rho_3$, $i = 1, 2$. By Liouville's formula, we also obtain that $0 < \rho_1\rho_2\rho_3 = \det Z(\varphi\omega) = e^{\int_0^{\varphi\omega}trace(\tilde{A}(s))ds} < 1$.

Let $z(t): = Z(t)e = (z_1(t), z_2(t), z_3(t))$, then $z(\varphi\omega) = Z(\varphi\omega)e = \rho_3(e_1, e_2, e_3)$ and $z(0) = Z(0)e = (e_1, e_2, e_3) > 0$. Noticing that $z(t)$ satisfies the equation

it follows that

Using the method of elimination, we have

Integrating the above equation for $t$ from $0$ to $\varphi\omega$, we then obtain

Note that

it follows that

Based on the fact that $\int_0^{\varphi\omega}\tilde{u}_2(t)z_2(t)dt > 0$, we have

${\rm(i)}$ If $\det \bar{A} > 0$, then $\rho_3 > 1$.

${\rm(ii)}$ If $\det \bar{A} < 0$, then $\rho_3 < 1.$

This implies that the proof is completed.

4.   Global stability

To obtain the existence and uniqueness of the positive fixed point for the Poincaré map $S$, we first give a lemma with respect to the index of fixed points. For the reader's convenience, we recall some known results on the fixed point index of a continuous map (see Amann ^[19] for a more detailed discussion).

Let $U \subseteq \mathbb{R}_+^n$ be open and $S: U\rightarrow \mathbb{R}_+^n$ be a continuous map such that Fix$(S, U)$ is compact, where Fix$(S, U)$ is defined by the set of all fixed points of $S$ in the subset $U$. The fixed point index of $S$ is denoted by

where $id$ is the identity map and $\deg(id-S, U, 0)$ is the Brouwer degree for $id-S$. The fixed point index of $S$ at an isolated fixed point $\theta \in U$ is defined by

where $B_\delta(\theta): = \{x\in \mathbb{R}^n: \|x-\theta\| < \delta\}$ is an open ball in $U$ such that Fix$(S, B_\delta(\theta)) = \{\theta\}$. In particular, if $S$ is differentiable at $\theta \in$ Fix$(S, U)$ and $1$ is not an eigenvalue of $DS(\theta)$, then

where $\beta$ is the sum of the multiplicities of all the eigenvalues of $DS(\theta)$ which are greater than one. When $S$ has only finitely many fixed points in $U$, one has

Lemma 4.1. Assume that each fixed point $\theta$ of the Poincaré map $S$ deduced by system (1.2) is hyperbolic, then

${\rm(i)}$ $i(S, \mathbb{R}^3_+, \mathbb{R}^3_+) = 1$;

${\rm(ii)}$ If $DS(\theta)$ has no eigenvalue whose modulus is larger than 1, then $i(S, \theta) = 1$;

${\rm(iii)}$ If $\theta \in {{\rm{Int}}} H_L^+$, $L\neq \Lambda = \{1, 2, 3\}$, then $DS(\theta)$ has at least one eigenvalue with modulus larger than $1$ $\Leftrightarrow$ $i(S, \theta) = 0$.

Proof. (ⅰ) Since $\mathbb{R}^3_+$ is nonempty closed convex set, it follows from Dugundjis theorem that $i(S, \mathbb{R}^3_+, \mathbb{R}^3_+)$ is well-defined. Let $x_0 \in \mathbb{R}^3_+$ be arbitrary and define a compact map

Then $g$ maps the product space into $\mathbb{R}^3_+$ and has no fixed points on the boundary of $\mathbb{R}^3_+$ (relative to $\mathbb{R}^3_+$) because this boundary is empty. By the homotopy invariance and the normalization property, we have

(ⅱ), (ⅲ) See the proof of Lemma 4.2 for $n = 3$ and $U = \mathbb{R}^3_+$ in Liang and Jiang ^[20].

Arguing as the proof of Theorem 2.3 in Niu et al. ^[12], it can easily be proved that the Poincaré map $S$ also has a carrying simplex.

Lemma 4.2. (The existence of the carrying simplex) Assume that $h_i > 0, i = 1, 2, 3$, then the Poincaré map $S$ admits a carrying simplex $\Sigma$ which attracts every nontrivial orbit in $\mathbb{R}_+^3$.

Proof. See the proof of Theorem 2.3 for $n = 3$ in Niu et al. ^[12].

For each coordinate plane, we write $\Pi_i = \{x\in \mathbb{R}^3_+ :x_i = 0\}, i = 1, 2, 3$. We denote by $S |_{\Pi_i}$ the restriction of $S$ to $\Pi_i$. To obtain the global stability of the positive fixed point, we also need the following lemma which is a special version of Theorem 2.4 in Balreira et al. ^[21] for maps defined on $\mathbb{R}^3_+$.

Lemma 4.3. ([21, Theorem 2.4]) Consider the map $S = (x_{1} G_{1}(x), x_{2} G_{2}(x), x_{3} G_{3}(x))$ defined on $\mathbb{R}_+^3$ with $G_i(x)\geq 0$, $i = 1, 2, 3$, which has a carrying simplex. Assume that

${\rm(a)}$ $\det DS(x) > 0$ for all $x\in \mathbb{R}^3_+$;

${\rm(b)}$ $DS(x)^{-1} > 0$ for all $x\in {{\rm{Int}} } \mathbb{R}^3_+$;

${\rm(c)}$ for each $i = 1, 2, 3$, $S|_{\Pi_i}$ has a unique interior fixed point $E_{\{i\}}$ that is globally asymptotically stable in ${\rm{Int}}$$\Pi_i$, but a saddle for $S$;

${\rm(d)}$ $S$ has a unique positive fixed point $p\in {{\rm{Int}} } \mathbb{R}^3_+$.

Then $P$ is globally asymptotically stable in ${{\rm{Int}}}\mathbb{R}^3_+$ for $S$.

Next, we will analyze the global dynamics of system (1.2). Applying Lemma 4.1 to Lemma 3.1-3.7, it is not difficult to calculate the index of each of axial and planar fixed points. If $S$ has a positive fixed point, it follows from Lemma 3.8 that $i(S, P) = 1$ under the condition $\det \bar{A}<0$. By using the index of each boundary fixed point and $i(S, \mathbb{R}^3_+, \mathbb{R}^3_+) = 1$, we can choose appropriate parameter values to ascertain whether the positive fixed point exists. We can further obtain the global dynamics for system (1.2).

Theorem 4.4. (Three species Coexistence) Suppose that system (1.2) satisfies

${\rm(i)}$ $h_i>0 \ (i = 1, 2, 3), \ \det \bar{A}<0;$

${\rm(ii)}$ $\frac{h_1}{a_{12}}>\frac{h_2}{a_{22}};\ \frac{h_3}{a_{32}}>\frac{h_2}{a_{22}};\ h_2>\frac{a_{21}}{a_{11}}h_1+\frac{a_{23}}{a_{33}}h_3;$

${\rm(iii)}$ $h_1(a_{22}a_{33}-a_{23}a_{32})>a_{12}(a_{33}h_2-a_{23}h_3);$

${\rm(iv)}$ $h_3(a_{11}a_{22}-a_{12}a_{21})>a_{32}(a_{11}h_2-a_{21}h_1);$

then there admits a unique positive fixed point, say $P = (\tilde{x}_1, \tilde{x}_2, \tilde{x}_3)$, for the Poincaré map $S$. Moreover, $P$ is globally asymptotically stable in ${\rm{Int}} \mathbb{R}_+^3$ .

Proof. By Lemma 2.1, $S|_{H_i^+}$ has a unique interior fixed point $R_i$ that is globally asymptotically stable in ${\rm{Int}} H_{i}^+$ for each $i = 1, 2, 3$ because $h_i>0$. And yet, $R_i$ is a saddle point for $S$ due to the assumption (ⅱ) and Lemmas 3.2–3.4. Under the assumptions (ⅱ)–(ⅳ), by Lemmas 3.5–3.7 and Theorem 3.6 in ^[12], $S|_{\Pi_i}$ has a unique interior fixed point $E_{\{i\}}$ for $i = 1, 2, 3$ that is globally asymptotically stable in ${\rm{Int}}$$\Pi_i$, but a saddle point for $S$. Using Lemma 4.1(ⅲ), one has

Since $i(S, \mathbb{R}^3_+, \mathbb{R}^3_+) = 1$ (Lemma 4.1(ⅰ)), it is easy to see that there exists at least a positive fixed point for $S$, say $P$. Note that $\det \bar{A} < 0$ and Lemma 3.8(ⅰ), so $P$ is an asymptotically stable fixed point for $S$. Lemma 4.1(ⅱ) implies that $i(S, P) = 1$. If there also exists another positive fixed point, say $P^*$, that is, $P^*\neq P$, then $P^*$ is also asymptotically stable, and hence $i(S, P^*) = 1$, which contradicts the fact that $i(S, \mathbb{R}^3_+, \mathbb{R}^3_+) = 1$. Consequently, the positive fixed point $P$ is unique. By the expression (3.1) of $DS(x)$ and the Eq (3.3), we can obtain that for any $x\in \mathbb{R}_+^3$,

Besides, it follows from the proof of Theorem 2.3 in ^[12] that $DS(x)^{-1} > 0, \ \ \forall \ x\in {{\rm{Int}} } \mathbb{R}^3_+$. So far, the conditions (a), (b), (c) and (d) of Lemma 4.3 are satisfied for $S$, which implies that $P$ is globally asymptotically stable in ${{\rm{Int}}}\mathbb{R}^3_+$. We have completed the proof.

Theorem 4.5. (Two species Coexistence) Suppose that system (1.2) satisfies

${\rm(i)}$ $h_i > 0 \ (i = 1, 2, 3), \ \det \bar{A} < 0;$

${\rm(ii)}$ $\frac{h_2}{a_{21}} < \frac{h_1}{a_{11}}; \ \frac{h_2}{a_{23}} < \frac{h_3}{a_{33}}; \ \frac{h_1}{a_{12}} > \frac{h_2}{a_{22}}; \ \frac{h_3}{a_{32}} > \frac{h_2}{a_{22}}; h_2 < \frac{a_{21}}{a_{11}}h_1+\frac{a_{23}}{a_{33}}h_3;$

then there is no positive fixed point for the Poincaré map $S$. Moreover, the planar fixed point $E_2$ is globally asymptotically stable in ${\rm{Int}}\mathbb{R}_+^3$.

Proof. By Lemma 2.1 and Theorem 3.6 in ^[12], there exist three axial fixed points $R_i \ (i = 1, 2, 3)$ and one planar fixed point $E_2$ under the assumptions (ⅰ) and (ⅱ). Moreover, $R_i$ is a saddle point for $S$ and $E_2$ is an asymptotically stable fixed point of $S$. In view of Lemma 4.1(ⅱ) and (ⅲ), one has

If there exists a positive fixed point for $S$, say $P$, it follows from $\det \bar{A} < 0$ and Lemma 3.8(i) that $P$ is an asymptotically stable fixed point of $S$. Lemma 4.1(ⅱ) implies that $i(S, P) = 1$. This contradicts the fact that $i(S, \mathbb{R}^3_+, \mathbb{R}^3_+) = 1$. So there is no positive fixed point for $S$. Lemma 4.2 states that the Poincaré map $S$ has a carrying simplex $\Sigma$ by which is homeomorphic to the probability simplex $\Delta^2$. We further get that $\Sigma$ is a topological disk and $S|_{\Sigma}$ is an orientation preserving homeomorphism from $\Sigma$ onto $\Sigma$ (see ^[22]). By Corollary 2.1 in ^[23], every trajectory on $\Sigma$ converges to some fixed point. In particular, $R_1$ and $R_3$ are saddle points, $R_2$ is an unstable node point, while $E_2$ is an asymptotically stable node point on $\Sigma$. Since $S|_{\Sigma}$ is an orientation preserving homeomorphism, every trajectory on $\Sigma$ converges to $E_2$. By using the property ($P_3$) of the carrying simplex, one has

Thus, $E_2$ is globally asymptotically stable in ${\rm{Int}} \mathbb{R}_+^3$. The proof is completed.

By suitable modification to the proof of Theorem 4.5, we can also obtain the following Theorems 4.6 and 4.7.

Theorem 4.6. (Two species Coexistence) Suppose that system (1.2) satisfies

${\rm(i)}$ $h_i>0 \ (i = 1, 2, 3), \ \det \bar{A}<0;$

${\rm(ii)}$ $\frac{h_3}{a_{32}}<\frac{h_2}{a_{22}};\ \frac{h_1}{a_{12}}>\frac{h_2}{a_{22}}; \ h_2>\frac{a_{21}}{a_{11}}h_1+\frac{a_{23}}{a_{33}}h_3;$

${\rm(iii)}$ $h_3(a_{11}a_{22}-a_{12}a_{21})<a_{32}(a_{11}h_2-a_{21}h_1);$

then there is no positive fixed point for the Poincaré map $S$. Moreover, the planar fixed point $E_3$ is globally asymptotically stable in ${\rm{Int}} \mathbb{R}_+^3$.

Theorem 4.7. (Two species Extinction) Suppose that system (1.2) satisfies

${\rm(i)}$ $h_i>0 \ (i = 1, 2, 3), \ \det \bar{A}<0;$

${\rm(ii)}$ $\frac{h_1}{a_{12}}<\frac{h_2}{a_{22}};\ \frac{h_3}{a_{32}}<\frac{h_2}{a_{22}}; \ h_2>\frac{a_{21}}{a_{11}}h_1+\frac{a_{23}}{a_{33}}h_3;$

then there is no positive fixed point for the Poincaré map $S$. Moreover, the axial fixed point $R_2$ is globally asymptotically stable in ${\rm{Int}} \mathbb{R}_+^3$.

Remark 4.1. By using our analytical approach in Theorems 4.5–4.7, we also take different sufficient conditions to obtain the global dynamics of system (1.2).

Next, we will investigate the global extinction for system (1.2). For simplicity, we introduce the following notations

Lemma 4.8. If $S$ has a positive fixed point, say $P$, then $y: = \int_0^{\varphi\omega}Q_t(LP)dt$ is a positive solution of the linear algebraic system $\tilde{A}y = \tilde{B}$. In other words, if the linear system $\tilde{A}y = \tilde{B}$ has no positive solution, then $S$ has no positive fixed point in ${\rm{Int}} \mathbb{R}_+^3$.

Proof. We write the positive fixed point $P: = (\tilde{x}_1, \tilde{x}_2, \tilde{x}_3)$ as Lemma 3.8. Let $Q_t(LP) = u(t, LP): = \big(\tilde{u}_1(t), \tilde{u}_2(t), \tilde{u}_3(t)\big)$, then $\big(\tilde{u}_1(t), \tilde{u}_2(t), \tilde{u}_3(t)\big)$ satisfies the following equations

Integrating the above equations of (4.1) for $t$ from $0$ to $\varphi\omega$, it follows that

Note that $y_i = \int_0^{\varphi\omega}\tilde{u}_i(t)dt$ and $h_i = \big(r_i\varphi-\lambda_i(1-\varphi)\big)\omega, i = 1, 2, 3$, we have

From the above equations, it is clear that $\tilde{A}y = \tilde{B}$ with $y_i > 0 \ (i = 1, 2, 3)$. Therefore, the conclusion is immediate. The proof is completed.

Theorem 4.9. (Global Extinction) Suppose that $h_i < 0$ $(i = 1, 2, 3)$. Then the trivial fixed point $O$ is globally asymptotically stable in $\mathbb{R}_+^3$.

Proof. Since $h_i < 0 \ (i = 1, 2, 3)$, it follows that $S$ has no interior fixed points on the coordinate axes of $\mathbb{R}_+^3$ due to Lemma 2.1(i). By appealing to Lemma 2.4 in ^[11], there are also no interior fixed points on the coordinate plane of $\mathbb{R}_+^3$. Lemma 4.8 implies that there is no positive fixed point in Int$\mathbb{R}_+^3$ for $S$. Therefore, $O$ is a unique fixed point for $S$ in $\mathbb{R}_+^3$. By the equations of system (1.4), one has

Since $h_i < 0$, by differential inequality theorem and Lemma 2.1(ⅰ), it follows that

Note that Lemma 3.1(ⅰ), $O$ is an asymptotically stable fixed point of $S$, and hence, $O$ is globally asymptotically stable in $\mathbb{R}_+^3$. We have completed the proof.

5.   Numerical simulation

In this section, we provide some numerical examples to illustrate our analytic results.

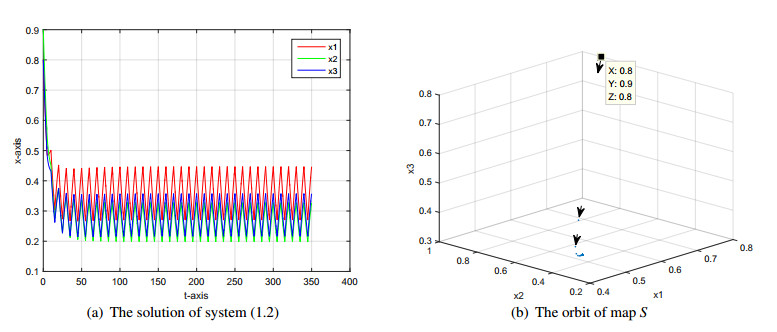
Example 1. (Three species Coexistence) Taking parameter values $\omega = 10$, $\phi = 0.5$, $\lambda_1 = 0.1$, $\lambda_2 = 0.1$, $\lambda_3 = 0.1$, $r_1 = 0.3$, $r_2 = 0.3$, $r_3 = 0.3$, $a_{11} = 0.4$, $a_{12} = 0.2$, $a_{21} = 0.1$, $a_{22} = 0.5$, $a_{23} = 0.1$, $a_{32} = 0.2$, $a_{33} = 0.5$, and initial values $x^0 = (0.8, 0.9, 0.8)$, system (1.2) satisfies the conditions of Theorem 4.4. The numerical simulations for the solution of system (1.2) and the orbit of the Poincaré map $S$ are shown in Figure 1, which imply that three species will be coexistence.

Example 2. (Two species coexistence) Taking parameter values $\omega = 10$, $\phi = 0.5$, $\lambda_1 = 0.1$, $\lambda_2 = 0.1$, $\lambda_3 = 0.1$, $r_1 = 0.3$, $r_2 = 0.3$, $r_3 = 0.3$, $a_{11} = 0.2$, $a_{12} = 0.2$, $a_{21} = 0.3$, $a_{22} = 1$, $a_{23} = 0.22$, $a_{32} = 0.1$, $a_{33} = 0.2$, and initial values $x^0 = (0.8, 0.9, 0.8)$, system (1.2) satisfies the conditions of Theorem 4.5. The numerical simulations for the solution of system (1.2) and the orbit of the Poincaré map $S$ are shown in Figure 2, which imply that Species 1 and Species 3 will be coexistence, and Species 2 will go to extinction.

Example 3. (Two species coexistence) Taking parameter values $\omega = 10$, $\phi = 0.5$, $\lambda_1 = 0.1$, $\lambda_2 = 0.1$, $\lambda_3 = 0.1$, $r_1 = 0.3$, $r_2 = 0.3$, $r_3 = 0.3$, $a_{11} = 0.6$, $a_{12} = 0.1$, $a_{21} = 0.1$, $a_{22} = 0.2$, $a_{23} = 0.2$, $a_{32} = 0.3$, $a_{33} = 0.5$, and initial values $x^0 = (0.8, 0.9, 0.8)$, system (1.2) satisfies the conditions of Theorem 4.6. The numerical simulations for the solution of system (1.2) and the orbit of the Poincaré map $S$ are shown in Figure 3, which imply that Species 1 and 2 will be coexistence, and Species 3 will go to extinction.

Example 4. (Two species extinction) Taking parameter values $\omega = 10$, $\phi = 0.5$, $\lambda_1 = 0.1$, $\lambda_2 = 0.1$, $\lambda_3 = 0.1$, $r_1 = 0.3$, $r_2 = 0.3$, $r_3 = 0.3$, $a_{11} = 0.6$, $a_{12} = 0.3$, $a_{21} = 0.1$, $a_{22} = 0.23$, $a_{23} = 0.2$, $a_{32} = 0.25$, $a_{33} = 0.5$, and initial values $x^0 = (0.8, 0.9, 0.8)$, system (1.2) satisfies the conditions of Theorem 4.7. The numerical simulations for the solution of system (1.2) and the orbit of the Poincaré map $S$ are shown in Figure 4, which imply that Species 2 will win the competition and Species 1 and Species 3 will go to extinction.

Example 5. (Global extinction) Taking parameter values $\omega = 10$, $\phi = 0.5$, $\lambda_1 = 0.3$, $\lambda_2 = 0.3$, $\lambda_3 = 0.3$, $r_1 = 0.1$, $r_2 = 0.1$, $r_3 = 0.1$, $a_{11} = 0.6$, $a_{12} = 0.1$, $a_{21} = 0.1$, $a_{22} = 0.2$, $a_{23} = 0.2$, $a_{32} = 0.3$, $a_{33} = 0.5$, and initial values $x^0 = (0.8, 0.9, 0.8)$, system (1.2) satisfies the conditions of Theorem 4.9. The numerical simulations for the solution of system (1.2) and the orbit of the Poincaré map $S$ are shown in Figure 5, which imply that three species will go to extinction.

6.   Discussion

In this paper, we focus on a tridiagonal three-species competition model with time $\omega$-periodic coefficients (called Seasonal Succession). By the stability analysis of equilibria, we estimate the Floquet multipliers of all nonnegative periodic solutions of system (1.2), and get the local dynamics of these periodic solutions. By using the Brouwer degree theory, we present an index result of the fixed points for the Poincaré map $S$. Based on this, we can verify the existence and uniqueness of the positive fixed point under appropriate conditions. Sufficient conditions of the global stability for coexistence and extinction of system (1.2) are provided via the local dynamics of all nonnegative fixed points. More precisely, three species will be existence under the assumptions of Theorem 4.4; Species 1 and 3 will be coexistence and Species 2 will go to extinction in the competition under the assumptions of Theorem 4.5; Species 1 and 2 will be coexistence and Species 3 will go to extinction in the competition under the assumptions of Theorem 4.6; Species 2 will win the competition and Species 1 and 3 will go to extinction under the assumptions of Theorem 4.7; three species will go to extinction under the assumptions of Theorem 4.9. From above analytic results, it is not difficult to see that the introduction of seasonal succession may lead to species' extinction.

On the other hand, there is no explicit expression of the Poincaré map $S$ for the time-periodic differential equations, even for the simplest form as system (1.2). This makes the researches on the dynamics the Poincaré map of the time-periodic Kolmogorov competitive systems become much more difficult and complicated. In future work, we will try to give a complete classification for the dynamics of system (1.2) and explore the influence of parameter values $\varphi$ and $\lambda_i, i = 1, 2, 3$ related to the seasonal succession on the dynamics of the system (1.2).

Acknowledgments

We are very grateful to the anonymous referees for their valuable comments and suggestions, which led to an improvement of our original manuscript. This work was supported by the National Natural Science Foundation of China (No.11871231) and Natural Science Foundation Fund of Fujian Province (No.2022J01305).

Conflict of interest

The authors declare there is no conflict of interest.