Dynamical analysis of an aquatic amensalism model with non-selective harvesting and Allee effect

1.   Introduction

Algae and some algicidal bacteria can constitute a unique micro-ecological environment system, and their interaction is similar to symbiosis ^[1]. However, some algicidal bacteria can release specific or non-specific extracellular substances to inhibit algae or kill algal cells, especially which can produce antibiotics to inhibit the growth of algae population. Thus, the relationship between algae and algicidal bacteria is similar to amensalism. Dakhama et al. ^[2] pointed out that Pseudomonas aeruginosa could produce a lot of antibiotics to inhibit the growth of other bacteria and algae. Kawano et al. ^[3] confirmed that bacterial strain pk654 could produce antibiotic thiotropocin to inhibit Skeletonema medialis and Heterosigma. Xiao et al. ^[4] concluded that the extracellular metabolites of actinomycete AN02 had a good inhibitory effect on Microcystis aeruginosa. Choi et al. ^[5] pointed out that the growth of Microcystis could be well inhibited by Streptomyces neogawaensis. Thus, it is obvious that because harmful algal blooms have become one of the most urgent ecological and environmental problems in the world, the algal-bacterial interaction will pose the possible of using some algicidal bacteria for biological control of algal blooms ^[6]. Although a large number of literatures have studied dynamic relationship between algae and bacteria, and some excellent research results have also been given, it is a pity that the lysing dynamic process and characteristics of algicidal bacteria are still unclear. Therefore, it is necessary to find a new way to better understand the dynamic mechanism between algae and algicidal bacteria. However, as far as we are concerned, it is possible to seek a relatively new way to reveal the dynamic inhibition mechanism of algicidal bacteria on algae by using mathematical models and dynamic analysis technology.

In natural ecosystems, the amensalism relationship is one of the symbiotic relationships between two kinds of organisms ^[7]. When two kinds of organisms live together, one of them does not get benefits, the other species is negatively by the presence of the first species, but it doesn't have to die. Even if a individual experiences death, the negatively-impacted species will not die. At the same time, in the amensalism relationship, one organism can inhibit and harm another organism, even kill each other, but it does not directly benefit or harm itself. For example, plants in the walnut family can secrete juglone to inhibit the growth of other plants nearby, and penicillin produced by Penicillium can inhibit Gram-positive bacteria ^[8].

In recent ten years, the research on amensalism relationship and population dynamic model has achieved many excellent results. Sun ^[9] firstly proposed the following population amensalism model:

where ${r_1}$ and ${r_2}$ are respectively the intrinsic growth rate of two species, ${k_1}$ and ${k_2}$ are respectively the environmental carrying capacity of two species, $T$ represents the coefficient of effect single $y$ on $x$. The author investigated the stability of all equilibrium points of this model (1.1). Based on the model (1.1), two population amensalism Lotka-Volterra model were proposed to investigate the permanence and extinction ^[10,11], and discuss the stability using the characteristic theory ^[12]. Wu ^[13] constructed a two species amensalism model with non-monotonic functional response argued by using the linear functional response to depict the influence of the second species to the first species, which can be depicted as follows:

where ${a_1}, {a_2}, {b_1}, {b_2}, c, d$ are all positive constants. He studied the global dynamics of all the possible equilibrium points of the model (1.2). Guan and Chen ^[14] proposed a two species amensalism model with Beddington-DeAngelis functional response and Allee effect on the second species, which can be described as follows:

where $\frac{y}{{u + y}}$ represents the Allee effect, and $u$ is a positive constant, which can describe the intense of the Allee effect. They discussed the global dynamics of all the possible equilibrium points of the model (1.3), and found that the Allee effect had no influence on the final density of the species, but could cause the trajectories of the model (1.3) approaching the equilibrium solution more slowly. Drawing lessons from the model (1.2) and (1.3), a large number of amensalism ecological models have been constructed and studied, such as: a three-species model with partial harm relations^[15], a Holling type commensal symbiosis model involving Allee effect^[16], a non-selective harvesting Lotka-Volterra amensalism discrete model^[17], a non-autonomous Lotka-Volterra amensalism model^[18], and an amensalism model with weak Allee effect^[19]. However, as far as we know, there is little research on aquatic amensalism model based on the inhibition mechanism of algicidal bacteria on algae.

Courchamp et al. ^[20] pointed out that the survival and reproduction of algicidal bacteria had a certain Allee effect, hence, the Allee effect must be taken into account in the process of establishing an aquatic amensalism model. Allee effect was first proposed by ecologist Allee in 1931 ^[21], since then, Stephens and other researchers summarized the previous research results and gave a clear definition of Allee effect. This definition was the positive correlation between the number or population density of the same species and any aspect of individual fitness ^[22,23,24]. Furthermore, such relationship was also known as inverse density dependence ^[23,24], depensation ^[25] and positive density dependence ^[26,27]. At the same time, as more and more scholars have paid attention to Allee effect in some mathematical biological models, and given a large number of excellent research results in the papers ^{[28,29,30,31,32]}. Rebelo and Soresina ^[28] gave some general conditions for coexistence of prey and predator in the case of weak and strong Allee effect. Kumar et al. ^[29] concluded that Allee effect could generate or destroy the interior attractor. Sen et al. ^[30] pointed out that increasing value of Allee parameter could turn the system more structurally stable. Bai et al. ^[31] pointed out that strong Allee effect in the basal prey could increase the extinction risk of not only the basal prey but also the intraguild prey. Tripathi et al. ^[32] found that the introduction of Allee effect could induce more rich dynamics and compel the system to be more sensitive to initial population densities. Obviously, Allee effect has some serious effects on the dynamic characteristics of ecological population. In addition, with more and more examples of Allee effect in the ecological nature, ecologists and biological mathematicians will pay more and more attention to the research of Allee effect, and it is possible that more and more excellent research results will be published in the near future.

During the experiment of algicidal bacteria inhibiting growth dynamics of algae population, in order to ensure the sustainability of this experiment, it is necessary to consider an ecological harvest in this aquatic amensalism model, which is a favorable economic tool to realize the high unity and sustainable development of economic take-off and environmental protection ^[33]. In ecological model, harvest is one of critical factors, which can grab the attention among researchers due to its importance in resource management and sustainable development from the biological and economic standpoints ^[34]. Therefore, a large number of excellent research results have been given in these papers. Ang et al. ^[34] derived some optimal thresholds for predator harvesting to give maximum financial profit for sustaining the fishery resources. Clark ^[35] systematically and orderly investigated harvesting behaviors of natural populations. Clark et al. ^[36] proposed mathematical models of exploited fish stocks through a dynamic aggregation process. Das et al. ^[37] investigated a prey-predator fishery model to explore the optimal harvesting policy. Das et al. ^[38] solved the problem of determining the optimal harvesting policy of a predator-prey model. Liu and Huang ^[39] inquired into a series of harvesting problems of a harvested predator-prey model. Bellier et al. ^[40] demonstrated that two different harvesting strategies were needed to optimize the annual yield of predators and prey. Datta et al. ^[41] found that the age-selective harvesting system could undergo a Hopf bifurcation. Li et al. ^[42] discussed stability and bifurcations of a bio-economic differential algebraic predator-prey model. Gupta et al. ^[43] investigated numerous kinds of bifurcations of a predator-prey model with nonlinear predator harvesting. Guin et al. ^[44] studied the effect of harvesting on the control of spatial pattern formation. Guin et al. ^[45] pointed out that harvesting effort could play a vital function for geological conservation. Guin and Acharya ^[46] proposed that the effect of harvesting could play a significant role on the control of spatial pattern formation. Thus, it can be seen from the above that harvesting behavior has an important impact on population dynamics. Moreover, with the circular development of ecological economics, we believe that more and more attention has been paid to ecological harvest in the implementation of ecological algae control strategy.

At present, it is useful to carry out ecological model to study the algae control behavior of algicidal bacteria. This is because that ecological model can form three basic of studying biological system, namely: trophic level analysis, system perspective and dynamic view ^[47,48], which can improve the understanding of the interactions between populations and their dependence on internal and external conditions ^[49,50]. Therefore, in recent decades, the research of ecological model has developed rapidly, and some excellent models have been obtained in the paper. Balram et al ^[51] proposed a two-dimensional prey-predator system, and found that the negative feedback delay and gestation delay could destabilize the behavior of the system. Guin et al. ^[52,53,54] investigated dynamics of predator-prey system, and achieved a series of important and meaningful results(Turing instability can be affected by nonlinear harvesting^[52], prey harvesting has a significant effect on the spatiotemporal pattern formations of predator-prey systems^[53], and prey harvesting can induce diffusion-driven instability resulting in stationary Turing patterns^[54]). Wang and Yu ^[55] obtained some sufficient and threshold conditions to guarantee the occurrence of saddle-node, pitchfork, and Hopf bifurcations. Li et al. ^[56] constructed a new aquatic ecological model to describe the aggregation effect of Microcystis aeruginosa, and investigated some critical threshold conditions through the discovery of transcritical bifurcation, saddle-node bifurcation, Hopf bifurcation and Bogdanov-Takens bifurcation. Han et al.^[57,58] investigated how fear effect and refuge to pattern formation in predator = prey model. However, facing more and more natural ecological environment problems, the research on ecological model and its related applications still needs to be strengthened and deepened. Based on the above overview, the main aim of the paper is to explore the inhibition mechanism of algicidal bacteria on algae by means of bifurcation dynamic behaviors of a new aquatic amensalism model. On this basis, we clarify biological meaning of transcritical bifurcation and saddle-node bifurcation from the aspect of algicidal bacteria controlling algae.

2.   Construction of ecological model

In order to describe the dynamic relationship between algae and algicidal bacteria (it can produce antibiotic) by using ecological mathematical modeling, some modeling assumptions are given as follows:

(1) The growth space of algicidal bacteria and algae is semi closed water environment, species concentration is always evenly distributed in space and changed instantaneously with time $t$, $x(t)$ and $y(t)$ represent respectively the density of algae and algicidal bacteria. Furthermore, we demand that algicidal bacteria can produce antibiotic to inhibit algal growth.

(2) The paper ^[59] pointed out that the growth curve of algicidal bacteria (Microbacterium oleivoran) is in line with logistic model, and then the survival and reproduction of algicidal bacteria have Allee effect ^[20]. Thus, we assume that the dynamic growth function of algicidal bacteria $y$ is $y({a_2} - {b_2}y)F(y)$ with intrinsic growth rate $a_{2}$ and maximum environmental capacity $\frac{{a_2}}{{b_2}}$ as well as the Allee effect $F(y) = \frac{y}{{u + y}}$, where $u$ is Allee effect constant, the larger the value of $u$ is, the stronger the Allee effect is. At the same time, we also suppose that the dynamic growth function of algae $x$ is $x({a_1} - {b_1}x)$ with intrinsic growth rate $a_{1}$ and maximum environmental capacity $\frac{{a_1}}{{b_1}}$, this is because that a large number of experiments have proved that logistic growth function is most consistent with the growth trend of algae in nature ^[60].

(3) In order to prevent algal blooms from breaking out or rotting later, the algae needs to be salvaged or harvested regularly. At the same time, because algicidal bacteria are attached to algae, the salvage or harvest of the algae will lead to the salvage or harvest of some algicidal bacteria. Hence, we presume that the harvesting functions of algae and algicidal bacteria are ${q_1}mEx$ and ${q_2}mEy$ respectively, where ${q_i}$ is the catchability co-efficient of the ith species, $E$ is the combined capture effort used to harvest, and $m(0 < m < 1)$ is the fraction of the stock available for harvesting.

(4) As everyone knows, the survival and reproduction of algicidal bacteria are dependent on a certain number of algae. Meanwhile, if the density of algicidal bacteria is relatively small, it needs to attract nutrients for rapid propagation. Thus algicidal bacteria can form a competitive relationship with algae, and also secrete a large number of antibiotics to inhibit the growth of algae. However, with the increase of algicidal bacteria density, the living space of algicidal bacteria will gradually reduce, and algicidal bacteria will form internal competition, which leads to the need for a large number of algae, and then the inhibition effect of algal growth will be weakened. In other words, the inhibition of algicidal bacteria on algae is a humped function and declines with the high densities of algicidal bacteria and internal competitive relationship. Thus, we give an inhibition function of algicidal bacteria on algal growth $\frac{{cxy}}{{d + {y^2}}}$ with the half saturation constant $d$ and the maximum value of the per capita reduction rate $c$.

Based on the above hypothesis and the inspiration of some works ^[9,13,14], we will propose an aquatic amensalism model with non-selective harvesting and Allee effect, which can be described by the following differential equation:

In this paper, we firstly give some sufficient conditions to guarantee the existence and stability of all possible equilibrium points of the model (2.1), which contain the survival mode of algae and algicidal bacteria, and analyze some critical conditions to ensure that the model (2.1) can occur saddle-node bifurcation and transcritical bifurcation, which mainly reflect the dynamic process of the survival and evolution of algae and algicidal bacteria. Secondly, the relevant dynamic simulation experiments of the model (2.1) are implemented to verify the feasibility of the theoretical results and reveal some specific dynamic behaviors, which can visually explain the interaction trend and dynamic evolution trend of algae and algicidal bacteria. Finally, based on the results of numerical simulation, we explore how Allee effect and harvest affect the intrinsic dynamics of the model (2.1), and analyze the biological significance of some particular bifurcation dynamics(saddle-node bifurcation and transcritical bifurcation.

3.   Results of mathematical analysis

3.1.   Equilibrium points and their stability

In this section, the existence and stability of all possible equilibrium points of the model (2.1) will be discussed carefully, this is because that equilibrium point is a special solution of the model (2.1), which has special ecosystem significance. In order to obtain all possible equilibrium points of the model (2.1), we consider the following equation:

All possible equilibrium points are now the points of intersection of these equations, thus it is easy to obtain that the model (2.1) has a trivial equilibrium point ${E_0}(0, 0)$ and algicidal bacteria extinction equilibrium point ${E_1}(\frac{{{a_1} - {q_1}mE}}{{{b_1}}}, 0)$ with ${a_1} - {q_1}mE > 0$. For other boundary and positive equilibrium of the model (2.1), we need consider the positive solutions of the following equations:

We suppose that $\Delta$ is denoted the discriminant of the second equation of (3.2) and express $\Delta$ in terms of $m$, i.e., $\Delta (m) = q_2^2{E^2}{m^2} - (2{a_2} + 4{b_2}u){q_2}mE + a_2^2,$ as well as ${m_1}$ and ${m_2}$ is some roots of $\Delta (m)$. Thus, we can easily calculate that

Since all the parameters are positive, then ${m_2} > {m_1} > 0$. Thus, we can acquire the following theorem.

Theorem 1. For algae population free equilibrium points:

(1) If $m > m_1$, then the model (2.1) has no algae population free equilibrium point.

(2) If $0 < m < m_1$, then the model (2.1) has two algae population free equilibrium points ${E_2}(0, y_1^*)$ and ${E_3}(0, y_2^*)$, where $y_{1, 2}^* = \frac{{{a_2} - {q_2}mE \pm \sqrt {{{({a_2} - {q_2}mE)}^2} - 4{b_2}{q_2}mEu} }}{{2{b_2}}}$.

(3) If $m = m_1$, then the model (2.1) has a unique algae population free equilibrium point ${E_4}(0, y_3^*)$, where $y_3^* = \frac{{{a_2} - {q_2}mE}}{{2{b_2}}}$.

Our method of proving this theorem is similar to Hu and Cao ^[61] (see Theorem 1). For the sake of brevity, here we omit it.

Theorem 2. For all interior equilibrium points, we have:

(1) If $m > m_1$ or $m = m_1$, ${q_1} \ge g(y_3^*)$ or $0 < m < m_1$, ${q_1} \ge \max \{ g(y_1^*), g(y_2^*)\}$, then the model (2.1) has no interior equilibrium point, where $g(y_1^*) = \frac{{{a_1}}}{{mE}} - \frac{{cy_1^*}}{{mE(d + y{{_1^*}^2})}}$, $g(y_2^*) = \frac{{{a_1}}}{{mE}} - \frac{{cy_2^*}}{{mE(d + y{{_2^*}^2})}}$, $g(y_3^*) = \frac{{{a_1}}}{{mE}} - \frac{{cy_3^*}}{{mE(d + y{{_3^*}^2})}}$.

(2) If $m = m_1$ and ${q_1} < g(y_3^*)$, then the model (2.1) has a unique interior equilibrium point $E_3^*(x_3^*, y_3^*)$, where $x_3^* = \frac{1}{{{b_1}}}({a_1} - {q_1}mE - \frac{{cy_3^*}}{{d + y{{_3^*}^2}}})$, $y_3^* = \frac{{{a_2} - {q_2}{m_1}E}}{{2{b_2}}}$.

(3) If $0 < m < m_1$, then:

(a) If ${q_1} < \min \{ g(y_1^*), g(y_2^*)\}$, then the model (2.1) has two distinct interior equilibrium points $E_1^*(x_1^*, y_1^*)$ and $E_2^*(x_2^*, y_2^*)$, where $x_{1, 2}^* = \frac{1}{{{b_1}}}({a_1} - {q_1}mE - \frac{{cy_{1, 2}^*}}{{d + y{{_{1, 2}^*}^2}}})$, $y_{1, 2}^* = \frac{{{a_2} - {q_2}mE \pm \sqrt {{{({a_2} - {q_2}mE)}^2} - 4{b_2}{q_2}mEu} }}{{2{b_2}}}$.

(b) If $g(y_1^*) > g(y_2^*)$, $g(y_2^*) \le {q_1} < g(y_1^*)$, then the model (2.1) has a unique interior equilibrium point $E_1^*(x_1^*, y_1^*)$, where $x_1^*$ and $y_1^*$ are the same as in case (a).

(c) If $g(y_1^*) < g(y_2^*)$, $g(y_1^*) \le {q_1} < g(y_2^*)$, then the model (2.1) has a unique interior equilibrium point $E_2^*(x_2^*, y_2^*)$, where $x_2^*$ and $y_2^*$ are the same as in case (a).

Note that this theorem follows immediately from Theorem 1 and some straightforward computation.

Theorem 3. For all positive parameters, the model (2.1) has a boundary equilibrium point $E_{0}$, if $0 < {q_1} < \frac{{{a_1}}}{{mE}}$, then the equilibrium point $E_{0}$ is a saddle; if ${q_1} > \frac{{{a_1}}}{{mE}}$, then the equilibrium point $E_{0}$ is a stable node; and if ${q_1} = \frac{{{a_1}}}{{mE}}$, then the equilibrium point $E_{0}$ is a saddle-node. Moreover, when $0 < {q_1} < \frac{{{a_1}}}{{mE}}$, the equilibrium point $E_{1}$ exists, and it is always a stable node.

Proof: The Jacobian matrix of the model (2.1) evaluated at the equilibrium point $E_0$ is given by

Clearly, if $0 < {q_1} < \frac{{{a_1}}}{{mE}}$, then the equilibrium point $E_{0}$ is a saddle; if ${q_1} > \frac{{{a_1}}}{{mE}}$, then the equilibrium point $E_{0}$ is a stable node; if ${q_1} = \frac{{{a_1}}}{{mE}}$, using the Theorem 7.1 in Chapter 2 in ^[62], we can obtain that the equilibrium point $E_{0}$ is a saddle-node. That is to say, two separators that can tend to the equilibrium point $E_{0}$ along the upside and underneath of the equilibrium point $E_{0}$ can separate the neighborhood of $E_{0}$ into two parts. One part is a parabolic sector, and the other part mainly consists two hyperbolic sectors. Moreover, here the parabolic sector is on the right halfplane because of $a_{m} > 0$.

When $0 < {q_1} < \frac{{{a_1}}}{{mE}}$, the equilibrium point $E_{1}$ exists. The Jacobian matrix of the model (2.1) at the equilibrium point $E_{1}$ is

It is easy to see that the equilibrium point $E_{1}$ is always a stable node. This completes the proof.

Theorem 4.

(1) Assume that $0 < m < m_1$ holds, then the model (2.1) has two boundary equilibrium points $E_{2}$ and $E_{3}$, then we have

(a) If ${q_1} < g(y_1^*)$, then the equilibrium point $E_2$ is a saddle; if ${q_1} > g(y_1^*)$, then the equilibrium point $E_2$ is a stable node; if ${q_1} = g(y_1^*)$, then the equilibrium point $E_2$ is an attracting saddle-node.

(b) If ${q_1} < g(y_2^*)$, then the equilibrium point $E_3$ is an unstable node; if ${q_1} > g(y_2^*)$, then the equilibrium point $E_3$ is a saddle; if ${q_1} = g(y_2^*)$, then the equilibrium point $E_3$ is a repelling saddle-node.

(2) Assume that $m = m_1$ and ${a_1} - {q_1}mE - \frac{{cy_3^*}}{{d + y{{_3^*}^2}}}\neq0$ hold, then the model (2.1) has a boundary equilibrium point $E_4$, which is a saddle-node, furthermore if ${q_1} < g(y_3^*)$ (or ${q_1} > g(y_3^*)$), then the equilibrium point $E_4$ is a repelling (attracting) saddle-node.

Proof: (1) The Jacobian matrix of the model (2.1) evaluated at the equilibrium point $E_2$ is given by

Apparently, if ${q_1} < g(y_1^*)$, then the equilibrium point $E_{2}$ is a saddle; if ${q_1} > g(y_1^*)$, then the equilibrium point $E_{2}$ is a stable node; if ${q_1} = g(y_1^*)$, we again utilize Theorem 7.1 in Chapter 2 in ^[62] to determinate the stability of the equilibrium point $E_{2}$, which is an attracting saddle-node.

The Jacobian matrix of the model (2.1) evaluated at the equilibrium point $E_3$ is given by

Similarly, if ${q_1} < g(y_2^*)$, the equilibrium point $E_{3}$ is an unstable node; if ${q_1} > g(y_2^*)$, the equilibrium point $E_{3}$ is a saddle; if ${q_1} = g(y_2^*)$, it is a repelling saddle-node.

(2) The Jacobian matrix of the model (2.1) evaluated at the equilibrium point $E_{4}$ is given by

It is easy to see that if ${a_1} - {q_1}mE- {\frac{{cy_3^*}}{{d + y{{_3^*}^2}}}}\neq0$, the equilibrium point $E_{4}$ is always a non-hyperbolic point, therefore according to the notations of Theorem 7.1 in Chapter 2 in ^[62], if ${q_1} < g(y_3^*)$, the equilibrium point $E_{4}$ is a repelling saddle-node, and if ${q_1} > g(y_3^*)$, then it is an attracting saddle-node. This completely ends the proof of Theorem 4.

Theorem 5.

(1) Assume that $0 < m < m_1$ and ${q_1} < g(y_1^*)$ hold, then the positive equilibrium point ${E_1^*}$ exists, which is always a stable node.

(2) Assume that $0 < m < m_1$ and ${q_1} < g(y_2^*)$ hold, then the positive equilibrium point ${E_2^*}$ exists, which is always a saddle.

(3) Assume that $m = m_1$ and ${q_1} < g(y_3^*)$ hold, then the model (2.1) has a unique positive equilibrium point ${E_3^*}$, which is an attracting saddle-node.

We know that Theorem 5 can be proved by the same analysis as Theorem 4 above, therefore the proof of this theorem is omitted for brevity.

Based on the above discussion, we can list all the possible cases, where the stability of all possible equilibrium points of the model (2.1) may occur in Table 1 (see Appendix). Furthermore, the stability of these equilibrium points is an important theoretical basis for the later study of bifurcation dynamics.

3.2.   Bifurcation analysis

In this section, we will try to discuss miscellaneous possible bifurcations of the model (2.1) and obtain some critical conditions for the occurrence of saddle-node bifurcation and transcritical bifurcation. The saddle-node bifurcation is a best known bifurcation dynamic mechanism, which can change the model (2.1) from an unstable mode to a coexistence mode of a local stable state and a local unstable state, and also make the equilibrium point type and dynamic behavior of the model (2.1) change essentially. The transcritical bifurcation is a special bifurcation dynamic mechanism, which can make the equilibrium point of the model (2.1) exchange only through the bifurcation point, and its shape does not change, but its stability has changed essentially. Thus, the saddle-node bifurcation and the transcritical bifurcation have special biological significance in the natural evolution of ecological population, which is worthy of our in-depth exploration.

3.2.1.   Saddle-node bifurcation

Here we will prove that the model (2.1) undergoes two saddle-node bifurcations, which can take place when $m$ satisfies $m = {m_{SN}} = \frac{{{a_2} + 2{b_2}u - 2\sqrt {{b_2}u({a_2} + {b_2}u)} }}{{{q_2}E}}$.

Theorem 6. When $m = {m_{SN}}$, then the model (2.1) undergoes a saddle-node bifurcation around the equilibrium point $E_{4}$, where $m$ is a bifurcation control parameter and ${m_{SN}} = {m_{1}} = \frac{{{a_2} + 2{b_2}u - 2\sqrt {{b_2}u({a_2} + {b_2}u)} }}{{{q_2}E}}$.

Proof: Based on Sotomayor's theorem ^[62,63], we can prove that the model (2.1) undergoes a saddle-node bifurcation at ${m_{SN}}$. From the previous analysis, we can easily verify that $Det[{J_{{E_4}}}] = 0$ when ${m_{SN}} = {m_{1}} = \frac{{{a_2} + 2{b_2}u - 2\sqrt {{b_2}u({a_2} + {b_2}u)} }}{{{q_2}E}}$, which means that ${J_{{E_4}}}$ has a zero eigenvalue ${\lambda _2} = 0$. Letting $V = {({v_1}, {v_2})^T}$, $W = {({w_1}, {w_2})^T}$ be the eigenvectors of ${J_{{E_4}}}$ and ${J_{E_4^T}}$ corresponding to the zero eigenvalue ${\lambda _2}$ respectively, then we can calculate

Moreover, we have

Using these expressions, we can get

Hence according to Sotomayor's theorem, we can acquire the occurrence of saddle-node bifurcation at the equilibrium point ${E_4}$ as the value of the parameter $m$ passes through the critical threshold $m = {m_{SN}}$.

Analogously, when ${q_1} < \min \{ g(y_1^*), g(y_2^*), g(y_3^*)\}$, we know that the model (2.1) has two distinct positive equilibrium points $E_1^*$ and $E_2^*$ if $0 < m < {m_1}$, and the equilibrium point ${E_1^*}$ can coincide with the equilibrium point ${E_2^*}$ if $m = {m_1}$ and ${q_1} < g(y_3^*)$, then they will vanish if $m > {m_1}$. Thus there is a chance of saddle-node bifurcation around the equilibrium point ${E_3^*}$ when $m = {m_{SN}}$. The following theorem will show the occurrence of saddle-node bifurcation around the equilibrium point ${E_3^*}$ in the model (2.1).

Theorem 7. When the value of some key parameters can satisfy $m = {m_{SN}}$ and ${q_1} < \min \{ g(y_1^*), g(y_2^*), g(y_3^*)\}$, then the model (2.1) undergoes a saddle-node bifurcation around the equilibrium point ${E_3^*}$.

Proof: We assume that ${q_1} < \min \{ g(y_1^*), g(y_2^*), g(y_3^*)\}$ holds, and also apply Sotomayor's theorem to prove that the model (2.1) undergoes a saddle-node bifurcation at ${m_{SN}}$ around the equilibrium point ${E_3^*}$. When ${m_{SN}} = {m_{1}} = \frac{{{a_2} + 2{b_2}u - 2\sqrt {{b_2}u({a_2} + {b_2}u)} }}{{{q_2}E}}$, it is easy to verify that $Det[{J_{{E_3^*}}}] = 0$ and ${J_{{E_3^*}}}$ has a zero eigenvalue ${\lambda _2} = 0$. Letting $V = {({v_1}, {v_2})^T}$, $W = {({w_1}, {w_2})^T}$ be the eigenvectors of ${J_{{E_3^*}}}$ and ${J_{{E_3^*}^T}}$ corresponding to the zero eigenvalue ${\lambda _2}$ respectively, then we can calculate

Moreover, we have

Using these expressions, we can get

Hence according to Sotomayor's theorem, we acquire the occurrence of saddle-node bifurcation at ${E_3^*}$ when the value of critical parameter $m$ passes through the critical threshold $m = {m_{SN}}$, which means that when the value of $m$ passes from one side of $m = {m_{SN}}$ to the other side, the number of interior equilibrium point of the model (2.1) changes from zero to two. This completely ends the proof.

3.2.2.   Transcritical bifurcation

From the above discussion, we can see that the boundary equilibrium ${E_0}$ may change its stability when the value of ${q_1}$ passes through the critical threshold ${q_1} = \frac{{{a_1}}}{{mE}}$, and the model (2.1) may possesses another boundary equilibrium ${E_1}$. Therefore, we have the following theorem.

Theorem 8. When ${q_1} = {q_{1TC1}}$, the model (2.1) undergoes a transcritical bifurcation around the equilibrium point ${E_0}$, where ${q_1}$ is a bifurcation control parameter and ${q_{1TC1}} = \frac{{{a_1}}}{{mE}}$.

Proof: We again use Sotomayor's theorem to prove that the model (2.1) undergoes a transcritical bifurcation at ${q_{1TC1}}$ around the equilibrium point ${E_0}$. We can easily verify that $Det[{J_{{E_0}}}] = 0$, when ${q_{1TC1}} = \frac{{{a_1}}}{{mE}}$, which means that $Det[{J_{{E_0}}}]$ has a zero eigenvalue ${\lambda _1} = 0$. Letting $V = {({v_1}, {v_2})^T}$, $W = {({w_1}, {w_2})^T}$ be the eigenvectors of ${J_{{E_0}}}$ and ${J_{{E_0}^T}}$ corresponding to the zero eigenvalue ${\lambda _1}$ respectively, then we can calculate

Moreover, we have

Using these expressions, we can get

Thus, using Sotomayor's theorem, we acquire the occurrence of transcritical bifurcation at the equilibrium point ${E_0}$ as the value of parameter ${q_1}$ passes through the critical threshold ${q_1} = {q_{1TC1}}$. This completely ends the proof.

Next, we discuss the existence of transcritical bifurcation at the equilibrium point ${E_2}$. Based on the previous demonstration, we can see that the number of interior equilibria will changes as the value of parameter ${q_1}$ varies. The interior equilibrium point ${E_1^*}$ can bifurcate from the equilibrium point ${E_2}$ when the value of ${q_1}$ passes through the critical threshold ${q_{1TC2}} = g({y_1^*})$. Thus, there is a chance of transcritical bifurcation around the equilibrium point ${E_2}$ and Theorem 9 can be obtained.

Theorem 9. If $0 < m < {m_1}$, when the parameters satisfy the qualification ${q_1} = {q_{1TC2}} = g({y_1^*})$, the model (2.1) undergoes a transcritical bifurcation around the equilibrium point ${E_2}$.

Proof: From the analysis of section 2, we can easily verify that when ${q_{1TC2}} = g({y_1^*})$, $Det[{J_{{E_2}}}] = 0$, which means that $Det[{J_{{E_2}}}]$ has a zero eigenvalue ${\lambda _1} = 0$. Letting $V = {({v_1}, {v_2})^T}$, $W = {({w_1}, {w_2})^T}$ be the eigenvectors of ${J_{{E_2}}}$ and ${J_{{E_2}^T}}$ corresponding to the zero eigenvalue ${\lambda _1}$ respectively, then we can count out

Moreover, we have

Using these expressions, we can get

Hence from Sotomayor's theorem, the model (2.1) undergoes a transcritical bifurcation around the equilibrium point ${E_2}$ as the value of parameter ${q_1}$ passes through the critical threshold ${q_1} = {q_{1TC2}}$. This completely ends the proof.

3.3.   Global dynamics

In this section, we will further investigate the global dynamics of the model (2.1), and give the following result.

3.3.1.   The dynamics near infinity

Now we discuss the qualitative properties of equilibrium point at infinity to study the global dynamics of the model (2.1), which can help us learn the behavior of orbits when either $x$ or $y$ is large. Firstly, we use the Poincar$\acute{e}$ transformation ^[62] $x = \frac{1}{z}$, $y = \frac{w}{z}$, to transform the model (2.1) into

where $ds = {{dt} / z}$. Then, it can see that, in the nonnegative w-axis, the model (5.1) has a unique equilibrium point $A(\frac{{{b_1}}}{{{b_2}}}, 0)$ in the first quadrant of $w-z$ plane.

Then by another Poincar$\acute{e}$ transformation $x = \frac{v}{z}$, $y = \frac{1}{z}$, the model (2.1) can be changed into

where $ds = {{dt} / z}$. Now, we just need to discuss the equilibrium point $B(0, 0)$ of the model (5.2) in the first quadrant of $v-z$ plane.

Next, we analysis the qualitative properties of $A(\frac{{{b_1}}}{{{b_2}}}, 0)$ of the model (5.1) and $B(0, 0)$ of the model (5.2), and obtain the following Theorem.

Theorem 10. The model (5.1) has a unique equilibrium point $A(\frac{{{b_1}}}{{{b_2}}}, 0)$, where $A$ is a saddle, and the model (5.2) has an equilibrium point $B(0, 0)$, which is an unstable node.

Proof: From the above analysis, the Jacobian matrix of the model (5.1) evaluated at $A(\frac{{{b_1}}}{{{b_2}}}, 0)$ is given by

Apparently, the eigenvalues of ${J_A}$ are ${\lambda _1} = - {b_1} < 0$ and ${\lambda _2} = {b_1} > 0$. Hence, $A$ is a saddle.

Similarly, the Jacobian matrix of the model (5.2) evaluated at $B(0, 0)$ is given by

We can easily see that the eigenvalues of ${J_B}$ are ${\lambda _1} = {b_2} > 0$ and ${\lambda _2} = {b_2} > 0$. Hence, $B$ is an unstable node. The proof of this theorem is completed.

3.3.2.   Nonexistence of closed orbits

In this section, we will discuss whether the model (2.1) has closed orbits. In order to study this, we divide the whole parameter plane $({a_1}, {a_2}, {b_1}, {b_2}, {q_1}, {q_1}, c, d, m, E, u) \in R_ + ^{11}$ into five regions as follows:

where

Then we can get the following result.

Theorem 11. The model (2.1) has no closed orbit.

Proof: We firstly consider $({a_1}, {a_2}, {b_1}, {b_2}, {q_1}, {q_1}, c, d, m, E, u) \in {S_1}$. From previous analysis, we know that the model (2.1) has no interior equilibrium point in ${S_1}$, then the model (2.1) has no closed orbit because $x = 0, y = 0$ and $y = {y_3^*}$ are invariant sets, otherwise, the closed orbit must intersect with one of these three orbits. Now we consider $({a_1}, {a_2}, {b_1}, {b_2}, {q_1}, {q_1}, c, d, m, E, u) \in {S_2}$, it can claim that there is no closed orbit in ${S_2}$. Indeed, supposing that the model (2.1) exists a closed orbit, then there is an equilibrium point in the interior of the closed orbit. Since $y = {y_3^*}$ is an invariant set and the interior equilibrium point ${E_3^*}$ lies on this line, hence the closed orbit must have two intersection points with $y = {y_3^*}$. This contradicts the existence and uniqueness of solutions. A similar argument, because $y = {y_1^*}$ and $y = {y_2^*}$ are also invariant sets, thus the proof of the case that $({a_1}, {a_2}, {b_1}, {b_2}, {q_1}, {q_1}, c, d, m, E, u) \in {S_3}\; or\; {S_4}\; or\; {S_5}$ is similar to that of ${S_2}$. Hence the model (2.1) has no closed orbits. This completely ends the proof.

According to the above mathematical analysis, some key threshold conditions have been derived to guarantee the existence and stability of all possible equilibrium points, the occurrence of saddle-node bifurcation and transcritical bifurcation, nonexistence of closed orbit, which can provide a theoretical basis for numerical simulation about dynamical behaviors of the model (2.1). Furthermore, it is worthy of our attention from the given related theorems that the parameter $m$ and $q_{1}$ can be regarded as key parameter to control dynamic evolution process of the model (2.1).

4.   Results of numerical simulations

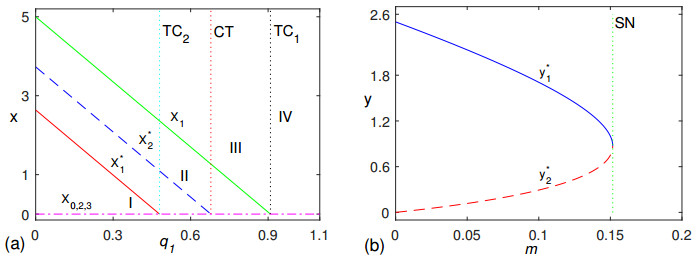
As we all know, Allee effect and harvest have a serious influence on the dynamic behavior of the model (2.1). In order to explore their influence mechanism and the inhibition of algicidal bacteria on algae, some numerical simulation works are carried out with the help of MATLAB software, which also in turn verify the correctness and feasibility of the theoretical results. Furthermore, some phase diagrams (see Figure 2, Figure 3, Figure 4 and Figure 5) can represent the survival mode of algicidal bacteria and algae, and bifurcation diagrams (see Figure 1) can represent the dynamic change process of algae inhibition by algicidal bacteria with the change of key ecological and environmental factors.

It is easy to find from table $1$ that the model (2.1) has two boundary equilibrium points ${E_2}$ and ${E_3}$ when $0 < m < {m_1}$, further when $0 < {q_1} < \min \{ g(y_1^*), g(y_2^*)\}$, the model(2.1) has two interior equilibrium points ${E_1^*}$ and ${E_2^*}$, hence the premise of parametric ranges are $0 < m < {m_1}$ in Figure 1(a) and $0 < {q_1} < \min \{ g(y_1^*), g(y_2^*)\}$ in Figure 1(b). Furthermore, it is worth emphasizing that in Figure 1(a) the three vertical lines passing through points $(\frac{{{a_1}}}{{mE}}, 0)$, $(g(y_1^*), 0)$ and $(g(y_2^*), 0)(g(y_1^*) < g(y_2^*))$ are the transcritical bifurcation lines $TC_{1}$ and $TC_{2}$ as well as a critical threshold line $CT$, respectively. Moreover, if ${q_1} < \min \{ g(y_1^*), g(y_2^*)\})$, which means that the model (2.1) has two interior equilibrium points ${E_1^*}$ and ${E_2^*}$, and four boundary equilibrium points ${E_0}$, ${E_1}$, ${E_2}$ and ${E_3}$ when the value of ${q_1}$ is positioned within region I of Figure 1(a), where ${E_2^*}$, ${E_0}$ and ${E_2}$ are saddle, ${E_1^*}$ and ${E_1}$ are stable node and ${E_3}$ is an unstable node, the corresponding phase diagram is shown in Figure 2(a). Now it is worth pointing out that the equilibrium point ${E_1^*}$ is a stable node, which suggests that algae and algicidal bacteria can form a stable coexistence regional mode, but ${E_1}$ is a stable node, which also suggests that algicidal bacteria will eventually go extinct if the density of algicidal bacteria is below a critical threshold. Thus, this result is in response to the Allee effect, and further reveals the influence mechanism of Allee effect on the dynamic change of algae and algicidal bacteria. That is to say, if the Allee effect threshold is too small and algicidal bacteria can not reproduce rapidly, the algal population will reproduce in large numbers, which can increase the possibility of algal bloom.

While when the value of key parameter ${q_1}$ is gradually increasing across the transcritical bifurcation $TC_{2}$ and enters into region II, there is a unique interior equilibrium point ${E_2^*}$ and four boundary equilibrium points ${E_0}$, ${E_1}$, ${E_2}$ and ${E_3}$ in the model (2.1), where ${E_2^*}$ and ${E_0}$ are saddle, ${E_1}$ and ${E_2}$ are stable node and ${E_3}$ is an unstable node, the corresponding phase diagram is shown in Figure 2(b). Thus, it should be pointed out by us that ${E_1}$ and ${E_2}$ are stable node, which hints that algicidal bacteria will eventually go extinct if the density of algicidal bacteria is below a critical threshold, but algae will eventually go extinct if the density of algicidal bacteria is greater than the critical threshold, these results reveal that algae and algicidal bacteria can not form a stable coexistence mode under the joint action of Allee effect and harvest. As the value of key parameter ${q_1}$ gradually increases across the critical threshold line $CT$ and enters into region III, there is no interior equilibrium point and only four boundary equilibrium points ${E_0}$, ${E_1}$, ${E_2}$ and ${E_3}$ in the model (2.1), where ${E_0}$ and ${E_3}$ are saddle, ${E_1}$ and ${E_2}$ are stable node, the corresponding phase diagram is shown in Figure 2(c). It is obvious to find by comparing Figure 2b with Figure 2c that algae and algicidal bacteria still can not form a stable coexistence mode under the joint action of Allee effect and harvest, but their influences have always existed. When the value of key parameter ${q_1}$ continuously increases across the transcritical bifurcation $TC_{1}$ and finally enters into region IV, there is no interior equilibrium point and only three boundary equilibrium points ${E_0}$, ${E_2}$ and ${E_3}$ in the model (2.1), where ${E_3}$ is a saddle, ${E_0}$ and ${E_2}$ are stable node, the corresponding phase diagram is shown in Figure 2(d), which suggests that if the density of algicidal bacteria is lower than a critical threshold, algicidal bacteria will become extinct, while algicidal bacteria can survive if the density of algicidal bacteria is higher than this critical threshold, but no matter what, algae is always going extinct. These results show that if the value of the harvesting parameter is too large, algae will go extinct, which is not conducive to the permanence of algae and algicidal bacteria.

In order to investigate the occurrence of the saddle-node bifurcation behavior in the model (2.1), a bifurcation diagram of the model (2.1) has been drawn by directly continuing the threshold value of $m_{SN}$ in Figure 1(b), where the vertical lines passing through points $({m_1}, 0)$ are the saddle-node bifurcation curve $SN$. If $0 < m < m_{SN}$ and ${q_1} < \min \{ g(y_1^*), g(y_2^*)\})$, then the model (2.1) has two interior equilibrium points ${E_1^*}$ and ${E_2^*}$ and four boundary equilibrium points ${E_0}$, ${E_1}$, ${E_2}$ and ${E_3}$ (see Figure 2(a)). If the value of $m$ gradually increases and reaches $m_{SN}$, then the equilibrium point ${E_1^*}$ and ${E_2^*}$ can coincide at the line $SN$. Thus, the model (2.1) will produce the coincident point ${E_3^*}$ when ${q_1} < g(y_3^*)$, and three boundary equilibrium points ${E_0}, {E_1}, {E_4}$ still exist, where ${E_0}$ is a saddle, ${E_1}$ is a stable node and ${E_3^*}$ and ${E_4}$ are saddle-node points, the corresponding phase diagram is shown in Figure 3(a). However when the value of $m$ continuously increases and acroses the threshold value $m_{SN}$, the model (2.1) has no interior equilibrium point and two boundary equilibrium points ${E_0}$ and ${E_1}$ still exist, where ${E_0}$ is a saddle, ${E_1}$ is a stable node, the corresponding phase diagram is shown in Figure 3(b). Therefore, it is worthwhile to be happy from Figure 2a, Figure 3a and Figure 3b that the model (2.1) have formed saddle-node bifurcation, that is to say, if the value of the fraction $m$ of the stock available for harvesting is lower than the key value $m_{SN}$, then algae and algicidal bacteria can form a stable coexistence regional mode when the density of algicidal bacteria is higher than a critical threshold, but if the value of the fraction $m$ of the stock available for harvesting is higher than the key value $m_{SN}$, algicidal bacteria always going extinct no matter what the density of algicidal bacteria is.

Furthermore, in order to explore the interaction mechanism of harvesting related parameters $m$ and ${q_1}$ on the permanence of algae and algicidal bacteria, some phase portraits of the model have been given. When $m = m_{SN} = 0.1516685226$, if ${q_1} = 0.48$, then the model (2.1) has no interior equilibrium point and three boundary equilibrium points ${E_0}$, ${E_1}$ and ${E_4}$ exist, where ${E_0}$ is a saddle, ${E_1}$ is a stable node and ${E_4}$ is a saddle-node point, which is shown in Figure 4(a). It is obvious to know from Figure 4(a) that if the density of algicidal bacteria is lower than a critical threshold, algicidal bacteria will become extinct, but algae can survive, while algicidal bacteria can survive if the density of algicidal bacteria is higher than this critical threshold, but algae will go extinct. If ${q_1} = 0.67$, then the model (2.1) has no interior equilibrium point and two boundary equilibrium points ${E_0}$ and ${E_4}$ exist, where ${E_0}$ is a stable node and ${E_4}$ is a saddle-node point, which is shown in Figure 4(b). It is easy to find that the density of algicidal bacteria is lower than a critical threshold, algae and algicidal bacteria will become extinct together, while algicidal bacteria can survive if the density of algicidal bacteria is higher than this critical threshold, but algae will become extinct. But it is worth emphasizing that the persistence and extinction of algicidal bacteria can reveal the existence of Allee effect. However, it is a pity that when $m = 0.35$ and ${q_1} = 0.3$, the model (2.1) only has one boundary equilibrium point ${E_0}$, which is a stable node, which is shown in Figure 4(c), and this suggests that when the overall harvest is excessive, algae and algicidal bacteria will eventually go extinct regardless of their initial density. In addition, we also investigate the impact of Allee effect on amensalism ecosystem by comparing Figure 2(a) and Figure 5 with different $u$ values, it is easy to find that the strength of Allee effect does not change the type and stability of equilibrium points, and only affects the internal dynamic evolution characteristics of algae and algicidal bacteria. In other words, it only affects the survival and reproduction of algicidal bacteria.

Based on the above dynamic analysis results, we will reveal biological significance of the dynamic behavior of the model (2.1). Firstly, it is easy to find from Figure 2 that the key threshold of Allee effect on algicidal bacteria has always been the $y$ coordinate value of the equilibrium point $E_{3}$, if the density of algicidal bacteria is lower than the critical value, then algicidal bacteria is always going extinct, if the density of algicidal bacteria is larger than the critical value, then algicidal bacteria always approaches to the $y$ coordinate value of equilibrium point $E_{2}$. That is to say, the initial density of algicidal bacteria determines whether algicidal bacteria can survive for a long time, and then affects whether algae and algicidal bacteria can coexist for a long time. However, it is a pity to find from Figure 5 that Allee effect has little effect on the specific dynamic behavior of the model (2.1), and does not change the dynamic characteristics of the model (2.1). Secondly, it is worthy of our attention from Figure 2(a), Figure 3 and Figure 4 that if the comprehensive harvest is moderate ($m < m_{SN}$ and ${q_1} < \min \{ g(y_1^*), g(y_2^*), g(y_3^*)\}$), then algae and algicidal bacteria can form a stable coexistence regional mode, which can be produced by saddle-node bifurcation, it stands for new stable and orderly formation. In other words, saddle-node bifurcation can lead to the coexistence of algae and algicidal bacteria, which is conducive to a good cycle of biological control of algae. However, if we over harvest or salvage algae, although we can effectively control the outbreak of algal bloom, but we also indirectly harvest or salvage a part of algicidal bacteria, which is not conducive to the rapid propagation and space survival of algicidal bacteria and can lead to the reproduce failure and the extinction of algicidal bacteria. Finally, it should be stressed from Figure 1(a) and Figure 2 that the model (2.1) can appear transcritical bifurcation, which can represent steady state transformation, that is to say, the intrinsic nature of amensalism dynamic relationship has not been changed, but only the coexistence mode of algae and algicidal bacteria has been changed. Moreover, all the numerical simulation results also indirectly prove the validity and feasibility of the theoretical results.

5.   Conclusion

In this paper, with the framework of studying how algicidal bacteria inhibit algal growth, we have proposed an aquatic amensalism model with a non-selective harvesting and Allee effect, Using relevant mathematical theory, we mainly gave some critical conditions for the existence and local stability of all possible equilibrium points and the occurrence of saddle-node bifurcation and transcritical bifurcation. Furthermore, the dynamics near infinity and nonexistence of closed orbits have also been probed. These theoretical results not only provide theoretical basis for numerical simulation, but also suggest that the model (2.1) has some equilibrium points of different properties and bifurcation dynamics.

In order to investigate how harvest and Allee effect affect the dynamic behavior of the aquatic amensalism model(2.1), some numerical simulation works have been carried out, which can not only make clear the feasibility of mathematical deduction results, but also reveal the dynamic trend of algae and algicidal bacteria, and is more able to show the changing characteristics of attributes and types of equilibrium points. The numerical simulation results show that the model (2.1) has different types of equilibrium points with the value of key parameter $q_{1}$ changing, which also can produce transcritical bifurcation. At the same time, as the value of key parameter $q_{1}$ increases, algae and algicidal bacteria have different coexistence modes, such regional stable state mode, algicidal bacteria gradual extinction mode and algae gradual extinction mode. Meanwhile, it is also worth explaining that the model (2.1) can generate saddle-node bifurcation dynamics, which mainly represents a new stable and orderly formation with the value of key parameter $m$ changing, and hints that algae and algicidal bacteria can survive with a stable manner in a certain range. Furthermore, it should be stressed that non-selective harvesting can strongly affect the survival and extinction of algae and algicidal bacteria, and over harvesting can eliminate the Allee effect on the dynamic behavior of the model (2.1). However, it needs our special emphasis that the Allee effect of algicidal bacteria is the key factor whether algicidal bacteria can effectively inhibit the growth of algae population.

Compared with the previous research on some amensalism models, the greatest advantage of this paper is to establish an aquatic amensalism model from the perspective of exploratory research on biological algae control technology, which can put the amensalism model into a certain ecological environment system, and make that the bifurcation dynamic behaviors have certain ecological and environmental significance. On this basis, the inhibition mechanism of algicidal bacteria on algae is explored from the perspective of population dynamics, which can not only explain the internal ecological significance of bifurcation dynamics, but also help us to deeply understand the algae dissolving mode and mechanism of algicidal bacteria.

In the follow-up research works, we will first deepen theoretical research of bifurcation dynamics, especially Bogdanov-Takens bifurcation, and then further modify the model to study the growth trend of algae population dynamics when the inhibitory effect of algicidal bacteria on algae is at a disadvantage, finally explore how to effectively repair the microbial flora structure and enhance the inhibitory ability of algicidal bacteria, which can effectively prevent the outbreak of algal bloom and maintain the basic ecological balance of water body. In a word, all these results are expected to be useful in studying dynamic behavior of the amensalism ecosystem and algae controlling technology by microorganism.

Acknowledgments

This work was supported by the key International Cooperation Projects of China (Grant No: 2018YFE0103700), the National Natural Science Foundation of China (Grant No: 31570364, 61871293, 41876124, 61901303), by the Zhejiang Provincial Natural Science Foundation of China (Grant No: 2Q18C030002), by key research and development projects of Zhejiang Province (Grant No: 2021C03166), by the Science and Technology program of Cangnan, China (Grant No: 2018ZG29), by Science and Technology Major Program of Wenzhou, China (Grant No: 2018ZG002).

Conflict of interest

The author declares that there are no conflicts of interest.

Appendix