Citation: Renji Han, Binxiang Dai, Lin Wang. Delay induced spatiotemporal patterns in a diffusive intraguild predation model with Beddington-DeAngelis functional response[J]. Mathematical Biosciences and Engineering, 2018, 15(3): 595-627. doi: 10.3934/mbe.2018027
| [1] | Xin-You Meng, Yu-Qian Wu . Bifurcation analysis in a singular Beddington-DeAngelis predator-prey model with two delays and nonlinear predator harvesting. Mathematical Biosciences and Engineering, 2019, 16(4): 2668-2696. doi: 10.3934/mbe.2019133 |
| [2] | Fang Liu, Yanfei Du . Spatiotemporal dynamics of a diffusive predator-prey model with delay and Allee effect in predator. Mathematical Biosciences and Engineering, 2023, 20(11): 19372-19400. doi: 10.3934/mbe.2023857 |
| [3] | Xiaoying Wang, Xingfu Zou . Pattern formation of a predator-prey model with the cost of anti-predator behaviors. Mathematical Biosciences and Engineering, 2018, 15(3): 775-805. doi: 10.3934/mbe.2018035 |
| [4] | Yan Wang, Minmin Lu, Daqing Jiang . Viral dynamics of a latent HIV infection model with Beddington-DeAngelis incidence function, B-cell immune response and multiple delays. Mathematical Biosciences and Engineering, 2021, 18(1): 274-299. doi: 10.3934/mbe.2021014 |
| [5] | Kalyan Manna, Malay Banerjee . Stability of Hopf-bifurcating limit cycles in a diffusion-driven prey-predator system with Allee effect and time delay. Mathematical Biosciences and Engineering, 2019, 16(4): 2411-2446. doi: 10.3934/mbe.2019121 |
| [6] | Yue Xing, Weihua Jiang, Xun Cao . Multi-stable and spatiotemporal staggered patterns in a predator-prey model with predator-taxis and delay. Mathematical Biosciences and Engineering, 2023, 20(10): 18413-18444. doi: 10.3934/mbe.2023818 |
| [7] | G.A.K. van Voorn, D. Stiefs, T. Gross, B. W. Kooi, Ulrike Feudel, S.A.L.M. Kooijman . Stabilization due to predator interference: comparison of different analysis approaches. Mathematical Biosciences and Engineering, 2008, 5(3): 567-583. doi: 10.3934/mbe.2008.5.567 |
| [8] | Zhihong Zhao, Yan Li, Zhaosheng Feng . Traveling wave phenomena in a nonlocal dispersal predator-prey system with the Beddington-DeAngelis functional response and harvesting. Mathematical Biosciences and Engineering, 2021, 18(2): 1629-1652. doi: 10.3934/mbe.2021084 |
| [9] | Yuhong Huo, Gourav Mandal, Lakshmi Narayan Guin, Santabrata Chakravarty, Renji Han . Allee effect-driven complexity in a spatiotemporal predator-prey system with fear factor. Mathematical Biosciences and Engineering, 2023, 20(10): 18820-18860. doi: 10.3934/mbe.2023834 |
| [10] | Ming Chen, Menglin Gong, Jimin Zhang, Lale Asik . Comparison of dynamic behavior between continuous- and discrete-time models of intraguild predation. Mathematical Biosciences and Engineering, 2023, 20(7): 12750-12771. doi: 10.3934/mbe.2023569 |
Competition and predation are two fundamental ecological relationships among species and have been widely studied [1]. Recently, it is been recognized that intraguild predation (IGP), which is a combination of competition and predation, has significant impacts on the distribution, abundance, persistence and evolution of the species involved [2]. As a result, growing attention has been paid to IGP models [3,4,5,6,7,8,9].
The general framework of IGP described below was established by Holt and Polis [5]
| {˙R(t)=R(φ(R)−ρ1(R,N,P)N−ρ2(R,N,P)P),˙N(t)=N(e1ρ1(R,N,P)R−ρ3(R,N,P)P−m1),˙P(t)=P(e2ρ2(R,N,P)R+e3ρ3(R,N,P)N−m2), | (1) |
where
Functional response describes how the consumption rate of individual consumers varies with respect to resource density and is often used to model predator-prey interactions. For IGP models, several functional response functions have been studied. For instance, Velazquez
Note that the reproduction of predator following the consumption of prey is not instantaneous, but rather is mediated by some reaction-time lag required for gestation. Time delay plays an important role in ecology and it can induce very complex dynamical behaviors [16,17,18,19,20,21,22]. For IGP models, it has been shown that a time delay greatly impacts their dynamics [23,24]. In [24], Shu
| {˙R(t)=rR(t)(1−R(t)K)−c1R(t)N(t)−c2R(t)P(t),˙N(t)=e1c1R(t−τ)N(t−τ)−c3N(t)P(t)−m1N(t),˙P(t)=e2c2R(t)P(t)+e3c3N(t)P(t)−m2P(t), | (2) |
where
Note that for each species, individuals tend to migrate towards regions with lower population densities. Hence the species are distributed over space and interact with each other within their spatial domains. To take spatial effects into consideration, reaction diffusion equations become a natural choice [25,26,27,28,29,30,31,32,33,34,35,36]. In this work, we consider a reaction diffusion IGP model with delay and Beddington-DeAngelis functional response.
Suppose
| {∂R(t,x)∂t=˜d1ΔR+R(r(1−RK)−c1N−c2P1+a1R+a2P),t>0,x∈Ω∂N(t,x)∂t=˜d2ΔN+e1c1N(t−τ,x)R(t−τ,x)−c3NP−m1N,t>0,x∈Ω,∂P(t,x)∂t=˜d3ΔP+P(e2c2R1+a1R+a2P+e3c3N−m2),t>0,x∈Ω,∂R∂ν=∂N∂ν=∂P∂ν=0,t>0,x∈∂Ω,R(t,x)=˜ϕ1(t,x)≥0,(t,x)∈[−τ,0]×Ω,N(t,x)=˜ϕ2(t,x)≥0,(t,x)∈[−τ,0]×Ω,P(t,x)=˜ϕ3(t,x)≥0,(t,x)∈[−τ,0]×Ω, | (3) |
where
| Symbol | Parameter Definition | Units |
| Basal resource intrinsic growth rate | [time] |
|
| Basal resource carrying capacity | [Basal resource density] | |
| Predation rate of IG prey on resource | [IG prey] |
|
| Predation rate of IG predator on resource | [IG predator] |
|
| Predation rate of IG predaotr on IG prey | [IG preys][IG predator] |
|
| [time] |
||
| Conversion rate from resource to IG prey | [IG preys][resource] |
|
| Conversion rate from resource to IG predator | [IG predators][resource] |
|
| Conversion rate from IG prey to IG predator | [IG predators][IG prey] |
|
| [Half saturation constant] |
[resource] |
|
| [Half saturation constant] |
[IG predator] |
|
| Mortality rate of IG prey | [time] |
|
| Mortality rate of IG predator | [time] |
|
| Diffusion coefficient of resource | [length] |
|
| Diffusion coefficient of IG prey | [length] |
|
| Diffusion coefficient of IG predatior | [length] |
|
| The size of spatial domain |
[length] |
For rescalling, we let
| u1(t,x)=R(t,x)K,u2(t,x)=c1N(t,x)r,u3(t,x)=c2P(t,x)r,γ1=m1r,γ2=m2r,β1=e1c1Kr,β2=e2c2Kr,α=c3c2,β=e3c3c1,b=a1K,c=a2rc2,d1=˜d1rL2,d2=˜d2rL2,d3=˜d3rL2,ˆx=xL,ˆt=tr,ˆτ=τr,ϕ1(t,x)=˜ϕ1(t,x)K,ϕ2(t,x)=c1˜ϕ2(t,x)r,ϕ3(t,x)=c2˜ϕ3(t,x)r. |
Then model (1.3) becomes
| {∂u1(t,x)∂t=d1Δu1(t,x)+f1(u,v),t>0,x∈Ω,∂u2(t,x)∂t=d2Δu2(t,x)+f2(u,v),t>0,x∈Ω,∂u3(t,x)∂t=d3Δu3(t,x)+f3(u,v),t>0,x∈Ω,∂u1(t,x)∂ν=∂u2(t,x)∂ν=∂u3(t,x)∂ν=0,t>0,x∈∂Ω,u1(t,x)=ϕ1(t,x)≥0,(t,x)∈[−τ,0]×Ω,u2(t,x)=ϕ2(t,x)≥0,(t,x)∈[−τ,0]×Ω,u3(t,x)=ϕ3(t,x)≥0,(t,x)∈[−τ,0]×Ω, | (4) |
where
| f1(u,v)=u1(t,x)(1−u1(t,x)−u2(t,x)−u3(t,x)1+bu1(t,x)+cu3(t,x)), |
| f2(u,v)=β1u1(t−τ,x)u2(t−τ,x)−αu2(t,x)u3(t,x)−γ1u2(t,x), |
| f3(u,v)=u3(t,x)(β2u1(t,x)1+bu1(t,x)+cu3(t,x)+βu2(t,x)−γ2). |
Throughout the paper, we denote
| C(ˉDT)≡C(ˉDT)×C(ˉDT)×C(ˉDT),Cγ(ˉDT)≡Cγ(ˉDT)×Cγ(ˉDT)×Cγ(ˉDT). |
Denote
| X={(u1,u2,u3)T∈H2(Ω)×H2(Ω)×H2(Ω):∂u1∂ν=∂u2∂ν=∂u3∂ν=0on∂Ω} |
with the usual inner product
The rest of the paper is organized as follows. In Section 2, we study the existence and uniqueness of solution of (4) and estimate the solution's priori bounds. In Section 3, we discuss the existence of nonnegative spatially homogeneous steady states. In Section 4, we carry out stability analysis and Hopf bifurcation analysis about the unique positive spatially homogeneous steady state of System (4). Numerical simulations are presented in Section 5 to illustrate the impacts of delay, diffusion and the functional response on the dynamics of our IGP model. We conclude this paper with a brief summary and discussion in Section 6.
Theorem 2.1. Consider System (4), we have the following conclusions.
(ⅰ) Given any initial condition
| 0≤ϕi(t,x)≤Li,(t,x)∈Q0,i=1,2,3, | (5) |
where
| 1≤L1≤γ1β1,L2<γ2β,L3≥1c[β2L1γ2−βL2−bL1−1], | (6) |
System (4) admits a unique solution
| 0≤ui(t,x)≤Li,fort>0,x∈Ω,i=1,2,3. |
(ⅱ) For any solution
| lim supt→∞u1(t,x)≤1,lim supt→∞∫Ωu2(t,x)dx≤J1,lim supt→∞∫Ωu3(t,x)dx≤J2 |
where
Furthermore, if
| lim supt→∞u2(t,x)≤β2α4κβ+β1κ(β14γ1+β1)+β2αβ,lim supt→∞u3(t,x)≤β24κ+β1βακ(β14γ1+β1)+β2. |
Proof. Note that
| {∂˜u1∂t≥d1Δ˜u1+˜u1(1−˜u1−ˆu2−ˆu31+b˜u1+cˆu3)∂~u2∂t≥d2Δ˜u2+β1˜u1˜u2−αˆu3˜u2−γ1˜u2,∂˜u3∂t≥d3Δ˜u3+β2˜u1˜u31+b˜u1+c˜u3+β˜u2˜u3−γ2˜u3 | (7) |
and
| {∂ˆu1∂t≤d1Δˆu1+ˆu1(1−ˆu1−˜u2−˜u31+bˆu1+c˜u3)∂ˆu2∂t≤d2Δˆu2+β1ˆu1ˆu2−α˜u3ˆu2−γ1ˆu2∂ˆu3∂t≤d3Δˆu3+β2ˆu1ˆu31+bˆu1+cˆu3+βˆu2ˆu3−γ2ˆu3. | (8) |
Take
| (0,0,0)≤(u1(t,x),u2(t,x),u3(t,x))≤(L1,L2,L3),t≥0,x∈Ω. |
This completes the proof of (ⅰ).
Next we establish the priori bound of solutions to System (4). To estimate
| {∂u1(t,x)∂t≤d1Δu1(t,x)+u1(t,x)(1−u1(t,x)),t>0,x∈Ω,∂u1(t,x)∂n=0,t>0,x∈∂Ω. |
It follows from the standard comparison principle [37,Lemma 3.4.2] of parabolic equations that
To estimate the priori bounds of
| U1(t)=∫Ωu1(t,x)dx,U2(t)=∫Ωu2(t,x)dx,U3(t)=∫Ωu3(t,x)dx. |
Then
| dU1(t)dt=∫Ω∂u1∂tdx=∫Ωd1Δu1dx+∫Ω[u1(t,x)(1−u1(t,x)−u2(t,x)−u3(t,x)1+bu1(t,x)+cu3(t,x))]dx, |
| dU2(t)dt=∫Ω∂u2∂tdx=∫Ωd2Δu2dx+∫Ω[β1u1(t−τ,x)u2(t−τ,x)−αu2(t,x)u3(t,x)−γ1u2(t,x)]dx, |
| dU3(t)dt=∫Ω∂u3∂tdx=∫Ωd3Δu3dx+∫Ω[u3(t,x)(β2u1(t,x)1+bu1(t,x)+cu3(t,x)+βu2(t,x)−γ2)]dx. |
From the Neumann boundary conditions, we further obtain
| d(β1U1(t)+U2(t+τ))dt=β1∫Ω∂u1∂tdx+∫Ω∂u2(t+τ,x)∂tdx=β1∫Ω(u1−u21)dx−∫Ωβ1u1u31+bu1+cu3dx−∫Ωαu2(t+τ,x)u3(t+τ,x)dx−∫Ωγ1u2(t+τ,x)dx≤β14|Ω|+γ1β1(1+ε)|Ω|−γ1(β1U1(t)+U2(t+τ)),t>T1. |
By the comparison principle, we have
| lim supt→∞(β1U1(t)+U2(t+τ))≤β14γ1|Ω|+β1|Ω|≡J1. |
Similarly, there exists
| d(β2U1(t)+βαU2(t)+U3(t))dt=β2∫Ω(u1−u21)dx+∫Ωβ1βαu1(t−τ,x)u2(t−τ,x)dx−βγ1α∫Ωu2dx−γ2∫Ωu3dx≤β24|Ω|+β1βα(1+ε)∫Ωu2(t−τ,x)dx−βγ1αU2−γ2U3≤β24|Ω|+β1βα(1+ε)(J1+ε)|Ω|+β2κ(1+ε)|Ω|−κ(β2U1+βαU2+U3), |
where
| lim supt→∞(β2U1+βαU2+U3)≤β24κ|Ω|+β1βακJ1|Ω|+β2|Ω|≡J2. |
and hence
For the case with
| {∂S∂t=dΔS+β2(u1−u21)−β2u1u2+ββ1αu1u2−βγ1αu2−γ2u3,t>T3,x∈Ω,∂S∂ν=0,t>T3,x∈∂Ω,S(T3,x)=β2u1(T3,x)+βαu2(T3,x)+u3(T3,x),x∈Ω. |
Thus for
| β2(u1−u21)−β2u1u2+ββ1αu1u2−βγ1αu2−γ2u3≤β24+β1βα(1+ε)(β14γ1+β1+ε)+β2κ(1+ε)−κS. |
Consider the system
| {∂W∂t=dΔW+β24+β1βα(1+ε)(β14γ1+β1+ε)+β2κ(1+ε)−κW,t>T3,x∈Ω∂W∂ν=0,t>T3,x∈∂Ω,W(T3,x)=β2u1(T3,x)+βαu2(T3,x)+u3(T3,x),x∈Ω. |
It follows from [37,Theorem 2.4.6] that the solution
| limt→∞W(t,x)=β24κ+β1βακ(1+ε)(β14γ1+β1+ε)+β2(1+ε). |
The comparison argument implies that
| lim supt→∞u2(t,x)≤lim supt→∞αβS(t,x)≤β2α4κβ+β1κ(β14γ1+β1)+β2αβ |
and
| lim supt→∞u3(t,x)≤lim supt→∞S(t,x)≤β24κ+β1βακ(β14γ1+β1)+β2. |
This completes the proof.
Same as in [24], we denote by
Proposition 1. (ⅰ) The trivial steady state
(ⅱ) There is a weakly semi-trivial steady state in the absence of IG Prey and IG Predator
(ⅲ) The IG Prey-only strong semi-trivial steady state
(ⅳ) The IG Predator-only strong semi-trivial steady state
| ˆu1=−(R2−cR2−b)+√(R2−cR2−b)2+4cR22cR2. |
System (4) admits a positive steady state
| 1−u1−u2−u31+bu1+cu3=0, | (9) |
| β1u1−αu3−γ1=0, | (10) |
| β2u11+bu1+cu3+βu2−γ2=0. | (11) |
It follows from (10) that
| u∗1=αβ1u∗3+γ1β1. | (12) |
Combining (9), (10) and (12), we obtain
| u∗32+pu∗3+q=0, | (13) |
where
| p=cγ2β21+bαβ1(γ2−β)+αβ1(β−β2)+cββ1(γ1−β1)+ββ21+2bαβγ1αβ(bα+cβ1),q=β21(γ2−β)+bβ1γ1(γ2−β)+β1γ1(β−β2)+β(bγ21−β21)αβ(bα+cβ1). |
For the distribution of roots of Eq. (13), we have the following results.
Lemma 3.1. (ⅰ) If
(ⅱ) If
(ⅲ) If
Remark 1. Set
According to Lemma 3.1, the following proposition is valid.
Proposition 2. (ⅰ) When
| R2<min{(bα+cβ1)(−p+√p2−4q)+2(bγ1+β1)α(−p+√p2−4q)+2γ1,(bα+cβ1)(−p−√p2−4q)+2(bγ1+β1)α(−p−√p2−4q)+2γ1} |
System (4) has two positive constant steady states
| E∗−=(u∗1−,u∗2−,u∗3−),whereu∗1+=αu∗3++γ1β1,u∗2+=γ2β−β2β×αu∗3++γ1(bα+cβ1)u∗3++bγ1+β1,u∗3+=−p+√p2−4q2andu∗1−=αu∗3−+γ1β1,u∗2−=γ2β−β2β×αu∗3−+γ1(bα+cβ1)u∗3−+bγ1+β1,u∗3−=−p−√p2−4q2. |
(ⅱ) When
(ⅲ) When
Remark 2. There exist parameter values such that Proposition 3.4 holds. For example, choosing
| E∗=(0.1891,0.7562,0.2344). |
Let
Lemma 4.1.
| X=∞⊕i=1Xi,whereXi=dimE(μi)⊕j=1Xij. |
In the following, we consider the stability of
Theorem 4.2.
(ⅰ) The trivial steady state
(ⅱ) The semi-trivial steady state
Proof. Linearizing System (4) at a constant steady state
| ∂u∂t=(DΔ+ˆJ1)u+ˆJ2uτ, | (14) |
where
| ˆJ1=[1−2u⋄1−u⋄2−u⋄3+cu⋄32(1+bu⋄1+cu⋄3)2−u⋄1−(1+bu⋄1)u⋄1(1+bu⋄1+cu⋄3)20−αu⋄3−γ1−αu⋄2β2(1+cu⋄3)u⋄3(1+bu⋄1+cu⋄3)2βu⋄3β2u⋄1(1+bu⋄1)(1+bu⋄1+cu⋄3)2+βu⋄2−γ2],ˆJ2=[000β1u⋄2β1u⋄10000]. |
From Lemma 4.1, we know that the eigenvalues of the System (4) is confined on the subspace
| det(λI3+μiD−J∗)=0, | (15) |
where
(ⅰ) If
| (λ+d1μi−1)(λ+d2μi+γ1)(λ+d3μi+γ2)=0, |
which gives three sets of eigenvalues, namely,
(ⅱ) If
| (λ+d1μi+1)(λ+d2μi−β1e−λτ+γ1)(λ+d3μi−β21+b+γ2)=0, |
which gives the eigenvalues
Theorem 4.3. Suppose that
Proof. Let
We claim that
| ˉu(m)1=ˉu(m−1)1+1K1[ˉu(m−1)1(1−ˉu(m−1)1−u_(m−1)2−u_(m−1)31+bˉu(m−1)1+cu_(m−1)3)],u_(m)1=u_(m−1)1+1K1[u_(m−1)1(1−u_(m−1)1−ˉu(m−1)2−ˉu(m−1)31+bu_(m−1)1+cˉu(m−1)3)],ˉu(m)2=ˉu(m−1)2+1K2[ˉu(m−1)2(β1ˉu(m−1)1−αu_(m−1)3−γ1)],u_(m)2=u_(m−1)2+1K2[u_(m−1)2(β1u_(m−1)1−αˉu(m−1)3−γ1)],ˉu(m)3=ˉu(m−1)3+1K3[ˉu(m−1)3(β2ˉu(m−1)11+bˉu(m−1)1+cˉu(m−1)3+βˉu(m−1)2−γ2)],u_(m)3=u_(m−1)3+1K3[u_(m−1)3(β2u_(m−1)11+bu_(m−1)1+cu_(m−1)3+βu_(m−1)2−γ2)], |
where
| ˆu≤u_(m)≤u_(m+1)≤ˉu(m+1)≤ˉu(m)≤˜u. | (16) |
It follows from (16) that the limits
| limm→∞ˉu(m)1=ˉu1,limm→∞ˉu(m)2=ˉu2,limm→∞ˉu(m)3=ˉu3,limm→∞u_(m)1=u_1,limm→∞u_(m)2=u_2,limm→∞u_(m)3=u_3 | (17) |
exist and satisfy the following equations
| f1(ˉu1,u_2,u_3)=0,f2(ˉu1,ˉu2,u_3)=0,f3(ˉu1,ˉu2,ˉu3)=0, | (18) |
| f1(u_1,ˉu2,ˉu3)=0,f2(u_1,u_2,ˉu3)=0,f3(u_1,u_2,u_3)=0, | (19) |
where
| ˆu=(ˆu1,ˆu2,ˆu3),u_(m)=(u_(m)1,u_(m)2,u_(m)3),u_(m+1)=(u_(m+1)1,u_(m+1)2,u_(m+1)3), |
| ˉu(m+1)=(ˉu(m+1)1,ˉu(m+1)2,ˉu(m+1)3),ˉu(m)=(ˉu(m)1,ˉu(m)2,ˉu(m)3),˜u=(˜u1,˜u2,˜u3). |
Since
Next, we consider the stability of the two strong semi-trivial steady states:
Theorem 4.4. Consider System (4) with
(ⅰ) If
(ⅱ) If
(ⅲ) If
Proof. For
| m_1(\lambda)[g_1(\lambda)+h_1(\lambda)e^{-\lambda\tau}] = 0, \label{Eq4.7} | (20) |
with
Denote
| \bar{E}_1 = d_1\mu_i+\frac{1}{\mathcal{R}_1}>0, \bar L_1 = d_2\mu_italic>0, \quad \quad \bar J_1 = \gamma_1(1-\frac{1}{\mathcal{R}_1})>0. |
Then we have
| \label{Eq4.8} g_1(\lambda)+h_1(\lambda) = \lambda^2+(\bar E_1+\bar L_1)\lambda+\bar E_1\bar L_1+\bar J_1. | (21) |
Since
Define
| \begin{split} \label{Eq4.9} G(u)\equiv&\mid g_1(i\sqrt{u})\mid^2-\mid h_1(i\sqrt u)\mid^2\\ = &u^2+(\bar L_1^2+2\bar L_1\gamma_1+\bar E_1^2)u+\bar E_1^2\bar L_1^2+2\bar E_1^2\bar L_1\gamma_1-\bar J_1^2+2\bar E_1\gamma_1\bar J_1. \end{split} | (22) |
Since
Next, we discuss the distribution of positive zeros of
If
| \mathcal{F}(\mu_i)\geq 0 \mbox{ for }\quad italic>N_1, \mbox{ and } \mathcal{F}(\mu_i)<0 \mbox{ for }\quad i\leq N_1. |
This implies that (22) has no positive root for
| \tau_{i}^j = \frac{1}{\omega_{i}}\left\{\arccos\left(\frac{\mathcal{B}_{1i}} {\sqrt{\mathcal{B}_{1i}^2+\mathcal{C}_{1i}^2}}\right)+2j\pi\right\}, \quad i = 1,2,\cdots,N_1, \quad j = 0,1,2,\cdots, |
where
| \begin{split} \mathcal{B}_{1i} = &(\frac{\beta_1}{\mathcal{R}_1})d_1\mu_i+\frac{\beta_1}{\mathcal{R}_1^2}-\frac{\beta_1}{\mathcal{R}_1} (1-\frac{1}{\mathcal{R}_1})((d_1\mu_i+\frac{1}{\mathcal{R}_1})(d_2\mu_i+\gamma_1)-\omega_i^2)+\\ &\frac{\beta_1}{\mathcal{R}_1}\omega_i^2(d_1\mu_i+d_2\mu_i+\frac{1}{\mathcal{R}_1}+\gamma_1), \end{split} |
| \begin{split} \mathcal{C}_{1i} = &-(\frac{\beta_1}{\mathcal{R}_1} d_1\mu_i+\frac{\beta_1}{\mathcal{R}_1^2}-\frac{\beta_1}{\mathcal{R}_1} (1-\frac{1}{\mathcal{R}_1}))(d_1\mu_i+d_2\mu_i+\frac{1}{\mathcal{R}_1}+\gamma_1)\omega_i+\\ &\frac{\beta_1}{\mathcal{R}_1}\omega_i((d_1\mu_i+\frac{1}{\mathcal{R}_1})(d_2\mu_i+\gamma_1)-\omega_i^2). \end{split} |
Denote
| \tau_{00} = \min\limits_{i = 1,2,\cdots,N_1}\{\tau_i^0\}. |
It follows easily from
For the IG predator-only strong semi-trivial steady state
Theorem 4.5. Consider System (4) with
(ⅰ) If
(ⅱ) If
Proof. For
| \label{Eq4.10} m_2(\lambda)g_2(\lambda) = 0, | (23) |
with
| m_2(\lambda) = (\lambda+d_2\mu_i+\alpha\hat u_3+\gamma_1-\beta_1\hat u_1e^{-\lambda\tau}), |
and
| \begin{array}{l} {g_2}(\lambda ) = (\lambda + {d_1}{\mu _i} + {{\hat u}_1} - \frac{{b{{\hat u}_1}{{\hat u}_3}}}{{{{(1 + b{{\hat u}_1} + c{{\hat u}_3})}^2}}})(\lambda + {d_3}{\mu _i} + {\gamma _2} - \frac{{{\beta _2}{{\hat u}_1}(1 + b{{\hat u}_1})}}{{{{(1 + b{{\hat u}_1} + c{{\hat u}_3})}^2}}}) + \\ \quad \quad \quad \quad \frac{{{\beta _2}{{\hat u}_1}{{\hat u}_3}(1 + b{{\hat u}_1})(1 + c{{\hat u}_3})}}{{{{(1 + b{{\hat u}_1} + c{{\hat u}_3})}^4}}}, \end{array} |
where
| \hat{u}_1 = \frac{-(\mathcal{R}_2-c\mathcal{R}_2-b)+\sqrt{(\mathcal{R}_2-c\mathcal{R}_2-b)^2+4c\mathcal{R}_2}}{2c\mathcal{R}_2}, \hat{u}_3 = \mathcal{R}_2(1-\hat{u}_1)\hat{u}_1. |
Since
Denote
| \begin{align*} &\bar E_2 = d_1\mu_i+\hat u_1-\frac{b\hat u_1\hat u_3}{(1+b\hat u_1+c\hat u_3)^2},\, \bar L_2 = d_3\mu_i+\gamma_2-\frac{\beta_2\hat u_1(1+b\hat u_1)}{(1+b\hat u_1+c\hat u_3)^2},\\ &\bar J_2 = \frac{\beta_2\hat u_1\hat u_3(1+b\hat u_1)(1+c\hat u_3)}{(1+b\hat u_1+c\hat u_3)^4}. \end{align*} |
Then we have
| g_2(\lambda) = \lambda^2+(\bar E_2+\bar L_2)\lambda+\bar E_2\bar L_2+\bar J_2. |
Since
In this subsection, by taking
| ({H_1})\;q < 0\;{\rm{and}}\;{{\cal R}_2} < \frac{{(b\alpha + c{\beta _1})( - p + \sqrt {{p^2} - 4q} ) + 2(b{\gamma _1} + {\beta _1})}}{{\alpha ( - p + \sqrt {{p^2} - 4q} ) + 2{\gamma _1}}}. |
The above assumption guarantees the uniqueness of the positive spatially homogeneous steady state
| \label{Eq4.11} \lambda^3+b_{2i}\lambda^2+b_{1i}\lambda+b_{0i}+e^{-\lambda\tau}(c_{2}\lambda^2+c_{1i}\lambda+c_{0i}) = 0, | (24) |
where
| \begin{align*} b_{2i} = d_1\mu_i+d_2\mu_i+d_3\mu_i+u_1^\ast-bA_3+\beta_1u_1^\ast+c\beta_2A_3,\\ b_{1i} = (d_1\mu_i+u_1^\ast-bA_3)(d_2\mu_i+\beta_1u_1^\ast+d_3\mu_i+c\beta_2A_3)+\\ \quad \quad (d_2\mu_i+\beta_1u_1^\ast)(d_3\mu_i+c\beta_2A_3)+\alpha\beta u_2^\ast u_3^\ast+A_1A_2\beta_2u_1^\ast u_3^\ast, \end{align*} |
| \begin{array}{l} b_{0i}=(d_1\mu_i+u_1^\ast-bA_3)(d_2\mu_i+\beta_1u_1^\ast)(d_3\mu_i+c\beta_2A_3)+(d_1\mu_i+u_1^\ast-bA_3)\alpha\beta u_2^\ast u_3^\ast\\ \quad \quad -\alpha\beta_2A_2u_1^\ast u_2^\ast u_3^\ast+(d_2\mu_i+\beta_1u_1^\ast)A_1A_2\beta_2u_1^\ast u_3^\ast,\\ c_2=-\beta_1u_1^\ast,\\ c_{1i}=\beta_1u_1^\ast u_2^\ast-\beta_1u_1^\ast(d_1\mu_i+d_3\mu_i+u_1^\ast-bA_3+c\beta_2A_3),\\ c_{0i}=-d_1d_3\beta_1u_1^\ast\mu_i^2+d_3\mu_i\beta_1u_1^\ast u_2^\ast-d_1\mu_ic\beta_2A_3\beta_1u_1^\ast-d_3\mu_i(u_1^\ast-bA_3)\beta_1u_1^\ast+\\ \quad \quad (c\beta_2A_3+A_1\beta u_3^\ast)\beta_1u_1^\ast u_2^\ast-(u_1^\ast-bA_3)c\beta_2A_3\beta_1u_1^\ast-A_1A_2\beta_2 u_1^\ast u_3^\ast\beta_1 u_1^\ast,\\ A_1=\frac{1+bu_1^\ast}{(1+bu_1^\ast+cu_3^\ast)^2}, A_2=\frac{1+cu_3^\ast}{(1+bu_1^\ast+cu_3^\ast)^2}, A_3=\frac{u_1^\ast u_3^\ast}{(1+bu_1^\ast+cu_3^\ast)^2}. \end{array} |
Denote
Furthermore, we assume that
| ({H_2}){\mkern 1mu} \;{M_1} > 0. |
| ({H_3}){\mkern 1mu} \;{M_6} - {M_2}{M_7} > 0. |
| ({H_4})\;{M_1}{M_4} + {M_2}{M_3}u_2^ * + {M_2}{M_7} - {M_6} > 0. |
| (H_3^\prime )\;{M_6} - {M_2}{M_7} \le 0. |
| (H_4^\prime )\;{M_1}({M_1}{M_3} + {M_5} + u_2^ * {M_2}) + {M_3}({M_1}{M_3} + {M_4} + {M_5}) + {M_6} - {M_2}{M_7} > 0. |
Theorem 4.6. (ⅰ) Assume that
(ⅱ) Assume that
Proof. When
| \label{Eq4.12} \lambda^3+(b_{2i}+c_2)\lambda^2+(b_{1i}+c_{1i})\lambda+b_{0i}+c_{0i} = 0. | (25) |
Since
| b_{2i}+c_2 = (d_1+d_2+d_3)\mu_i+M_1+M_3>0. |
| \begin{array}{l} {b_{1i}} + {c_{1i}} = ({d_1}{d_2} + {d_1}{d_3} + {d_2}{d_3})\mu _i^2 + ({M_3}{d_1} + {M_3}{d_2} + {M_1}{d_2} + {M_1}{d_3}){\mu _i} + \\ {M_1}{M_3} + {M_4} + {M_5} + u_2^*{M_2} > 0. \end{array} |
| \begin{split} b_{0i}+c_{0i} = &d_1d_2d_3\mu_i^3+(M_3 d_1d_2+M_1 d_2d_3)\mu_i^2+\\ &(M_2u_2^\ast d_3+M_1M_3d_2+M_4d_1+M_5d_2)\mu_i+M_1M_4+M_2M_3u_2^\ast+\\ &M_2M_7-M_6. \end{split} |
| \begin{equation*}\begin{split} (b_{2i}+c_2)(b_{1i}+c_{1i})-(b_{0i}+c_{0i})=&d_1\mu_i(b_{1i}+c_{1i}-d_2d_3\mu_i^2-M_3d_2\mu_i-M_4)+\\ &d_3\mu_i(b_{1i}+c_{1i}-u_2^\ast M_2)+\\ &d_{2}\mu_i(b_{1i}+c_{1i}-M_1d_3\mu_i-M_1M_3-M_5)+\\ &M_1(b_{1i}+c_{1i}-M_4)+M_3(b_{1i}+c_{1i}-u_2^\ast M_2)+\\ &M_6-M_2M_7. \end{split}\end{equation*} |
Since
It follows from
Next, we discuss the effect of the delay
Let
| -\mathrm{i}\omega^3-b_{2i}\omega^2+\mathrm{i}b_{1i}\omega+b_{0i}+(-c_2\omega^2+\mathrm{i}\omega c_{1i}+c_{0i})e^{-\mathrm{i}\omega\tau} = 0, |
which implies that
| \label{Eq4.13} b_{2i}\omega^2-b_{0i} = (c_{0i}-c_2\omega^2)\cos\omega\tau+c_{1i}\omega\sin\omega\tau, | (26) |
| \label{Eq4.14} -\omega^3+b_{1i}\omega = (c_{0i}-c_2\omega^2)\sin\omega\tau-c_{1i}\omega\cos\omega\tau. | (27) |
It follows from (26) and (27) that
| \label{Eq4.15} \omega^6+(b^2_{2i}-2b_{1i}-c_2^2)\omega^4+(b_{1i}^2-2b_{0i}b_{2i}+2c_{0i}c_2-c_{1i}^2)\omega^2+b_{0i}^2-c_{0i}^2 = 0. | (28) |
Let
| \label{Eq4.16} h(s)\equiv s^3+p_is^2+q_is+r_i = 0. | (29) |
From (24), we get
| \begin{array}{l} \quad \quad {p_i} = (d_1^2 + d_2^2 + d_3^2)\mu _i^2 + 2({M_1}{d_1} + {M_2}{d_2} + {M_3}{d_3}){\mu _i} + \\ \quad \quad \quad \quad M_1^2 + M_3^2 - 2{M_4} - 2{M_5},\\ {b_{0i}} - {c_{0i}} = {d_1}{d_2}{d_3}\mu _i^3 + ({d_1}{d_2}{M_3} + {d_2}{d_3}{M_1} + 2{d_1}{d_3}{M_2})\mu _i^2 + \\ \quad \quad \quad \quad (2{d_1}{M_2}{M_3} + {d_2}{M_1}{M_3} + 2{d_3}{M_1}{M_2} + {d_1}{M_4} + {d_2}{M_5} - {d_3}u_2^ * {M_2}){\mu _i}\\ \quad \quad \quad \quad + 2{M_1}{M_2}{M_3} + 2{M_2}{M_5} + {M_1}{M_4} - u_2^ * {M_2}{M_3} - {M_2}{M_7} - {M_6}. \end{array} |
In the following, we need to seek conditions required for Eq. (29) to have at least one positive root. For this purpose, we further make the following hypotheses:
| \begin{array}{l} ({H_6})\;2{d_1}{M_2}{M_3} + {d_2}{M_1}{M_3} + 2{d_3}{M_1}{M_2} + {d_1}{M_4} + {d_2}{M_5} - {d_3}u_2^ * {M_2} \ge 0. \end{array} |
Since
| b_{0i}-c_{0i}<0 \quad \mathrm{for}\quad 1\leq i\leq N_2 \quad \mathrm{and} \quad b_{0i}-c_{0i}\geq 0\quad \mathrm{for}\quad i\geq N_2+1,\quad i\in\mathbb{N}. |
According to the above analysis, we have the following lemma.
Lemma 4.7.(ⅰ) Assume that
(ⅱ) Assume that and
Proof. It follows from
Remark 3. From Lemma 4.2, without loss of generality, for each
| \cos\omega_{k,i}\tau_{k,i} = \frac{(c_{1i}-b_{2i}c_2)\omega_{k,i}^4+(b_{2i}c_{0i}+b_{0i}c_2-c_{1i}b_{1i})\omega_{k,i}^2-b_{0i}c_{0i}}{(c_{0i}-c_2\omega_{k,i}^2)^2+c_{1i}^2\omega_{k,i}^2}. |
Let
| \label{Eq4.17} \tau_{k,j}^i = \frac{\left(\arccos\left(\frac{(c_{1i}-b_{2i}c_2)\omega_{k,i}^4+(b_{2i}c_{0i}+b_{0i}c_2-c_{1i}b_{1i})\omega_{k,i}^2-b_{0i}c_{0i}}{(c_{0i}-c_2\omega_{k,i}^2)^2+c_{1i}^2\omega_{k,i}^2}\right)+2j\pi\right)}{\omega_{k,i}} | (30) |
for
| \label{Eq4.18} \tau^\ast = \tau_{k_0,0}^{i_0} = \min\limits_{k = {1,2,3},\,i = {1,2,\cdots,N_2}}\tau_{k,0}^i, \quad \quad \omega^\ast = \omega_{k_0,i_0}. | (31) |
Lemma 4.8. Let
| \mathrm{sign}\left \{\frac{d(\mathrm{Re}\lambda(\tau))}{d\tau}\right\}_{\tau = \tau^\ast,\lambda = \mathrm{i}\omega^\ast}>0. |
Proof. We denote
| \label{Eq4.19} P(\lambda)+Q(\lambda)e^{-\lambda\tau} = 0. | (32) |
It is easy to know (26) and (27) are equivalent to the following equations
| \begin{split} &\mathrm{Re}P(\mathrm{i}\omega) = -\mathrm{Re}Q(\mathrm{i}\omega)\cos\omega\tau-\mathrm{Im} Q(\mathrm{i}\omega)\sin\omega\tau,\\ &\mathrm{Im}P(\mathrm{i}\omega) = \mathrm{Re}Q(\mathrm{i}\omega)\sin\omega\tau-\mathrm{Im} Q(\mathrm{i}\omega)\cos\omega\tau. \end{split} |
Thus
| \label{Eq4.20} h(\omega^2) = (\mathrm{Re}P(\mathrm{i}\omega))^2+(\mathrm{Im}P(\mathrm{i}\omega))^2- ((\mathrm{Re}Q(\mathrm{i}\omega))^2+(\mathrm{Im}Q(\mathrm{i}\omega))^2). | (33) |
Differentiating both sides of (33) with respect to
| \label{Eq4.21} 2\omega h^\prime(\omega^2) = \mathrm{i}[P^\prime(\mathrm{i}\omega)\bar{P}(\mathrm{i}\omega)-\bar P^\prime(\mathrm{i}\omega)P(\mathrm{i}\omega)-Q^\prime(\mathrm{i}\omega)\bar{Q}(\mathrm{i}\omega)+\bar Q^\prime(\mathrm{i}\omega)Q(\mathrm{i}\omega)]. | (34) |
Substituting
| \label{Eq4.22}|P(\mathrm{i}\omega^\ast)| = |Q(\mathrm{i}\omega^\ast)|. | (35) |
If
| \begin{split} \mathrm{Im}\tau^\ast = &\mathrm{Im}\left[\frac{Q^\prime(\mathrm{i}\omega^\ast)}{Q(\mathrm{i}\omega^\ast)}-\frac{P^\prime(\mathrm{i}\omega^\ast)}{P(\mathrm{i}\omega^\ast)}\right] = \mathrm{Im}\left[\frac{Q^\prime(\mathrm{i}\omega^\ast)\bar Q(\mathrm{i}\omega^\ast)}{Q(\mathrm{i}\omega^\ast)\bar Q(\mathrm{i}\omega^\ast)}-\frac{P^\prime(\mathrm{i}\omega^\ast)\bar P(\mathrm{i}\omega^\ast)}{P(\mathrm{i}\omega^\ast)\bar P(\mathrm{i}\omega^\ast)}\right]\\ & = \frac{1}{Q(\mathrm{i}\omega^\ast)\bar Q(\mathrm{i}\omega^\ast)}\mathrm{Im}\left[Q^\prime(\mathrm{i}\omega^\ast)\bar Q(\mathrm{i}\omega^\ast)-P^\prime(\mathrm{i}\omega^\ast)\bar P(\mathrm{i}\omega^\ast)\right]\\ & = \frac{Q^\prime(\mathrm{i}\omega^\ast)\bar Q(\mathrm{i}\omega^\ast)-P^\prime(\mathrm{i}\omega^\ast)\bar P(\mathrm{i}\omega^\ast)-\bar{Q}^\prime(\mathrm{i}\omega^\ast)Q(\mathrm{i}\omega^\ast)+\bar{P}^\prime(\mathrm{i}\omega^\ast) P(\mathrm{i}\omega^\ast)}{2\mathrm{i}|Q(\mathrm{i}\omega^\ast)|^2}\\ & = \frac{\omega^\ast h^\prime((\omega^{\ast})^2)}{|Q(\mathrm{i}\omega^\ast)|^2}. \end{split} |
This is a contradiction. Thus
Since
| P^\prime(\mathrm{i}\omega^\ast)+[Q^\prime(\mathrm{i}\omega^\ast)-\tau^\ast Q(\mathrm{i}\omega^\ast)]e^{-\mathrm{i}\omega^\ast\tau^\ast}\neq 0, |
we may consider
| \frac{d\lambda(\tau)}{d\tau} = \frac{\lambda Q(\lambda)}{P^\prime(\lambda)e^{\lambda\tau}+Q^\prime(\lambda)-\tau Q(\lambda)}. |
Using (32) again, we obtain
| \label{Eq4.23} \left(\frac{d\lambda(\tau)}{d\tau}\right)^{-1} = -\frac{P^\prime(\lambda)}{\lambda P(\lambda)}+\frac{Q^\prime(\lambda)}{\lambda Q(\lambda)}-\frac{\tau}{\lambda}. | (36) |
Thus, from (36), we have
| \begin{split} \mathrm{sign}\left \{\frac{d(\mathrm{Re}\lambda(\tau))}{d\tau}\right\}_{\tau = \tau^\ast,\lambda = \mathrm{i}\omega^\ast} = &\mathrm{sign}\left\{\mathrm{Re}\left[-\frac{P^\prime(\lambda)}{\lambda P(\lambda)}+\frac{Q^\prime(\lambda)}{\lambda Q(\lambda)}-\frac{\tau}{\lambda}\right]\right\}_{\tau = \tau^\ast,\lambda = \mathrm{i}\omega^\ast}\\ = &\mathrm{sign}\left\{\mathrm{Re}\bar\lambda\left[Q^\prime(\lambda)\bar{Q(\lambda)}-P^\prime(\lambda)\bar{P(\lambda)}\right]\right\}_{\lambda = \mathrm{i}\omega^\ast}\\ = &\mathrm{sign}\left\{(\omega^\ast)^2h^\prime((\omega^\ast)^2)\right\}>0. \end{split} |
This completes the proof.
When
Theorem 4.9. Assume that either
(ⅰ) The spatially homogeneous positive steady state
(ⅱ) Furthermore, suppose that
Remark 4. There exist parameter values such that hypotheses
In this section, we investigate the direction of Hopf bifurcation and the stability of bifurcated periodic solutions by using the normal form theory and center manifold reduction. For convenience, for fixed
Let
| \dot U(t) = \hat\tau D\triangle U(t)+L(\hat\tau)(U_t)+F(U_t,\mu), \label{Eq4.24} | (37) |
where
| L(\delta)\phi = \delta\left[ \begin{array}{c} (\frac{bu_1^\ast u_3^\ast}{(1+bu_1^\ast+cu_3^\ast)^2}-u_1^\ast)\phi_1(0)-u_1^\ast\phi_2(0)-\frac{(1+bu_1^\ast)u_1^\ast}{(1+bu_1^\ast+cu_3^\ast)^2}\phi_3(0)\\ \beta_1u_2^\ast\phi_1(-1)+\beta_1u_1^\ast\phi_2(-1)-\beta_1u_1^\ast\phi_2(0)-\alpha u_2^\ast\phi_3(0)\\ \frac{\beta_2(1+cu_3^\ast)u_3^\ast}{(1+bu_1^\ast+cu_3^\ast)^2}\phi_1(0)+\beta u_3^\ast\phi_2(0)-\frac{c\beta_2u_1^\ast u_3^\ast}{(1+bu_1^\ast+cu_3^\ast)^2}\phi_3(0) \end{array}\right] |
and
| F(\phi,\mu) = \mu D\Delta\phi(0)+L(\mu)\phi+f(\phi,\mu), |
where
| \begin{split} f(\phi,\mu) = &(\hat\tau+\mu)\times\\ &\left[\begin{array}{l} (\phi_1(0)+u_1^\ast)(-\phi_1(0)-\phi_2(0)-\frac{\phi_3(0)+u_3^\ast}{1+b(\phi_1(0)+u_1^\ast)+c(\phi_3(0)+u_3^\ast)}+\\ \frac{u_3^\ast}{1+bu_1^\ast+cu_3^\ast})-((\frac{bu_1^\ast u_3^\ast}{(1+bu_1^\ast+cu_3^\ast)^2}-u_1^\ast)\phi_1(0)-u_1^\ast\phi_2(0)-\\ \frac{(1+bu_1^\ast)u_1^\ast}{(1+bu_1^\ast+cu_3^\ast)^2}\phi_3(0))\\\\ \beta_1\phi_1(-1)\phi_2(-1)-\alpha \phi_2(0)\phi_3(0)\\\\ (\phi_3(0)+u_3^\ast)(\frac{\beta_2(\phi_1(0)+u_1^\ast)}{1+b(\phi_1(0)+u_1^\ast)+c(\phi_3(0)+u_3^\ast)}+\beta \phi_2(0)-\frac{\beta_2 u_1^\ast}{1+bu_1^\ast+cu_3^\ast})\\ -(\frac{\beta_2(1+cu_3^\ast)u_3^\ast}{(1+bu_1^\ast+cu_3^\ast)^2}\phi_1(0)+\beta u_3^\ast\phi_2(0)-\frac{c\beta_2u_1^\ast u_3^\ast}{(1+bu_1^\ast+cu_3^\ast)^2}\phi_3(0)) \end{array}\right] \end{split} |
for
| \dot U(t) = \hat\tau D\triangle U(t)+L(\hat\tau)(U_t). |
Thus Eq. (37) can be written in the following abstract form
| \frac{dU_t}{dt} = \mathcal{A}U_t+X_0F(U_t,\mu), |
where
| -\hat\tau D\mu_i\phi(0)+L(\hat\tau)(\phi) = \int^0_{-1}d[\eta(\theta, \hat\tau)]\phi(\theta), |
for
| \begin{equation*}\begin{split} \eta(\theta, \hat\tau)=& \hat\tau\left[ \begin{array}{ccc} -d_1\mu_i+\frac{bu_1^\ast u_3^\ast}{(1+bu_1^\ast+cu_3^\ast)^2}-u_1^\ast&-u_1^\ast&-\frac{(1+bu_1^\ast)u_1^\ast}{(1+bu_1^\ast+cu_3^\ast)^2}\\ 0&-d_2\mu_i-\beta_1u_1^\ast&-\alpha u_2^\ast\\ \frac{\beta_2(1+cu_3^\ast)u_3^\ast}{(1+bu_1^\ast+cu_3^\ast)^2}&\beta u_3^\ast&-d_3\mu_i-\frac{c\beta_2u_1^\ast u_3^\ast}{(1+bu_1^\ast+cu_3^\ast)^2} \end{array}\right]\\ &\times\delta(\theta)+\hat\tau\left[ \begin{array}{ccc} 0&0&0\\ -\beta_1u_2^\ast&-\beta_1 u_1^\ast&0\\ 0&0&0 \end{array}\right]\delta(\theta+1), \end{split}\end{equation*} |
where
Let us define
For
| \mathcal{A}(\Phi(\theta)) = \left\{\begin{array}{ll} \frac{d\Phi(\theta)}{d\theta}, \quad \quad \quad \quad \ \theta\in[-1,0),\\ \int_{-1}^0[d\eta(\theta, \hat\tau)]\Phi(\theta), \quad \theta = 0, \end{array}\right. |
and
| \mathcal{A}^\ast(\Psi(s)) = \left\{\begin{array}{ll} -\frac{d\Psi(s)}{ds},&s\in(0,1],\\ \int_{-1}^0\Psi(-\theta)[d\eta(\theta, \hat\tau)],&s = 1. \end{array}\right. |
Then
| \begin{align*}\begin{split} \langle\psi, \phi\rangle = &\psi(0)\phi(0)-\int^0_{-1}\int^\theta_0\psi(\xi-\theta)d[\eta(\theta, \hat\tau)]\phi(\xi)d\xi\\ = &\bar\psi(0)\phi(0)+\hat\tau\int^0_{-1}\bar\psi(\xi+1) \left[ \begin{array}{ccc} 0&0&0\\ \beta_1u_2^\ast&\beta_1u_1^\ast&0\\ 0&0&0 \end{array}\right]\phi(\xi)d\xi, \end{split}\end{align*} |
for
In view of the definition of the two infinitesimal generators
Lemma 4.10. Let
| \begin{split} \eta_1 = &\frac{(d_3\mu_i+M_3)(G_2^2+H_2^2)-\beta u_3^\ast(G_1G_2+H_1H_2)}{u_3^\ast\beta_2A_2(G_2^2+H_2^2)}+\\ &\mathrm{i}\frac{(\hat\omega(G_2^2+H_2^2)-\beta u_3^\ast(G_2H_1-G_1H_2))}{u_3^\ast\beta_2A_2(G_2^2+H_2^2)}, \end{split} |
| \begin{split} \eta_1^\ast = &\frac{-\alpha u_2^\ast(G_3G_4+H_3H_4)-(d_3\mu_i+M_3)(G_4^2+H_4^2)}{u_1^\ast A_1(G_4^2+H_4^2)}-\\ &\mathrm{i}\frac{\alpha u_2^\ast(G_4H_3-G_3H_4)+\hat\omega(G_4^2+H_4^2)}{u_1^\ast A_1(G_4^2+H_4^2)}, \end{split} |
| \eta_2 = \frac{G_1G_2+H_1H_2+\mathrm{i}(G_2H_1-G_1H_2)}{G_2^2+H_2^2},\eta_2^\ast = \frac{G_3G_4+H_3H_4+\mathrm{i}(G_4H_3-G_3H_4)}{G_4^2+H_4^2}, |
where
Then
Proof. The proof is standard and we omit it here.
Clearly, from Lemma 4.10, we know the center subspace of Eq. (37) is
| \mathcal{P} = \mathrm{span}\{ p(\theta), \bar {p(\theta)}\}, \mathcal{P}^\ast = \mathrm{span}\{p^\ast(s), \bar {p^\ast (s)}\}. |
Then
| \mathcal{Q} = \{\psi\in\mathcal{C}: \langle\widehat\psi, \psi\rangle = 0 \quad \mathrm{for}\,\mathrm{all}\, \widehat\psi\in \mathcal{P}^\ast \}. |
It follows from Lemma 4.12 that
| \begin{split} \langle p^\ast(s), p(\theta)\rangle = &\bar{ p^\ast(0)}p(0)+\\ &\hat\tau\int_{-1}^0e^{-\mathrm{i}\hat\omega\hat\tau(\xi+1)}(\bar{\eta^\ast_1}, \bar{\eta^\ast_2}, 1)\left[ \begin{array}{ccc} 0&0&0\\ \beta_1u_2^\ast&\beta_1u_1^\ast&0\\ 0&0&0 \end{array}\right](\eta_1, \eta_2, 1)^Te^{\mathrm{i}\hat\omega\hat\tau\xi}d\xi\\ = &\bar{\eta^\ast_1}\eta_1+\bar{\eta^\ast_2}\eta_2+1+\beta_1u_2^\ast\hat\tau e^{-\mathrm{i}\hat\omega\hat\tau}\eta_1\bar{\eta_2^\ast}+\beta_1u_1^\ast\hat\tau e^{-\mathrm{i}\hat\omega\hat\tau}\eta_2\bar{\eta_2^\ast}. \end{split} |
Thus we choose
In what follows, in order to determine the bifurcation direction and stability, we compute the coordinates to describe the center manifold
| W(z, \bar z)(\theta) = W_{20}(\theta)\frac{z^2}{2}+W_{11}(\theta)z\bar z+W_{02}(\theta)\frac{\bar z^2}{2}+\cdots \label{Eq4.25} | (38) |
with the range in
| U_t = \Phi\cdot(z(t), \bar {z(t)})^T+W(z(t),\bar {z(t)}). \label{Eq4.26} | (39) |
Moreover, from (39),
| \begin{split} \dot z(t) = &\frac{d}{dt}\langle q(s), U_t\rangle = \langle q(s), \mathcal{A}U_t\rangle+\langle q(s), X_0F(U_t,0)\rangle\\ = &\mathrm{i}\hat\omega\hat\tau z+\bar {q(0)}f(W(z,\bar z)+2\mathrm{Re}\{zp(\theta)\},0)\\ = &\mathrm{i}\hat\omega\hat\tau z+g(z,\bar z), \label{Eq4.27} \end{split} | (40) |
where
| \begin{split} g(z, \bar z) = g_{20}\frac{z^2}{2}+g_{11}z\bar z+g_{02}\frac{\bar z^2}{2}+g_{21}\frac{z^2\bar z}{2}+\cdot\cdot\cdot. \end{split} |
By the Taylor expansion
| \begin{array}{l} \frac{{v + {v^ * }}}{{1 + b(u + {u^ * }) + c(v + {v^ * })}} = \frac{{{v^ * }}}{{1 + b{u^ * } + c{v^ * }}} - \frac{{b{v^ * }u}}{{{{(1 + b{u^ * } + c{v^ * })}^2}}} + \frac{{(1 + b{u^ * })v}}{{{{(1 + b{u^ * } + c{v^ * })}^2}}} + \\ {C_{11}}{u^2} + {C_{12}}uv + {C_{13}}{v^2} + {C_{14}}{u^2}v + {C_{15}}u{v^2} + \\ {C_{16}}{u^3} + {C_{17}}{v^3} + O(4),\\ \frac{{u + {u^ * }}}{{1 + b(u + {u^ * }) + c(v + {v^ * })}} = \frac{{{u^ * }}}{{1 + b{u^ * } + c{v^ * }}} + \frac{{(1 + c{v^ * })u}}{{{{(1 + b{u^ * } + c{v^ * })}^2}}} - \frac{{c{u^ * }v}}{{{{(1 + b{u^ * } + c{v^ * })}^2}}}\\ + {C_{21}}{u^2} + {C_{22}}uv + {C_{23}}{v^2} + {C_{24}}{u^2}v + {C_{25}}u{v^2} + \\ {C_{26}}{u^3} + {C_{27}}{v^3} + O(4), \end{array} | (41) |
where
| \begin{array}{l} {C_{11}} = \frac{{{b^2}{v^ * }}}{{{{(1 + b{u^ * } + c{v^ * })}^3}}},{C_{12}} = \frac{{bc{v^ * } - b(1 + b{u^ * })}}{{{{(1 + b{u^ * } + c{v^ * })}^3}}},{C_{13}} = - \frac{{c(1 + b{u^ * })}}{{{{(1 + b{u^ * } + c{v^ * })}^3}}},\\ {C_{14}} = \frac{{{b^2}(1 + b{u^ * } - 2c{v^ * })}}{{{{(1 + b{u^ * } + c{v^ * })}^4}}},{C_{15}} = \frac{{bc(2(1 + b{u^ * }) - c{v^ * })}}{{{{(1 + b{u^ * } + c{v^ * })}^4}}},{C_{16}} = \frac{{ - {b^3}{v^ * }}}{{{{(1 + b{u^ * } + c{v^ * })}^4}}}, \end{array} |
| \begin{array}{l} {C_{17}} = \frac{{{c^3}(1 + b{u^ * })}}{{{{(1 + b{u^ * } + c{v^ * })}^4}}},{C_{21}} = - \frac{{b(1 + c{v^ * })}}{{{{(1 + b{u^ * } + c{v^ * })}^3}}},{C_{22}} = \frac{{bc{u^ * } - c(1 + c{v^ * })}}{{{{(1 + b{u^ * } + c{v^ * })}^3}}},\\ {C_{23}} = \frac{{{c^2}{u^ * }}}{{{{(1 + b{u^ * } + c{v^ * })}^3}}},{C_{24}} = \frac{{bc(2(1 + c{v^ * }) - b{u^ * })}}{{{{(1 + b{u^ * } + c{v^ * })}^4}}},{C_{25}} = \frac{{{c^2}(1 + c{v^ * } - 2b{u^ * })}}{{{{(1 + b{u^ * } + c{v^ * })}^4}}},\\ {C_{26}} = \frac{{{b^3}(1 + c{v^ * })}}{{{{(1 + b{u^ * } + c{v^ * })}^4}}},{C_{27}} = - \frac{{{c^3}{u^ * }}}{{{{(1 + b{u^ * } + c{v^ * })}^4}}}. \end{array} |
Noting that (40), we get
| g(z,\bar z) = \bar {\mathfrak{D}}(\bar{\eta_1^\ast}, \bar{\eta_2^\ast}, 1)f(W(z,\bar z)+zp(\theta)+\bar z\bar {p(\theta)},0). \label{Eq4.29} | (42) |
Substituting (38) into (42) and combining (41) yield
| \begin{split} g_{20} = &2\bar{\mathfrak{D}}\hat\tau[\bar{\eta_1^\ast}\left(({bA_3}/{u_1^\ast}-C_{11}u_1^\ast-1)\eta_1^2-\eta_1\eta_2-C_{13}u_1^\ast-(A_1+C_{12}u_1^\ast)\eta_1\right) +\\ &\bar{{\eta_2^\ast}}(\beta_1\eta_1\eta_2e^{-2\mathrm{i}\hat\omega\hat\tau}-\alpha\eta_2) +\bar{{\eta_3^\ast}}(u_3^\ast\beta_2C_{21}\eta_1^2+C_{23}u_3^\ast\beta_2-M_3/u_3^\ast+\\ &(\beta_2A_2+\beta_2C_{22}u_3^\ast)\eta_1+\beta\eta_2)], \end{split} |
| \begin{split} g_{11} = &\bar{\mathfrak{D}}\hat\tau[\bar{\eta_1^\ast}(2({bA_3}/{u_1^\ast}-C_{11}u_1^\ast-1)\eta_1\bar{\eta_1}-(\eta_1\bar{\eta_2}+\eta_2\bar{\eta_1})-2C_{13}u_1^\ast-\\ &(A_1+C_{12}u_1^\ast)(\eta_1+\bar{\eta_1}))+\bar{\eta_2^\ast}(\beta_1(\eta_1\bar{\eta_2}+\bar{\eta_1}\eta_2)-\alpha(\eta_2+\bar{\eta_2}))+\\ &\bar{\eta_3^\ast}(2u_3^\ast\beta_2C_{21}\eta_1\bar{\eta_1}+2(C_{23}u_3^\ast\beta_2-M_3/u_3^\ast)+\\ &(\beta_2A_2+\beta_2C_{22}u_3^\ast)(\eta_1+\bar{\eta_1})+\beta(\eta_2+\bar{\eta_2}))], \end{split} |
| \begin{split} g_{21} = &2\bar{\mathfrak{D}}\hat\tau[\bar{\eta_1^\ast}(2({bA_3}/{u_1^\ast}-C_{11}u_1^\ast-1)(\eta_1W_{11}^{(1)}(0)+\frac{\bar{\eta_1}}{2}W_{20}^{(1)}(0)) -(\frac{W_{20}^{(1)}(0)}{2}\bar{\eta_2}+\\ &W_{11}^{(2)}(0)\eta_1+W_{11}^{(1)}(0)\eta_2+\frac{W_{20}^{(2)}(0)}{2}\bar{\eta_1})-2C_{13}u_1^\ast(W_{11}^{(3)}(0)+\frac{W_{20}^{(3)}(0)}{2})-\\ &(A_1+C_{12}u_1^\ast)(\frac{W_{20}^{(1)}(0)}{2}+W_{11}^{(1)}(0)+W_{11}^{(3)}(0)\eta_1+\frac{W_{20}^{(3)}(0)}{2}\bar{\eta_1})-\\ &3(C_{11}+C_{16}u_1^\ast)\eta_1\eta_1\bar{\eta_1}-(C_{12}+C_{14}u_1^\ast)(\eta_1\eta_1+\eta_1\bar{\eta_1}+\bar{\eta_1}\eta_1)-3C_{17}u_1^\ast-\\ &(C_{13}+C_{15}u_1^\ast)(2\eta_1+\bar{\eta_1}))+\bar{\eta_2^\ast}(\beta_1\eta_1W_{11}^{2}(-1)e^{-\mathrm{i}\hat\omega\hat\tau}+ \beta_1\bar{\eta_1}e^{\mathrm{i}\hat\omega\hat\tau}\frac{W_{20}^{2}(-1)}{2}+\\ &\beta_1\bar{\eta_2}e^{\mathrm{i}\hat\omega\hat\tau}\frac{W_{20}^{1}(-1)}{2}+\beta_1\eta_2W_{11}^{1}(-1)e^{-\mathrm{i}\hat\omega\hat\tau}-\alpha\eta_2W_{11}^{(3)}(0) -\alpha\bar{\eta_2}\frac{W_{20}^{(3)}(0)}{2}-\\ &\alpha\frac{W_{20}^{(2)}(0)}{2}-\alpha W_{11}^{(2)}(0))+\bar{\eta_3^\ast}(2u_3^\ast\beta_2C_{21}(W_{11}^{(1)}(0)\eta_1+\frac{\bar {\eta_1}}{2}W_{20}^{(1)}(0))+\\ &2(C_{23}u_3^\ast\beta_2-M_3/u_3^\ast)(W_{11}^{(3)}(0)+\frac{W_{20}^{(3)}(0)}{2})+(\beta_2A_2+\beta_2C_{22}u_3^\ast)(\frac{W_{20}^{(1)}(0)}{2}+\\ &\eta_1W_{11}^{(3)}(0)+\bar{\eta_1}\frac{W_{20}^{(3)}(0)}{2}+W_{11}^{(1)}(0))+\beta(\eta_2W_{11}^{(3)}(0)+\bar{\eta_2}\frac{W_{20}^{(3)}(0)}{2} + \end{split} |
| \begin{split}&\frac{W_{20}^{(2)}(0)}{2}+W_{11}^{(2)}(0))+3C_{26}u_3^\ast\beta_2\eta_1\eta_1\bar{\eta_1}+3(C_{23}\beta_2+u_3^\ast\beta_2C_{27})+\\ &(C_{21}\beta_2+u_3^\ast\beta_2C_{24})(\eta_1^2+2\eta_1\bar{\eta_1})+(C_{22}\beta_2+u_3^\ast\beta_2C_{25})(2\eta_1+\bar{\eta_1}))], \end{split} |
| g_{02} = 2\bar{g_{20}}\bar{\mathfrak{D}}/\mathfrak{D}. |
Since
| \begin{split} \dot W = &\mathcal{A}W+X_0f(\Phi\cdot(z, \bar z)^T+W(z,\bar z),0)-\Phi\Psi(0)f(\Phi\cdot(z,\bar z)^T+W(z,\bar z),0)\\ = &\mathcal{A}W+H_{20}\frac{z^2}{2}+H_{11}z\bar z+H_{02}\frac{\bar z^2}{2}+\cdot\cdot\cdot \label{Eq4.30} \end{split} | (43) |
we obtain
| \left\{\begin{array}{ll} (2\mathrm{i}\hat\omega\hat\tau-\mathcal{A})W_{20} = H_{20},\\ -\mathcal{A}W_{11} = H_{11},\\ (-2\mathrm{i}\hat\omega\hat\tau-\mathcal{A})W_{02} = H_{02}. \end{array}\right. \label{Eq4.31} | (44) |
Since
We first compute
| H(z, \bar z) = -\Phi\Psi(0)f(\Phi\cdot(z,\bar z)^T+W(z,\bar z), 0). |
Therefore, by comparing the coefficients, and notice that
| H(z, \bar z)(0) = f(\Phi\cdot(z, \bar z)^T+W(z,\bar z), 0)-\Phi\Psi(0)f(\Phi\cdot(z,\bar z)^T+W(z,\bar z), 0), |
we obtain
| \begin{split} &H_{20}(\theta) = \\ &\left\{\begin{array}{ll} -(g_{20}p(\theta)+\bar{g_{02}}\bar{p(\theta)}),\quad \quad \theta\in[-1,0),\\ 2\hat\tau\left[\begin{array}{c} ({bA_3}/{u_1^\ast}-C_{11}u_1^\ast-1)\eta_1^2-\eta_1\eta_2-C_{13}u_1^\ast-(A_1+C_{12}u_1^\ast)\eta_1\\ \beta_1\eta_1\eta_2e^{-2\mathrm{i}\hat\omega\hat\tau}-\alpha\eta_2\\ u_3^\ast\beta_2C_{21}\eta_1^2+C_{23}u_3^\ast\beta_2-M_3/u_3^\ast+(\beta_2A_2+\beta_2C_{22}u_3^\ast)\eta_1+\beta\eta_2 \end{array}\right]\\ -[g_{20}p(0)+\bar{g_{02}}\bar {p(0)}], \quad \quad \theta = 0. \end{array}\right. \end{split} |
| \begin{split} &H_{11}(\theta) = \\ &\left\{\begin{array}{ll} -(g_{11}p(\theta)+\bar{g_{11}}\bar{p(\theta)}),\quad \quad \theta\in[-1,0),\\ 2\hat\tau\left[\begin{array}{c} (\frac{bA_3}{u_1^\ast}-C_{11}u_1^\ast-1)\eta_1\bar{\eta_1}-\mathrm{Re}\{\eta_1\bar{\eta_2}\}-C_{13}u_1^\ast -(A_1+C_{12}u_1^\ast)\mathrm{Re}\{\eta_1\}\\ \beta_1\mathrm{Re}\{\eta_1\bar{\eta_2}\}-\alpha\mathrm{Re}\{\eta_2\}\\ u_3^\ast\beta_2C_{21}\eta_1\bar{\eta_1}+C_{23}u_3^\ast\beta_2-\frac{M_3}{u_3^\ast}+(\beta_2A_2+\beta_2C_{22}u_3^\ast)\mathrm{Re}\{\eta_1\}+\beta\mathrm{Re}\{\eta_2\} \end{array}\right]\\ -[g_{11}p(0)+\bar{g_{11}}\bar{p(0)}], \quad \quad \theta = 0. \end{array}\right. \end{split} |
It follows from (44) and the definition of
| \begin{split} &\dot W_{20}(\theta) = 2\mathrm{i}\omega_n\hat\tau W_{20}(\theta)+[g_{20}p(\theta)+\bar{g_{02}}\bar p(\theta)], -1\leq\theta\leq 0,\\ &-\dot W_{11}(\theta) = -[g_{11}p(\theta)+\bar{g_{11}}\bar{p(\theta)}], -1\leq \theta\leq 0. \end{split} |
Noting that
| \begin{split} &W_{20}(\theta) = [\frac{\mathrm{i}g_{20}}{\hat\omega\hat\tau}p(\theta)+\frac{\mathrm{i}\bar{g_{02}}}{3\hat\omega\hat\tau}\bar{p(\theta)}] +E_1e^{2\mathrm{i}\hat\omega\hat\tau\theta},\\ &W_{11}(\theta) = [\frac{-\mathrm{i}g_{11}}{\hat\omega\hat\tau}p(\theta)+\frac{\mathrm{i}\bar{g_{11}}}{\hat\omega\hat\tau}\bar{p(\theta)}] +E_2. \label{Eq4.32} \end{split} | (45) |
Utilizing the definition of
| \begin{split} &E_1 = 2\left[\begin{array}{c} ({bA_3}/{u_1^\ast}-C_{11}u_1^\ast-1)\eta_1^2-\eta_1\eta_2-C_{13}u_1^\ast-(A_1+C_{12}u_1^\ast)\eta_1\\ \beta_1\eta_1\eta_2e^{-2\mathrm{i}\hat\omega\hat\tau}-\alpha\eta_2\\ u_3^\ast\beta_2C_{21}\eta_1^2+C_{23}u_3^\ast\beta_2-M_3/u_3^\ast+(\beta_2A_2+\beta_2C_{22}u_3^\ast)\eta_1+\beta\eta_2 \end{array}\right]\times\\ &\left[ \begin{array}{ccc} 2\mathrm{i}\hat\omega+d_1\mu_i+M_1&u_1^\ast&u_1^\ast A_1\\ -\beta_1u_2^\ast e^{-2\mathrm{i}\hat\omega\hat\tau}&2\mathrm{i}\hat\omega+d_2\mu_i+M_2(1-e^{-2\mathrm{i}\hat\omega\hat\tau})&\alpha u_2^\ast\\ -\beta_2A_2u_3^\ast &-\beta u_3^\ast&2\mathrm{i}\hat\omega+d_3\mu_i+M_3 \end{array}\right ]^{-1}\\ \end{split} |
and
| \begin{array}{l} {E_2} = 2{\left[ {\begin{array}{*{20}{c}} {{d_1}{\mu _i} + u_1^ * - b{A_3}}&{u_1^ * }&{u_1^ * {A_1}}\\ { - {\beta _1}u_2^ * }&{{d_2}{\mu _i}}&{\alpha u_2^ * }\\ { - {\beta _2}{A_2}u_3^ * }&{ - \beta u_3^ * }&{{d_3}{\mu _i} + c{\beta _2}{A_3}} \end{array}} \right]^{ - 1}} \times \\ \quad \left[ {\begin{array}{*{20}{c}} {(\frac{{b{A_3}}}{{u_1^ * }} - {C_{11}}u_1^ * - 1){\eta _1}\overline {{\eta _1}} - {\rm{Re}}\{ {\eta _1}\overline {{\eta _2}} \} - {C_{13}}u_1^ * - ({A_1} + {C_{12}}u_1^ * ){\rm{Re}}\{ {\eta _1}\} }\\ {{\beta _1}{\rm{Re}}\{ {\eta _1}\overline {{\eta _2}} \} - \alpha {\rm{Re}}\{ {\eta _2}\} }\\ {u_3^ * {\beta _2}{C_{21}}{\eta _1}\overline {{\eta _1}} + {C_{23}}u_3^ * {\beta _2} - \frac{{{M_3}}}{{u_3^ * }} + ({\beta _2}{A_2} + {\beta _2}{C_{22}}u_3^ * ){\rm{Re}}\{ {\eta _1}\} + \beta {\rm{Re}}\{ {\eta _2}\} } \end{array}} \right]. \end{array} |
Now, we can compute the following values
| \begin{split} &c_1(0) = \frac{\mathrm{i}}{2\hat\omega\hat\tau}(g_{20}g_{11}-2|g_{11}|^2-\frac{|g_{02}|^2}{3})+\frac{ g_{21}}{2}, \nu_2 = -\frac{\mathrm{Re}(c_1(0))}{\mathrm{Re}(\lambda^\prime(\hat\tau))},\\ &\beta_2 = 2\mathrm{Re}(c_1(0)), T_2 = -\frac{\mathrm{Im}(c_1(0))+\nu_2\mathrm{Im}(\lambda^\prime(\hat\tau))}{\hat\omega\hat\tau}, \end{split} |
which determine the properties of bifurcating periodic solutions at critical value
Theorem 4.11. Assume that the conditions of Theorem 4.5 are satisfied, we have
(ⅰ) If
(ⅱ)If
(ⅲ) If
In this subsection, we numerically explore the dynamic behavior of System (4) with one-dimensional space, namely
For the choice of the parameter values in System (4), we refer to [10,11,12,15] and choose parameter values as follows
and the initial conditions as
With these parameter values, System (4) admits a unique positive spatially homogeneous steady state
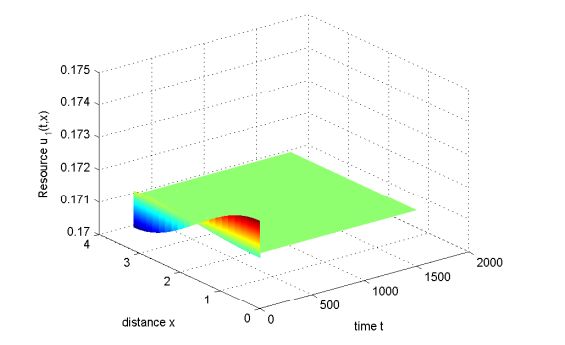 Figure 1. Numerical solutions of (4) with
Figure 1. Numerical solutions of (4) with 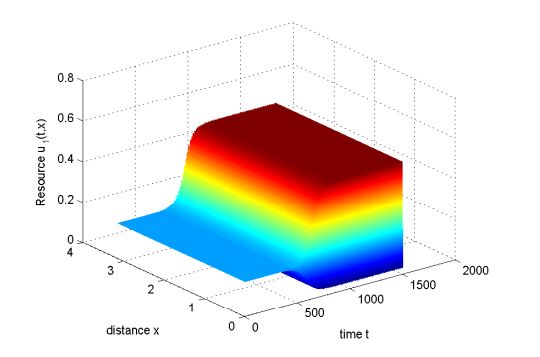 Figure 2. Numerical solutions of (4) with
Figure 2. Numerical solutions of (4) with Consider System (4) with
| \begin{split} \Delta u|_{(i,j)} = &\frac{1}{s^2}[A_l(i,j)u(i-1,j)+A_r(i,j)u(i+1,j)+\\ &A_d(i,j)u(i,j-1)+A_u(i,j)u(i,j+1)-4u(i,j)], \end{split} |
where
With the given Neumann boundary conditions, the eigenvalues of
We consider two types of different initial conditions:
and
| (IC_2^\prime):\left\{\begin{array}{lll} u_1(t,x,y) = 0.1754-\varepsilon_1(x-0.1y-225)(x-0.1y-675),\\ u_2(t,x,y) = 0.7419-\varepsilon_2(x-450)-\varepsilon_3(y-150),\\ u_3(t,x,y) = 0.2029-\varepsilon_4(x-350)-\varepsilon_5(y-200)\\ \end{array}\right. |
for
Under
Figure 3 depicts the population dynamics of the temporal model and the spatiotemporal model at
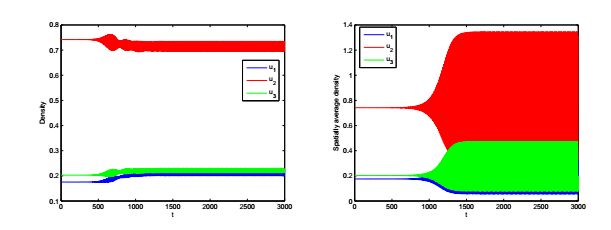 Figure 3. Numerical solutions of the temporal model (left) and numerical solutions of the spatiotemporal model (right) with
Figure 3. Numerical solutions of the temporal model (left) and numerical solutions of the spatiotemporal model (right) with If we increase
 Figure 4. Numerical solutions of the temporal model (left) and numerical solutions of the spatiotemporal model (right) with
Figure 4. Numerical solutions of the temporal model (left) and numerical solutions of the spatiotemporal model (right) with Figure Figure 5 depicts the snapshots of the contour maps of specie
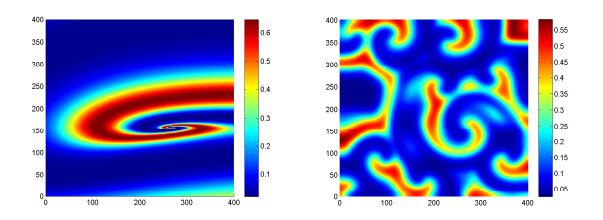 Figure 5. Snapshots of contour maps of the basal resource
Figure 5. Snapshots of contour maps of the basal resource 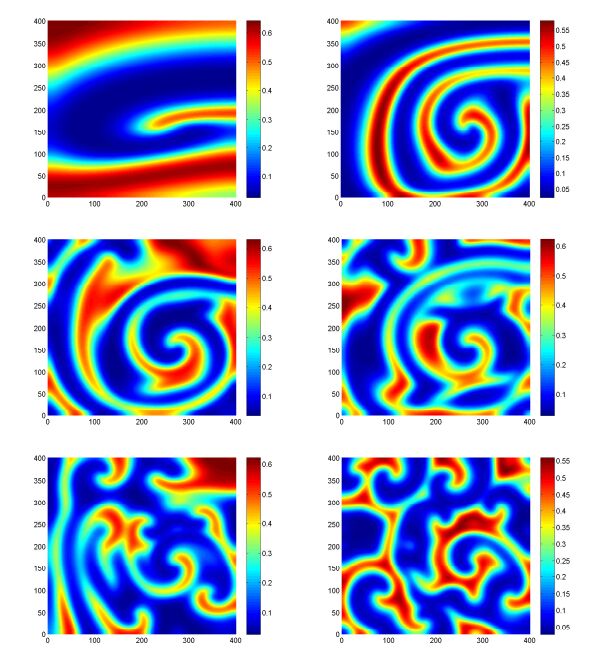 Figure 6. Snapshots of contour maps of the time evolution of the specie
Figure 6. Snapshots of contour maps of the time evolution of the specie To explore the impact of delay, in Figure Figure 7, we take the snapshots of the contour maps of specie
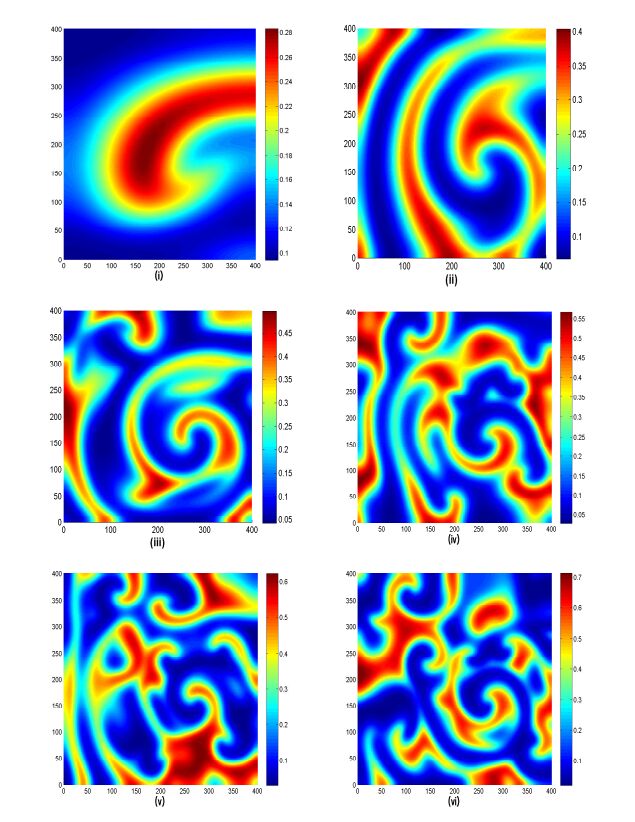 Figure 7. Snapshots of contour maps of the time evolution of the basal resource
Figure 7. Snapshots of contour maps of the time evolution of the basal resource As seen from Figure Figure 6, System (4) has a regular spiral wave pattern when
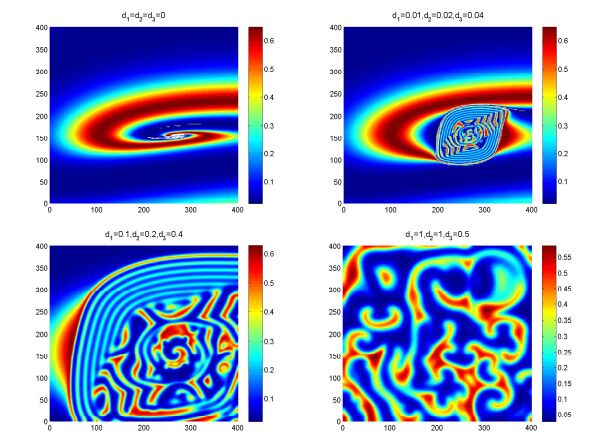 Figure 8. Snapshots of contour maps of the basal resource
Figure 8. Snapshots of contour maps of the basal resource Figure 9 demonstrates how the prey saturation constant constant
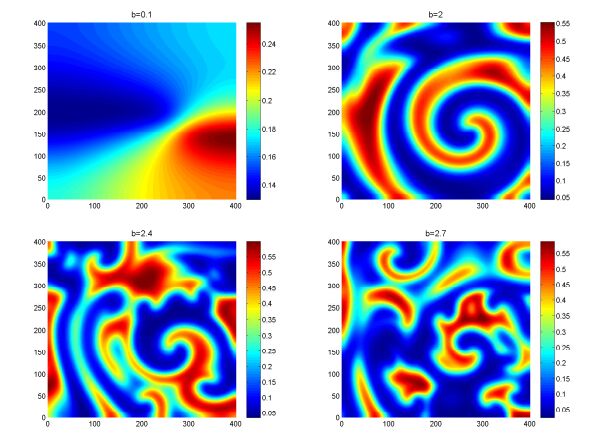 Figure 9. Snapshots of contour maps of the time evolution of the basal resource
Figure 9. Snapshots of contour maps of the time evolution of the basal resource To see how the predator interference constant
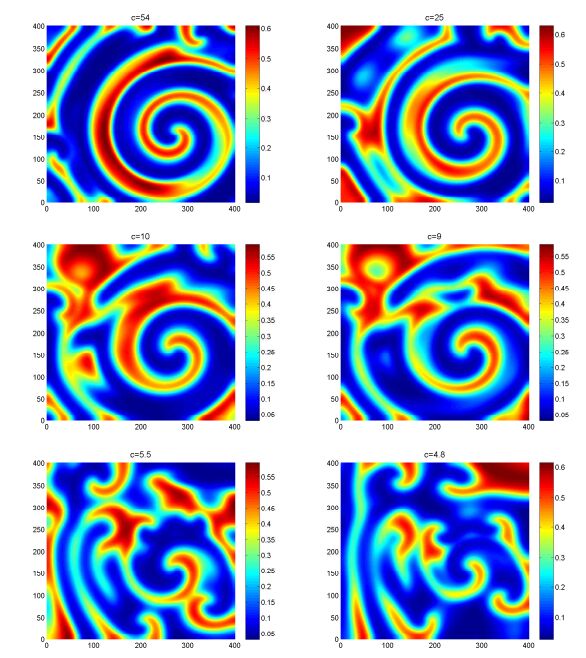 Figure 10. 10. Snapshots of contour maps of the time evolution of the basal resource
Figure 10. 10. Snapshots of contour maps of the time evolution of the basal resource In this work, we have investigated the spatiotemporal dynamics of a diffusive IGP model with delay and the Beddington-DeAngelis functional response. we have established locally asymptotically stability results of the trivial, semi-trivial and strong semi-trivial steady states. In the case that there is a unique positive spatially homogeneous steady state
Compared with the temporal model in [24], we also observe bistability is possible in System (4). In addition, the diffusion also has impacts on the formation of spatiotemporal patterns as it can change the distribution of characteristic roots of the corresponding characteristic equations, and hence has an important effect on the dynamics for the constant steady state of System (4). This has been illustrated via numerical simulations as well (See Figure 8). Moreover, we have observed that the functional response can also influence the formation of complex patterns. As demonstrated in Figures 9 and 10, the functional responses can also trigger the emergence of spiral wave pattern and chaotic wave spatial pattern.
The authors were very grateful to two anonymous reviewers' very helpful comments and suggestions. The project was partially supported by the National Natural Science Foundation of China (No.51479215, No. 11401577) and the Natural Sciences and Engineering Research Council of Canada (RGPIN-2015-05686).
| [1] | [ J. D. Murray, Mathematical Biology II: Spatial Models and Biomedical Applications, Spring-Verlag, New York, 2003. |
| [2] | [ G. A. Polis,C. A. Myers,R. D. Holt, The ecology and evolution of intraguild predation: Potential competitors that each other, Ann. Rev. Ecol. Sys., 20 (1989): 297-330. |
| [3] | [ M. H. Posey,A. H. Hines, Complex predator-prey interactions within an estuarine benthic community, Ecol., 72 (1991): 2155-2169. |
| [4] | [ G. A. Polis,R. D. Holt, Intraguild predation: The dynamics of complex trophic interactions, Trends Ecol. Evol., 7 (1992): 151-154. |
| [5] | [ R. D. Holt,G. A. Polis, A theoretical framework for intraguild predation, Am. Nat., 149 (1997): 745-764. |
| [6] | [ M. Arim,P. A. Marquet, Intraguild predation: A widespread interaction related to species biology, Ecol. Let., 7 (2004): 557-564. |
| [7] | [ P. Amarasekare, Trade-offs, temporal, variation, and species coexistence in communities with intraguild predation, Ecol., 88 (2007): 2720-2728. |
| [8] | [ R. Hall, Intraguild predation in the presence of a shared natural enemy, Ecol., 92 (2011): 352-361. |
| [9] | [ Y. S. Wang,D. L. DeAngelis, Stability of an intraguild predation system with mutual predation, Commun. Nonlinear Sci. Numer. Simulat., 33 (2016): 141-159. |
| [10] | [ I. Velazquez,D. Kaplan,J. X. Velasco-Hernandez,S. A. Navarrete, Multistability in an open recruitment food web model, Appl. Math. Comp., 163 (2005): 275-294. |
| [11] | [ S. B. Hsu,S. Ruan,T. H. Yang, Analysis of three species Lotka-Volterra food web models with omnivory, J. Math. Anal. Appl., 426 (2015): 659-687. |
| [12] | [ P. A. Abrams,S. R. Fung, Prey persistence and abundance in systems with intraguild predation and type-2 functional response, J. Theor. Biol., 264 (2010): 1033-1042. |
| [13] | [ A. Verdy,P. Amarasekare, Alternative stable states in communities with intraguild predatiion, J. Theor. Biol., 262 (2010): 116-128. |
| [14] | [ M. Freeze,Y. Chang,W. Feng, Analysis of dynamics in a complex food chain with ratio-dependent functional response, J. Appl. Anal. Comput., 4 (2014): 69-87. |
| [15] | [ Y. Kang,L. Wedekin, Dynamics of a intraguild predation model with generalist or specialist predator, J. Math. Biol., 67 (2013): 1227-1259. |
| [16] | [ H. I. Freedman,V. S. H. Rao, Stability criteria for a system involving two time delays, SIAM J. Appl. Math., 46 (1986): 552-560. |
| [17] | [ G. S. K. Wolkowicz,H. X. Xia, Global asymptotic behavior of chemostat model with discrete delays, SIAM J. Appl. Math., 57 (1997): 1019-1043. |
| [18] | [ Y. L. Song,M. A. Han,J. J. Wei, Stability and Hopf bifurcation analysis on a simplified BAM neural network with delays, Physica D, 200 (2005): 185-204. |
| [19] | [ S. Ruan, On nonlinear dynamics of predator-prey models with discrete delay, Math. Mod. Nat. Phen., 4 (2009): 140-188. |
| [20] | [ X. Y. Meng,H. F. Huo,X. B. Zhao,H. Xiang, Stability and Hopf bifurcation in a three-species system with feedback delays, Nonlinear Dyn., 64 (2011): 349-364. |
| [21] | [ M. Y. Li,H. Shu, Multiple stable periodic oscillations in a mathematical model of CTL-response to HTLV-I infection, Bull. Math. Biol., 73 (2011): 1774-1793. |
| [22] | [ H. Shu,L. Wang,J. Watmough, Sustained and transient oscillations and chaos induced by delayed antiviral inmune response in an immunosuppressive infective model, J. Math. Biol., 68 (2014): 477-503. |
| [23] | [ M. Yamaguchi,Y. Takeuchi,W. Ma, Dynamical properties of a stage structured three-species model with intra-guild predation, J. Comput. Appl. Math., 201 (2007): 327-338. |
| [24] | [ H. Shu,X. Hu,L. Wang,J. Watmough, Delayed induced stability switch, multitype bistability and chaos in an intraguild predation model, J. Math. Biol., 71 (2015): 1269-1298. |
| [25] | [ A. Okubo and S. A. Levin, Diffusion and Ecological Problems: Modern perspectives, Springer-Verlag, New York, 2001. |
| [26] | [ T. Faria, Stability and bifurcation for a delayed predator-prey model and the effect of diffusion, J. Math. Anal. Appl., 254 (2001): 433-463. |
| [27] | [ C. V. Pao, Systems of parabolic equations with continuous and discrete delays, J. Math. Anal. Appl., 205 (1997): 157-185. |
| [28] | [ C. V. Pao, Convergence of solutions of reaction-diffusion systems with time delays, Nonlinear Anal., 48 (2002): 349-362. |
| [29] | [ J. Wang,J. P. Shi,J. J. Wei, Dyanmics and pattern formation in a diffusive predator-prey system with strong Allee effect in prey, J. Diff. Equat., 251 (2011): 1276-1304. |
| [30] | [ C. Tian, Delay-driven spatial patterns in a plankton allelopathic system, Chaos, 22(2012), 013129, 7 pp. |
| [31] | [ C. Tian,L. Zhang, Hopf bifurcation analysis in a diffusive food-chain model with time delay, Comput. Math. Appl., 66 (2013): 2139-2153. |
| [32] | [ W. Zuo,J. Wei, Global stability and Hopf bifurcations of a Beddington-DeAngelis type predator-prey system with diffusion and delay, Appl. Math. Comput., 223 (2013): 423-435. |
| [33] | [ J. Zhao,J. Wei, Dynamics in a diffusive plankton system with delay and toxic substances effect, Nonlinear Anal., 22 (2015): 66-83. |
| [34] | [ L. Zhu,H. Zhao,X. M. Wang, Bifurcation analysis of a delay reaction-diffusion malware propagation model with feedback control, Commun. Nonlinear Sci. Numer. Simulat., 22 (2015): 747-768. |
| [35] | [ Y. Li,M. X. Wang, Hopf bifurcation and global stability of a delayed predator-prey model with prey harvesting, Comput. Math. Appl., 69 (2015): 398-410. |
| [36] | [ H. Y. Zhao,X. Zhang,X. Huang, Hopf bifurcation and spatial patterns of a delayed biological economic system with diffusion, Appl. Math. Comput., 266 (2015): 462-480. |
| [37] | [ Q. X. Ye, Z. Y. Li, M. X. Wang and Y. P. Wu, Introduction to Reaction-diffusion Equations (Second Edition), Science Press, Bei Jing, 2011. |
| [38] | [ D. Henry, Geometric Theory of Semilinear Parabolic Equations, Springer-Verlag, Berlin/New York, 1981. |
| [39] | [ S. Ruan,J. Wei, On the zeros of a third degree exponential polynomial with applications to a delayed model for the control of testoterone secretion, Math. Med. Biol., 18 (2001): 41-52. |
| [40] | [ J. Wu, Theory and Applications of Partial Functional Differential Equations, Springer-Verlag, New York, 1996. |
| [41] | [ B. Hassard, N. Kazarinoff and Y. Wan, Theory and Applications of Hopf bifurcation, Cambridge University Press, Cambridge, 1981. |
| [42] | [ J. Y. Wakano,C. Hauert, Pattern formation and chaos in spatial ecological public goods games, J. Theor. Biol., 268 (2011): 30-38. |
| [43] | [ M. Banerjee, S. Ghoral and N. Mukherjee, Approximated spiral and target patterns in Bazykin's prey-predator model: Multiscale perturbation analysis, Int. J. Bifurcat. Chaos, 27 (2017), 1750038, 14 pp. |
| [44] | [ H. Malchow, S. V. Petrovskii and E. Venturino, Spatiotemporal Patterns in Ecology and Epidemiology: Theory, Models, Simulations, Chapman & Hall / CRC Press, 2008. |
| [45] | [ Q. Ouyang, Pattern Formation in Reaction-Diffusion Systems Shanghai Scientific and Technological Education Publishing House, SHANGHAI, 2000. |
| 1. | Jingen Yang, Sanling Yuan, Tonghua Zhang, Complex dynamics of a predator–prey system with herd and schooling behavior: with or without delay and diffusion, 2021, 0924-090X, 10.1007/s11071-021-06343-0 | |
| 2. | Dawei Zhang, Binxiang Dai, Spreading and vanishing in a diffusive intraguild predation model with intraspecific competition and free boundary, 2019, 42, 0170-4214, 6917, 10.1002/mma.5797 | |
| 3. | Dawei Zhang, Binxiang Dai, A free boundary problem for the diffusive intraguild predation model with intraspecific competition, 2019, 474, 0022247X, 381, 10.1016/j.jmaa.2019.01.050 | |
| 4. | Dawei Zhang, Binxiang Dai, The diffusive intraguild predation model with intraspecific competition and double free boundaries, 2020, 0003-6811, 1, 10.1080/00036811.2020.1716971 | |
| 5. | Zhenzhen Li, Binxiang Dai, ANALYSIS OF DYNAMICS IN A GENERAL INTRAGUILD PREDATION MODEL WITH INTRASPECIFIC COMPETITION, 2019, 9, 2156-907X, 1493, 10.11948/2156-907X.20180296 | |
| 6. | Renji Han, Binxiang Dai, Yuming Chen, Pattern formation in a diffusive intraguild predation model with nonlocal interaction effects, 2019, 9, 2158-3226, 035046, 10.1063/1.5084948 | |
| 7. | Juping Ji, Russell Milne, Hao Wang, Stoichiometry and environmental change drive dynamical complexity and unpredictable switches in an intraguild predation model, 2023, 86, 0303-6812, 10.1007/s00285-023-01866-z | |
| 8. | Zhenzhen Li, Binxiang Dai, Renji Han, Hopf Bifurcation in a Reaction–Diffusion–Advection Population Model with Distributed Delay, 2022, 32, 0218-1274, 10.1142/S0218127422502479 | |
| 9. | Juping Ji, Lin Wang, Competitive exclusion and coexistence in an intraguild predation model with Beddington–DeAngelis functional response, 2022, 107, 10075704, 106192, 10.1016/j.cnsns.2021.106192 | |
| 10. | Renji Han, Global classical solvability and stabilization in a reaction–diffusion intraguild predation model with chemotaxis, 2022, 73, 0044-2275, 10.1007/s00033-022-01777-x | |
| 11. | 小宁 王, Stability and Bifurcation Analysis of a Spatiotemporal Intraguild PredationModel with Fear Effect and Beddington-DeAngelis Functional Response, 2022, 12, 2160-7583, 2081, 10.12677/PM.2022.1212225 | |
| 12. | Heping Jiang, Stable spatially inhomogeneous periodic solutions for a diffusive Leslie–Gower predator–prey model, 2024, 1598-5865, 10.1007/s12190-024-02018-2 | |
| 13. | Renji Han, Sanaa Moussa Salman, Nonlinear dynamics and pattern formation in a space-time discrete diffusive intraguild predation model, 2024, 01672789, 134295, 10.1016/j.physd.2024.134295 | |
| 14. | Suparna Dash, Kankan Sarkar, Subhas Khajanchi, Spatiotemporal Dynamics of an Intraguild Predation Model with Intraspecies Competition, 2024, 34, 0218-1274, 10.1142/S0218127424300301 | |
| 15. | Liqiong Pu, Haotian Tang, Jiashan Zheng, Smooth Solution in an N$$ N $$‐Dimensional Chemotaxis Model With Intraguild Predation, 2025, 0170-4214, 10.1002/mma.10694 |
| Symbol | Parameter Definition | Units |
| Basal resource intrinsic growth rate | [time] |
|
| Basal resource carrying capacity | [Basal resource density] | |
| Predation rate of IG prey on resource | [IG prey] |
|
| Predation rate of IG predator on resource | [IG predator] |
|
| Predation rate of IG predaotr on IG prey | [IG preys][IG predator] |
|
| [time] |
||
| Conversion rate from resource to IG prey | [IG preys][resource] |
|
| Conversion rate from resource to IG predator | [IG predators][resource] |
|
| Conversion rate from IG prey to IG predator | [IG predators][IG prey] |
|
| [Half saturation constant] |
[resource] |
|
| [Half saturation constant] |
[IG predator] |
|
| Mortality rate of IG prey | [time] |
|
| Mortality rate of IG predator | [time] |
|
| Diffusion coefficient of resource | [length] |
|
| Diffusion coefficient of IG prey | [length] |
|
| Diffusion coefficient of IG predatior | [length] |
|
| The size of spatial domain |
[length] |
| Symbol | Parameter Definition | Units |
| Basal resource intrinsic growth rate | [time] |
|
| Basal resource carrying capacity | [Basal resource density] | |
| Predation rate of IG prey on resource | [IG prey] |
|
| Predation rate of IG predator on resource | [IG predator] |
|
| Predation rate of IG predaotr on IG prey | [IG preys][IG predator] |
|
| [time] |
||
| Conversion rate from resource to IG prey | [IG preys][resource] |
|
| Conversion rate from resource to IG predator | [IG predators][resource] |
|
| Conversion rate from IG prey to IG predator | [IG predators][IG prey] |
|
| [Half saturation constant] |
[resource] |
|
| [Half saturation constant] |
[IG predator] |
|
| Mortality rate of IG prey | [time] |
|
| Mortality rate of IG predator | [time] |
|
| Diffusion coefficient of resource | [length] |
|
| Diffusion coefficient of IG prey | [length] |
|
| Diffusion coefficient of IG predatior | [length] |
|
| The size of spatial domain |
[length] |