Caputo-Hadamard fractional boundary-value problems in ${\mathfrak{L}}^\mathfrak{p}$-spaces

1.   Introduction

Fractional differential equations have attracted much attention and been widely used in engineering, physics, chemistry, biology, and other fields. For more details, see ^[1,2,3]. The theory is a beautiful mixture of pure and applied analysis. Over the years, the theory of fixed points has been revealed as a very powerful and important tool in the study of nonlinear phenomena.

In particular, fixed-point techniques have been applied in many areas of mathematics, sciences, and engineering. Various fixed-point theorems have been utilized to establish sufficient conditions for the existence and uniqueness of solutions for different types of fractional differential problems; see, for example, ^{[4,5,6,7,8,9,10]}.

The Caputo-Hadamard fractional differential equations ($\mathcal{CHFDE}s$), with their non-integer order derivatives, offer a distinctive perspective on modeling complex phenomena. Incorporating boundary conditions adds depth to the study by constraining solutions, and this is essential for practical applications and system analysis. There have been investigations into the existence, uniqueness, and properties of solutions under specific constraints by using diverse techniques like Laplace transformation and numerical methods. Unveiling new insights into fractional dynamics under constraints has broad applications in physics, engineering, biology, and finance. The development of tailored analytical and numerical tools for fractional contexts presents hurdles and opportunities for solving real-world problems effectively. Ongoing research promises advancements in mathematical methods, algorithms, and theoretical frameworks, potentially refining existing models and solving complex problems. Moreover, researchers have made great efforts in the study of the properties of Caputo-Hadamard fractional derivatives, and they have established the existence of the ($\mathcal{CHFDE}s$) by applying some fixed-point theorems; see ^{[11,12,13,14,15,16,17]}.

Furthermore, the ${\mathfrak{L}}^\mathfrak{p}$-integrable solutions for fractional differential equations have been intensively studied by many mathematicians. For example, the authors of ^[18] discussed the existence of fractional boundary-value problems in ${\mathfrak{L}}^\mathfrak{p}$-spaces. Agrwal et al. ^[19] derived the existence of ${\mathfrak{L}}^\mathfrak{p}$-solutions for differential equations with fractional derivatives under compactness conditions. The investigations of ${\mathfrak{L}}^\mathfrak{p}$-integrable solutions can be see in ^{[20,21,22,23,24]}. Nowadays, the Ulam-Hyers stability is a crucial topic in nonlinear differential equation research, as it studies the effective flexibility of solutions along small perturbations and focuses on how the differential equations behave when the initial state or parameters are slightly changed. Several articles have been published related to this subject; see ^{[25,26,27,28,29,30,31]}.

Dhaigude and Bhairat ^[32] discussed the existence and Ulam-type stability of solutions for the following fractional differential equation:

where $p-1 < {\mathfrak{W}} \leq p$ and $\; {\mathcal{D}}^{{\mathfrak{W}}}$ denotes the Caputo-Hadamard derivative of order ${\mathfrak{W}}$.

In ^[33], utilizing the O'Regan fixed-point theorem and Burton-Kirk fixed-point theorem, Derbazi and Hammouche presented a new result on the existence and stability for the boundary-value problem of nonlinear fractional differential equations, as follows:

where ${\mathcal{D}}^{{\mathfrak{W}}}, \; {\mathcal{D}}^{\beta},$ and ${\mathcal{D}}^{\beta_1}$ are the Caputo fractional derivatives such that $0 < \beta, \; \beta_1\leq1$ and $I_{0^+}^{\delta_1}, I_{0^+}^{\delta_2}$ are the Riemann-Liouville fractional integral and $m_1, m_2, \delta_1, \delta_2$ are real constants. In ^[34], Hu and Wang investigated the existence of solutions of the following nonlinear fractional differential equation:

with the following integral boundary conditions:

where $D_{t}^{\alpha}$ is the Riemann-Liouville fractional derivative, ${\mathcal{M}}:[0, 1]\times \mathfrak{R} \times \mathfrak{R}\rightarrow \mathfrak{R}$, and $g\in{{\mathfrak{L}}^{1}}[0, 1]$.

In ^[35], Murad and Hadid, by means of the Schauder fixed-point theorem and the Banach contraction principle, considered the boundary-value problem of the fractional differential equation:

where ${\mathcal{D}}^{{\mathfrak{W}}}, \; {\mathcal{D}}^{{\mathfrak{H}}}$ are Riemann-Liouville fractional derivatives, $1 < {\mathfrak{W}} \leq2$, $0 < {\mathfrak{H}} \leq 1$, and $\; 0 < {\mathfrak{U}} \leq 1$.

The focal point of the originality of this work is that we deal with the existence of ${\mathfrak{L}}^{\mathfrak{p}}$-integrable solutions for $\mathcal{CHFDE}$s by applying the rarely used Burton-Kirk fixed-point theorem under sufficient conditions with the help of the Kolmogorov compactness criterion and Hölder inequality. The Burton-Kirk fixed-point theorem, a pivotal result in the field of functional analysis and nonlinear analysis, is a tool for addressing existence problems in systems of differential equations; see ^[36,37,38]. This theorem combines Krasnoselskii's fixed-point theorem on the sum of two operators with Schaefer's fixed-point theorem. Schaefer's theorem eliminates a difficult hypothesis in Krasnoselskii's theorem, but it requires an a priori bound on solutions. Motivated by the above works, we extended the previous results obtained in ^[34,35] to study the existence, uniqueness, and Ulam stability of solutions for fractional differential equations of the Caputo-Hadamard type with integral boundary conditions of the following form:

where $^{\mathcal{CH}}{\mathcal{D}}^{{\mathfrak{W}}}, \; \; ^{\mathcal{CH}}{\mathcal{D}}^{{\mathfrak{H}}}$ are Caputo-Hadamard fractional derivatives of order ${\mathfrak{W}} \in(1, 2]$, ${\mathfrak{H}} \in (0, 1]$, ${I}_{\hat{a}}^{{{\mathfrak{T}} }_{{\mathfrak{U}}}}$ is the Caputo-Hadamard fractional integral, ${\mathfrak{U}} \in (0, 1]$, and ${\mathcal{M}}:{\mathfrak{I}} \times \mathfrak{R} \times \mathfrak{R} \rightarrow \mathfrak{R}$.

To the best of our knowledge, up to now, no work has been reported to drive the ($\mathcal{CHFDE}s$) with the rarely used Bourten-Kirk fixed-point in Lebesgue space (${\mathfrak{L}}^{\mathfrak{p}}$). The main contribution is summarized as follows:

1) ($\mathcal{CHFDE}s$) with integral boundary conditions are formulated.

2) Initially, we establish the uniqueness result by applying the Banach fixed-point theorem together with the Hölder inequality.

3) The arguments are based on the Bourtin-Kirk fixed-point theorem, in combination with the technique of measures of noncompactness, to prove the existence of ${\mathfrak{L}}^{\mathfrak{p}}$-integrable solutions for Eq (1.1). A necessary and sufficient condition for a subset of Lebesgue space to be compact is given in what is often called the Kolmogorov compactness theorem.

4) Ulam-Hyers stability is also investigated by applying the Hölder inequality for the ${\mathfrak{L}}^{\mathfrak{p}}$-integrable solutions.

5) Appropriate examples with figures and tables are also provided to demonstrate the applicability of our results.

The paper is organized as follows. In Section 2, we recall some definitions and results required for this study. Section 3 deals with the existence and uniqueness of ${\mathfrak{L}}^\mathfrak{p}$-integrable solutions for $\mathcal{CHFDE}$s. In Section 4, we show the stability of this solution by using the Ulam-Hyers with Ulam-Hyers-Rassias stability. Examples are given to illustrate our main results in Section 5.

2.   Preliminaries

Definition 2.1. ^[2] Let ${\mathfrak{S}}:[\hat{a}, {\mathfrak{T}}] \rightarrow \mathfrak{R}$ be a continuous function. Then, the Hadamard fractional integral is defined by

provided that the integral exists.

Definition 2.2. ^[2] Let ${\mathfrak{S}}$ be a continuous function. Then, the Hadamard fractional derivative is defined by

where $\nu = [{\mathfrak{W}}]+1$, [${\mathfrak{W}}$] denotes the integer part of the real number ${\mathfrak{W}},$ and $\Gamma$ is the gamma function.

Definition 2.3. ^[2] Let ${\mathfrak{S}}$ be a continuous function. Then, the Caputo-Hadamard derivative of order ${\mathfrak{W}}$ is defined as follows

where $\nu = [{\mathfrak{W}}]+1$, $\Delta = (\zeta\frac{d}{d\zeta})$, and [${\mathfrak{W}}$] denotes the integer part of the real number ${\mathfrak{W}}$.

Lemma 2.4. ^[2] Let ${\mathfrak{W}}\in\mathfrak{R^+}$ and $\nu = [{\mathfrak{W}}]+1$. If ${\mathfrak{S}} \in AC^{\nu}_{{\mathfrak{W}}}([\hat{a}, {\mathfrak{T}}], \mathfrak{R})$, then the Caputo-Hadamard differential equation $^{\mathcal{CH}}D^{{\mathfrak{W}}}_{a} {\mathfrak{S}}(\zeta) = 0$ has a solution

and the next formula hold:

where $\mathfrak{g}_{p}\in {\mathfrak{R} }, \; p = 0, 1, 2, ..., \nu-1$.

Definition 2.5. ^[39] If there exists a real number $c_f > 0$ such that $\hat{\varepsilon} > 0$, for each solution $\hat{\Psi} \in {\mathfrak{L}}^{\mathfrak{p}}([\hat{a}, {\mathfrak{T}}], \mathfrak{R})$ of the inequality

there exists a solution ${\mathfrak{S}} \in {\mathfrak{L}}^{\mathfrak{p}}([\hat{a}, {\mathfrak{T}}], \mathfrak{R})$ of Eq (1.1) with

Then, Eq (1.1) is Ulam-Hyers-stable

Definition 2.6. ^[39] If there exists a real number $c_{f\hat{\Phi}} > 0$ such that $\hat{\varepsilon} > 0$, for each solution $\hat{\Psi} \in {\mathfrak{L}}^{\mathfrak{p}}([\hat{a}, {\mathfrak{T}}], \mathfrak{R})$ of the inequality

there exists a solution ${\mathfrak{S}} \in {\mathfrak{L}}^{\mathfrak{p}}([\hat{a}, {\mathfrak{T}}], \mathfrak{R})$ of Eq (1.1) with

Then, Eq (1.1) is Ulam-Hyers-Rassias-stable with respect to $\hat{\Phi}$.

Theorem 2.7. ^[40] (Kolmogorov compactness criterion)

Let $\hat{\nu} \subseteq {\mathfrak{L}}^\mathfrak{p}[\hat{a}, {\mathfrak{T}}]$, $1\leq\mathfrak{p} < \infty$. If

(ⅰ) $\hat{\nu}$ is bounded in ${\mathfrak{L}}^\mathfrak{p}[\hat{a}, {\mathfrak{T}}]$ and

(ⅱ) $\hat{\nu}$ is compact (relatively) in ${\mathfrak{L}}^\mathfrak{p}[\hat{a}, {\mathfrak{T}}]$ then $\hat{\psi}_\mathfrak{h}\rightarrow \hat{\psi}$ as $\mathfrak{h}\rightarrow 0$ uniformly with respect to $\hat{\psi} \in \hat{\nu}$, where

Theorem 2.8. ^[41] (Burton-Kirk fixed-point theorem)

Assume that $H$ is a Banach space and that there are two operators ${\mathfrak{F}}_1, {\mathfrak{F}}_2:H \rightarrow H$ such that ${\mathfrak{F}}_1$ is a contraction and ${\mathfrak{F}}_2$ is completely continuous. Then, either

- $\Xi = \{{\mathfrak{S}} \in H:\hat{\gamma}\; {\mathfrak{F}}_2(\dfrac{{\mathfrak{S}}}{\hat{\gamma}})+\hat{\gamma}{\mathfrak{F}}_1({\mathfrak{S}}) = {\mathfrak{S}}\; \; \text{is unbounded for}\; \hat{\gamma} \in(0, 1) \}$, or

- the operator equation ${\mathfrak{S}} = {{\mathfrak{F}}_1}({\mathfrak{S}})+{\mathfrak{F}}_2({\mathfrak{S}})$ has a solution.

Then, $z \in H$ exists such that $z = {\mathfrak{F}}_1z+{\mathfrak{F}}_2z$.

Lemma 2.9. ^[42] (Bochner integrability)

If $||\hat{V}||$ is Lebesgue integrable, then a measurable function $\hat{V}:[\hat{a}, {\mathfrak{T}}]\times \mathfrak{R} \rightarrow \mathfrak{R}$ is Bochner integrable.

Lemma 2.10. ^[43] (Hölder's inequality)

Assume that $\hat{Q}$ is a measurable space and that $\mathfrak{a}$ and $\mathfrak{b}$ satisfy the condition that $\frac{1}{\mathfrak{a}}+\frac{1}{\mathfrak{b}} = 1.\; 1\leq\mathfrak{a} < \infty, \; 1\leq \mathfrak{b} < \infty$ and $(ej)$ belongs to ${\mathfrak{L}}(\hat{Q})$, which is satisfied if $e\in {\mathfrak{L}}^{\mathfrak{a}}(\hat{Q})$ and $j\in {\mathfrak{L}}^{\mathfrak{b}}(\hat{Q})$.

Lemma 2.11. ^[44] If $0 < {\mathfrak{W}} < 1$, then

where $\; 1 < \mathfrak{a} < 1/(1-{\mathfrak{W}}).$

Lemma 2.12. A function ${\mathfrak{S}}\in {\mathfrak{L}}^{\mathfrak{p}}({\mathfrak{I}}, \mathfrak{R})$ is a unique solution of the boundary-value problem given by Eqs (1.1) and (1.2) if and only if ${\mathfrak{S}}$ satisfies the integral equation

Proof. Equation (1.1) can be reduced to the corresponding integral equation by using Lemma 2.4:

for $\mathfrak{g}_{0}, \mathfrak{g}_{1}\in\mathfrak{R}$ and ${\mathfrak{S}}(\hat{a}) = 0$; we can obtain $\mathfrak{g}_{0} = 0$. Then, we can write Eq (2.3) as

and it follows from the condition ${\mathfrak{S}}(\hat{{\mathfrak{T}}}) = {I}_{\hat{a}}^{{{\mathfrak{T}} }_{{\mathfrak{U}}}}{{\mathfrak{S}}}$ that

where $\hat{\mho} = \dfrac{\Gamma({\mathfrak{U}})}{(\ln\frac{{\mathfrak{T}}}{\hat{a}})\; \bigg(\Gamma({\mathfrak{U}})-(\ln\frac{{\mathfrak{T}}}{\hat{a}})^{{\mathfrak{U}}}\; \beta({\mathfrak{U}}, 2)\bigg)}$.

Hence, the solution of the problem defined by Eqs (1.1) and (1.2) is given by

□

3.   Existence and uniqueness results

In this section, we study the existence of a solution for the boundary-value problem given by Eqs (1.1) and (1.2) under certain conditions and assumptions. For measurable functions denoted by ${\mathcal{M}}:{\mathfrak{I}} \times \mathfrak{R} \times \mathfrak{R} \rightarrow \mathfrak{R}$, define the space $\beth_* = \{\zeta : {\mathfrak{S}} \in {\mathfrak{L}}^\mathfrak{p} ({\mathfrak{I}}, \mathfrak{R}), ^{\mathcal{CH}}{\mathcal{D}}^{{\mathfrak{H}}} {\mathfrak{S}}\in {\mathfrak{L}}^\mathfrak{p} ({\mathfrak{I}}, \mathfrak{R})\},$

equipped with the norm

where ${\mathfrak{L}}^\mathfrak{p} ({\mathfrak{I}}, \mathfrak{R})$ represents the Banach space containing all Lebesgue measurable functions.

Our results are based on the following assumptions:

(O1) $\exists$ a constant ${\mathfrak{Z}} > 0$ such that $\qquad\big|{\mathcal{M}}(\zeta, {\mathfrak{S}}_1, {\mathfrak{S}}_2)\big|\leq \mathfrak{Z}\; \big(|{\mathfrak{S}}_1|+|{\mathfrak{S}}_2|\big),$

for each $\zeta \in {\mathfrak{I}}$ and for all ${\mathfrak{S}}_1, {\mathfrak{S}}_2 \in \mathfrak{R}.$

(O2) ${\mathcal{M}}$ is continuous and $\exists$ a constant ${\mathfrak{Q}}_1 > 0$ such that

for each ${\mathfrak{S}}_1, {\mathfrak{S}}_2, \bar{{\mathfrak{S}}_1}, \bar{{\mathfrak{S}}_2} \in \mathfrak{R}$.

To make things easier, we set the notation as follows:

The first theorem is based on Banach contraction mapping.

Theorem 3.1. Let ${\mathcal{M}}:[\hat{a}, {\mathfrak{T}}] \times \mathfrak{R} \times \mathfrak{R} \rightarrow \mathfrak{R}$ be a continuous function that satisfies the conditions (O1) and (O2). If $\; \omega < 1$, then the problem defined by Eqs (1.1) and (1.2) has only one solution.

Proof. First, define the operator ${\mathfrak{F}}$ by

It is necessary to derive the fixed-point of the operator ${\mathfrak{F}}$ on the following set:

${\mathfrak{Y}}_{\mathfrak{A}} = \{{\mathfrak{S}} \in {\mathfrak{L}}^{\mathfrak{p}}({\mathfrak{I}}, \mathfrak{R}):||{\mathfrak{S}}||^\mathfrak{p}_{\mathfrak{p}{\beth_*}}\leq {\mathfrak{A}}^\mathfrak{p}, {\mathfrak{A}} > 0 \}$. For ${\mathfrak{S}} \in {\mathfrak{Y}}_{\mathfrak{A}}$, we have

From Hölder's inequality and Lemma 2.11, the first term of Eq (3.1) can be simplified as follows:

Now, by the same technique, the second term can be found as follows

where $\beta({\mathfrak{W}}, {\mathfrak{U}})$ and $\beta^{\frac{\mathfrak{p}-1}{\mathfrak{p}}}\big({\frac{\mathfrak{p}({\mathfrak{W}}+{\mathfrak{U}})-1}{\mathfrak{p}-1}}, 1\big)$ are beta functions. Now, the last term of Eq (3.1) needs to be found, as follows:

Thus, $\bigg(\int_{\hat{a}}^{{\mathfrak{T}}}(\ln\frac{{\mathfrak{T}}}{\theta})^{{\mathfrak{W}}-1}|{\mathcal{M}}(\theta, {\mathfrak{S}}(\theta), {\mathcal{D}}^{{\mathfrak{H}}}{\mathfrak{S}}(\theta))|\frac{d\theta}{\theta} \bigg)^\mathfrak{p}, \; \bigg(\int_{\hat{a}}^{\zeta}(\ln\frac{\zeta}{\theta})^{{\mathfrak{W}}-1}|{\mathcal{M}}(\theta, {\mathfrak{S}}(\theta), {\mathcal{D}}^{{\mathfrak{H}}}{\mathfrak{S}}(\theta))|\frac{d\theta}{\theta} \bigg)^\mathfrak{p}$ and $\bigg(\int_{\hat{a}}^{{\mathfrak{T}}} \int_{\theta}^{{\mathfrak{T}}}{(\ln\frac{{\mathfrak{T}}}{\varpi})^{{\mathfrak{U}}-1}(\ln\frac{\varpi}{\theta})^{{\mathfrak{W}}-1}}|{\mathcal{M}}(\theta, {\mathfrak{S}}(\theta), {\mathcal{D}}^{{\mathfrak{H}}}{\mathfrak{S}}(\theta))|\frac{d\varpi}{\varpi}\frac{d\theta}{\theta}\bigg)^\mathfrak{p}$ are Lebesgue-integrable; by Lemma 2.9, we conclude that $(\ln\frac{{\mathfrak{T}}}{\theta})^{{\mathfrak{W}}-1}{\mathcal{M}}(\theta, {\mathfrak{S}}(\theta), {\mathcal{D}}^{{\mathfrak{H}}}{\mathfrak{S}}(\theta))$, $\int_{\theta}^{{\mathfrak{T}}}{(\ln\frac{{\mathfrak{T}}}{\varpi})^{{\mathfrak{U}}-1}(\ln\frac{\varpi}{\theta})^{{\mathfrak{W}}-1}}\frac{d\varpi}{\varpi}{\mathcal{M}}(\theta, {\mathfrak{S}}(\theta), {\mathcal{D}}^{{\mathfrak{H}}}{\mathfrak{S}}(\theta))$ and $(\ln\frac{\zeta}{\theta})^{{\mathfrak{W}}-1}{\mathcal{M}}(\theta, {\mathfrak{S}}(\theta), {\mathcal{D}}^{{\mathfrak{H}}}{\mathfrak{S}}(\theta))$ are Bochner-integrable with respect to $\theta \in [\hat{a}, \zeta]$ for all $\zeta \in {\mathfrak{I}}$. From Eqs (3.2)–(3.4), Eq (3.1) gives

By using integration by parts, Eq (3.5) becomes

and

By (O1) and the Hölder inequality, we can find that

Combining Eq (3.6) with Eq (3.7), we get

which implies that ${\mathfrak{F}} {\mathfrak{Y}}_{\mathfrak{A}} \subseteq {\mathfrak{Y}}_{\mathfrak{A}}$. Hence, ${\mathfrak{F}} ({\mathfrak{S}})(\zeta)$ is Lebesgue-integrable and ${\mathfrak{F}}$ maps ${\mathfrak{Y}}_{\mathfrak{A}}$ into itself.

Now, to show that ${\mathfrak{F}}$ is a contraction mapping, considering that ${\mathfrak{S}}_1, {\mathfrak{S}}_2 \in {\mathfrak{L}}^{\mathfrak{p}}({\mathfrak{I}}, \mathfrak{R})$, we obtain

Some computations give

Using similar techniques, we obtain

Then,

Combining Eqs (3.8) and (3.9), we get

If $\omega < 1$, then the Banach theorem guarantees that there is only one fixed-point which is a solution of the problem defined by Eqs (1.1) and (1.2). □

The following outcome is the Burton-Kirk theorem.

Theorem 3.2. Suppose that (O1) and (O2) hold. Then, the problem defined by Eqs (1.1) and (1.2) has at least one solution.

Proof. Let ${\mathfrak{F}}:\beth_* \rightarrow \beth_*$; we define the operators as follows

Step 1: The operator ${\mathfrak{F}}_1$ is continuous.

It follows from the Hölder inequality and the integration by parts that Eq (3.10) becomes

for all $\zeta \in {\mathfrak{I}}$. In a similar manner, we obtain

Then,

According to the Lebesgue dominated convergence theorem, since ${\mathcal{M}}$ is of Caratheodory type, we have that $\quad||({\mathfrak{F}}_1{\mathfrak{S}}_{\mathfrak{j}})-({\mathfrak{F}}_1{\mathfrak{S}})||_{\mathfrak{p}\beth_*} \rightarrow 0 \qquad \text{as} \qquad \mathfrak{j}\rightarrow \infty$.

Step 2: Consider the set $\aleph_{ \Upsilon} = \{{\mathfrak{S}}\in {\mathfrak{L}}^{\mathfrak{p}}({\mathfrak{I}}, \mathfrak{R}):||{\mathfrak{S}}||^\mathfrak{p}_{\mathfrak{p}{\beth_*}}\leq{ \Upsilon}^\mathfrak{p}, \Upsilon > 0\}$.

For ${\mathfrak{S}} \in \aleph_{ \Upsilon}$ and $\zeta \in {\mathfrak{I}}$, we will prove that ${\mathfrak{F}}_1(\aleph_{ \Upsilon})$ is bounded and equicontinuous, and that

In a like manner,

Then,

Hence, ${\mathfrak{F}}_1(\aleph_{ \Upsilon})$ is bounded.

Now, Theorem 2.7-(Kolmogorov compactness criterion) will be applied to prove that ${\mathfrak{F}}_1$ is completely continuous. Assume that a bounded subset of $\aleph_{\Upsilon}$ is $\hat{\delta}$. Hence, ${\mathfrak{F}}_1 (\hat{\delta})$ is bounded in ${\mathfrak{L}}^{\mathfrak{p}}({\mathfrak{I}}, \mathfrak{R})$ and condition (i) of Theorem 2.7 is satisfied. Next, we will demonstrate that, uniformly with regard to $\zeta \in \hat{\delta}$, $({\mathfrak{F}}_1 {\mathfrak{S}})_\mathfrak{h} \rightarrow ({\mathfrak{F}}_1 {\mathfrak{S}})$ in ${\mathfrak{L}}^{\mathfrak{p}}({\mathfrak{I}}, \mathfrak{R})$ as $\mathfrak{h} \rightarrow 0$. We estimate the following:

Similar, the following is obtained:

Since ${\mathcal{M}}\in {\mathfrak{L}}^{\mathfrak{p}}({\mathfrak{I}}, \mathfrak{R})$, we get that $I^{{\mathfrak{W}}}{\mathcal{M}}, \; I^{{\mathfrak{W}}-{\mathfrak{H}}} {\mathcal{M}} \in {\mathfrak{L}}^{\mathfrak{p}}({\mathfrak{I}}, \mathfrak{R})$, as well as that

and

Hence, $\; \; ||({\mathfrak{F}}_1 {\mathfrak{S}})_\mathfrak{h}- ({\mathfrak{F}}_1 {\mathfrak{S}})||_{\mathfrak{p}\beth_*}\rightarrow 0.$

Then, we conclude that ${\mathfrak{F}}_1(\hat{\delta})$ is relatively compact, i.e., ${\mathfrak{F}}_1$ is a compact, by using Theorem 2.7.

Step 3: ${\mathfrak{F}}_2$ is contractive. For all ${\mathfrak{S}}, {\mathfrak{S}}_c \in {\mathfrak{L}}^{\mathfrak{p}}({\mathfrak{I}}, \mathfrak{R})$, we have

and

Combining Eqs (3.11) and (3.12), the following is obtained:

Step 4: Let $\Xi = \{{\mathfrak{S}} \in {\mathfrak{L}}^\mathfrak{p}({\mathfrak{I}}):\hat{\gamma}\; {\mathfrak{F}}_2(\dfrac{{\mathfrak{S}}}{\hat{\gamma}})+\hat{\gamma}{\mathfrak{F}}_1({\mathfrak{S}}) = {\mathfrak{S}}, \; \hat{\gamma} \in(0, 1) \}$. For all $\; {\mathfrak{S}} \in \Xi$, there exists $\hat{\gamma} \in(0, 1)$ such that

With the same arguments, we have

Then,

Hence, the set $\Xi$ is bounded, and, by Theorem 3.2, the problem defined by Eqs (1.1) and (1.2) has a solution. □

4.   Stability results

In this section, we establish the Ulam-Hyers and Ulam-Hyers-Rassias stability of the problem defined by Eqs (1.1) and (1.2); we set the following condition.

(O3) $\hat{\Phi} \in {\mathfrak{L}}^{\mathfrak{p}}({\mathfrak{I}}, \mathfrak{R})$ is an increasing function and $\exists$ $\hat{\lambda}_{\hat{\Phi}}, \hat{\Omega}_{\hat{\Phi}} > 0$ such that, for any $\zeta \in {\mathfrak{I}}$, we have

Theorem 4.1. Let ${\mathcal{M}}$ be a continuous function and (O2) hold with

Then, the problem defined by Eqs (1.1) and (1.2) is Ulam-Hyers-stable.

Proof. For $\hat{\varepsilon} > 0$, $\hat{\Psi}$ is a solution that satisfies the following inequality:

There exists a solution ${\mathfrak{S}} \in {\mathfrak{L}}^{\mathfrak{p}}({\mathfrak{I}}, \mathfrak{R})$ of the boundary-value problem defined by Eqs (1.1) and (1.2). Then, ${\mathfrak{S}} (\zeta)$ is given by Eq (2.4); from Eq (4.1), and for each $\zeta \in {\mathfrak{I}}$, we have

and

For each $\zeta \in {\mathfrak{I}}$, we have

Then, from Eq (4.2), we conclude that

By (O2) and the Hölder inequality, it follows that

Hence,

Now, from Eq (4.3), we have

and

Combining Eq (4.4) with Eq (4.5), we have

Hence,

where

Then, the problem is Ulam-Hyers-stable. □

Theorem 4.2. Let ${\mathcal{M}}$ be a continuous function and (O2) and (O3) hold. Then, the problem defined by Eqs (1.1) and (1.2) is Ulam-Hyers-Rassias-stable.

Proof. Let $\hat{\Psi}\in {\mathfrak{L}}^\mathfrak{p}({\mathfrak{I}}, \mathfrak{R})$ be a solution of Eq (2.2) and there exist a solution ${\mathfrak{S}} \in {\mathfrak{L}}^\mathfrak{p}({\mathfrak{I}}, \mathfrak{R})$ of Eq (1.1). Then, we have

From Eq (2.2), for each $\zeta \in {\mathfrak{I}}$, we get

and

On the other hand, for each $\zeta \in {\mathfrak{I}}$, from Eq (4.6), the below is found:

Thus, by condition (O2) and the Hölder inequality, Eq (4.8) becomes

Now, from Eq (4.7), one has

Similarly, we have

Combining Eq (4.9) with Eq (4.10), we have

Hence,

where    $c_f = \frac{2\; \big({\mathcal{V}}_3+{\mathcal{V}}_4\big)^{\frac{1}{\mathfrak{p}}}\; {\mathfrak{T}}^{\frac{1}{\mathfrak{p}}} }{\big(1-2^{2\mathfrak{p}}{\mathfrak{Q}}_1^\mathfrak{p}({\mathcal{V}}_1+{\mathcal{V}}_2)\big)^\frac{1}{\mathfrak{p}}}$.

Then, the problem defined by Eqs (1.1) and (1.2) is Ulam-Hyers-Rassias-stable. □

5.   Examples

In this section, we present two examples to illustrate the utility of our main results.

Example 5.1. Consider the following Bagley-Torvik equation:

Here, $\hat{a} = 1, \; {\mathfrak{T}} = 2, \; \theta = 1/25$, ${\mathfrak{W}} = 2, \; {\mathfrak{H}} = 0.4$ and $\; {\mathfrak{U}} = 0.7$. Also, let $\mathfrak{p} = 2$, by the condition (O2), we have that ${\mathfrak{Q}}_1 = 0.04$. Then, from Theorem 3.1,

This indicates that the solution to the problem defined by Eq (5.1) is unique.

Example 5.2. Consider the following boundary-value problem:

Here, $\hat{a} = 1, \; {\mathfrak{T}} = e$, ${\mathfrak{W}} = 1.7, \; {\mathfrak{H}} = 0.3$, and ${\mathfrak{U}} = 0.6$. By the Lipschitz condition, we have that ${\mathfrak{Q}}_1 = 0.00817509$. Now, to check the obtained results for the Banach contraction mapping and Ulam-Hyers and Ulam-Hyers-Rassias stability, we examine the following cases:

Case Ⅰ: Let $\mathfrak{p} = 2$; by a direct calculation and by Theorem 3.1, one can obtain that

We get that the problem defined by Eq (2) has a unique solution.

At this moment, to examine the stability, let ${\mathfrak{S}} = 1$; we show that Eq (2.1) hold. Indeed,

From Theorem 4.1, we have

which shows that the problem defined by Eq (5.2) is Ulam-Hyers-stable.

Next, let $\hat{\Phi}(\zeta) = \zeta-1.8$; by applying Theorem 4.2, we have

Hence, the problem defined by Eq (5.2) is Ulam-Hyers-Rassias-stable with

Case Ⅱ: Let $\mathfrak{p} = 3, \; \hat{\varepsilon} = 0.08443313, \; \text{and}\; \hat{\Phi}(\zeta) = \zeta-1.8$; we have that $\omega = 0.24914024 < 1.$

Then, the boundary-value problem defined by Eq (2) has a unique solution.

Now, according Theorems 4.1 and 4.2, the Ulam-Hyers and Ulam-Hyers-Rassias stability for the boundary-value problem defined by Eq (2) are respectively given as follows

Case Ⅲ: Let $\mathfrak{p} = 4$. From Theorem 3.1, we start by computing the following:

$2\; {\mathfrak{Q}}_1\; \big({\mathcal{V}}_1+{\mathcal{V}}_2\big)^\frac{1}{\mathfrak{p}} = 0.209580118 < 1.$ Hence, the boundary-value problem defined by Eq (2) has a unique solution. Also, it has Ulam-Hyers and Ulam-Hyers-Rassias stable with

6.   Discussion

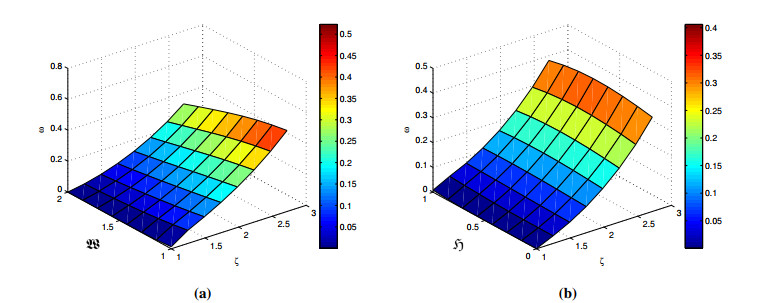
To show the efficiency of the Banach contraction principle and that the problem has a unique solution, we will evaluate the value of $\omega$ for some different fractional orders, i.e., $1 < {\mathfrak{W}}\leq 2$ and $0 < \; {\mathfrak{H}}\le 1$. Table 1 presents the value of $\omega$ when $\mathfrak{p} = 2$ and $\zeta \in [1, e]$ for some specific orders, such as when ${\mathfrak{W}} = 1.2$, ${\mathfrak{H}} = 0.2, 0.8$, when ${\mathfrak{W}} = 1.5$, $\; {\mathfrak{H}} = 0.2, 0.5, 0.8$, and when ${\mathfrak{W}} = 1.8$, $\; {\mathfrak{H}} = 0.2, 0.5$. Furthermore, the behavior of $\omega$ at some selected points is illustrated in Figure 1.

Figure 2 shows that the problem has a unique solution at $\mathfrak{p} = 3$ when $1 < {\mathfrak{W}} < 2$, $\; {\mathfrak{H}} = 0.5$, and when ${\mathfrak{W}} = 1.5$, $0 < \; {\mathfrak{H}}\leq1$. In addition, for $\mathfrak{p} = 4$ and $1 < {\mathfrak{W}} < 2$, $\; {\mathfrak{H}} = 0.8$ or ${\mathfrak{W}} = 1.2$, $0 < \; {\mathfrak{H}}\leq1$, $\omega$ has been plotted in Figure 3 and is presented in Table 2. To illustrate the sufficiency of our results to find the solution and its uniqueness, we chose $\mathfrak{p} = 15$ as shown in Figure 4.

7.   Conclusions

In this paper, we examined the ${\mathfrak{L}}^{\mathfrak{p}}$-integrable solutions of nonlinear $\mathcal{CHFDE}s$ with integral boundary conditions. We applied the Burton-Kirk fixed-point theorem and Banach contraction principle with the Kolmogorov compactness criterion and Hölder's inequality technique to demonstrate the main results. In addition, the Ulam-Hyers and Ulam-Hyers-Rassias stability of the problem defined by Eqs (1.1) and (1.2) have been studied. Finally, examples have been provided to demonstrate the validity of our conclusions. In future works, one can extend the given fractional boundary-value problem to more fractional derivatives, such as the Hilfer and Caputo-Fabrizio fractional derivatives.

Author contributions

Shayma Adil Murad: Conceptualization, Methodology, Formal analysis, Investigation, Writing-original draft, Validation, Writing-review and editing; Ava Shafeeq Rafeeq: Methodology, Formal analysis, Investigation, Writing-original draft, Validation; Thabet Abdeljawad: Investigation, Validation, Supervision, Writing-review and editing. All authors have read and agreed to the published version of the manuscript.

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgments

The author T. Abdeljawad would like to thank Prince Sultan University for the support through the TAS research lab.

Conflict of interest

The authors declare no conflict of interest.