A characterization of Wolf and Schechter essential pseudospectra

Sara Smail; Chafika Belabbaci; Sara Smail; Chafika Belabbaci

doi:10.3934/math.2024832

AIMS Mathematics

2024, Volume 9, Issue 7: 17146-17153. doi: 10.3934/math.2024832

Previous Article Next Article

Research article Special Issues

A characterization of Wolf and Schechter essential pseudospectra

Sara Smail ^,,
Chafika Belabbaci

Laboratory of pure and applied mathematics, Amar Teledji University, Laghouat 3000, Algeria

Received: 22 December 2023 Revised: 18 April 2024 Accepted: 09 May 2024 Published: 17 May 2024
MSC : 47A53, 47A55, 47A13

The aim of this paper is to provide new results on the Wolf and Schechter essential pseudospectra of bounded linear operators on a Banach space. More precisely, we characterize the Wolf and Schechter essential pseudospectra by using the notion of Fredholm perturbation. Also, we state the condition under which the Wolf (respectively, Schechter) essential pseudospectrum of two different bounded linear operators coincides. Furthermore, we give some characterizations of the Wolf and Schechter essential pseudospectra of $3\times 3$ upper triangular block operator matrices.

Keywords:

Citation: Sara Smail, Chafika Belabbaci. A characterization of Wolf and Schechter essential pseudospectra[J]. AIMS Mathematics, 2024, 9(7): 17146-17153. doi: 10.3934/math.2024832

Related Papers:

[1]	Ihtisham Ul Haq, Nigar Ali, Hijaz Ahmad . Analysis of a chaotic system using fractal-fractional derivatives with exponential decay type kernels. Mathematical Modelling and Control, 2022, 2(4): 185-199. doi: 10.3934/mmc.2022019
[2]	Rashid Jan, Normy Norfiza Abdul Razak, Sania Qureshi, Imtiaz Ahmad, Salma Bahramand . Modeling Rift Valley fever transmission: insights from fractal-fractional dynamics with the Caputo derivative. Mathematical Modelling and Control, 2024, 4(2): 163-177. doi: 10.3934/mmc.2024015
[3]	Saïd Abbas, Mouffak Benchohra, Johnny Henderson . Random Caputo-Fabrizio fractional differential inclusions. Mathematical Modelling and Control, 2021, 1(2): 102-111. doi: 10.3934/mmc.2021008
[4]	Anil Chavada, Nimisha Pathak, Sagar R. Khirsariya . A fractional mathematical model for assessing cancer risk due to smoking habits. Mathematical Modelling and Control, 2024, 4(3): 246-259. doi: 10.3934/mmc.2024020
[5]	Ihtisham Ul Haq, Nigar Ali, Shabir Ahmad . A fractional mathematical model for COVID-19 outbreak transmission dynamics with the impact of isolation and social distancing. Mathematical Modelling and Control, 2022, 2(4): 228-242. doi: 10.3934/mmc.2022022
[6]	Muhammad Nawaz Khan, Imtiaz Ahmad, Mehnaz Shakeel, Rashid Jan . Fractional calculus analysis: investigating Drinfeld-Sokolov-Wilson system and Harry Dym equations via meshless procedures. Mathematical Modelling and Control, 2024, 4(1): 86-100. doi: 10.3934/mmc.2024008
[7]	Lusong Ding, Weiwei Sun . Neuro-adaptive finite-time control of fractional-order nonlinear systems with multiple objective constraints. Mathematical Modelling and Control, 2023, 3(4): 355-369. doi: 10.3934/mmc.2023029
[8]	Naveen Kumar, Km Shelly Chaudhary . Position tracking control of nonholonomic mobile robots via $H_\infty$ -based adaptive fractional-order sliding mode controller. Mathematical Modelling and Control, 2025, 5(1): 121-130. doi: 10.3934/mmc.2025009
[9]	M. Haripriya, A. Manivannan, S. Dhanasekar, S. Lakshmanan . Finite-time synchronization of delayed complex dynamical networks via sampled-data controller. Mathematical Modelling and Control, 2025, 5(1): 73-84. doi: 10.3934/mmc.2025006
[10]	Abduljawad Anwar, Shayma Adil Murad . On the Ulam stability and existence of $L^p$ -solutions for fractional differential and integro-differential equations with Caputo-Hadamard derivative. Mathematical Modelling and Control, 2024, 4(4): 439-458. doi: 10.3934/mmc.2024035

Abstract

1. Introduction

As per medical experts, breast cancer is characterized by the abnormal development and division of cells inside the breast tissue. This condition poses a significant risk to health and is mostly seen in the female population. According to ^[1], compared to other types of cancer, the most people get breast cancer. It kills breast tissue and cells, causing the breasts to grow out of control and change shape. Several risk factors have been found that put women at a higher chance of getting breast cancer. Dietary habits, personal experience of malignancy, alcohol consumption, smoking, weight status, dense breast tissue, physical inactivity, reproductive history (including pregnancy and breastfeeding), menstrual history, genetic predisposition, racial background, life span history, specific breast changes, and getting older are all factors to consider. The main clinical presentations of breast cancer include lymphadenopathy, nipple discharge, nipple retraction, breast/nipple pain, presence of flaky skin on the breast/nipple, skin irritation, appearance of skin dimpling with erythema, changes in breast form or size, localized breast tissue thickening, and either complete or partial breast swelling. Tumor, node, and metastatic stages are used by medical professionals to evaluate a patient's cancer state and calculate their prospects of remission.

The odds of recovery are higher at earlier stages. Bisphosphonates, bone marrow transplants, gene therapy, hormone therapy, immunotherapy, surgery, stem cell therapy, targeted cancer medications, radiation, and complementary and alternative treatments are only a few of the therapeutic methods that have been created to prevent cancer. Chemotherapy is the most popular kind of treatment for the aforementioned conditions, and it uses medications to destroy cancer cells. The medications used in chemotherapy are either administered intravenously to the patient or taken orally, with some efficacy but potential cardiac risks. Cardiotoxicity is a negative side effect that may affect both adults and children ^[2]. Mathematical models are employed to analyze the complicated dynamics of illnesses and offer precise outcomes for infection reduction and control.

One of the most rapidly expanding subfields of mathematical analysis is called fractional calculus, and it is concerned with the study of both derivatives and integrals of any arbitrary order ^[3,4]. The nonlocality is a major advantage of the nonclassical derivatives that helps in the depiction of memory and hereditary characteristics of the system. As most of the biological processes involve the memory effect in the study of the epidemiological model fractional derivatives play a vital role.

Recent advancements suggest that fractional interpreters exhibit superior accuracy, utility, and reliability compared to the conventional derivative method. Contemporary fractional derivative operators are now being formulated in order to elucidate practical phenomena. Many mathematical models including integer and fractional order have been created in the literature for the detailed analysis of various diseases such as diabetes ^[5,6], breast cancer ^[7], cancer treatment model ^[8,9], HIV/AIDS ^[10,11], lung cancer ^[12], Covid-19 ^[13,14,15], and in several other scientific and technological disciplines such as biology ^[16,17,18], chemistry ^[19], engineering ^{[20,21,22,23,24,25,26]}, and most of the references cited therein.

The various techniques, such as the analytical methods ^[27,28,29], the semi-analytic methods ^{[30,31,32,33,34,35]}, and the numerical methods ^{[36,37,38,39,40,41]} are found in the literature for solving fractional differential equations. Moreover, in ^[42], the solution of the fractional Sawada-Kotera-Ito equation using Caputo and Atangana-Baleanu (AB) derivatives have been derived. In ^[43], a robust computational analysis of residual power series involving general transform to solve fractional differential equations has been proposed. Abdeljawad et al. in ^[44] derived a higher-order extension of AB fractional operators with respect to another function and a Gronwall-type inequality. In ^[45], the authors provide studies on the coupled snap system with integral boundary conditions in the G-Caputo sense. The authors in ^[46], studied the nonlocal multi-order implicit differential equation involving Hilfer fractional derivative on unbounded domains. In ^[47], an efficient variable step-size rational method for stiff, singular, and singularly perturbed problems has been given. The development of the reproducing kernel Hilbert space algorithm for the numerical pointwise solution of the time-fractional nonlocal reaction-diffusion equation is provided in ^[48].

In fractional calculus, a number of fractional operators are offered for the study of real issues. In contrast, these operators have a power law kernel and can merely partly simulate physical obstacles. Caputo and Fabrizio (CF) ^[49] introduced a unique fractional operator with a nonsingular kernel to address these issues and constraints. This revolutionary operator's results are more appropriate and have several applications. In ^[50], the authors studied the fractional mathematical modeling of the human liver. Ullah et al. in ^[51] analyzed a fractional model for the dynamics of tuberculosis infection. In ^[52], the authors proposed fractional cancer treatment. In ^[53], the authors studied the application of the CF derivative to a cancer model with unknown parameters. Ngungu et al. in ^[54] focused on mathematical epidemiological modeling and analysis of monkeypox dynamics with non-pharmaceutical intervation using real data from the UK. In ^[55], the authors developed the mathematical modeling of the immune-chemotherapeutic treatment of breast cancer under some control parameters, and the majority of the references listed therein have adequately represented the diseases transmission process with therapy and unidentified parameters.

The breast cancer classical mathematical model was categorized into five epidemiological groups by authors in ^[56]. However, it fails to optimally capture nonlocal behavior for the dynamics of the cancer, and the deviation between the real data and the data obtained through the simulation of the classical model is found to be large. Moreover, the freedom of having access to the real data is the chief motivation behind detailed analysis of the breast cancer model under the CF fractional-order differential operator. These precise fractional calculus findings and consequences drive us to examine and analyze the dynamics of breast cancer using actual data, which are reported cases in Saudi Arabia from 2004 to 2016 ^[57]. It is noteworthy to mention that, unlike several previous studies, we have diligently ensured dimensional uniformity throughout the process of fractionalization.

The following is the outline of article: Section 2 explains the fractional calculus requirements for system analysis. Section 3 uses a CF framework to develop a fractional order model for breast cancer. Section 4 gives the Picard-Lindelof (PL) technique and the fixed-point theorem to investigate the existence and uniqueness of a proposed system. In Section 5, we numerically investigate the dynamics of the proposed model with variation in input parameters. Finally, final observations are offered in the concluding portion.

2. Preliminaries

Definition 2.1. Let $f \in \mathbb{H}^{1}(\iota_1, \iota_2)$ and $0 < r < 1$ , then the CF fractional derivative ^[49] is defined as follows:

$\begin{equation} ({}^{\mathsf{cf}}_{0} D^{r}_{t} N)(t) = \frac{P(r)}{1-r} \int_{\iota_1}^{t}N^{'}(\delta) \mathsf{\exp}\left [{-\frac{r(t-\delta)}{1-r}}\right]d\delta, \end{equation}$

(2.1)

where $P(r)$ is a normalizing function with the property that $P(0) = P(1) = 1$ .

Definition 2.2. ^[58] If $0 < r < 1$ , then the CF fractional integral of order $r$ of a function $N$ is:

$\begin{equation} \begin{aligned} ({}^{\mathsf{cf}}_{0} I^{r}_{t} N)(t) = \frac{2(1-r)}{(2-r)P(r)}N(t) + \frac{2r}{(2-r)P(r)} \int_{0}^{t} N(\delta) d\delta.\end{aligned} \end{equation}$

(2.2)

3. Formulation of fractional mathematical model

Authors in ^[56] classified the breast cancer model into five epidemiological categories. During the initial medical report, the overall population of breast cancer patients was divided into phases 1 and 2 ( $\mathbb{S}_{12}$ ), phase 3 ( $\mathbb{S}_{3}$ ), phase 4 ( $\mathbb{S}_{4}$ ), disease-free state ( $\mathbb{S}_{R}$ ), and cardiotoxic ( $\mathbb{S}_{E}$ ) subpopulations. The traditional system is described as:

$\begin{align} \left\{\begin{array}{ll} \frac{d\mathbb{S}_{12}}{dt} = \Delta -(\rho +\nu)\mathbb{S}_{12}, \\ \quad \frac{d\mathbb{S}_3}{dt} = \Gamma +\nu \mathbb{S}_{12}+\psi {\mathbb{S}_{R}} - (\sigma+\mu+\kappa+\chi )\mathbb{S}_3, \\ \quad \frac{d\mathbb{S}_4}{dt} = \Omega +\mu \mathbb{S}_3+\phi {\mathbb{S}_{R}} - (\tau+\omega+\delta ){\mathbb{S}_{4}}, \\ \quad \frac{d\mathbb{S}_R}{dt} = \rho \mathbb{S}_{12} +\sigma \mathbb{S}_3 +\tau {\mathbb{S}_{4}} - (\psi +\phi +\zeta){\mathbb{S}_{R}}, \\ \quad \frac{d\mathbb{S}_E}{dt} = \zeta {\mathbb{S}_{R}}+\omega {\mathbb{S}_{4}}+\kappa\mathbb{S}_3-\eta {\mathbb{S}_{E}}, \end{array}\right. \end{align}$

(3.1)

where the following are the parameters of the system (3.1): $\Delta$ : people who have been diagnosed with cancer at stages 1 and 2; $\Gamma$ : people suffering with stage 3 cancer; $\Omega$ : cancer patients in the fourth stage; $\rho$ : chemotherapy recovery in phases 1 and 2; $\sigma$ : chemotherapy recovery at stage 3; $\tau$ : chemotherapy recovery at stage 4; $\mu$ : people in worse health enter the stage 4 population; $\nu$ : people in worse health enroll in class $\mathbb{S}_3$ ; $\kappa$ : patients undergoing severe treatment that induces cardiotoxicity; $\omega$ : people undergoing treatment for stage 4 cancer who suffer cardiotoxicity; $\zeta$ : at the disease-free stage, patients who have had extensive chemotherapy, which leads to cardiotoxicity; $\chi$ : cancer-related death at stage 3; $\delta$ : cancer-related death at stage 4; $\eta$ : cardiotoxic patients' mortality rate; $\psi$ : people who regress to stage 3; $\phi$ : people who regress to stage 4.

Now, to better approximate the spread of breast cancer with varying treatment rates into various compartments and to quantitatively demonstrate the influence of the above-mentioned parameters, the integer order model must be replaced by a fractional order model. The goal of this research is to extend the traditional system (3.1) by adding a fractional time derivative operator that allows for the analysis of memory effects in an arbitrary-order system. During the process of fractionalization, the dimensional consistency for each of the equations in the model has been maintained. We place the fractional order power $r$ on each time-dimensional parameter to make the equal time dimension ( $time^{-r}$ ) on both sides of the model. The proposed breast cancer transmission model, which includes the CF derivative, is offered as:

$\begin{equation} \begin{aligned} \begin{cases} {}^{\mathsf{cf}}_{0} D^{r}_{t} \mathbb{S}_{12} = \Delta^{r} -(\rho^{r} +\nu^{r}) \mathbb{S}_{12}, \\ \quad {}^{\mathsf{cf}}_{0} D^{r}_{t} \mathbb{S}_3 = \Gamma^{r} +\nu^{r} \mathbb{S}_{12}+\psi^{r} {\mathbb{S}_{R}} - (\sigma^{r} +\mu^{r} +\kappa^{r} +\chi^{r} )\mathbb{S}_3, \\ \quad {}^{\mathsf{cf}}_{0} D^{r}_{t} {\mathbb{S}_{4}} = \Omega^{r} +\mu ^{r} \mathbb{S}_3+\phi^{r} {{\mathbb{S}_{R}}} - (\tau^{r} +\omega^{r} +\delta^{r} ){\mathbb{S}_{4}}, \\ \quad {}^{\mathsf{cf}}_{0} D^{r}_{t} {\mathbb{S}_{R}} = \rho^{r} \mathbb{S}_{12} +\sigma^{r} \mathbb{S}_3 +\tau^{r} {\mathbb{S}_{4}} - (\psi^{r} +\phi^{r} +\zeta^{r}){\mathbb{S}_{R}}, \\ \quad {}^{\mathsf{cf}}_{0} D^{r}_{t} {\mathbb{S}_{E}} = \zeta^{r} {\mathbb{S}_{R}}+\omega^{r} {\mathbb{S}_{4}}+\kappa^{r} \mathbb{S}_3-\eta^{r} {\mathbb{S}_{E}}, \end{cases}\end{aligned} \end{equation}$

(3.2)

with initial conditions: $\mathbb{S}_{12}(0) = {\mathbb{S}_{12_0}}, \mathbb{S}_3(0) = {\mathbb{S}_{3_0}}, {\mathbb{S}_{4}}(0) = {\mathbb{S}_{4_0}}, {\mathbb{S}_{R}}(0) = {\mathbb{S}_{R_0}}, {\mathbb{S}_{E}}(0) = {\mathbb{S}_{E_0}}$ .

4. Qualitative analysis of fractional system

The present study aims to thoroughly examine the fractional system. The PL technique was used to investigate the presence and singularity of the offered solutions for the system (3.2). To begin the process, the system (3.2) is transformed into an integral equation with arbitrary order by applying (2.2) to both sides, resulting in

$\begin{gather} \left\{\begin{array}{ll} \mathbb{S}_{12}(t) - \mathbb{S}_{12}(0) = {}^{\mathsf{cf}} I^{r}_{t} \left\{N_1 (t,\mathbb{S}_{12})\right\}, \\ \quad \mathbb{S}_3(t) - \mathbb{S}_3(0) = {}^{\mathsf{cf}} I^{r}_{t} \left\{N_2 (t,\mathbb{S}_3)\right\}, \\ \quad {\mathbb{S}_{4}}(t) - {\mathbb{S}_{4}}(0) = {}^{\mathsf{cf}} I^{r}_{t} \left\{N_3 (t,{\mathbb{S}_{4}})\right\},\\ \quad {\mathbb{S}_{R}}(t) - {\mathbb{S}_{R}}(0) = {}^{\mathsf{cf}} I^{r}_{t} \left\{N_4 (t,{\mathbb{S}_{R}})\right\}, \\ \quad {\mathbb{S}_{E}}(t) - {\mathbb{S}_{E}}(0) = {}^{\mathsf{cf}} I^{r}_{t} \left\{N_5 (t,{\mathbb{S}_{E}})\right\}, \end{array}\right. \end{gather}$

(4.1)

where

$\begin{align*} N_1(t,\mathbb{S}_{12}) & = \Delta^{r} -(\rho^{r} +\nu^{r}) \mathbb{S}_{12}, \\ N_2(t,\mathbb{S}_3) & = \Gamma^{r} +\nu^{r} \mathbb{S}_{12}+\psi^{r} {\mathbb{S}_{R}} - (\sigma^{r} +\mu^{r} +\kappa^{r} +\chi^{r} )\mathbb{S}_3,\\ N_3(t,{\mathbb{S}_{4}}) & = \Omega^{r} +\mu ^{r} \mathbb{S}_3+\phi^{r} {{\mathbb{S}_{R}}} - (\tau^{r} +\omega^{r} +\delta^{r}){\mathbb{S}_{4}}, \\ N_4(t,{\mathbb{S}_{R}})& = \rho^{r} \mathbb{S}_{12} +\sigma^{r} \mathbb{S}_3 +\tau^{r} {\mathbb{S}_{4}} - (\psi^{r} +\phi^{r} +\zeta^{r}){\mathbb{S}_{R}}, \\N_5(t,{\mathbb{S}_{E}}) & = \zeta^{r} {\mathbb{S}_{R}}+\omega^{r} {\mathbb{S}_{4}}+\kappa^{r} \mathbb{S}_3-\eta^{r} {\mathbb{S}_{E}}, \end{align*}$

are contractions with respect to the functions $\mathbb{S}_{12}, \mathbb{S}_3, {\mathbb{S}_{4}}, {\mathbb{S}_{R}}$ , and ${\mathbb{S}_{E}}$ , respectively. Applying (2.2) on (4.1), we obtain

$\begin{gather} \begin{aligned} \left\{\begin{array}{ll} \mathbb{S}_{12}(t)-\mathbb{S}_{12}(0) = \Omega(r)N_1(t,\mathbb{S}_{12}) + \omega(r) \int_{0}^{t} N_1(y,\mathbb{S}_{12})dy, \\ \quad \mathbb{S}_3(t)-\mathbb{S}_3(0) = \Omega(r)N_2(t,\mathbb{S}_3) + \omega(r) \int_{0}^{t} N_2(y,\mathbb{S}_3)dy, \\ \quad {\mathbb{S}_{4}}(t)-{\mathbb{S}_{4}}(0) = \Omega(r)N_3(t,{\mathbb{S}_{4}}) + \omega(r) \int_{0}^{t} N_3(y,{\mathbb{S}_{4}})dy,\\ \quad {\mathbb{S}_{R}}(t)-{\mathbb{S}_{R}}(0) = \Omega(r)N_4(t,{\mathbb{S}_{R}}) + \omega(r) \int_{0}^{t} N_4(y,{\mathbb{S}_{R}})dy, \\ \quad {\mathbb{S}_{E}}(t)-{\mathbb{S}_{E}}(0) = \Omega(r)N_5(t,{\mathbb{S}_{E}}) + \omega(r) \int_{0}^{t} N_5(y,{\mathbb{S}_{E}})dy, \end{array}\right.\end{aligned} \end{gather}$

(4.2)

where

$\Omega(r) = \frac{2(1-r)}{(2-r)P(r)},\; \; \; \; \omega(r) = \frac{2r}{(2-r)P(r)}.$

By Picard's iterative algorithm,

$\begin{gather} \begin{aligned} \left\{\begin{array}{ll} {\mathbb{S}_{12_{n+1}}}(t) = \Omega(r)N_1(t,{\mathbb{S}_{12_n}}) + \omega(r) \int_{0}^{t} N_1(y,{\mathbb{S}_{12_n}})dy, \\ \quad {\mathbb{S}_{3_{n+1}}}(t) = \Omega(r)N_2(t,{\mathbb{S}_{3_n}}) + \omega(r) \int_{0}^{t} N_2(y,{\mathbb{S}_{3_n}})dy,\\ \quad {\mathbb{S}_{4_{n+1}}}(t) = \Omega(r)N_3(t,{\mathbb{S}_{4_n}}) + \omega(r) \int_{0}^{t} N_3(y,{\mathbb{S}_{4_n}})dy,\\ \quad {\mathbb{S}_{R_{n+1}}}(t) = \Omega(r)N_4(t,{\mathbb{S}_{R_n}}) + \omega(r) \int_{0}^{t} N_4(y,{\mathbb{S}_{R_n}})dy, \\ \quad {\mathbb{S}_{E_{n+1}}}(t) = \Omega(r)N_5(t,{\mathbb{S}_{E_n}}) + \omega(r) \int_{0}^{t} N_5(y,{\mathbb{S}_{E_n}})dy. \end{array}\right.\end{aligned} \end{gather}$

(4.3)

The solutions are achieved as follows:

$\begin{align*} & \lim\limits_{n \to \infty}{\mathbb{S}_{12_{n}}}(t) = \mathbb{S}_{12}(t),\ \lim\limits_{n \to \infty}{\mathbb{S}_{3_{n}}}(t) = \mathbb{S}_3(t),\ \lim\limits_{n \to \infty}{\mathbb{S}_{4_{n}}}(t) = {\mathbb{S}_{4}}(t), \\ &\ \lim\limits_{n \to \infty}{\mathbb{S}_{R_{n}}}(t) = {\mathbb{S}_{R}}(t),\ \ \lim\limits_{n \to \infty}{\mathbb{S}_{E_{n}}}(t) = {\mathbb{S}_{E}}(t). \end{align*}$

To demonstrate the existence of the solution, we use the PL approach and the Banach fixed point theorem.

Let

$\begin{align*} & N_1^{*} = \sup\limits_{\mathbb{C}[a,b_1]} \left \|N_1(t,\mathbb{S}_{12}) \right \|,\; \; \; N_2^{*} = \sup\limits_{\mathbb{C}[a,b_2]} \left \|N_2(t,\mathbb{S}_3) \right \|, \\ & N_3^{*} = \sup\limits_{\mathbb{C}[a,b_3]} \left \|N_1(t,{\mathbb{S}_{4}}) \right \|,\; \; \; \; \, N_4^{*} = \sup\limits_{\mathbb{C}[a,b_4]} \left \|N_1(t,{\mathbb{S}_{R}}) \right \|, \\ & N_5^{*} = \sup\limits_{\mathbb{C}[a,b_5]} \left \|N_1(t,{\mathbb{S}_{E}}) \right \|, && \end{align*}$

where

$\begin{align*} \mathbb {C}[a,b_1] & = [t-a, t+a]\times [\mathbb{S}_{12}-b_1, \mathbb{S}_{12}+b_1] = \mathbb{A} \times \mathbb {B}_1, \\ \mathbb {C}[a,b_2] & = [t-a, t+a]\times [\mathbb{S}_3-b_2, \mathbb{S}_3+b_2] = \mathbb{A} \times \mathbb {B}_2, \\ \mathbb {C}[a,b_3] & = [t-a, t+a]\times [{\mathbb{S}_{4}}-b_3, {\mathbb{S}_{4}}+b_3] = \mathbb{A} \times \mathbb {B}_3, \\ \mathbb {C}[a,b_4] & = [t-a, t+a]\times [{\mathbb{S}_{R}}-b_4, {\mathbb{S}_{R}}+b_4] = \mathbb{A} \times \mathbb {B}_4, \\ \mathbb {C}[a,b_5] & = [t-a, t+a]\times [{\mathbb{S}_{E}}-b_5, {\mathbb{S}_{E}}+b_5] = \mathbb{A} \times \mathbb {B}_5. \end{align*}$

Consider a uniform norm on $\mathbb {C}[a, b_\iota], (\iota = 1, 2, 3, 4, 5)$ as given by

$\left \| U(t) \right \|_\infty = \sup\limits_{t \in [t-a,t+a]}\left | U(t) \right |.$

We define the Picard operator as:

$\triangle : \mathbb {C}(\mathbb{A},\mathbb {B}_1, \mathbb {B}_2, \mathbb {B}_3, \mathbb {B}_4, \mathbb {B}_5)\rightarrow \mathbb {C}(\mathbb{A},\mathbb {B}_1, \mathbb {B}_2, \mathbb {B}_3, \mathbb {B}_4, \mathbb {B}_5)$

described by

$\triangle (U(t)) = U_{0}(t) + \Omega(r) N(t, U(t)) + \omega (r) \int_{0}^{t} N(y,U(y))dy,$

where

$\begin{align*} \begin{aligned} U(t)& = \left\{\mathbb{S}_{12}(t), \mathbb{S}_3(t), {\mathbb{S}_{4}}(t), {\mathbb{S}_{R}}(t), {\mathbb{S}_{E}}(t)\right\},\\ U_0(t)& = \left\{ \mathbb{S}_{12}(0), \mathbb{S}_3(0), {\mathbb{S}_{4}}(0), {\mathbb{S}_{R}}(0), {\mathbb{S}_{E}}(0)\right\}, \\ N(t,U(t))& = \left\{ N_1(t,\mathbb{S}_{12}),N_2(t,\mathbb{S}_3),N_3(t,{\mathbb{S}_{4}}),N_4(t,{\mathbb{S}_{R}}),N_5(t,{\mathbb{S}_{E}}) \right\}.\end{aligned} \end{align*}$

We consider the solutions to the problem under examination are bounded within a time interval, that is,

$\left \| U(t) \right \|_\infty \leqslant max \left\{ b_1,b_2,b_3,b_4,b_5\right\} = b.$

Let

$N^{*} = max \left\{ N^{*}_1,N^{*}_2,N^{*}_3,N^{*}_4,N^{*}_5\right\},$

and $\exists$ $t_0$ , so $t\leqslant t_0$ , then

$\begin{align*} & \left \| \triangle U(t)-U_{0}(t) \right \| \\ & = \left \| \Omega(r)N(t,U(t))+\omega(r) \int_{0}^{t} N(y,U(y))dy \right \|\\ & \leqslant \Omega(r)\left \| N(t,U(t)) \right \|+\omega(r) \int_{0}^{t} \left \| N(y,U(y))\right \|dy \\ & \leqslant \left ( \Omega(r)+\omega(r)t \right )N^{*} \\ & \leqslant \left ( \Omega(r)+\omega(r)t_{0} \right )N^{*}\\ & \leqslant \mu^{*}N^{*} \\&\leqslant b, \end{align*}$

where

$\mu^{*} = \left ( \Omega(r)+\omega(r)t_{0} \right ) \leqslant \frac{b}{N^{*}}.$

Further, we prove the following equality:

$\left \| \triangle U_1 - \triangle U_2 \right \| = \sup\limits_{t \in \mathbb{A}} \left | U_1(t)-U_2(t) \right |.$

Using the Picard operator, we get

$\begin{align*} &\left \| \triangle U_1 - \triangle U_2 \right \|\\ & = \left \| \Omega(r) \left\{N(t,U_1(t)) -N(t,U_2(t))\right\} \right.\\ & \left . \quad +\omega(r) \int_{0}^{t} \left\{N(y,U_1(y))-N(y,U_2(y))\right\} dy \right \|\\ & \leqslant \Omega(r) \left \| N(t,U_1(t)) -N(t,U_2(t)) \right \| \\ &\quad +\omega(r) \int_{0}^{t}\left \| N(y,U_1(y))-N(y,U_2(y)) \right \| dy\\ & \leqslant \Omega(r) \gamma^{*} \left \| U_1(t) -U_2(t)\right \| +\omega(r) \gamma^{*}\int_{0}^{t}\left \|U_1(y)-U_2(y)\right \| dy\\ & \leqslant \left(\Omega(r)+\omega(r)t_{0}\right)\gamma^{*}\left \|U_1(y)-U_2(y)\right \| \\ & \leqslant \mu^{*} \gamma^{*}\left \|U_1(y)-U_2(y)\right \| \end{align*}$

with $\gamma^{*} < 1.$ Since $N$ is a contraction, then $\mu^{*}\gamma^{*} < 1$ , so the discussed operator $\Delta$ is a contraction. Hence, system (3.2) has a unique solution.

5. Stability analysis of the proposed fractional model

In this section, we discuss the Ulam-Hyres (UH) stabiity (such as ^[59,60]) of the proposed fractional model (3.2) using the notion of nonlinear functional analysis. For the sake of simplicity we consider the proposed model (3.2) as:

$\begin{gather} \left\{\begin{array}{ll} {}^{\mathsf{cf}}_{0} D^{r}_{t} \mathbb{B}(t) = \Theta \left(t, \mathbb{B}(t)\right),\\ \quad \mathbb{B}(0) = \mathbb{B}_0 \geq 0, \end{array}\right. \end{gather}$

(5.1)

where

$\begin{align*} \mathbb{B}(t)& = \left(\mathbb{S}_{12}(t), \mathbb{S}_3(t), {\mathbb{S}_{4}}(t), {\mathbb{S}_{R}}(t), {\mathbb{S}_{E}}(t)\right)^{T},\\ \mathbb{B}_0& = \left(\mathbb{S}_{12_0},\mathbb{S}_{3_0}, {\mathbb{S}_{4_0}},{\mathbb{S}_{R_0}}, {\mathbb{S}_{E_0}}\right)^{T},\\ \Theta (t, \mathbb{B}(t))& = \left( N_1, N_2, N_3, N_4, N_5\right)^{T}. \end{align*}$

Applying fractional integral (2.2) on (5.1), we get

$\begin{equation} \mathbb{B}(t) = \mathbb{B}_{0} + \Omega(r) \Theta (t, \mathbb{B}(t)) + \omega (r) \int_{0}^{t} \Theta (\delta, \mathbb{B}(\delta))d\delta. \end{equation}$

(5.2)

Definition 5.1. The proposed system (3.2) is UH stable if $\exists$ $\overline{\mu} > 0$ with the following property. For any $\epsilon > 0$ and $\overline {\mathbb{B}} \in \mathsf{B}$ (Banach space), if

$\begin{equation} \left | {}^{\mathsf{cf}}_{0} D^{r}_{t} \overline{\mathbb{B}}(t) - \Theta \left(t, \overline{\mathbb{B}}(t)\right) \right |\leq \epsilon, \end{equation}$

(5.3)

then $\exists$ $\mathbb{B} \in \mathsf{B}$ satisfies system (3.2) with initial condition

$\mathbb{B}(0) = \overline{\mathbb{B}}(0) = \overline{\mathbb{B}}_0,$

such that

$\left \| \overline{\mathbb{B}}-\mathbb{B} \right \| \leq \overline{\mu}\epsilon.$

where

$\begin{gather*} \label{CFMP} \left\{\begin{array}{ll} \quad \overline{\mathbb{B}}(t) = \left(\overline{\mathbb{S}}_{12}(t), \overline{\mathbb{S}}_3(t), \overline{\mathbb{S}}_{4}(t), \overline{\mathbb{S}}_{R}(t), \overline{\mathbb{S}}_{E}(t)\right)^{T},\\ \quad \mathbb{B}_0 = \left( \overline{\mathbb{S}}_{12_0}, \overline{\mathbb{S}}_{3_0}, {\overline{\mathbb{S}}_{4_0}}, {\overline{\mathbb{S}}_{R_0}},{\overline{\mathbb{S}}_{E_0}}\right)^{T},\\ \Theta (t, \overline{\mathbb{B}}(t)) = \left(\overline{N}_1, \overline{N}_2, \overline{N}_3, \overline{N}_4, \overline{N}_5\right)^{T},\\ \quad \epsilon = max (\epsilon_{j})^T; \; \; \; j = 1,2,3,4,5, \\ \quad \overline{\mu} = max (\overline{\mu}_{j})^T;\; \; \; j = 1,2,3,4,5. \end{array}\right. \end{gather*}$

Remark 5.1. Consider a small perturbation $k \in \mathsf{C}[0, \mathsf{T}]$ , such that $k(0) = 0$ along with the following property : $\left | k(t) \right |\leq \overline{\epsilon }$ , for $t \in [0, \mathsf{T}]$ and $\overline{\epsilon} > 0$ ,

Lemma 5.1. ^[10] The solution $\overline{\mathbb{B}}_k(t)$ of the perturbed system

$\begin{align} {}^{\mathsf{cf}}_{0} D^{r}_{t} \overline{\mathbb{B}}(t) = \Theta \left(t, \overline{\mathbb{B}}(t)\right)+ k(t),\quad \overline{\mathbb{B}}(0) = \overline{\mathbb{B}}_{0}, \end{align}$

(5.4)

saisfies the relation:

$\left \| \overline{\mathbb{B}}_k(t)- \overline{\mathbb{B}}(t) \right\|\leq \Phi\overline{\epsilon},$

where

$\begin{align*} \Phi & = \Omega (r)+\omega (r)T,\\ k(t) & = \left( k_1(t), k_2(t), k_3(t), k_4(t), k_5(t)\right)^T. \end{align*}$

Proof. Applying fractional integral (2.2) on (5.4), we get

$\begin{align} \overline{\mathbb{B}}_k(t) = & \overline{\mathbb{B}}_{0} + \Omega(r) \Theta \left(t, \overline{\mathbb{B}}(t)\right) + \omega (r) \int_{0}^{t} \Theta \left (\delta, \overline{\mathbb{B}}(\delta)\right)d\delta\\& + \Omega(r) k(t) + \omega (r) \int_{0}^{t} k(\delta) d\delta. \end{align}$

(5.5)

Also,

$\begin{align} \overline{\mathbb{B}}(t) & = \overline{\mathbb{B}}_{0} + \Omega(r) \Theta \left(t, \overline{\mathbb{B}}(t)\right) + \omega (r) \int_{0}^{t} \Theta \left (\delta, \overline{\mathbb{B}}(\delta)\right)d\delta. \end{align}$

(5.6)

Using Remark 5.1,

$\begin{align*} \left \| \overline{\mathbb{B}}_k(t)- \overline{\mathbb{B}}(t) \right \| &\leq \Omega(r) \left |k(t) \right| + \omega (r) \int_{0}^{t} \left |k(\delta) \right| d\delta \\ &\leq \left ( \Omega (r)+\omega (r)T \right)\overline{\epsilon} \\& = \Phi \overline{\epsilon}. \end{align*}$

This completes the proof. □

Theorem 5.1. ^[10] The proposed fractional system (3.2) is UM stable if

$\left \| \overline{\mathbb{B}}(t)-\mathbb{B}(t) \right \| \leq \overline{\mu} \overline{\epsilon}.$

Proof. Let $\overline{\mathbb{B}}$ be the solution of (5.3) and, due to uniqueness, $\mathbb{B}$ be a unique solution of the system (5.1), then

$\begin{align*} \begin{aligned} \left\|\overline{\mathbb{B}}(t)- {\mathbb{B}}(t) \right\| \leq& \left\|\overline{\mathbb{B}}_h (t)- \overline{\mathbb{B}}(t) \right\| +\left\|\overline{\mathbb{B}}_h (t) - {\mathbb{B}}(t) \right\| \\ \leq& \Phi \overline{\epsilon}+\Omega(r) \left \| \Theta ( t,\overline{\mathbb{B}}(t)) - \Theta ( t,\mathbb{B}(t))\right\| \\ & +\omega (r) \int_{0}^{t} \left \| \Theta ( \delta,\overline{\mathbb{B}}(\delta)) - \Theta ( \delta,\mathbb{B}(\delta))\right\| d\delta + \Phi \overline{\epsilon}\\ \leq& 2 \Phi \overline{\epsilon} + \Phi \overline{\delta} \left \|\overline{\mathbb{B}}(t))-\mathbb{B}(t)) \right \|,\end{aligned} \end{align*}$

which implies that

$\left\|\overline{\mathbb{B}}(t)- {\mathbb{B}}(t) \right\|\leq \frac{2 \Phi \overline{\epsilon}}{1-\Phi \overline{\delta}} = \overline{\mu}\overline{\epsilon},$

where

$\overline{\mu} = \frac{2 \Phi}{1-\Phi \overline{\delta}}.$

Hence, the considered fractional system (3.2) is UM stable. □

6. Numerical algorithm on CF frame for dynamics of breast cancer model

The present part of the paper provides an approximate solution for the fractional order model (3.2) using two-step fractional Adams-Bashforth technique ^[37]. We discretized model (3.2) as follows:

$\begin{equation} \begin{aligned} {\mathbb{S}_{12}}_{\iota+1}& = {\mathbb{S}_{12}}_\iota + M_1(r) N_1(t_\iota,{\mathbb{S}_{12}}_\iota,{\mathbb{S}_3}_\iota,{\mathbb{S}_{4}}_\iota,{\mathbb{S}_{R}}_\iota,{\mathbb{S}_{E}}_\iota)- M_2(r)\\& \quad N_1(t_{\iota-1},{\mathbb{S}_{12}}_{\iota-1},{\mathbb{S}_3}_{\iota-1},{\mathbb{S}_{4}}_{\iota-1},{\mathbb{S}_{R}}_{\iota-1},{\mathbb{S}_{E}}_{\iota-1}),\\ {\mathbb{S}_3}_{\iota+1}& = {\mathbb{S}_3}_\iota + M_1(r) N_2(t_\iota,{\mathbb{S}_{12}}_\iota,{\mathbb{S}_3}_\iota,{\mathbb{S}_{4}}_\iota,{\mathbb{S}_{R}}_\iota,{\mathbb{S}_{E}}_\iota)- M_2(r) \\& \quad N_2(t_{\iota-1},{\mathbb{S}_{12}}_{\iota-1},{\mathbb{S}_3}_{\iota-1},{\mathbb{S}_{4}}_{\iota-1},{\mathbb{S}_{R}}_{\iota-1},{\mathbb{S}_{E}}_{\iota-1}),\\ {\mathbb{S}_{4}}_{\iota+1}& = {\mathbb{S}_{4}}_\iota + M_1(r) N_3(t_\iota,{\mathbb{S}_{12}}_\iota,{\mathbb{S}_3}_\iota,{\mathbb{S}_{4}}_\iota,{\mathbb{S}_{R}}_\iota,{\mathbb{S}_{E}}_\iota)- M_2(r) \\& \quad N_3(t_{\iota-1},{\mathbb{S}_{12}}_{\iota-1},{\mathbb{S}_3}_{\iota-1},{\mathbb{S}_{4}}_{\iota-1},{\mathbb{S}_{R}}_{\iota-1},{\mathbb{S}_{E}}_{\iota-1}),\\ {\mathbb{S}_{R}}_{\iota+1}& = {\mathbb{S}_{R}}_\iota + M_1(r) N_4(t_\iota,{\mathbb{S}_{12}}_\iota,{\mathbb{S}_3}_\iota,{\mathbb{S}_{4}}_\iota,{\mathbb{S}_{R}}_\iota,{\mathbb{S}_{E}}_\iota)- M_2(r)\\& \quad N_4(t_{\iota-1},{\mathbb{S}_{12}}_{\iota-1},{\mathbb{S}_3}_{\iota-1},{\mathbb{S}_{4}}_{\iota-1},{\mathbb{S}_{R}}_{\iota-1},{\mathbb{S}_{E}}_{\iota-1}),\\ {\mathbb{S}_{E}}_{\iota+1}& = {\mathbb{S}_{E}}_\iota + M_1(r) N_5(t_\iota,{\mathbb{S}_{12}}_\iota,{\mathbb{S}_3}_\iota,{\mathbb{S}_{4}}_\iota,{\mathbb{S}_{R}}_\iota,{\mathbb{S}_{E}}_\iota)- M_2(r)\\& \quad N_5(t_{\iota-1},{\mathbb{S}_{12}}_{\iota-1},{\mathbb{S}_3}_{\iota-1},{\mathbb{S}_{4}}_{\iota-1},{\mathbb{S}_{R}}_{\iota-1},{\mathbb{S}_{E}}_{\iota-1}),\\ \end{aligned} \end{equation}$

(6.1)

where

$M_1(r) = \left(\frac{1-r}{P(r)}+\frac{3rh}{2P(r)}\right)$

and

$M_2(r) = \left(\frac{1-r}{P(r)}+\frac{rh}{2P(r)}\right).$

7. Numerical simulation and discussion

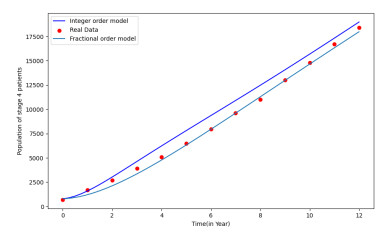
The dynamical behavior of the proposed fractional system (3.2) is investigated numerically using (6.1) for the approximate solution of the state variables ${\mathbb{S}_{12}}(t), \mathbb{S}_3(t), {\mathbb{S}_{4}}(t), {\mathbb{S}_{R}}(t)$ , and ${\mathbb{S}_{E}}(t)$ in (3.2). We take the initial values as ${\mathbb{S}_{12}}(0) = 30000, \ \mathbb{S}_3(0) = 12300, \ {\mathbb{S}_{4}}(0) = 783, \ {\mathbb{S}_{R}}(0) = 334, \ {\mathbb{S}_{E}}(0) = 10$ and parameters values: $\Delta = 14000, \ \Gamma = 80, \ \Omega = 90, \ \mu = 0.01$ , $\ \nu = 0.034, \ \psi = 0.03, \ \phi = 0.3, \ \omega = 0.1, \ \zeta = 0.2$ , $\ \chi = \delta = \eta = 0.0256$ ^[56], $\rho = 0.149 (fitted), \ \kappa = 0.09 (fitted), \ \sigma = 0.47 (fitted)$ , and $\tau = 0.01(fitted)$ , as fitted with real data. In Figure 1, we used real data ^[57] from Kingdom of Saudi Arabia from 2004 to 2016 to fit the classical model (3.1) and the suggested fractional order model (3.2). This demonstrates that the fractional model better matches the actual data and may be used to forecast future instances than the classical model. Figure 2 shows a long-term estimate of the cases based on a fractional model. Here, we can see from Figure 2 that the data fits the model curve well, and we can also see that the number of long-term behavior cases grows in an exponential way over time. This case could be scary because the number of cases could go up even more in the next few years if the health department does not use the right treatment methods to get rid of breast cancer.

Figure 1. Fitting real data of breast cancer with integer and fractional order(

$r = 1$ ) models.

[1]	B. Abdelmoumen, S. Yengui, Perturbation theory, M-essential spectra of operator matrices, Filomat, 34 (2020), 1187–1196. https://doi.org/10.2298/FIL2004187A doi: 10.2298/FIL2004187A
[2]	Y. A. Abramovich, C. D. Aliprantis, An invitation to operator theory, Providence: Am. Math. Soc., 2002. https://doi.org/10.1090/gsm/050
[3]	A. Ammar, B. Boukettaya, A. Jeribi, A note on essential pseudospectra and application, Linear Multilinear A., 64 (2016), 1474–1483. https://doi.org/10.1080/03081087.2015.1091436 doi: 10.1080/03081087.2015.1091436
[4]	A. Ammar, A. Jeribi, A characterization of the essential pseudospectra on a Banach space, Arab. J. Math., 2 (2013), 139–145. https://doi.org/10.1007/s40065-012-0065-7 doi: 10.1007/s40065-012-0065-7
[5]	Q. Bai, J. Huang, A. Chen, Essential, Weyl and Browder spectra of unbounded upper triangular operator matrices, Linear Multilinear A., 64 (2016), 1583–1594. https://doi.org/10.1080/03081087.2015.1111290 doi: 10.1080/03081087.2015.1111290
[6]	C. Belabbaci, New characterizations of the Jeribi essential spectrum, Int. J. Anal. Appl., 21 (2023), 109–109. https://doi.org/10.28924/2291-8639-21-2023-109 doi: 10.28924/2291-8639-21-2023-109
[7]	C. Belabbaci, The S-Jeribi essential spectrum, Ukrainian Math. J., 73 (2021), 359–366. https://doi.org/10.1007/s11253-021-01929-8 doi: 10.1007/s11253-021-01929-8
[8]	E. B. Davies, Spectral theory and differential operators, Cambridge: Cambridge University Press, 1995. https://doi.org/10.1017/CBO9780511623721
[9]	D. E. Edmunds, W. D. Evans, Spectral theory and differential operators, New York: Oxford University Press, 2018. https://doi.org/10.1093/oso/9780198812050.002.0005
[10]	A. Jeribi, A characterization of the Schechter essential spectrum on Banach spaces and applications, J. Math. Anal. Appl., 271 (2002), 343–358. https://doi.org/10.1016/S0022-247X(02)00115-4 doi: 10.1016/S0022-247X(02)00115-4
[11]	A. Jeribi, Linear operators and their essential pseudospectra, Canada: Apple Academic Press, 2018. https://doi.org/10.1201/9781351046275
[12]	A. Jeribi, Some remarks on the Schechter essential spectrum and applications to transport equations, J. Math. Anal. Appl., 275 (2002), 222–237. https://doi.org/10.1016/S0022-247X(02)00323-2 doi: 10.1016/S0022-247X(02)00323-2
[13]	A. Jeribi, Spectral theory and applications of linear operators and block operator matrices, New York: Springer-Verlag, 2015. https://doi.org/10.1007/978-3-319-17566-9
[14]	A. Jeribi, N. Moalla, A characterization of some subsets of schechter's essential spectrum and application to singular transport equation, Math. Anal. Appl., 358 (2009), 434–444. https://doi.org/10.1016/j.jmaa.2009.04.053 doi: 10.1016/j.jmaa.2009.04.053
[15]	A. Jeribi, N. Moalla, S. Yengui, S-essential spectra and application to an example of transport operators, Math. Method. Appl. Sci., 37 (2014), 2341–2353. https://doi.org/10.1002/mma.1564 doi: 10.1002/mma.1564
[16]	N. Karapetiants, S. Samko, Equations with involutive operators, United states: Birkhäuser Boston, 2012. http://dx.doi.org/10.1007/978-1-4612-0183-0
[17]	V. Müller, Spectral theory of linear operator and spectral systems in Banach algebras, operator theory: Advances and applications, Basel: Birkhäuser Verlag, 2007. https://doi.org/10.1007/978-3-7643-8265-0
[18]	M. Schechter, Principles of functional analysis, Providence: Am. Math. Soc., 2002. https://doi.org/10.1090/gsm/036
[19]	L. N. Trefethen, Spectra and pseudospectra: The behavior of nonnormal matrices and operators, Princeton University Press, Princeton, NJ, 2005. https://doi.org/10.1515/9780691213101
[20]	F. Wolf, On the invariance of the essential spectrum under a change of boundary conditions of partial differential boundary operators, Indag. Math., 21 (1959), 142–147. https://doi.org/10.1016/S1385-7258(59)50016-5 doi: 10.1016/S1385-7258(59)50016-5

1.	Anil Chavada, Nimisha Pathak, Sagar R. Khirsariya, Fractional‐order modeling of Chikungunya virus transmission dynamics, 2024, 0170-4214, 10.1002/mma.10372
2.	Parveen Kumar, Sunil Kumar, Badr Saad T Alkahtani, Sara S Alzaid, A mathematical model for simulating the spread of infectious disease using the Caputo-Fabrizio fractional-order operator, 2024, 9, 2473-6988, 30864, 10.3934/math.20241490
3.	Twinkle R Singh, A novel analytical iterative approach to time-fractional Vibration equation using Aboodh transform, 2025, 100, 0031-8949, 015296, 10.1088/1402-4896/ad9e4c
4.	A. M. Alqahtani, Shivani Sharma, Arun Chaudhary, Aditya Sharma, Application of Caputo-Fabrizio derivative in circuit realization, 2025, 10, 2473-6988, 2415, 10.3934/math.2025113
5.	Akanksha Singh, Anil Chavada, Nimisha Pathak, Fractional Calculus Approach to Pancreatic Cancer Therapy: Modeling Tumor and Immune Interactions with siRNA Treatment, 2025, 11, 2349-5103, 10.1007/s40819-025-01873-2

AIMS Mathematics

A characterization of Wolf and Schechter essential pseudospectra

Related Papers:

Abstract

1. Introduction

2. Preliminaries

3. Formulation of fractional mathematical model

4. Qualitative analysis of fractional system

5. Stability analysis of the proposed fractional model

6. Numerical algorithm on CF frame for dynamics of breast cancer model

7. Numerical simulation and discussion

8. Conclusions

Use of AI tools declaration

Acknowledgments

Conflict of interest

References

This article has been cited by:

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Other Articles By Authors

Related pages

Tools

Export File

Citation

Format

Content

Catalog