Abbreviation
N: nutrient, it is produced from a source at a fixed rate, cells grow as a result of consuming nutrient; M: epithelial cells, the type of cells in the lungs that are infected by SARS-CoV-2; C cancer cells: the cells characterized by uncontrolled growth that become infected by SARS-CoV-2; V: the free SARS-CoV-2 particles, the virus responsible for COVID-19, these particles infect epithelial and cancer cells; T: anti-cancer CTLs, the immune cells that specifically target and eliminate cancer cells; A: antibodies, they are used by the immune system to eliminate virus particles from the body
1.
Introduction
COVID-19 is a disease caused by severe acute respiratory syndrome coronavirus 2 (SARS-CoV-2). While there has been a decline in reported cases and deaths globally, SARS-CoV-2 is still spreading in many countries [1]. SARS-CoV-2 passes into host cells by way of a transmembrane protein known as the angiotensin-converting enzyme 2 receptor [2]. It principally causes infection in alveolar epithelial type-II cells of the lungs [3,4]. However, other organs can be infected by SARS-CoV-2. The impact of COVID-19 on cancer patients has opened up a wide field of research. This group of patients is vulnerable to COVID-19 due to weakened immune system or ongoing anti-cancer treatments [5]. The question of whether SARS-CoV-2 induces remission in cancer patients or exacerbates the severity of the disease remains controversial [5,6].
The interconnection between cancer and viruses has become one of the most important topics in oncology and virology [7]. Some viruses, called oncolytic viruses (OVs), have the ability to infect cancer cells. These viruses can be found in nature or genetically modified to replicate in cancer cells without infecting normal cells [5,8]. OVs kill cancer cells after massive replication inside them and by inducing a specific antitumor immune response [5,8]. Examples of OVs include adenovirus, vaccinia virus, Coxsackievirus, and herpes simplex virus [8,9]. Talimogene laherparepvec is the only approved oncolytic virotherapy [6,10]. Talimogene laherparepvec is an engineered herpes virus used to treat advanced melanoma through immediate injection into the tumor [10].
The impact of COVID-19 on cancer patients is bidirectional. It has been indicated that SARS-CoV-2 infection can enhance cancer progression [5,7,8,11]. On the other hand, cancer remission after SARS-CoV-2 infection has been reported in many patients [6,12,13,14]. For example, Pasin et al. [15] reported the case of a patient with refractory natural killer (NK)/T-cell lymphoma who experienced a transient remission during SARS-CoV-2 infection. As angiotensin-converting enzyme 2 is expressed in NK cells, the authors in [15] proposed that SARS-CoV-2 could own some oncolytic properties. Challenor and Tucker [16] presented the case of a remission in a patient with classical Hodgkin lymphoma after SARS-CoV-2 infection. Another case was reported by Sollini et al. [17] involving a patient with follicular lymphoma who achieved full remission after SARS-CoV-2 infection. The authors in [16,17] supposed that the infection stimulated an antitumor immune response. Kandeel et al. [18] reported the remission of two cases with acute leukemia. Antwi-Amoabeng et al. [19] presented a case in which a patient with multiple myeloma had remission following SARS-CoV-2 infection. The patient received a single dose of chemotherapy. However, the authors mentioned that the remission in this case was parallel to the remission in patients who got four doses of chemotherapy. Ohadi et al. [20] reported a case of mycosis fungoides that went into remission after the coronavirus infection. Other cases of remission were reported in [6].
The above remission cases suggest that SARS-CoV-2 could have an oncolytic role in many types of cancer. It may infect and destroy cancer cells to expose the tumor-associated antigens. These antigens stimulate an immune response against cancer cells, leading to cancer remission [18]. Hence, there is an urgent need to understand the relationship between SARS-CoV-2 infection and cancer. This understanding may enable the engineering of SARS-CoV-2 for use as an efficient therapy against certain types of cancer. Mathematical modeling is a strong tool that is often employed to assist experiments and medical research [21,22]. Mathematical models have been used to understand the dynamics of many infectious diseases and test hypotheses that may be challenging to assess experimentally. The analysis of these models can offer predictions of outcomes and aid in identifying optimal treatment strategies.
Many mathematical models about SARS-CoV-2 [23,24,25], cancer [26], SARS-CoV-2/cancer [27], and oncolytic virotherapy [28,29,30,31,32] have been constructed and studied. However and to the best of our knowledge, no oncolytic SARS-CoV-2 models have been established yet. Such models are important to understand the effect of the infection of cancer cells by SARS-CoV-2 and the role of different immune responses during this coinfection. In this work, we propose an oncolytic SARS-CoV-2 virotherpy model. The construction of this model follows similar principles of those used in [33]. We conduct a comprehensive mathematical analysis of this model including assessments of boundedness, nonnegativity, and global stability of equilibrium points. In addition, we implement some numerical simulations.
This paper is structured as follows. Section 2 introduces the model under consideration. Section 3 demonstrates that all solutions are bounded and have zero or positive values. Furthermore, it computes the equilibrium solutions of the proposed model. Section 4 verifies the global properties of these solutions. Section 5 is dedicated to numerical simulations. The last section discusses the results and provides a glimpse of the future vision.
2.
Oncolytic SARS-CoV-2 model with immune responses
In formulating the model, we consider the following assumptions:
(i) The nutrient is produced from a source at a fixed rate, while it is depleted due to its consumption by epithelial cells and cancer cells. Additionally, depletion occurs as a result of natural death;
(ii) Epithelial cells proliferate as a result of nutrient consumption, and their numbers decrease due to either viral infection or natural death;
(iii) Cancer cells replicate as a result of nutrient utilization, and their numbers decline due to viral infection, attacks by cytotoxic T lymphocytes (CTLs), or natural cell death;
(iv) Free virus particles increase as a consequence of infecting epithelial cells and cancer cells, but they diminish due to the removal by antibodies or natural death;
(v) CTLs are stimulated by infected cancer cells, while antibodies are stimulated by free virus particles;
(vi) CTLs and antibodies undergo decay through natural processes;
(vii) The induction of CTLs by SARS-CoV-2 infection is implied in the stimulation rate of CTLs;
(viii) The model does not contain infected components for epithelial cells and cancer cells.
The proposed ordinary differential equation (ODE) model consists of six nonlinear equations and takes the form
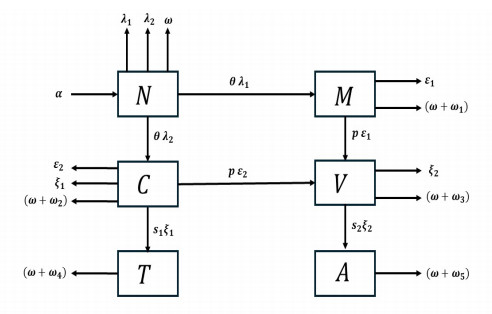
where N(t), M(t), C(t), V(t), T(t), and A(t) typify the concentrations of nutrient, epithelial cells, cancer cells, SARS-CoV-2 particles, anti-cancer CTLs, and antibodies. The nutrient is released from its source at rate α and declines at rate ωN. Epithelial cells expend nutrient at rate λ1NM, reproduce at rate θλ1NM, and get infected by SARS-CoV-2 at rate ϵ1MV. Cancer cells expend nutrient at rate λ2NC, grow at rate θλ2NC, and become infected at rate ϵ2CV. SARS-CoV-2 replicates as a result of infecting epithelial cells and cancer cells at rates pϵ1MV and pϵ2CV, respectively. CTLs kill cancer cells at rate ξ1CT and reproduce at rate s1ξ1CT. Antibodies eliminate SARS-CoV-2 at rate ξ2VA and get stimulated at rate s2ξ2VA. Epithelial cells, cancer cells, SARS-CoV-2 particles, CTLs, and antibodies die at natural rates ω1M, ω2C, ω3V, ω4T, and ω5A, respectively. The parameters in model (2.1) are postulated to take positive values. Figure 1 provides a schematic diagram of the model. For simplicity, we consider the following
3.
Basic properties
The following theorem demonstrates the existence and uniqueness of the solutions of model (2.1).
Theorem 1. Assume that the initial values (N0,M0,C0,V0,T0,A0)∈R6 are given. There exists t0>0 and continuously differentiable functions N,M,C,V,T,A: [0,t0)→R such that (N,M,C,V,T,A) satisfies model (2.1) and
Proof. As the system of ODEs given in (2.1) is autonomous, it is enough to prove that the function f: R6→R6 defined by
is locally Lipschitz in its z argument. We observe that the Jacobian matrix
is linear in z and consequently locally bounded for all z∈R6. Therefore, f has a continuous and bounded derivative on any compact subset of R6, and so f is locally Lipschitz in z. According to the classical Picard-Lindelöf theorem [34], there exists a unique solution z(t) to the ODE
on the time interval [0,t0] for some t0>0 [35]. □
Next, we prove the nonnegativity and boundedness of the solutions of model (2.1).
Theorem 2. Let τi>0 (i=1,2,3,4,5), then the set
is positively invariant set for system (2.1).
Proof. For system (2.1), we obtain
This shows that
for t≥0 whenever
To prove the boundedness, we consider the function
By computing dχ(t)dt, we get
where
This implicates that
where
Consequently, we have N(t)≤τ1, M(t)≤τ2, C(t)≤τ2, V(t)≤τ3, T(t)≤τ4, and A(t)≤τ5, where τ2=θτ1, τ3=θpτ1, τ4=θs1τ1, and τ5=θps2τ1. Thus, the set Ω is positively invariant [36]. □
Theorem 3. Model (2.1) has thirteen equilibrium points as follows:
(1) The trivial equilibrium E0 always exists;
(2) The uninfected-epithelial equilibrium E1 exists if R0>1;
(3) The uninfected-cancer equilibrium E2 exists if R1>1;
(4) The infected-epithelial equilibrium E3 exists if
(5) The infected-cancer equilibrium E4 exists if
(6) The uninfected-cancer equilibrium with CTLs E5 exists if
(7) The infected epithelial-cancer equilibrium without immunity E6 exists if
(8) The uninfected epithelial-cancer equilibrium with CTLs E7 exists if
(9) The infected-epithelial equilibrium with antibodies E8 exists if
(10) The infected-cancer equilibrium with antibodies E9 exists if
(11) The infected-cancer equilibrium with CTLs and antibodies E10 exists if
(12) The infected epithelial-cancer equilibrium with CTLs E11 exists if
and
(13) The infected epithelial-cancer equilibrium with CTLs and antibodies E12 exists if
and
Proof. To get the equilibria of system (2.1), we solve the following system:
Then, we obtain
(1) The trivial equilibrium
This point always exists. This point has no biological meaning as all components vanish except for the nutrient.
(2) The uninfected-epithelial equilibrium E1=(N1,M1,0,0,0,0). The components N1 and M1 are defined as:
where
As N1>0, the equilibrium E1 exists if R0>1. This equilibrium represents a healthy individual without cancer or SARS-CoV-2 infection.
(3) The uninfected-cancer equilibrium E2=(N2,0,C2,0,0,0), where
where
As N2>0, the point E2 exists if R1>1. This equilibrium represents the case of a person who has cancer, but without SARS-CoV-2 infection.
(4) The infected-epithelial equilibrium E3=(N3,M3,0,V3,0,0), where
and
Clearly, N3>0, M3>0, and V3>0 if
Hence, E3 exists if
The person here suffers from SARS-CoV-2 infection, but he is cancer-free.
(5) The infected-cancer equilibrium E4=(N4,0,C4,V4,0,0), where
and
Notably, N4>0, C4>0, and V4>0 if
Thus, E4 exists if
In this scenario, the cancer patient is experiencing a SARS-CoV-2 infection with the disappearance of healthy epithelial cells.
(6) The uninfected-cancer equilibrium with CTLs E5=(N5,0,C5,0,T5,0), where
and
Clearly, N5>0, C5>0, and T5>0 if
Hence, E5 exists if
CTLs are activated to eliminate cancer cells in a patient who has experienced the disappearance of healthy epithelial cells.
(7) The infected epithelial-cancer equilibrium without immunity E6=(N6,M6,C6,V6,0,0), where
We note that the components are positive if
Thus, E6 is defined when the above conditions are met. In this scenario, the cancer patient has SARS-CoV-2 infection with inactive immune responses.
(8) The uninfected epithelial-cancer equilibrium with CTLs E7=(N7,M7,C7,0,T7,0), where
Accordingly, N7>0, C7>0, M7>0 if
and T7>0 if
Therefore, E7 exists when
Here, the cancer patient has active CTL immunity that works on killing cancer cells.
(9) The infected-epithelial equilibrium with antibodies E8=(N8,M8,0,V8,0,A8), where
Thus, the components are positive and E8 exists if
Notably, the first condition is naturally satisfied when the second condition is met.
The SARS-CoV-2 patient has active antibody immunity against the virus.
(10) The infected-cancer equilibrium with antibodies E9=(N9,0,C9,V9,0,A9), where
We note that E9 is defined when
where the other condition
is naturally met when the previous condition is satisfied.
The infected cancer patient who suffers from the disappearance of epithelial cells has active antibody immunity against the virus.
(11) The infected-cancer equilibrium with CTLs and antibodies E10=(N10,0,C10,V10,T10,A10), where
Thus, E10 is biologically accepted when
This point represents the case of a cancer patient who is infected, with active immune responses, but experiencing the disappearance of epithelial cells.
(12) The infected epithelial-cancer equilibrium with CTLs E11=(N11,M11,C11,V11,T11,0), where
It is easy to observe that N11, V11, and T11 are positive and E11 exists when
and
At this point, the infected cancer patient has active CTLs and inactive antibody immune response.
(13) The infected epithelial-cancer equilibrium with CTLs and antibodies E12=(N12,M12,C12,V12,T12,A12), where
We observe that the components are positive and E12 is defined if
and
This point imitates the case of cancer patient with SARS-CoV-2 infection and active immune responses. □
In the next sections, we will focus our analysis on the equilibria E0, E1, E3, E6, E7, E8, E11, and E12 as we are interested in the points where the epithelial cells component (M) does not vanish.
4.
Global properties
The following theorems are aimed to establish the global stability of equilibria through nominating Lyapunov functions. Let Y′i be the largest invariant subset of
where i=0,1,3,6,7,8,11,12.
Theorem 4. The equilibrium E0 is globally asymptotically stable (GS) when R0≤1 and R1≤1.
Proof. We consider
Then, we get
We see that
if R0≤1 and R1≤1. Furthermore,
when N=N0 and M=C=V=A=0. This gives
From the third equation of model (2.1), we obtain T=0. Hence, Y′0={E0} and by LaSalle's invariance principle (LP) [37], E0 is GS if R0≤1 and R1≤1. □
Theorem 5. Let R0>1. Then, the equilibrium E1 is GS if
and
Proof. We opt
Then, we get
At equilibrium, E1 solves the system
Applying the above equations to collect (4.1) gives
Hence,
if
In addition,
if N=N1 and C=V=T=A=0. Consequently,
and the first equation of (2.1) gives M=M1. Therefore, Y′1={E1} and E1 is GS when
according to LP [37]. □
Theorem 6. Let
Then, the equilibrium E3 is GS if
and
Proof. We construct
Then, we get
The equilibrium conditions at E3 are
By using (6), Eq (4.2) becomes
We observe that
if
and
In addition, dΣ3dt=0 when N=N3, M=M3, V=V3 and C=T=A=0. Thus, Y′3={E3} and LP [37] implies that E3 is GS when
with the above conditions. □
Theorem 7. Let the existence conditions of E6 be satisfied. Then, the equilibrium E6 is GS if
and
Proof. See Appendix A. □
Theorem 8. Let
Then, the equilibrium E7 is GS if
Proof. See Appendix B. □
Theorem 9. Let
Then, E8 is GS if
Proof. See Appendix C. □
Theorem 10. Let
and
Then, E11 is GS if
Proof. See Appendix D. □
Theorem 11. Let
and
Then, the equilibrium E12 is GS.
Proof. We nominate
Then, we get
By using the conditions of equilibrium state at E12
Equation (4.4) is transformed into
We note that dΣ12dt≤0 and dΣ12dt=0 at E12. Based on LP [37], E12 is GS when the existence conditions are met. □
5.
Numerical simulations
The ode45 solver of Matlab is utilized to effectuate the numerical simulations. ode45 is the default solver for ODEs in Matlab. It utilizes an explicit Runge-Kutta formula and generally performs well with a wide range of ODE problems. Nevertheless, when dealing with stiff problems or situations demanding high accuracy, alternative solvers like ode15s, ode23s, and ode23t may prove more efficient. We consider three different groups of initial conditions:
(1) (N(0),M(0),C(0),V(0),T(0),A(0))=(0.0001,0.01,0.03,0.01,0.001,0.001);
(2) (N(0),M(0),C(0),V(0),T(0),A(0))=(0.1,0.03,0.06,0.05,0.002,0.003);
(3) (N(0),M(0),C(0),V(0),T(0),A(0))=(0.6,0.06,0.1,0.06,0.03,0.01).
The chosen sets of initial conditions are arbitrary, as the global stability is guaranteed for any initial values. To affirm the global stability of E0, E1, E3, E6, E7, E8, E11, and E12, we partition the numerical simulations into eight classes. We change the values of λ1, λ2, s1, s2, ϵ1, ϵ2, ξ2, ω2, ω3, and ω5 to obtain the global stability of the equilibrium in each case. The other values are fixed and given in Table 1.
Thus, we have
(i) We opt λ1=0.03, λ2=0.03, s1=0.1, s2=0.2, ϵ1=0.55, ϵ2=0.55, ξ2=4.88×10−8, ω2=0.17, ω3=0.6, and ω5=0.05. This yields R0=0.8<1 and R1=0.1263<1. Thus, E0=(1,0,0,0,0,0) is GS as indicated in Theorem 4 (see Figure 2). This point has no significant biological interpretation as all populations, except the nutrient's component, tend to zero.
(ii) We choose λ1=0.05, λ2=0.03, s1=0.1, s2=0.2, ϵ1=1×10−5, ϵ2=0.55, ξ2=4.88×10−8, ω2=0.17, ω3=0.9, and ω5=0.05. The corresponding thresholds are
Thus, E1=(0.75,0.1333,0,0,0,0) is GS which matches with Theorem 5 (see Figure 3). This simulates the case of an individual who neither has SARS-CoV-2 infection nor cancer.
(iii) We nominate λ1=0.07, λ2=0.03, s1=0.1, s2=0.2, ϵ1=0.5, ϵ2=0.55, ξ2=4.88×10−8, ω2=0.17, ω3=1×10−4, and ω5=0.2. This gives
and
This causes E3=(0.6304,0.1675,0,0.01061,0,0) to be GS as verified in Theorem 6 (see Figure 4). This imitates the case of a patient with SARS-CoV-2 infection but without cancer.
(iv) We pick out λ1=0.06, λ2=0.05, s1=0.1, s2=0.2, ϵ1=1×10−5, ϵ2=0.55, ξ2=4.88×10−8, ω2=0.001, ω3=0.001, and ω5=0.05. This corresponds to
and
In parallel with Theorem 7, E6=(0.625,0.06742,0.1591,0.0073,0,0) is GS (see Figure 5). This simulates the case of a cancer patient who has SARS-CoV-2 infection with inactive immune responses.
(v) We opt λ1=0.06, λ2=0.05, s1=1.2, s2=0.2, ϵ1=1×10−5, ϵ2=0.55, ξ2=4.88×10−8, ω2=1×10−4, ω3=0.9, and ω5=0.05. The resultant thresholds are
and
In agreement with Theorem 8, E7=(0.625,0.0333,0.2,0,0.0098,0) is GS (see Figure 6). In this scenario, the cancer patient does not have SARS-CoV-2 infection, and the CTL immunity against cancer cells is active.
(vi) We select λ1=0.07, λ2=0.03, s1=0.4, s2=1.6, ϵ1=0.5, ϵ2=0.55, ξ2=1.6, ω2=0.17, ω3=1×10−6, and ω5=1×10−8. This gives
and
As indicated in Theorem 9, E8=(0.6055,0.1862,0,0.0078,0,0.0015) is GS (see Figure 7). Here, the patient is solely affected by SARS-CoV-2 infection with an active immune response against the virus.
(vii) We consider λ1=0.06, λ2=0.06, s1=1.6, s2=0.2, ϵ1=1.4×10−1, ϵ2=0.55, ξ2=4.88×10−8, ω2=0.001, ω3=0.001, and ω5=0.05. This gives
and
As proved in Theorem 10, E11=(0.6422,0.0357,0.15,0.0059,0.01316,0) is GS (Figure 8). The CTL immunity against cancer cells is activated in the cancer patient infected with SARS-CoV-2.
(viii) We pick up λ1=0.06, λ2=0.06, s1=1.6, s2=1.9, ϵ1=1.4×10−1, ϵ2=1.2, ξ2=1.9, ω2=0.001, ω3=0.001, and ω5=0.000001. The corresponding thresholds are
and
As indicated in Theorem 11, E12=(0.641,0.03657,0.1499,0.006,0.006,0.0123) is GS (see Figure 9). The CTL and antibody immune responses are activated in the cancer patient infected with SARS-CoV-2.
To observe the impact of ϵ2 (the infection rate of cancer cells by SARS-CoV-2) on the concentration of cancer cells before stimulating any immune responses, we increase the value of ϵ2 in case (iv). When we set ϵ2=0.7, we get C6=0.125. Additionally, if we raise ϵ2 to 0.9, we find C6=0.097. Figure 10 shows the impact of increasing ϵ2 on the decrease in the concentration of cancer cells for other values of ϵ2. Thus, the infection of cancer cells by SARS-CoV-2 can lead to a reduction in cancer cells concentration, consequently resulting in a remission or an improvement in the patient's situation. Similarly, when we increase the value of ξ1 (the killing rate of cancer cells by CTLs) in case (viii), the concentration of cancer cells decreases to lower values (See Figure 11). In fact, these results align with many studies that suggest the ability of SARS-CoV-2 to infect cancer cells and induce immune responses, leading to cancer remission [15,16,17,18,19].
6.
Conclusions and discussion
Cancer remission after SARS-CoV-2 infection has been observed in many patients. This remission has been transient or complete, and it has been recorded with various types of cancer such as NK/T-cell lymphoma [15], Hodgkin lymphoma [16], follicular lymphoma [17], acute leukemia [18], and other types of cancer [6]. This has raised an urgent need to understand the relationship between cancer and SARS-CoV-2. This paper proposes and analyzes an oncolytic SARS-CoV-2 model. The model has 13 equilibrium points, and we focused our analysis on the points with the most important biological significance as follows:
(1) The trivial equilibrium E0 which is GS if R0≤1 and R1≤1. At this point, all populations disappear except for the nutrient.
(2) The uninfected-epithelial equilibrium E1 is GS if R0>1, R0≤1+λ1a3ωpϵ1, and a1λ2a2λ1≤1. Here, the patient is free from both SARS-CoV-2 infection and cancer.
(3) The infected-epithelial equilibrium E3 is GS if
and
The patient here has only SARS-CoV-2 infection.
(4) The infected epithelial-cancer equilibrium without immunity E6 is GS when
and
Here, the cancer patient has SARS-CoV-2 infection with inactive immunity.
(5) The uninfected epithelial-cancer equilibrium with CTLs E7 is GS when
and
Here, the cancer patient with active CTL immunity does not have SARS-CoV-2 infection.
(6) The infected-epithelial equilibrium with antibodies E8 is GS if
and
The patient is cancer-free and suffers only from SARS-CoV-2 infection with active immunity against the virus.
(7) The infected epithelial-cancer equilibrium with CTLs E11 is GS when
and
In this case, the cancer patient has SARS-CoV-2 with active CTLs against the cancer cells.
(8) The infected epithelial-cancer equilibrium with CTLs and antibodies E12 is GS if
and
The cancer patient has SARS-CoV-2 infection with active immune responses against the cancer cells and virus particles.
We found complete agreement between the theoretical contributions and numerical simulations. The global stability conditions of equilibrium points determine various infection scenarios, such as patients having only SARS-CoV-2, cancer, both SARS-CoV-2 and cancer, or no infections. These conditions are dependent on the parameters of model (2.1), emphasizing the importance of carefully selecting their values. Furthermore, our findings indicate that the infection rate of cancer cells by SARS-CoV-2 (ϵ2) and the killing rate of these cells by CTLs (ξ1) contribute to the reduction in the concentration of cancer cells. Based on these results, SARS-CoV-2 has the potential to lead to cancer remission or improve health conditions by either infecting cancer cells or inducing an anti-cancer immune response. This outcome aligns with recent studies suggesting an oncolytic role of SARS-CoV-2 [15,16,17,18,19]. In comparison to existing works, our model is the first to propose and analyze the oncolytic effect of SARS-CoV-2 in cancer patients. As such, these results warrant further investigation and comparison with the outcomes of experimental studies. Then, the model can be utilized in studies aiming to employ SARS-CoV-2 as oncolytic virotherapy to target cancer cells. However, a main limitation of this work is the absence of real data to estimate the values of the parameters in model (2.1), given the limited availability of such data in this direction. We utilized values from the literature and made assumptions for some parameters. Consequently, model (1) can be developed by:
(i) Estimating parameter values through fitting with real data once sufficient information becomes available;
(ii) Testing the model results against real data;
(iii) Studying the effect of immune responses on the oncolytic role of SARS-CoV-2 and when they can be supportive;
(iv) Including the direct induction of CTLs by SARS-CoV-2;
(v) Adding more components to the model, such as infected cancer cells and infected epithelial cells, for a deeper understanding of the model's dynamics;
(vi) Considering time delays that occur during different biological processes;
(vii) Accounting for parameters and model uncertainties by performing sensitivity analysis and other methods once experimental or real data becomes available.
These enhancements would contribute to a better understanding of the model and facilitate improved predictions.
Use of AI tools declaration
The authors declare they have not used artificial intelligence (AI) tools in the creation of this article.
Acknowledgments
The authors acknowledge that this project was funded by the Deanship of Scientific Research (DSR), University of Business and Technology, Jeddah 21361, Saudi Arabia. The authors, therefore, gratefully acknowledge the DSR technical and financial support.
Conflict of interest
The authors declare that there are no conflicts of interest.
Appendix
Appendix A
Proof of Theorem 7. We nominate
By evaluating dΣ6dt, we get
At equilibrium, E6 fulfills the equations:
Thus, Eq (A.1) can be collected as
Thus,
if
and
Also, it is easy to observe that dΣ6dt=0 when (N,M,C,V,T,A)=(N6,M6,C6,V6,0,0). Hence, Y′6={E6} and E6 is GS when the existence conditions and the global stability conditions are met based on LP [37]. □
Appendix B
Proof of Theorem 8. We select
Then, we obtain
By applying the equilibrium conditions at E7
Equation (B.1) can be collected as
Thus,
if
Also, dΣ7dt=0 when (N,M,C,V,T,A)=(N7,M7,C7,0,T7,0). Hence, Y′7={E7} and by LP [37], E7 is GS if
and
□
Appendix C
Proof of Theorem 9. We nominate
Then, we have
At equilibrium, E8 fulfills the following:
Thus, Eq (C.1) can be collected as
Hence,
if
when (N,M,C,V,T,A)=(N8,M8,0,V8,0,A8). Therefore, Y′8={E8} and based on LP [37], E8 is GS when it exists and
Appendix D
Proof of Theorem 10. We select
Then, we have
At equilibrium, E11 fulfills the system
Thus, Eq (D.1) can be collected as
Thus,
if
Also,
when (N,M,C,V,T,A)=(N11,M11,C11,V11,T11,0). Therefore, Y′11={E11} and by LP [37], E11 is GS when the existence and stability conditions are met. □









 DownLoad:
DownLoad:













