The multiplicative degree-Kirchhoff index and complexity of a class of linear networks

1.   Introduction

Graph categories considered in this study are simple, finite, and linked. Allow the graph $G$ to be made up of $V_G$ and edge set $E_G$, i.e., $G = (V_G, E_G)$. For more graph notations, readers should refer to ^[1].

If and only if two neighbouring verticies $i$ and $j$ of $G$, the adjacency matrix $A(G) = (a_{ij})$ is a (0, 1)-matrix, $a_{ij} = 1$. The diagonal degree matrix of $G$ is

where $d_{i}$ represents the degree of vertex $i$ in $G$. The difference between the degree matrix $D_{G}$ and adjacency matrix $A_{G}$ of $G$ gives rise to the Laplace matrix, denoted as $L_{G}$. The normalized Laplacian ^[2] is defined as

The $(m, n)$th-entry of $\mathcal{L}(G)$, which is designated

The distance between both $v_{i}$ and $v_{j}$, known as $d_{ij} = d_{G}(v_i, v_j)$, represents the length of the smallest path in question. Wiener and Dobrynin ^[3,4] introduced the Wiener index for the first time in 1947. In addition, the Wiener index is denoted as

For further information on the Wiener index, please refer to ^[5,6,7,8,9].

The Gutman index of a simple graph $G$ is introduced ^[10] and denoted as

taking into account the degree $d_{i}$ of vertex $v_{i}$.

The Kirchhoff index^[11,12] characterizes graph $G$ by summing the resistance distances between every pair of vertices, similar to the Wiener index, namely

The multiplicative degree-Kirchhoff index, initially introduced by Chen and Zhang^[13] in 2007. It is an extension of the traditional Kirchhoff index, which is expressed as

The techniques of the Kirchhoff index and multiplication degree-Kirchhoff index can be found in ^{[14,15,16,17,18]}. The multiplication degree-Kirchhoff index has garnered significant attention due to its remarkable contributions in academia and practical applications in computer network science, epidemiology, social economics, and other fields. Further research results on the Kirchhoff index and multiplication degree-Kirchhoff index can be explored through ^{[19,20,21,22,23]}.

The spanning tree of a graph $G$, also known as complexity, denoted as $\tau(G)$, refers to the number of subgraphs that encompass all vertices in $G$. This measure serves as a crucial indicator for network stability and plays a significant role in assessing the structural characteristics of graphs. For further insights into related topics such as the count of spanning trees, interested readers are encouraged to consult ^[24,25,26].

With the rapid advancement of scientific research and the successful application of topology in practical scenarios, topological theory has gained increasing recognition worldwide. The calculation problem concerning the phenylene Wiener index has been effectively resolved by Pavlović and Gutman ^[27]. Chen and Zhang ^[28] have developed a precise equation for predicting the Wiener index of random phenylene chains. Additionally, Liu et al. ^[29] have identified both the degree-Kirchhoff index and the number of spanning trees for $L_{n}$ dicyclobutadieno derivatives of [$n$] phenylenes.

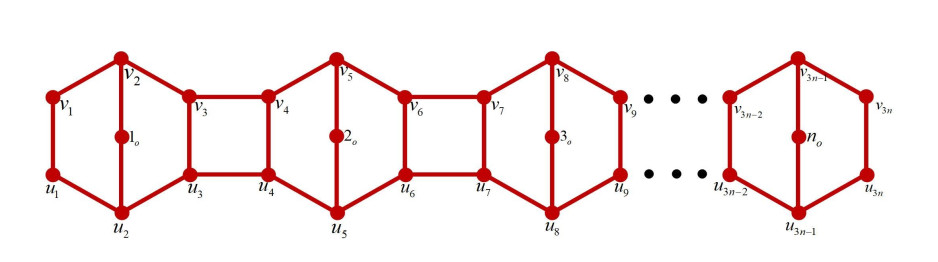
Given two automorphic graphs $S$ and $K$, we define the symbol ${S}\boxtimes{K}$ to represent the strong product of these two graphs with ${V(S)}\times{V(K)}$, which is commonly referred to as the strong product in graph theory literature. Readers can refer to ^[30] for more comprehensive definitions and concepts. Recently, Pan et al.^[25] utilized the resistance distance of a strong prism formed by $P_{n}$ and $C_{n}$ to determine the Kirchhoff index. Similarly, Li et al.^[31] derived graph invariants and spanning trees from the strong prism of a star $S_{n}$. Motivated by ^{[30,31,32,33]}, we obtain the pentagonal network $R_n$ and its strong product $P_n^2$. The pentagonal network consists of numerous adjacent pentagons and quadrilaterals with each quadrangle having a maximum of two non-adjacent pentagons, as shown in Figure 1. The $P^{2}_{n}$ is the strong product of $R_{n}$, as depicted in Figure 2. It obviously that

In this paper, we focus on the strong product of pentagonal networks, specifically examining the graph $P^{2}_{n}$ with $n\geq1$. The subsequent sections are organized as follows: Section 2 provides a comprehensive review of relevant research materials, presenting illustrations, concepts and lemmas. In Section 3, we derive the normalized Laplacian spectrum and present an explicit closed formula for the multiplicative degree-Kirchhoff index. Additionally, we calculate the complexity of $P^{2}_{n}$. In Section 4, we conclude the paper.

2.   Preliminary results

In this section, let $R_{n}$ represent the penagonal-quadrilateral networks, as illustrated in Figure 1. $P^{2}_{n}$ is composed of $R_{n}$ and its copy $R_{n}^{\prime}$, positioned one in front and one behind, as shown in Figure 2. Moreover,

represents the characteristic polynomial of matrix $A$.

The fact that

is an automorphism deserves attention. Let

Subsequently, the normalized Laplacian matrix can be represented as a block matrix, that is

in which

Let

then,

where

Observe that $W'$ and $W$ are transpose matrices of each other.

The characteristic polynomial of the matrix $R$, is denoted as

The decomposition theorem process for $P_{n}^{2}$ is obtained in a similar manner to Pan and Li^[34], thus we omit this proof and present it as follows:

Lemma 2.1. ^[35] Assuming that the determination of $\mathcal{L}_{A}$ and $\mathcal{L}_{S}$ has been previously described, then

Lemma 2.2. ^[13] The graph $G$ is an undirected connected graph with $n$ vertices and $m$ edges. Then

Lemma 2.3. ^[2] The number of spanning trees in $G$, referred to as the graph's complexity, can be considered a fundamental measure in graph theory. Then

3.   Main results

In this section, we explore the methodology for deriving an explicit analytical expression of the multiplicative Kirchhoff index by traversing the normalized Laplacian matrix. Meanwhile, we determine the computational complexity of $P^{2}_{n}$. Subsequently, employing the normalized Laplacian, we derive matrices of order $6n$ as

and

Due to

and

it can be convincingly argued that

and

Utilizing Lemma 2.1, it is revealed that the $P^{2}_{n}$ normalized Laplacian spectrum consists of the eigenvalues from $\mathcal{L}_{A}$ and $\mathcal{L}_{S}$. It is established that the $\mathcal{L}_{S}$ possesses eigenvalues $\frac{6}{5}$ and $\frac{8}{7}$ with multiplicities of $4$ and $(6n-4)$, respectively.

Let

and

where the matrices $M$ and $N$ are both of order $3n$.

The matrices $M$ and $N$ are combined to form a block matrix, denoted as $\frac{1}{2}\mathcal{L}_{A}$, in the following manner:

Suppose that

is a block matrix. Hence, we can obtain

Let $J = M+N$ and $K = M-N$. Then,

and

in which the diagonal elements are

Based on Lemma $2.1$, it is evident and demonstrable that the eigenvalues of $\frac{1}{2}\mathcal{L}_{A}$ are identical to those of $J$ and $K$. Assume that the eigenvalues of $J$ and $K$ are $\sigma_{i}$ and $\varsigma_{j} \; (i, j = 1, 2, \cdots, 3n)$, respectively, with

We verify $\sigma_{1} \geq0$ and $\varsigma_{1}\geq0$. In addition, it is easy to know that the normalized Laplacian spectrum of $P^{2}_{n}$ is $\{ 2\sigma_{1}, 2\sigma_{2}, \cdots, 2\sigma_{3n}, 2\varsigma_{1}, 2\varsigma_{2}, \cdots, 2\varsigma_{3n} \}$. Note that

we can obtain Lemma 3.1 according to Lemma 2.2.

Lemma 3.1. Assume that $P^{2}_{n}$ is the strong product of the pentagonal network. Then,

Subsequently, we partition the computation of the aforementioned equation into two distinct components and prioritize the initial calculation of $\sum_{i = 2}^{3n}\frac{1}{\sigma_{i}}$.

Lemma 3.2. Suppose that $\sigma_{i}(i = 1, 2, \cdots, 3n)$ is defined as described previously.

Proof. Suppose that

Then $\sigma_{2}, \sigma_{3}, \cdots, \sigma_{3n}$ fulfil the following equation

and we observe that $\frac{1}{\sigma_{2}}, \frac{1}{\sigma_{3}}, \cdots, \frac{1}{\sigma_{3n}}$ are the roots of the following equation

By Vieta's Theorem, one has

For each value of $i$ from $1$ to $3n + 1$, we consider $J_{i}$ and assign $j_{i}$ as the determinant of $J_{i}$. We will derive the formula for $j_{i}$, which can be utilized to calculate $(-1)^{3n-2}a_{3n-2}$ and $(-1)^{3n-1}a_{3n-1}$. Then one has

and

Through a straightforward computation, one can derive the following general formulas:

According to Eq (3.1), we divide the numerator and denominator into two facts and reveal them later. For the sake of convenience, we represent the diagonal elements of $J$ as $l_{ii}$ in a simplified manner. □

Fact 3.3.

Proof. Given that $J$ is a left-right symmetric matrix, the sum of all principal minors can be represented by the number $(-1)^{3n-1}a_{3n-1}$, where these minors correspond to rows and columns with indices equal to $(3n-1)$.

where

By Eqs (3.2) and (3.3), we have

This is the completion of the proof. □

Fact 3.4.

Proof. We note that the sum of all principal minors of order $3n-2$ in $J$ can be expressed as $(-1)^{3n-2}a_{3n-2}$. After that,

where

and

Note that

According to Eq (3.4), the determinant of $Z$ varies depending on the values of $i$ and $j$, as well as $s$ and $t$. Consequently, we can categorize the primary scenarios into six distinct classifications.

Case 1. $i = 3s$, $j = 3t$ for $1\leq{s} < t\leq{n}$, and

Case 2. $i = 3s$, $j = 3t+1$ for $1\leq{s}\leq{t}\leq{n}$, and

or $i = 3s+2$, $j = 3t$ for $0\leq{s} < t\leq{n}$, and

Case 3. In the same way, $i = 3s$, $j = 3t+2$ for $1\leq{s}\leq{t}\leq{n}$, and

or $i = 3s+1$, $j = 3t$ for $0\leq{s} < t\leq{n}$, and

Case 4. Similarly, $i = 3s+1$, $j = 3t+1$ for $0\leq{s} < t\leq{n}$, and

or $i = 3s+2$, $j = 3t+2$ for $0\leq{k} < l\leq{n}$, and

Case 5. $i = 3s+1$, $j = 3t+2$ for $0\leq{s}\leq{t}\leq{n}$, and

Case 6. $i = 3s+2$, $j = 3t+1$ for $0\leq{k} < l\leq{n}$, and

Therefore, we can obtain

where

and

By substituting $\wp_{1}$, $\wp_{2}$, and $\wp_{3}$ into Eq (3.5), the desired outcome can be deduced.

This is the completion of the proof. □ Let

represent the eigenvalues of $J$. By Facts $3.3$ and $3.4$, we can further investigate Lemma 3.2. According to Eq (3.1), it is evident that

Considering Lemma 3.1, we will focus on the calculations of $\sum\limits_{j = 1}^{3n}\frac{1}{\varsigma_{j}}$. Let

and

Lemma 3.5. The variable $\varsigma_{j}$ (where $j$ ranges from $1$ to $3n+2$) is assumed to be defined as previously described. One has

where

and

Proof. The representation of $\Phi(K)$ can be expressed as

where $\varsigma_{1}, \varsigma_{2}, \cdots, \varsigma_{3n}$ represent the roots of the equation.

and the equation is determined to possess $\frac{1}{\varsigma_{1}}, \frac{1}{\varsigma_{2}}, \cdots, \frac{1}{\varsigma_{3n}}$ as its solutions

By Vieta's Theorem, one holds that

To simplify the analysis, let $R_{p}$ denote the $p$-th order principal minors of matrix $K$ and $k_{p}$ represent the determinant of $R_{p}$. We will derive an equation for $k_{p}$ that can be utilized to calculate $(-1)^{3n-1}b_{3n-1}$ and $det \; K$ for values of $p$ ranging from $1$ to $3n-1$. Subsequently, we arrive at

and

After a straightforward computation, the following general formulas can be derived

□ Subsequently, we proceed by examining the following Facts:

Fact 3.6.

Proof. By expanding $det\; K$ with respect to its last row, we obtain

The desired outcome has been achieved. □

Fact 3.7.

where

and

Proof. Considering that $K$ has a $(3n-1)$-row and $(3n-1)$-column structure, the sum of all principal minors can be expressed as $(-1)^{3n-1}b_{3n-1}$. Here, $l_{ii}$ represents the diagonal entries of $K$. It is noteworthy that $K$ exhibits bilateral symmetry, which enables us to derive specific information.

where

In line with Eq (3.7), we have

Immediately, we can get

and

By incorporating Eqs (3.9)–(3.12) into Eq (3.8), we can achieve Lemma 3.7. Utilizing Eq (3.6), in conjunction with Facts 3.6 and 3.7, Lemma 3.5 can be promptly derived. □

By integrating Lemmas 3.1, 3.2 and 3.5, we can readily deduce the Theorems 3.8 and 3.9.

Theorem 3.8. Assume that $P^{2}_{n}$ is the strong product of the pentagonal network. One has

where

additionally,

and

Theorem 3.9. Let $P^{2}_{n}$ be the strong product of pentagonal network. Then

Proof. Based on the proof of Lemma $2.2$, it is evident that $\sigma_{1}, \sigma_{2}, \cdots \sigma_{2n+1}$ constitute the roots of the equation

Accordingly, one has

By Fact 3.3, we have

By the same method,

Note that

and

In conjunction with Lemma $2.3$, we get

This is the completion of the proof. □

4.   Conclusions

In this study, we have derived explicit expressions for the multiplicative degree-Kirchhoff index and complexity of $P^{2}_{n}$ based on the spectrum of the Laplacian matrix, where $P^{2}_{n} = R_{n} \boxtimes R_{n}^{\prime}$. These two fundamental calculations serve as simple yet reliable graph invariants that effectively capture the stability of diverse networks. Future research should focus on applying our methodology to determine spectra for strong products of automorphic and symmetric networks.

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgments

The authors would like to express their sincere gratitude to the editor and anonymous referees for providing valuable suggestions, which significantly contributed to the substantial improvement of the original manuscript. This work was supported by Anhui Jianzhu University Funding under Grant KJ212005, and by Natural Science Fund of Education Department of Anhui Province under Grant KJ2020A0478.

Conflict of interest

No potential confilcts of interest were reported by the authors.