Limit cycles in an $m$-piecewise discontinuous polynomial differential system

1.   Introduction

One of the main topics in the qualitative theory of differential equations is to determine the cyclicity of a given differential system. The cyclicity refers to the maximum number of limit cycles that the system possesses. The well-known second part of Hilbert's 16th problem (proposed by David Hilbert in 1900) is concerned with the cyclicity of planar polynomial systems of a specific degree. Over the past few decades, several results ^[1,2,3] have been obtained regarding the cyclicity of planar polynomial systems of degrees 2 and 3.

Recently, attention has been focused on discontinuous differential systems (see ^[4]). The problem of cyclicity in discontinuous differential systems has been re-examined. In 2001, Coll, Gasull, and Prohens ^[5] conducted a comprehensive investigation on switching systems, including FF-type, FP-type, and PP-type, for degenerate Hopf bifurcations near a weak focus. In the FF-type case, they computed five Lyapunov quantities for a switching quadratic system and proved that at least four limit cycles can bifurcate from the weak focus $O:(0, 0)$. Subsequently, Gasull and Torregrosa ^[6] discovered five limit cycles for a switching quadratic system. For the PP-type case, Novaes and Silva ^[7] provided a general recursive formula for the Lyapunov coefficients for monodromic tangential singularities in Filippov vector fields, which encompasses the PP-type singularities studied in ^[5]. In 2010, Han and Zhang ^[8] proved that a planar switching linear system may have two limit cycles and they conjectured that such systems could have at most two limit cycles. However, in 2012, Huan and Yang ^[9] refuted this conjecture by presenting an example where three limit cycles could be numerically observed. In the same year, Llibre and Ponce ^[10] analytically proved the existence of these numerically observed limit cycles. Since then, many other works have provided examples with three limit cycles (see, for instance, ^[11,12,13]). In 2013, Llibre, Ord'o nez, and Ponce ^[14] extended some techniques used to demonstrate the existence and uniqueness of limit cycles, originally stated for smooth vector fields, to continuous piecewise-linear differential systems. They obtained new results for systems with three linearity zones without symmetry and with one equilibrium point in the central region. In 2015, Chen, Romanovski, and Zhang ^[15] introduced the fractional order for weak foci in FF-type switching systems and proved that the cyclicity of these FF-type switching systems is at least five for weak foci and eight for centers, respectively.

The averaging theory, as proposed in the classical work in ^[16], is a well-established tool for studying the existence of periodic solutions in nonlinear smooth dynamical systems. Building upon this theory, Llibre, Novaes, and Teixeira ^[17] extended its applicability to non-smooth systems with two zones in 2015. In 2017, Llibre, Novaes, and Camila ^[18] further expanded the averaging theory to encompass discontinuous differential systems with multiple zones. In their work, they considered discontinuous differential systems in $\mathbb{R}^2$ that were defined in two half-planes separated by a straight line. By employing the averaging theory, Chen, Llibre, and Zhang ^[19] established that the cyclicity of a Hopf bifurcation in such systems is at least 5.

Efforts have also been made to determine the number of limit cycles bifurcated from the periodic annulus of a linear center under a switching polynomial Liénard perturbation

where $f$ is a polynomial of degree $n\in\mathbb{N}$ and $\kappa_1, \kappa_2\in\mathbb{ R}$, sgn$(y)$ is the sign of $y$. In ^[20], Martins and Mereu studied the number of limit cycles of system (1.1), and obtained that for any $n\ge1$ the cyclicity of the differential system (1.1), is $\left[\frac{n}{2}\right]+1$. In 2023, Tiago M.P. De Abreu and Ricardo M. Martins^[21] considered the piecewise smooth system of differential equations $\dot{x} = y, \ \dot{y} = -x-\varepsilon\cdot(f(x)y+{\rm sgn}(y)g(x))$, where $f(x)$ and $g(x)$ be real polynomials of degrees $n\ge 1$ and $m\ge 1$, respectively. Using the averaging method, concluded that for sufficiently small values of $|\varepsilon |$, a lower bound for the maximum number of limit cycles in this system is $\left[\frac{n}{2}\right]+\left[\frac{m}{2}\right]+1$.

In recent years, the interest on this topic was extended to the $m$-piecewise discontinuous polynomial Liénard differential system

where $f(x) = a_0+a_1x+\cdots+a_nx^n$ and the zero set of the function $g_m(x, y)$ with positive even number $m$ is the union of $m/2$ different straight lines passing through the origin of coordinates dividing the plane into sectors of angle $2\pi/m$. This system is commonly encountered in many applications such as control theory (see ^[4]), economics (see ^[22]), mechanical systems (see ^[23]), and nonlinear oscillations (see ^[24]). In ^[25], Llibre and Teixeira proved that the cyclicity $L(m, n)$ of the system (1.2) satisfies

where $[z]$ denotes the integer part of $z$, i.e., the greatest integer less than or equal to $z$, and conjectured that

for any even $m\ge 6$. In ^[26], Dong and Liu proved this conjecture. In ^[27], Novaes showed that if the discontinuity set $\{(x, y):g_m(x, y) = 0\}$ consists of $m$ rays starring at the origin whose slopes can be taken freely, many other limit cycles can appear; then, $L(m, n)\ge n$. Finally, it has been proven in ^[28] that the maximum number of limit cycles of such a planar switching linear system is indeed uniformly bounded by 8.

In this paper, I study the number of limit cycles that can bifurcate from the periodic orbits of the linear center $\dot{x} = y, \dot{y} = -x$ perturbed inside the following $m$-piecewise discontinuous polynomial differential system

where

I assume that $g_m$ is a sign-switching function on the $(x, y)$-plane partitioned equally by $m/2$ lines $\ell_{k}: y = \tan(2k\pi/m) x$, $k = 0, ..., m/2-1$ such that $g_m(x, y) = 0$ on those $\ell_k$-lines and $g_m(x, y) = (-1)^k$ if $(x, y)$ lies in the angular region between $\ell_k$ and $\ell_{k+1}$. For convenience, I call system (1.3) an $m$-piecewise discontinuous generalized Liénard systems of degree $(n, l)$ if $a_{ij}\ne 0$ for some $i, j$ with $i+j = n$ and $b_l\ne 0$. Let $L_m(n, l)$ denote lower bound for the maximun number of limit cycles which bifurcating from the annulus of the origin of this system. I will prove the following.

Theorem 1.1. For the system (1.3), $L_m(n, l)$ satisfies

for any even number $m\ge2$.

Theorem 1.1 includes the result of Theorem 1.1 of ^[26] and the result of Corollary 2 of ^[20]. In fact, in the case that $f(x, y) = 0$, system (1.3) is equivalent to system (1.2) in ^[26]. From Theorem A, if $4\mid m, \left[(l-1)/2\right]\ge(m-4)/4$ or $4\nmid m, \left[l/2\right]\ge(m-2)/4$, then system (1.3) can has at least $\left[\left(l-(m-2)/2\right)/2\right]$ limit cycles, i.e., the same result of Theorem 1.1 in ^[26]. In the case that $f(x, y) = \sum_{i = 0}^{i = n}a_ix^iy, m = 2, h(x) = \kappa_1x+\kappa_2$, system (1.3) is equivalent to system (1.1) in ^[20]. By Theorem 1.1, system (1.3) can has at least $\left[n/2\right]+1$ limit cycles, i.e., the same result of Corollary 2 in ^[20].

2.   Preliminary results

In this section, I will introduce some preliminary results on the averaging theory and the zeros of function, which will be applied to studying the $m$-piecewise discontinuous polynomial differential equations (1.3). For the proof, refer the reader to ^[16,18].

Let $m > 1$ be a positive integer, $\alpha_m = 2\pi$ and $\alpha = (\alpha_0, \alpha_1, \cdots, \alpha_{m-1})$ is a $m$-tuple of angles such that $0 = \alpha_0 < \alpha_1 < \cdots < \alpha_{m-1} < \alpha_m = 2\pi$. For $j = 1, 2, \cdots, m$ let $L_j$ be the intersection between the open bounded neighborhood $U\subset \mathbb{R}^2$ of the origin with the ray starting at the origin and passing through the point $(\cos \alpha_j, \sin \alpha_j)$, and take $\Sigma = \bigcup\limits_{j = 1}^mL_j$. Note that $\Sigma$ splits the set $U\setminus\Sigma\subset\mathbb{R}^2$ in $m$ disjoint open sectors. Denote the sector delimited by $L_j$ and $L_{j+1}$, in counterclockwise sense, by $C_j$, for $j = 1, 2, \cdots, m$.

Let $D$ be an open bounded subset of $\mathbb{R}_+$ and $\mathbb{S}^1\equiv \mathbb{R}\setminus(2\pi\mathbb{Z})$, consider the following differential equation

where $F_i^j:\mathbb{S}^1\times D\rightarrow \mathbb{R}$ and $R^j:\mathbb{R}^1\times D\times(-\varepsilon_0, \varepsilon_0)\rightarrow \mathbb{R}(i = 0, 1, \cdots, k, j = 1, 2, \cdots, m)$ are both $C^{k+1}$ functions and $2\pi$-periodic in the first variable. The characteristic function $\chi_J(\theta)$ of an interval $J$ is defined as

Then system (2.1) becomes

where

Clearly, system (2.2) is a periodic system having a discontinuity set $\sum = \bigcup\limits_{j = 0}^{m-1}\{\theta = \alpha_j\}$. Denote by $\varphi(\theta, \rho)$ the solution of the system $r'(\theta) = F_0(\theta, r)$ such that $\varphi(0, \rho) = \rho$. From now on, system $r'(\theta) = F_0(\theta, r)$ will be called unperturbed system. I need the following hypothesis,

(H) For each $\rho\in D$ the solution $\varphi(\theta, \rho)$ of the unperturbed system is well defined for all $\theta\in \mathbb{S}^1$, $2\pi$-periodic, and reaches $\Sigma$ only at crossing points.

Then, let $y_i : \mathbb{R}\times D\rightarrow \mathbb{R}$ for $i = 1, 2, \cdots, k$, be defined recurrently by

where $\partial^LG(\phi, \rho)$ denotes the $L$-th order derivative of $G$ with respect to $\rho$ and $S_l$ is the set of all $l$-tuples of non-negative integers $(b_1, b_2, \cdots, b_l)$ satisfying $b_1 + 2b_2 + \cdots + lb_l = l$, and $L = b_1 + b_2 + \cdots + b_l$. Thus, as shown in ^[18], I can define $f_i: D\to \mathbb{R}$ such that

called the $i$-th order averaged function.

Lemma 2.1. (Theorem 1 of ^[18]) Assume that for some $l\in\{1, 2, \cdots, k\}$ the functions defined in (2.4) satisfy $f_s = 0$ for $s = 1, 2, \cdots, l-1$ and $f_l\not\equiv 0$. If there exists $\rho^*$ such that $f_l(\rho^*) = 0$ and $f'_l(\rho^*)\neq0$, then for $|\varepsilon|\neq0$ sufficiently small there exists a $2\pi$-periodic solution $r(\theta, \varepsilon)$ of system (2.2) such that $r(0, \varepsilon)\rightarrow \rho^*$ when $\varepsilon\rightarrow0$.

I recall the Descartes Theorem about the number of zeros of a real polynomial. For a proof, see pages 81-83 of book ^[29].

Descartes Theorem. Consider the real polynomial $p(x) = a_{i_1}x^{i_1}+a_{i_2}x^{i_2}+\cdots+a_{i_r}x^{i_r}$, where $r > 1$ and $0\leq i_1 < i_2 < \cdots < i_r$ are all integers and the coefficients $a_{i_1}, \cdots, a_{i_r}$ do not vanish simultaneously. If coefficients of $p$ have $m$ variations of sign, i.e., there are $m$ consecutive pairs $a_{i_j}$ and $a_{i_j+1}$, $j\in \{1, ..., r\}$, such that $a_{i_j}a_{i_j+1} < 0$, then $p(x)$ has at most $m$ positive real roots. Moreover, it is always possible to choose the coefficients of $p(x)$ in such a way that $p(x)$ has exactly $r-1$ positive real roots.

In order to obtain the simple zeros of a real polynomial, I need

Lemma 2.2. (Lemma 2.1 of ^[30]) Consider $p+1$ linearly independent analytical functions $f_i:U\rightarrow \mathbb{R}$, $i = 0, 1, \cdots, p$, where $U\subset\mathbb{R}$ is an interval.

(1) Given p arbitrary values $x_i\in U, i = 1, 2, \cdots, p,$ there exist $p+1$ constants $C_i, i = 0, 2, \cdots, p$ such that

is not the zero function and $f(x_i) = 0$ for $i = 1, 2, \cdots, p.$

(2) Furthermore, there exist $f(x)$ in (2.5) such tant it has at least $p$ simple zeroes in $U$.

3.   Proof of Theorem 1.1

3.1.   The first order averaged function

Using the polar coordinates transformation $x = r\cos\theta, y = r\sin\theta$ and taking $\theta$ as the new variable, I change system (1.3) into the following equivalent equation

where

and

Clearly, Eq (3.1) is of the form (2.2) with $k = 1, F_0(\theta, r) = 0, \varphi(\theta, r) = r$ and $F_1(\theta, r) = F(\theta, r)$. One can check that (3.1) satisfies hypothesis (H). Thus, as in (2.4), I can compute the first order averaged function of the system (3.1)

where

First, I compute $I_1$.

Obviously, $\int_0^{2\pi}\cos^{2s+1}\theta\sin^{j+1}\theta{\rm d}\theta = 0$, $\int_0^{2\pi}\cos^{i}\theta\sin^{2t+1}\theta{\rm d}\theta = 0$, so, I have

Proposition 3.1.

where $c_{2i, 2(k-i)+1} = \int_{0}^{2\pi}\cos^{2i}\theta\sin^{2(k-i)+2}\theta{\rm d}\theta = \frac{(2k-2i+2)!!(2i)!!}{(2k+2)!!}\cdot 2\pi > 0$ $(i = 0, 1, \cdots, k)$ is a positive constant and $A_k = \sum_{i = 0}^{k}a_{2i, 2(k-i)+1}c_{2i, 2(k-i)+1}$ can be chosen arbitrarily.

Second, I compute $I_2$. In order to simplify the notation, I define the following function:

Let $v = {\rm e}^{\frac{\pi}{k}i}, i = \sqrt{-1}$, by Lemma 2.1 of ^[26], I have

Lemma 3.1. If $j = (2p+1)k$, $p$ is an integer, then

and if $j$ is other integer, then

Using Lemma 3.1, I have

Lemma 3.2. For $t\in\mathbb{N}$, the following results hold:

(i) if $m = 4s, s = 1, 2, \cdots$, then $d_{4s, 2t} = 0$ for all $t$, $d_{4s, 2t+1} = 0$ for $0\le t < s-1$ and $d_{4s, 2t+1} > 0$ for $t\ge s-1$;

(ii) if $m = 4s+2, s = 0, 1, 2, \cdots$, then $d_{4s+2, 2t+1} = 0$ for all $t$, $d_{4s+2, 2t} = 0$ for $0\le t < s$ and $d_{4s+2, 2t} > 0$ for $t\ge s$.

Proof. (ⅰ) If $m = 4s, s = 1, 2, \cdots$, then

where

Let $\theta = \varphi+\pi$, have

and in the same manner, have

moreover,

Hence, if $j = 2t, t\in\mathbb{N}$, then $d_{4s, 2t} = 0.$ Let $j = 2t+1, t\in\mathbb{N}$, and let

So,

and

Hence

Thus, by Lemma 3.1, I obtain

If $0\le t < s-1$, then there does not exist $p\in\mathbb{Z}$ such that $0\le (2p+1)s\le t+1$. Thus,

and this implies $d_{4s, 2t+1} = 0$.

If $t\ge s-1$, then

(ⅱ) If $m = 4s+2, s = 0, 1, 2, \cdots$, then

where

Let $\theta = \varphi+\pi$, have

so, $d_{4s+2, j} = \left(1+(-1)^j\right)K_1$. Hence, if $j = 2t+1, t\in\mathbb{N}$, then $d_{4s+2, 2t+1} = 0$. Let $j = 2t, t\in\mathbb{N}$. Now, take

Hence

and

So, by Lemma 3.1, the following equalities hold:

If $0\le t < s$, then there does not exist $p\in\mathbb{Z}$ so that $0\le p\le\frac{t-s}{2s+1}$, thus,

and this implies $d_{4s+2, 2t} = 0$.

If $t\ge s$, then

Hence, the Lemma 3.1 is proved. □

Hence, according to Lemma 3.2, have

Proposition 3.2.

where $d_{m, 2k+1} > 0(4\mid m, k = (m-4)/4, \cdots, [(l-1)/2]), d_{m, 2k} > 0(4\nmid m, k = (m-2)/4, \cdots, [l/2])$.

From Propositions 3.1 and Propositions 3.2, have

Proposition 3.3. The first order averaged function of the system (3.1) is

3.2.   Proof of Theorem 1.1

Now, I am going to prove Theorem 1.1 and divide it into two cases:

3.2.1.   The even number $m$ is multiple of $4$

Let $m = 4s, s = 1, 2, \cdots$. From Proposition 3.1, the first order averaged function of the system (3.1) is

If $\left[\frac{n-1}{2}\right] < \frac{m-4}{4}$ and $\left[\frac{l-1}{2}\right]\le\frac{m-8}{4}$, the term $\sum_{k = \frac{m-4}{4}}^{\left[\frac{l-1}{2}\right]}b_{2k+1}d_{4s, 2k+1}r^{2k+1}$ does not appear, the monomials which appear in the polynomial $f_1(r)$ in (3.3) are the following $N+1$ monomials

where $N = \left[\frac{n-1}{2}\right]$.

If $\left[\frac{n-1}{2}\right] < \frac{m-4}{4}$ and $\left[\frac{l-1}{2}\right]\geq \frac{m-4}{4}$, the monomials which appear in the polynomial $f_1(r)$ in (3.3) are the following $N+1$ monomials

where $N = \left[\frac{n-1}{2}\right]+\left[\frac{l-1}{2}\right]-\frac{m-8}{4}$.

If $\left[\frac{n-1}{2}\right]\geq \frac{m-4}{4}$, the monomials which appear in the polynomial $f_1(r)$ in (3.3) are the following $N+1$ monomials

where $N = \max\left\{\left[\frac{n-1}{2}\right], \left[\frac{l-1}{2}\right]\right\}$.

By Descartes Theorem and Lemma 2.2, the polynomial $f_1(r)$ in (3.3) has exactly $N$ simple positive real roots. Hence, by Lemma 2.1, if $m = 4s, s = 1, 2, \cdots$, then for $\varepsilon > 0$ sufficiently small the system (3.1) has $N$ limit cycles, i.e.,

3.2.2.   The even number $m$ is not multiple of $4$

Let $m = 4s+2, s = 0, 1, 2, \cdots$. From Proposition 3.1, the first order averaged function of the system (3.1) is

If $\left[\frac{l}{2}\right]\le\frac{m-2}{4}-1$, then $d_{4s+2, 2k} = 0, k = 0, 1, 2, \cdots, \left[\frac{l}{2}\right]$. So, the term $\sum_{k = t}^{\left[\frac{l}{2}\right]}b_{2k}d_{4s+2, 2k}r^{2k}$ does not appear, the monomials which appear in the polynomial $f_1(r)$ in (3.5) are the following $N+1$ monomials

where $N = \left[\frac{n-1}{2}\right]$.

If $\left[\frac{l}{2}\right]\geq \frac{m-2}{4}$, the monomials which appear in the polynomial $f_1(r)$ in (3.5) are the following $N+1$ monomials

where $N = \left[\frac{n-1}{2}\right]+\left[\frac{l}{2}\right]-\frac{m-6}{4}$.

By Descartes Theorem and Lemma 2.2, the polynomial $f_1(r)$ in (3.5) has exactly $N$ simple positive real roots. Hence, by Lemma 2.1, if $m = 4s+2, s = 0, 1, 2, \cdots$, then for $\varepsilon > 0$ sufficiently small the system (3.1) has $N$ limit cycles, i.e.,

As discussed in the above two parts, for any even number $m\ge2$, the cyclicity $L_m(n, l)$ of system (3.1) satisfies

The proof of Theorem 1.1 is completed.

Remark 3.1. If $f(x, y) = 0$, then (3.2) can be simplified as

Remark 3.2. (ⅰ) If $f(x, y) = 0$ and $m = 4s, s = 1, 2, \cdots$, from Remark 3.1 and Theorem 1.1, for $\varepsilon$ sufficiently small the system (3.1) has at least $L(m, l)$ limit cycles, where as defined in ^[25],

So, those are the results conjectured in ^[25].

(ⅱ) If $f(x, y) = 0$ and $m = 4s+2, s = 0, 1, 2, \cdots$, from Remark 3.1 and Theorem A, for $\varepsilon$ sufficiently small the system (3.1) has at least $L(m, l)$ limit cycles, where as defined in ^[25],

So, those are the results conjectured in ^[25].

(ⅲ) If $f(x, y) = 0$, from (i) (ii), for $\varepsilon$ sufficiently small the system (3.1) has at least $\left[\frac{1}{2}\left(l-\frac{m-2}{2}\right)\right]$ limit cycles for any positive even number $m$. So, those are the results of Theorem 1.1 in ^[26].

Remark 3.3. If $f(x, y) = \sum_{i = 0}^{n}a_ix^iy, m = 2, h(x) = \kappa_1x+\kappa_2$, from (3.6), for $\varepsilon$ sufficiently small the system (3.1) has $\left[\frac{n}{2}\right]+1$ limit cycles. So, it's the result of Corollary 2 in ^[20].

4.   Examples

Example 4.1. Consider the following system

where, $\varepsilon = 0.02, m = 4, n = 3, l = 1$. The first order averaged function $f_1(r)$ of the system (4.1) is

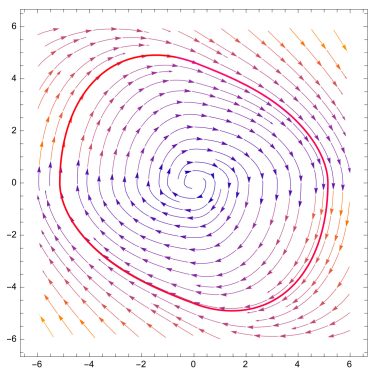
So, $f_1(r)$ has unique positive real root $r_1 = \sqrt{22}$ and $f_1'(r_1) = 44\pi > 0$. The system (4.1) has a stable limit cycle, as shown in Figure 1. Then $L_4(3, 1)\ge\max\left\{\left[\frac{n-1}{2}\right], \left[\frac{l-1}{2}\right]\right\} = 1$.

Example 4.2. Consider the following system

where, $\varepsilon = 0.17, m = 2, n = 3, l = 1$. The first order averaged function $f_1(r)$ of the system (4.2) is

So, $f_2(r)$ has two positive real roots $r_1 = \sqrt{3}-1, r_2 = 2$ and $f_1'(r_1) = 6(1-\sqrt{3})\pi < 0, f_1'(r_2) = 6 > 0$. The system (4.2) has two limit cycles, as shown in Figure 2.

Then $L_2(3, 1)\ge\left[\frac{n-1}{2}\right]+\left[\frac{l}{2}\right]-\frac{m-6}{4} = 2$.

5.   Conclusions

In this paper, I discuss the lower bound of the maximum number of limit cycles for a class of $m$-piecewise discontinuous polynomial systems and obtain the lower bound of the maximum number of limit cycles for this class of differential systems. This result generalizes the results of the existing literature.

Use of AI tools declaration

The author declares that he has not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgments

The author expresses sincere gratitude to Professor Weinian Zhang of Sichuan University for providing invaluable guidance throughout the research process. Additionally, this work was supported by the Natural Science Foundation of China (No. 11861001).

Conflict of interest

The author declares no conflicts of interest in this paper.