Stability analysis of Caputo fractional time-dependent systems with delay using vector lyapunov functions

1.   Introduction

Fractional calculus extends traditional differentiation and integration concepts to non-integer orders, and it has gained considerable academic interest in recent decades due to its efficacy in modeling various real-world systems. Fractional derivatives are instrumental in describing mechanical and electrical properties of materials, as well as the behaviors of gases, liquids, and minerals across diverse fields. For foundational understanding, refer to the monographs ^[1,2,3,4] and their cited references.

Fractional time-dependent systems with delays have gained prominence for their enhanced accuracy in capturing memory and hereditary behaviors. Studies have explored the existence and uniqueness of solutions for fractional differential systems, both with and without delays, in works such as ^[5,6,7,8]. For instance, Deng et al. ^[9] derived stability criteria for fractional differential systems with multiple time delays by employing the Laplace transform to convert fractional differential equations (FDEs) into algebraic equations in the Laplace domain, analyzing stability based on the poles of the resulting transfer function.

Cermak et al. ^[10] investigated the stability of solutions to FDEs with constant delays using Lyapunov functional methods and fractional calculus tools. They derived stability conditions based on the nonlinear function $f$, the delay $\tau$, and the fractional order $\alpha$. Their results indicate that if specific conditions on $f$ and $\tau$ are met, the zero solution of the FDE is asymptotically stable. They also explored the asymptotic behavior of solutions, providing estimates for the rate of decay over time, which depends on the fractional order $\alpha$. Specifically, for $0 < \alpha < 1$, the solution decays at a rate proportional to $t^{-\alpha}$ as $t \to \infty$.

Tuan ^[11] focused on the stability analysis of nonlinear delay fractional differential equations (DFDEs) by developing a linearized stability theorem that extends classical results to FDEs with delays. This theorem offers conditions under which equilibrium solutions of such systems are asymptotically stable, providing a robust framework for analyzing equilibrium solutions in systems with fractional dynamics and time delays. Similarly, Li and Wang ^[12] addressed the stability analysis of fractional delay differential equations (FDDEs) by exploring delayed Mittag-Leffler type matrix functions to determine conditions for convergence to an equilibrium point within a finite time. Thanh ^[13] proposed new criteria for finite-time stability of systems with singular FDEs and time-varying delays. Using a Lyapunov-Krasovskii functional designed to handle fractional orders and time-varying delays, stability conditions are expressed in terms of linear matrix inequalities (LMIs), which offer a convenient computational framework.

Following the discussion so far on stability, its critical importance in the dynamics of systems, especially those with feedback control, deserves further attention. For linear fractional systems, various reliable methods have been established to maintain stability (see ^{[14,15,16,17]}). Meanwhile, Lyapunov stability theory provides a strong foundation for analyzing nonlinear systems. In particular, Lyapunov's second method, or direct method, is highly effective because it allows for stability assessment without requiring the explicit solution of the system's differential equations, making it a versatile tool for stability analysis (see ^[19,20,21]).

In ^[22], Argawal et al. identified three types of fractional derivatives of Lyapunov functions used in stability analysis of time-dependent systems with delay: the Dini fractional derivative, Caputo fractional Dini derivative, and Dini fractional derivative. The Caputo fractional derivative is commonly used and is defined as:

However, this derivative has limitations, as it requires the use of the Razumikhin criterion over the entire delay interval and differentiable Lyapunov functions. For studying stability characteristics, primarily quadratic Lyapunov functions are used (see ^[23]). The Dini fractional derivative does not have this drawback, maintaining the concept of fractional derivatives due to its memory property, and is defined as:

and the Caputo fractional Dini derivative is defined as:

The Caputo fractional Dini derivative has been used to analyze various stability types of Caputo fractional time-dependent systems with and without delay (see ^{[22,24,25,26,27,28]}). Scalar Lyapunov functions may not fully capture interactions among dimensions. Vector Lyapunov functions, on the other hand, offer greater flexibility and precision in constructing stability criteria for complex systems, providing a more detailed analysis of subsystems and their interactions. They are particularly useful for examining nonlinear systems where interactions can be intricate and nonlinear relationships are prevalent (see ^{[29,30,31,32]}).

Let $\mathbb{R_+} = [0, \infty)$ and assume that $t_0\geq 0\in \mathbb{R_+}$. Let $J_0 = [-\gamma, 0]$, $J = [-\gamma, \infty), \gamma > 0$ and $I = [t_0, T]$ be intervals in $\mathbb{R}$. Let $\mathfrak{D}^N = C(J_0, \mathbb{R}^N)$ be the space of all continuous maps on $J_0$, where $\mathbb{R}^N$ is the N-dimensional Euclidean vector space endowed with norm $\|.\|$. For any $\phi \in \mathfrak{D}^N$, we define the norm of $\phi$ by

In this paper, we consider the retarded Caputo fractional time-dependent system of the form

where $^CD^\alpha$ denotes the Caputo fractional derivative of order $\alpha\in(0, 1)$, $t\in J$, $g\in \mathbb{R}^N$, $\omega_0 \in \mathfrak{D}^N$, and $f\in C (\mathbb{R}\times B_\rho \times \mathfrak{D}^N, \mathbb{R}^N)$. Here, $g_t\in \mathfrak{D}^N$ represents the history of the state from time $t-\gamma$ to the present time $t$, defined by $g_t(s) = g(t+s), \, s\in J_0$. In other words, $g_t = \{g(\tau):\tau\leq t\}$ represents the trajectory of the solution in the past.

We assume that the following conditions hold:

(1) The function $f$ guarantees that for any initial condition $(t_0, \omega_0)\in \mathbb{R}_+\times \mathfrak{D}^N$, the system (1.3) possesses a solution $g(t_0, \omega_0)(t)\in C^q([t_0, T], \mathbb{R}^N)$.

(2) $f(t, 0, 0) = 0$ for $t\geq t_0$.

We will utilize comparison results for the Caputo fractional time-varying system of the form

where $u\in \mathbb{R}^n$, $\zeta\in C[\mathbb{R_+}\times \mathbb{R}^n\times \mathfrak{D}^n, \mathbb{R}^n]$, $\mathfrak{D}^n = C(J_0, \mathbb{R}^n)$ and $\zeta(t, 0, 0)\equiv 0$. The function $\zeta$ ensures that for any initial values $(t_0, \theta_0) \in \mathbb{R}_+ \times \mathfrak{D}^n$, the system (1.4) with the given initial condition has a solution $u(t_0, \theta_0)(t) \in C^\alpha([t_0, T], \mathbb{R}^n)$.

This paper's primary goal is to use vector Lyapunov functions to examine the stability characteristics of Caputo fractional time-dependent systems with delay. This study utilizes the definition of the Caputo fractional Dini derivative for Lyapunov-like functions as introduced in ^[22,25], along with the application of the comparison theorem and differential inequalities.

2.   Preliminaries

In this paper, we adopt the Caputo (C) definition for fractional derivative, which is expressed as follows:

It is important to note that the Caputo approach has the advantage that the initial conditions for fractional differential equations using the Caputo derivative are expressed in the same form as those for integer-order differentiation, which have well-established physical meanings. There exist various definitions for fractional derivatives. Among the widely used definitions is the Grunwald-Letnikov (GL) fractional derivative, which is expressed as:

The Riemann-Liouville (RL) fractional derivative is of the form:

In all the definitions given above, we have that $n-1 < \alpha < n, \alpha > 0$, where $n$ is a natural number and $\Gamma(\cdot)$ represents the gamma function. In most applications, the order of $\alpha$ is often less than 1, so that $\alpha \in (0, 1)$. For simplicity of notation, we will use $^CD^\alpha$ instead of $^C_{t_0}D_t^\alpha$ so that the Caputo fractional derivative of order $\alpha$ of the function $g(t)$ is given as

In this paper, we define the following sets:

Remark 2.1. In the definitions mentioned above and throughout this paper, $n\leq N$.

Definition 2.1. ^[2] The Grunwald-Letnikov (GL) fractional Dini derivative is given by

Definition 2.2. A function $V(t, g_t): J \times C_\rho \rightarrow \mathbb{R}_+^N$ is considered a vector Lyapunov function for (1.3) if it is continuous on $J \times C_\rho$, satisfies $V(t, 0) = 0$, and is locally Lipschitz continuous with respect to the second argument.

Definition 2.3. ^[22,25] Let $(t_0, \omega_0) \in \mathbb{R_+} \times C[J_0, B_\rho]$ represent the initial condition of the initial value problem (IVP) (1.3) with $f\in C (\mathbb{R}\times B_\rho \times \mathfrak{D}^N, \mathbb{R}^N)$. The Caputo fractional Dini Derivative of the Lyapunov function $V(t, g_t)$ is defined as

where it is understood that $\omega(0) = g(t_0, \omega_0)(t)$ is the state of the system (1.3) at the current time $t$. $\omega_0$(0) is the initial condition of the system (1.3) at the beginning $t = 0$. Equivalently, (2.2) can be written as

Definition 2.4. A function $G \in C[\mathbb{R}^n, \mathbb{R}^n]$ is considered quasi-monotone nondecreasing in $x$ if, whenever $x \leq y$ and $x_i = y_i$ for $1 \leq i \leq n$, it follows that $G_i(x) \leq G_i(y)$ for all $i$.

Definition 2.5. ^[30] A function $a(r)$ is considered to be in the class $\mathcal{K}$ if $a$ is a continuous function on $[0, \rho)$ with values in $\mathbb{R_+}$, $a(0) = 0$, and $a(r)$ is strictly increasing in $r$.

Definition 2.6. ^[33] The zero solution of (1.3) is considered

(1) stable if, for every initial time $t_0 \in \mathbb{R_+}$ and any $\epsilon > 0$, there exists a $\delta = \delta(\epsilon, t_0) > 0$, continuous in $t_0$, such that for any initial function $\omega_0 \in \mathfrak{D}^N$ with $\|\omega_0\|_0 \leq \delta$, it follows that $\|g(t_0, \omega_0)(t)\| < \epsilon$ for $t \geq t_0$.

(2) asymptotically stable if, for every initial time $t_0 \in \mathbb{R_+}$ and any $\epsilon > 0$, there exists a $\delta = \delta(\epsilon, t_0) > 0$, continuous in $t_0$, such that for any initial function $\omega_0 \in \mathfrak{D}^N$ with $\|\omega_0\|_0 \leq \delta$, it follows that $\|g(t_0, \omega_0)(t)\| < \epsilon$ for $t \geq t_0$ and $\lim\limits_{t\rightarrow \infty}\|g(t_0, \omega_0)(t)\| = 0$.

3.   Main result

In this section, we present our findings on the stability and asymptotic stability of Caputo fractional time-dependent systems with delay. Our results are structured around lemmas and theorems that define the necessary conditions for stability and asymptotic stability.

Lemma 3.1. Assume $p(t), r(t)\in C([t_0, T), \mathbb{R}^N)$ and suppose there exists $\tau_*\in (t_0, T]$ such that $p(\tau_*) = r(\tau_*)$ and $p(t) < r(t)$ for $t\in [t_0, \tau_*)$. The inequality $^C D^\alpha_+ p(\tau_*) > ^C D^\alpha_+ r(\tau_*)$ holds if the Caputo fractional Dini derivative of $p$ and $r$ exists at $t = \tau_*$ for $\alpha\in(0, 1)$.

Proof. Applying the definition of the Caputo Dini derivative in (2.3), we have

It is clear from the hypothesis of the lemma that for $\tau_*\in(\tau_0, T], \, p(\tau_*)-r(\tau_*) = 0$ so that

Taking limit as $h\rightarrow0^+$, we have

Again by the hypothesis of the lemma, for $\tau = \tau_0, \, p(\tau_0)-r(\tau_0) < 0$ together with the fact that $\frac{(\tau-\tau_0)^{-\alpha}}{\Gamma(1-\alpha)} > 0$, leads to

hence the result. □

Lemma 3.2. Let $w, s : [t_0- \gamma, T] \rightarrow \mathbb{R}^n$ be continuous on $[t_0, T]$, and let $\zeta \in C([t_0, T] \times \mathbb{R}^n \times C_q, \mathbb{R}^n)$ be quasi-monotone nondecreasing in $w_t$ for each $(t, w) \in \mathbb{R}^n$. Additionally, for each $t$, we have

(i) $^CD^\alpha_+w(t)\leq \zeta(t, w, w_t)$,

(ii) $^CD^\alpha_+s(t) > \zeta(t, s, s_t), \, t\in[t_0, T]$.

Then

implies

Proof. Assume that the conclusion (3.2) of the theorem is false. Then, there would be a $t_1 > t_0$ such that

Applying Lemma 3.1, we obtain

Furthermore, from (3.1) and (3.3), we deduce that

Combining condition (ⅰ), (3.4), condition (ⅱ), (3.5), and the quasi-monotonicity of $G$, we have that at $t = t_1$

which is a contradiction, thus (3.5) is true. □

Theorem 3.1. Let $\zeta\in C[R_c, \mathbb{R}^n]$, where $R_c\subset \mathbb{R_+}\times\mathbb{R}^n\times C_q$ such that $R_c: = \{(t, u, \xi):t_0\leq t\leq t_0+a, \, \|u-\theta_0(0)\|\leq b, \, \|\xi-\theta_0\|_0\leq b, \, u\in \mathbb{R}^n, \, \xi \in C_q: = \{\xi\in \mathfrak{D}^n:\|\xi\| < q, q > 0\}, \, \theta_0 \in \mathfrak{D}^n, \, a, b > 0\}$ and $\|\zeta(t, u, u_t)\|\leq H$ on $R_c$. Assume that $\zeta(t, u, u_t)$ is quasi-monotone nondecreasing in $u_t$ for every $(t, u) \in \mathbb{R}_+ \times \mathbb{R}^n$. Then, the IVP (1.4) has a maximal solution $h(t, (t_0, \theta_0))$ defined on the interval $[t_0, t_0 + q]$, where $q = \text{min} \left\{a, \left(\frac{b \Gamma (\alpha + 1)}{2H + b}\right)^{\frac{1}{\alpha}}\right\}$ and $\alpha\in (0, 1)$.

Proof. Let $\eta \in \mathbb{R}_+^n$ be a small arbitrary vector, such that $\|\eta\| < \frac{b}{2}e$, where $e = (1, 1, ...1)^T$ with $\|e\| = 1$.

Consider the IVP for the following Caputo fractional time-dependent system of the form:

where $\zeta_\eta(t, u, u_t)+\epsilon$ is continuous on $R_\eta$ and is given by $R_\eta = \{R_\eta: = \{(t, u, \xi):t_0\leq t\leq t_0+a, \, \|u-(\theta_0(0)+\eta)\|\leq \frac{b}{2}, \, \|\xi-(\theta_0+\eta)\|_0\leq \frac{b}{2}, \, u\in \mathbb{R}^n, \, \xi \in C_q, \, \theta \in \mathfrak{D}^n, \, a, b > 0\}$ and $\|\zeta_\eta(t, u, u_t)+\eta\|\leq H$ on $R_\eta$ with $R_\eta \subset R_c$.

Integrating (3.6) from $t_0$ to $t$ in the Caputo sense, we obtain

Now, consider the family of solutions $\{u_\eta(t_0, \theta_0)(t)\}$ on $[t_0, t_0+q]$. Then from (3.7)

Therefore

Thus, the set of solutions $\{u_\eta(t_0, \theta_0)(t)\}$ has a uniform bound with bound $K$. We take $t_1, t_2\in [t_0, t_0+q]$, with $t_1 < t_2$, and produce the following estimate to demonstrate that the family of solutions $\{u_\eta(t_0, \theta_0)(t)\}$ is equi-continuous.

provided $|t_2-t_1| < \delta(\epsilon) = (\frac{\epsilon\Gamma(\alpha+1)}{2H+b})^{\frac{1}{\alpha}}$, hence the family $\{u_\eta(t_0, \theta_0)(t)\}$ is equi-continuous on $[t_0, t_0+q]$. Then, by the Arzela-Ascoli theorem, $\lim\limits_{i\rightarrow \infty}u_{\eta_i}(t_0, \theta_0)(t) = h(t_0, \theta_0)(t)$ uniformly on $[t_0, t_0+q]$ for every decreasing sequence $\{\eta_i\}, \, \eta_i\rightarrow 0$ as $i\rightarrow \infty$.. The uniform continuity of $\zeta$ implies that $\zeta(t, u_t(t_0, \theta_0))+\eta_i$ tends uniformly to $\zeta(t, h_t(t_0, \theta_0))$ as $\eta_i\rightarrow \infty$. Taking limit as $i\rightarrow \infty$ in (3.7) leads to

which demonstrates that the limit $h(t_0, \theta_0)(t)$ is truly a solution of (1.4) on the interval $[t_0, t_0+q]$.

It is left to show that $h(t_0, \theta_0)(t)$ is the maximal solution of the comparison system (1.4). Let $u(t_0, \theta_0)(t)$ be any solution of the IVP (1.4) on $[t_0, t_0+q]$. Then in light of Lemma 3.2, we have that

Then $\theta_0 < \theta_0+\eta, \, \eta > 0$ implies that $u(t_0, \theta_0)(t) < u_{\eta_i}(t_0, \theta_0)(t)+\eta_i$.

Since $\lim\limits_{i\rightarrow \infty}u_{\eta_i}(t_0, \theta_0)(t) = h(t_0, \theta_0)(t)$ uniformly on $[t_0, t_0+q]$, it follows by taking limits that $u(t_0, \theta_0)(t) < \lim\limits_{i\rightarrow \infty }\{u_{\eta_i}(t_0, \theta_0)(t)+\eta_i\} = h(t_0, \theta_0)(t)$ and so the result follows. □

Theorem 3.2. Assume that

(1) $V \in C[(-\gamma, \infty) \times C_\rho, \mathbb{R}^N_+]$, where $V(t, g_t)$ is locally Lipschitz continuous with respect to the second argument.

(2) $\zeta \in C[\mathbb{R_+} \times \mathbb{R}^n \times \mathfrak{D_q}, \mathbb{R}^n]$ and $\zeta(t, u, u_t)$ is quasi-monotone nondecreasing with respect to $u_t$.

(3) $^CD^\alpha_+ V(t, \omega(0), \omega) \leq \zeta(t, V(t, \omega(0)), V_t)$ for all $t \in \mathbb{R}_+$, where $V_t = V(t + s, \omega(s))$, $\xi \in J_0$.

If $h(t_0, \theta_0)(t)$ is the maximal solution of (1.4) and $g(t_0, \omega_0)(t)$ is any solution of (1.3) defined in the future such that

then the inequality

holds.

Proof. Let $g(t_0, \omega_0)(t)$ be any solution of (1.3) such that (3.8) holds.

For an arbitrary vector $\eta \in \mathbb{R}_+^n$ of sufficiently small magnitude, we examine the IVP associated with the Caputo fractional time-dependent system with delay as follows.

for $t \in \mathbb{R}_+$, where the solution $u_\eta(t_0, \theta_0)(t)$ exists as long as the maximal solution $h(t_0, \theta_0)(t)$ to the right of $t_0$ and satisfies the Volterra integral equation

Let $y(t) = V(t, g(t_0, \omega_0))$.

Since $\lim\limits_{\eta \rightarrow 0} u_\eta(t_0, \theta_0)(t) = h(t_0, \theta_0)(t)$, it is sufficient to show that

In the event that the inequality (3.12) is false, there would be a point $\tau > t_0$ such that

It follows from Lemma (3.1) that

Thus,

and using (3.10) we obtain

Therefore,

Let $g(t) = g(t_0, \omega_0)(t)$ be any solution of (1.3) such that (3.8) holds. Using the Caputo fractional Dini derivative (1) for $y(t)$, we then obtain for $t \in [t_0, T]$ the following

Given that $V$ is locally Lipschitz in the second variable with a Lipschitz constant $L > 0$, we derive

Let

so that

Therefore, using condition 3 of the theorem, we have that

Now (3.14) with $t = \tau$ gives

which contradicts (3.13), and hence (3.12) is true.

From the proof of Theorem 3.1, it can be concluded that the set of solutions $\{u_\eta(t_0, \theta_0)(t)\}$ is uniformly bounded and equi-continuous on the interval $[t_0, T]$. Therefore, according to the Arzelà-Ascoli theorem, there exist a decreasing subsequence $\{u_{\eta_k}(t_0, \theta_0)(t)\}$ and a continuous function $p(t_0, \theta_0)(t)$ that serves as the uniform limit of $u_{\eta_k}(t_0, \theta_0)(t)$ on the interval $[t_0, T]$. From (3.11) we have

Taking the limit as $k \rightarrow \infty$ in (3.16) leads to

which demonstrates that $p(t_0, \theta_0)(t)$ serves as a solution to (1.4) over the interval $[t_0, T]$. We claim that $p(t_0, \theta_0)(t)$ converges to the maximal solution $h(t_0, \theta_0)(t)$ on $[t_0, T]$. In order to demonstrate this, we take the limit in (3.12) for $\eta = \eta_k$ as $k \rightarrow \infty$. From there, we get $V(t, (t_0, \omega_0)(t)) \leq h(t_0, \theta_0)(t)$. □

Theorem 3.3. Assume that

(1) $\zeta \in C(\mathbb{R_+} \times \mathbb{R}^n \times \mathfrak{D}^n, \mathbb{R}^n)$, and $\zeta(t, u, u_t)$ is quasi-monotone nondecreasing in $u_t$ with $\zeta(t, 0, 0) = 0$.

(2) $V \in C[(-\gamma, \infty) \times C_\rho, \mathbb{R}^N_+]$, $V(t, 0) = 0$, and $V(t, g_t)$ is locally Lipschitz continuous in $g_t$ such that

holds for all $(t, g)\in \mathbb{R_+}\times B_\rho$.

(3) $a(\|g\|)\leq V_0(t, g_t)$, where $a\in \mathcal{K}$ and $V_0(t, g_t) = \sum_{i = 1}^{N}V_i(t, g_t)$.

The stability of the trivial solution $g = 0$ of the system (1.3) is therefore implied by the stability of the trivial solution $u = 0$ of the system (1.4).

Proof. Given $\epsilon \in (0, \rho]$ and $t_0 \in \mathbb{R}_+$, the stability of the trivial solution $u = 0$ of (1.4) indicates that for any $a(\epsilon) > 0$, $t_0 \in \mathbb{R}_+$, and initial function $\theta_0 \in \mathfrak{D}^n$, there exists a $\delta = \delta(t_0, \epsilon) > 0$ which is continuous in $t_0$ such that

where $u(t_0, \theta_0)(t)$ is any solution of (1.4). With $V(t, 0) = 0$ and the continuity of $V(t_0, \theta_0(0))$, it is ensured that there exists a $\delta_1 = \delta_1(t_0, \delta) > 0$ such that

Let $g(t_0, \omega_0)(t)$ be any solution of (1.3), with $\|\omega_0\|_0 < \delta_1$.

Claim:

Assuming (3.21) does not hold, there exists a $\tau > t_0$ such that $\|g(t_0, \omega_0(0))(\tau)\|_0 = \epsilon$ and $\|g(t_0, \omega_0(0))(t)\|_0 < \epsilon$ for $t \in [t_0, \tau)$.

Let $\theta_0 = V_0(t_0, \omega_0)$. Then, from (3.19), we have $V_0(t_0, \omega_0) < \delta < \epsilon$.

Let $h_m(t_0, \theta_0)(t) = \sum_{i = 1}^{n}h_i(t_0, \theta_0)(t)$ with $h_0(t_0, \theta_0) < \delta$ be the maximal solution of (1.4) such that

Therefore at $t = \tau$, we have that $\|g(t_0, \omega_0(0))(\tau)\|_0 = \epsilon$. Combining condition 3 of the theorem, (3.19) and (3.22) we obtain

This yields

which is a contradiction. Thus, (3.21) holds, leading us to conclude that the trivial solution $g = 0$ of (1.3) is stable. □

Theorem 3.4. Assume that

(1) $\zeta \in C(\mathbb{R_+} \times \mathbb{R}^n \times \mathfrak{D}^n, \mathbb{R}^n)$, and $\zeta(t, u, u_t)$ is quasi-monotone nondecreasing in $u_t$ with $\zeta(t, 0, 0) = 0$.

(2) $V \in C[(-\gamma, \infty) \times C_\rho, \mathbb{R}^N_+]$, $V(t, 0) = 0$, and $V(t, g_t)$ is locally Lipschitzian in $g_t$ such that

holds for all $(t, g)\in \mathbb{R_+}\times B_\rho$.

(3) $a(\|g\|)\leq V_0(t, g_t)$, where $a\in \mathcal{K}$ and $V_0(t, g_t) = \sum_{i = 1}^{N}V_i(t, g_t)$.

Consequently, the asymptotic stability of the trivial solution $g = 0$ of the system (1.3) is implied by the asymptotic stability of the trivial solution $u = 0$ of the system (1.4).

Proof. According to Theorem (1.3), the trivial solution of (1.4) is stable. Condition (ⅱ) of the theorem ensures that $V(t, \omega(0))$ is monotonically decreasing, and condition (ⅲ) further ensures that it is bounded below by zero. Therefore, there exists a limit

Claim: $G_0 = 0$

Assume that the claim is false. In other words, if we assume $G_0 \neq 0$, then $c(G_0) \neq 0$ because $c \in \mathcal{K}$. $V(t, \omega(0))$ being monotonically decreasing combined with (3.24) guarantees that $V(t, \omega(0)) > G_0$. Given that $c(r)$ is a monotonically increasing function of $r$, we can state that

so that

In terms of (3.23) we have

Integrating (3.25) from $t_0$ to $t$ we have

where $\mathbb{I}_N$ denotes an identity matrix of order $N$.

This implies that

so that as $t\rightarrow \infty$ in (3.26), we have that $\frac{c(G_0)}{\alpha\Gamma(q)}\bigg((t-t_0)^\alpha \bigg)\mathbb{I}_N\rightarrow \infty$ so that $V(t, \omega(0), \omega)\rightarrow -\infty$. This contradicts condition (3) of the theorem and so our claim that $V_0 = 0$ is true, that is $\lim\limits_{t\rightarrow \infty}V(t, \omega(0), \omega) = 0$. This demonstrates that the zero solution $u = 0$ of (1.4) is asymptotically stable. □

4.   Example

We demonstrate the benefit of employing the vector Lyapunov function over the scalar Lyapunov function with this example.

Consider the system of retarded nonlinear Caputo fractional differential equations

for $t\geq t_0$, with initial functions

where $\omega_1(s)$ and $\omega_2(s)$ are the initial functions defined on $-2 \leq s\leq 0$. We recall that the initial function $\omega_1(s)$ and $\omega_2(s)$ captures the state of the system at time $t+s$. In this example, $g_1(t) = \omega_1(s) = g_1(t+s)$, so that at $s = -2$ we have $g_1(t) = \omega_1(-2) = g_1(t-2)$. Similarly, $g_2(t) = \omega_2(-2) = g_2(t-2)$. With these, the system (4.1) can therefore be written as

Now we consider a scalar Lyapunov function for (4.1) given by

Then according to (2.3) we obtain

Applying Eqs (3.7) and (3.8) in ^[26], we obtain

As $t \rightarrow \infty$, the first two terms tend to zero, and using (4.2) we have

Therefore, we have

Now consider the scalar comparison equation

where $\theta_0 = 2$ remains constant throughout the given interval. Solving (4.4) by the Laplace transform method and noting that $u(t-2)$ is a Heaviside step function, we obtain the following:

This implies that

so that

Taking the inverse Laplace transforms we obtain

so that

Using the fact that $\mathcal{L}(t^\alpha) = \frac{\Gamma(\alpha+1)}{s^{\alpha+1}},$ we have

We observe that

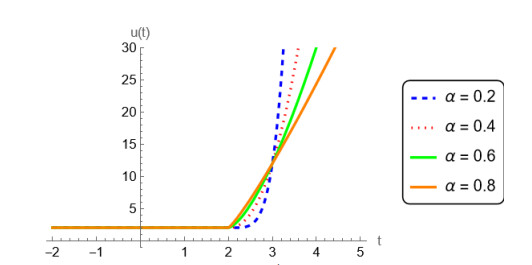
As $t$ increases, the term $10(t-2)^{\frac{1}{\Gamma(\alpha)}}$ grows causing $u(t)$ to be unbounded. This indicates that for any nonzero initial condition $\theta_0$, $u(t)$ will eventually grow without bound as $t$ increases. Hence for any small $\delta > 0$ such that $\|\theta_0\|_0 < \delta$, there exist some $t > 0$ at which $u(t)$ becomes unbounded. This means that no matter how small we choose $\delta$, $u(t)$ will eventually exceed any prescribed $\epsilon$.

All the conditions of Theorem 3.3 are satisfied, except that the trivial solution $u = 0$ of (4.5) is not stable (also see Figure 1). Therefore Theorem 3.3 cannot yield any stability information for the zero solution of (4.4).

We now examine a vector Lyapunov function with the following form:

where $V_1 = |\omega_1(-2)|$ and $V_2 = |\omega_2(-2)|$, with $\omega = (\omega_1, \omega_2)\in \mathbb{R}^2$, so that the associated norm $\|\omega\| = \sqrt{\omega_1^2+\omega_2^2}$.

Now,

and so $a(\|\omega\|)\leq V_0(t, x_t)$ with $a(r) = r$, implying that $a\in \mathcal{K}$. We compute the Caputo fractional Dini derivative for $V_1 = |\omega_1(-2)|$ using (2.3) as follows:

Applying Eqs (3.7) and (3.8) in ^[26], we obtain

As $t \rightarrow \infty$, the first term tends to zero, and using (4.2) we obtain

Therefore

Similarly, we compute the Caputo fractional Dini derivative for $V_2 = |\omega_2(-2)|$ using (2.3) as follows:

Applying Eqs (3.7) and (3.8) in ^[26], we obtain

As $t \rightarrow \infty$, the first term tends to zero, and using (4.2) we obtain

Therefore,

Combining (4.7) and (4.8), we obtain

Now consider the comparison system

where $A = \begin{pmatrix} -8 & 1 \\ 2 & -4 \end{pmatrix}, \, u(\xi) = \theta_0 \, \text{for} \, \xi\in[-2, 0]$, with $\theta_0 = (2, 2)^T$ being a constant function defined over the interval.

The vector inequality (4.9), along with the required conditions for using vector Lyapunov functions as outlined in Theorem 3.3, is fulfilled by (4.6). In fact, the eigenvalues of $A$ have negative real components. Consequently, by Theorem 3.3, we can conclude that the zero solution $g = 0$ of the system (4.1) is not only stable but also asymptotically stable.

5.   Conclusions

Due to the increasing scholarly interest in fractional time-dependent systems with delay, known for their improved accuracy in modeling problems with hereditary and memory behaviors, this paper examines the stability and asymptotic stability dynamics of Caputo fractional time-dependent systems with delays using vector Lyapunov functions. By applying the Caputo fractional Dini derivative and introducing a new comparison theorem, we have established robust stability and asymptotic stability conditions for these systems. Our method advances beyond traditional scalar Lyapunov function approaches and enhances existing stability results. The provided example highlights the practical benefits and improved accuracy of our approach, representing a significant advancement in the field.

Author contributions

Jonas Achuobi: Conceptualization, methodology and writing of the original draft of the manuscript. Edet Akpan: Conceptualization, methodology and supervision. Reny George: Conceptualization, review, editing, validation and funding acquisition. Autine Ofem: Validation. All authors have read and agreed to the published version of the manuscript.

Acknowledgments

The authors extend their appreciation to Prince Sattam bin Abdulaziz University for funding this research work through the project number (2024/01/922572).

Conflict of interest

The authors declare that they have no conflict of interest.