Adaptive event-triggered reachable set control for Markov jump cyber-physical systems with time-varying delays

1.   Introduction

The integration of physical systems and network systems has become a trend. This integration forms cyber-physical systems (CPSs) ^[1]. CPSs realize real-time information interaction between cyber and physical modules, which have the advantages of efficiency, convenience, and transparency. A typical CPS consists of three parts: sensing part, network part, and control part. In the practical application of some CPSs, external disturbances, internal failures, and changes in the working environment often occur ^[2]. Those situations can lead to abrupt changes in the modes of the CPS, while also causing the system to not operate safely ^[3]. Markov jump cyber-physical systems (MJCPSs) introduce Markov jump properties to describe the abrupt change of system modes. Scholars have paid increasing attention to MJCPSs in recent years and have obtained some research results, such as attack-compensated output control ^[4], sliding mode control ^[5], and adaptive resilient control ^[6].

MJCPSs are characterized by the openness of network transmission, thus making them vulnerable to the threat of cyber attacks during information transmission. Common cyber attacks include deception attacks ^[7] and denial of service (DOS) attacks ^[8]. Deception attacks are related to the state of the system and carefully designed by the attacker. As a result, deception attacks tend to be more destructive than DOS attacks ^[9], the most typical of which are false data injection (FDI) attacks. When the system state information of MJCPSs is transmitted between physical and network modules, the attacker tampers with the system state information by secretly injecting false information, which leads to system paralysis. Reachable set control is an important branch of system security research and the reachable set control can defend against FDI attacks. The existence of FDI attacks and external disturbances will destroy the security of the system and make the system unstable. The system can be restored to normal by reachable set control. Therefore, reachable set control has great significance in theoretical research and practical application. The reachable set is the set of all states of the system that can be reached from the original state under the influence of bounded disturbances ^[10]. Scholars have conducted reachable set research on stealth attacks ^[11], deception attacks ^[12], and hybrid attacks ^[13] that MJCPSs may be subjected to. However, there are few studies on the reachable set of discrete MJCPSs, and even fewer on the reachable set of MJCPSs under FDI attacks. Therefore, obtaining more applicable reachable set control results for discrete MJCPSs under FDI attacks is a motivation for this paper.

Influenced by physical factors such as material and temperature, the transmission of signals at actual MJCPSs creates time delays that cannot be eliminated^[14]. In the transmission of the signal, most of the time delays are not constant. The magnitude of time delays varies with time because of the physical factors, such as material and temperature change. This kind of delay is called time-varying delay. It makes the current state information of the system also contain the state information of the previous time ^[15]. The presence of system delays increases the conservatism of research results in the reachable set of MJCPSs^[16]. Therefore, reducing the conservatism that system delays bring to the study of reachable sets of MJCPSs is another motivation for this paper.

Since MJCPSs are characterized by data exchange and sharing, remote monitoring and control, they require continuous network transmission. However, network bandwidth is usually limited, leading to problems such as information congestion and inefficient utilization of network resources during data transmission. Event-triggered control strategies can effectively save network resources. Moreover, event-triggered control strategies can also reduce the impact of cyber attacks and external disturbances on the MJCPS ^[17]. There are many kinds of event-triggered control strategies, including but not limited to hybrid-triggered security control strategies ^[18], dynamic event-triggered security control strategies ^[19], and memory-based event-triggered security control strategies ^[20]. Ordinary event-triggered control strategies give fixed trigger thresholds and consider global information. Adaptive event-triggered control strategies introduce dynamic trigger thresholds that eliminate the need for global data and provide more flexible trigger conditions. It can flexibly change the trigger threshold when FDI attacks and external disturbances occur. This reduces the number of triggers and increases the security of the MJCPS. Obtaining more effective reachable set control effects through adaptive event-triggered control strategies is also the research motivation.

The contributions of the paper are as follows:

1) In this paper, the reachable set problem of MJCPS with time-varying delays and external disturbances under FDI attacks is studied for the first time. Compared to other papers^[21], this paper considers a variety of adverse effects on the MJCPS, and the results are more applicable.

2) The adaptive event-triggered control strategy is added to save network resources during data transmission. It also reduces the impact of FDI attacks and external disturbances on the MJCPS.

3) By constructing the new Lyapunov-Krasovskii (L-K) functional with system time-varying delays and utilizing the Wirtinger inequality and the improved extended reciprocally convex matrix inequality, sufficient conditions of less conservative for reachable set estimation are derived. It is transformed into the form of the linear matrix inequality (LMI) and solved to obtain the optimal solution of the reachable set controller.

4) The validity of the results is proved by a boost converter circuit system example.

Notations: $\mathbb{R}^{n}$ stands for an $n$-dimensional real matrix; $\mathbb Z$ stands for nonnegative integers; and $\mathbb E\left\{ \cdot \right\}$ represents the mathematical expectation. The symmetric term in a symmetric matrix is represented by the symbol $*$, and $\left\| \cdot \right\|$ indicates the Euclidean norm. The diagonal matrix is represented by the symbol $\rm diag\left\{ \cdots \right\}$.

2.   Problems formulation and preliminaries

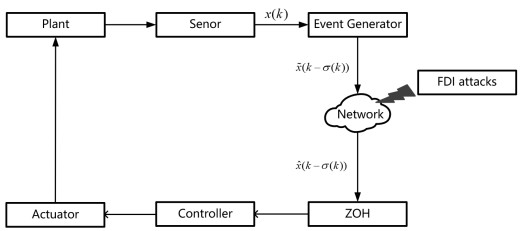
Figure 1 shows the design diagram of the reachable set controller of the MJCPS under FDI attacks. As can be seen from the figure, the sensor obtains the sampled signal $x(k)$ and sends it to the event generator. The event generator is compared the sampled signal with the trigger conditions. The signal which meets the conditions will be sent to the network transmission. When the signal does not meet the trigger conditions, the zero-order hold (ZOH) will keep the information of the previous time unchanged to ensure the continuity of the signal. In the transmission process, it is assumed that the network-induced delay of the network transmission is $\sigma (k)$, which satisfies $\sigma (k) \in [0, {\sigma _M}]$, and the attacker randomly carries out FDI attacks. Finally, the controller processes the signal $\hat x(k - \sigma (k))$.

2.1.   Cyber-physical system model description

Consider the following MJCPS:

where $x(k)\in \mathbb{R}^{n}, u(k)\in \mathbb{R}^{m}, K_{r_k}$ represent the state vector, the controller input, and the controller gain; $d(k)$ is a time-varying delay; $d(k) \in \left[ {{d_m}, {d_M}} \right]$, where $d_m$ and $d_M$ are constant; $w(k)\in \mathbb{R}^{l}$ represents the external disturbance, and the following mean bounded condition is met:

where $\varpi$ is a constant. $A_{r_k}, B_{r_k}, C_{r_k}, D_{r_k}, F_{r_k}$ are constant matrices of known suitable dimensions. Here, $\{{r_k}, k\in \mathbb Z\}$ is a discrete-time Markov chain, which takes from a finite space $\mathbb N = \{1, 2, \dots, N\}$. The system transition probability is $\pi_{pq} = Pr\{r_{k+1} = q|r_k = p\}$, where $0\leq \pi_{pq} \leq 1$ for all $p, q\in \mathbb N$, and $\sum_{q = 1}^{N}\pi_{pq} = 1$ for all $p\in \mathbb N$. $\Pi = \left[ {{\pi _{{\rm{pq}}}}} \right]$ is a matrix of transition probability.

2.2.   Adaptive event-triggered control strategy

The adaptive event-triggered control strategy is adopted to reduce the waste of network resources. The corresponding trigger condition is:

where $k_m$ represents the most recent trigger time; $x(k_m)$ denotes the latest transmitted state; and ${Q}$ is the undetermined positive definite weighting matrix. The adaptive triggered threshold $\vartheta(k)$ meets:

where $\Delta \vartheta (k) = \vartheta (k + 1) - \vartheta (k)$ and $\vartheta _0 > 0$. It is inevitable that there is transmission delay in the transmission process. The delay existing in network transmission is analyzed as in references ^[22] and ^[23]. The mechanism (2.3) can be rewritten as:

where $e_x = x(k)-x(k_m)$. The delay is $0\leq\sigma(k)\leq \sigma_M$, and $\sigma_M$ is a constant. So, $x(k)$ after passing the adaptive event-triggered control strategy can be expressed as: $\tilde x(k-\sigma(k)) = x(k-\sigma(k)) - {e_x}$.

Remark 1. The common event-triggered control strategy relies on global information to set a fixed trigger threshold, which may cause the loss of important information. The adaptive event-triggered control strategy in this paper not only preserves system data as much as possible, but also helps the system recover quickly after FDI attacks and external disturbances happened. Compared with other adaptive laws, this adaptive law is adjusted by focusing on the difference in state near the trigger time. When the FDI attack or the disturbance occur, $e_x$ will increase, then $\Delta \vartheta (k)$ will increase, consequently causing an increase in $\vartheta (k)$. The trigger condition will become harder, and the system will maintain its previous trigger state unchanged under the ZOH, thereby effectively mitigating the impact of FDI attacks and disturbances on MJCPSs state. In addition, in order to avoid Zeno behavior, the sampling period of the system is used as the minimum time interval between two events.

2.3.   FDI attacks

FDI attacks occur during network communications such as data sharing and remote control in the MJCPS (2.1). Different from the previous attacks from ^[24], the attacker eliminates the original correct data in the system while carrying out the attacks signal input, which is more destructive to the system. The attacks signal model can be expressed as:

where $\alpha(k) = 0$ or $\alpha(k) = 1$ represent failure or success of the attack launched by the attacker at time $k$. $\alpha(k)$ satisfies the Bernoulli distribution; $\mathbb E\{\alpha(k)\} = Prob\{\alpha{(k)} = 1\} = \hat{{\alpha}}, \mathbb E\{1-\alpha(k)\} = Prob\{\alpha{(k) = 0}\} = 1-\hat{{\alpha}}$; and ${\xi(\tilde x(k-\sigma(k)))}$ denotes the FDI attacks signal in discrete time, satisfying:

where $G_M$ is a known matrix.

Remark 2. FDI attacks pose a huge threat to the security of the MJCPS as they are well-designed by the attackers. The attack model in this paper is to erase the original state information of the MJCPS and inject error information. In order to avoid the attacks signal being detected and improve the success rate of the FDI attack, the attacker sets an energy upper limit for the attacks signal. In the actual MJCPS, the attacks signal will be affected by the external environment, signal disturbances, and other factors. Therefore, it is random whether the FDI attacks signal can be injected into the MJCPS. In this paper, the Bernoulli distribution function is used to describe the probability distribution of whether an attacker launches a successful FDI attack. In order to be closer to the actual MJCPS, the system state in this paper introduces the corresponding delay in the network transmission after the event-triggered control strategy processing, so the FDI attacks signal should contain the corresponding delay.

2.4.   Preliminary

When ${r_k} = p$, there is the system such that:

Here are the definition and a few lemmas.

Definition 1. ^[25] For $\forall k \ge 0$, the reachable set of the MJCPS is denoted as follows:

and the ellipsoid bound of the MJCPS can be given as:

Lemma 1. ^[26] For a given symmetric matrix $R > 0$, a sequence of discrete-time variable $\delta:Z[\gamma_1, \gamma_2]\to \mathbb{R}^n$, and integers $0\leq\gamma_1\leq \gamma_2,$

where

Lemma 2. ^[27] For a real scalar $0 < \chi < 1$, real matrices $D_1 > 0, D_2 > 0$ and an arbitrary matrix $N$, we obtain

Lemma 3. ^[28] Consider the MJCPS (2.1) with a well-squared bounded external disturbance, assuming that $V(x_k, r_k)$ is an L-K functional satisfying the initial condition $V(x_0, r_0) = 0$ and $w(k)$ satisfies (2.2). If there exists a scalar $\beta \in(0, 1)$, for any $\forall k\ge0$: $\mathbb E [V(x_{k+1}, r_{k+1})|x_k, r_k]-\beta V(x_k, r_k)-\frac{1-\beta}{\varpi}{w} ^T(k){w}(k)\leq0$, then $\mathbb E [ V(x_k, r_k)|x_0, r_0]\leq 1$.

3.   Reachable set estimation analysis

In this part, we give the analysis of reachable set estimation for the MJCPS (2.1). First, the range of the reachable set for the MJCPS is obtained by estimation in Theorem 1. Second, the controller gain of the MJCPS reachable set controller is obtained in Theorem 2. Define the following symbols:

with

3.1.   Reachable set estimation of the MJCPS

Theorem 1. For given scalars $d_M, d_m, \sigma_M, \vartheta _0, \hat{\alpha}$, and the matrix $G_M$, if there is a scalar $0 < \beta < 1$, positive definite matrices $P_i{(i = 1, \ldots, 7)}, U_p\in \mathbb{R}^{n\times n}$, ${N_i(i = 1, \ldots, 4)} \in \mathbb{R}^{2n \times 2n}$, such that, for $\forall p \in \mathbb N$ :

where

the ellipsoid set $\varepsilon _x(U_p, 1)$ is a reachable set of the MJCPS (2.8).

Proof: We choose the L-K functional:

where

Define $\Delta V(k, x_k, r_k)$ as the forward difference, then we have:

where ${\Phi}_0^p = \sum_{q\in N}\pi_{pq}l_p^T{U_q} l_p$.

where

Those can be obtained by Lemma 1:

It is estimated by Lemma 2 that:

and using the same processing method as (3.12), the another integral term can be estimated as:

Therefore, it can be obtained that:

where

In addition, assuming the attacks signal $\xi (x(k-\sigma(k)))$ satisfies (2.7), we have:

Meanwhile, from (2.5), we can get:

Combining (3.7)–(3.17), we get:

where

To obtain the reachable set of the MJCPS (2.1), the following function is defined:

Combining (3.18) and (3.19), we get:

where $\bar{\Phi}^p = {\Phi}^p-\frac{1-\beta}{\varpi}w(k) ^Tw(k)$.

By using the Schur complement, if (3.1)–(3.4) hold, $\bar{\Phi}^p\leq0$ holds, which means $\mathbb E[V(k+1, x_{k+1}, r_{k+1})|k, x_k, r_k]-\beta V(k, x_k, r_k)-\frac{1-\beta}{\varpi}w^Tw$ $\leq0$ holds, then based on Lemma 3, $\mathbb E[x^T(k)U_px(k)]\leq 1$ holds. This completes the proof.

Remark 3. In general, after constructing the L-K functional, the reachable set estimation result, which is obtained by applying the Jensen summation inequality to handle summation terms, tends to be conservative. In order to reduce conservatism, researchers have adopted many methods, which also increase the difficulty of computation ^[29]. This paper employs the Wirtinger inequality and improved extended reciprocally convex matrix inequality to estimate the integral term, which reduce the conservatism of the results and the complexity of the calculation. At the same time, by designing $V_2(k, x_k)$, two summation terms are cleverly eliminated in $\Delta V_2(k, x_k)$. In addition, more time-varying delays and system information are introduced. These further reduce conservatism of the results and calculation difficulty.

Remark 4. In this paper, the problem of reachable sets for the discrete-time MJCPS under FDI attacks is studied for the first time. Unlike the result of previous studies, this paper considers the effect of unfavorable factors such as time-varying delays information, mode jumps, and FDI attacks. Considering these factors makes it difficult to design the controller. In addition, it is also difficult to reduce the conservatism of the results and the complexity of the calculation at the same time. In order to solve these difficulties, the L-K functional with more time-varying delays is constructed, and the satisfactory results are obtained through the Wirtinger inequality and improved extended reciprocally convex matrix inequality techniques.

3.2.   Reachable set controller design

In this part, the reachable set controller is designed and obtained.

Theorem 2. For given scalars $d_M, d_m, \sigma_M, \vartheta_0, \hat{\alpha}$, and matrices $G_M, \Theta$, if there is a scalar $0 < \beta < 1$, positive definite matrices are $\hat{P}_{i}^p\; (i = 1, \ldots, 7), {H_p}\in \mathbb{R}^{n\times n},$ $\hat{N}_{ip}\; (i = 1, \ldots, 4)\in \mathbb{R}^{2n \times 2n}, \hat{K}_p\in \mathbb{R}^{m \times n}$, such that, for $\forall p \in \mathbb N$:

where

Then, controller gains are given by $K_p = \hat{K_p}H_p$ and the reachable set of (2.8) is defined by $\varepsilon _x(\Theta, 1)$.

Proof. Define $H_p = U_P^{-1}, \hat{P}_{i}^p = H_p^T{P_i}H_p\; (i = 1, \ldots, 7), \hat{N}_{ip} = H_p^T{N_i}H_p\; (i = 1, \ldots, 4),$ $\hat{Q} = H_p^T{Q}H_p, \hat{K_p} = K_p H^{-1}_p, h_p = {\rm diag}\{\underbrace{H_p\ldots H_p}_{13}, I, \underbrace{H_p\ldots H_p}_{N+5}\}.$

By pre-multiplying and post-multiplying LMIs (3.1)–(3.4) with $h_p^T$ and $h_p$, we get:

where $\hat{\Psi}_i^p\; (i = 1, \ldots, 4)$ are defined in (3.22)-(3.25). If $\hat{\Psi}_i^p < 0\; (i = 1, \ldots, 4)$ hold, that means $h_{p}^T \Psi_i^p h_p < 0\; (i = 1, \ldots, 4)$ are true, so $\Psi_i^p < 0\; (i = 1, \ldots, 4).$

Then, combining Theorem 1:

from LMI (3.21), we obtain $-H_p+H^T_p\Theta H_p < 0$. Due to $H_p = U_p^{-1}$, we get $-U_p+\Theta < 0$. Eventually, we get:

Therefore, when (3.21)–(3.25) are satisfied, the reachability set of the system can be obtained, and the controller gain $K_p$ of the MJCPS can be obtained by solving $K_p = \hat{K_p}H_p$. This completes the proof.□

4.   A practical example

In this part, a practical example is given to verify the validity of the reachable set control method to the MJCPS (2.1).

Example. (Boost converter circuit system) In order to verify the validity of the result, example Boost converter circuit system is used for verification ^[30]. The corresponding parameters and known matrices are set as follows:

Also, given scalars $d_M = 2, d_m = 1, \sigma_M = 1, \vartheta_0 = 0.15, \hat{\alpha} = 0.5$, and matrices $G_M = \left[ {\begin{array}{*{20}{c}} {0.01} & 0\\ 0 & {0.02} \end{array}} \right]$, $\Theta = \left[ {\begin{array}{*{20}{c}} {0.5389} & 0\\ 0 & {0.5389} \end{array}} \right], \Pi = \left[ {\begin{array}{*{20}{c}} {0.8} & {0.2}\\ {0.3} & {0.7} \end{array}} \right],$ one of the Markov jump processes is shown in Figure 2.

The random external disturbances signal conforming to $\left\| {w(k)} \right\| \le 0.3$ is selected and shown in Figure 3. The FDI attacks signal is ${\left[ {\begin{array}{*{20}{c}} { - \tanh (0.1{x_1}(k))}\\ {\tanh (0.01{x_2}(k))} \end{array}} \right]^T}$ and shown in Figure 4.

The resident time and trigger time of adaptive event-triggered control strategy are shown in Figure 5. It can be found that after the adaptive event-triggered control strategy is added to the MJCPS, the event generator triggers a total of 59 times within 100 seconds, effectively saving network resources and improving transmission efficiency.

By using LMI solving technology, the value range of $\beta$ is [ 0.875, 1). We select $\beta = 0.88$, then the controller gain $K_p$ can be solved.

The ellipsoid reachable set $\varepsilon (\Theta, 1)$ of all states that the MJCPS can reach from the initial state is shown in Figure 6. It can be seen that all states of the MJCPS are in the ellipsoid range, which proves that the designed controller is effective.

5.   Conclusions

In this paper, the reachable set problem of MJCPS (2.1) with time-varying delays and external disturbances under FDI attacks is studied for the first time. To begin, by selecting the L-K functional with delays information and combining the discrete Wirtinger inequality and the improved extended reciprocally convex matrix inequality, the conservatism and computational difficulty of the result are reduced. Additionally, the adaptive event-triggered control strategy is introduced to reduce the waste of network resources. At the same time, it reduces the impact of FDI attacks and external disturbances on the MJCPS security. What's more, the controller gain of the MJCPS is obtained by using LMI technology. Finally, the validity of the research results is verified by the circuit simulation of the boost converter circuit system.

Author contributions

All authors contributed to the study conception and design. The first manuscript was written by Sheng-Ran Jia. Wen-Juan Lin provided guidance and comments on the manuscript. All authors have read and approved the final version of the manuscript for publication.

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgments

This work was supported in part by the National Natural Science Foundation of China (Grant No. 62103213), the Shandong Provincial Natural Science Foundation (Grant No. 2023HWYQ-086, ZR2021QF002), the China Postdoctoral Science Foundation (Grant No. 2022M721748), and in part by Young Talent of Lifting engineering for Science and Technology in Shandong, China.

Conflict of interest

The authors declare no conflict of interest.