1.
Introduction
String algebras play an important role in representation theory; they are closely related to many algebras, such as biserial algebras, gentle algebras, and Nakayama algebras. In [17], Wald and Waschbüsch described all indecomposable modules over tame biserial algebras by V-sequences and primitive V-sequences; and provided an important theorem that shows an arbitrary indecomposable module over tame biserial algebra can be corresponded by some V-sequences or some pairs of primitive V-sequences and Jordan blocks. But this corresponding is not bijective, except for the case all projective–injective modules over tame biserial algebras to be uniserial. In [3], Butler and Ringel introduced strings and bands on bound quiver, which are special V-sequences and primitive V-sequences, and show that if the tame biserial algebras are string algebras, then the above descriptions given by Wald and Waschbüsch provide a bijection M to describe the indecomposable modules over string algebra. This result points out that a string algebra is representation-finite, that is, the number of isoclasses of an indecomposable module is finite; if and only if its bound quiver does not contain bands.
With the research of Wald, Waschbüsch, Butler, and Ringel, we can conduct further research on string algebras and gentle algebras. For example, the tensor algebras and Clebsch–Gordan problems of string algebras [11,14] derived representation-types of gentle algebras [18,19], the (co)homologies and homological dimensions of string and gentle algebras [8,12], Cohen–Macaulay–Auslander algebras of string and gentle algebras [4,5,13], the tilting and silting theories of gentle and skew-gentle algebras [1,6,10] and so on.
In [7], Gabriel showed that a finite-dimensional connected basic hereditary algebra is representation-finite if and only if the underlying graph of its quiver is one of the Dynkin diagrams Am with m≥1, Dn with n≥4, E6, E7, and E8, that also appear in Lie theory (see, for example, [9]). Later, Bernstein, Gelfand, and Ponomarev [2] gave a very elegant and conceptual proof underlining the links between the two theories by applying the nice concept of reflection functors, and, furthermore, they showed that the number of isoclasses of indecomposable modules over A, a path algebra of Dynkin quiver Am, Dn, E6, E7, and E8, equals to m(m+1)2, n2−n, 36, 63, and 120, respectively. c.f. [16, Chap IIV, Theorem 5.10, (c)]. In this paper, we focus on how to compute the number of isoclasses of indecomposable modules over string algebra and show the following result:
Theorem 1.1. Let A be a basic and connected string algebra.
(1) (Theorem 3.7) If for each vertex v of its bound quiver (Q,I), there exists at most one arrow ending at v and there exist at most two arrows starting from v, then the number of indecomposable modules over A is
where P(v) is the indecomposable projective module corresponded by v∈Q0, and Dv,1 and Dv,2 are direct summands of radP(v)=Dv,1⊕Dv,2.
(2) (Theorem 3.8) If for each vertex v of its bound quiver (Q,I), there exist at most two arrows ending at v and there exists at most one arrow starting from v, then the number of indecomposable modules over A is
where E(v) is the indecomposable injective module corresponding to v∈Q0, and Dv,1 and Dv,2 are direct summands of E(v)/socE(v)=Dv,1⊕Dv,2.
Furthermore, we obtain a corollary from the above theorem as follows:
Corollary 1.2 (Example 4.2). The number of isoclasses of indecomposable modules over A=kQ/I is dimkA, where the underlying graph of Q is type A and I is an arbitrary admissible ideal of kQ.
2.
Preliminaries
In this section, we recall the definition and some properties of string algebras. We refer the readers to [3] for more details. Throughout this paper, we always assume that: k is an algebraically closed; Q=(Q0,Q1,s,t) is a finite connected quiver; s and t are the functions Q1→Q0 sending any arrow in the arrow set Q1 of Q to its starting point and its ending point lying in the vertex set Q0 of Q; for any two paths p1 and p2 with t(p1)=s(p2), the composition is denoted by p1p2; I, the ideal of the path algebra kQ of Q, is admissible; and, for the algebra A=kQ/I of bound quiver (Q,I), all modules we considered are right A-module.
The bound quiver (Q,I) is said to be a string pair if it satisfies the following conditions:
(1) Any vertex of Q is the source and target of at most two arrows;
(2) For each arrow β, there is at most one arrow γ such that βγ∉I;
(3) For each arrow β, there is at most one arrow α such that αβ∉I;
(4)I is generated by paths of length great than or equal to 2.
Furthermore, (Q,I) is said to be a gentle pair if it is a string pair such that the following conditions hold:
(5) For each arrow β, there is at most one arrow γ such that βγ∈I;
(6) For each arrow β, there is at most one arrow α such that αβ∈I.
(7)I is generated by paths of length 2.
Definition 2.1. A finite-dimensional algebra A=kQ/I is called a string (resp. gentle) algebra if its bound quiver is a string (resp. gentle) pair (Q,I).
For any arrow a∈Q1, we denote by a−1 the formal inverse of a. Then s(a−1)=t(a), and t(a−1)=s(a). We denote by Q−11:={a−1∣a∈Q1} the set of all formal inverses of arrows. Any path p=a1a2⋯aℓ in (Q,I) naturally provides a formal inverse path p−1=a−1ℓa−1ℓ−1⋯a−11 of p. For any path ev of length one corresponding to v∈Q0, we define e−1v=ev.
Definition 2.2. A string on a string pair (Q,I) is a sequence s=(p1,p2,…,pn) such that:
(1) For any 1≤i≤n, pi or p−1i is a path in (Q,I);
(2) If pi is a path, then pi+1 is a formal inverse path;
(3) If pi is a formal inverse path, then pi+1 is a path;
(4) t(pi)=s(pi+1) holds for all 1≤i≤n−1, which are called turning points.
A band b=(p1,p2,…,pn) is a string such that:
(5)t(pn)=s(p1), and if pn and p1 are paths, then pnp1∉I, if pn and p1 are formal inverse paths, then (pnp1)−1∉I;
(6)b is not a non-trivial power of some strings, i.e., there is no string s such that b=sm for some m≥2.
A vertex v on a string s is called a source if one of the following conditions holds:
● v is a turning point t(pi)=s(pi+1) such that pi is a formal inverse path and pi+1 is a path;
● p1 is a path, and v=s(s)=s(p1);
● pn is a formal inverse path, and v=t(s)=t(pn).
We can define sink in a dual way.
If n=1, then we call s a direct string. In particular, s is called a trivial string if it is empty. Two strings s and s′ are called equivalent if s′=s or s′=s−1; two bands b=α1⋯αn and b′=α′1⋯α′t are called equivalent if b[t]=b′ or b[t]−1=b′, where b[t]=α1+t⋯αnα1⋯α1+t−1. We denote by Str(A) the set of all equivalent classes of strings and by Band(A) the set of all equivalent classes of bands on the bound quiver of A. In [3], Butler and Ringel showed that all indecomposable modules over a string algebra can be described by strings and bands. To be more precise, we have the following result:
Theorem 2.3 (Butler–Ringel). Let A=kQ/I be a string algebra. Then there is a bijection
where ind(modA) is the set of all isoclasses of indecomposable A-modules and J is the set of all indecomposable k[x,x−1]-modules.
Usually, if M−1(N) is a (direct) string, then we say N is a (direct) string module; if M−1(N) is a band with some pairs (n,λ), we say it is a band module. The original definition of string and band modules over string algebra can be referred to [3].
3.
The number of all isoclasses of indecomposable modules
Now, for simplification, we always assume that all bound quivers we considered are string pairs whose underlying graph ¯Q is a tree (i.e., ¯Q does not contain a cycle), and all algebras we considered are string algebras, which are of the form kQ/I (up to isomorphism) in this section.
3.1. The string with unique source/sink
Lemma 3.1.
(1) If a string s on (Q,I) has a unique source, then it is one of the following:
(A) s is a direct string, that is,
(B) s=p1p2, where p1 is a formal inverse path and p2 is a path, that is,
(2) Dually, if a string s on (Q,I) has a unique sink, then it is one of the following forms:
(A') s is a direct string, that is,
(B') s=p1p2, where p1 is a path and p2 is a formal inverse path, that is,
Proof. We only prove (1); the proof of (2) is similar. If s has at least two sources, then it has at least one sink. So, it has a substring, which is of the form
It is easy to see that s has at least two sources in this case, a contradiction. □
Let p=a−1r⋯a−12a−11b1b2⋯bt be a string with r,t≥0, a−11,…,a−1r∈Q−11 and b1,…,bt∈Q1. If it satisfies the following conditions:
(P1) t(a−11)=s(b1);
(P2) For any α∈Q1 with t(ar)=s(α), ar′ar′+1⋯arα∈I for some 1≤r′≤r;
(P3) For any β∈Q1 with t(bt)=s(β), bt′bt′+1⋯btβ∈I for some 1≤t′≤t.
Then M(p) is an indecomposable projective A-module; we call that p is a projective string in this case. Dually, we can define any indecomposable injective string.
Lemma 3.2. If a string s on (Q,I) has a unique source, then it is a substring of some projective string p such that s and p have the same source.
Proof. If s is projective, we have finished. If s is not projective, assume that s lies in Lemma 3.1 (B), i.e., s=a−1r′⋯a−11b1⋯bt′ (a1,…,ar′, b1,…,bt′∈Q1), then there are arrows ar′+1,…,ar, r≥r′, and bt′+1,…,bt, t≥t′ such that
is projective (consider the string corresponded by the indecomposable projective module P(s(b1))=P(s(a1))), where at least one of t−t′ and r−r′ is positive. In this case, s is a subsrting of p, and the sources of s and p equal to t(a−11)(=s(a1))=s(b1) as required.
The case of s belongs to Lemma 3.1 (A) is similar. □
Denoted by ℘(s) the projective string p is given by (3.1). If s is a string with a unique source on (Q,I), then the projective cover of M(s) is an A-homomorphism pM:M(℘(s))≅P(v)→M(s), and v is the unique source of ℘(s). Indeed, the string module M(℘(s)) corresponded by ℘(s)=a−1r⋯a−1r′+1sbt′+1⋯bt is an indecomposable projective module whose top is isomorphic to the simple module S(v), which corresponds to the unique source v of s. Notice that the top of M(s) is isomorphic to S(v), thus pM is of the form M(℘(s))→M(s). Furthermore, the kernel Ker(pM) of pM is a direct sum of at most two direct string modules; see [15, Lemma 2.9] and [20, Lemma 3.4].
3.2. The indecomposable modules over string tree algebras
We call a vertex v of a quiver (tinv,1,toutv,2)-vertex if the number of arrows ending at v is tv,1 and that of arrows starting from v is tv,2. We call a bound (Q,I) is a bound ((≤1)in,(≤2)out)-quiver (resp. bound ((≤2)in,(≤1)out)-quiver) if any vertex v of Q0 is a (tinv,1,toutv,2)-vertex, where tinv,1≤1 (resp. ≤2) and tinv,2≤2 (resp. ≤1). Obviously, if (Q,I) is a string pair, then tinv,1≤2 and toutv,2≤2 hold for all v∈Q0. Furthermore, a string algebra A is said to be a string ((≤1)in,(≤2)out)-algebra (resp. string ((≤2)in,(≤1)out)-algebra) if its bound quiver is a bound ((≤1)in,(≤2)out)-quiver (resp. {bound ((≤2)in,(≤1)out)-quiver}).
Lemma 3.3. If A is a string ((≤1)in,(≤2)out)-algebra, then any indecomposable module is isomorphic to M(s), where s is either a string lying in Lemma 3.1 (A) or a string lying in Lemma 3.1 (B).
Proof. By Theorem 2.3, we show that any string s on (Q,I) lies in either Lemma 3.1 (A) or 3.1 (B) in this proof. Since each vertex v∈Q0 satisfies that tv,1≤1 and tv,2≤2, a string crossing v is one of
If s lies in (a), then all sources of s are on the left of v. Indeed, if there is a source on the right of v, then we can find a vertex, say z, between v and w such that tz,1=2, a contradiction. Furthermore, if s has two sources, say w1 and w2, which are left to v, then s is of the form
It is easy to see that there is a vertex z between w1 and w2 such that tz,1=2, a contradiction. Thus, s has a unique source that is left to v, and, if v=s(s), then s lies in Lemma 3.1 (A); otherwise, s lies in Lemma 3.1 (B). The case of s lies in (b) can be obtained in a dual way.
For the case of s lies (c), we show that v is the unique source of s. Otherwise, there is another source w on the left (resp. right) of v, and then we can find a vertex z between v and w such that tz,1=2, a contradiction. In this case s is a string lying in Lemma 3.1 (B). □
Lemma 3.4. String ((≤1)in,(≤2)out)-algebras are representation-finite.
Proof. Indeed, a string algebra is representation-finite if and only if its bound quiver does not contain bands. This well-known result can be proved by Theorem 2.3 and Brauer–Thrall Theorem.
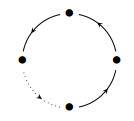
Next, we show that the bound quiver (Q,I) of any string ((≤1)in,(≤2)out)-algebra does not contain bands. First, if Q contains at least one cycle, then all cycles must be oriented cycles, i.e., the subquiver of the following form.
Otherwise, there are two vertex v and w on the cycle such that tinv,1=2 and toutw,2=2. This is a contradiction. In this case, if (Q,I) contains a band b, then it can be seen in the following form:
(note that x and y are ((≤1)in,(≤2)out)-points). If b has a sink v, then v, as a vertex of Q, must be a vertex on the string  lying in the shadow part "
lying in the shadow part " " (x and y are two end points of
" (x and y are two end points of 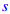 ). In this case, v is a (2in,toutv,2)-vertex, this is a contradiction. □
). In this case, v is a (2in,toutv,2)-vertex, this is a contradiction. □
For any projective string p, we define its vertices pair as the following:
● If p lies in Lemma 3.1 (A), then it is of the form
For any vi, 0≤i≤l, a vertices pair, written as (vi,vi)p, is the triple (p,vi,vi).
● If p lying in Lemma 3.1 (B), then it is of the form
For any xi, yj, where 0≤i≤r, 0≤j≤t, a vertices pair, written as (xi,yj)p, is the triple (p,xi,yi).
In this case, we define (xi,yj)p=(yi,xj)p.
Proposition 3.5. If A is a string ((≤1)in,(≤2)out)-algebra, then there is a bijection
from the set VP(A) of all vertices pairs of projective strings to the set of all isoclasses of indecomposable A-modules.
Proof. By Lemma 3.4, A is representation-finite. Thus, the bijection M given in Theorem 2.3 is a bijection between ind(modA) and Str(A). Then we need to construct a bijection from VP(A) to Str(A) in this proof.
For any projective string p lying in Lemma 3.1 (A), assume that it is of the form given by (3.2). By Lemma 3.2, we define a corresponding ¯ΨA that sends any vertices pair (vi,vi)p, (0≤i≤l), to the direct string a1⋯ai, here, ¯ΨA((vi,vi)p) is a substring of p and the sources of ¯ΨA((vi,vi)p) and p coincide (if i=0, take ¯ΨA((v0,v0)p) the string ev0 of length zero corresponded by the vertex v0).
For any projective string p lying in Lemma 3.1 (B), assume that it is of the form given by (3.3). By Lemma 3.2, we define a corresponding ¯ΨB which sends any vertices pair (xi,yj)p, (0≤i≤r, 0≤j≤t), to the string a−1i⋯a−11b1⋯bj, here, ¯ΨB((xi,yi)p) is a substring of p, and the sources of ¯ΨB((xi,yi)p) and p coincide (if i=0, then ¯ΨB((xi,yj)p)=b1⋯bj is a direct string; the case for j=0 is dual; if i and j are zero, then ¯ΨB((xi,yj)p) is the string ev of length zero corresponded by the vertex v).
Then, by Lemma 3.3, all strings on the string pair (Q,I), a bound ((≤1)in,(≤2)out)-quiver, of A are corresponded by vertices pair by
One can check that ¯Ψ is a bijection. Then, by Theorem 2.3, ˉΨ∘M is a bijection from VP(A) to ind(modA), as required. □
3.3. The countering formula
Now we provide the main result of our paper. In this part, we use ♯S to represent the number of elements of the set S.
First, we show the following corollary:
For any projective string p, we define
The following corollary describes all indecomposable A-modules that are quotients of M(p).
Corollary 3.6. Let p be a projective string on a given bound ((≤1)in,(≤2)out)-quiver (Q,I), and assume that v is the unique source of p. Then the following statements hold:
(1) Ψp is the set of all isoclasses of indecomposable A-modules whose top is isomorphic to the simple A-module S(v) corresponding to v.
(2) If p is a projective string lying in Lemma 3.1 (A), then ♯Ψp=dimkP(v);
(3) If p is a projective string lying in Lemma 3.1 (B), assume that p equals to of the form given by (3.3), then ♯Ψp=(r+1)(t+1).
Proof. (1) is a direct corollary of the formula (3.4) in the proof of Proposition 3.5.
(2) Assume that p is of the form given by (3.2), then Ψp={a1⋯ai∣0≤i≤l}. Thus, ♯Ψp=l+1=dimkM(p)=dimkP(v) (v=s(a1) in this case).
(3) By the definition of a vertices pair of p, we have ♯Ψp=(r+11)(t+11)=(r+1)(t+1) in the case of p lying in Lemma 3.1 (B). □
Let pStr(A) be the set of all equivalent classes of projective strings. Now we provide the main result of our paper.
Theorem 3.7. If A is a string ((≤1)in,(≤2)out)-algebra, then
where Dp,1 and Dp,2 are the direct summands of rad(M(p))(p∈pStr(A) lies in Lemma 3.1 (B)).
Proof. All projective strings can be divided in two parts:
(I) The set pStr(A)I of all equivalent classes of projective strings lying in Lemma 3.1 (A);
(II) The set pStr(A)II of all equivalent classes of projective strings lying in Lemma 3.1 (B).
By Proposition 3.5, we have ♯ind(modA)=♯VP(A). Let VPp(A) be the set of all vertices pairs of p, then
is a disjoint union, see Corollary 3.6 (1), and so,
By Corollary 3.6 (2), we have:
● ♯VPp(A)=dimkM(p) if p is a projective string lying in pStr(A)I;
● Let rp=dimkDp,1 and tp=dimkDp,2. By Corollary 3.6 (3), we have ♯VPp(A)=(rp+1)(tp+1)=dimkM(p)+rptp if p is a projective string
lying in pStr(A)II.
Therefore,
□
Let iStr(A) be the set of all equivalent classes of injection strings. We can prove the following result in a dual way.
Theorem 3.8. If A is a string ((≤2)in,(≤1)out)-algebra, then
where Dp,1 and Dp,2 are the direct summands of M(ı)/sco(M(ı))(ı∈iStr(A) lies in Lemma 3.1 (B′)).
4.
Examples
We provide some examples in this section.
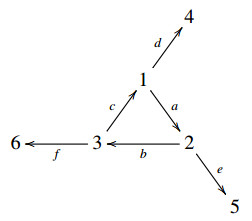
Example 4.1. Let A=kQ/I given by Q=
and I=⟨abc,bca,cab,ae,bf,cd⟩. Then P(1)=(2314), P(2)=(3125), P(3)=(1236), P(4)=S(4)=(4), P(5)=S(5)=(5), and P(6)=S(6)=(6). Furthermore, the Auslander–Reiten quiver of A is shown in Figure 1, and then ♯ind(modA)=21. On the other hand, dimkA=dimk6⨁i=1P(i)=15, and radP(1)=(23)⊕(4), radP(2)=(31)⊕(5) and radP(3)=(12)⊕(6). We obtain
Example 4.2. Take A=kQ/I with Q= 1a1−−−⟶2a2−−−⟶⋯an−1−−−⟶n and I be an arbitrary admissible ideal. Then the number of indecomposable A-modules equals dimkA (up to isomorphism). In particular, if I=radt(kQ) (2≤t≤n), then we have
by Theorem 3.7. In particular, if t=n, then kQ is hereditary; we have ♯ind(modA)=n(n+1)2 in this case.
5.
Conclusions
We obtained the counting formula for indecomposable modules over some string algebras in this paper.
Author contributions
Haicun Wen: Conceptualization, Methodology, Validation, Writing-review and editing, Visualization; Mian-Tao Liu: Methodology, Validation, Writing-original draft preparation; Yu-Zhe Liu: Conceptualization, Methodology, Validation, Writing-review and editing; All authors have read and agreed to the published version of the manuscript.
Use of AI tools declaration
The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.
Acknowledgements
The authors thank the editor and referees for their careful reading and valuable comments. Mian-Tao Liu is supported by CSC 202206190153 and {Postgraduate Research & Practice Innovation Program of Jiangsu Province Grant KYCX24_0123}. Yu-Zhe Liu is supported by the National Natural Science Foundation of China (Grant No. 12171207), Guizhou Provincial Basic Research Program (Natural Science) (Grant No. ZK[2024]YiBan066) and Scientific Research Foundation of Guizhou University (Grant Nos. [2022]53, [2022]65).
Conflict of interest
All authors declare no conflicts of interest in this paper.









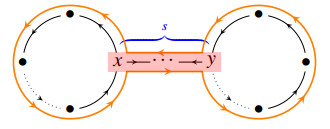
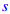 lying in the shadow part "
lying in the shadow part " " (
" (
 DownLoad:
DownLoad:



