On the equiform geometry of special curves in hyperbolic and de Sitter planes

1.   Introduction

Many important results in the theory of the curves in $\mathbb{R}^{3}$ were initiated by G. Monge and G. Darboux pioneered the moving frame idea. Thereafter, Frenet defined his moving frame and his special equations which play important role in mechanics and kinematics as well as in differential geometry. At the beginning of the twentieth century, A. Einstein's theory opened a door to the use of new geometries. One of them, Minkowski space-time, which is simultaneously the geometry of special relativity and the geometry induced on each fixed tangent space of an arbitrary Lorentzian manifold, was introduced and some of classical differential geometry topics have been treated by the researchers. In recent years, the theory of degenerate submanifolds has been treated by researchers and some classical differential geometry topics have been extended to Lorentz manifolds. For instance, in ^[1,2,3,4], the authors extended and studied involute-evolute curves in Minkowski, hyperbolic, and de Sitter spaces.

The involute of a given curve is a well-known concept in Euclidean 3-space $\mathbb{R}^{3}$. It is well-known that, if a curve is differentiable at each point of an open interval, a set of mutually orthogonal unit vectors can be constructed and called Frenet frame or moving frame vectors. The rates of these frame vectors along the curve define the curvatures of the curves. The set, whose elements are frame vectors and curvatures of a curve, is called the Frenet apparatus of the curve. An evolute and its involute, are defined in mutual pairs. The evolute and involute of the curve pair are well known by mathematicians, especially differential geometry scientists (see for example ^[5,6,7]). We can find more motivations for our work from several papers (see ^{[8,9,10,11,12,13,14,15,16,17,18,19]}).

The geometry of space is associated with the mathematical groups. The idea of invariance of geometry under transformation group may imply that on some spacetimes of maximum symmetry, there should be a principle of relativity, which requires the invariance of physical laws without gravity under the transformations among inertial systems.

The equiform geometry of Cayley-Klein space is defined by requesting that the similarity group of the space preserves angles between planes and lines, respectively. Cayley-Klein geometries are studied for many years. However, they recently have become interesting again since their importance for other fields, like soliton theory, have been rediscovered. Although the equiform geometry has minor importance related to the usual one, the curves that appear here in the equiform geometry can be seen as generalizations of well-known curves from the above-mentioned geometries and therefore could have been of research interest. Besides, the theory of curves and the curves of constant curvature in the equiform differential geometry of the isotropic spaces $\mathbb{I}_{3}^{2}$ and $\mathbb{I}_{3}^{1}$ and the Galilean space $\mathbb{G}_{3}$ are described in ^[20,21], respectively.

In this work, we introduce a visualization for the equiform geometry of frontal curves in the hyperbolic and de Sitter planes. Also, we define the equiform geometry of the involute-evolute curve couple in $\mathbb{H}_{0}^{2}$ and $\mathbb{S}_{1}^{2}$.

2.   Basis concepts and geometric meanings

To meet the requirements in the next sections, here, the basic elements of the theory of curves in the hyperbolic and de Sitter planes are briefly presented. We adopt $\mathbb{H}_{0}^{2}$ and $\mathbb{S}_{1}^{2}$ as models of hyperbolic and de Sitter spheres in Minkowski 3-space $\mathbb{E}_{1}^{3}$, respectively. Since $\mathbb{H}_{0}^{2}$ and $\mathbb{S}_{1}^{2}$ are Riemannian manifolds, so the explicit differential geometry of the curves in these spheres is analogous to the differential geometry of the curves in Euclidean space (see for more details ^[3,4,22]).

Let $\mathbb{R}^{3} = \{(x_{1}, x_{2}, x_{3})\mid x_{1}, x_{2}, x_{3}\in \mathbb{R} \}$ be a 3-dimensional vector space, and ${\bf{x}} = (x_{1}, x_{2}, x_{3})$ and ${\bf{y}} = (y_{1}, y_{2}, y_{3})$ two vectors in $\mathbb{R}^{3}$. The pseudo scalar product of ${\bf{x}}$ and ${\bf{y}}$ is defined by $\langle {\bf{x}}, {\bf{y}}\rangle = -x_{1}y_{1}+x_{2}y_{2}+x_{3}y_{3}$. We call $(\mathbb{R}^{3}, \langle, \rangle)$ a 3-dimensional pseudo Euclidean space, or Minkowski 3-space. We write $\mathbb{E}_{1}^{3}$ instead of $(\mathbb{R}^{3}, \langle, \rangle)$ and a vector ${\bf{x}}$ in $\mathbb{E}_{1}^{3}$ is spacelike, lightlike or timelike if $\langle {\bf{x}}, {\bf{x}}\rangle > 0$, $\langle {\bf{x}}, {\bf{x}}\rangle = 0$ or $\langle {\bf{x}}, {\bf{x}}\rangle < 0$, respectively. We define spheres in $\mathbb{E}_{1}^{3}$ as follows:

and we take

where $\mathbb{H}_{0}^{2} = \mathbb{H}_{+}^{2}\cup \mathbb{H}_{-}^{2}$. We call $\mathbb{H}_{0}^{2}$ a hyperbolic sphere and $\mathbb{S}_{1}^{2}$ a de Sitter sphere. For any two vectors ${\bf{x}} = (x_{1}, x_{2}, x_{3})$, ${\bf{y}} = (y_{1}, y_{2}, y_{3})$ of $\mathbb{R}_{1}^{3}$, the vector product is defined by the following.

We call $\boldsymbol{\gamma}_{h} :I\longrightarrow \mathbb{H}^{2}_{0}\subset \mathbb{E}_{1}^{3}; \boldsymbol{\gamma}_{h} (s) = (x_{1}(s), x_{2}(s), x_{3}(s))$ a spacelike frontal curve in $\mathbb{H}^{2}_{0}$ $(i.e., \dot{\boldsymbol{\gamma}}_{h}(s)\neq 0)$ for any $s\in I$, where $I$ is an open interval and $\langle \dot{\boldsymbol{\gamma}}_{h}(s), \dot{\boldsymbol{\gamma}}_{h}(s)\rangle > 0$. Also, we call $(\boldsymbol{\gamma}_{h}, \boldsymbol{\nu}_{\gamma_{h}}):I\longrightarrow \mathbb{H}_{0}^{2}\times\mathbb{S}_{1}^{2}$ a framed curve. If $\boldsymbol{\gamma}_{h}$ is singular at $s_{0}$, we can't define a frame in a traditional way. However, $\boldsymbol{\nu}_{\gamma_{h}}$ always exists even if $s$ is a singular point of $\boldsymbol{\gamma}_{h}$. We consider $\boldsymbol{\mu}_{\gamma_{h}} = \boldsymbol{\nu}_{\gamma_{h}}\wedge\boldsymbol{\gamma}_{h}$ and therefore, the pair $\{\boldsymbol{\gamma}_{h}, \boldsymbol{\nu}_{\gamma_{h}}, \boldsymbol{\mu}_{\gamma_{h}}\}$ is a moving frame of $\boldsymbol{\gamma}_{h}$. Then, the hyperbolic Serret-Frenet formulae are read as follows:

where $\ell(t) = \langle \dot{\boldsymbol{\nu}}_{\gamma_{h}}(s), \boldsymbol{\mu}_{\gamma_{h}}(s)\rangle$, $\boldsymbol{\nu}_{\gamma_{h}}(s)$ and $\boldsymbol{\mu}_{\gamma_{h}}(s)$ are both unit spacelike vectors. We declare that $(\boldsymbol{\gamma_{h}}, -\boldsymbol{\nu}_{\gamma_{h}})$ is also a framed curve. If $(\boldsymbol{\gamma}_{h}, \boldsymbol{\nu}_{\gamma_{h}})$ is a framed immersion, we have $(\beta(s), \ell(s))\neq(0, 0)$ for each $t\in I$. The pair $(\beta, \ell)$ are geodesic curvatures of the framed curve ^[23,24].

Definition 2.1. ^[24] Under the assumption $\ell^{2}(s)\neq\beta^{2}(s)$, the evolute of the frontal curve $\boldsymbol{\gamma}_{h}$ in the hyperbolic plane is expressed as

where $\boldsymbol{\gamma}_{h}$ is the involute curve of $\boldsymbol{\mathcal{E}_{v}}(\boldsymbol{\gamma}_{h})$ in the hyperbolic plane.

Further, let $\boldsymbol{\gamma}_{d}:I\longrightarrow \mathbb{S}^{2}_{1}\subset \mathbb{E}_{1}^{3}; \boldsymbol{\gamma}_{d} (s) = (x_{1}(s), x_{2}(s), x_{3}(s))$ be a timelike frontal curve in $\mathbb{S}^{2}_{1}$ $(i.e., \dot{\boldsymbol{\gamma}}_{d}(s)\neq 0)$ for any $s\in I$, where $I$ is an open interval. It is easy to show that $\langle \dot{\boldsymbol{\gamma}}_{d}(s), \dot{\boldsymbol{\gamma}}_{d}(s)\rangle < 0$. Let $(\boldsymbol{\gamma}_{d}, \boldsymbol{\nu}_{\gamma_{d}}):I\longrightarrow \mathbb{S}_{1}^{2}\times\mathbb{S}_{1}^{2}$ be a framed curve. If $\boldsymbol{\gamma}_{d}$ is singular at $s_{0}$, then we can't define a frame in a traditional way. However, $\boldsymbol{\nu}_{\gamma_{d}}$ always exists even $s$ is a singular point of $\boldsymbol{\gamma}_{d}$. Also, we consider $\boldsymbol{\mu}_{\gamma_{d}} = \boldsymbol{\nu}_{\gamma_{d}}\wedge\boldsymbol{\gamma}_{d}$. The pair $\{\boldsymbol{\gamma}_{d}, \boldsymbol{\nu}_{\gamma_{d}}, \boldsymbol{\mu}_{\gamma_{d}}\}$ is a moving frame of $\boldsymbol{\gamma}_{d}$ and the de Sitter Frenet-Serret formulae are expressed as follows:

where $\ell(t) = -\langle \dot{\boldsymbol{\nu}}_{\gamma_{d}}(s), \boldsymbol{\mu}_{\gamma_{d}}(s)\rangle$; $\boldsymbol{\nu}_{\gamma_{d}}(s)$ and $\boldsymbol{\mu}_{\gamma_{d}}(s)$ are both unit timelike vectors. We declare that $(\boldsymbol{\gamma_{d}}, -\boldsymbol{\nu}_{\gamma_{d}})$ is also a framed curve. If $(\boldsymbol{\gamma}_{d}, \boldsymbol{\nu}_{\gamma_{d}})$ is a framed immersion, then we have $(\beta(s), \ell(s))\neq(0, 0)$ for each $t\in I$, such that the pair $(\beta, \ell)$ are geodesic curvatures of the framed curve^[25,26].

Definition 2.2. Under the assumption $\ell^{2}(s)\neq-\beta^{2}(s)$, the evolute of the frontal curve $\boldsymbol{\gamma}_{d}$ in de Sitter plane is expressed as

where $\boldsymbol{\gamma}_{d}$ is the involute curve of $\boldsymbol{\mathcal{E}_{v}}(\boldsymbol{\gamma}_{d})$ in the hyperbolic plane.

Throughout this work, we assume that the pairs $(\boldsymbol{\gamma}_{h}, \boldsymbol{\nu}_{\gamma_{h}})$ and $(\boldsymbol{\gamma}_{d}, \boldsymbol{\nu}_{\gamma_{d}})$ are co-orientable, and the singular points of $\boldsymbol{\gamma}_{h}$ and $\boldsymbol{\gamma}_{d}$ are finite. Also, the derivative with respect to the arc length $s$ is denoted by (dot) and to the other parameters by (prime).

3.   Equiform geometry of frontal curves in hyperbolic plane

Let $\boldsymbol{\gamma}_{h}(s) :I\rightarrow \mathbb{H}_{0}^{2}(-1)$ be a frontal curve in a hyperbolic plane. We define the equiform parameter of $\boldsymbol{\gamma}_{h}$ as

where $\rho = \frac{\beta}{\ell}$, is the radius of curvature of the frontal curve $\boldsymbol{\gamma}_{h}$ ^[27,28,29].

From Eq (5), we get

Let $\mathcal{H}$ is a homothety with a center at the origin and $\varepsilon$ as a coefficient. So, if we put $\boldsymbol{\gamma}_{h}^{\ast } = \mathcal{H}\left(\boldsymbol{\gamma}_{h}\right),$ then

where $s^{\ast }$ is the arc length parameter of $\boldsymbol{\gamma}_{h} ^{\ast }$ and $\beta^{\ast }/\ell^{\ast}$ is the radius of curvature of $\boldsymbol{\gamma}_{h} ^{\ast }$. Hence, $\sigma$ is an equiform invariant parameter of $\boldsymbol{\gamma}_{h}$. Let $\ell/\beta$ be not an invariant of the homothety group, then $\frac{\ell^{\ast }}{\beta^{\ast}} = \frac{1}{\varepsilon }\frac{\ell}{\beta}$. If we take ${\bf T}_{h}(\sigma) = d\boldsymbol{\gamma}_{h}(s)/d\sigma$ as a tangent vector of $\boldsymbol{\gamma}_{h}$ in the equiform geometry of $\mathbb{H}_{0}^{2}$, therefore we get

Furthermore, we define the vector ${\bf N}_{h}$ as

It is easy to see that $\left\{ \boldsymbol{\gamma}_{h}, {\bf T}_{h}, {\bf N}_{h}\right\}$ is an equiform invariant trihedron of the curve $\boldsymbol{\gamma}_{h}$. The derivatives of these vectors with respect to $\sigma$ are given as follows:

and

Let $\mathcal{K}_{h}:I\rightarrow \mathbb{R}$ be a function defined by $\mathcal{K}_{h} = \frac{d}{ds}(\beta^{2}/\ell)$ is called the equiform curvature of the curve $\boldsymbol{\gamma}_{h}$. Then, the analogous formulae to Frenet formulae in the equiform geometry of the hyperbolic plane have the following form

and they can be written in a matrix form as follows:

Therefore, according to the equiform Frenet formulae (9), the equiform curvature of the curve $\boldsymbol{\gamma}_{h}$ is given by

3.1.   Equiform geometry of height function in hyperbolic plane

In this section, we introduce the families of functions on a curve $\boldsymbol{\gamma}_{h}(\sigma) :I\rightarrow \mathbb{H}^{2}_{0}$. We call $\mathcal{H}^{h}$ the hyperbolic height function of the curve $\boldsymbol{\gamma}_{h}(\sigma)$ in $\mathbb{H}_{0}^{2}$.

Theorem 3.1. Assume that $\mathcal{H}^{h}(\sigma):I\wedge\mathbb{H}^{2}(-1)\rightarrow R$; $(\sigma, \boldsymbol{u})\rightarrow (\boldsymbol{\gamma}_{h}(\sigma), \boldsymbol{u})$, and $\lVert {\bf T}_{h}^{\prime }(\sigma)\rVert\neq0$, then the equation of the evolute curve in the equiform geometry is given by:

where $\ell^{2}\neq\beta^{2}$.

Proof. With the aid of Frenet formulae (9), we obtain

since $\boldsymbol{u}\in \mathbb{H}_{0}^{2}$, there are $\lambda, \mu\in \mathbb{R}$ such that $\boldsymbol{u} = \lambda \boldsymbol{\gamma}_{h}(\sigma)+\mu {\bf N}_{h}(\sigma)$, therefore $\langle \boldsymbol{u}, \boldsymbol{u}\rangle = -1$, hence $-\lambda^{2}+\mu^{2}\left(\frac{\beta^{4}}{\ell^{2}} \right) = -1$, and if $\dfrac{\partial\mathcal{H}^{h}}{\partial\sigma} = 0$, then

which implies $\lambda = -\beta\mu$, therefore $\partial\mathcal{H}^{h}/\partial\sigma = \partial^{2}\mathcal{H}^{h}/\partial\sigma^{2} = 0$, if and only if $\boldsymbol{u} = \lambda \boldsymbol{\gamma}_{h}(\sigma)+\mu {\bf N}_{h}(\sigma)$, $-\lambda^{2}+\mu^{2}\left(\frac{\beta^{4}}{\ell^{2}} \right) = -1$ and $\lambda = -\beta\mu$, it leads to

Under the condition $\ell^{2}\neq\beta^{2}$, we have

it follows that the evolute curve in equiform geometry of the hyperbolic plane is given by

Thus, it completes the proof. □

4.   Equiform geometry of frontal curves in de Sitter plane

Let $\boldsymbol{\gamma}_{d}(s) :I\rightarrow \mathbb{S}_{1}^{2}$ be a frontal curve in de Sitter plane. The equiform parameter of $\boldsymbol{\gamma}_{d}$ is expressed as

where $\rho = \frac{\beta}{\ell}$, is the radius of curvature of the frontal curve $\boldsymbol{\gamma}_{d}$. From which, we get

Let $\mathcal{F}$ be a homothety with the center at the origin and a coefficient $\bar{\varepsilon}$, so if we put $\bar{\boldsymbol{\gamma}}_{d} = \mathcal{F}\left(\boldsymbol{\gamma}_{d}\right),$ then

where $\bar{s}$ is the arc length parameter of $\bar{\boldsymbol{\gamma}}_{d}$ and $\bar{\beta}/\bar{\ell}$ is the radius of curvature of $\bar{\boldsymbol{\gamma}}_{d}$. Hence, $\sigma$ is an equiform invariant parameter of $\boldsymbol{\gamma}_{d}$. Also, let $\ell/\beta$ be not an invariant of the homothety group, then $\frac{\bar{\ell}}{\bar{\beta}} = \frac{1}{\bar{\varepsilon} }\frac{\ell}{\beta}$. If we take ${\bf T}_{d} = d\boldsymbol{\gamma}_{d}(s)/d\sigma$ as a tangent vector of $\boldsymbol{\gamma}_{d}$ in the equiform geometry of $\mathbb{S}_{1}^{2}$, then we get

and we define the vector ${\bf N}_{d}$ as follows

It is easy to check that the trihedron $\left\{ \boldsymbol{\gamma}_{d}, {\bf T}_{d}, {\bf N}_{d}\right\}$ is an equiform invariant trihedron of the curve $\boldsymbol{\gamma}_{d}$.

Now, we find the derivatives of these vectors with respect to $\sigma$. So, from Eqs (12)–(14), we find

also, we get

Let $\mathcal{K}_{d}:I\rightarrow \mathbb{R}$ be a function defined by $\mathcal{K}_{d} = d/ds(\beta^{2}/\ell)$ is called the equiform curvature of the curve $\boldsymbol{\gamma}_{d}$. The formulae analogous to Frenet formulae in the equiform geometry of de Sitter plane are read as follows:

and in the matrix form are

Hence, according to the equiform Frenet formulae (15), the equiform curvature of the curve $\boldsymbol{\gamma}_{d}$ is given by

4.1.   Equiform geometry of height function in de Sitter plane

Here, we introduce families of functions on the curve $\boldsymbol{\gamma}_{d}(\sigma) :I\rightarrow \mathbb{S}_{1}^{2}$. We call $\mathcal{H}^{d}$ the de Sitter height function of the curve $\boldsymbol{\gamma}_{d}(\sigma)$ in $\mathbb{S}_{1}^{2}$.

Theorem 4.1. Let $\mathcal{H}^{d}(\sigma):I\wedge\mathbb{S}^{2}_{1}\rightarrow R$; $(\sigma, \boldsymbol{v})\rightarrow (\boldsymbol{\gamma}_{d}(\sigma), \boldsymbol{v})$, and suppose that $\lVert {\bf T}_{d}^{\prime }(\sigma)\rVert\neq0$, then the equation of the evolute curve in the equiform geometry is given by

where $\ell^{2}\neq\beta^{2}$.

Proof. By using Frenet formulae (15), we get

since $\boldsymbol{v}\in \mathbb{S}_{1}^{2}$, there are $\lambda, \mu\in \mathbb{R}$ such that $\boldsymbol{v} = \lambda \boldsymbol{\gamma}_{d}(\sigma)+\mu {\bf N}_{d}(\sigma)$, therefore $\langle \boldsymbol{v}, \boldsymbol{v}\rangle = 1$. Hence, $-\lambda^{2}+\mu^{2}\left(\frac{\beta^{4}}{\ell^{2}} \right) = 1$, if $\dfrac{\partial\mathcal{H}^{d}}{\partial\sigma} = 0$, then

which implies $\lambda = \beta\mu$, therefore $\partial\mathcal{H}^{d}/\partial\sigma = \partial^{2}\mathcal{H}^{d}/\partial\sigma^{2} = 0$, if and only if $\boldsymbol{v} = \lambda \boldsymbol{\gamma}_{d}(\sigma)+\mu {\bf N}_{d}(\sigma)$, $-\lambda^{2}+\mu^{2}\left(\frac{\beta^{4}}{\ell^{2}} \right) = 1$ and $\lambda = \beta\mu$, which implies that

Under the condition $\ell^{2}\neq\beta^{2}$, we get

it follows that the evolute curve in the equiform geometry of de Sitter plane is expressed as

and thus, the proof is completed. □

5.   Equiform geometry of involute-evolute curve couple in $\mathbb{H}_{0}^{2}$

In this section, we introduce the definitions of front and frontal curves in the hyperbolic plane. Also, we investigate the equiform geometry of the Frenet apparatus of an evolute-involute curve couple ^{[30,31,32,33,34]}.

Definition 5.1. The curve denoted by $(\boldsymbol{\mathcal{E}_{v}}, \boldsymbol{\nu}_{h_{\mathcal{E}_{v}}}):I\longrightarrow \mathbb{H}_{0}^{2}\times\mathbb{S}_{1}^{2}$ is said to be a framed Legendrian curve if $\langle \boldsymbol{\mathcal{E}_{v}}(s), \boldsymbol{\nu}_{h_{\mathcal{E}_{v}}}(s)\rangle = 0$, and $\langle \dot{\boldsymbol{\mathcal{E}_{v}}}(s), \boldsymbol{\nu}_{h_{\mathcal{E}_{v}}}(s)\rangle = 0$ for all $s\in I$, and if $\left(\boldsymbol{\mathcal{E}_{v}}, \boldsymbol{\nu}_{h_{\mathcal{E}_{v}}}\right)$ is an immersion, namely, $\left(\dot{\boldsymbol{\mathcal{E}_{v}}}(s), \dot{\boldsymbol{\nu}}_{h_{\mathcal{E}_{v}}}(s)\right) \neq(0, 0)$, we call $(\boldsymbol{\mathcal{E}_{v}}, \boldsymbol{\nu}_{h_{\mathcal{E}_{v}}})$ a framed immersion Legendrian curve.

Definition 5.2. The curve $\boldsymbol{\mathcal{E}_{v}} :I\longrightarrow \mathbb{H}_{0}^{2}$ is said to be a frontal curve if there exists a smooth mapping $\boldsymbol{\nu}_{h_{\mathcal{E}_{v}}} :I\longrightarrow \mathbb{S}_{1}^{2}$ such that $(\boldsymbol{\mathcal{E}_{v}}, \boldsymbol{\nu}_{h_{\mathcal{E}_{v}}})$ is a framed curve. Also, we say that $\boldsymbol{\mathcal{E}_{v}} :I\longrightarrow \mathbb{H}_{0}^{2}$ is a front curve if there exists a smooth mapping $\boldsymbol{\nu}_{h_{\mathcal{E}_{v}}} :I\longrightarrow \mathbb{S}_{1}^{2}$ such that $(\boldsymbol{\mathcal{E}_{v}}, \boldsymbol{\nu}_{h_{\mathcal{E}_{v}}})$ is a framed immersion Legendrian curve.

Theorem 5.1. Let $\boldsymbol{\gamma}_{h} :I\longrightarrow \mathbb{H}_{0}^{2}$ and $\boldsymbol{\mathcal{E}_{v}} (\boldsymbol{\gamma}_{h}) :I\longrightarrow \mathbb{H}_{0}^{2}$ be unit speed spacelike frontal curves and $\boldsymbol{\mathcal{E}_{v}} (\boldsymbol{\gamma}_{h})$ an evolute of $\boldsymbol{\gamma}_{h}$. The equiform Frenet apparatus of $\boldsymbol{\mathcal{E}_{v}} (\boldsymbol{\gamma}_{h})$ $\left\lbrace \boldsymbol{\mathcal{E}_{v}} (\boldsymbol{\gamma}_{h}); {\bf T}_{h_{\mathcal{E}_{v}} }, {\bf N}_{h_{\mathcal{E}_{v}} }, \mathcal{K}_{h_{\mathcal{E}_{v}}}\right\rbrace$ can be formed according to Frenet apparatus of $\boldsymbol{\gamma}_{h}$ $\left\lbrace \boldsymbol{\gamma}_{h}; \boldsymbol{\mu}_{\gamma_{h}}, \boldsymbol{\nu}_{\gamma_{h}}, (\frac{\ell}{\beta})_{\gamma_{h}}\right\rbrace$.

Proof. From the definition of the evolute frontal curve in $\mathbb{H}_{0}^{2},$ and by differentiating both sides of Eq (2) with respect to $s$, we get

which can be written as

where

and from the relation $\beta_{h_{\mathcal{E}_{v}}}(s) = \lVert\boldsymbol{\dot{\mathcal{E}}_{v}}(s)\rVert$, we find

From Definition 5.2, there exists a smooth mapping $\boldsymbol{\nu}_{h_{\mathcal{E}_{v}}} :I\longrightarrow \mathbb{S}_{1}^{2}$ such that $(\boldsymbol{\mathcal{E}_{v}}, \boldsymbol{\nu}_{h_{\mathcal{E}_{v}}})$ is a framed curve, and we get $\ell_{h_{\mathcal{E}_{v}}}$ from the equation $\ell_{h_{\mathcal{E}_{v}}} = \langle\dot{\boldsymbol{\nu}}_{h_{\mathcal{E}_{v}}}, \boldsymbol{\mu}_{h_{\mathcal{E}_{v}}}\rangle$, where $\left(\beta_{h_{\mathcal{E}_{v}}}, \ell_{h_{\mathcal{E}_{v}}}\right) \neq(0, 0)$.

From Eqs (7), (8), (18), and (20), we obtain the equiform geometry of Frenet apparatus of an evolute curve according to the apparatus of the involute curve as follows:

Hence, the proof is completed. □

6.   Equiform geometry of involute-evolute curve couple in $\mathbb{S}_{1}^{2}$

Now, we introduce the definitions of front and frontal curves in the de Sitter plane. Further, we study the equiform geometry of the Frenet apparatus of an evolute curve according to the Frenet apparatus of an involute curve.

Definition 6.1. The curve $(\boldsymbol{\mathcal{E}_{v}}, \boldsymbol{\nu}_{d_{\mathcal{E}_{v}}}):I\longrightarrow \mathbb{S}_{1}^{2}\times\mathbb{S}_{1}^{2}$ is said to be a framed Legendrian curve, if $\langle \boldsymbol{\mathcal{E}_{v}}(s), \boldsymbol{\nu}_{d_{\mathcal{E}_{v}}}(s)\rangle = 0$, and $\langle \dot{\boldsymbol{\mathcal{E}_{v}}}(s), \boldsymbol{\nu}_{d_{\mathcal{E}_{v}}}(s)\rangle = 0$ for all $s\in I$. Also, if $\left(\boldsymbol{\mathcal{E}_{v}}, \boldsymbol{\nu}_{d_{\mathcal{E}_{v}}}\right)$ is an immersion, namely, $\left(\dot{\boldsymbol{\mathcal{E}_{v}}}(s), \dot{\boldsymbol{\nu}}_{d_{\mathcal{E}_{v}}}(s)\right) \neq(0, 0)$, we call $(\boldsymbol{\mathcal{E}_{v}}, \boldsymbol{\nu}_{d_{\mathcal{E}_{v}}})$ a framed immersion Legendrian curve.

Definition 6.2. The $\boldsymbol{\mathcal{E}_{v}} :I\longrightarrow \mathbb{S}_{1}^{2}$ is a frontal curve if there exists a smooth mapping $\boldsymbol{\nu}_{d_{\mathcal{E}_{v}}} :I\longrightarrow \mathbb{S}_{1}^{2}$ such that $(\boldsymbol{\mathcal{E}_{v}}, \boldsymbol{\nu}_{d_{\mathcal{E}_{v}}})$ is a framed curve. Also, the curve $\boldsymbol{\mathcal{E}_{v}} :I\longrightarrow \mathbb{S}_{1}^{2}$ is said to be a front curve if there exists a smooth mapping $\boldsymbol{\nu}_{d_{\mathcal{E}_{v}}} :I\longrightarrow \mathbb{S}_{1}^{2}$ such that $(\boldsymbol{\mathcal{E}_{v}}, \boldsymbol{\nu}_{d_{\mathcal{E}_{v}}})$ is a framed immersion Legendrian curve.

Theorem 6.1. Let $\boldsymbol{\gamma}_{d} :I\longrightarrow \mathbb{S}_{1}^{2}$ and $\boldsymbol{\mathcal{E}_{v}} (\boldsymbol{\gamma}_{d}) :I\longrightarrow \mathbb{S}_{1}^{2}$ be unit speed spacelike frontal curves and $\boldsymbol{\mathcal{E}_{v}} (\boldsymbol{\gamma}_{d})$ an evolute of $\boldsymbol{\gamma}_{d}$. Then, the equiform Frenet apparatus of $\boldsymbol{\mathcal{E}_{v}} (\boldsymbol{\gamma}_{d})$ $\left\lbrace \boldsymbol{\mathcal{E}_{v}} (\boldsymbol{\gamma}_{d}); {\bf T}_{d_{\mathcal{E}_{v}} }, {\bf N}_{d_{\mathcal{E}_{v}} }, \mathcal{K}_{d_{\mathcal{E}_{v}}}\right\rbrace$ can be formed according to Frenet apparatus of $\boldsymbol{\gamma}_{d}$ $\left\lbrace \boldsymbol{\gamma}_{d}; \boldsymbol{\mu}_{\gamma_{d}}, \boldsymbol{\nu}_{\gamma_{d}}, (\frac{\ell}{\beta})_{\gamma_{d}}\right\rbrace$.

Proof. From the definition of the evolute frontal curve in $\mathbb{S}_{1}^{2}$, and by differentiating both sides of Eq (4) with respect to $s$, we get

which can be written as

where

Further, from the relation $\beta_{d_{\mathcal{E}_{v}}}(s) = \lVert\boldsymbol{\dot{\mathcal{E}}_{v}}(s)\rVert$, we obtain

From Definition 6.2, there exists a smooth mapping $\boldsymbol{\nu}_{d_{\mathcal{E}_{v}}} :I\longrightarrow \mathbb{S}_{1}^{2}$, such that $(\boldsymbol{\mathcal{E}_{v}}, \boldsymbol{\nu}_{d_{\mathcal{E}_{v}}})$ is a framed curve, and then we get $\ell_{d_{\mathcal{E}_{v}}}$ from the equation $\ell_{d_{\mathcal{E}_{v}}} = \langle\dot{\boldsymbol{\nu}}_{d_{\mathcal{E}_{v}}}, \boldsymbol{\mu}_{d_{\mathcal{E}_{v}}}\rangle$, where $\left(\beta_{d_{\mathcal{E}_{v}}}, \ell_{d_{\mathcal{E}_{v}}}\right) \neq(0, 0)$.

Also, from Eqs (13), (14), and (21), we find the equiform geometry of Frenet apparatus of an evolute curve according to the apparatus of the involute curve as follows:

Hence, this completes the proof. □

7.   Computational examples

Finally, in what follows we give two illustrative examples for the frontal curves and obtain their equiform differential geometric properties in the hyperbolic and de Sitter planes.

Example 7.1. Consider the hyperbolic astroid curve $\boldsymbol{\gamma}_{h}:I\longrightarrow \mathbb{H}_{0}^{2}$, parameterized by

therefore, we get

It is obvious that $\boldsymbol{\gamma}_{h}$ is a singular curve at $t = 0$, $\pi/2$, $\pi$ and $3\pi/2$.

If we take ${\mathit{\boldsymbol{\nu }}_{{\mathit{\boldsymbol{\gamma }}_h}}} = ({\mathit{\boldsymbol{\nu }}_{\bf{1}}}_{{\mathit{\boldsymbol{\gamma }}_h}},{\mathit{\boldsymbol{\nu }}_{\bf{2}}}_{{\mathit{\boldsymbol{\gamma }}_h}},{\mathit{\boldsymbol{\nu }}_{\bf{3}}}_{{\mathit{\boldsymbol{\gamma }}_h}})$, where

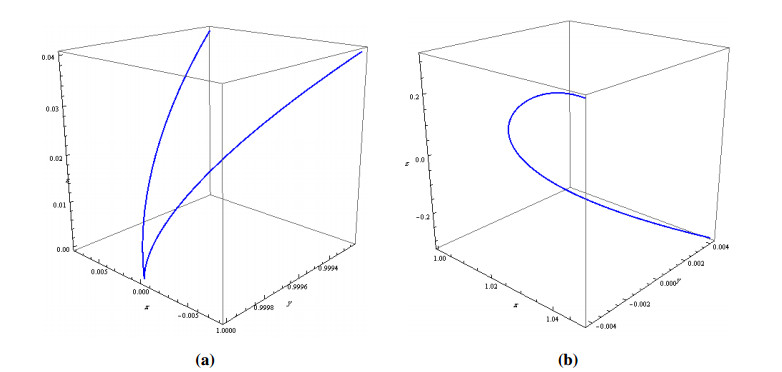
such that $\mathcal{Q}_{1}(t) = \sqrt{ 1+\sin^{2}(t)\cos^{2}(t)}$, then by a straightforward calculation, we obtain $\langle\boldsymbol{\gamma}_{h} (t), \boldsymbol{\nu}_{\boldsymbol{\gamma}_{h}}(t)\rangle = \langle\boldsymbol{\gamma}_{h} ^{\prime}(t), \boldsymbol{\nu}_{\boldsymbol{\gamma}_{h}}(t)\rangle = 0$ and $\langle\boldsymbol{\nu}_{\boldsymbol{\gamma}_{h}}(t), \boldsymbol{\nu}_{\boldsymbol{\gamma}_{h}}(t)\rangle = 1$. Hence, $(\boldsymbol{\gamma}_{h}, \boldsymbol{\nu}_{\boldsymbol{\gamma}_{h}})$ is a framed curve (see Figures 1a and 1b).

Thereafter, from the relation $\boldsymbol{\mu}_{\boldsymbol{\gamma}_{h}} = \boldsymbol{\nu}_{\boldsymbol{\gamma}_{h}}\wedge\boldsymbol{\gamma}_{h}$, we find

and then, we get

it follows that $\langle \boldsymbol{\mu}_{\boldsymbol{\gamma}_{h}}(t), \boldsymbol{\mu}_{\boldsymbol{\gamma}_{h}}(t)\rangle = 1$. So, we obtain

Also, from Eq (25), we have

thus, we get

Furthermore, we have $(\beta(0), \ell(0))\neq(0, 0)$, and hence $\boldsymbol{\gamma}_{h}$ is a frontal curve.

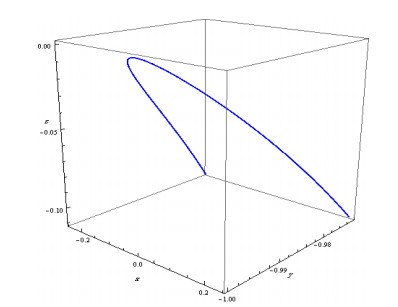
Moreover, from Eqs (2), (24), (25), (27), and (28), we obtain the evolute curve $\boldsymbol{\mathcal{E}_{v}}(t)$ (see Figure 2) as follows: $\boldsymbol{\mathcal{E}_{v}}(t) = \left(\boldsymbol{\mathcal{E}_{v_{1}}}, \boldsymbol{\mathcal{E}_{v_{2}}}, \boldsymbol{\mathcal{E}_{v_{3}}} \right)$, where

such that

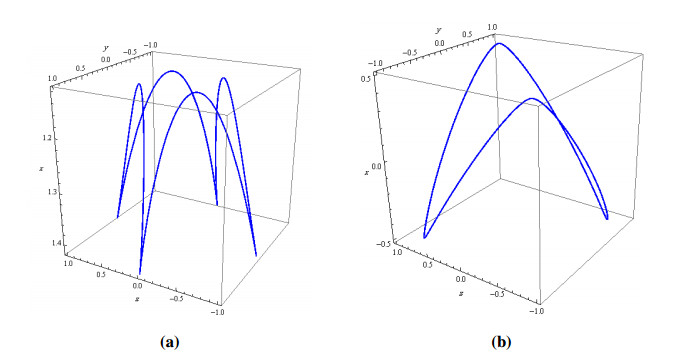
Also, from Eqs (7), (8) and (29), we obtain the equiform geometry of Frenet apparatus of the curve $\boldsymbol{\gamma}_{h}$ in the hyperbolic plane as follows:

and ${\bf N}_{h}(\sigma) = ({\bf N}_{h_{1}}, {\bf N}_{h_{2}}, {\bf N}_{h_{3}})$, where

such that

Example 7.2. We assume that the curve $\boldsymbol{\gamma}_{d} :I\longrightarrow \mathbb{S}_{1}^{2}$ is given by

where $\boldsymbol{\gamma}_{d}$ is a singular curve at $t = 0$. If we take $\boldsymbol{\nu}_{\boldsymbol{\gamma}_{d}} = ({\boldsymbol{\nu_{1}}}_{\boldsymbol{\gamma}_{d}}, {\boldsymbol{\nu_{2}}}_{\boldsymbol{\gamma}_{d}}, {\boldsymbol{\nu_{3}}}_{\boldsymbol{\gamma}_{d}})$, where

and $\mathcal{P}_{1} = \sqrt{\left| 9t^{2}-4\cosh^{2}(t^{3})\right| }$.

Then, we obtain $\langle\boldsymbol{\gamma}_{d}(t), \boldsymbol{\nu}_{\boldsymbol{\gamma}_{d}}(t)\rangle = \langle\boldsymbol{\gamma}_{d}^{\prime}(t), \boldsymbol{\nu}_{\boldsymbol{\gamma}_{d}}(t)\rangle = 0$ and $\langle\boldsymbol{\nu}_{\boldsymbol{\gamma}_{d}}(t), \boldsymbol{\nu}_{\boldsymbol{\gamma}_{d}}(t)\rangle = 1$. Therefore, $(\boldsymbol{\gamma}_{d}, \boldsymbol{\nu}_{\boldsymbol{\gamma}_{d}})$ is a framed curve (see Figures 3a and 3b).

Now, from the relation $\boldsymbol{\mu}_{\boldsymbol{\gamma}_{d}} = \boldsymbol{\nu}_{\boldsymbol{\gamma}_{d}}\wedge\boldsymbol{\gamma}_{d}$, we find

it follows that

where $\langle \boldsymbol{\mu}_{\boldsymbol{\gamma}_{d}}(t), \boldsymbol{\mu}_{\boldsymbol{\gamma}_{d}}(t)\rangle = 1$, so we get

Further, from Eq (31), we have

which leads to

it follows that $(\beta(0), \ell(0))\neq(0, 0)$, and then $\boldsymbol{\gamma}_{d}$ is a frontal curve.

Also, from Eqs (4), (30), (31), (33), and (34), we obtain the evolute curve $\boldsymbol{\mathcal{E}_{v}}(t)$ (see Figure 4) as follows: $\boldsymbol{\mathcal{E}_{v}}(t) = \left(\boldsymbol{\mathcal{E}_{v_{1}}}, \boldsymbol{\mathcal{E}_{v_{2}}}, \boldsymbol{\mathcal{E}_{v_{3}}} \right)$, where

such that $\mathcal{P}_{2} = -18t^{3}\sinh(t^{3})+4t\sinh(t^{3})\cosh(t^{3})+3\cosh(t^{3})$.

Now, from Eqs (13), (14), (31), (32), (33), and (34), we get the equiform geometry of Frenet apparatus of the curve $\boldsymbol{\gamma}_{d}$ in the de Sitter plane as under:

where

and ${\bf N}_{d}(\sigma) = ({\bf N}_{d_{1}}, {\bf N}_{d_{2}}, {\bf N}_{d_{3}})$, such that

also, we obtain

8.   Conclusions

The equiform differential geometric properties of the evolute and involute frontal curves in the hyperbolic and de Sitter planes have been studied. We have introduced the relevance between evolute and involute frontal curves that relate to symmetry properties. Also, under the viewpoint of symmetry, these notions to the frontal curves have been expanded. Furthermore, we have looked at the classification of these curves and introduce the notion of frontalisation for its singularities. Finally, two numerical examples through which we authenticate our theoretical results are given and plotted.

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgments

The authors gratefully acknowledge the approval and support of this research study by the grant no SCAR-2022-11-1780 from the Deanship of Scientific Research at Northern Border University, Arar, K.S.A.

Conflict of interest

The authors declare no conflict of interest.