Analysis study on multi-order $\varrho$-Hilfer fractional pantograph implicit differential equation on unbounded domains

1.   Introduction and objectives

Fractional calculus expands upon traditional calculus by extending the concept of integer order to any real order. This leads to a variety of definitions for integrals and derivatives within the field of fractional calculus. The Caputo and Riemann-Liouville (R-L) ^[1] are considered the most popular among of the fractional operators. Lately, Hilfer ^[2] has linked the Caputo and R-L derivatives by so-call Hilfer or generalized R-L derivatives, which has attracted the attention of many authors in the literature, such as an equivalent form of the Hilfer derivative derived by Kamocki ^[3]. Authors in ^[4], conducted research on the Ulam stability and existence results for Hadamard-Hilfer differential equations. Bulavatsky ^[5] proved the closed solutions for the anomalous diffusion equation in the sense of Hilfer fractional derivative. Thabet et al. ^[6] established existence, uniqueness and continuous dependence of $\epsilon$-approximate solutions for an abstract Hilfer fractional integrodifferential equation via technique of measure of noncompactness and generalized Gronwall's inequality. More recently, ^[7] presented the Hilfer fractional derivatives of a function relative to another function $\varrho$, which is called $\varrho$-Hilfer fractional derivatives. In 2020, by utilizing Schaefer, Banach and Schauder theorems helped generalize Gronwall's inequality and the sufficient conditions of $\mathcal{UH}$ stability and the existence and uniqueness of solutions for $\psi$-Hilfer fractional integrodifferential equations investigated by Abdo et al, ^[8]. Furthermore, the fixed point topic has a large popularity in mathematics areas and may be considered a kernel of nonlinear analysis. It is used to study many mathematical, physical and real phenomena problems, we refer the readers to these references ^{[9,10,11,12,13,14,15,16,17]}. In fact, the existence, uniqueness and $\mathcal{UH}$ stability is an ideal approaches to deal with nonlinear fractional differential equations, for example see ^{[18,19,20,21,22,23,24,25,26,27,28,29,30,31,32]}.

The pantograph is a tool utilized in trains of electricity in order to congregates electric current from the overload lines. Indeed, pantograph equation has an essential part in physics, applied and pure mathematics, such as electrodynamics, control systems, quantum mechanics, probability and number theory. Ockendon and Tayler ^[33], modeled the pantograph differential equations which is a particular type of delay differential equation and is defined by the form:

Recently, the pantograph and implicit equations have attracted increasing interesting, for example see the papers ^{[34,35,36,37,38,39,40,41,42,43,44,45,46]} and the references cited within them. In particular, the authors ^[11], studied the existence, uniqueness and stability of the solution for the following $\varrho$-Hilfer implicit differential equation via a bounded interval $[a, T]$:

Thabet et al. ^[47], investigated the existence criteria of solutions for three-point Caputo conformable fractional pantograph differential inclusion, given by

where ${}^{ CC }\mathfrak{D}_a^{\upsilon, \varrho }$ is Caputo conformable fractional derivative and ${\mathfrak{G}} : [a, T]\times \mathbb{R} \times \mathbb{R} \to \mathcal{P}(\mathbb{R})$ is a set-valued map. They studied also the Snap system ^[48].

In 2021, the existence and uniqueness results for the following Hilfer-Katugampola fractional pantograph implicit differential equation studied by Almalahi et al. ^[49]:

Furthermore, in 2021, the authors of these works ^[50,51] established sufficient conditions of the existence and uniqueness solution and discussed various types of $\mathcal UH$ stability for initial $\varrho-$ Hilfer fractional integro-differential equations. Very recently, Xie et al. ^[52], investigated some qualitative properties of multi-order differential equations with initial condition involving R-L fractional derivatives of the form:

Motivated by the above mentioned research papers, this paper aims to study the existence and uniqueness solution as well as $\mathcal UH$, $\mathcal UHR$ and s-$\mathcal UHR$ stability for the following multi-order $\varrho$-Hilfer fractional pantograph implicit differential equation on unbounded domains:

where $p_1 < p_2 < \dots < p_n, q_1 < q_2 < \dots < q_n, \; c_i, a_0, a_1\in\mathbb{R}, (i = 1, 2, \dots, n-1), n\in \mathbb{N},$ ${}^{ H}\mathfrak{D}^{p_n, q_n; \varrho}_{a^+}, {}^{ H}\mathfrak{D}^{p_i, q_i; \varrho}_{a^+}, {}^{ H}\mathfrak{D}^{\mu, \nu; \varrho}_{a^+},$ are the $\varrho$-Hilfer fractional derivatives of order $p_n, p_i\in (1, 2], \mu\in (0, 1]$ and types $q_n, q_i, \nu\in[0, 1]$, respectively, such that $\mu < p_i, p_i+\mu\leq p_n,$ $\mathfrak{I}^{x; \varrho}_{a^+}$ is $\varrho-$R-L fractional integrals of order $x = \{1-\rho_n, 2-\rho_n\}, \; where\; p_n\leq\rho_n = p_n+2q_n-p_n q_n,$ and a function $g : I\times \Upsilon\times \Upsilon\times\Upsilon \rightarrow \Upsilon$ is continuous in the real Banach space $\Upsilon$.

Throughout this paper, $\mathcal{C}(I, \Upsilon)$ denotes to the Banach space of all continuous functions from $I$ to $\Upsilon,$ which is gifted by the norm $\|{Q}\, \|_\Upsilon = \sup\limits_{ u\in I}\|{Q}\, (u)\|.$ For an appropriate analysis, we define the following an applicable basic Banach space:

equipped with the following norm:

where $\sigma:I\to (0, \infty)$ is an increasing, non-negative and continuous function. Similar to the procedures in these works ^[53,54,55], it can easily be proven that $(\Pi, \|\cdot\|_\Pi)$ is Banach space.

The contributions and novelty of this paper are to study, for the first time, the existence, uniqueness solution as well as $\mathcal UH$, $\mathcal UHR$ and s-$\mathcal UHR$ stability for the problem described in equation (1.1) on unbounded domains $(a, \infty), a\geq0$ in a special applicable Banach space $\Pi$. Additionally, the multi-order fractional problem (1.1) is considering a more general problem and includes many of cases. In particular, it reduces to the sense of Hilfer derivatives ^[2] for $\varrho (u) = u$; Hilfer-Katugampola derivatives ^[56] for $\varrho (u) = u^{r }, r > 0;$ Hilfer-Hadamard derivatives ^[57] for $\varrho (u) = \log u$; $\varrho-$R-L derivatives ^[1] for $q _{n}, q _{i}, \nu\rightarrow 0;$ $\varrho-$Caputo derivatives ^[58] for $q _{n}, q _{i}, \nu\rightarrow 1$; R-L derivatives ^[1] for $\varrho (u) = u,$ $q _{n}, q _{i}, \nu\rightarrow 0$; Caputo derivatives ^[1] for $\varrho (u) = u,$ $q _{n}, q _{i}, \nu\rightarrow 1$; and integer order derivatives for $\varrho (u) = u,$ $p _{n}, p _{i}, \mu\rightarrow n\in\mathbb N, q _{n}, q _{i}, \nu\rightarrow 1$. Furthermore, worth mentioning that our approach in this paper is different about used in the work ^[49]. Also, those works ^[50,51] considered integro-differential problems at one initial boundary conditions and fractional order belongs to $(0, 1]$, and the work ^[52] studied implicit problem in the sense of R-L fractional derivatives of order $p_n\in(0, 1]$, while this work consider the delay and implicit problem (1.1) in the framework of more general $\varrho$-Hilfer fractional derivatives of order $p_n, p_i\in (1, 2]$ with two boundary conditions.

The rest of this paper is arranged as follows: In Section 2, we will review some definitions and basic concepts. In Section 3, we prove the existence and uniqueness solution of the multi-order fractional problem (1.1), by utilizing Banach fixed point theorem in an applicable Banach space. In Section 4, we introduce various types of $\mathcal{UH}$ stability by using the nonlinear analysis topics. At the last, an example is given to showcase our main outcomes.

2.   Preliminaries

Throughout this section, we present some interesting preliminaries, in order to use them in achieving the desired results.

Definition 2.1. (see ^[1]) Let $g$ be an integrable function on $J = [a, b],$ and $\varrho \in C^{1}(J)$ be a non-decreasing function with $\varrho ^{\prime }(u)\neq 0$ for all $u\in J.$ Then, the R-L fractional integral of $g$ of order $\vartheta_{1} > 0,$ with respect to another function $\varrho$ is given by

where $\Gamma (\cdot)$ is the Euler Gamma function and $a\in \mathbb{R}$.

Definition 2.2. (see ^[1]) The R-L fractional derivative of a function $g$ of order $\vartheta _{1}\in (n-1, n]$ with respect to another increasing and integrable function $\varrho \in C^{n}(J)$ with $\varrho ^{\prime }(u)\neq 0, \forall u\in J$, is defined by

such that $n = [\vartheta _{1}]+1$ and $[\vartheta _{1}]$ is the integer part of $\vartheta _{1}.$

Lemma 2.1. (^[1]) Let $\zeta > 0$. Then

and

Definition 2.3. (^[7]) The $\varrho$-Hilfer fractional derivative of a function $g$ of order $\vartheta _{1}\in(n-1, n]$ and type $\vartheta _{2}\in[0, 1],$ with respect to another function $\varrho \in C^{n}(J)$ with $\varrho ^{\prime }(u)\neq 0$ for all $u\in J,$ is given by

Moreover, the operator ${}^{ H}\mathfrak{D}_{a^{+}}^{\vartheta _{1}, \vartheta _{2};\varrho},$ can be written as

Lemma 2.1. (^[1,7]) If $\vartheta _{1}\in(n-1, n]$, $\vartheta_2 \in[0, 1], 0\leq\vartheta < 1, \vartheta = \vartheta _{1}+n\vartheta _{2}-\vartheta _{1}\vartheta _{2}$ and $g^n\in C(J)$, $\mathfrak I_{a^{+}}^{n-\vartheta; \varrho }g\in C^n(J)$, then

Theorem 2.1. (^[59]) Assume that the generalized complete metric space denoted by $(\Xi, \mathfrak{d}),$ and let the operator $\Gamma:\Xi\to\Xi$ is contractive with the Lipschitz constant $\ell < 1$. If there is a positive integer $r$, where $\mathfrak{d}(\Gamma^{r+1}u, \Gamma^{r}u) < \infty,$ for some $u\in\Xi$. Then the following hold:

(i) The sequence $\{\Gamma^r\}$ converges to a fixed point $u_0$ of $\Xi$;

(ii) $u_0$ is the unique fixed point of $\Gamma$ in $\Xi^* = \{v\in\Xi| \mathfrak{d}(\Gamma^{r}u, v) < \infty\}$;

(iii) if $v\in\Xi^*,$ then $\mathfrak{d}(v, u_0)\leq \dfrac{1}{1-\ell} \mathfrak{d}(\Gamma v, v).$

3.   Existence and uniqueness results

At the beginning of this section, we derive the factional integral equation which is equivalent to the multi-order fractional problem specified in (1.1) as follows:

Lemma 3.1. Let a function ${Q}\,$ be continuously differentiable. Then, the solution of the multi-order fractional problem specified in (1.1) is equivalent to the Volterra factional integral equation:

Proof. By applying $\mathfrak{I}^{p_n; \varrho}_{a^+}$ on both sides of (1.1), then by using Lemma 2.1 with boundary conditions, we get

Hence, the proof is completed.

In the following, using Banach fixed point theorems, we establish the existence and uniqueness of a solution for the multi-order fractional problem defined in Eq (1.1).

Theorem 3.1. Let the following assumptions are fulfilled:

$(A_1)$ Suppose that $x_j(\cdot)\geq0, (j = 1, 2)$ are continuous functions and the continuously differentiable function $g:I\times\Pi\times\Pi\times\Pi\to\Pi$ such that

far all ${Q}\, _j, \bar{{Q}\, }_j \in\Pi, (j = 1, 2, 3)$ and $u\in I.$

$(A_2)$ There exist the constants $Q, L > 0,$ such that $Q < 1,$ which are verifying the following requirements:

where $\eta = p_n \; or\; p_n-\mu, \; and\; \bar{\eta} = \rho_n \; or\; \rho_n-\mu.$

Then, the multi-order fractional problem specified in (1.1) has one and only one solution on unbounded interval $I$.

Proof. Due to Lemma 3.1, we consider the map $\Xi:\Pi\to\Pi$ given by:

By using $(A_1)$ and $(A_2)$, we have

Also, by helping of Eq (2.1), we obtain

Next, we investigate that $\Xi$ is contractive operator on $\Pi$. By using $(A_1)$ and $(A_2)$, for any ${Q}\, , \bar{{Q}\, }\in\Pi,$ we get

Similarly,

Hence, we deduce that $\|\Xi{Q}\, -\Xi\bar{{Q}\, }\|_{\Pi}\leq Q \|{Q}\, -\bar{{Q}\, }\|_{\Pi},$ which implies that $\Xi$ is a contractive mapping, since $Q\in(0, 1).$ In the light of Banach fixed point theorem, $\Xi$ has an one and only one fixed point ${Q}\, _0$ in $\Xi,$ which is verifying $\Xi{Q}\, _0 = {Q}\, _0.$ Therefore, the problem specified in (1.1) has an one and only one solution on unbounded interval $(a, \infty)$.

4.   Stability results

In this section, we discuss $\mathcal UHR$ Stability, $\mathcal UH$ Stability and s-$\mathcal UHR$ Stability. Regarding this, we need to present the applicable metrics $\mathfrak{d}_1(\cdot)$ and $\mathfrak{d}_2(\cdot)$ on Banach space $\Pi.$ Regarding this, for non-negative increasing continuous function $\varphi(u)$ on unbounded interval $I$, the metric $\mathfrak{d}_1(\cdot)$ is given by

Also, for non-negative decreasing continuous function $\varphi(u)$ on unbounded interval $I$, the metric $\mathfrak{d}_2(\cdot)$ is given by

We can guarantee that $\mathfrak{d}_1(\cdot)$ and $\mathfrak{d}_2(\cdot)$ are metrics on Banach space $\Pi,$ as given in the work ^[60] and references therein.

In the following, we present the definitions of $\mathcal UHR, \mathcal UH$ and s-$\mathcal UHR$ stability, then state and prove their theorems.

Definition 4.1. (^[61]) The solution of the multi-order fractional problem specified in (1.1) is $\mathcal UHR$ stable, if for every continuously differentiable function ${Q}\, :I = (a, \infty)\to\Pi$ verifying

where $\varphi(u)$ is a non-negative non-decreasing continuous function on unbounded interval $I$, there is a unique solution ${Q}\, _0$ of the multi-order fractional problem specified in (1.1), and a constant $M > 0$ independent of ${Q}\, , {Q}\, _0,$ where

Moreover, if we replace $\varphi(u)$ by $\omega\geq0$, then the solution of the multi-order fractional problem specified in (1.1) is $\mathcal UH$ stable.

Definition 4.2. (^[61]) The solution of the multi-order fractional problem specified in (1.1) is s-$\mathcal UHR$ stable, if for every continuously differentiable function ${Q}\, :I = (a, \infty)\to\Pi$ verifying

where $\omega\geq0$, there is a unique solution ${Q}\, _0$ of the multi-order fractional problem specified in (1.1), and a constant $M > 0$ independent of ${Q}\, , {Q}\, _0$ for some positive decreasing continuous function $\varrho(u)$ on unbounded interval $I$, where

Theorem 4.1. Suppose that $(A_1)$ and $(A_2)$ are fulfilled, $\varphi(u)$ be a non-negative continuous increasing function on unbounded interval $I$, and ${Q}\, :I = (a, \infty)\to\Pi$ is continuously differentiable function verifying

Then, there is one and only one solution ${Q}\, _0\in\Pi,$ such that

where $\sup\limits_{u\in I} \dfrac{(\varrho(u)-\varrho(a))^{\eta}}{\Gamma(\eta+1)(\sigma(u))}\leq K < \infty, \; for\; \eta = (p_n \; or\; p_n-\mu),$ which yields that the solution of problem specified in (1.1) is $\mathcal UHR$ stable and consequently is $\mathcal UH$ stable.

Proof. Consider $\Xi:\Pi\to\Pi$ be the contractive operator as given in (3.2).

Now, for ${Q}\, , \bar{{Q}\, }\in\Pi,$ it follows from metric $\mathfrak{d}_1(\cdot)$ and the assumptions $(A_1)$–$(A_2)$ that

and

Then, we obtain

In the light of inequalities (4.1) and (4.2), we have

Due to inequalities (4.3) and (4.4), we get

Based on $(i) \; and \; (ii)$ of Theorem 2.1, there is an one and only one fixed point ${Q}\, _0$ such that $\Xi{Q}\, _0 = {Q}\, _0.$ As consequence of $(iii)$ of Theorem 2.1, we can conclude that

According to the above conclusions, the solution of problem specified in (1.1) is $\mathcal UHR$ stable. Along with this, if $\varphi(u) = 1$, then the solution of problem specified in (1.1) is $\mathcal UH$ stable.

Theorem 4.2. Suppose that $(A_1)$ and $(A_2)$ are fulfilled, $\varphi(u)$ be a non-negative decreasing continuous function on unbounded interval $I$, and ${Q}\, :I = (a, \infty)\to\Pi$ is continuously differentiable function verifying

where $\omega > 0$. Then, there is one and only one solution ${Q}\, _0\in\Pi$, and a constant $\Psi > 0$ such that

where $\sup\limits_{u\in I} \dfrac{(\varrho(u)-\varrho(a))^{\eta}}{\Gamma(\eta+1)\sigma(u)}\leq K < \infty, \; for\; \eta = p_n \; or\; p_n-\mu,$ which yields that the solution of problem specified in (1.1) is s-$\mathcal UHR$ stable.

Proof. Similar to Theorem 4.1, take the contractive operator $\Xi:\Pi\to\Pi$ as given in (3.2). From metric $\mathfrak{d}_2(\cdot)$ and assumptions $(A_1)$–$(A_2)$, we present that

and

Then, we get

Due to non-negativeness, continuity of a decreasing function $\varphi(u), \forall u\in I$, we find

Based on inequalities (4.5) and (4.6), we obtain

From inequalities (4.7) and (4.8), we have

From $(i) \; and \; (ii)$ of Theorem 2.1, there is an unique fixed point ${Q}\, _0$ such that $\Xi{Q}\, _0 = {Q}\, _0$. As consequence from $(iii)$ of Theorem 2.1, we can deduce that

According to the above conclusions, the solution of the multi-order fractional problem specified in (1.1) is s-$\mathcal UHR$ stable, and the proof is completed.

5.   Example

Herein, we present an example to illustrate validity of main results.

Now, consider the following multi-order $\varrho$-Hilfer fractional pantograph implicit differential equation:

Here, $n = 3, p_3 = \frac{9}{5}, p_2 = \frac{6}{4}, p_1 = \frac{5}{4}, q_3 = \frac{3}{4}, q_2 = \frac{1}{3}, q_1 = \frac{1}{4}, c_2 = 2, c_1 = 3, \mu = \frac{1}{5}, \nu = \frac{1}{6}, a_1 = \frac{1}{2}, a_2 = \frac{1}{3}, \rho_3 = \frac{31}{16}, \delta = \frac{1}{3} \; and\; \sigma(u) = 20+u^6.$

The applicable Banach space is given as follows:

Clearly, the assumption $(A_1)$ is satisfied for $x_1(u) = \frac{u^2}{200}$ and $x_2(u) = \frac{\sqrt{u}}{100}$. In the following, we will introduce some particular cases:

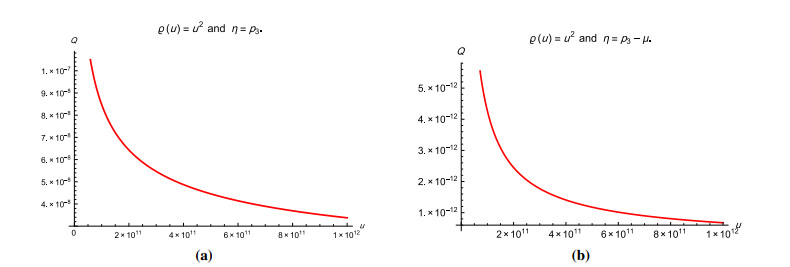
${\rm{(i)}}$ Hilfer-Katugampola Case: Let $\varrho(u) = u^2$ for $u\in (0, \infty)$, by using Mathematica software, we have

Figure 1, shows the graphical representation of $Q$ which is less than $1$ in the Hilfer-Katugampola sense, namely $\varrho(u) = u^2$ for $\eta = p_3$ in Figure 1a, or $p_3-\mu$ in Figure 1b.

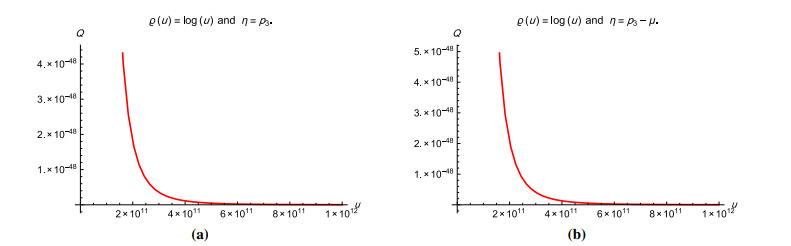
$({\rm{ii}})$ Hilfer-Hadamard Case: Let $\varrho (u) = \log u$ for $u\in(1, \infty)$, then we obtain

Figure 2, shows the graphical representation of $Q$ which is less than $1$ in the Hilfer-Hadamard sense, namely $\varrho(u) = \log(u)$ for $\eta = p_3$ in Figure 2a, or $p_3-\mu$ in Figure 2b.

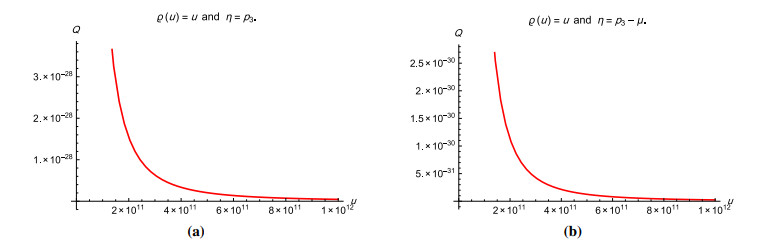
$({\rm{iii}})$ Hilfer Case: Let $\varrho(u) = u$ for $u\in (0, \infty)$, by the same process in $(i)$, we get

Figure 3, shows the graphical representation of $Q$ which is less than $1$ in the Hilfer sense, namely $\varrho(u) = u$ for $\eta = p_3$ in Figure 3a, or $p_3-\mu$ in Figure 3b.

Therefore, we observe in all cases that the assumption $(A_2)$ is satisfied. Thus by Theorem 3.1, we deduce that the multi-order fractional problem (5.1) possesses a unique solution in all cases on corresponding unbounded domains in applicable Banach space $\Pi_1$.

6.   Conclusions

This paper announced that, by utilizing the Banach fixed point theorem and nonlinear analysis topics in an applicable Banach space on unbounded domains $(a, \infty)$, for $a\geq 0$, the multi-order $\varrho$-Hilfer fractional pantograph implicit differential equation provides existence and uniqueness results as well as $\mathcal UH$, $\mathcal UHR$ and s-$\mathcal UHR$ stability. Also, an example includes some particular cases is provided to illustrate the validity of our results.

There are two important notes which young researchers can focus on those for their future works. First, can proportional delays bring out some difficulties when you deal with the existence and stability of a multi-order Hilfer fractional pantograph implicit differential equation on unbounded domains? Second, it is good idea that young researchers try to find new sufficient conditions by changing the Banach fixed point theorem with another contraction fixed point results.

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgments

This study is supported via funding from Prince Sattam bin Abdulaziz University project number (PSAU/2023/R/1444). The authors express their gratitude to dear unknown referees for their helpful suggestions which improved the final version of this paper. One of the unknown respected reviewers arise some queries which completed the conclusion section by adding two new ideas for young researchers.

Conflict of interest

The authors declare that they have no competing interests.