Fitted mesh method for singularly perturbed fourth order differential equation of convection diffusion type with integral boundary condition

1.   Introduction

A singularly perturbed problem (SPP) is a differential equation, for which the solution is affected by a small parameter that multiplies the leading derivative term. The solutions to these problems undergo fast changes in small portions of the domain, hence the traditional numerical approaches are ineffective for SPP. For these types of situations, it is required to develop suitable numerical approaches such that the error estimates are independent of the parameter. A technique that provides parameter-uniform numerical solutions is found in ^[6,19]. In such cases, the most effective and simplest approach is the Shiskin mesh (piecewise uniform mesh).

Nonlocal boundary value issues are differential equations having conditions that link the values of the unknown solution at the boundary with values inside the equation. Of these, the differential equation with IBC is the essential problem that has been researched so far. A few years ago, research was carried out by the authors of ^{[4,7,8,12,16,17,28,32]} to analyze the behavior of solutions to the higher-order differential equations with IBC that are not singularly perturbed. This article concentrates on singularly perturbed fourth-order convection diffusion differential equations with IBC.

Later, Debela and Duressa suggested a computational method for the class of SPCDE with IBC using the fitted operator method and the Richardson extrapolation technique in ^[3]. A singularly perturbed linear system of the Fredholm integro-differential problem with IBC is considered in ^[5]. They investigated a second-order convergent by applying the finite difference approach using the trapezoidal rule in both the equation and the initial condition. In ^[14], a uniform first-order solution is obtained by imposing a Bakhvalov mesh for the first order parameterized singularly perturbed differential equation with IBC, whereas the authors of ^[15] prove a second order convergent. Raja and Tamilselvan ^[22] discussed the finite difference scheme on Shishkin mesh for a system of singularly perturbed convection diffusion equations with IBC.

The numerical method for the fourth-order SPP is considered in ^[9,23,27]. A quintic B-spline approach for solving the fourth-order singularly perturbed boundary value problem provides a solution without reducing the order. An initial value technique for the fourth-order singularly perturbed boundary value problems was examined by Mishra in ^[20]. Research articles ^{[18,21,24,25,26]} deal with third-order convection differential equations. Zen et al. ^[2] presented a numerical technique to solve a nonlinear SPP with IBC. The authors of ^[11] discussed SPP with boundary turning points. The numerical methods for higher-order differential equations, fractional integro-differential equations, and biharmonic problems are discussed in ^{[1,10,13,29,30,31]}.

The organisation of this article is as follows: Section 2 defines the problem's statement, as supported to Section 3, which establishes the maximum principle, the stability finding, and the estimations of the analytical solutions that were produced. The numerical scheme is provided in Section 4, while Section 5 yields the error estimates for each numerical solution and Section 6 offers the numerical experiments. The research article is concluded with a discussion given in Section 7.

2.   Problem description

Inspired by ^[7,20,27] works, we take into account the ensuing fourth order SPCDE with IBC:

where $0 < \varepsilon < < 1,$ $a(t)\geq\alpha_1 > \alpha+2 > 3$, $b(t)\geq\beta\geq0$, $\theta\leq c(t)\leq \theta_0\leq0$, $\beta+2\theta > 0$, $g(t)$ is non negative with $\int\limits_0^1g(t) dt < 1$, $a(t), b(t), c(t), f(t), g(t)$ are sufficiently smooth on $[0, 1] = \bar\Omega$ and $y\in C^4(\Omega)\cap C^2(\bar\Omega)$.

Through out the paper, we assume that $\varepsilon\leq CN^{-1}$ and $\| y\|_D = \sup\limits_{x\in D}|y(x)|$. $C$ is independent constant of $\varepsilon$.

3.   Analytical results

The Eqs (2.1)–(2.3) may be changed into the following problem:

where $\bar y(t) = (y_1(t), y_2(t))$ with the boundary conditions

Theorem 3.1. (Maximum Principle) Let $\bar y(t)$ be any function satisfying $y_1(0)\geq 0, y_1(1)\geq0$, $y_2(0)\geq 0, By_2(1)\geq0$, $L_1\bar y(t)\geq0$ and $L_2\bar y(t)\geq0, \; t\in\Omega$. Then $\bar y(t)\geq 0$, $t\in \overline{\Omega}$.

Proof. Define $\bar s(t) = (s_1(t), s_2(t))\; \text{as} \; s_1(t) = 2(1-\frac{t^2}{2}), s_2(t) = 1 \label{s1}$. Note that $\bar s(t) > 0, t \in\overline{\Omega}$, $\bar L_1 \bar s(t) > 0, L_2\bar s(t) > 0 \; t\in\Omega$, $s_1(0) > 0, s_2(0) > 0, s_1(1) > 0$ and $Bs_2(1) > 0$. Further we define

Then there exists at least one $t_0\in \Omega,$ such that $\left(\dfrac{-y_1(t_0)}{s_1(t_0)}\right) = \gamma$ or $\left(\dfrac{-y_2(t_0)}{s_2(t_0)}\right) = \gamma$ or both. Also $(\bar y+\gamma \bar{s})(t)\geq \bar{0}, \; t\in \bar{\Omega}.$ Without loss of generality we assume that $\left(\dfrac{-y_1(t_0)}{s_1(t_0)}\right) = \gamma$. Then $(y_1 + \gamma s_1)$ attains its minimum at $t = t_0$. It is simple to see that for every $t\in \overline{\Omega}$, $\bar y(t)\geq0$ if $\gamma\leq0$. Now we will show that indeed $\gamma\leq0$. Suppose $\gamma > 0$.

Case (ⅰ): If $(y_1+\gamma s_1)(t_0) = 0$, for $t_0 = 0$, Then,

Case (ⅱ): If $(y_1+\gamma s_1)(t_0) = 0, \;$ for $t_0\in \Omega$, Then,

Case (ⅲ): If $(y_1+\gamma s_1)(1) = 0$, Then,

Case (ⅳ): If $(y_2+\gamma s_2)(1) = 0$, Then,

Case (ⅴ): If $(y_2+\gamma s_2)(t_0) = 0, \;$ for $t_0\in \Omega$. Therefore $(y_2+\gamma s_2)$ attains its minimum at $t = t_0$. Then,

Case (ⅵ): Assume that $(y_2+\gamma s_2)(1) = 0, \;$ Then,

Be aware that we reached a contradiction in each and every situation. Therefore, $\gamma > 0$ cannot exist. This demonstrates that $y_1(t)\geq0$, $y_2(t)\geq0$. Hence $\bar y(t)\geq0, t\in\bar\Omega.$ □

Lemma 3.2. (Stability Result) The solution to the problem (3.1)–(3.4) is $\bar y(t)$, fulfils the condition.

Proof. Define $\psi^{\pm}_i(t) = CMs_i(t)\pm u_i(t), t\in\overline{\Omega}, i = 1, 2$, where

Note that $\psi^{\pm}_1(0)\geq 0,$ $\psi^\pm_1(1)\geq 0,$ $\psi^\pm_2(0)\geq 0$ and $B\psi^\pm_2(1)\geq 0$. Observe that $L_1\bar\psi^\pm(t)\geq 0$, $L_2\bar \psi^\pm(t)\geq 0$. The desired outcome is then obtained using the maximal principle. □

The following lemma gives bounds for the derivatives of $\bar y(t)$.

Lemma 3.3. Let (2.1) and (2.2) be the solution, and let $\bar y(t)$ be the solution. Then, for $1\leq k\leq3$,

Proof. This lemma may be demonstrated by applying the arguments provided in ^[6] and corollary 3.2 in that order. □

The sharper constraints on the derivatives of the solution $\bar y(t)$ may be used to calculate the uniform error estimate. We formulate the analytical solution in the form $\bar y(t) = \bar v(t)+\bar w(t)$ to get sharper constraints, where $\bar v(t) = (v_1(t), v_2(t))$ and $\bar w(t) = (w_1(t), w_2(t))$. The regular component $\bar v(t)$ can be written as $\bar v(t) = \bar v_0(t)+\varepsilon \bar v_1(t)+\varepsilon^2\bar v_2(t)$, where $\bar v_0(t) = (v_{01}(t), v_{02}(t))$, $\bar v_1(t) = (v_{11}(t), v_{12}(t))$, $\bar v_2(t) = (v_{21}(t), v_{22}(t))$ respectively satisfy the following equations:

Consequently, the regular component $\bar v(t)$ is the solution to

and layer component $\bar w(t)$ is the solution of

Theorem 3.4. Let $\bar v_0(t)$ be the solution to the reduced problem $\bar v_0(t)$ and $\bar y(t)$ be the solution to the problem (2.1) and (2.2). Then

Proof. Consider $\bar\psi^\pm(t) = (\psi^\pm_1(t), \psi_2^\pm(t))$, where

Note that $\psi_1^{\pm}(0)\geq 0$, $\psi_1^{\pm}(1)\geq 0$, $\psi_2^{\pm}(0)\geq 0$ Further

Let $t\in(0, 1)$. Then

The maximal principle then gives us $\bar\psi^\pm(x)\geq0, \; t\in\overline{\Omega}.$ □

Lemma 3.5. The solution $\bar y(t)$ to the problem (3.1)–(3.4) satisfies the following constraints in its regular and singular components, respectively:

Proof. With the method described in ^[6], the necessary limits on $\bar v$, $\bar w$, and their derivatives are obtained. □

Note: It is simple to observe from the aforementioned theorem that,

4.   Discretization problems

On $\bar{\Omega}$, a piecewise uniform Shishkin mesh with mesh intervals of $N$ ($\geq4$) is produced. Two subintervals are created for the domain $\bar{\Omega}$: $[0, 1-\sigma]$ and $[1-\sigma, 1]$ where $\sigma$ is the transitional parameter and $\sigma = \min\{\frac{1}{2}, \frac{2\varepsilon\ln N}{\alpha}\}$ specifies it. With regard to $[0, 1-\sigma]$ and $[1-\sigma, 1]$. A homogeneous mesh with $\frac{N}{2}$ mesh spacing is put in place. The interior mesh points are shown by

Clearly, $\bar\Omega^N = \{t_i\}_0^N$.The mesh step should be $h_i = t_i-t_{i-1}$, and the bar step should be $\hbar_i = \frac{h_{i+1}+h_i}{2}$.

The discrete problem that results from (2.1) and (2.2) is:

Find $\bar Y = (Y_1(t_i), Y_2(t_i))$ such that

where

Theorem 4.1. (Discrete maximum principle) Let $\bar\Psi(t_i) = (\Psi_1(t_i), \Psi_2(t_i))$ be the mesh function satisfying $\Psi_1(t_0)\geq0, \Psi_2(t_0)\geq0, \Psi_1(t_N)\geq0, B^N\Psi_2(t_N)\geq0, L_1^N\bar\Psi(t_i)\geq0,$ and $L_2^N\bar\Psi(t_i)\geq0$. Then $\bar\Psi(t_i)\geq0$, $t_i\in\overline\Omega^N$.

Proof. Let $\bar S(t_i) = (S_1(t_i), S_2(t_i))$ where

$S_1(t_i) = 2(1-\frac{t_i^2}{2}), t_i \in\overline{\Omega}^N,$

$S_2(t_i) = 1, t_i \in\overline{\Omega}^N$.

Note that $\bar S(t_i) > 0, \forall t_i \in\overline{\Omega}^N$, $S_1(t_0) > 0, S_1(t_N) > 0, S_2(t_0) > 0, B^NS_2(t_N) > 0, L_1^N\bar S_1(t_i) > 0$ and $L_1^N\bar S_1(t_i) > 0$, $\forall t_i\in\Omega^N$. Let

Then there exists one $t_k\in\overline{\Omega}^N$ such that $\Psi_1(t_k)+\gamma S_1(t_k) = 0$ or $\Psi_2(t_k)+\gamma S_2(t_k) = 0$ or both. We have $\Psi_j(t_i)+\gamma S_j(t_i)\geq0, t_i\in\overline{\Omega}^N, j = 1, 2.$ Therefore either $(\Psi_1+\gamma S_1)$ or $(\Psi_1+\gamma S_1)$ attains minimum at $t_i = t_k$. Suppose the theorem does not hold true, then $\gamma > 0.$

Case (ⅰ): If $(\Psi_1+\gamma S_1)(t_0) = 0$, Then,

Case (ⅱ): If $(\Psi_1+\gamma S_1)(t_k) = 0$, for $t_k\in {\Omega}^N$. Then,

Case (ⅲ): If $(\Psi_1+\gamma S_1)(t_N) = 0$, Then,

Case (ⅳ): If $(\Psi_2+\gamma S_2)(t_0) = 0$, Then,

Case (ⅴ): If $(\Psi_2+\gamma S_2)(t_k) = 0$, for $t_k\in {\Omega}^N$. Then,

Case (ⅵ): If $(\Psi_2+\gamma S_2)(t_N) = 0$, Then,

Note that we got to a contradiction in each and every situation. In light of this, $\gamma > 0$ cannot exist. According to this, $\Psi_1(t_i)\geq 0$, $\Psi_2(t_i)\geq0$. Hence $\bar\Psi(t_i)\geq0, t_i \in\overline{\Omega}^N.$ □

Lemma 4.2. (Discrete stability result) Let $\bar Y(t_i) = (Y_1(t_i), Y_2(t_i))$ be any mesh function. Then

Proof. One may prove the aforementioned inequality by utilising Theorem 4.1, and proper barrier functions. □

Similar to the continuous case, the discrete solution $\bar U(x_i)$ may be broken down into $\bar Y(t_i) = \bar V(t_i)+\bar W(t_i)$, where $V(t_i)$ and $W(t_i)$ are the answers to the following problems, respectively:

and

The theory that follows provides an estimation for the difference between the answers of (4.1)–(4.3).

Theorem 4.3. Let $\bar Y$ be the numerical solution to the equation (2.1) and (2.2) as specified by (4.1) and (4.2). A numerical solution of (3.8) defined by (4.3) and $\bar V$. Then

Proof. Consider $\bar\Psi^\pm(t_i) = (\Psi_1^\pm(t_i), \Psi_2^\pm(t_i))$, where

Now

Then we arrive at the conclusion using Theorem 4.1. □

5.   Estimated errors for the solution

For each component of the numerical solution, we get a different estimate of the error.

Lemma 5.1. Assume that $\bar V(t_i)$ is a numerical solution to the Eq (3.8) as specified by (4.3). Then

Proof. Now

Therefore

Further

where $t_{i-1}\leq\chi_i\leq t_i$, $1\leq i\leq N$. Then, as a result of discrete stability, we have $|(v_j(t_i)-V_j(t_i))|\leq CN^{-1}, \; t_i\in \bar{\Omega}^N, \; \; j = 1, 2$. □

Lemma 5.2. Let (3.9) defined in (3.9) have a numerical solution, $\bar W(t_i)$. Then

Proof. Observe that

Then by (3.14), Theorem 3.4 and Lemma 5.1, we have

Therefore

Consider $\bar\Psi^\pm(t_i) = (\Psi_1^\pm(t_i), \Psi_2^\pm(t_i)), \; t_i\in[1-\sigma, 1]$, where

In consideration of the discrete maximum concept, we therefore have $\Psi_j^{\pm}(t_i)\geq 0$, $t_i\in[1-\sigma, 1], \; j = 1, 2$.

Therefore $|w_j(t_i)-W_j(t_i)|\leq CN^{-1}(\ln N)^2, \; t_i\in[1-\sigma, 1], \; j = 1, 2.$ □

Theorem 5.3. Let (2.1) and (2.2) be the solution as defined in (4.1) and (4.2), and let $\bar Y(t_i)$ be the result. Then

Proof. The proof is finished by combining the Lemmas 5.1 and 5.2. □

6.   Numerical results

Example 6.1.

Example 6.2.

There is no analytical answer for the aforementioned situations. As a result, we use the double mesh principle, which is defined as $D_\varepsilon^N = \max\limits_{x_i\in \overline{\Omega}_\varepsilon^N}|U^N(x_i)-{U}^{2N}(x_i)|$ and $D^N = \max\limits_\varepsilon D_\varepsilon^N$, where the numerical solution calculated using $N$ and $2N$ mesh points is denoted by $U^N(x_i)$ and $U^{2N}(x_i)$. The order of convergence is given by $P^N = \log_2(\frac{D^N}{D^{2N}})$ based on these values.

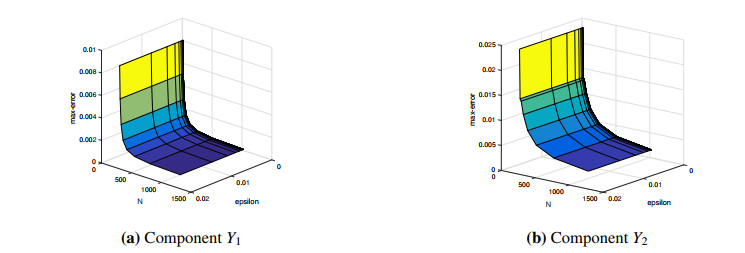
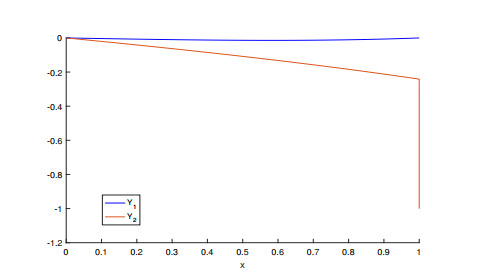
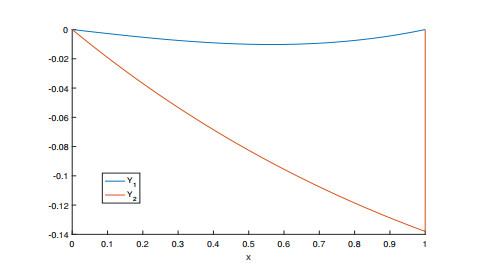
For different values of $\varepsilon$, the approximate solutions for Example 6.1 are plotted in Figure 1 on a Shishkin mesh. Numerical solutions of Example 6.1 are plotted in Figure 2. These figures show that when $\varepsilon$ decreases, boundary layers are present near $x = 1$. The most possible pointwise error in the numerical solution is plotted in Figures 3 and 4 for Examples 6.1 and 6.2.

7.   Conclusions

This article has provided the fitted mesh method for the fourth-order SPCDE with IBC. As a consequence, the approach converges in the discrete maximum norm with $\varepsilon$-uniformity with respect to the perturbation parameter. Maximum absolute errors and convergence rates have been reported for the given numerical results (see Tables 1–4). According to these observations, the proposed method is uniformly convergent and has almost first-order accuracy on the piecewise uniform mesh. Concisely, the strategy is reliable and well-suited for dealing with such problems. Further, higher-order difference schemes can be proposed to promote numerical investigations.

Conflict of interest

The author declares that there is no conflict of interest regarding the publication of this paper.