W-shaped soliton solutions to the modified Zakharov-Kuznetsov equation of ion-acoustic waves in (3+1)-dimensions arise in a magnetized plasma

1.   Introduction

Nonlinear partial differential equations (NLPDEs) depict a wide range of phenomena not only in physics but also in other fields in science such as chemistry, biology, and engineering. Physical phenomena involving nonlinear waves play an important role in research on NLPDEs. In previous decades, several strong numerical and analytical methods have been introduced for formulating explicit solutions for several NLPDEs in physics and mathematics, for instance, shooting techniques with 4th-order Runge-Kutta method ^[1,2,3,4], the homotopy analysis scheme ^[5], the modified Rusanov scheme ^[6], the homotopy perturbation scheme ^[7,8], the finite forward difference scheme ^[9,10], the Adomian decomposition method ^[11,12], the extension exponential rational function method ^[13], the modified exponential function method ^[14], the sine-Gordon expansion method ^[15,16], the $\left(m+{}^{{{G}'}}/{}_{G} \right)$ expansion method ^[17,18], the sinh-Gordon expansion method ^[19,20,21], the extended auxiliary equation mapping method ^[22,23], the Bernoulli sub-equation function method ^[24,25], the improved Bernoulli sub-equation function method ^[26,27,28], the modified Jacobi elliptic function expansion method ^[29], the modified auxiliary expansion method ^[30], the Hirota bilinear method ^[31], the improved $\tan \left(\phi \left(\xi \right)/2 \right)$-expansion method ^[32], the improved generalized Riccati equation mapping method ^[33,34], the F-expansion method ^[35,36,37], and the Riccati equation rational expansion method ^[38].

Munro and Parkes introduced the new Zakharov-Kuznetsov (ZK) in 1999 ^[39], they viewed the most realistic state if particles are non-isothermal. With the correctly modified form of the electron number density proposed by Schamel, it has been said that the reductive disturbance procedure tends to result in the modified ZK equation. Several reports have been presented to find the analytical solutions to the nonlinear modified ZK equation, such as, the extended tanh method ^[40], the Hirota method ^[41], the sine-cosine method ^[42], the extended direct algebraic method ^[43], fractional sub-equation technique ^[44], and the homogeneous balance technique ^[45].

One form of the envelope that is particularly interesting is the W-shaped soliton, which was first described in optical fiber mediums with higher-order effects ^[46], and afterward achieved in a variety of higher-order NLSEs ^[47,48,49]. More specifically, there are two valleys on the sides of one hump of this pulse shape. A soliton of this kind is relatively rare in nonlinear science compared to the fundamental bright and dark soliton.

According to our understanding, the features of nonlinearly W-shaped soliton pulses propagating in magnetized plasmas have not been previously studied. Three novel forms of W-shaped soliton solutions are presented in this research, apart from the one previously reported in magnetized plasmas applications. In this research paper, we utilize the $\tan \left(\phi \left(\zeta \right)/2 \right)$-expansion ^[50], $(m+G'(\zeta)/G(\zeta))$-expansion ^[17] and He exponential function ^[51] methods to formulate novel solutions to the modified ZK equation. Assume the following nonlinear modified ZK equation in three dimensions ^[52]:

where the constant $c > 0$.

This is how the paper is arranged. The instructions of the methods that use to solve the modified ZK equation of ion-acoustic waves in a magnetized plasma will be presented in Section 2. Last section presents some exact solutions to the nonlinear differential equation that governs the amplitude dynamics of fields based on the traveling wave method. These solutions include W-shaped soliton solutions. In Section 4, we present the results and discuss the gained solutions. Section 5 of the paper offers a conclusion.

2.   Instructions of the methods

2.1.   The $\tan \left(\phi \left(\zeta \right)/2 \right)$-expansion method

Suppose a NPDE follows the following form:

where $\phi = (x, y, z, t)$ and it can be transformed into an ODE

By using the wave transformation $\phi = u(\zeta)$ and $\zeta = kx+ly+\rho z-wt$. Consider the following as an expression for the traveling wave solution of Eq (2.2)

where the constants ${{a}_{k}}\ne 0$ to be evaluated later and satisfies the ODE

Where $\xi = {{A}^{2}}+{{B}^{2}}-{{C}^{2}}$, here are a class of solutions to Eq (2.4):

Family 1: In case $\xi < 0$ and $B-C\ne 0$, the solution is

Family 2: In case $\xi > 0$ and $B-C\ne 0$, the solution become

Family 3: In case $\xi > 0$, $B\ne 0$ and $C = 0$, the solution is

Family 4: In case $\xi < 0$, $C\ne 0$ and $B = 0$, the solution become

Family 5: In case $\xi > 0$, $B-C\ne 0$ and $A = 0$, the solution is

Family 6: In case $A = 0$ and $C = 0$, the solution is

Family 7: In case $B = C = 0$, the solution is

Family 8: In case ${{A}^{2}}+{{B}^{2}} = {{C}^{2}}$, the solution is

Family 9: In case $A = B = C = kA$, the solution is

Family 10: In case $A = C = kA$ and $B = -kA$, the solution is

Family 11: In case $C = A$, the solution is

Family 12: In case $A = C$, the solution is

Family 13: In case $C = -A$, the solution is

Family 14: In case $B = -C$, the solution is

Family 15: In case $B = 0$ and $A = C$, the solution is

Family 16: In case $A = 0$ while $B = C$, the solution is

Family 17: In case $A = 0$ while $B = -C$, the solution is

Family 18: In case $A = B = 0$, the solution is

Family 19: In case $B = C$, the solution is

Here the constants $A, B$ and $C$ will be determined later. The value of $m$ will found by using the balance principle. Inserting Eq (2.3) into Eq (2.2) and collecting the coefficients of $\tan {{\left(\phi /2 \right)}^{m}}, \left(m = 1, 2, 3, ... \right),$ then the zeroing out of each coefficient, one can obtain a set of equations that are over-determined for $A, B, C, {{a}_{m}}, \left(m = 1, 2, .., k \right)$ and $w$. Mathematica Package has been used to perform symbolic computations.

2.2.   The He Exp-function method

Consider the following as an expression for the traveling wave solution of Eq (2.2)

where $a_n$ and $b_m$ are unidentified constants, while $i, j, g$ and $h$ are positive integers that need to be found later. We match the highest order linear term in Eq (2.6) with the highest order nonlinear term to reach the values of $i$ and $g$. Collecting the coefficients of exponential function with the same power then the zeroing out of each coefficient, we can obtain a set of equations that are over-determined for $a_n, b_n \, (n = 0, \mp 1, \mp 2, ...)$, $k, l, \rho$ and $w$. Mathematica Package has been used to perform symbolic computations.

2.3.   The $(m+\frac{G'}{G})$-expansion method

Let's assume that the following equation satisfies Eq (2.2):

where ${{a}_{i}}, \, \, i = 0, \pm 1, ..., \pm n,$ are scalars and the constant $m\ne 0$. We determine the value of "n" while keeping in mind the rules of balance. In this research paper, we let

where $G\left(\zeta \right)$ verify $G''+\left(\lambda +2m\mu \right)G'+\mu G = 0$. Putting Eq. (2.7) into Eq. (2.2) with using Eq (2.8) and zeroing out all terms that have the same power of the ${{\left(m+\varphi \right)}^{n}}$, the system of algebraic equations for $\omega, {{a}_{n}}, \, \, n = 0, \, 1, \, ...\, , \, n$, $\lambda$ and $\mu$ will be obtained. Solving the system, one can evaluate ${{a}_{n}}, \, \, n = 0, \, 1, \, ...\, , \, n$, $k, l, \rho$ and $w$. Using these numbers and put a general solution of the LODE Eq (2.8) into equation Eq (2.7), we can find the precise solution of Eq (1.1).

3.   On solving the (3+1)-dimensional modified ZK

3.1.   Application on the $\tan \left(\phi \left(\zeta \right)/2 \right)$-expansion method

Consider the following traveling wave solution

Where $k, l, \rho$ are wave numbers and $w$ is a frequency. By using Eq (3.1) into Eq (1.1), we get

Integrate Eq (3.2) with the constant of integration is $e_1$, we have

By taking ${{u}^{\frac{1}{2}}} = v$, Eq (3.3) can be rewrite as

Taking the balance between the nonlinear term $v{v}''$ and the highest order derivative ${{v}^{3}}$ in Eq (3.4), gives m = 2. Therefore Eq (2.3) becomes

A system of equations is derived by inserting Eq (3.5) into Eq (3.4). Solution of this system provides the following class of solutions:

Case 1. In case ${{a}_{0}} = -\frac{\left(2{{A}^{2}}-{{B}^{2}}+{{C}^{2}} \right)e_{{1}^{1/3}}}{2\sqrt[3] {4}{{\left(-{{\left({{A}^{2}}+{{B}^{2}}-{{C}^{2}} \right)}^{3}}k \right)}^{1/3}}}$, ${{a}_{1}} = \frac{3A\left(B-C \right){{e}_{1}}^{1/3}}{\sqrt[3] {4}{{\left(-{{\left({{A}^{2}}+{{B}^{2}}-{{C}^{2}} \right)}^{3}}k \right)}^{1/3}}}$, ${{a}_{2}} = -\frac{3{{\left(B-C \right)}^{2}}e_{{1}^{1/3}}}{2\sqrt[3] {4}{{\left(-{{\left({{A}^{2}}+{{B}^{2}}-{{C}^{2}} \right)}^{3}}k \right)}^{1/3}}}$, $c = -\frac{3\left({{A}^{2}}+{{B}^{2}}-{{C}^{2}} \right){{e}_{1}}^{1/3}}{2\sqrt[3] {4}{{\left(-{{\left({{A}^{2}}+{{B}^{2}}-{{C}^{2}} \right)}^{3}}k \right)}^{1/3}}}-\frac{\omega }{k}$, the outcomes solutions are:

Solution 1: When $-{{A}^{2}}-{{B}^{2}}+{{C}^{2}} > 0$ and $-{{\left({{A}^{2}}+{{B}^{2}}-{{C}^{2}} \right)}^{3}}k\ne 0$ then according to family 1, we gain periodic-singular solution as seen in Figure 1.

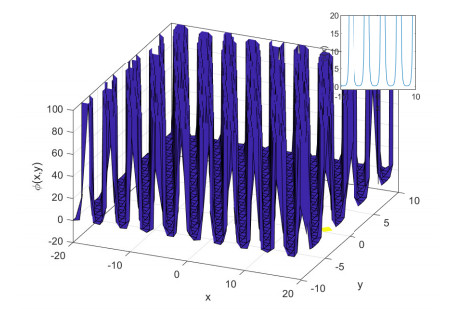
Solution 2: When ${{A}^{2}}+{{B}^{2}}-{{C}^{2}} > 0$ and $-{{\left({{A}^{2}}+{{B}^{2}}-{{C}^{2}} \right)}^{3}}k\ne 0$ then according to family 2, we gain w-shaped solution as presented in Figure 2.

Solution 3: When $\left({{A}^{2}}+{{B}^{2}} \right)k\ne 0$ then according to family 3, we gain w-shaped solution as shown in Figure 3.

Solution 4: When ${{C}^{2}}-{{A}^{2}} > 0$ and $k\ne 0$ then according to family 4, we gain bright-singular solution

Solution 5: When ${{B}^{2}}-{{C}^{2}} > 0$ and $k\ne 0$ then according to family 5, we get w-shaped soliton solution as seen in Figure 4.

Solution 6: When $A\ne 0$ and $k\ne 0$ then according to family 10, one can construct singular solution as seen in Figure 5.

Solution 7: When $B\ne 0$ and $k\ne 0$ then according to family 11, we gain singular solution

Solution 8: When $B\ne 0$ and $k\ne 0$ then according to family 12, we gain singular solution

Solution 9: When $B\ne 0$ and $k\ne 0$ then according to family 13, we gain singular solution

Solution 10: When $A\ne 0$ and $k\ne 0$ then according to family 14, we gain singular solution

Solution 11: When $C\ne 0$ and $k\ne 0$ then according to family 18, we gain bright-singular solution

Case 2: In case ${{a}_{0}} = -\frac{\sqrt[3] {{{e}_{1}}}\left(2A{{1}^{2}}-B{{1}^{2}}+C{{1}^{2}} \right)}{2\sqrt[3] {4}{{\left(-{{\left({{A}^{2}}+{{B}^{2}}-{{C}^{2}} \right)}^{3}}k \right)}^{1/3}}}, {{a}_{1}} = \frac{3A\sqrt[3] {{{e}_{1}}}\left(B-C \right)}{\sqrt[3] {4}{{\left(-{{\left({{A}^{2}}+{{B}^{2}}-{{C}^{2}} \right)}^{3}}k \right)}^{1/3}}}$, ${{a}_{2}} = -\frac{3{{\left(B-C \right)}^{2}}\sqrt[3] {{{e}_{1}}}}{2\sqrt[3] {4}{{\left(-{{\left({{A}^{2}}+{{B}^{2}}-{{C}^{2}} \right)}^{3}}k \right)}^{1/3}}}$, $\rho = \frac{\sqrt{{{\left(B-C \right)}^{2}}\left(-{{k}^{2}}+\frac{3\sqrt[3] {2{{e}_{1}}}}{{{\left(-{{\left({{A}^{2}}+{{B}^{2}}-{{C}^{2}} \right)}^{3}}k \right)}^{1/3}}}-{{l}^{2}} \right)}}{\sqrt{{{\left(B-C \right)}^{2}}}}$, $\omega = -ck+\frac{3\sqrt[3] {{{e}_{1}}}{{\left(-{{\left({{A}^{2}}+{{B}^{2}}-{{C}^{2}} \right)}^{3}}k \right)}^{2/3}}}{2\sqrt[3] {4}{{\left({{A}^{2}}+{{B}^{2}}-{{C}^{2}} \right)}^{2}}}$, we gain the following solutions:

Solution 1: When $\xi < 0$ and $k\ne 0$ then according to family 1, we have periodic-singular solution as shown in Figure 6.

Solution 2: When $\xi > 0$ and $k\ne 0$ then according to family 2, we gain

This solution is a W-shaped solution as presented in Figure 7.

Solution 3: When ${{A}^{2}}+{{B}^{2}}\ne 0$ and $k\ne 0$ then according to family 3, we gain W-shaped solution

Solution 4: When ${{C}^{2}}-{{A}^{2}} > 0$ and $k\ne 0$ then according to family 4, we gain bright-singular solution

Solution 5: When ${{B}^{2}}-{{C}^{2}} > 0$ and $k\ne 0$ then according to family 5, we gain W-shaped solution

Solution 6: When $A\ne 0$ and $k\ne 0$ then according to family 10, we gain singular solution

This is singular solution as shown in Figure 8.

Solution 7: When $B\ne 0$ and $k\ne 0$ then according to family 11, we gain W-shaped solution

Solution 8: When $B\ne 0$ and $k\ne 0$ then according to family 12, we gain singular solution

Solution 9: When $B\ne 0$ and $k\ne 0$ then according to family 13, we gain singular solution

Solution 10: When $A\ne 0$ and $k\ne 0$ then according to family 14, we gain singular solution

Solution 11: When $C\ne 0$ and $k\ne 0$ then according to family 18, we gain singular solution

3.2.   Application on the He Exp-function method

This method is extremely easy to use and is based on the premise in Eq (2.5). Suppose that the formula for the solution to Eq (3.4) is

Taking the balance of the linear term of the highest order in Eq (3.4) with the highest order nonlinear term. Calculating simply, we get

and

By taking the balance between the highest order of Exp-function in Eqs (3.29) and (3.30), in a simplified form we get

Taking only consider the simplest case $i = j = 1$ and as a result $g = h = 1$. Now the ansatz (3.28) can be rewrite as:

Substituting Eq (3.32) into Eq (3.4), collecting the coefficients of exponential function that have the same power to zero, we have the following case

Case: When ${{a}_{-1}} = 0, a_1 = 0, b_1 = \frac{{b_0}^{2}}{4{{b}_{-1}}}, l = \frac{\sqrt{2a_0-b_0\left({{k}^{2}}+{{\rho }^{2}} \right)}}{\sqrt{b_0}}, c = \frac{a_0}{2b_0}-\frac{\omega }{k}, e_1$, a result is

This solution is a bright soliton as seen in Figure 9.

3.3.   Application on the $(m+\frac{G'}{G})$-expansion method

Consider Eq (3.4) and balance the linear term of the highest order in Eq (2.5) with the highest order nonlinear term. By using balance principle and simple calculation, we get $n = 2$. Now the ansatz (2.6) can be rewrite as:

Substituting Eq (3.34) into Eq (3.4), collecting the coefficients of $(m+\varphi)^i, \, i = 0, 1, ...$ that have the same power to zero, we have the following cases:

Case 1: When ${{a}_{-1}} = 0, {{a}_{-2}} = 0$, ${{a}_{0}} = \left(m\left(m+\lambda \right)-\mu \right)\left({{k}^{2}}+{{l}^{2}}+{{\rho }^{2}} \right)$, ${{a}_{2}} = -{{k}^{2}}-{{l}^{2}}-{{\rho }^{2}}$, ${{a}_{1}} = -\lambda \left({{k}^{2}}+{{l}^{2}}+{{\rho }^{2}} \right)$, $c = \frac{1}{4}\left({{\left(2m+\lambda \right)}^{2}}-4\mu \right)\left({{k}^{2}}+{{l}^{2}}+{{\rho }^{2}} \right)-\frac{\omega }{k}, e_1$, we obtain the following solutions:

provided that $\gamma = {{\left(2m+\lambda \right)}^{2}}-4\mu > 0$. When $\gamma < 0$, the solution is

In case $\gamma = 0$, the solution is

These provided solutions are singular as shown in Figures 10–12.

Case 2: When ${{a}_{-1}} = \lambda \left(m\left(m+\lambda \right)-\mu \right)\left({{k}^{2}}+{{l}^{2}}+{{\rho }^{2}} \right)$, ${{a}_{-2}} = -{{\left(\mu -m\left(m+\lambda \right) \right)}^{2}}\left({{k}^{2}}+{{l}^{2}}+{{\rho }^{2}} \right)$, ${{a}_{0}} = \left(m\left(m+\lambda \right)-\mu \right)\left({{k}^{2}}+{{l}^{2}}+{{\rho }^{2}} \right)$, ${{a}_{2}} = 0$, ${{a}_{1}} = 0$, $c = \frac{1}{4}\left({{\left(2m+\lambda \right)}^{2}}-4\mu \right)\left({{k}^{2}}+{{l}^{2}}+{{\rho }^{2}} \right)-\frac{\omega }{k}, e_1 = 0$, one can offer the below solutions:

provided that $\gamma > 0$. When $\gamma < 0$, the solution is

In case $\gamma = 0$, the solution is

These provided solutions are singular.

4.   Results and discussion

Three powerful methods are used to study the considered equation. In this paper, we present W-shaped soliton solutions, in comparison with the solutions reported in Refs. ^{[36,37,38,39,40,41,42]}. This pulse propagation is new and can be constructed rarely in nonlinear science. From the results obtained, we can conclude that the $\tan \left(\phi \left(\zeta \right)/2 \right)$-expansion method provides more novel and different types of solutions in comparison with solutions constructed via other suggested methods.

5.   Conclusions

In the current research paper, we have constructed some novel analytical solutions to the (3+1)-dimensional modified Zakharov-Kuznetsov equation. This equation presents the ion-acoustic waves in a magnetized plasma. Three methods namely; the $\tan \left(\phi \left(\zeta \right)/2 \right)$-expansion, $(m+G'(\zeta)/G(\zeta))$-expansion and He exponential function methods are used to offer different solutions for this model. Various soliton solutions are constructed such as W-shaped, singular, bright and periodic bright-singular. To verify the existence of the solutions, we have inserted them into Eq (1.1) and they satisfy it.

Acknowledgments

This research received funding support from the NSRF via the Program Management Unit for Human Resources & Institutional Development, Research and Innovation (grant number B05F650018).

Conflict of interest

We declare that all the authors have no any conflicts of interest about this submission and publication of this article.

Author contributions

Conceptualization, H.R.N. and N.A.S.; methodology, H.F.I.; software, H.F.I.; validation, H.R.N., N.A.S. and W.W.; formal analysis, W.W.; investigation, H.R.N, H.F.I. and N.A.S.; A.A.H.; writing—original draft preparation, H.R.N., and H.F.I.; writing—review and editing, All authors. Harivan R. Nabi and Nehad Ali Shah contributed equally to this work and are co-first authors.