Determine unknown source problem for time fractional pseudo-parabolic equation with Atangana-Baleanu Caputo fractional derivative

1.   Introduction

The fractional diffusion equation has been of significant interest for many decades and has many important applications in practice, where mathematical formulas are abstract and can be used to provide procedures for converting outside measurements into information about inside properties thus increasingly interested in the study of inverse problems and unknown sources for partial differential equations ^{[1,2,3,4,5,6,7,8,9,10,11]}. So, PDEs with fractional derivatives are a generalization of equations with integer-order partial derivatives, please see in the reference ^{[12,13,14,15,16,17,18,19,20,21,22]}. In this paper, we consider time-fractional PDE

Here the Atangana-Baleanu Caputo derivative $^{\rm{ABC}}_{0} D_{t}^{\alpha}u(t, x)$ is defined by

where $\mathcal{L}(\alpha)$ satisfies $\mathcal{L}(\alpha) = 1 - \alpha + \dfrac{\alpha}{\Gamma(\alpha)}$, and $\mathcal{L}(0) = \mathcal{L}(1) = 1$, and $E_{\alpha, 1}\Big(-\frac{\alpha(t-s)^{\alpha}}{1-\alpha} \Big)$ is the Mittag-Leffler function is introduced in Section 2. The problem (1.1) can be considered in the following two cases:

The case $\alpha = 1$ : System (1.1) is called pseudo-parabolic. There are many works on the well-posedness of the pseudo-parabolic equation with classical derivative, see ^{[23,24,25,26]}, here the condition $u(T, x)$ is replaced by the initial condition $u(0, x)$. An important goal in the scientific community is to investigate the existence, uniqueness, and stability of fractional differential equations (1.1).

The case $\alpha \neq 1$: System (1.1) is called the fractional pseudo-parabolic equation. From the given data $\theta$ and the measured data at the final time $g \in L^{2}(\Omega)$, we recovered the source function $f(x)$. The problem (1.1) is severely ill-posed. The problem (1.1) is called the classical pseudo-parabolic equation. The problem (1.1) is considered with the right hand side of function $F(t, x) = \theta(t)f(x)$. In this case, we would like to take a look at some related issues as follows:

● In case $\theta(t) = 1, k = 0$ and $D_{t}^{\alpha}$ is the Caputo derivative, then (1.1) is called the fractional diffusion equation, and this type has been surveyed a lot, see in ^[27,28,29].

● In case $\theta(t) \neq 1, k = 0$, and $D_{t}^{\alpha}$ is the Caputo derivative, there are a number of studies on this case, see in ^[30,31].

● In case $\theta(t) \neq 1, k \neq 0$, with $D_{t}^{\alpha}$ is Atangana - Baleanu fractional derivative, until now, according to our understanding, we did not find papers to solve this case.

Our problem recover of a space-dependent source function $f(x)$ along with subject to the homogeneous initial condition. When exact data $(\theta, g)$ is given, observed data $(\theta_{\epsilon}, g_{\epsilon})$ will inevitably be noisy by

Regarding the regularization methods, in ^[32], Ting Wei used a quasi boundary value method. In ^[33], Tuan-Long-Thinh used the Tikhonov regularization method. In ^[34], with a general filter method, the authors studied the problem of finding the source distribution for the linear bi-parabolic equation when we have the final observation. In ^[35], Zhang with the truncation regularization method. In ^[36], Yang and his group used fractional Tikhonov to identify the initial value problem for a time-fractional diffusion equation. In ^[37], Cheng and co-authors used iteration regularization to solve a time-fractional inverse diffusion problem in the frequency domain. The Landweber regularization method, see in ^[38,39]. In ^[40], Binh and co-authors studied an inverse source problem for the Rayleigh–Stokes problem using the Tikhonov method. In ^[41], Ma and his group identified the unknown space-dependent source term in a time-fractional diffusion equation by applying the generalized and revised generalized Tikhonov regularization methods.

However, to the best acknowledgment, the Tikhonov regularization method is very famous. Over the past decade, some modified Tikhonov methods have begun to be researched by mathematicians, such as the Fractional Tikhonov regularization method used in this article was considered by Smina Djennadi and his colleagues, see ^[42], and the generalized Tikhonov method can be found in reference ^[41]. For the reader's convenience, we would like to outline the main results:

● We give the ill-posedness of our inverse source problem.

● The first goal of this paper is to provide the fractional Tikhonov method to solve this inverse space-dependent source problem for the fractional pseudo-parabolic equation.

● The second goal of this paper is to provide the A generalized Tikhonov to solve the (1.1).

● And then finally, we provide an example of the illustration of the correctness of our theory and conclude this article.

The layout of this article is as follows. We show the preliminaries and Lemma is used. In Section 3, we have the mild solution of Problem (1.1) and the condition stability for Problem (1.1) in Theorem 3.1. In Sections 4 and 5, we have the error estimate between the exact solution and its approximation by the Fractional Tikhonov and The generalized Tikhonov method. In Section 6, we have one example numerical experiment.

2.   Preliminaries

Let us recall that the spectral problem

admits a family of eigenvalues

For all $r\geq 0$, we define by $A^{r}$ the following operator

The domain $\mathcal{H}(A^{r})$ is the Banach space equipped with the norm

Definition 2.1 (^[43]). The definition of the Mittag-Leffler function as follows

where $\alpha, \beta$ are arbitrary constants.

Lemma 2.1. (^[43]) Let $0 < \alpha < 1$. Then there exist positive constants $\mathcal A_{1}, \mathcal A_{2}, \mathcal A_{3}$ such that

Lemma 2.2. For $\lambda > 0, \alpha > 0$ and $m \in \mathbb N$, then

Lemma 2.3. ^[33] For $t > 0$, and $\lambda > 0$, and $0 < \alpha < 1$, we get

Lemma 2.4. For $\alpha \in (0, 1)$, we put $\mathcal{D}_{\alpha}(\lambda_{n}, k) = \xi_{n} \alpha \mathcal{L}(\alpha) \big(\mathcal{L}(\alpha) + \lambda_{n} \xi_{n}(1-\alpha) \big)^{-2}$, it gives

Proof. First of all, we notice that $\lambda_{n} \xi_{n} = \frac{\lambda_{n}}{1+k\lambda_{n}} = \frac{\lambda_{n}}{ \lambda_{n} \big(\frac{1}{\lambda_{n}} + k\big) } \geq \frac{1}{ \frac{1}{\lambda_{1}} + k} = \frac{1}{ \lambda_{1}^{-1} + k}$, and we have

Lemma 2.5. Let $\alpha \in (0, 1)$, by denoting

and $\xi_{n} = (1+k\lambda_{n})^{-1}$, then we get the following estimate

Proof. For $E_{\alpha, \alpha}(-y) \geq 0$ for $0 < \alpha < 1$ and $y \geq 0$, and using Lemma 2.1, we obtain

Lemma 2.6 (^[42]). For constant $\mathcal{N} > 0$, $s \geq \lambda_{1}$, and $\frac{1}{2} \leq \sigma < 1$, then we get

where $\mathcal{C}_{2} = \mathcal{C}_{2}(\sigma, \mathcal{N}) > 0$ is independent on $s$ and $\sigma$.

Lemma 2.7 (^[42]). For constant $s \geq \lambda_{1}$, with $\frac{1}{2} \leq \sigma < 1$, we obtain

where $\mathcal{C}_{4} = \mathcal{C}_{4}(\sigma, r, \mathcal{N})$ and $\mathcal{C}_{5} = \mathcal{C}_{5}(\sigma, r, \mathcal{N}, \lambda_{1})$.

Lemma 2.8 (^[42]). For constant $s \geq \lambda_{1} > 0$, and $\frac{1}{2} \leq \sigma < 1$, we get

Lemma 2.9. Let $\theta_{0}, \theta_{1}$ are positive constants such that $\theta_{0} < \theta < \theta_{1}$. By choosing $\epsilon \in \Big(0, \dfrac{\theta_{1}}{4}\Big)$, and $B(\theta_{0}, \theta_{1}) = \theta_{1} + \dfrac{\theta_{0}}{4}$, we obtain

Proof. This provision was detailed in ^[5]. We omit it here.

3.   The inverse source problem in $L^{2}$ case

In this section, we consider a linear problem as follows

where $u_0$ and $F$ are given functions. Let $u(t, x) = \sum_{n = 1}^\infty u_{n} (t) e_n(x)$ be the Fourier series in $L^2(\Omega)$ with $u_n(t) = \int\limits_{\mathcal{D}} u(t, x){e}_{n}(x)dx$, then we have the fractional integro-differential equation involving the Atangana-Baleanu fractional derivative in the form

along with the following condition $u_{n}(0) = \int\limits_{\mathcal{D}} u(0, x) \mathit{e}_{n}(x)dx$, and $F_{n}(0) = \lambda_{n} u_{n}(0)$, using Lemma $3.4$ in ^[43], the solution (3.1) is written by Fourier series as follows

From now on, for a shorter, we put $\xi_{n} = \big(1+k\lambda_{n} \big)^{-1}$, this implies that

Let $t = T$ and noting that $u(0, x) = 0$, $F(t, x) = \theta(t)f(x)$, and $\theta(T) = 0$, we find that

Let us denote and (2.10), we have

From (3.5) and (3.6), this leads to

and we can rewrite the formula (3.8) as follows:

and

with $\mathcal{D}_{\alpha}(\lambda_{n}, k)$ are defined as (3.6).

3.1.   The ill-posedness of problem (1.1)

Theorem 3.1. The inverse problem $(1.1)$ is ill-posed.

Proof. A linear operator $\mathcal{K}:L^{2}(\mathcal{D}) \to L^{2}(\mathcal{D})$ as follows.

where

It is obvious that $p(x, \xi) = p(\xi, x)$, we know that $\mathcal{K}$ is self-adjoint operator. Next, its compactness is considered and the finite rank operators as follows:

From (3.8), we find that the problem of seeking $f(x)$ can be transformed into the following operator equation:

where $\mathcal{K}_{N} : f \to g$ is a linear operator. To prove that $\mathcal{K}_{N}$ is a compact operator, defining $\mathcal{K}_{N}$ the finite rank operator as

Using the estimate of Lemma 2.5, we notice that $\mathcal{D}_{\alpha, \beta}(\lambda_{n}, k)\; \int\limits_{0}^{T}G_{\alpha, \beta}(\lambda_{n}, k, s)\theta(s)ds \leq \dfrac{\theta_{1} }{\lambda_{n}} \cdot$

So, the $\big\|\mathcal{K}_{N} f - \mathcal{K} f \big\|_{L^{2}(\mathcal{D})}$ can be bounded as follows:

Hence, $\big\| \mathcal{K}_{N} f - \mathcal{K} f \big\|_{L^{2}(\mathcal{D})} \to 0$ in the sense of $L(L^{2}(\mathcal{D}), L^{2}(\mathcal{D}))$as $N \to \infty$ and $\mathcal{K}$ is a linear operator. Next, we show one example to illustrate the non-well posed of problem (1.1). Considering the input final data $g_{m}(x) = \mathit{e}_{m}(x) (\lambda_{m})^{-\frac{1}{2}}$ and $g_{m} = 0$ and the corresponding source solution $f_{m}(x) = \mathit{e}_{m}(x) \; \Big(\sqrt{\lambda_{m}} \mathcal{D}_{\alpha}(\lambda_{m}, k) \int\limits_{0}^{T} G_{\alpha}(\lambda_{m}, k, s)\theta(s)ds \Big)^{-1}$ and $f(x) = 0$. The error estimate in $L^{2}$ between $g_{m}$ and $g$ is

Applying Lemma 2.5, then the corresponding error between source $f_{m}$ and $f$ is

From the condition (3.15) and (3.16), we conclude that the problem (3.13) is non-well posed.

3.2.   The conditional stability of the solution for problem (1.1)

Theorem 3.2. Let $r > 0$, and $f \in \mathcal{H}(A^{r}(\mathcal{D}))$ such that

Then we have

where

Proof. This theorem can refer similarly in ^[43]. We omit here.

4.   Fractional Tikhonov regularization method and error estimate

To get the regularized solution with the given data $g_{\epsilon}(x) \in L^{2}(\mathcal{D})$, minimizing the quantity $J^{\sigma}_{[\gamma(\epsilon)]}(f) \in L^{2}(\mathcal{D})$ such that

whereby $[\gamma(\epsilon)]$ is a regularization parameter, and $\|.\|_{\sigma}$ is a weighted seminorm defined as $\|\xi\|_{\sigma} = \big\|\mathcal{W}^{\frac{1}{2}} \xi \big\|_{L^{2}(\mathcal{D})}$ for any $\xi$ with $\mathcal{W} = \big(\mathcal{K}^{*} \mathcal{K} \big)^{\sigma-1}$ some of parameter $\frac{1}{2} \leq \sigma < 1$ called the fractional parameter. In here, we have some reviews as follows:

● In case $\sigma = \frac{1}{2}$, it is the quasi-boundary value method, interested readers can find a view in the document ^[42].

● In case $\sigma = 1$, it is classical Tikhonov regularization method, this method is fully described in the following documents ^[33].

● For $\frac{1}{2} < \sigma < 1$, it is the new fractional Tikhonov regularization.

The minimizer $f$ satisfy the norm equation

Using the singular decomposition for the compact self-adjoint operator, we get

For the observed data, we have

Next, with two formulas of regularized in (4.3) and (4.4), we consider the convergent rate of $\|f - f^{\sigma}_{ \varepsilon, \gamma(\varepsilon)}\|_{L^{2}(\mathcal{D})}$, by discussing how to select the regularization parameter, with a-priori strategies.

4.1.   A priori parameter choice rule

In this subpart, under a suitable choice for the regularization parameter $[\gamma(\epsilon)]$, we will give an error estimate for $\|f - f^{\sigma}_{\epsilon, \gamma(\epsilon)}\|_{L^{2}(\mathcal{D})}$.

Lemma 4.1. Assume that the condition $(1.3)$ holds, then we have

Proof. To prove this Lemma, we have used two formulas (4.3), (4.4), for $\dfrac{1}{2} \leq \sigma < 1$, we have

Now, we need establish the upper bound of $\big\| f^{\sigma}_{\gamma(\epsilon)} - f^{\sigma}_{ \varepsilon, \gamma(\varepsilon)} \big\|_{L^{2}(\mathcal{D})}$. For convenience, we consider the following step.

$\underline {Step\; \mathit{1:}}$ Estimate of $E_{1}$, with $\mathcal{V}$ depends on $\alpha, \lambda_{1}, k, T, \mathcal{A}_{2}$ and $\|g_{\epsilon}-g\|_{L^{2}(\mathcal{D})} \leq \epsilon$, applying Lemma 2.6 with $s = \lambda_{n}$, we get

whereby $\mathcal{V}_{\alpha}$ is defined as (3.19) and $\mathcal{C}_{2}$ depends on $\mathcal{V}, \sigma$ and $\mathcal{B}$.

$\underline {Step\; \mathit{2:}}$ Estimate of $E_{2}$, applying the inequality $(a+b)^{2} \leq 2a^{2}+2b^{2}$, after simple transformation steps, we have

From (4.8), the right hand side can be bounded as follow:

Combining (4.6) to (4.9), we have

Lemma 4.2. Suppose that $f \in \mathcal{H}^{ r}(\mathcal{D})$, then it gives

Proof. From (3.8) and (4.3), we received

Due to Lemma 4.2, the upper bound of the right hand side of (4.12) as follows:

● If $r \in (0, 2\sigma)$, then we have

● If $r \geq 2 \sigma$, then we have

Theorem 4.1. Assume that the noise assumption $(1.3)$ are hold, and the a-priori bound condition $(3.17)$. Then we have the following estimate:

● If $r \in (0, 2\sigma)$, by choosing $[\gamma(\epsilon)] = \Big(\dfrac{\epsilon}{E}\Big)^{\frac{2\sigma}{r+2}}$, then we have

where $\mathcal{P}_{1}$ depends on $\mathcal{C}_{2}, E, \theta_{0}, \theta_{1}, \mathcal{V}_{\alpha}, \mathcal{C}_{4}.$

● If $r \geq 2\sigma$, by choosing $[\gamma(\epsilon)] = \Big(\dfrac{\epsilon}{E}\Big)^{\frac{\sigma}{\sigma+1}}$, then we have

and $\mathcal{P}_{2}$ depends on $\mathcal{C}_{2}, E, \theta_{0}, \theta_{1}, \lambda_{1}, \mathcal{C}_{5}$.

Proof. Using the triangle inequality, we get

From (4.3), (4.4), results from Lemma 4.1 and Lemma 4.2, Theorem 4.1 is proven.

5.   A generalized Tikhonov regularization method

5.1.   A priori parameter choice rule

From ^[41], we have a stable solution to problem (1.1) with the observed data $\big(g_{\epsilon}, \theta_{\epsilon}\big)$ by generalized Tihkhonov regularization method which minimizes the quantity

Let $f_{\epsilon, [\gamma(\epsilon)]}$ be a solution of the problem (5.1) which satisfies

Using the SVD for compact self-adjoint operator, we get

and

Lemma 5.1. Assume that $f \in \mathcal{H}^{ r}(\mathcal{D})$ holds, assume that the couple $(g, \theta)$ is noised by $(g_{\epsilon}, \theta_{\epsilon})$ such that the condition $(1.3)$ satisfies, then we have

Proof. From (5.3) and (5.4), we have

whereby $\mathcal{Z}_{1}, \mathcal{Z}_{2}$ and $\mathcal{Z}_{3}$ are defined in (5.7) as follows:

The proof falls naturally into three parts.

$\underline {Step\; \mathit{1:}}$ Estimate of $\mathcal{Z}_{1}$, we have

$\underline {Step\; \mathit{2:}}$ Estimate of $\mathcal{Z}_{2}$, it gives

$\underline {Step\; \mathit{3:}}$ Estimate of $\mathcal{Z}_{3}$, we have

Combining (5.8)–(5.10), we get

Lemma 5.2. Suppose that $f \in \mathcal{H}^{ r}(\mathcal{D})$, then we have

● If $r > 1$, then we have

● If $r \in (0, 1)$, then we have

whereby $\mathcal{R}_{1}(\theta_{0}, \mathcal{V}_{\alpha}, E) = \big(\big(2\theta_{0} \mathcal{V}_{\alpha} \big)^{-2} E^2 + E^2 \big)$ and $\mathcal{R}_{2}(\lambda_1, r, \theta_{0}, \mathcal{V}_{\alpha}, E) = \dfrac{ \lambda_{1}^{ (1-r)} }{ 2 \theta_{0} \mathcal{V}_{\alpha} }E$.

Proof. From (5.4) and (3.8), we get:

Hence, $\mathcal{W}(n)$ is given by

Next, Estimate of $\mathcal{V}(n)$, we have

We divide into two following cases

● If $r \ge 1$, then we have

● If $r \in (0, 1)$, then we consider $\lambda_{n}^{1-r}$ satisfies the following two cases :

then we rewrite $\mathbb{N}$ by $\mathbb{N} = \mathcal{Q}_{1} \cup \mathcal{Q}_{2}$ where

From $(5.15)$ and $(5.18)$, we have:

Choose $\ell = \frac{1-r}{2(1+r)}$, we have

Theorem 5.1. Let $\theta_{\epsilon}, \theta \in L^{\infty}(0, T)$ such that $\|\theta_{\epsilon} - \theta\|_{L^{\infty}(0, T)} \leq \epsilon$ and $(\theta, \theta_{ \varepsilon})$ satisfy the condition in Lemma $(2.9)$, and the noise assumption $(3.17)$ hold. By choosing the regularization parameter $[\gamma(\epsilon)] = \Big(\dfrac{\epsilon}{E}\Big)$, we receive

● If $r > 1$, then we have

● If $r \in (0, 1)$, then we have

whereby $\mathcal{R}_{1}(\theta_{0}, \mathcal{V}_{\alpha}, E) = \big(\big(2\theta_{0} \mathcal{V}_{\alpha} \big)^{-2} E^2 + E^2 \big)$ and $\mathcal{R}_{2}(\lambda_1, r, \theta_{0}, \mathcal{V}_{\alpha}) = \dfrac{ \lambda_{1}^{ (1-r)} }{ 2 \theta_{0} \mathcal{V}_{\alpha} }$.

Proof. By the triangle inequality, we have

Combining Lemma 5.1 and Lemma 5.2, we have

● If $r > 1$, then we have

● If $r \in (0, 1)$, then we have

This theorem is proven.

6.   Simulation

In this section, we present one numerical example. By choosing $\Omega = (0, \pi)$, $T = 1$, $\alpha = 0.95$, and $\sigma = 0.45$, and $r = 0.25$ $k = 0.01$ are shown in this section, respectively. For computing the generalized Mittag-Leffler function and the accuracy control in computing is $10^{-10}$, we have the Matlab codes given by Podlubny ^[44]. To do this, we consider the problem as follows:

where $\text{ABC}_{0} D_{t}^{\alpha}u(x, t)$ is the Atangana-Baleanu fractional derivative is given by (1.2). In this calculation, we chose the operator $\Delta u = \dfrac{\partial^2}{\partial x^2} u$, we have chosen $\lambda_n = n^2, \; n = 1, 2, ...$ and $\mathit{e}_n(x)$ = $\sqrt{\dfrac{2}{\pi}}\sin(nx)$, respectively. We have the function

In general, the whole numerical procedure is summarized in the following steps:

$\underline {{\bf{Step}}\; {\bf{1:}}}$ Finite difference to discretize the time and spatial variable for $x \in (0, \pi)$ as follows:

$\underline {{\bf{Step}}\; {\bf{2:}}}$ The input data $(g, \theta)$ is noised by observation data $(g_{ \varepsilon}, \theta_{ \varepsilon})$ such that

$\underline {{\bf{Step}}\; {\bf{3:}}}$ The relative error estimation is defined by

where $\text{Error}_{1}$ is the error estimate between the exact solution and the regularized solution by the Fractional Tikhonov method, and $\text{Error}_{2}$ is the error estimate between the exact solution and the regularized solution by generalized Tikhonov method. In addition, taking for the priori parameter choice rule of $E$ is large enough. By ^[43], we get

From (6.5), by replacing $\beta = \alpha$, and $z = -\frac{\alpha \frac{n^{2}}{1+kn^{2}} }{\mathcal{L}(\alpha) + \frac{n^{2}}{1+kn^{2}}(1-\alpha)}$, we can find that

From (3.9), we have the exact solution

From (4.4), by choosing the regularization parameter $\gamma(\epsilon) = \Big(\dfrac{\epsilon}{E}\Big)^{\frac{1}{2}}$, with $N$ is a truncation number large enough, we have the regularized solution with Fractional Tikhonov as follows

From (5.3), with the Generalized Tikhonov method, and $\gamma(\epsilon) = \frac{\epsilon}{E}$, we receive

and $\mathcal{D}_{\alpha}(n^{2}, k)$ is defined in Lemma $2.4$. From (6.6), the integral $\int_{0}^{1} G_{\alpha}(n^{2}, k, s) \theta_{\epsilon}(s) ds$ calculated

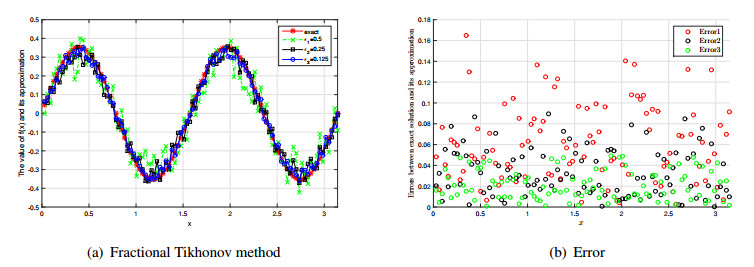
In these calculations, we choose $N = 100$ and $\epsilon = 0.5$, $\epsilon = 0.25$ and $\epsilon = 0.125$. Figure 1(a) shows the 2D graphs of the source function with the exact solution and its approximation for the case by the Fractional Tikhonov method. Figure 1(b) shows the error estimate between the exact solution and regularized solution for the case by the Fractional Tikhonov method. Figure 2(a) shows the 2D graphs of the source function with the exact solution and its approximation for the case by the generalized Tikhonov method. Figure 2(b) shows the error estimate between the exact solution and regularized solution for the case by the generalized Tikhonov method, respectively. Figure 3 shows the 2D graphs of the source function and its approximation, by two methods with $\epsilon = 0.5$, $\epsilon = 0.25$ and $\epsilon = 0.125$, respectively. The error estimates between the source function with the exact data and the measurement data are presented in Table 1. From the observations above, the approximation results are acceptable.

7.   Conclusions

In this study, we applied the Fractional Tikhonov method and the Generalized Tikhonov method to regularize the inverse problem to identify an unknown source term in fractional diffusion equations involving fractional derivative with Atangana-Baleanu Caputo derivative. This problem (1.1) is ill-posed in the sense of Hadamard. So, Under a priori parameter choice rule, we show the result for the convergent estimate between the sought solution and the regularized solution. Finally, we show an example to illustrate our proposed regularization.

Acknowledgements

This research is supported by Industrial University of Ho Chi Minh City (IUH) under grant number 130/HD-DHCN. Le Dinh Long is thankful to Van Lang University.

Conflict of interest

The authors declare no conflict of interest.