Fuzzy fractional estimates of Swift-Hohenberg model obtained using the Atangana-Baleanu fractional derivative operator

1.   Introduction

Recently, some researchers in statistics have been interested in generating new flexible statistical models based on different techniques such as the weight distributions which are commonly used in many fields of life situations such as sciences, ecology, biostatistics, medicine, engineering, pharmacy and environment and so on.

The concept of weighted distribution is suggested by ^[15] to study how verification methods can affect the form of the distribution of recorded observations. Later, the weighted distributions are unified and formulated by ^[31] in general terms in connection with modeling statistical data.

Suppose X is a non-negative random variable with a probability density function (pdf) $f(x)$. Let $W(x)$ be a non negative weight function, then the probability density function of the weighted random variable $X_{w}$ is given by:

If the weight function has the form $W(x) = x^{\lambda}$, the resulting distribution is known as a size biased distribution of order $\lambda$ with pdf given by:

If $\lambda$ = or 2, the yielded distributions are known as the length biased and area biased distributions, respectively.

For example, the length-biased Suja distribution is proposed by ^[5] as a generalization of the Suja distribution. The size biased Ishita distribution is offered by ^[6] as a new modification of the Ishita distribution. The Marshall-Olkin length-biased exponential distribution is suggested by ^[18]. The length-biased weighted generalized Rayleigh distribution with its properties is proposed by ^[2]. The weighted Lomax distribution is introduced by ^[23]. Other types of distributions are also suggested based on other procedures as ^[8] for the exponentiated new Weibull-Pareto distribution. The Topp-Leone Mukherjee-Islam distribution is offered by ^[7]. Also, see ^{[27,16,17,26]}.

To the best of our knowledge, the use of the weighted method to extend the generalized Quasi Lindley distribution introduced by ^[9] is still unexplored in the literature. In this study, the weighted generalized Quasi Lindley distribution is proposed. Indeed, the importance of the suggestion of the WGQLD arises from the fact it is a modification of the well known Quasi Lindley distribution which is considered by many researchers in different life situations.

The layout of this paper is organized as follows. Section 2 concerns with the pdf and cumulative distribution function (cdf) of the WGQLD and its shapes. Moment generating function and moments includes the $r$th moment, variance, the coefficient of skewness, kurtosis, and variation are presented in Section 3 theoretically and supported by some simulations. The distribution of order statistics, median deviations and harmonic mean are presented in Section 4. The stochastic ordering, reliability, hazard, reversed hazard rate and odds functions are given in Section 5. Bonferroni and Lorenz curves as well as the Gini index are provided in Section 6. In Section 7, different methods of estimation for the distribution parameters are discussed including maximum likelihood, maximum product of spacing's, ordinary least squares, weighted least squares, Cramer-von-Mises, and Anderson-Darling methods. A simulation study is conducted to compare the performance of the proposed estimators in Section 8. Illustrative examples of real data applications are given in Section 9. The paper is concluded with some suggestions for future works in Section 10.

2.   The WGQLD distribution

This section introduces the pdf and cdf of the WGQLD along with the shapes of the model. The probability density function of the generalized Quasi Lindley distribution is given by:

and the corresponding cdf is:

With reference to Eq 1.1, and in this work without loss of generality we considered the weight function as $W(x) = x$ given that the mean of the GQLD is $\mathbb{E}(X) = \frac{2(2+\alpha)}{\theta(1+\alpha)}$. Hence, the weighted generalized Quasi Lindley distribution can be obtained by substituting $f_{GQLD}(x; \theta, \alpha)$, $E(X)$ and $W(x)$ in Eq 1.1 to get:

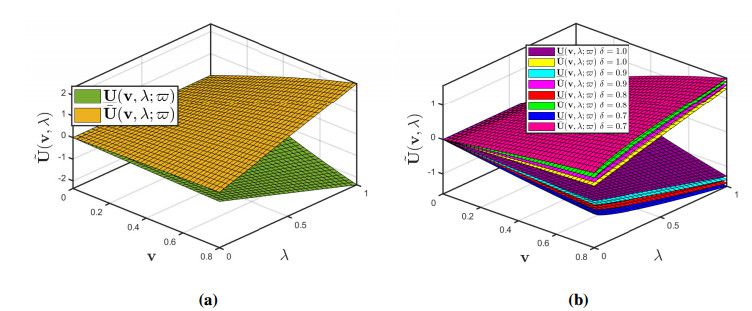
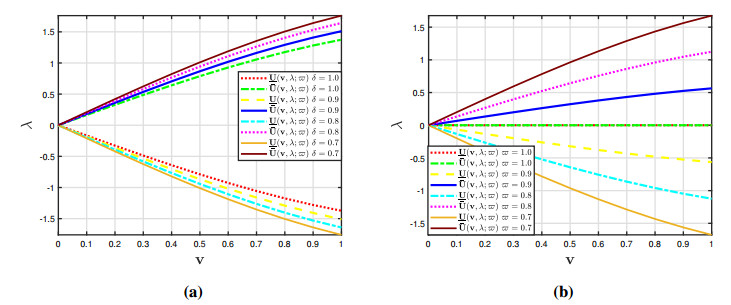
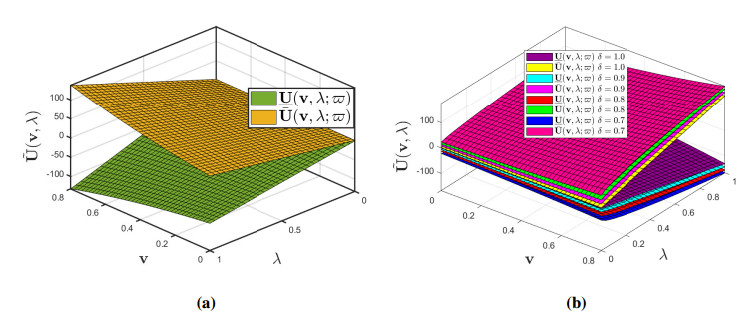
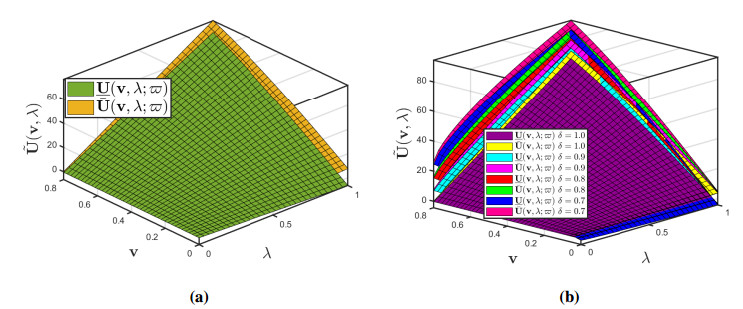
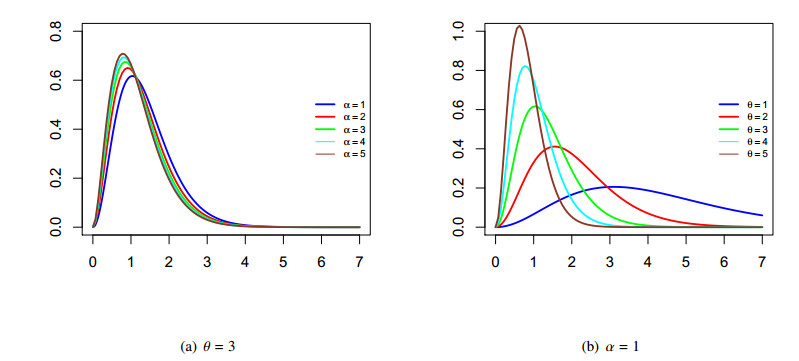
Figures 1 and 2 demonstrate the graphs of the pdf of the WGQLD for different values of $\theta$ and $\alpha$. Also, Figures 3 and 4 show various curves of the cdf of WGQLD for selected distribution parameters.

Based on Figures 1 and 2, it can be observed that the WGQLD is a unimodel and positively skewed. Also, it is approximately symmetric as in Figure 2 when $\theta = 1$ for some parameters. The curve of the distribution function is more flat for $\alpha = 1, \theta = 0.25$ and when $\alpha = \theta = 1.$ than other values.

The corresponding cdf equation of the WGQLD is given by

Figure 3 reveals that the cdf plots are approach 1 when $\theta = 3$ (a) faster than that of $\alpha = 1$ (b). In comparing Figure 3(b) with Figure 4(b), it can be observed that the cdf plots are more spread with smaller values of $\theta$. However, the same thing can be concluded in comparing Figure 3(a) with Figure 4(a).

3.   Moments and some related measures

Theorem 1. : Let $X\thicksim f_{WGQLD}(x, \theta, \alpha)$, then the $r$th moment of $X$ about the origin is:

Proof. : The proof is direct by using $E(X^{r}) = \int_{0}^{\infty}x^{r}f(x, \theta, \alpha)dx$ as

Based on Eq 7, it is simple to have the first, second, third and fourth moments of the WGQLD, respectively, as

Therefore, the variance of the WGQLD can be obtained as

The distribution shape analysis can be performed by studying the coefficient of skewness, coefficient of kurtosis, and coefficient of variation. For the WGQLD, these coefficients, respectively, are given by:

It is of interest to note here that the coefficient of skewness, coefficient of variation and coefficient of kurtosis are free of $\theta$. Table (1) presents some values of the mean, standard deviation, coefficient of variation, coefficient of skewness and coefficient of kurtosis of WGQLD for various values of the parameters $\alpha$ and $\theta$.

It can be noted that the mean and the standard deviation are decreasing when values of $\alpha$ and $\theta$ are increasing for fixed values of $\theta$ and $\alpha$, respectively. The results of simulation emphasize that the coefficient of skewness, coefficient of variation and coefficient of kurtosis don't depend on $\theta$ and increasing when $\alpha$ values are increasing.

The moment generating function (MGF) of the WGQLD is given in the following theorem.

Theorem 2. : Let $X\thicksim f_{WGQLD}(x, \theta, \alpha)$, then the MGF of $X$ about the origin is:

Proof. : To prove the MGF of the WGQLD, let

4.   Order statistics, median deviations and harmonic mean

4.1.   Order statistics

Let $X_{1}, X_{2}, \ldots, X_{m}$ be a random sample of size $m$ from a distribution with pdf $f(x)$ and cdf $F(x)$. The pdf of the $i$th order statistic $X_{(j:m)}$ for $j = 1, 2, \ldots, m$ are defined by ^[14] as

Based on Eq (4.1), the pdf of the $i$th order statistic, $X_{(i:m)}$, from the WGQLD, will be

where

and

Based on Eq (18), the pdf of the minimum and maximum order statistics, respectively, are given by

where $B = \left\{ {6\alpha (\theta x(\theta x(\theta x + 3) + 6) + 6) + \theta x(\theta x(\theta x(\theta x + 4) + 12) + 24) + 24} \right\}$, and

where

.

4.2.   Median deviations

To measure the scatter in the population, the mean deviation about the median denoted by $\mathbb{MD}(x)$ can be used, where

where $M$ is the population median. The mean deviation about the median for the WGQL distribution is defined as:

4.3.   Harmonic mean

Let $X\thicksim f_{WGQLD}(x, \theta, \alpha)$, then

Therefore, the harmonic mean of the WGQLD is given by

Table 2 presents some values of the harmonic mean of WGQLD for various values of the parameters $\theta$ and $\alpha$.

Based on Table 2, we can conclude that: the harmonic mean values are decreasing in $\alpha$ when $\theta = 5$ and are decreasing in $\theta$ when $\alpha = 2$. Also, in general the harmonic mean values when $\theta < \alpha$ are larger than the case of $\theta > \alpha$.

5.   Stochastic ordering and reliability analysis

5.1.   Stochastic ordering

The stochastic ordering is an important tool in finance and reliability theory to evaluate the comparative behaviour of the models or systems. Let the random variables $X$ and $Y$ having the probability density functions, cumulative distribution functions and survival functions $f(x), f(y), F(x), F(y), \overline{F}(x) = 1-F(x)$, and $\overline{F}(y) = 1-F(y)$, respectively, then $X\leq Y$ in:

1. Mean residual life order denoted by $X\leq_{MRLO} Y$, if $m_{X}(x)\leq m_{Y}(x)$ for all $x,$

2. Hazard rate order denoted by $X\leq_{HRO} Y$, if $\overline{F}_{X}(x)/\overline{F}_{Y}(x)$ is decreasing in $x\geq 0,$

3. Stochastic order denoted by $X\leq_{SO}Y$, if $\overline{F}_{X}(x)\leq\leq \overline{F}_{Y}(x)$ for all $x,$

4. Likelihood ratio order denoted by $X\leq_{LRO} Y$, if $\frac{fx(x)}{f_{Y}(x)}$ is decreasing in $x\geq 0.$

All these stochastic orders defined above are related to each other and ^[29] showed the following relation is hold.

Theorem 3. : Let the random variables $X$ and $Y$ be independent follow the pdf $f_{X}(x, \theta, \alpha)$ and $f_{Y}(x, \mu, \omega)$, respectively. If $\theta > \mu$ and $\alpha > \omega$, then $X\leq_{LRO}Y, X\leq_{HRO}Y, X\leq_{MRLO}Y$ and $X\leq_{SO}Y.$

Proof: Let $X\thicksim f_{X}(x, \theta, \alpha)$, $Y\thicksim f_{Y}(x, \mu, \omega)$, then

and

Taking the derivative of the last equation with respect to $x$ yields

Hence, if $\theta > \mu$, $\alpha > \omega$, then $\frac{d}{dx}\log(\frac{f_{X}(x, \theta, \alpha)}{f_{Y}(x, \mu, \omega)}) < 0.$ Therefore, $X\leq_{LRO}Y$, $X\leq_{HRO}Y$, $X\leq_{MRLO}Y$ and $X\leq_{SO}Y$.

5.2.   Reliability analysis

The corresponding reliability and hazard functions of the WGQLD distribution are given, respectively by:

and

Figure 5, reveals that the reliability of the WGQLD are decreasing while the hazard rate function is increasing for $\alpha = 1, 2, \ldots, 5$. The reversed hazard rate and odds functions for the WGQLD distribution, respectively, are defined as

and

As it is shown in Figure 6, the reversed hazard function is decreasing taking inverse J shape while the odds functions is increasing with J shape.

6.   Bonferroni and Lorenz curves and Gini index

Assume that the random variable $X$ is a non-negative with continuous and twice differentiable cumulative distribution function $F(x)$. The Bonferroni curve of the random variable $X$ is defined as

where $q = F^{-1}(p)$ and $p\in(0, 1$]. The Lorenz curve is defined as

The Gini index is given by

Now, for the WGQL distribution, the Bonferroni curve, Lorenz curve and Gini index are given, respectively, as

Table 3 presents some values of the Gini Index for WGQLD for different values of $\alpha$

Table 3 explains that the Gini index values for the WGQL distribution are increasing as $\alpha$ values are increasing, and on the average its value is about 0.31.

7.   Methods of estimation

In this section, we consider six methods of estimation for estimating the unknowns parameters $\alpha$ and $\theta$ of the WGQLD distribution. These methods include the (1) maximum likelihood (ML) method, (2) method of maximum product of spacings, (3) ordinary least square method, (4) weight least square method, (5) method of Cramer-Von-Mises, and (6) method of Anderson-Darling.

7.1.   Maximum likelihood estimation

First of all, we investigate the ML estimates (MLEs) of $\theta$ and $\alpha$. Let $X_{1}, X_{2}\ldots, X_{n}$ be a random sample of size $n$ selected from the WGQLD. The likelihood function is given by:

and the log-likelihood function $\Xi = \ln L\ (x; \theta, \ \alpha)$ is:

The derivatives of $\Xi$ with respect to $\theta$ and $\alpha$ are:

Since there is no closed form for these equations, then the MLEs $\hat{\theta}$ and $\hat{\alpha}$ of $\theta$ and $\alpha,$ respectively, can be solved simultaneously using a numerical method as Newton Raphson method.

7.2.   Method of maximum product of spacings

The maximum product of spacing (MPS) method is proposed by ^[11,12] as an alternative to the maximum likelihood method. The MPS method requires a maximization of the geometric mean of the spacings in the data with respect to the parameters. Consider a random sample of size $n$, $X_{1}, X_{2}, \ldots, X_{n}$ from WGQLD distribution, then uniform spacings is given as:

where $F(x_{0:n}|\theta, \alpha) = 0$ and $F(x_{n+1:n}|\theta, \alpha) = 1$. Clearly $\sum_{i = 1}^{n+1}\Upsilon_{i}(\theta, \alpha) = 1.$

The MPSs, $\hat{\alpha}_{MPS}$ and $\hat{\theta}_{MPS}$, are the values of $\alpha$ and $\theta$, which maximize the geometric mean of the spacing:

The natural logarithm of (7.5) is:

The MPSs estimators $\hat{\alpha}_{MPS}$ and $\hat{\theta}_{MPS}$ of the parameters $\alpha$ and $\theta$, respectively, can also be obtained by solving the nonlinear equations:

where

which can be obtained numerically.

7.3.   Methods of least squares

The least square methods are introduced by ^[28] to estimate the parameters of beta distribution. Let $X_{i:n}$ be the $i$th order statistic of the random sample $X_{1}, X_{2}, \ldots, X_{n}$ with distribution function $F(x)$, then a main result in probability theory indicates that $F(X_{i:n})\sim Beta(i, n-i+1)$. Moreover, we have

$E[F(X_{i:n})] = \frac{i}{n+1}$ and $\mathrm{V}\mathrm{a}\mathrm{r}[F(X_{i:n})] = \frac{i(n-i+1)}{(n+1)^{2}(n+2)}$.

Using the expectations and variances, we obtain two variants of the least squares methods.

7.3.1.   Ordinary least squares

For the WGQLD distribution parameters estimation, the ordinary least square estimators $\hat{\theta}_{OLS}$ and $\hat{\alpha}_{OLS}$ of the parameters $\theta$ and $\alpha$, respectively can be obtained by minimizing the function:

with respect to $\theta$ and $\alpha$. Alternatively, these estimates can also be obtained by solving the following nonlinear equations:

where $\Delta_{1}(x_{i:n}|\theta, \alpha)$ and $\Delta_{2}(x_{i:n}|\theta, \alpha)$ are defined as in 7.6 and 7.7, respectively.

7.3.2.   Weighted least squares

For the WGQLD distribution, the weighted least square estimators of $\theta$ and $\alpha$ say, $\hat{\theta}_{WLS}$ and $\hat{\alpha}_{WLS}$, respectively can be obtained by minimizing the function:

with respect to $\theta$ and $\alpha$. Equivalently, these estimators are the solution of the following nonlinear equations:

where $\Delta_{1}(x_{i:n}|\theta, \alpha)$ and $\Delta_{2}(x_{i:n}|\theta, \alpha)$ are specified as in 7.6 and 7.7, respectively.

7.4.   Methods of minimum distances

Here, we use two popular methods based on the minimization of test statistics between the theoretical and empirical cumulative distribution functions. The methods are Cramer-von-Mises method and the method of Anderson-Darling (for more details see ^[13] and ^[24]).

7.4.1.   Cramer-von-Mises method

The Cramer-von-Mises estimators (CVEs) $\hat{\theta}$ and $\hat{\alpha}$ of $\theta$ and $\alpha$ respectively, are obtained by minimizing the following function:

with respect to $\theta$ and $\alpha$. Equivalently, these estimators are the solution of the following nonlinear equations:

where $\Delta_{1}(x_{i:n}|\theta, \alpha)$ and $\Delta_{2}(x_{i:n}|\theta, \alpha)$ are given in 7.6 and 7.7, respectively.

7.4.2.   Method of Anderson-Darling

The Anderson-Darling (AD) estimates of the WGQLD distribution parameters $\theta$ and $\alpha$ denoted by $\hat{\theta}_{AD}$ and $\hat{\alpha}_{AD}$ can be obtained by minimizing the following function

with respect to $\theta$ and $\alpha$, or by solving the following two equations

and

where $\Delta_{1}(x_{i:n}|\theta, \alpha)$ and $\Delta_{2}(x_{i:n}|\theta, \alpha)$ are specified in 7.6 and 7.7, respectively.

8.   Simulation

This section compares the performances of the proposed estimators of the WGQLD parameters $\alpha$ and $\theta$. This comparison is carried out by taking random samples of different sizes ($n = 20$, 40, 60, 80,100 and 200) with various pairs of parameters values $(\theta, \alpha)$ = (0.25, 1), (0.5, 1.5), (0.75, 2), (1, 1), (0.3, 2), (0.2, 3), (0.8, 1), (1, 3). The estimators are compared in terms of there mean square errors (MSE) and the estimated (Es) values of the parameters. The results are summarized in the Tables 4–7.

Based on Tables 4–7 it is clear that:

● The MSEs values are decreasing as the sample sizes values are increasing for all cases considered in this section. As an example, for $\alpha = 1, \theta = 3$, with $n = 50$ based on the AD, the MSEs are 0.7566 and 0.0570 compared to 0.2669 and 0.0273 for $n = 100$, respectively.

● The bias values of the suggested estimators are decreasing as the sample sizes are increasing, and approaches zero for all cases for large n. For illustration, for $\alpha = 0.3, \theta = 2$, with $n = 100$ the Es values are 0.302 and 2.012 using the MPS as compared to 0.291 and 2.007 for $n = 200$, respectively.

● It can be observed that for most of the cases, the MLEs method has the smallest values of the MSEs among all methods of estimation.

9.   Application on real data

In this section, we use data sets to illustrate the usefulness of the WGQL model, where four different data sets are used related to the environment, engineering and two medical data sets are considered. We compare the WGQL distribution to some well known distributions of two parameters as the generalized Quasi Lindley distribution, Quasi Lindley distribution, two-parameter Sujatha distribution, and the Pareto distribution.

The first data set is taken from ^[22] represents the 100 annual maximum precipitation (inches) for one rain gauge in Fort Collins, Colorado, from 1900 through 1999. The data are given below:

Data Set 1: 239,232,434, 85,302,174,170,121,193,168,148,116,132,132,144,183,223, 96,298, 97,116,146, 84,230,138,170,117,115,132,125,156,124,189,193, 71,176,105, 93,354, 60,151,160,219,142,117, 87,223,215,108,354,213,306,169,184, 71, 98, 96,218,176,121,161,321,102,269, 98,271, 95,212,151,136,240,162, 71,110,285,215,103,443,185,199,115,134,297,187,203,146, 94,129,162,112,348, 95,249,103,181,152,135,463,183,241 5.

The second data set from ^[25] consists of 100 observations on breaking stress of carbon fibers (in Gba). The data are as follows:

Data Set 2: 3.7, 2.74, 2.73, 2.5, 3.6, 3.11, 3.27, 2.87, 1.47, 3.11, 4.42, 2.41, 3.19, 3.22, 1.69, 3.28, 3.09, 1.87, 3.15, 4.9, 3.75, 2.43, 2.95, 2.97, 3.39, 2.96, 2.53, 2.67, 2.93, 3.22, 3.39, 2.81, 4.2, 3.33, 2.55, 3.31, 3.31, 2.85, 2.56, 3.56, 3.15, 2.35, 2.55, 2.59, 2.38, 2.81, 2.77, 2.17, 2.83, 1.92, 1.41, 3.68, 2.97, 1.36, 0.98, 2.76, 4.91, 3.68, 1.84, 1.59, 3.19, 1.57, 0.81, 5.56, 1.73, 1.59, 2, 1.22, 1.12, 1.71, 2.17, 1.17, 5.08, 2.48, 1.18, 3.51, 2.17, 1.69, 1.25, 4.38, 1.84, 0.39, 3.68, 2.48, 0.85, 1.61, 2.79, 4.7, 2.03, 1.8, 1.57, 1.08, 2.03, 1.61, 2.12, 1.89, 2.88, 2.82, 2.05, 3.655.

Due to the importance of the studies about the Covid-19 in the last years, we considered two sets of Covid-19 related to Algeria and Saudi Arabia in various times. The third data set is the Covid-19 data for the daily new cases in Algeria from 12 August 2020 to 09 November 2020 and it is available on the following electronic address https://sehhty.com/dz-covid/. It is given as follows

Data Set 3: 642,670,581,631,642,548,405,302,330,291,319,306,320,287,276,263,250,273,276,252,213,214,199,205,221,193,185,174,153,132,136,146,138,121,129,134,141,148,157,160,162,155,146,153,160,175,179,186,191,197,203,210,219,228,232,238,242,247,255,264,272,278,285,289,293,298,304,311,325,339,348,365,378,387,397,391,370,398,392,401,409,411,403,419,442,450,469,477,488,495.

The box and TTT plots for the above data are given in Figures 7 and 8, respectively.

The fourth data set is calling the Covid-19 data which present the daily new cases in Saudi Arabia from 24 March 2020 to 24 April 2020 and it is given by

Data Set 4: 1, 1, 1, 0, 1, 4, 0, 2, 6, 5, 4, 4, 5, 4, 3, 0, 3, 3, 5, 7, 6, 8, 6, 4, 4, 5, 5, 6, 6, 5, 7, 6. The descriptive statistics for the data is given in Table 8.

The WGQLD distribution is fitted to these two real data sets and compared with the following models:

● The generalized Quasi Lindley distribution: $f(x) = \frac{{\theta}^2\left(\frac{{\theta}^2x^3}{6}+{\alpha}{\theta}x^2+{\alpha}^2x\right)\mathrm{e}^{-{\theta}x}}{\left({\alpha}+1\right)^2}.$

● Quasi Lindley distribution: $f(x) = \frac{\theta(\alpha+ x\theta)}{\alpha+1}\mathrm{e}^{-\theta x}.$

● The Pareto distribution: $f(x) = \frac{\alpha \theta^ \alpha}{x^{\alpha+1}}.$

● Two-parameter Sujatha distribution: $f(x) = \frac{{\theta}^3\left(x^2+{\alpha}x+1\right)\mathrm{e}^{-{\theta}x}}{{\theta}^2+{\alpha}{\theta}+2}.$

To choose the best model fitting, we considered Akaike information criterion (AIC) introduced by ^[1], Baysian information criterion (BIC) proposed by ^[30], Hannan Quinn Information Criterion (HQIC) suggested by ^[21], Consistent Akaike Information Criterion (CAIC) by ^[10], Kolmogorov-Smirnov (KS), where AIC = $-2L+k,$, CAIC = $-2L+2\frac{2kn}{n-k-1}$, HQIC = $2\log{\log(n)[k-2L]}$, BIC = $-2L+k\log(n)$, KS = $\sup|F_{n}(x)-F(x)|, F_{n}(x) = \frac{1}{n}\sum_{i}^{n}{ł}_{x_{i}\leq x}$, where k is the number of parameters and n is the sample size and L is the value of maximum log-likelihood function.

Based on the results reported in Tables 9, 10, 11 and 12, we observe that the WGQLD provides the better fit with the smallest values of AIC, AICc, BIC, HQIC and K-S with maximum P-values as compared to its competitive models considered in this study. Figures 9, 10, 11 and 12 support this claim.

10.   Conclusions

In this article, we proposed the WGQLD distribution along with some of its properties such as, stochastic ordering, Median deviation, Harmonic mean, some plots of the pdf and cdf, Bonferroni and Lorenz curves and Gini index moments, coefficient of variation, coefficient of skewness and coefficient of kurtosis. Also, the hazard rate function, reliability function, reversed hazard rate and odds functions are presented. The maximum likelihood estimates is computed as well as the maximum product of spacing's, ordinary least squares, weighted least squares, Cramer-von-Mises, and Anderson-Darling methods are obtained. The results show that the best method of estimation is the MLE method. Applications of various real data sets are analyzed for illustration. It is proved that the WGQLD is empirically better than other competitors models considered in this study including the base GQLD. Therefore, in the future, the authors intend to investigate the performance of different estimators of the WGQLD based on ranked set sampling method and its modifications, see ^{[3,4,19,20,32]}.

Conflict of interest

The author declare that they have no conflict of interest

Acknowledgement

The authors extend their appreciation to the deputy ship for research and innovation, "Ministry of Education" in Saudi Arabia for funding this research work through project No. IFKSURG-1438-086.