Soliton solutions for some nonlinear models in mathematical physics via conservation laws

F. A. Mohammed; F. A. Mohammed

doi:10.3934/math.2022826

AIMS Mathematics

2022, Volume 7, Issue 8: 15075-15093. doi: 10.3934/math.2022826

Previous Article Next Article

Research article Special Issues

Soliton solutions for some nonlinear models in mathematical physics via conservation laws

F. A. Mohammed ^{1,2
,
,}

1.
Department of Mathematics, College of Science and Arts, Jouf university, Al-Gurayat, Kingdom of Saudi Arabia
2.
Department of Mathematics, Faculty of Sciences, South Valley university, Qena, Egypt

Received: 06 April 2022 Revised: 18 May 2022 Accepted: 22 May 2022 Published: 15 June 2022
MSC : 70S10, 35Q53, 76M60

In this paper, we derive the soliton solutions from conserved quantities for the Benjamin-Bona-Mahoney equation with dual-power law nonlinearity (BBM), modified regularized long wave (MRLW) equation, modified nonlinearly dispersive KdV equations 2K(2, 2, 1) and 3K(3, 2, 2) equation, which are constructed by the multiplier approach (variational derivative method). Finally, we give the numerical simulations to illustrate this method.

Keywords:

Citation: F. A. Mohammed. Soliton solutions for some nonlinear models in mathematical physics via conservation laws[J]. AIMS Mathematics, 2022, 7(8): 15075-15093. doi: 10.3934/math.2022826

Related Papers:

[1]	Ghazala Akram, Maasoomah Sadaf, Mirfa Dawood, Muhammad Abbas, Dumitru Baleanu . Solitary wave solutions to Gardner equation using improved tan $\left(\frac{\Omega(\Upsilon)}{2}\right)$ -expansion method. AIMS Mathematics, 2023, 8(2): 4390-4406. doi: 10.3934/math.2023219
[2]	Naher Mohammed A. Alsafri, Hamad Zogan . Probing the diversity of kink solitons in nonlinear generalised Zakharov-Kuznetsov-Benjamin-Bona-Mahony dynamical model. AIMS Mathematics, 2024, 9(12): 34886-34905. doi: 10.3934/math.20241661
[3]	Imran Siddique, Khush Bukht Mehdi, Sayed M Eldin, Asim Zafar . Diverse optical solitons solutions of the fractional complex Ginzburg-Landau equation via two altered methods. AIMS Mathematics, 2023, 8(5): 11480-11497. doi: 10.3934/math.2023581
[4]	Noor Alam, Mohammad Safi Ullah, Jalil Manafian, Khaled H. Mahmoud, A. SA. Alsubaie, Hamdy M. Ahmed, Karim K. Ahmed, Soliman Al Khatib . Bifurcation analysis, chaotic behaviors, and explicit solutions for a fractional two-mode Nizhnik-Novikov-Veselov equation in mathematical physics. AIMS Mathematics, 2025, 10(3): 4558-4578. doi: 10.3934/math.2025211
[5]	Islam Samir, Hamdy M. Ahmed, Wafaa Rabie, W. Abbas, Ola Mostafa . Construction optical solitons of generalized nonlinear Schrödinger equation with quintuple power-law nonlinearity using Exp-function, projective Riccati, and new generalized methods. AIMS Mathematics, 2025, 10(2): 3392-3407. doi: 10.3934/math.2025157
[6]	Mohammad Alqudah, Safyan Mukhtar, Albandari W. Alrowaily, Sherif. M. E. Ismaeel, S. A. El-Tantawy, Fazal Ghani . Breather patterns and other soliton dynamics in (2+1)-dimensional conformable Broer-Kaup-Kupershmit system. AIMS Mathematics, 2024, 9(6): 13712-13749. doi: 10.3934/math.2024669
[7]	Naveed Iqbal, Muhammad Bilal Riaz, Meshari Alesemi, Taher S. Hassan, Ali M. Mahnashi, Ahmad Shafee . Reliable analysis for obtaining exact soliton solutions of (2+1)-dimensional Chaffee-Infante equation. AIMS Mathematics, 2024, 9(6): 16666-16686. doi: 10.3934/math.2024808
[8]	Nafissa T. Trouba, Huiying Xu, Mohamed E. M. Alngar, Reham M. A. Shohib, Haitham A. Mahmoud, Xinzhong Zhu . Soliton solutions and stability analysis of the stochastic nonlinear reaction-diffusion equation with multiplicative white noise in soliton dynamics and optical physics. AIMS Mathematics, 2025, 10(1): 1859-1881. doi: 10.3934/math.2025086
[9]	M. Ali Akbar, Norhashidah Hj. Mohd. Ali, M. Tarikul Islam . Multiple closed form solutions to some fractional order nonlinear evolution equations in physics and plasma physics. AIMS Mathematics, 2019, 4(3): 397-411. doi: 10.3934/math.2019.3.397
[10]	Zhi-Ying Feng, Xiang-Hua Meng, Xiao-Ge Xu . The data-driven localized wave solutions of KdV-type equations via physics-informed neural networks with a priori information. AIMS Mathematics, 2024, 9(11): 33263-33285. doi: 10.3934/math.20241587

Abstract

1. Introduction

In all areas of physics, conservation laws ^[1,2] are essential and important since they allow us to draw conclusions of a physical system under study in an efficient way. Knowledge of conservation laws are important for numerical integration of partial differential equations (PDEs). The existence of a large number of conservation laws for a nonlinear partial differential equation (system) is a strong indication of its integrability. Many powerful methods have been developed for the construction of conservation laws, such as the Noethers theorem ^[3] for variational problems, Laplaces Direct method ^[4], characteristic form introduced by Stuedel ^[5], multiplier approach ^[6,7], Kara and Mahomed ^[8] symmetry condition, partial Noether approach ^[9,10,11] and Cheviakov ^[12] developed powerful software packages to compute conservation laws for partial differential equations.

The multiplier approach (also known as the variational derivative method) was successfully applied to the construction of conservation laws for nonlinear partial differential equations ^{[13,14,15,16]}.

Soliton solutions for nonlinear partial differential equations (PDEs) are play a significant role in soliton theory ^[17]

In ^[18] the authors obtained the novel exact solutions in form of dark, bright, combined dark-bright, combined singular and other soliton solutions solitons for the meta materials model having third and fourth order dispersions, the authors in ^[19] obtained investigation for the optical solitons solution for the nonlinear Schr $\ddot{o}$ dinger equation in magneto-optic waveguides with anti-cubic nonlinearity and in ^[20] the author obtained the new exact soliton solutions for the two deformed nonlinear Schr $\ddot{o}$ dinger (NLS) type equations.

Based on He's variational approach ^[21,22], which depends on the Lagrange function and the finding of conservation laws of system of differential equations (DEs), which is often the first step towards finding the solution the variational formula from the conserved quantities for Benjamin-Bona-Mahoney equation with dual-power law nonlinearity (BBM), modified regularized long wave (MRLW) equation and modified nonlinearly dispersive KdV equations 2K(2, 2, 1) and 3K(3, 2, 2) equation and new kinds of solitary wave solutions for these models and numerical simulations were obtained in Sections 3–6. in Section 5. This method helps to explore the new soliton solutions for new phenomena of nonlinear evolution equations. So we proposed a new method to derive the functional from conserved quantities since it depends on the Lagrange function and its derivatives ^[23] and then find the soliton solutions by Ritz method ^[24].

The Benjamin-Bona-Mahoney equation with dual-power law nonlinearity (BBM) equation describes the dynamics of shallow water waves in the presence of advection, the modified regularized long wave (MRLW) equation was first introduced by Peregrine ^[25] to describe the development of an undular bore, the modified nonlinearly dispersive KdV equations 2K(2, 2, 1) equation and the modified nonlinearly dispersive KdV equations 3K(3, 2, 2) equation are derived from the well-known KdV equation which describes the mathematical modeling of traveling wave solutions, known as solitary water waves (also called solitons) in a shallow water domain.

The proposed method derived the soliton solutions from the conserved quantities for the models while the existing method derived the soliton solutions from the Lagrangian for the models. In ^[26], He's variational iteration method was applied to obtain the numerical solution for various kinds of Newell-Whitehead-Segel nonlinear diffusion equation. This paper is organized as follows: In Section 2, we give brief definitions related to the multiplier approach and main steps for deriving the soliton solutions. In Sections 3–6, we apply this method to Benjamin-Bona-Mahoney equation with dual-power law nonlinearity (BBM), modified regularized long wave (MRLW) equation and modified nonlinearly dispersive KdV equations 2K(2, 2, 1) and 3K(3, 2, 2) equation and give the soliton solutions by using a proposed method and the figures for each models to illustrate the properties of these solitons solutions. Finally, results are summarized in Section 7.

2. The proposed method

For giving an independent variable $t, x$ and dependent variable $u$

(1) The total derivative operator $D$ with respect to $t, x$ are

$\begin{array}{l} D_{t} = \frac{\partial}{\partial t}+u_{t}\frac{\partial}{\partial u}+u_{tt}\frac{\partial}{\partial u_{t}}+u_{tx}\frac{\partial}{\partial u_{x}}+u_{txx}\frac{\partial}{\partial u_{xx}}+......\\ D_{x} = \frac{\partial}{\partial x}+u_{x}\frac{\partial}{\partial u}+u_{xx}\frac{\partial}{\partial u_{x}}+u_{xt}\frac{\partial}{\partial u_{t}}+u_{xxt}\frac{\partial}{\partial u_{xt}}+...... \end{array}$

(2.1)

(2) The Euler operator is defined

$\begin{array}{l} \frac{\delta}{\delta u} = \frac{\partial}{\partial u}-D_{t}\frac{\partial}{\partial u_{t}}-D_{x}\frac{\partial}{\partial u_{x}}+D_{t}^{2}\frac{\partial}{\partial u_{tt}}+D_{x}^{2}\frac{\partial}{\partial u_{xx}}+D_{x}D_{t}\frac{\partial}{\partial u_{xt}}-D_{t}^{3}\frac{\partial}{\partial u_{ttt}}-..... \end{array}$

(2.2)

Consider a mth-order partial differential equation of $t, x$

$\begin{array}{l} E(t,x,u,u_{t},u_{x},u_{tt},u_{tx},u_{xx},......) = 0. \end{array}$

(2.3)

(3) A vector $T = (T^{1}, T^{2})$ such that

$\begin{array}{l} D_{t}T^{1}+D_{x}T^{2} = 0, \end{array}$

(2.4)

holds for all solutions of (2.3) is known as the conserved vector of (2.3).

(4) The multiplier $\Lambda$ for (2.3) has the property

$\begin{array}{l} D_{t}T^{1}+D_{x}T^{2} = \Lambda E, \end{array}$

(2.5)

for arbitrary function $u(x, t)$ ^[5,6]

(5) The determining equations for multiplier are obtained by taking the variational derivative of (2.5) ^[6]

$\begin{array}{l} \frac{\delta}{\delta u}(\Lambda E) = 0. \end{array}$

(2.6)

Equation (2.6) holds for arbitrary function $u(x, t)$ not only for solutions of (2.3). We can derive the conserved vectors using (2.5) after computing the multiplier from (2.6)

The main steps of the proposed method are given as follows:

For giving a nonlinear equation

Step 1. By using the wave transformation $u(x, t) = u(\zeta)$ , $\zeta = k x-\omega t+\zeta_0$ , we can convert Eq (2.3) into an ordinary differential equation (ODE)

$\begin{array}{l} H(u,-\omega u_{\zeta},k u_{\zeta},\omega^2 u_{\zeta\zeta},-k \omega u_{\zeta\zeta},k^2 u_{\zeta\zeta},.....) = 0. \end{array}$

(2.7)

Step 2. Equation (2.4) can be converted into

$\begin{array}{l} D_{\zeta}T^{\zeta} = 0, \end{array}$

(2.8)

Step 3. The multiplier $\Lambda$ for (2.7) has the property

$\begin{array}{l} D_{\zeta}T^{\zeta} = \Lambda(\zeta,u(\zeta)) H, \end{array}$

(2.9)

for arbitrary function $U(\zeta)$ .

Step 4. The determining equations for multiplier are obtained by taking the variational derivative of (2.9)

$\begin{array}{l} \frac{\delta}{\delta u}(\Lambda(\zeta,u(\zeta)) H) = 0. \end{array}$

(2.10)

Equation (2.10) holds for arbitrary function $u(\zeta)$ not only for solutions of (2.7). We can derive the conserved vectors using (2.9) after computing the multiplier from (2.10)

Step 5. We construct the following functional

$\begin{array}{l} J = \int^{\infty}_{0}T^{\zeta} d\zeta,\qquad\qquad or \qquad\qquad J = \int^{\infty}_{-\infty}T^{\zeta} d\zeta, \end{array}$

(2.11)

where $T^{\zeta}$ is the conserved quantity for the Eq (2.7).

Step 6. By a Ritz method ^[24], we can obtain solitary wave solutions, such as $u(\zeta) = A sech(\zeta), u(\zeta) = A sech(\zeta)^2$ , where $A$ is a constant to be determined.

Substituting $u(\zeta) = A sech(\zeta), u(\zeta) = A sech(\zeta)^2$ into Eq (2.11) and making $J$ stationary with respect to $A$ we obtain

$\begin{array}{l} \frac{\partial J}{\partial A} = 0. \end{array}$

(2.12)

Solving Eq (2.12) we obtain the constant $A$ . Hence the solitary wave solution is well determined.

3. Conservation laws and soliton solutions for the Benjamin-Bona-Mahoney equation with dual-power law nonlinearity (BBM) equation

We consider the Benjamin-Bona-Mahoney equation with dual-power law nonlinearity (BBM) equation ^[27]

$\begin{array}{l} u_t+a u_x+(b u^n+c u^{2 n}) u_x+l u_{xxx} = 0. \end{array}$

(3.1)

The first term represents the evolution term while $b$ and $c$ represent the coefficients of dual-power law nonlinearity. Then $a$ and $l$ are the coefficients of dispersion terms. The parameter $n$ is the power law parameter while $u$ is the wave profile. The independent variables $x$ and $t$ represent spatial and temporal variables respectively.

The two conserved vectors ^[28]

$\begin{array}{l} T_1^t = \frac{u^2}{2}, \\T_1^x = \frac{a}{2}u^2+\frac{b}{n+2}u^{n+2}+\frac{c}{2(n+1)}u^{2(n+1)}+luu_{xx}-\frac{l}{2}u_x^2,\\T_2^t = u, \\T_2^x = au+\frac{b}{n+1}u^{n+1}+\frac{c}{2n+1}u^{2n+1}+lu_{xx}. \end{array}$

(3.2)

Using the wave variable transformation $u(x, t) = u(\zeta)$ , $\zeta = k x-\omega t+\zeta_0$ to convert Eq (3.1) into ODE

$\begin{array}{l} (k a-\omega)u'+k (b u^n+c u^{2 n}) u'+k^3 l u''' = 0. \end{array}$

(3.3)

The determining equation for multiplier $\Lambda(\zeta, u(\zeta))$ , from (2.10), is

$\begin{array}{l} \Lambda_u((k a-\omega)u'+k (b u^n+c u^{2 n}) u'+k^3 l u''')+k(n b u^{n-1}+2 n c u^{2n-1})u' \Lambda\\-D_\zeta((k a-\omega)+k(b u^n+c u^{2n}))\Lambda -k^3l D_\zeta^3\Lambda = 0. \end{array}$

(3.4)

Equation (3.4) is separated according to different combinations of derivatives of $u$ and and an overdetermined system of equations for multiplier $\Lambda$ is obtained which gives

$\begin{array}{l} \Lambda(\zeta,u) = c_1u+c_2. \end{array}$

(3.5)

From (2.9) and (3.5), we obtain the conserved quantities

$\begin{array}{l} T^\zeta = (a k-\omega ) \left(\frac{1}{2} c_1 u(\zeta )^2+c_2 u(\zeta )\right)+\frac{b c_2 k u(\zeta )^{n+1}}{n+1}+\frac{b c_1 k u(\zeta )^{n+2}}{n+2}+\frac{c_2 d u(\zeta )^{2 n+1}}{2 n+1}\\\qquad+\frac{c_1 d u(\zeta )^{2 n+2}}{2 n+2}+c_2 k^3 l u''(\zeta )+c_1 k^3 l \left(u(\zeta ) u''(\zeta )-\frac{1}{2} u'(\zeta )^2\right). \end{array}$

(3.6)

We can construct the following functional

$\begin{array}{l} J = \int^{\infty}_{0}((a k-\omega ) \left(\frac{1}{2} c_1 u(\zeta )^2+c_2 u(\zeta )\right)+\frac{b c_2 k u(\zeta )^{n+1}}{n+1}+\frac{b c_1 k u(\zeta )^{n+2}}{n+2}+\frac{c_2 d u(\zeta )^{2 n+1}}{2 n+1}\\\qquad+\frac{c_1 d u(\zeta )^{2 n+2}}{2 n+2}+c_2 k^3 l u''(\zeta )+c_1 k^3 l \left(u(\zeta ) u''(\zeta )-\frac{1}{2} u'(\zeta )^2\right)) d\zeta. \end{array}$

(3.7)

According to the Ritz-like method, we look for a solitary wave solution in the form

$\begin{array}{l} u(\zeta) = A sech(\zeta). \end{array}$

(3.8)

Substituting Eq (3.8) into Eq (3.7), we have for $n = 1$

$\begin{array}{l} J = \frac{1}{12} A \left(A c_1 \left(6 a k+2 A^2 d+\pi A b k-6 \left(k^3 l+\\ \omega \right)\right)+c_2 \left(6 \pi a k+\pi A^2 d+6 A b k-6 \pi \omega \right)\right). \end{array}$

(3.9)

To find the constant $A$ , we solve the following equation

$\begin{array}{l} \frac{\partial J}{\partial A} = \frac{1}{4} c_2 \left(2 \pi a k+\pi A^2 d+4 A b k-2 \pi \omega \right)+\\ c_1 \left(A \left(a k+k^3 (-l)-\omega \right)+\frac{2 A^3 d}{3}+\frac{1}{4} \pi A^2 b k\right) = 0. \end{array}$

(3.10)

From (3.10), we get

$\begin{equation} A = \left\{\begin{array}{ll}\frac{-3 \pi b k\mp \sqrt{3(3 \pi ^2 b^2 k^2-128 a d k+128 d k^3 l+128 d \omega) }}{16 d},& c_1 = 1,\qquad c_2 = 0,\\\frac{-\sqrt{2(2 b^2 k^2-\pi ^2 a d k+\pi ^2 d \omega) }-2 b k}{\pi d} ,& c_1 = 0,\qquad c_2 = 1.\end{array}\right. \end{equation}$

(3.11)

Therefore, the solitary wave solutions for the BBM equation with dual-power law nonlinearity are

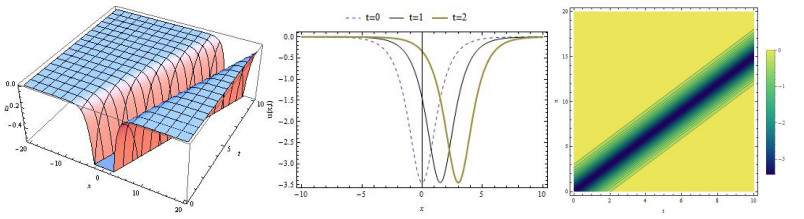
$\begin{equation} u(x,t) = \left\{\begin{array}{ll}\frac{-3 \pi b k\mp \sqrt{3(3 \pi ^2 b^2 k^2-128 a d k+128 d k^3 l+128 d \omega) }}{16 d}sech(k x-\omega t+\zeta_{0}),& c_1 = 1, c_2 = 0,\\\frac{-\sqrt{2(2 b^2 k^2-\pi ^2 a d k+\pi ^2 d \omega) }-2 b k}{\pi d} sech(k x-\omega t+\zeta_{0}) ,& c_1 = 0, c_2 = 1.\end{array}\right. \end{equation}$

(3.12)

The 3D graphs in and illustrate the trough propagating to the right, while 2D graphs show the movement of the wave along $x$ -direction as time increases for Eq (3.12), a contour plot could also be useful for describing the movement of the solitons.

Figure 1. Shows the soliton solution of Eq (3.12) for

$c_1 = 1, c_2 = 0$ .

DownLoad: Full-Size Img PowerPoint

Figure 2. Shows the soliton solution of Eq (3.12) for

$c_1 = 0, c_2 = 1$ .

DownLoad: Full-Size Img PowerPoint

For another soliton solution in the form ^[29]

$\begin{array}{l} u(\zeta) = F sech^2(\zeta). \end{array}$

(3.13)

where $F$ is a constant to be determined. Substituting Eq (3.13) into Eq (3.9), we obtain

$\begin{array}{l} J = \frac{F}{315} (F c_1 \left(21 \left(5 a k-12 k^3 l-5 \omega \right)+36 F^2 d+56 F b k\right))\\\qquad+\frac{F}{315}(7 c_2 \left(45 a k+8 F^2 d+15 F b k-45 \omega) \right)). \end{array}$

(3.14)

To find the constant $F$ , we solve the following equation

$\begin{array}{l} \frac{\partial J}{\partial F} = \frac{1}{105} (2 F c_1 \left(35 a k+24 F^2 d+28 F b k-84 k^3 l-35 \omega \right))\\\qquad\quad+\frac{1}{105} (7 c_2 \left(15 a k+8 F^2 d+10 F b k-15 \omega) \right)) = 0. \end{array}$

(3.15)

From Eq (3.15), we have

$\begin{equation} F = \left\{\begin{array}{ll}\frac{-7 b k\mp \sqrt{7(72 d k^3 l-30 a d k+7 b^2 k^2+30 d \omega )}}{12 d},& c_1 = 1,\qquad c_2 = 0,\\\frac{-5 b k\mp \sqrt{5(5 b^2 k^2-24 a d k+24 d \omega) }}{8 d},& c_1 = 0,\qquad c_2 = 1.\end{array}\right. \end{equation}$

(3.16)

Therefore, the solitary wave solutions for the BBM equation with dual-power law nonlinearity are as follows:

$\begin{equation} u(x,t) = \left\{\begin{array}{ll}\frac{-7 b k\mp \sqrt{7(72 d k^3 l-30 a d k+7 b^2 k^2+30 d \omega )}}{12 d}sech^2(k x-\omega t+\zeta_{0}),& c_1 = 1, c_2 = 0,\\\frac{-5 b k\mp \sqrt{5(5 b^2 k^2-24 a d k+24 d \omega) }}{8 d}sech^2(k x-\omega t+\zeta_{0}) ,& c_1 = 0, c_2 = 1.\end{array}\right. \end{equation}$

(3.17)

The 3D graphs in Figures 3 and 4 describe nonlinear wave propagation of soliton solutions, while 2D graphs depict the movement of the solitons obtained from Eq (3.17) for different times and a contour plot describes the movement of the solitons.

Figure 3. Shows the soliton solution of Eq (3.17) for

$c_1 = 1, c_2 = 0$ .

DownLoad: Full-Size Img PowerPoint

Figure 4. Shows the soliton solution of Eq (3.17) for

$c_1 = 0, c_2 = 1$ .

DownLoad: Full-Size Img PowerPoint

4. Conservation laws and soliton solutions for the modified regularized long wave (MRLW) equation

We consider the modified regularized long wave (MRLW) equation ^[30]

$\begin{array}{l} u_t+ u_x+6u^2u_x-\mu u_{xxt} = 0, \end{array}$

(4.1)

where $\mu$ is a positive constants, was originally introduced to describe the behavior of the undular bore by Peregrine ^[31], and later by Benjamin et al. ^[32]. This equation is very important in physics media since it describes a phenomenon with weak nonlinearity and dispersion waves, including nonlinear transverse waves in shallow water, ion-acoustic and magneto hydrodynamic waves in plasma and phonon packets in nonlinear crystals.

The two conserved vectors ^[28]

$\begin{array}{l} T_1^t = \frac{u^2}{2}-\mu(uu_{xx}-\frac{1}{2}u_x^2),\\ T_1^x = \frac{1}{2}u^2+\mu u_xu_t+\frac{3}{2}u^4,\\T_2^t = u, \qquad\qquad T_2^x = u+2u^3. \end{array}$

(4.2)

To find the soliton solutions, we convert Eq (4.1) into an ODE

$\begin{array}{l} (k -\omega)u'+6 k u^2 u'+\mu k^2 \omega u''' = 0. \end{array}$

(4.3)

The multiplier $\Lambda(\zeta, u(\zeta))$ , can be obtained as follows

$\begin{equation} \Lambda_u((k -\omega)u'+6 k u^2 u'+\mu k^2 \omega u''')+12k u u' \Lambda-\\ D_\zeta((k -\omega)+6k u^2)\Lambda -\mu \omega k^2 D_\zeta^3\Lambda = 0. \end{equation}$

(4.4)

Solving Eq (4.4) for multiplier $\Lambda$ , we obtain

$\begin{array}{l} \Lambda(\zeta,u) = c_1u+c_2. \end{array}$

(4.5)

From (2.9) and (4.5), we obtain the conserved quantities

$\begin{array}{l} T^\zeta = -k^2 \mu \omega \left(c_2 u''(\zeta )+c_1 \left(u(\zeta ) u''(\zeta )\\ -\frac{1}{2} u'(\zeta )^2\right)\right)+\frac{1}{2} (k-\omega ) u(\zeta ) \left(c_1 u(\zeta )+2 c_2\right)\\\qquad+\frac{3}{2} k u(\zeta )^3 \left(c_1 u(\zeta )+\frac{4 c_2}{3}\right). \end{array}$

(4.6)

From (4.6), we can obtain the functional

$\begin{array}{l} J = \int^{\infty}_{0}(-k^2 \mu \omega \left(c_2 u''(\zeta )+c_1 \left(u(\zeta ) u''(\zeta )\\ -\frac{1}{2} u'(\zeta )^2\right)\right)+\frac{1}{2} (k-\omega ) u(\zeta ) \left(c_1 u(\zeta )+2 c_2\right)\\\qquad+\frac{3}{2} k u(\zeta )^3 \left(c_1 u(\zeta )+\frac{4 c_2}{3}\right)) d\zeta. \end{array}$

(4.7)

According to the Ritz-like method, we search a solitary wave solution in the form

$\begin{array}{l} u(\zeta) = A sech(\zeta). \end{array}$

(4.8)

Substituting Eq (4.8) into Eq (4.7), we have

$\begin{array}{l} J = \frac{1}{2} A \left(A c_1 \left(2 A^2 k+k^2 \mu \omega +k-\omega \right)+\pi c_2 \left(A^2 k+k-\omega \right)\right). \end{array}$

(4.9)

To find the constant $A$ , we solve the following equation

$\begin{array}{l} \frac{\partial J}{\partial A} = \frac{1}{2} \left(2 A c_1 \left(4 A^2 k+k^2 \mu \omega +k-\omega \right)+\pi c_2 \left(3 A^2 k+k-\omega \right)\right) = 0. \end{array}$

(4.10)

From (4.10), we obtain

$\begin{equation} A = \left\{\begin{array}{ll}\mp \sqrt{\frac{\omega-k-\mu \omega k^2}{4k}},& c_1 = 1,\qquad c_2 = 0,\\\mp\sqrt{\frac{\omega-k}{3k}},& c_1 = 0,\qquad c_2 = 1.\end{array}\right. \end{equation}$

(4.11)

Therefore, the solitary wave solutions for the MRLW equation are

$\begin{equation} u(x,t) = \left\{\begin{array}{ll}\mp \sqrt{\frac{\omega-k-\mu \omega k^2}{4k}}sech(k x-\omega t+\zeta_{0}),& c_1 = 1, c_2 = 0,\\\mp\sqrt{\frac{\omega-k}{3k}}sech(k x-\omega t+\zeta_{0}) ,& c_1 = 0, c_2 = 1.\end{array}\right. \end{equation}$

(4.12)

The 3D graphs in and describe the shape of solitons for waves $u(x, t)$ , while 2D graphs depict the movement of the soliton waves along $x$ -direction as time increases for Eq (4.12), a contour plot can also be useful for deseribing the movement of the solitons for (4.12).

Figure 5. Shows the soliton solution of Eq (4.12) for

$c_1 = 1, c_2 = 0$ .

DownLoad: Full-Size Img PowerPoint

Figure 6. Shows the soliton solution of Eq (4.12) for

$c_1 = 0, c_2 = 1$ .

DownLoad: Full-Size Img PowerPoint

For another soliton solution (3.13), we obtain

$\begin{equation} J = \frac{1}{105} F \left(F c_1 \left(\left(72 F^2+35\right) k+84 k^2 \mu \omega -35 \omega \right)+\\ 7 c_2 \left(\left(16 F^2+15\right) k-15 \omega \right)\right). \end{equation}$

(4.13)

Solving the following equation to find $F$

$\begin{equation} \frac{\partial J}{\partial F} = \frac{2}{105} F c_1 \left(\left(144 F^2+35\right) k+84 k^2 \mu \omega -35 \omega \right)+\\ \frac{1}{5} c_2 \left(\left(16 F^2+5\right) k-5 \omega \right) = 0. \end{equation}$

(4.14)

From Eq (4.14), we have

$\begin{equation} F = \left\{\begin{array}{ll}\mp\frac{1}{12} \sqrt{\frac{7(5 \omega-12 k^2 \mu \omega -5 k) }{k}},& c_1 = 1,\qquad c_2 = 0,\\\mp\frac{1}{4} \sqrt{\frac{5(\omega -k)}{k}},& c_1 = 0,\qquad c_2 = 1.\end{array}\right. \end{equation}$

(4.15)

Therefore, the solitary wave solutions for the MRLW equation are as follows

$\begin{equation} u(x,t) = \left\{\begin{array}{ll}\mp\frac{1}{12} \sqrt{\frac{7(5 \omega-12 k^2 \mu \omega -5 k) }{k}}sech^2(k x-\omega t+\zeta_{0}),& c_1 = 1, c_2 = 0,\\\mp\frac{1}{4} \sqrt{\frac{5(\omega -k)}{k}}sech^2(k x-\omega t+\zeta_{0}) ,& c_1 = 0, c_2 = 1.\end{array}\right. \end{equation}$

(4.16)

The 3D graphs in Figures 7 and 8 describe nonlinear wave propagation of soliton solutions to the right, while 2D graphs depict the movement of the solitons obtained from Eq (4.16) for different times and a contour plot describes the movement of the solitons.

Figure 7. Shows the soliton solution of Eq (4.16) for

$c_1 = 1, c_2 = 0$ .

DownLoad: Full-Size Img PowerPoint

Figure 8. Shows the soliton solution of Eq (4.16) for

$c_1 = 0, c_2 = 1$ .

DownLoad: Full-Size Img PowerPoint

5. Conservation laws and soliton solutions for the modified nonlinearly dispersive KdV equations 2K(2, 2, 1) equation

The modified nonlinearly dispersive KdV equations 2K(2, 2, 1) equation ^[33] takes the form

$\begin{array}{l} uu_t+a (u^2)_x+b u_{xxx} = 0, \end{array}$

(5.1)

where $a$ and $b$ are constants.

The three conserved vectors ^[28]

$\begin{array}{l} T_1^t = \frac{u^2}{2}(t-\frac{x}{2a}),\\ T_1^x = u^2(at-\frac{x}{2})+u_{xx}(bt-\frac{x}{2})+\frac{1}{2a}u_x,\\T_2^t = \frac{u^3}{3}, \qquad\qquad T_2^x = \frac{2}{3}au^3-\frac{b}{2}(u_x)^2+buu_{xx},\\T_3^t = \frac{u^2}{2}, \qquad\qquad T_3^x = au^2+bu_{xx}. \end{array}$

(5.2)

By converting Eq (5.1) into an ODE

$\begin{array}{l} (2ak -\omega)u u'+b k^3 u''' = 0. \end{array}$

(5.3)

The multiplier $\Lambda(\zeta, u(\zeta))$ , can be obtained as follows

$\begin{equation} \Lambda_u((2ak -\omega)uu'+b k^3 u''')+(2ak -\omega)(u'\Lambda -D_\zeta(u\Lambda))-b k^3D_\zeta^3\Lambda = 0. \end{equation}$

(5.4)

Solving Eq (5.4) for multiplier $\Lambda$ , we obtain

$\begin{array}{l} \Lambda(\zeta,u) = c_1u+c_2. \end{array}$

(5.5)

From (2.9) and (5.5), we obtain the conserved quantities

$\begin{array}{l} T^\zeta = \frac{1}{6} u(\zeta )^2 (2 a k-\omega ) \left(2 c_1 u(\zeta )+3 c_2\right)+\\ b k^3 \left(c_2 u''(\zeta )+c_1 \left(u(\zeta ) u''(\zeta )-\frac{1}{2} u'(\zeta )^2\right)\right). \end{array}$

(5.6)

From (5.6), we can obtain the functional

$\begin{equation} J = \int^{\infty}_{0}(\frac{1}{6} u(\zeta )^2 (2 a k-\omega ) \left(2 c_1 u(\zeta )+3 c_2\right)+b k^3 \\ \left(c_2 u''(\zeta )+c_1 \left(u(\zeta ) u''(\zeta )-\frac{1}{2} u'(\zeta )^2\right)\right)) d\zeta. \end{equation}$

(5.7)

According to the Ritz-like method, we search a solitary wave solution in the form

$\begin{array}{l} u(\zeta) = A sech(\zeta). \end{array}$

(5.8)

Substituting Eq (5.8) into Eq (5.7), we have

$\begin{array}{l} J = \frac{1}{12} A^2 \left(c_1 \left(-\pi A (\omega -2 a k)-6 b k^3\right)+6 c_2 (2 a k-\omega )\right). \end{array}$

(5.9)

To find the constant $A$ , we solve the following equation

$\begin{array}{l} \frac{\partial J}{\partial A} = \frac{1}{4} A \left(c_1 \left(-\pi A (\omega -2 a k)-4 b k^3\right)+4 c_2 (2 a k-\omega )\right) = 0. \end{array}$

(5.10)

From (5.10), we obtain

$\begin{equation} A = \left\{\begin{array}{ll}\frac{4 b k^3}{\pi (2 a k-\omega )},& c_1 = 1,\qquad c_2 = 0,\\\frac{4 \left(-2 a k+b k^3+\omega \right)}{\pi (2 a k-\omega )},& c_1 = 1,\qquad c_2 = 1.\end{array}\right. \end{equation}$

(5.11)

Therefore, the solitary wave solutions for the 2K(2, 2, 1) equation are

$\begin{equation} u(x,t) = \left\{\begin{array}{ll}\frac{4 b k^3}{\pi (2 a k-\omega )}sech(k x-\omega t+\zeta_{0}),& c_1 = 1, c_2 = 0,\\\frac{4 \left(-2 a k+b k^3+\omega \right)}{\pi (2 a k-\omega )}sech(k x-\omega t+\zeta_{0}) ,& c_1 = 1, c_2 = 1.\end{array}\right. \end{equation}$

(5.12)

The 3D graphs in and describe the shape of solitons for waves $u(x, t)$ , while 2D graphs depict the movement of the soliton waves along $x$ -direction as time increases for Eq (5.12), a contour plot can also be useful for describing the movement of the solitons for (5.12).

Figure 9. Shows the soliton solution of Eq (5.12) for

$c_1 = 1, c_2 = 0$ .

DownLoad: Full-Size Img PowerPoint

Figure 10. Shows the soliton solution of Eq (5.12) for

$c_1 = 1, c_2 = 1$ .

DownLoad: Full-Size Img PowerPoint

For another soliton solution (3.13), we obtain

$\begin{equation} J = \frac{1}{45} F^2 \left(4 c_1 \left(4 a F k-2 F \omega -9 b k^3\right)+15 c_2 (2 a k-\omega )\right). \end{equation}$

(5.13)

Solving the following equation to find $F$

$\begin{equation} \frac{\partial J}{\partial F} = \frac{2}{15} F \left(4 c_1 \left(2 a F k-F \omega -3 b k^3\right)+5 c_2 (2 a k-\omega )\right) = 0. \end{equation}$

(5.14)

From Eq (5.14), we have

$\begin{equation} F = \left\{\begin{array}{ll}\frac{3 b k^3}{2 a k-\omega },& c_1 = 1,\qquad c_2 = 0,\\\frac{-10 a k+12 b k^3+5 \omega }{4 (2 a k-\omega )},& c_1 = 1,\qquad c_2 = 1.\end{array}\right. \end{equation}$

(5.15)

Therefore, the solitary wave solutions for the 2K(2, 2, 1) equation are as follows:

$\begin{equation} u(x,t) = \left\{\begin{array}{ll}\frac{3 b k^3}{2 a k-\omega }sech^2(k x-\omega t+\zeta_{0}),& c_1 = 1, c_2 = 0,\\\frac{-10 a k+12 b k^3+5 \omega }{4 (2 a k-\omega )}sech^2(k x-\omega t+\zeta_{0}) ,& c_1 = 1, c_2 = 1.\end{array}\right. \end{equation}$

(5.16)

The 3D graphs in Figures 11 and 12 describe nonlinear wave propagation of soliton solutions to the right, while 2D graphs depict the movement of the solitons obtained from Eq (5.16) for different times and a contour plot describes the movement of the solitons.

Figure 11. Shows the soliton solution of Eq (5.16) for

$c_1 = 1, c_2 = 0$ .

DownLoad: Full-Size Img PowerPoint

Figure 12. Shows the soliton solution of Eq (5.16) for

$c_1 = 1, c_2 = 1$ .

DownLoad: Full-Size Img PowerPoint

6. Conservation laws and soliton solutions for the modified nonlinearly dispersive KdV equations 3K(3, 2, 2) equation

The modified nonlinearly dispersive KdV equations 3K(3, 2, 2) equation ^[33] take the form

$\begin{array}{l} u^2u_t+a (u^2)_x+b (u^2)_{xxx} = 0, \end{array}$

(6.1)

where $a$ and $b$ are constants.

The four conserved vectors ^[28]

$\begin{array}{l} T_1^t = \frac{u^5}{5},\\ T_1^x = \frac{a}{2}u^4+bu^2(u^2)_{xx}-2bu^2u_x^2,\\T_2^t = au^2, \qquad\qquad T_2^x = au^2+2(uu_{xx}+u_x^2),\\T_3^t = \frac{1}{3}u^3\sin(\sqrt{\frac{a}{b}}x), \\ T_3^x = b(2(uu_{xx}+u_x^2)\sin(\sqrt{\frac{a}{b}}x)-2\sqrt{\frac{a}{b}}uu_x\cos(\sqrt{\frac{a}{b}}x)),\\T_4^t = \frac{1}{3}u^3\cos(\sqrt{\frac{a}{b}}x), \\ T_4^x = b(2(uu_{xx}+u_x^2)\cos(\sqrt{\frac{a}{b}}x)+2\sqrt{\frac{a}{b}}uu_x\sin(\sqrt{\frac{a}{b}}x)). \end{array}$

(6.2)

By converting Eq (6.1) into an ODE

$\begin{array}{l} (2ak -\omega u)u u'+2 k^3(u u'''+3 u' u'') = 0. \end{array}$

(6.3)

The multiplier $\Lambda(\zeta, u(\zeta))$ , can be obtained as follows

$\begin{array}{l} \Lambda_u((2ak -\omega u)u u'+2 k^3(u u'''+3 u' u''))+((2ak -2\omega u)u'+2k^3u''')\Lambda\\-D_\zeta((2ak -\omega u)u+6k^3u'')\Lambda)-2 k^3D_\zeta^3(u\Lambda) = 0. \end{array}$

(6.4)

Solving Eq (6.4) for multiplier $\Lambda$ , we obtain

$\begin{array}{l} \Lambda(\zeta,u) = c_1+c_2u^2. \end{array}$

(6.5)

From (2.9) and (6.5), we obtain the conserved quantities

$\begin{array}{l} T^\zeta = \frac{1}{2} a k u(\zeta )^2 \left(c_2 u(\zeta )^2+2 c_1\right)\\\qquad+2 k^3 \left(c_2 u(\zeta )^3 u''(\zeta )+\frac{3}{2} c_1 u'(\zeta )^2+c_1 \left(u(\zeta ) u''(\zeta )-\frac{1}{2} u'(\zeta )^2\right)\right)\\\qquad-\frac{1}{15} \omega u(\zeta )^3 \left(3 c_2 u(\zeta )^2+5 c_1\right). \end{array}$

(6.6)

From (6.6), we can get the functional

$\begin{array}{l} J = \int^{\infty}_{0}(\frac{1}{2} a k u(\zeta )^2 \left(c_2 u(\zeta )^2+2 c_1\right)\\\qquad+2 k^3 \left(c_2 u(\zeta )^3 u''(\zeta )+\frac{3}{2} c_1 u'(\zeta )^2+c_1 \left(u(\zeta ) u''(\zeta )-\frac{1}{2} u'(\zeta )^2\right)\right)\\\qquad-\frac{1}{15} \omega u(\zeta )^3 \left(3 c_2 u(\zeta )^2+5 c_1\right)) d\zeta. \end{array}$

(6.7)

According to the Ritz-like method, we search a solitary wave solution in the form

$\begin{array}{l} u(\zeta) = A sech(\zeta). \end{array}$

(6.8)

Substituting Eq (6.8) into Eq (6.7), we have

$\begin{array}{l} J = c_1 \left(a A^2 k-\frac{1}{12} \pi A^3 \omega \right)-\frac{1}{240} A^4 c_2 \left(-80 a k+9 \pi A \omega +192 k^3\right). \end{array}$

(6.9)

To find the constant $A$ , we solve the following equation

$\begin{array}{l} \frac{\partial J}{\partial A} = -\frac{1}{240} A^3 c_2 \left(-320 a k+45 \pi A \omega +768 k^3\right)-\frac{1}{4} A c_1 (\pi A \omega -8 a k) = 0. \end{array}$

(6.10)

From (6.10), we obtain

$\begin{equation} A = \left\{\begin{array}{ll}\frac{8 a k}{\pi \omega },& c_1 = 1,\qquad c_2 = 0,\\\frac{64 \left(5 a k-12 k^3\right)}{45 \pi \omega },& c_1 = 0,\qquad c_2 = 1.\end{array}\right. \end{equation}$

(6.11)

Therefore, the solitary wave solutions for the 3K(3, 2, 2) equation are

$\begin{equation} u(x,t) = \left\{\begin{array}{ll}\frac{8 a k}{\pi \omega }sech(k x-\omega t+\zeta_{0}),& c_1 = 1, c_2 = 0,\\\frac{64 \left(5 a k-12 k^3\right)}{45 \pi \omega }sech(k x-\omega t+\zeta_{0}) ,& c_1 = 0, c_2 = 1.\end{array}\right. \end{equation}$

(6.12)

The 3D graphs in and describe the shape of solitons for waves $u(x, t)$ , while 2D graphs depict the movement of the soliton waves along $x$ -direction as time increases for Eq (6.12), a contour plot can also be useful for describing the properties of the solitary waves for (6.12).

Figure 13. Shows the soliton solution of Eq (6.12) for

$c_1 = 1, c_2 = 0$ .

DownLoad: Full-Size Img PowerPoint

Figure 14. Shows the soliton solution of Eq (6.12) for

$c_1 = 0, c_2 = 1$ .

DownLoad: Full-Size Img PowerPoint

For another soliton solution (3.13), we obtain

$\begin{equation} J = \frac{2 F^2 \left(35 c_1 (15 a k-4 F \omega )-4 F^2 c_2 \left(-45 a k+16 F \omega +240 k^3\right)\right)}{1575}. \end{equation}$

(6.13)

Solving the following equation to find $F$

$\begin{equation} \frac{\partial J}{\partial F} = \frac{4}{315} A \left(21 c_1 (5 a k-2 A \omega )-8 A^2 c_2 \left(-9 a k+4 A \omega +48 k^3\right)\right) = 0. \end{equation}$

(6.14)

From Eq (6.14), we have

$\begin{equation} F = \left\{\begin{array}{ll}\frac{5 a k}{2 \omega },& c_1 = 1,\qquad c_2 = 0,\\\frac{3 \left(3 a k-16 k^3\right)}{4 \omega },& c_1 = 0,\qquad c_2 = 1.\end{array}\right. \end{equation}$

(6.15)

Therefore, the solitary wave solutions for the 3K(3, 2, 2) equation are as follows:

$\begin{equation} u(x,t) = \left\{\begin{array}{ll}\frac{5 a k}{2 \omega }sech^2(k x-\omega t+\zeta_{0}),& c_1 = 1, c_2 = 0,\\\frac{3 \left(3 a k-16 k^3\right)}{4 \omega }sech^2(k x-\omega t+\zeta_{0}) ,& c_1 = 0, c_2 = 1.\end{array}\right. \end{equation}$

(6.16)

The 3D graphs in Figures 15 and 16 describe nonlinear wave propagation of soliton solutions, while 2D graphs depict the movement of the solitons obtained from Eq (6.16) for different times and a contour plot describes the movement of the solitons.

Figure 15. Shows the soliton solution of Eq (6.16) for

$c_1 = 1, c_2 = 0$ .

DownLoad: Full-Size Img PowerPoint

Figure 16. Shows the soliton solution of Eq (6.16) for

$c_1 = 0, c_2 = 1$ .

DownLoad: Full-Size Img PowerPoint

7. Conclusions

In this paper we derive the soliton solutions directly from the conserved quantities for Benjamin-Bona-Mahoney equation with dual-power law nonlinearity (BBM), modified regularized long wave (MRLW) equation and modified nonlinearly dispersive KdV equations 2K(2, 2, 1) and 3K(3, 2, 2) equation, which are constructed by multiplier approach (variational derivative method). The multiplier approach on Benjamin-Bona-Mahoney equation with dual-power law nonlinearity (BBM) and modified regularized long wave (MRLW) equation gave two multipliers of $\Lambda (x, t, u)$ , and thus two conserved vectors obtained for each case. For the modified nonlinearly dispersive KdV equations 2K(2, 2, 1) equation, the multiplier approach yielded three multipliers and thus three conserved vectors were obtained. For the modified nonlinearly dispersive KdV equations 3K(3, 2, 2) equation, the multiplier approach yielded four multipliers and thus four conserved vectors were obtained. So, we can say that the proposed method is effective and an alternative mathematical tool for generating soliton solutions. The main results is derived the functional (2.11) using the conserved quantities and then the soliton solutions for the models. This is another method for deriving the variational principles for the nonlinear evolution equations and we can easily extend this work to all soliton equations in future works. Finally, we give the numerical simulations for these solutions.

Conflict of interests

The author declares no conflicts of interest in this paper.

References

[1]	A. Akbulut, M. Kaplan, M. K. A. Kaabar, New conservation laws and exact solutions of the special case of the fifth-order KdV equation, J. Ocean Eng. Sci., 2021. https://doi.org/10.1016/j.joes.2021.09.010
[2]	A. Akbulut, F. Tascan, E. Zel, Trivial conservation laws and solitary wave solution of the fifth order Lax equation, Part. Differ. Eq. Appl. Math., 4 (2021), 100101. https://doi.org/10.1016/j.padiff.2021.100101 doi: 10.1016/j.padiff.2021.100101
[3]	G. S. F. Frederico, D. F. M. Torres, Fractional isoperimetric Noether's Theorem in the Riemann-Liouville sense, Rep. Math. Phys., 71 (2013), 291–304. https://doi.org/10.1016/S0034-4877(13)60034-8 doi: 10.1016/S0034-4877(13)60034-8
[4]	P. S. Laplace, Trait de Mecanique Celeste, Celest. Mech., New York, 1966.
[5]	H. Steudel, Uber die Zuordnung zwischen invarianzeigenschaften und Erhaltungssatzen, Z. Naturforsch. A, 17 (1962), 129–132.
[6]	P. J. Olver, Applications of Lie groups to differential equations, New York: Springer, 1993,435–458.
[7]	S. C. Anco, G. W. Bluman, Direct construction method for conservation laws of partial differential equations. Part Ⅰ: Examples of conservation law classifications, Eur. J. Appl. Math., 13 (2002), 545–566. https://doi.org/10.1017/S095679250100465X doi: 10.1017/S095679250100465X
[8]	A. H. Kara, F. M. Mahomed, Relationship between symmetries and conservation laws, Int. J. Theor. Phys., 39 (2000), 23–40.
[9]	A. H. Kara, F. M. Mahomed, Noether-type symmetries and conservation laws via partial Lagrangians, Nonlinear Dyn., 45 (2006), 367–383. https://doi.org/10.1007/s11071-005-9013-9 doi: 10.1007/s11071-005-9013-9
[10]	H. Z. Liu, J. B. Li, Lie symmetries, conservation laws and exact solutions for two rod equations, Acta Appl. Math., 110 (2010), 573–587.
[11]	O. M. Khudaverdian, Double complexes and cohomological hierarchy in a space of weakly invariant lagrangians, Acta Appl. Math., 56 (1999), 181–215.
[12]	A. F. Cheviakov, GeM software package for computation of symmetries and conservation laws of differential equations, Comput. Phys. Commun., 176 (2007), 48–61. https://doi.org/10.1016/j.cpc.2006.08.001 doi: 10.1016/j.cpc.2006.08.001
[13]	R. Naz, D. P. Mason, F. M. Mahomed, Conservation laws and conserved quantities for laminar two-dimensional and radial jets, Nonlinear Anal. RWA, 10 (2009), 2641–2651. https://doi.org/10.1016/j.nonrwa.2008.07.003 doi: 10.1016/j.nonrwa.2008.07.003
[14]	R. Naz, F. M. Mahomed, D. P. Mason, Comparison of different approaches to conservation laws for some partial differential equations in fluid mechanics, Appl. Math. Comput., 205 (2008), 212–230. https://doi.org/10.1016/j.amc.2008.06.042 doi: 10.1016/j.amc.2008.06.042
[15]	G. T. Bekova, G. N. Shaikhova, K. R. Yesmakhanova, R. Myrzakulov, Conservation laws for two dimensional nonlinear Schrodinger equation, AIP Conf. Proc., 2159 (2019), 030003. https://doi.org/10.1063/1.5127468 doi: 10.1063/1.5127468
[16]	Y. Tian, K. L. Wang, Conservation laws for partial differential equations based on the polynomial characteristic method, Therm. Sci., 24 (2020), 2529–2534. https://doi.org/10.2298/TSCI2004529T doi: 10.2298/TSCI2004529T
[17]	F. A. Mohammed, M. K. Elboree, Soliton solutions and periodic solutions for two models arises in mathematical physics, AIMS Math., 7 (2022), 4439–4458. https://doi.org/10.3934/math.2022247 doi: 10.3934/math.2022247
[18]	T. Mathanaranjan, D. Kumar, H. Rezazadeh, L. Akinyemi, Optical solitons in meta materials with third and fourth order dispersions, Opt. Quant. Electron., 54 (2022), 1–15.
[19]	T. Mathanaranjan, H. Rezazadeh, M. Enol, L. Akinyemi, Optical singular and dark solitons to the nonlinear Schr $\ddot{o}$ dinger equation in magneto-optic waveguides with anti-cubic nonlinearity, Opt. Quant. Electron., 53 (2021), 1–16.
[20]	T. Mathanaranjan, Soliton solutions of deformed nonlinear Schr $\ddot{o}$ dinger equations using ansatz method, Int. J. Appl. Comput. Math., 7 (2021), 159
[21]	H. Najafi, M. Najafi, S. A. Mohammad-Abadi, New soliton solutions for Kaup-Boussinesq system, Int. J. Appl. Math. Res., 1 (2012), 402–408. https://doi.org/10.14419/ijamr.v1i4.310 doi: 10.14419/ijamr.v1i4.310
[22]	K. H. Mohammedali, N. A. Ahmad, F. S. Fadhel, Hes variational iteration method for solving Riccati matrix delay differential equations of variable coefficients, AIP Conf. Proc., 1830 (2017), 020029. https://doi.org/10.1063/1.4980892 doi: 10.1063/1.4980892
[23]	L. H. Zhang, Conservation laws of the (2 + 1)-dimensional KP equation and Burgers equation with variable coefficients and cross terms, Appl. Math. Comput., 219 (2013), 48654879. https://doi.org/10.1016/j.amc.2012.10.063 doi: 10.1016/j.amc.2012.10.063
[24]	W. Ritz, Uber eine neue methode zur lösung gewisser variationsprobleme der mathematischen physik, J. Reine Angew. Math., 135 (1909), 1–67. https://doi.org/10.1515/crll.1909.135.1 doi: 10.1515/crll.1909.135.1
[25]	D. H. Peregrine, Calculations of the development of an undular bore, J. Fluid Mech., 25 (1966), 321–330. https://doi.org/10.1017/S0022112066001678 doi: 10.1017/S0022112066001678
[26]	A. Prakash, M. Kumar, He's variational iteration method for the solution of nonlinear Newell-Whitehead-Segel equation, J. Appl. Anal. Comput., 6 (2016), 738–748. https://doi.org/10.11948/2016048 doi: 10.11948/2016048
[27]	X. L. Yang, J. S. Tang, Z. Qiao, Traveling wave solutions of the generalized BBM equation, Pacific J. Appl. Math., 1 (2009), 22134.
[28]	F. A. Mohammed, M. K. Elboree, Conservation laws for classes of nonlinear evolution equations using multiplier approach, Appl. Math. Sci., 24 (2019), 1259–1266. https://doi.org/10.12988/ams.2019.911156 doi: 10.12988/ams.2019.911156
[29]	Y. H. Ye, L. F. Mo, He's variational method for the Benjamin Bona equation and the Kawahara equation, Comput. Math. Appl., 58 (2009), 2420–2422. https://doi.org/10.1016/j.camwa.2009.03.026 doi: 10.1016/j.camwa.2009.03.026
[30]	A. K. Khalifa, K. R. Raslan, H. M. Alzubaidi, Numerical study using ADM for the modified regularized long wave equation, Appl. Math. Model., 32 (2008), 2962–2972. https://doi.org/10.1016/j.apm.2007.10.014 doi: 10.1016/j.apm.2007.10.014
[31]	D. H. Peregrine, Calculations of the development of an undular bore, J. Fluid. Mech., 25 (1966), 321–330. https://doi.org/10.1017/S0022112066001678 doi: 10.1017/S0022112066001678
[32]	A. K. Khalifa, K. R. Raslan, H. M. Alzubaidi, Numerical study using ADM for the modified regularized long wave equation, Appl. Math. Model., 32 (2008), 2962–2972. https://doi.org/10.1016/j.apm.2007.10.014 doi: 10.1016/j.apm.2007.10.014
[33]	Z. Y. Yan, Modified nonlinearly dispersive $mK(m, n, k)$ equations: Ⅱ. Jacobi elliptic function solutions, Comput. Phys. Commun., 22 (2003), 325–341.

This article has been cited by:

1.	Md. Nur Alam, Soliton solutions to the electric signals in telegraph lines on the basis of the tunnel diode, 2023, 7, 26668181, 100491, 10.1016/j.padiff.2023.100491
2.	Marwan Alquran, Omar Najadat, Mohammed Ali, Sania Qureshi, New kink-periodic and convex–concave-periodic solutions to the modified regularized long wave equation by means of modified rational trigonometric–hyperbolic functions, 2023, 12, 2192-8029, 10.1515/nleng-2022-0307

Reader Comments

Your name:*

Email:*
© 2022 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)

通讯作者: 陈斌, bchen63@163.com

1.
沈阳化工大学材料科学与工程学院沈阳 110142

AIMS Mathematics

1.8 3.4

Metrics

Article views(2713) PDF downloads(92) Cited by(2)

Preview PDF

Download XML

Export Citation

Article outline

Show full outline

Figures and Tables

Figures(16)

AIMS Mathematics

Soliton solutions for some nonlinear models in mathematical physics via conservation laws

Related Papers:

Abstract

1. Introduction

2. The proposed method

3. Conservation laws and soliton solutions for the Benjamin-Bona-Mahoney equation with dual-power law nonlinearity (BBM) equation

4. Conservation laws and soliton solutions for the modified regularized long wave (MRLW) equation

5. Conservation laws and soliton solutions for the modified nonlinearly dispersive KdV equations 2K(2, 2, 1) equation

6. Conservation laws and soliton solutions for the modified nonlinearly dispersive KdV equations 3K(3, 2, 2) equation

7. Conclusions

Conflict of interests

References

This article has been cited by:

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Figures and Tables

Other Articles By Authors

Catalog

AIMS Mathematics

Soliton solutions for some nonlinear models in mathematical physics via conservation laws

Related Papers:

Abstract

1. Introduction

2. The proposed method

3. Conservation laws and soliton solutions for the Benjamin-Bona-Mahoney equation with dual-power law nonlinearity (BBM) equation

4. Conservation laws and soliton solutions for the modified regularized long wave (MRLW) equation

5. Conservation laws and soliton solutions for the modified nonlinearly dispersive KdV equations 2K(2, 2, 1) equation

6. Conservation laws and soliton solutions for the modified nonlinearly dispersive KdV equations 3K(3, 2, 2) equation

7. Conclusions

Conflict of interests

References

This article has been cited by:

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Figures and Tables

Other Articles By Authors

Related pages

Tools

Export File

Citation

Format

Content

Catalog