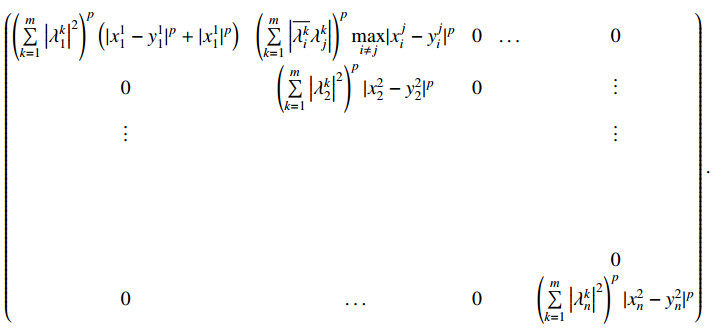On fixed point theorems in $C^{*}$-algebra valued $b$-asymmetric metric spaces

1.   Introduction

Fixed point theory has been the topic of extensive recent research due to the growing interest in its applications in various theoretical and applied fields, such as fractal theory, game theory, and mathematical modeling, the theory of approximation, nonlinear analysis, variational and linear inequalities, integral equations, differential equations, and dynamic systems.

The primary goal of fixed point theoretical basis is to improve and extend the conditions imposed on the spaces or applications under consideration. Several authors proved the Banach contraction principle in various generalized metric spaces. Bakhtin ^[3] introduced the concept of $b$-metric space and proved some fixed point theorems for some contraction mappings in this space. Other generalizations have been proven in the quasi-metric space introduced by Wilson ^[12]. This concept has many recent applications in both pure and applied mathematics. For example, the Hamilton-Jacobi equations ^[7], rate-independent plasticity models ^[5], shape memory alloys ^[8] and material failure models ^[11]. Several authors have made search in other directions, for example weaken assumptions or consider different contractions, see ^[1,2]. Ma et al. ^[6] established the concept of a $C^*$-algebra valued metric space and demonstrated certain fixed point theorems in 2014. They also used their results to determine the existence and uniqueness of solution for an integral type operator. Mlaiki et al. ^[9], have recently introduced the class of $C^*$-algebra valued partial $b$-metric spaces, which extends the class of partial metric spaces in $C^*$-algebra as well as the class of $b$-metric spaces in $C^*$-algebra.

Inspired by all the above concepts, we introduce the class of $C^{*}$-algebra valued $b$-asymmetric metric spaces and establish certain fixed point theorems. We present our non-trivial examples with an application whose $C^{*}$-algebra is noncommutative and an application for a certain integral equation.

2.   Preliminaries

Let us recall some basic definitions. $\mathbb{A}$ is a unitary $\mathbb{C}^{*}$-algebra with a unit $I_{\mathbb{A}}$ provided with a involution such that $a^{* *} = a$ and $(a b)^{*} = b^{*} a^{*}$ for all $a$ and $b$ in $\mathbb{A}$ and a complete multiplicative norm such that $\left\|a^{*}\right\| = \|a\|$ and $\left\|a^{*} a\right\| = \|a\|^{2}$ for all $a$ in $\mathbb{A}$. $\mathbb{A}_{h}$ is the set of all elements $a$ satisfying $a^{*} = a$, and $\mathbb{A}^{+}$ is the set of positive elements of $\mathbb{A}$, i.e., the elements $a \in \mathbb{A}_{h}$ having the spectrum $\sigma(a)$ contained in $[0, +\infty)$.

Note that $\mathbb{A}^{+}$ is a cone in the normed space $\mathbb{A}$ ^[10], which infers a partial order $\leq$ on $\mathbb{A}_{h}$ by $a \leq b$ if and only if $b-a \in \mathbb{A}^{+}$.

The following lemma will used to proof our main results.

Lemma 2.1. ^[10] Suppose that $\mathbb{A}$ is a unital $C^{*}-$ algebra with a unit $I_{\mathbb{A}}$.

(i) $\mathbb{A}^{+} = \left\{a^{*} a: a \in \mathbb{A}\right\}$;

(ii) if $a, b \in \mathbb{A}_{h}, a \leq b,$ and $x \in \mathbb{A},$ then $x^{*} a x \leq x^{*} b x$;

(iii) for all $a, b \in \mathbb{A}_{h},$ if $0_{\mathbb{A}} \leq a \leq b$ then $\|a\| \leq\|b\|$;

(iv) $0\leq a \leq I_{\mathbb{A}} \Leftrightarrow\|a\| \leq 1$.

3.   Motivation

Let $\mathbb{A} = L^{\infty}(\mathbb{R}) = \lbrace f:\mathbb{R} \rightarrow \mathbb{C}\, |\, \Vert f \Vert_{\infty} < \infty \rbrace$. Note that

$(i)$ $f \stackrel{\sigma}{\longrightarrow} \overline{f} \quad: t \rightarrow \overline{f(t)}$ is the involution

$(ii)$ $\left(\mathbb{A}, \Vert\: \Vert _{\infty}, \sigma \right)$ is a $C^{*}$-algebra

$(iii)$ $\leq$ denotes the partial order on $\mathbb{A}$ $\left(f\leq g \Leftrightarrow Re(f)\leq Re(g)\quad and \quad Im(f)\leq Im(g)\right)$.

Let $a$ and $b$ be two real numbers such that $a < b$, $X$ be the set of stepped functions defined on the interval $\left[a, b\right]$ i.e., $X = \lbrace f:\left[a, b\right] \rightarrow \mathbb{R}\:|\; \exists\:c_{0} = a < c_{1}\ldots < c_{n} = b\; and \; f\vert_{\left[ c_{i}, c_{i+1}\right[} = f_{i}\in \mathbb{R}\rbrace$. $\;{\rm{ Define }}\;d: X \times X \longrightarrow \mathbb{A}$ by

where $\lbrace I _{i}\rbrace_{n} = \left(\left[ c_{i}, c_{i+1}\right[ \right) _{0\leqslant i \leqslant n}$ is a subdivision adapted to $f$ and $g$ and $\chi_{\lbrace f\leqslant g\rbrace}$ indicator function of a set $\lbrace f\leqslant g\rbrace = \lbrace t \in\mathbb{R}/ f(t)\leqslant g(t)\rbrace$. Note that $d$ satisfies the following:

$(i)$ $d(f, g) = 0\Leftrightarrow f = g$

$(ii)$ $d$ is asymmetric. We can take $\left\{ \begin{array}{ll} f(t) = 2 \\ g(t) = 3 \end{array}\right. \Rightarrow d(f, g)(t) = 5 \; and\; d(g, f)(t) = 1$

$(iii)$ $d(f, g) \leq b\left[ d(f, h)+d(h, f)\right]$ for all $g, f, h \in X$ and a certain $b \in \mathbb{A^{+}}$ with $\Vert b\Vert > 1$.

We check the last assertion. Let $\left(\left[ c_{i}, c_{i+1}\right[ \right) _{i}$ be a subdivision adapted to $g, f \rm {and}\: h$ and $t$ in $X$. We can assume that t belongs in $\left[ c_{0}, c_{1}\right[$ then $f = f_{0};$ $g = g_{0}$ and $h = h_{0}$. We have 6 cases of which we are going to treat three cases (see Table 1). The other cases are done in the same way.

For the first case, we have

Since

then

For the second case, we have

Since

we have

For the third case, we have

Since

we have

4.   Main results

Definition 4.1. Let $X$ be a nonempty set and $b\in \mathbb{A^{+}}$ where $b\succeq 1_{\mathbb{A} }$. Suppose the mapping $d: X \times X \rightarrow \mathbb{A}$ satisfies:

(i) $0_{\mathbb{A}} \leq d(x, y)$ for all $x, y \in X$ and $d(x, y) = 0_{\mathbb{A}} \Leftrightarrow x = y;$

(ii) $d(x, y) \leq b\left[ d(x, z)+d(z, y)\right]$ for all $x, y, z \in X$.

Then $d$ is called a $C^{*}$-algebra valued $b$-asymmetric metric on $X$ and $(X, \mathbb{A}, d)$ is called a $C^{*}$-algebra valued asymmetric metric space.

It is obvious that a $C^{*}$-algebra-valued $b$-asymmetric metric space generalizes the concept of $C^{*}$-algebra valued asymmetric metric space ^[4].

Example 4.1. We consider the $C^{*}$-algebra as $\mathbb{R}^{2}$ provided with the partial order

Let $X = \lbrace a, b, c\rbrace$ ($a, b$ and $c$ are real numbers). We define $d : X\times X \longrightarrow \mathbb{R}^{2}$

$d$ is a $b$-asymmetric metric with $b = (3, 3)$ and not asymmetric metric

Proposition 4.1. Let $(X, \mathbb{A}, d_{i})$ be a $C^{*}$-algebra valued $b_{i}$-asymmetric metric space and $i\in\lbrace1, 2\rbrace$. Then the mapping $d: X \times X \rightarrow \mathbb{A}$ defined by

is $b_{1}+b_{2}$-asymmetric metric.

Proof. It is easy to verify that $d(x, y) = 0 \Leftrightarrow x = y.$ To verify condition $(ii)$ of Definition 4.1, we have (for all $x, y, z \in A$)

Proposition 4.2. Let $(X, \mathbb{A}, d)$ be a $C^{*}$-algebra valued $b$-asymmetric metric space and $\lambda$ a positive element in $Z(\mathbb{A}^{+})$ such that $\Vert\lambda\Vert\geqslant 1$. Then the mapping $d_{\lambda}: X \times X \rightarrow \mathbb{A}$ defined by

is $\lambda b$-asymmetric metric.

Proof. We recall that the product of two positive elements which commutes in a $C^{*}$-algebra is also positive. It is easy to verify that the conditions $(i)$ and $(ii)$ of Definition 4.1 are satisfied.

Definition 4.2. Let $(X, d, \mathbb{A})$ be a $C^{*}$-algebra valued b-asymmetric metric space, $x \in X$ and $\left\{x_{n}\right\}$ a sequence in $X.$ We say that

(i) $\left\{x_{n}\right\}$ $b$-forward (respectively $b$-backward) converges to $x$ with respect to $\mathbb{A}$ and we write $x_{k} \stackrel{F}{\rightarrow } x$ (respectively $x_{n} \stackrel{B}{\rightarrow } x$), if and only if for given $\epsilon > 0_{\mathbb{A}}$, there exists $k \in \mathbb{N}$ such that for all $n \geqslant k\,$

(ii) $\left\{x_{n}\right\}$ converges to $x$ if $\left\{x_{n}\right\}$ $b$-forward converges and $b$-backward converges to $x$.

(iii) $\left\{x_{n}\right\}$ $b$-forward (respectively $b$-backward) Cauchy sequence with respect to $\mathbb{A}$, if for given $\epsilon > 0_{\mathbb{A}}$, there exists $k$ belonging to $\mathbb{N}$ such that for all $n > p \geqslant k$

Definition 4.3. Let $(X, d, \mathbb{A})$ be a $C^{*}$-algebra valued $b$-asymmetric metric space. $X$ is said to be $b$-forward (respectively $b$-backward) complete if every $b$-forward (respectively $b$-backward) Cauchy sequence $\left\{x_{n}\right\}_{n \in \mathbb{N}}$ in X, converges to $x \in X$.

Definition 4.4. Let $(X, d, \mathbb{A})$ be a $C^{*}$-algebra valued $b$-asymmetric metric space. $X$ is said to be $b$-complete if $X$ is $b$-forward and $b$-backward complete.

Example 4.2. Let $G$ a nonempty set and $X = L^{\infty}(G), H = L^{2}(G)$ and $\mathbb{A} = B(H)$ the set of all bounded linear operators on the Hilbert space $H$. Note that $B(H)$ is a unitary $C^{*}$-algebra. We define a $b$-asymmetric metric $d_{b}: X \times X \rightarrow \mathbb{A}$ as

$(X, B(H), d_{b})$ is a complete $C^{*}$-valued $b$-asymmetric metric space with respect to $B(H)$. Indeed, $(f_{n})$ is a Cauchy sequence in $L^{\infty}(G)$ with respect to $B(H)$ then

We observe that for $A$ and $B$ included in a nonempty set $E$ such that and $A \amalg B$,

then for every $n > m\geqslant k$

then $(f_{n})$ is a $b$-Cauchy sequence in the space $X$. This implies that there is a function $f \in X$ and a natural number $N^{'}$ such that $\left\|f_{n}-f\right\|_{\infty} \leq \varepsilon$ for every $n \geq N^{'}$. It follows that

Therefore, the sequence $(f_{n})$ converges to the function $f$ in $X$ with respect to $L(H)$, that is, $(X, L(H), d_{b})$ is complete with respect to $L(H)$.

Definition 4.5. Let $(X, d, \mathbb{A})$ be a $C^{*}$-algebra valued asymmetric metric space. A mapping $T: X \rightarrow X$ is said to be forward (respectively backward) $C^{*}$-algebra valued contractive mapping on $X,$ if there exists $a$ in $\mathbb{A}$ with $\|a\| < 1$ such that

Example 4.3. Define $d:\mathbb{R}\times \mathbb{R}\rightarrow \mathbb{R}^{2}$ by

We consider $T :\, \mathbb{R}\rightarrow \mathbb{R}$ such as $Tx = \dfrac{x}{4}$. Then

As a result

So $T$ is forward and backward $C^{*}$-algebra valued contractive mapping on $\mathbb{R}.$

Theorem 4.1. If $(X, \mathbb{A}, d)$ is a $b$-complete $C^{*}$-algebra-valued $b$-asymmetric metric space and $T: X \rightarrow X$ is a $C^{*}$-algebra valued contractive mapping on $X,$ then T admit a unique fixed point in $X$.

Proof. Choose an $x_{0} \in X$ and set $x_{n+1} = T x_{n} = \cdots = T^{n+1} x_{0}, n = 1, 2, \ldots$.

For any $m \geq 1$ and $p \geq 1$, it follows that

In the same way $d\left(x_{m}, x_{m+p}\right)\rightarrow \theta_{\mathbb{A}} \quad(m \rightarrow \infty)$. Therefore $\left\{x_{n}\right\}$ is $b$-forward and $b$-backward Cauchy sequence with respect to $\mathbb{A}$. By the completeness of $(X, \mathbb{A}, d)$ there exists an $x \in X$ such that $x_{n}$ converges to $x$. Since

hence, $T x = x$, i.e., $x$ is a fixed point of $T.$ For uniqueness, we consider $x$ and $y$ two fixed points such that $x\neq y$ then

Thus, we get $1\leq\Vert a\Vert^{2}$, which is a contradiction.

5.   Application

As an application of Theorem 4.1, we find an existence and uniqueness solution for a type of matrix equation.

Let $M_{n}(\mathbb{C})$ be the set of all $n\times n$ matrices with complex entries with $n\geqslant 3$. $M_{n}(\mathbb{C})$ is a $C^{*}$-algebra with the operator norm $\Vert B\Vert = \underset{1\leqslant i, j\leqslant n}\max \vert b^{i}_{j}\vert$. Let $B_{1}, B_{2}, \ldots, B_{m} \in M_{n} (\mathbb{C})$ are diagonal matrices which satisfy $\sum\limits_{k = 1}^{m}\Vert B_{k}\Vert^{2} < 1.$ $M_{n}(\mathbb{C})^{+}$ is the set of all positive definite matrices "hermitian and the eigenvalues are non-negative". Then the matrix equation

has a unique solution.

Proof. Let $A = \left(a_{i}^{j}\right)_{1 \leq i, j \leq n}$ and $B = \left(b_{i}^{j}\right)_{1 \leq i, j\leq n}.$ If $\sum\limits_{k = 1}^{n}\Vert B_{k}\Vert^{2} = 0$, then it is clear that the equations has a unique solution in $M_{n}(\mathbb{C}).$

Suppose that $\sum\limits_{k = 1}^{n}\Vert B_{k}\Vert^{2} > 0.$ For $p \geq 1$, define $d_{b} : M_{n}(\mathbb{C}) \times M_{n}(\mathbb{C}) \rightarrow M_{n}(\mathbb{C})^{+}$ as

with

Then $\left(M_{n}(\mathbb{C}), M_{n}(\mathbb{C}), d \right)$ is a $C^{*}$-algebra valued $b$-asymmetric metric space and it $b$-complete with $b$ = $2^{p-1}I_{n}$.

Consider the map $T : M_{n}(\mathbb{C}) \rightarrow M_{n}(\mathbb{C})$ defined by $T\left(X \right) = \sum_{k = 1}^{m} B_{k}^{*} X B_{k}.$ Let $X = \left(x_{i}^{j}\right)_{1 \leq i, j \leq n}$ and $B_{k} = diag\left(\lambda^{k} _{1}, \lambda^{k} _{2}......., \lambda^{k} _{n}\right)$, observe that

so $if \left|x_{1}^{1}\right| < \left|y_{1}^{1}\right|$ $d(T X, T Y) =$

Then, we have

with

Therefore, $T$ satisfies the condition of Theorem 4.1. So it has a fixed point. So the matrix equations has a unique solution on $M_{n}(\mathbb{C})$. As a second application of Theorem 4.1, we find the existence and uniqueness solution for a type of following integral equation

where $G$ is a multiplicative group with its left invariant Haar measure $\mu$, $K: G \times G \times \mathbb{R} \rightarrow \mathbb{R}$ and $h \in L^{\infty}(G)$.

Let $X = L^{\infty}(G), H = L^{2}(G)$ and $\mathbb{A} = B(H)$ the set of all bounded linear operators on the Hilbert space $H$. Note that $B(H)$ is a unitary $C^{*}$-algebra. We define a $b$-asymmetric metric $d_{b}: X \times X \rightarrow \mathbb{A}$ (see example 4.2) by

Suppose that

$(i)$ There exist a continuous function $\psi: G \times G \rightarrow \mathbb{R}$ and $\alpha \in(0, \dfrac{1}{2})$ such that

$(ii)$ $\sup \limits_{u \in G} \int_{G}|\psi(u, v)| d \mu(v) \leq 1$.

Then, the integral equation $f(u) = \int_{G} K(u, v, f(u)) d\mu(u)+h(u), u, v \in E$ has a unique solution in $X$.

Proof. Define $T: X \rightarrow X$ by

Thus the integral Eq (5.1) admits a solution.

Acknowledgments

The authors Thabet Abdeljawad and Aiman Mukheimer would like to thank Prince Sultan University for paying the APC and for the support through the TAS research lab.

Conflict of interest

The authors declare no conflicts of interest.