1.
Introduction
Business cycle models have since time immemorial, encapsulated strong flavor of non linearity no matter what portion of economy they are serving. Prima facie, monetary events and the movement of economy makes the former idea digestible. Demand and supply shocks, an unpredictable market and dual structure of financial system obviously do not follow a pattern and are best left to non linear business cycle models. Recent exploration (Lopes and Zsurkis (2019)) however debates this perception and does not show inclination towards the slapdash acceptance of non linearity of business cycles. Their results glorify that fact of business cycle models not being non-linear every time. Authors suggest that India and seven other countries have weak evidence of non linearity at certain frequencies. Obviously this study (Lopes and Zsurkis (2019)) had limitations and authors have adopted a purely testing approach and their study was model independent. While we do not wish to endorse linearity over non linearity or vice-versa, it is important to understand that business cycles replicate the phenomenon or event for which they are created imbibing respective arguments. It can be both linear and non linear. However, linking of important events like gold price fluctuations with business cycle asymmetries (Apergis and Eleftheriou (2016)) have placed non linear business cycle models at an intangible elevated space. Linear models await such an exploration which puts them at an exposition which is equal if not greater than non linear business cycle models.
To follow our pursuit we have chosen an augmented Investment Saving-Liquidity Preference Money Supply model. The kaleidoscope of Indian markets is to a considerable extent captured by the IS-LM model (Ahmed (2005)). The author (Ahmed (2005)) takes a firm dig on the audacity of IS-LM model to explain macroeconomic cycles in our country. In the wake of these facts, we have proceeded with the same. This model has then been subjected to linear and non linear arguments for investment, savings and liquidity and their dynamics have been illustrated with relevant comparison.
Research on IS-LM models has been resonating under similar perspectives in the last few years and needs an uplift. We now discuss in brief how the proposed research work provides an increased edge to the existing works and paves way for new avenues to be explored under the following domains:
● Nature of arguments In recent works a delayed IS-LM model with general investment function but linear functions for liquidity and savings has been proposed (Riad et al. (2019)) with single delay in investment function. The arguments are mixed in nature. In another proposal (Kaddar et al. (2008)), a mixed IS-LM business cycle model has been considered with single delay in gross product, capital stock and rate of interest but again functions for investment, savings and liquidity function are linear in nature. A similar model (Kaddar and Alaoui (2008)) with anticipated capital stock has also been explored with linear arguments. In an attempt, Hattaf et al. (2017) have considered a generalized business cycle model with delays in gross product and capital stock and general investment function. To quote Abta et al. (2008), Cai (2005) and all the aforementioned works have explored different augmented versions of IS-LM model under similar umbrella of linear arguments and general investment functions. There has been trifle discussion on non linearity of liquidity function and almost negligible on non linearity of savings function. Latter involving complex numerical simulations and hence less preferred. This limits the scope of research done so far. Savings never develop at regular intervals and appropriate portions and ask for a spatial view in the form of non linear relationships. Encapsulating unusual demand and supply events, preference for money or liquidity is not assumed to follow a pattern. Our proposed work considers this issue, encapsulating nonlinear arguments for investment, liquidity and savings.
IS-LM model with dual delays have not been explored in abundance and there are few works to quote. In an attempt, Hattaf et al. (2017) have considered a generalized business cycle model with delays in gross product and capital stock but with a generalized investment function. We believe that a clearer picture of economy is possible pertaining to specific functions as it aids in narrowing down target economy which may be applicable to various cities within a country or various countries globally. Increasing the number of delays into the system creates a more realistic picture of economy. Moreover, a system with dual delay can stabilize the system earlier, elevating sensitivity of the same, than a similar system with single delay. This statement has been discussed in detail later in the numerical section of this paper. Our work involves dual delays in the equation for capital accumulation.
● Parameter sensitivity analysis In all of the works discussed above system stability is investigated and various conditions under which the system undergoes hopf bifurcation have been discussed but there is trifle emphasis or elaboration on the parameter sensitivity of the same. It is of paramount importance to understand the interaction of parameters such as the adjustment coefficient in goods market (α), adjustment coefficient in money market (β), depreciation of capital stock (δ)etc with the IS-LM model as they contribute in majority to the dynamics of the system. This arena has been neglected and has a lacklustre appearance so far. We have performed parameter sensitivity analysis for parameters common and most sensitive to both the models. Also, we have shed some light on the individual personality of the aforementioned parameters.
● Choice of linear or nonlinear model To the best of our knowledge, no work has been considerably attempted in this arena. In all of the research data so far on IS-LM model, never have a linear and nonlinear model been compared under a similar economic framework. Comparative analysis of such a kind not only accentuates importance of choosing of linear/nonlinear models in given economic environment but also aids in policy making, once an economy is identified as either of the one. In an neophyte attempt, we have unravelled the dynamics of an amalgamated IS-LM model while comparing its linear and non linear counterparts simultaneously.
Classification of this paper is as follows. Section 2 explains the amalgamated IS-LM model and two time delays in the equation of capital accumulation. We have introduced linear and non linear arguments in Section 2.2 and Section 2.3 classifying them as M1 and M2. Qualitative behavior of both the models including steady state and local stability has been fairly discussed in Sections 2.2 and 2.3. Three cases have been investigated pertaining to two time delays and conditions for hopf bifurcation have been put up. Numerical simulation for both the models finds a fair portion in Section 3. Also, this section is gratified by comparison resulting from the dynamics of both the models. Decent discussion on model behavior for different values of τ1 and τ2 has been attempted. A detailed overview on the sensitivity of the major parameters that are common to both the models is also attached with this section. Conclusion is laid out in Section 4. Proof of common lemmas and theorems used in Section 2.2 and Section 2.3 is attached with portion of Appendices.
2.
Mathematical model and qualitative analysis
Following the proposed idea, we proceed with the model inspired from Zhou and Li (2009). Considering an augmented IS-LM model with dual delay, this model accentuates the factor of capital accumulation as the proposed delays reside in the equation for capital stock. The delays depict the time frame when capital is utilised for production process and the time period when capital is available for production. Cai (2005) studied the augmented IS-LM model (Combination of the standard IS-LM model and Kaldor's model) with time lag in capital accumulation inspired from Kalecki's idea of delay. Here the author made an assumption that saved part of the profit is being invested and capital growth is attributed to past investment decisions. In the equation for capital stock, investment depends on the income at the time investment decisions are taken and on the capital stock as well, at the time when investment is finished.
On the contrary, Zak (1999) explored Solow growth model coupled with time delay and assumed that investment depends only on capital stock at the past time. An incubation period is required in order to produce and install capital goods and the author further considers that a portion of capital stock depreciates during this incubation period.
Here, at time "t" the productive capital stock is k(t−r).
Amalgamating this concept and that proposed in Cai (2005), Zhou and Li (2009) assumed that the investment function given in the capital accumulation equation depends on income as well as capital stock at past period and maturity period.
where −1<β1<0 is propensities to investment I2(K) with respect to capital stock. Time delays are demonstrated by τ1 and τ2. Hence, the resulting IS-LM model under investigation is
Here, the delay "τ1" represents the time period in which decisions for investment were taken and delay "τ2" represents the time employed in order so that the capital is used for production. I, S, L, K, Y, r and ¯M represent investment, savings, liquidity preference (demand for money), capital stock, National income, interest rate and constant money supply. Here, α implies adjustment coefficient in the goods market (α>0), β implies adjustment coefficient in the money market (β>0), μ or β1 implies propensities to investment (−1<μ or β1<0) and δ implies depreciation rate of the capital stock (0≤δ≤1). We have used different symbols for the same parameter i.e propensities to investment, as Model M1 already contains β1 as a positive parameter in the form of linear argument for investment. In order to avoid any confusion 'propensities to investment' is denoted by μ in Model M1 and is denoted by β1 in Model M2.
2.1. Model M1:Linearmodel: steady state and local stability of the model
Cai (2005) constructed an augmented IS-LM model by incorporating kalecki's idea of delay into it. It has single delay in investment function while arguments for National Income (Y), rate of interest (r) and capital stock(K) depend linearly on their variables. The arguments for our linear model are inspired from Cai (2005) and are given by
Following parameters have been used in model M1 (Table 1),
The system (4) on combination with (5) has an equilibrium (Y∗,r∗,K∗) given by,
We linearize the system (4) around E∗=(Y∗,r∗,K∗) by taking y=Y−Y∗,k=K−K∗ and r=R−R∗.
The characteristic equation corresponding to the system (5) becomes:
where
We know consider three cases pertaining to two time delays,
Case 1: τ1=τ2=0
The characteristic equation (9) transforms into
Using Routh-Hurwitz criterion, it is easily understood that
When condition K1 is satisfied, equilibrium E∗(Y∗,r∗,K∗) is locally asymptotically stable.
Case 2: τ1=0,τ2≠0
Putting τ1=0 in (9), we obtain the characteristic equation
Let λ=iω be the solution of (15). Putting it in (15) and separating the real and imaginary parts, we obtain:
It is relevant to mention here that in order to have ω as a solution of (15), ω must be a root of the equation below:
Let x=ω2, we obtain
where P,Q and R stand for,
Inspired from De Cesare and Sportelli (2005), following assumptions are taken
Here △=F(k)24+F′(k)39,k=−43 following with the reduced form (19) and obtain that,
Lemma 2.1. We consider the following results for Equation (19)
a. Equation (19) will have only one positive real root ω1 provided, (K2) exists;
b. Equation (19) will have two distinct positive real roots ω2,ω3(settingω2>ω3), provided (K3) exists;
c. Equation (19) will have three distinct positive real roots ω1<ω2<ω3, provided (K4) exists;
d. Equation (19) will have no positive real root, given (K5) exists.
It is pertinent to note here that a solution ω belonging to Equation (19) is also a solution of Equation (15) (characteristic equation) provided τ∗2=ϕ(ω∗)+2nπω∗n ϵN,ϕ∈[0,2π].
Lemma 2.2. Let f(λ,τ)=λ2+aλ+bλe−λτ+c+de−λτ where a, b, c, d and τ are real numbers and τ≥0. Then, as τ varies, the sum of the multiplicities of zeroes of "f" in the open right half-plane can change only if a zero appears on or crosses the imaginary axis.
Following Lemma 2.1 and in accordance with Lemma in Ruan and Wei (2003) which is stated above as Lemma 2.2, we deduce that.
Lemma 2.3. Following Equation (15), we have
a. Equation (15) has only one pair of purely imaginary roots ±ιω1, provided (K2) exists and τ2=τ12,n.
b. Equation (15) has two pairs of purely imaginary roots ±ιω2,±ιω3, provided (K3) exists and τ2=τ22,n,τ32,n.
c. Equation (15) has three pairs of purely imaginary roots ±ιω1,±ιω2,±ιω3, provided (K4) exists and τ2=τ12,n,τ22,n,τ32,n.
d. Equation (15) has no purely imaginary roots, provided (K5) exists.
We have,
Lemma 2.4. Let τ∗2 demonstrates an element belonging to either sequence {τ12,n} or {τ22,n} or {τ32,n} we then obtain the following transversality conditions, which are satisfied:
Proof. Refer Appendix A
Lemma 2.5. For Equation (15), we proceed with the following
a. Provided (K1) and (K2) exists, all roots of Equation (15) have negative real parts when τ2∈[0,τ12,0) and when τ2>τ12,0 there exist at least one root with positive real part for Equation (15).
b. Provided (K1) and (K3) exists, then there will be k switches from stability to instability when parameters such that τ32,0<τ22,0<τ32,1<......<τ32,k−2<τ22.k−2<τ32,k−1<τ32,k<τ32,k−1, all roots of Equation (15) possess negative real parts when τ2∈(τ22,n,τ32,n+1),τ22,k−1=0,n=−1,0,.......,k−1. Equation (15) has at least one root with positive real parts when τ2∈[τ32,n,τ22,n),n=0,1.....k−1 and τ2>τ32,k.
c. Provided (K3) exists and (K1) ceases, when the parameters such that τ22,0<τ32,0<τ22,1<......<τ22,k−1<τ32,k−1<τ32,k<τ22,k, there may exist k switches from instability to stability when τ2∈[τ32,n,τ22,n+1) and τ2>τ32,k−1,τ32,k−1=0,n=−1,0,.......,k−2. Equation (15) has at least one root with positive real parts. All roots of Equation (15) have negative real parts when τ2∈[τ22,n,τ32,n),n=0,1,........k−1.
d. Provided (K1) and (K4) exists, there exists at least one stability switch.
Proof. Refer Appendix B
In accordance with the dynamic analysis above and emphasising on the theorem (Hale (1971)) we put forward the following results:
Theorem 2.1. When the conditions occurring in Lemma 2.5 (a, b, c) are satisfied then,
a. When τ2∈[0,τ12,0), we obtain a locally asymptotically stable equilibrium (Y∗,r∗,K∗) and Hopf bifurcation occurs when τ2=τ12,0 at (Y∗,r∗,K∗).
b. When τ2∈0⋃[τ22,n,τ32,n+1),τ22,k−1=0,n=−1,0……..k−2 we obtain a locally asymptotically stable equilibrium (Y∗,r∗,K∗) and hopf bifurcation occurs when τ2=τm2,n⋃τ32,k−1m=2,3,n=0,1........,k−2.
c. When τ2∈[τ22,n,τ32,n),n=0,1......k−1 we obtain a locally asymptotically stable equilibrium (Y∗,r∗,K∗), hopf bifurcation occurs when τ2=τm2,n,m=2,3,n=0,1…….,k−1.
Case 3: τ1≠0,τ2≠0 For this case we consider τ1 as parameter and take τ2 in stable region, pertaining to Equation (9). Following Ruan and Wei (2003) we obtain,
Lemma 2.6. There exists a τ∗1(τ2)>0, provided for τ2>0 all roots of Equation (9) have negative real parts, such that when 0≤τ1<τ∗1(τ2) all roots of Equation (9) have negative real parts.
Proof. Refer Appendix C
Theorem 2.2. Considering (K1) holds true,
a. Provided (K2) exists, then for any τ2∈[0,τ12,0), there exists a τ∗1(τ2), such that we obtain a locally asymptotically stable equilibrium for (4) when τ1∈[0,τ∗1(τ2)).
b. Provided (K3) exists, then for any τ2∈[τ22,n,τ32,n+1), there exists a τ∗1(τ2), such that we obtain a locally asymptotically stable equilibrium for (4) when τ1∈[0,τ∗1(τ2)).
c. Provided (K5) exists, then for any any τ2≥0, there exists a τ∗1(τ2), such that we obtain a locally asymptotically stable equilibrium for (4) when τ1∈[0,τ∗1(τ2)).
Proof. Refer Appendix D
Remark 2.1. Indeed, Hopf bifurcation occurs at τ∗1(τ2) if conditions of Lemma 2.6 or Theorem 2.2 are fulfilled. Multiple stability switches may exist. Also, there may exist no τ∗1(τ2) if we let τ2 in unstable region such that when the system (4) is stable in τ∗1(τ2)<τ1 it's unstable in 0≤τ1<τ∗1(τ2).
Now, we proceed with the analysis of model M2.
2.2. Model M2:nonlinearmodel: steady state and local stability of the model
In Rocsoreanu and Sterpu (2009) the author imbibes single delay in equation for capital accumulation in a modulated IS-LM model. We adhere to nonlinear arguments, inspired from the same Rocsoreanu and Sterpu (2009)
where ˆr is very small rate of interest generating the liquidity trap.
Following parameters have been used in model M2 (Table 2),
The system (30) has an equilibria (Y∗,r∗,K∗) given by,
We linearize the system (30) around E∗=(Y∗,r∗,K∗) by considering x=Y−Y∗,y=K−K∗andz=R−R∗ and obtain,
where
Characteristic equation pertaining to system (34) is given by
For investigating the root distribution of system (39), we recall the lemma Ruan and Wei (2003) stated in previous Section (Lemma 2.2). Following the methodology used in Song and Wei (2004), three cases have been elaborated,
Case1: τ1=τ2=0
Substituting τ1 = τ2=0 in (39), characteristic equation becomes,
It can be easily verified that (p3+q2+r3)>0,(p1+r1)>0. Thus, following Routh-Hurwitz criterion all the roots of (42) have negative real parts if the following condition holds:
We can say that when condition (C1) is satisfied, equilibrium point(Y∗,r∗,K∗) is locally asymptotically stable.
Case2: τ1=0,τ2>0
(39) transforms into
Assuming λ=ιω(ω>0) to be a root of (45), we have,
leading to,
Assuming ω2=v, then (48) is reproduced as,
We know that f(0)=C, lim→f(v)=+∞ and from (49) it follows that,
Discussing about the roots of (50) as done in Song and Wei (2004), we put forward the following lemma.
Lemma 2.7. We hold the following results for polynomial (49):
If (C2):C≥0,△=A2−3B≤0 holds, then (49) has no positive root;
If (C3):C≥0,△=A2−3B>0,v∗=−B+√△3>0,f(v∗)≤0
or
(C4):C<0 holds, then (49) has a positive root.
Proof. Let us assume that (49) has positive roots. Without loss of generality, we suppose that it has three positive roots, namely v1,v2 and v3.
(48) then has three positive roots ωk=√vk,k=1,2,3.. Corresponding critical value of time delay τ(j)2k is given by
k = 1, 2, 3; j = 0, 1, 2
Hence, ±ωk is a pair of purely imaginary roots of (51) with τ2=τ(j)2k and let τ2.0=mink∈[1,2,3]{τ(0)2k},ω0=ωk0
Lemma 2.8. Suppose that (C5) f′(ω20)≠0 then we have the following condition for transversality: {d(Reλ)dτ2}λ=ιω0
Proof. Refer Appendix E
In accordance with Lemmas 2.7–2.8, Lemma in Ruan and Wei (2003) and considering the Hopf bifurcation theorem Hale (1971), Hassard BD et al. (1981), Zhang and Bi, (2012) we put forward the following results.
Theorem 2.3. Pertaining to system (30), τ1=0.
(1) The positive equilibrium (Y∗,r∗,K∗) is asymptotically stable for all τ2≥0, provided (C2) holds.
(2) If the positive equilibrium (Y∗,r∗,K∗) is asymptotically stable for all τ2∈[0,τ2.0] and unstable for τ2>τ2.0, provided (C3) or (C4) and (C5) hold. Also, when τ2=τ2.0 the system (30) undergoes a Hopf bifurcation at the positive equilibrium (Y∗,r∗,K∗).
Pertaining to the methodology inspired from Li (2019), we put up that
Define
Theorem 2.4. a. Let us assume that (48) has at least one positive root depicted by ¯νk(1≤k≤3). There exists a nonempty set Sτ2and [0,τ20)⊆Sτ2], it follows that when τ2∈Sτ2 the positive equilibrium of (30) is locally stable, and a Hopf bifurcation exists when
b. Let us assume that (48) has only one positive root given by ¯νk. There exists a nonempty set Sτ2and Sτ2=[0,τ(0)2), it follows that when τ2∈Sτ2 the positive equilibrium (30) is locally stable and unstable when τ2>τ(0)2 and system (30) undergoes Hopf bifurcation when τ2=τ(n)2(n=0,1,2,.....).
Assuming that the condition (p3+r3+q2)(p3+r3−q2)<0 holds (refer (49)), then
In the context of stability switches, it is relevant to mention here that if there exist more than one positive root for (49), it will give rise to finite stability switches when τ2 time delay passes through critical points
and [0,τ20)⊆Sτ2]. If A,B>0 and condition (p3+r3+q2)(p3+r3−q2)<0 holds, (49) has only one positive root, then there exist no stability switch when τ2 the time delay passes through critical points when τ2=τ(n)2(n=0,1,2,.....) and Sτ2=[0,τ(0)2).
Case 3: τ1,τ2≠0
Here τ1>0 and τ2 is fixed, taken in stable region and τ2∈Sτ2,τ1≠τ2 Characteristic equation (39) is given by
Pertaining to the methodology used in Li (2019), we now assume that λ=ιω(ω>0) is a root of (56). Solving on the lines of Case 2, we obtain
where E1=(p1ω2−p3),F1=(r1ω2−r3),G1=r2ω,H1=q2,I1=q1ω,J1=(ω3−p2ω). we have,
The values are given by,
f5=−2r1sinωτ2,f4=p21+r21−2p2+2(p1r1−r2)cosωτ2,f3=(−2p1r2+2r3+2p2r1)sinωτ2
f2=−2p1p3+p22−2r1r3+r22−q21+(−2p1r3−2p3r1+2p2r2)cosωτ2,f1=2(p3r2−p2r3)sinωτ2
f0=p23+r23+2p3r3cosωτ2−q22
Assuming that the condition (p3+r3+q2)(p3+r3−q2)<0 holds, then
and Fτ1(τ2)(+∞)=+∞. Hence, (58) possess at least one positive root. We now assume that (58) has N1(N1∈N+) different positive roots, depicted by ωk=√ωk(k=1,2,.....,N1) and we obtain
Hence, we have
When ω=ωk, the direction of τ(n)1k(τ2) passing through the imaginary axis (Wang (2012)) is determined by
Then sign(△kτ1(τ2))≠=0, since ωk(k=1,2......N1) are N1 distinct positive roots belonging to (58). And a Hopf bifurcation occurs for the system (30) when τ1=τ(n)1k(τ2). Define
when τ1∈Sτ1(τ2) the positive equilibrium is locally stable. Its relevant to mention here that if there exist more than one positive root for (58), it will give rise to finite stability switches when τ1 time delay passes through critical points
and [0,τ10(τ2)⊆Sτ1(τ2)]. If fi>0(i=1,2,.....,5) and the condition (p3+r3+q2)(p3+r3−q2)<0 holds, (58) has only one positive root, then there exist no stability switch when τ1 the time delay passes through critical points when τ1=τ(0)1(τ2)(n=1,2.....) and Sτ1(τ2)=[0,τ(0)1(τ2)).
Theorem 2.5. a. Let us assume that (58) has at least one positive root depicted by ωk(1≤k≤N1). There exists a nonempty set Sτ1(τ2)and [0,τ10(τ2)⊆Sτ1(τ2)], it follows that when τ1∈Sτ1(τ2) the positive equilibrium of (30) is locally stable, and the same undergoes Hopf bifurcation at the positive equilibrium when
b. Let us assume that (58) has only one positive root given by ω1. There exists a nonempty set Sτ1(τ2) and Sτ1(τ2)=[0,τ(0)1(τ2)), it follows that when τ1(τ2)∈Sτ1(τ2) the positive equilibrium (58) is locally stable and unstable when τ1>τ(0)1(τ2) and system (30) undergoes Hopf bifurcation at positive equilibrium when τ1=τ(n)1(τ2))(n=0,1,2,.....).
3.
Numerical simulation for model M1 and model M2
3.1. Model M1
The dynamics of the model are studied for the following set of parameters (Table 3).
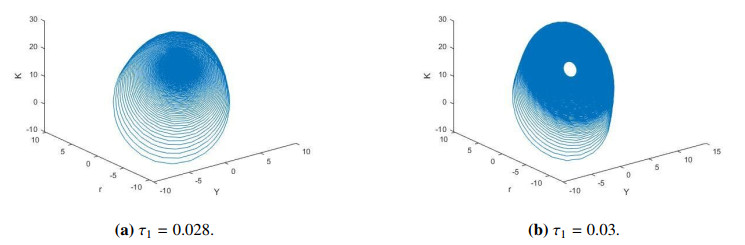
In the absence of delay, for initial condition [10,5,6], we have a positive equilibrium E∗ resting at E∗=(0.5596,0.1225,1.5161). However, in Case Ⅱ (Table 4), when τ1=0 and τ2≠0, we observe that the system initially stable, exhibits bifurcation for some period of time and then enters a stable window and remains there (Figure 1). System observes two stability switches (Lemma 2.5).
Now, we consider case 3 and consider τ2=0.3 for our numerical example and obtain a positive equilibrium (Y∗,r∗,K∗) resting at (0.0179,0.0237,0.0250). Pertaining to Case Ⅲ (Table 5), we keep τ2=0.3 in the stable region and modulate τ1 and observe that the system fluctuates in terms of stability and gives rise to many stable and unstable small periodic windows leading to four stability switches (Lemma 2.5). Consequently, we obtain a stable channel (Figure 2).
Remark 3.1. The delay τ2 demonstrates capital available after time τ2 has passed or to be precise, the capital available for production at given instance of time. Nevertheless to state that capital cannot immediately be consumed for production and requires time to process. When a single delay is acting upon the system, it becomes stable after a brief period of bifurcation.This clearly states that only a specific amount of delay can be applied to capital stock. Hence, the capital stock should be made available within a certain time period so that it can ensure considerable output.
The above (Table 5) shows that incorporating second delay in investment function, destabilizes the system at alternate intervals. Investments are a result of decisions taken in the past which then affect National Income. Also, capital is the accumulation of past investment or more likely to say from the return of past investment. Our dynamics reveal that system stabilization takes considerable amount of time in Case 3 in comparison to Case 2 which means that range of delay should not be so long that it brings chaos in the behaviour of the system. Failing to implement investment after its decision has been made, can cause system equilibrium to disrupt (Figure 3).
Now, in the next subsection, we would be discussing Model M2.
3.2. Model M2
All the parametric values have been taken same except those involved in non-linear terms and β=2 which is an exception. Still for convenience all the parametric values (Table 6) are given below.
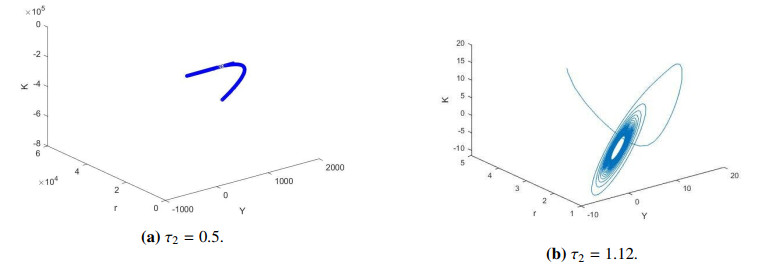
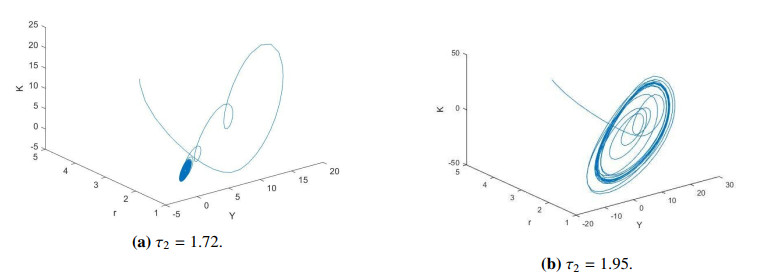
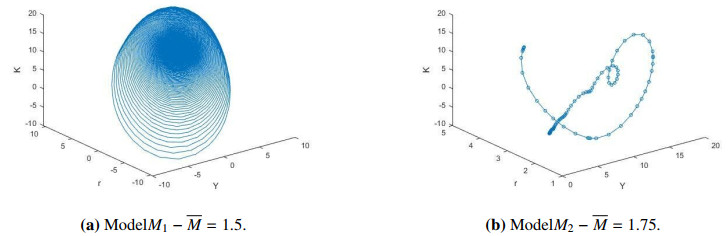
For the same initial conditions, t=(0 500), we have positive equilibrium E∗ at E∗=(11.7172,1.6072,13.2037) for Case 1 when no delay is present. Considering Case Ⅱ (Table 7), we observe that the system is again fluctuating in terms of stability and creates ample number of stable and unstable small periodic windows leading to four stability switches (Theorem 2.4). Here it is pertinent to mention that the instability of the system arises little late in comparison to Model M1 (Table 4) but its stabilization also occurs late which (Figure 4, Figure 5) can be attributed to the non-linearity of the system. It can be said that in our model, the linear system achieves stability earlier without much conflict when single delay is present. The equilibrium point obtained is (0.025396,0.000298,0.018889) at τ2=1.7. The periodic windows of stability and instability are wide which implies that a non-linear system gives considerable amount of time for decision making and policy implementation in this case. Also, pertaining to these wide windows policy makers can find some extra time to rectify anomalies in economic policies when detected.
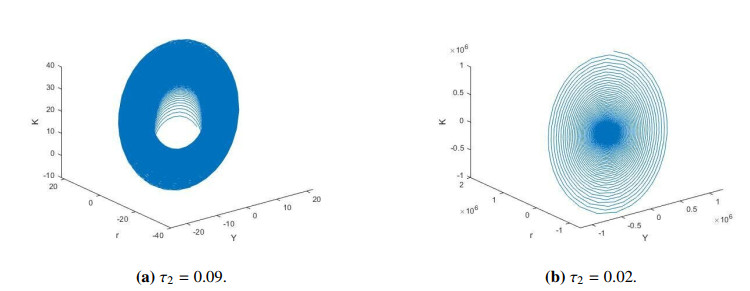
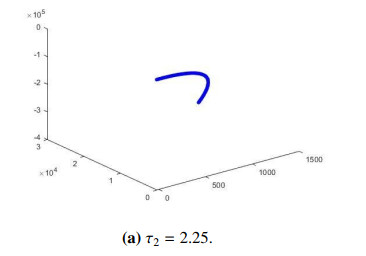
Remark 3.2. When only capital stock imbibes delay, the system fluctuates from stability to instability finally leading to a stable system. Numerical results vouch that out of all the stable windows (Figure 4, Figure 5, Figure 6) i.e 0≤τ2≤0.9, 1.14≤τ2≤1.72 and τ2≥2.19 only τ2=1.7 yields positive equilibrium value. Although the system exhibits stability for others value of τ2, but encapsulates negative equilibrium points which cannot reflect a practical picture of economy. Hence, we have chosen τ2=1.7 as our value in stable region. This picture again gives rise to the scene of only a specific amount of delay being applicable to capital stock. Failing to which, we will not be able to achieve desired income even when capital is available.
Now, we consider the last case of M2 and obtain positive equilibrium E∗(0.0800,0.0009,0.0287) for τ1=0.02 and τ2=1.7.
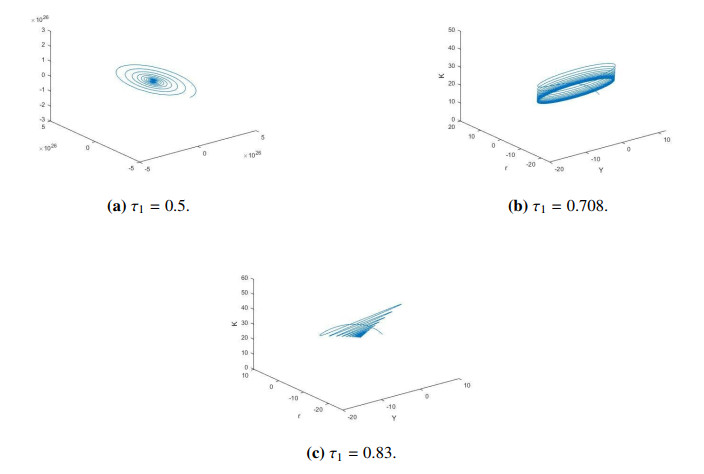
Remark 3.3. CaseIII (Table 8) numerical simulation of Model M2 shows that when both delays come into picture the stability disappears at very initial level. System portrays bifurcation for a while and then proceeds towards stability, residing there(Figure 7). As compared to M1 (Table 5), there are fewer windows of changing system dynamics and stability returns to place soon(Figure 7c). As mentioned previously, in case of single delay linear model M1 achieves stability sooner whereas in case of dual delay non linear model has this feather in its cap.
A non linear savings function apart from output and capital accumulation function contribute to a more realistic situation in economic framework. Savings never happen equally at equal intervals of time and follow a non linear pattern throughout the given tenure. Our results vouch that stability can be achieved with ease provided investment decisions be taken on time and quickly since the periodic windows in this case are short and require immediate action.
Introduction of non linearity stabilizes the system (τ1=0.44) as compared to its linear counterpart (τ1=0.72).
Now, we would be discussing the comparison of both the systems based on a few parameters which we consider important to discuss. In this novice attempt, we have managed to sustain this exploration of dynamics with same parametric values i.e consider same values for parameters that are common to both the models namely α=1,δ=0.9,β1orμ=−0.5,¯M=2 with β being an exception as its value is different for both. Narrowing down to the same initial conditions (Y=10,r=5,K=6) and time frame (0,500) we have tried to keep the economic framework as similar as possible through rigorous mathematical work for better investigation. Along with that, we would also try to investigate which model is more sensitive to the parameters stated above. To quote, we restrict ourselves to the scope of this paper and our vested interests.
1. Initial condition Although we have managed to cater to same set of initial condition values i.e (10, 5, 6) for both models, our results advocate the fact of linear model being less sensitive to change in initial conditions. Values discussed below stand in the case of absence of both the delays.
Remark 3.4. Nonlinear model shows sharp change (Table 9) in equilibrium points as subjected to change in initial values vouching for the sensitivity of the same. Its counter part, model M1 shows a very minor change in equilibrium points when exposed to a similar condition providing concrete evidence for it being less sensitive in general.
2. Parameters and Sensitivity Analysis Through intensive MATLAB simulations we obtain that out of all the parameters common to both the models the ones that are most sensitive stand to be ¯M, δ, β1 or μ. They alter system dynamics significantly when modulated even to the slightest number. We study these effects when both the delays are present in the system and lie in their respective stable regions. Following parametric values (Table 10, Table 11) have been considered for both the models.
● δ - Depreciation of capital stock (δ=0.9)
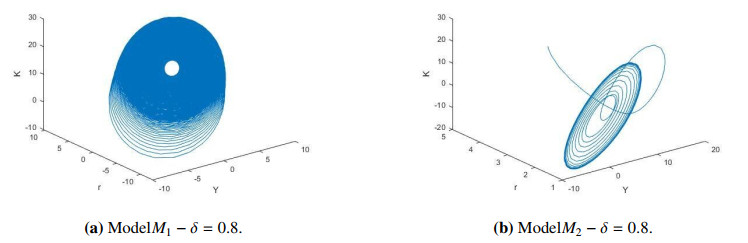
Naturally, capital starts depreciating as soon as it is formed. Under the umbrella of capital depreciation many heterogeneous factors find place. Either arising out of deterioration(associated with ageing, use, maintenance etc) or obsolescence(technological issues, evolving international trade etc), capital stock depreciates at a rate δ. On Increasing the value we have the following dynamics (Table 12).
Remark 3.5. It is pertinent from the above values that system proceeds towards stability (Figure 8) on increasing the parameter under discussion. We have observed the dynamics for both the models in previous section at δ=0.9 and the range for the same varies between 0≤δ≤1. As we start increasing the value form δ=0.9 to δ=1, naturally there is less scope for both the models to show drastic changes in system dynamics. Both the models M1 and M2 continue to follow stable dynamics for δ>0.9 as their predecessor δ=0.9.
On Decreasing the value we have the following dynamics (Table 13),
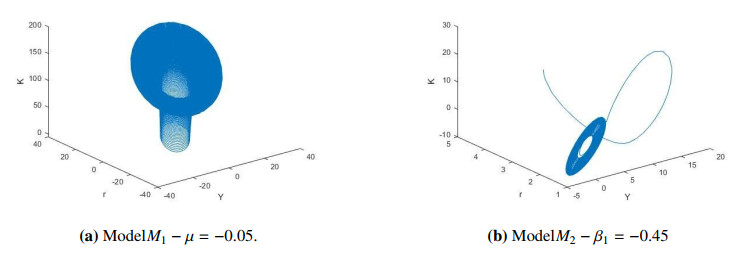
Remark 3.6. Ref 4, as the value of depreciation constant decreases it leads to an increase in capital stock. However, this capital is not readily available for production and would be available after a time period. Hence, the system shows bifurcation initially when the capital stock is increasing readily and becomes stable afterwards as the capital starts being utilised. For model M2, non linearity induces instability in the system finally leading to chaotic behaviour for lower values of δ.
● ¯M - Money Supply (¯M=2)
As per the liquidity preference theory (Keynes' Liquidity Preference Theory of Interest Rate Determination (2016)), people prefer to dwell in liquid or cash every time given an option. We require money for transactionary, precautionary and speculative motives. However, this demand for money is fulfilled either by the currency issued by the government or the policies introduced by the central bank which increase money supply and cannot be done privately. This fulfillment of money done by the government is termed as "Money Supply". Rate of interest and money supply are negatively related and this relation can be very well understood by the expression, PriceofBonds=1RateofInterest.
On Increasing the value (Table 14) we have the following dynamics,
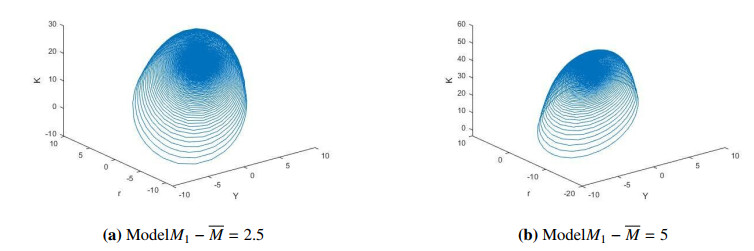
In model M1, as we increase money supply the system lies in a stable window (Figure 10) following the liquidity preference curve. An increased money supply means decrease in rate of interest (See 4). For better understanding of this concept, we proceed in reverse direction of the result "Increased money supply will result in decreased rate of interest".
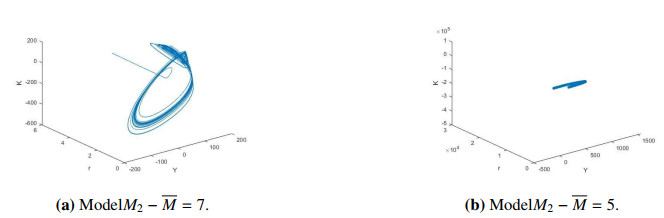
Rate of interest if is decreasing, then people speculate that it will increase in near future. Following the relation PriceofBonds=1RateofInterest, an increase in rate of interest would mean that prices of bond would decrease. Hence people will start selling their bonds and keep cash with them because the value of their bonds will decrease in near future. This results in greater demand for money (Liquidity Preference Curve) which would ask for an increased money supply and our results are in accordance with the model. The value of equilibrium for ¯M=2.5 is (0.0051, 0.0106, 0.0106) and for ¯M=5 is (−0.0127,−0.0302,−0.0297) which further advocates our results in favor of the model i.e the value of r∗ decreases with increasing value of ¯M.
In model M2, as soon as non linearity enters the scenario we see periodic windows (Table 14) of stability and instability. Majority of them being unstable. An adept explanation of such a nature, while its linear counterpart stands to stable lies in the movement of LM curve of the IS-LM model. LM curve shifts due to two reasons namely change in money demand and change in money supply. Here, however change in money supply takes place in form of increased money supply which will shift LM curve to the right. Shifting of LM curve to the right brings with it lower interests rate, increased investment and increased level of national income. Taking a closer look at equilibrium values of National income or output (Y), we observe that they occur in a bizarre pattern (Table 15) rather than following an increased income trend. Hence, non linearity disrupts the system and induces bifurcations (Figure 11).
On Decreasing the value (Table 16) we have the following dynamics.
In model M1, as we decrease money supply the system lies in a stable window (Figure 12) again. A decreased money supply ensures increase in rate of interest (See 4). We rely on the reverse direction of this result "Decreased money supply will result in increased rate of interest".
Rate of interest if is increasing, then people speculate that it will decrease in near future. Following the relation PriceofBonds=1RateofInterest, a decrease in rate of interest would mean that prices of bond would increase. Hence people will start purchasing bonds and securities to adhere to profits of increased bond values in near future. Hence, there is lesser demand for money as per the LPC and the system is stable.
In model M2, as we decrease money supply it gives rise to a stable region. In this arena non linearity is stabilizing the system. We adhere to a similar course of events related to shifting of LM curve but to the left in this case. A decreased money supply will eventually shift LM curve to the left paving way for high interest rates, investments to fall and income to shrink. Narrowing down to the values of equilibrium for National income (Table 17) we observe that value of Y∗ decreases and that our results follow characteristics associated with the model.
Remark 3.7. It is interesting to note here that non linearity destabilises the system in case of increased value of money supply and induces stability when money supply decreases. This behaviour is totally attributed to large number of parameters working in the argument for liquidity and presence of two time delays.
● β1 or μ - Propensities (β1=μ=−0.5)
We recall the assumption taken for investment function in the equation (3) of capital accumulation, I(Y,K,r)=I1(Y,r)+I2(K)=I1(Y(t−τ1),r(t))+I2(K(t−τ2))=I1(Y(t−τ1),r(t))+μ(K(t−τ2)). This μ or β1 serves as coefficient which is in flow with the investment corresponding to the capital stock or more appropriately symbolizes level of decrement of investment in capital stock. On Decreasing the value (Table 18) of depreciation we have the following dynamics.
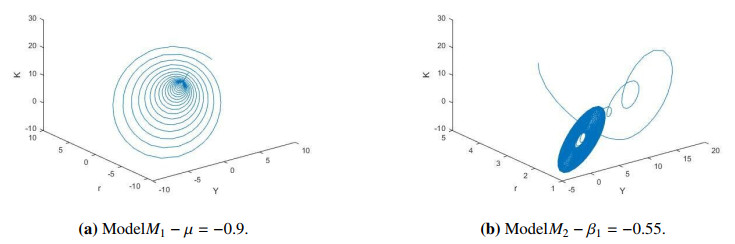
For model M1, as we decrease the value of μ, (See 4), the system is stable (Figure 13a). It implies that the level of decrement of investment in capital stock is reduced. Hence, there is substantial investment in the capital stock, however the capital stock so formed continues to depreciate at a rate δ=0.9.
On Increasing the value we have the following dynamics (Table 19).
For model M1, as we increase the value of μ, (See 4), it increases the level of decrement of investment in capital stock and there is negligible investment in capital stock. If there is negligible investment in capital stock and all that is produced is consumed then future productive capacity of the economy will start falling as the present capital wears out owing to a depreciation rate of δ=0.9. This process causes the system to behave in an unstable way (Figure 14a).
For model M2, the system fluctuates between stability and bifurcations not depicting any trend (Figure 13b, Figure 14b). A decreased or increased value of β1 interferes with the investment function. Since we are following the basic assumption of all that is saved is invested and the savings function is non-linear in nature, our model behaves in such a manner. Nevertheless to mention the effect overall non linearity of the model induces. While saving linearly would mean having appropriate portions at particular time period which are invested then, a non linear pattern for savings does not guarantee the same. The portion saved will vary in magnitude and may not occur in a favorable time period.
4.
Discussion
Ostensibly, non linear arguments alter system dynamics drastically. We have braced a customized IS-LM model which incorporates two time delays in the equation for capital accumulation. We distribute the findings of this paper to be beneficial to the following arenas broadly. Firstly, it can aid the government in stabilization of economy. In the wake of sudden crisis like events in any domain like health, social, technological, educational etc investor sentiment becomes fragile and they wish to flee away from risk. Such events lead to scarcity of money in financial markets. How? With any disaster natural or instigated hitting the economy results in reduced consumer business. To support a declining economy, people assume that government will reduce the rate of interest in order to revive investors participation. Rate of interest if will decrease now, then speculations rise that it will increase in near future. Following an inverse relation between price of bonds and rate of interest, an increase in rate of interest would imply that prices of bond will decrease. Consequently, people will start selling their bonds and prefer liquidity or cash because bond value will decrease in near future. Hence, the money in markets starts dwindling asking for increased money supply. With the intent of stabilizing the economy, government introduces money into the market which disturbs the equilibrium of IS-LM curve. In order to achieve equilibrium again LM curve shifts to the right accompanied by lower interests rate, increased investment and increased level of national income. However, as we see that model M2 is sensitive towards increase in value of Money supply (Table 14). Taking a note of parameters and the constraints involved can help in treating bottlenecks that may arise in achieving stability even when money has been released in the respective markets.
Secondly, talking of economic policies, once an economy has been identified as falling in line with linear framework, its capital stock and invest decisions can follow discourse inspired from model M1. In this case, capital stock can imbibe only specific amount of delay in order to ensure considerable output. Also, implementation of investment decision requires precise observation failing to which the system may show stability for a while and enter instability again (Table 2). Obviously, time delays stand as a pertinent control parameter in economic framework and can many a times cause an otherwise stable system to fluctuate (Ballestra LV et al., (2013)). Through Section 3 (Parameter Sensitivity and analysis) it is clear that model M1 shows vulnerability when (a)value of depreciation of capital stock is decreased (Table 13) (b) propensities to investment are increased (Table 19).Hence, while curating economic policies for a linear economy this information can be of immense help. If an economy is identified as non linear, policy makers should frame policies that can be brought to effect much sooner as the periodic windows in this case are smaller and demand prompt action. Both capital stock and investment decisions are greatly dependent on the time on which they are taken. Model M2 is sensitive towards (a) decrease in value of depreciation of capital stock (Table 13) (b) increase in value of Money supply (Table 14) (c) increment or decrements in value of propensities to investment (Table 18, Table 19). Imbibing these sentiments policy makers can take note of the parameters and value constraints so as to avoid instability.
Thirdly, the shaping up of dynamical behaviour of both the models clearly indicates that model M2 is in better capacity to serve the economical environment set in this case as introduction of non linearity to the model stabilizes the system (τ1=0.44(M2),τ1=0.72(M1)). Model M1 either converges to stability or explodes to instability and is in no capacity to demonstrate recurring cyclical behaviour primarily as seen in (Table 12, Table 14, Table 16, Table 18 and Table 19). Model M2 displays ambiguous prints of recurring cyclical behaviour (Table 14, Table 18, Table 19) in majority. According to the works of Turnovsky (2019) such a behaviour is best suited for capturing economic timeline and validates the exposition of our non linear model. The intent here is not to accord the supremacy of nonlinear models over linear models but to observe from a distance and through diving in, how the two models rise to the occasion under similar framework.
We have made first step towards such a comparison and parameter sensitivity analysis although this arena demands greater exploration. Future works may include involving more parameters in parameter sensitivity analysis or choosing different base models and then subjecting them to various linear and non linear counterparts.
Acknowledgments
The authors would like to appreciate the effort from editors and reviewers. The following research did not claim any particular or general grant from any of the funding agencies i.e. public, commercial or not-for-profit sectors.
Conflict of interest
All authors declare no conflicts of interest in this paper.









 DownLoad:
DownLoad: