1.
Introduction
The soliton solutions of nonlinear evolution equations (NLEE) play an important role to understand the various advantages of physical phenomena in fluids, plasmas, etc [1,2]. Rational solutions are a particular type of soliton solutions, which include lump and rogue waves.
A rogue wave which is a type of rational solution is isolated. It is reported in [3,4] that the mean height of rogue waves is at least double times the height of the neighboring waves. Rogue waves come from nowhere and disappear with no trace [5,6] and superfluids [7]. The applications of rogue waves with their rational solutions for the Boussinesq equation can be found in [8].
Rogue waves can be seen in the thick ocean [7,9], water tanks [10,11], and optical fibers [10,12].
The purpose for the rogue wave is to understand the physics of the huge waves appearance and its relations to the environmental conditions (wind and atmospheric pressure).
In the literature, many methods were proposed to derive the rogue wave solutions such as an inverse scattering method [13], Hirota bilinear method [14], Darboux transformation method [15], B¨acklund transformation method [16], variational direct method, simplified extended tanh-function method, Exp-function method, extended rational Sin-Cos and sinh-cosh methods. In [17] the author derives the periodic wave solution for the fractional complex nonlinear Fokas-Lenells equation via an ancient Chinese algorithm so called the Ying Bu Zu Shu. Also in [18] the authors deduced the abundant solitons and periodic solutions of the (1+2)-dimensional chiral nonlinear Schr¨odinger equation using the extended He's variational method. In [19] the same authors gave the explicit solutions for the Benney-Luke equation in dark solitary, dark-like solitary, kinky dark solitary and periodic wave solutions by the variational direct method (VDM).
The KP equation is a nonlinear partial differential equation in one temporal and two or three spatial coordinate, describes the evolution of nonlinear long waves for small amplitudes.
Furthermore, the (KP) equation was proposed to deal with slowly varying perturbation wave in dispersion media. This equation has been studied in a variety of scientific fields, such as solid state, physics, plasma physics, fiber optics, propagation of waves, oceanography [20].
The importance of the nonlinear terms is to obtain the rogue waves which are nonlinear waves phenomena, so they can be represented with a variety of nonlinear wave equations. Bilinear equation was obtained for soliton equation only and can be used to handle the nonlinear wave equations.
The novelty of this work is handling the higher-order rogue wave solution by the (KP)equation in form of two (3+1)-dimensional extensions
The multi-rogue wave solutions are found for the (3+1)-dimensional Jimbo-Miwa equation [21] and (3+1)-dimensional Hirota bilinear equation based on the bilinear form for this equation using a symbolic computation approach [22]. It is noted that there are similarity between the results in the references [21,22] and the obtained results in this paper which prove the correctness of the proposed approach.
N-soliton solutions are obtained based on the simplified Hirota approach and kinky-lump breather, combo line kink and kinky-lump breather are derived for (3+1)-dimensional Sharma-Tasso-Olver-like (STOL) model [23]. By the bilinear form for the extended BKPA-Boussinesq equation the abundant breather waves, multi-shocks waves and localized excitation solutions are obtained [24].
The symbolic computation approach method was chosen because it is a simple method to obtain the higher order rogue wave solution without need to obtain a Darboux transformation [25].
Multi rogue waves of the Boussinesq type equation [26,27,28].
A rogue wave can be formed when wave energy is focused, usually during a storm. When the storm produces waves that go against the prevailing ocean current, the wave frequency shortens.
In [29] the authors constructed the multiple lump solutions of the (3+1)-dimensional potential Yu-Toda-Sasa-Fukuyama equation in fluid dynamics, in [30] the authors studied (2+1)-dimensional asymmetrical Nizhnik-Novikov-Veselov equation. By adding some new constraints to the N-soliton solutions and the resonance Y-type soliton and in [31] the authors investigated the multiple lump solutions for the generalized (3+1)-dimensional KP equation. With the aid of the variable transformation
In this work, section 2 introduces the description of the symbolic computation approach [32]. Section 3 introduces the multi-rogue waves and bilinear system of the first extended (3+1)-dimensional KP equation. The first, second, and third-order rogue waves for this equation are derived in subsections 3.1–3.3. Section 4 introduces the multi-rogue waves and bilinear form of the second extended (3+1)-dimensional KP equation. Subsections 4.1–4.3 introduces the derivations of the first, second, and third-order rogue waves for this equation. Section 5 introduces the results and conclusions of the outcome results.
2.
Materials and methods
The nonlinear partial differential equations (NLEEs) are:
Here, H is a function in u(x,y,z,t) and its derivatives.
The main steps of the proposed approach are:
Step 1. Following the Painlève analysis
Step 2. By using (2.2) in NLEEs (2.1), the Hirota's bilinear form is:
where ζ=x+y−et. The D-operator is defined by [33]
Step 3. Let
where
F0=1,F−1=P0=Q0=0, α,β,am,l,bm,l and cm.l,(m,l=0,2,4,....,n(n+1)) are real numbers. α,β are used to control the rogue-wave center.
Step 4. By substituting (2.5) into (2.3) and taking the coefficients of z and ζ equal to zero, a system of polynomials is obtained.
Step 5. For getting the multi rogue wave solutions in terms of z and ζ, the values of am,l,bm,l and cm,l are substituted into (2.5).
3.
The bilinear form and multi rogue waves for the first extended (3+1)-dimensional KP equation
The first extended (3+1)-dimensional KP equation [34]:
where δ,μ and χ are plasma parameters. And u is a wave amplitude functions in x,y,z and t. The rogue-waves solutions for (3.1) can be obtained by finding the Hirota bilinear form.
In (3.1) setting ζ=x+dy−et,
Using the following variable transformation
Where u0 is a constant, the Hirota bilinear form for (3.1) is obtained by substituting (3.3) into (3.2):
If χ=1,μ=−(1/3),e=d2+δu0, then (3.1) converts to the Boussinesq equation [8].
The multi rogue wave solutions of the first extended (3+1)-dimensional (KP) equation (3.1) can be obtained as follows.
3.1. First-order rogue waves n=0
Here, F is selected as
Let a2,0=1 with no loss of generalization.
The coefficients a0,0 and a0,2 are obtained by substituting (3.5) into (3.4) and taken zero value for the coefficients of all powers of z and ζ
The first-order rogue waves of Eq (3.1) is obtained by inserting (3.6) in (3.5) we get
where
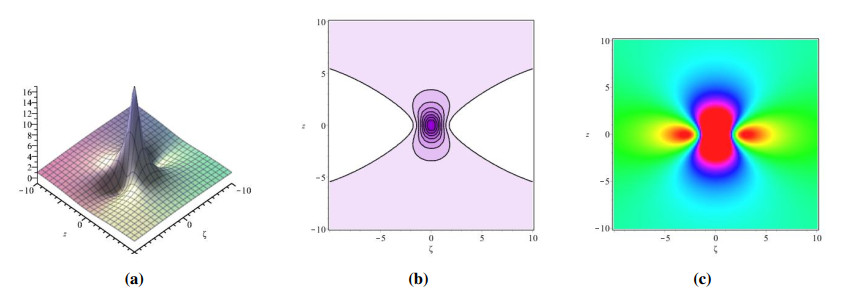
Figure 1 shows first-order rogue wave solutions (3.7) at α=β=0. These solutions have three centers (0,0) and (±3√−μd2χ+δu0+χ−e,0). In three-dimensional, contour plot and the corresponding density plot is presented. It is remarked that there is one peak, and the first-order rogue wave has the minimum amplitude −8d2χ+7δu0+8χ−8eδ at (0,0) and maximal amplitude d2χ+2δu0+χ−eδ at (3√−μd2χ+δU0+χ−e,0) when μ>0,d2χ+δu0+χ<e. The first-order rogue wave solutions (3.7) at α=−5,β=−5 the center of rogue wave will be (−5,−5) and (−5d2χ−5δu0+3√−χd2μ−δμu0−χμ+eμ−5χ+5ed2χ+δu0+χ−e,−5) as shown in Figure 2, moreover, the minimal and maximal amplitudes also change into −8d2χ+7δu0+8χ−8eδ and d2χ+2δu0+χ−eδ respectively.
3.2. Second-order rogue waves n=1
The second-order rogue wave solutions of Eq (3.1) can be found by setting n=1 in Eq (2.5) as:
Substituting (3.9) in (3.4) and taking the coefficients of all powers of ζ and z equal to zero, the set of parameters am,l,bm,l,cm.l,(m,l=0,2,4,6) can be obtained as:
where b2,0 and c2,0 {are} arbitrary parameters.
The second-order rogue wave of Eq (3.1) can be found as:
Figures 3 and 4 show the two high peaks of the second-order rogue waves for (3.11) at α=β=0. The second-order peak breaks apart and for sufficiently big parameters at α=β=1000. The set of three first order rogue waves are forming a triangle called rogue wave triplet.
3.3. Third-order rogue waves n=2
The third-order rogue wave of Eq (3.1) is given by establishing n=2 in Eq (2.5) as follows:
Substituting (3.12) in (3.4) and taken all coefficients of all powers of ζ and z equal to zero, the set of parameters am,l,bm,l,cm.l,(m,l=0,2,4,6) are found as:
where b4,0 and c4,0 are arbitrary constants.
Then the third-order rogue wave solution for Eq (3.1) is defined as:
Figures 5 and 6 show three high peaks of the third-order rogue waves for (3.14) at α=β=0. The third-order peak breaks apart and for sufficiently big parameters at α=β=108, the third-order rogue waves consist of five first-order rogue waves. These waves are located in the corners of a pentagon and the other sit in the center.
4.
The multi rogue waves for the second extended (3+1)-dimensional (KP) equation
The second extended (3+1)-dimensional (KP) equation [34] is:
where δ,μ,χ and ρ are constants and u is a wave amplitude functions in x,y,z and t.
The rogue-waves solutions for (4.1) can be obtained by finding the Hirota bilinear form by setting ζ=x+dy−et. Then, the ODE of (4.1) can be obtained as:
Using the following variable transformation
Then we can obtain the Hirota bilinear form for (4.1) by inserting (4.3) into (4.2) as
The multi rogue wave solutions of the second extended (3+1)-dimensional KP equation (4.1) are given as Figures 7 and 8.
4.1. Case 1. n=0
The coefficients a0,0 and a0,2 in Eq (3.5) can be give as follows
Inserting (4.5) into (3.5), the first-order rogue waves for Eq (4.1) can be obtained in the form
where
The first-order rogue wave solutions (4.6) when α=β=0 are shown in Figure 7. This figure has three centers (0,0) and (±3√−μδu0+2χ−e−ρ,0) in three-dimensional, contour plot and the corresponding density plot. It is remarked that, there is one peak only because energy of the rogue wave is focused on the high peaks. The first-order rogue wave has the minimal amplitude −7δu0−16χ+8e+8ρδ at (0,0) and maximal amplitude 2δu0+2χ−e−ρδ at (±3√−μδu0+2χ−e−ρ,0) where μ>0,χ<12(−δu0+e+ρ). The first-order rogue wave solutions (4.6) at α=−5,β=−5 with the centers of rogue wave will be at (−5,−5) and (5δu0−5e+10χ−5ρ−3√μ(−δu0−2χ+e+ρ)−δu0−2χ+e+ρ,−5) as shown in Figure 8. The minimal and maximal amplitudes are changing into −7δu0−16χ+8e+8ρδ and 2δu0+2χ−e−ρδ respectively.
4.2. Case 2. n=1
For this case the second-order rogue wave solutions of Eq (4.1) is:
where G2(ζ,z;α,β)) is given by (3.9) with n=1 and
where b2,0 and c2,0 is an arbitrary parameters.
In Figures 9 and 10, the two high peaks of the second-order rogue waves of (4.6) at α=β=0 are shown. At sufficiently big parameters, the set of three first order rogue waves forms and the centers are formed a triangle entitled a rogue wave triplet.
4.3. Case 3. n=2
The third-order rogue wave solutions for this case of Eq (4.1) can be obtained as follows
where G3(ζ,z;α,β)) is given by (3.12) with n=2 and
where b4,0 and c4,0 are arbitrary constants.
In Figures 11 and 12, the three high peaks of the third-order rogue waves for (4.6) at α=β=0 are introduced. The third-order peak breaks apart and for sufficiently big parameters for α=β=108, the third-order rogue waves consists of five first-order rogue waves are located in the corners of a pentagon and other one sites in the center.
5.
Conclusions
In this paper, we investigated the first, second, and third-order rogue waves for two (3+1)-dimensional extensions of the (KP) equation by the bilinear method via the symbolic computation approach. The properties of the two (3+1)-dimensional extensions of the (KP) equation are examined by introducing several figures. The obtained higher-order rogue waves have the property limx→±∞=limy→±∞=limz→±∞=limt→±∞=u0. The figures were depicted in three dimensional, contour and density with the center controlled by the parameters α and β. The results obtained in this work are useful to understand the dynamic behaviors of higher-rogue waves in the deep ocean and nonlinear optical fibers. Thus, the characteristics of these solutions are discussed through some diverting graphics under different parameter choices. The dynamics behaviors of higher-rogue waves related to the optical rogue waves are pulses of light similar to rogue or freak ocean waves. Rogue waves in optical fibers can be described mathematically by the nonlinear Schr¨odinger equation and its extensions that take into account third-order dispersion. We can apply this technique to completely integrable nonlinear evolution equations.
Acknowledgments
The authors extend their appreciation to the Deanship of Scientific Research at King Khalid University, Abha, Saudi Arabia, for funding this work through the Research Group Project under Grant Number (RGP. 2/36/43). This research was funded by Princess Nourah bint Abdulrahman University Researchers Supporting Project number (PNURSP2022R229), Princess Nourah bint Abdulrahman University, Riyadh, Saudi Arabia.
Conflict of interest
All authors declare that they have no conflicts of interest.









 DownLoad:
DownLoad:














