Nonlocal coupled system for $\psi$-Hilfer fractional order Langevin equations

1.   Introduction

Fractional calculus deals with the study of fractional order integral and derivative operators over real or complex domains. The subject of fractional differential equations has become a hot topic for the researchers due to its intensive development and applications in the field of physics, mechanics, chemistry, engineering, etc. For a reader interested in the systematic development of the topic, we refer the books ^{[1,2,3,4,5,6,7,8]}.

Since the beginning of the fractional calculus there are numerous definitions of integrals and fractional derivatives, and over time, new derivatives and fractional integrals arise. Hilfer in ^[9] generalized both Riemann-Liouville and Caputo fractional derivatives to Hilfer fractional derivative of order $\alpha\in (0, 1)$ and a type $\beta\in [0, 1]$ which can be reduced to the Riemann-Liouville and Caputo fractional derivatives when $\beta = 0$ and $\beta = 1$, respectively. For more details see ^[10,11] and references cited therein.

In ^[2], a fractional derivative of a function with respect to another function were introduced, by using the fractional derivative in the Riemann-Liouville sense. Almeida ^[12] using the idea of the fractional derivative in the Caputo sense, proposes a new fractional derivative, called $\psi$-Caputo derivative with respect to another function $\psi$, which generalizes a class of fractional derivatives. In ^[13], the authors, by using the Hilfer fractional derivative idea, proposed a fractional differential operator of a function with respect to another $\psi$-function, the so-called $\psi$-Hilfer fractional derivative. The $\psi$-Hilfer fractional derivative has as advantage the freedom of choice of the classical differential operator, see, for example, ^[14,15].

Initial value problems involving Hilfer fractional derivatives were studied by several authors, see for example ^[16,17,18] and references therein. In ^[19], the authors initiated the study of nonlocal boundary value problems for Hilfer fractional derivative. Recently, in ^[20], the results in ^[19] was extended to $\psi$-Hilfer nonlocal implicit fractional boundary value problems. In ^[21], the existence and uniqueness of solutions were studied, for a new class of boundary value problems of sequential $\psi$-Hilfer-type fractional differential equations with multi-point boundary conditions.

The Langevin equation (first formulated by Langevin in $1908$ to give an elaborate description of Brownian motion) is found to be an effective tool to describe the evolution of physical phenomena in fluctuating environments ^[22]. For some new developments on the fractional Langevin equation, see, for example, ^[23], ^[24], ^[25].

In ^[26], the authors considered a boundary value problem of Langevin fractional differential equations with $\psi$-Hilfer fractional derivative and nonlocal integral boundary conditions, given by

where $\mathcal{D}^{\chi_{i}, \beta_{i}; \psi}, \ i = 1, 2$ is the $\psi$-Hilfer fractional derivative of order $\chi_{i}$, $0 < \chi_{i} < 1$ and type $\beta_{i}$, $0\leq \beta_{i}\leq 1$, $i = 1, 2$, $1 < \chi_1+\chi_2\le 2,$ $k\in \mathbb{R}$, $a\geq 0$, $f: J\times \mathbb{R}\rightarrow \mathbb{R}$ is a continuous function, $I^{\delta_{i}; \psi}$ is $\psi$-Riemann-Liouville fractional integral of order $\delta_{i} > 0$, $\lambda_{i}\in \mathbb{R}$, $i = 1, 2, \ldots, m$ and $0\leq a\leq \tau_{1} < \tau_{2} < \ldots < \tau_{m}\leq b$. Existence and uniqueness results are established by using Krasnosel'ski$\mathop {\rm{i}}\limits^ \vee$'s fixed point theorem, Leray-Schauder nonlinear alternative and Banach contraction mapping principle.

In the present work, we study a coupled system consisting by $\psi$-Hilfer fractional order Langevin equations supplemented with nonlocal integral boundary conditions of the form

where ${}^{H}\mathfrak{D}_{a^+}^{u, v;\psi }$ is $\psi$-Hilfer fractional derivatives of order $u \in \{\alpha_{1}, \alpha_{2}, p_{1}, p_{2}\}$ with $0 < u \leq 1$ and $v \in \{\beta_{1}, \beta_{2}, q_{1}, q_{2}\}$ with $0 \leq v \leq 1$, $\mathcal{I}_{a^+}^{w; \psi}$ is $\psi$-Riemann-Liouville fractional integral of order $w = \{\delta_{i}, \kappa_{j}\},$ $w > 0$, the points $\theta_i$, $\xi_j \in [a, b]$, $i = 1, 2, \ldots, m$, $j = 1, 2, \ldots, n$, $\lambda_{1}$, $\lambda_{2} \in\mathbb{R}$, $f$, $g \in \mathcal{C}([a, b]\times \mathbb{R}^{2}, \mathbb{R})$ and $b > a \geq 0$.

The rest of the paper is organized as follows. In Section 2 we outline the basic concepts from fractional calculus and prove an auxiliary lemma for the linear variant of the problem (1.2). Then, by applying Banach's fixed point theorem, we derive the existence and uniqueness result for the problem (1.2), while the existence result is established via Leray-Schauder alternative. Example illustrating the main results are also presented.

2.   Background material and auxiliary results

This section, is assigned to recall some notation in relation to fractional calculus.

Let $\mathcal{S} = \mathcal{C}([a, b], \mathbb{R})$ be the space equipped with the norm defined by $\| x\| = \sup\{|x(t)|: t\in J\}$. Obviously $(\mathcal{S}, \|\cdot\|)$ is a Banach space and, consequently, the product space $(\mathcal{S}\times\mathcal{S}, \|\cdot\|)$ is a Banach space with the norm $\|(x, y)\| = \|x\| + \|y\|$ for $(x, y) \in \mathcal{S}\times\mathcal{S}$ and the $n$-times absolutely continuous functions given by

Definition 2.1. ^[2] Let $(a, b)$, $(-\infty \leq a < b \leq \infty)$, be a finite or infinite interval of the half-axis $\mathbb{R}^+$ and $\alpha \in \mathbb{R}^+$. Also let $\psi(x)$ be an increasing and positive monotone function on $(a, b]$, having a continuous derivative $\psi^{\prime}(x)$ on $(a, b)$. The $\psi$-Riemann-Liouville fractional integral of a function $f$ with respect to another function $\psi$ on $[a, b]$ is defined by

where $\Gamma(\cdot)$ is represent the Gamma function.

Definition 2.2. ^[2] Let $\psi^{\prime}(t)\neq 0$ and $\alpha > 0$, $n \in \mathbb{N}$. The Riemann–Liouville derivatives of a function $f$ with respect to another function $\psi$ of order $\alpha$ correspondent to the Riemann–Liouville, is defined by

where $n = [\alpha]+1$, $[\alpha]$ is represent the integer part of the real number $\alpha$.

Definition 2.3. ^[13] Let $n-1 < \alpha < n$ with $n \in \mathbb{N}$, $[a, b]$ is the interval such that $-\infty \leq a < b \leq \infty$ and $f, \psi \in \mathcal{C}^n ([a, b], \mathbb{R})$ two functions such that $\psi$ is increasing and $\psi^{\prime}(t)\neq 0$, for all $t \in [a, b]$. The $\psi$-Hilfer fractional derivative of a function $f$ of order $\alpha$ and type $0\leq\rho\leq1$, is defined by

where $n = [\alpha]+1$, $[\alpha]$ represents the integer part of the real number $\alpha$ with $\gamma = \alpha + \rho(n - \alpha)$.

Lemma 2.4. ^[2] Let $\alpha, \beta > 0$. Then we have the following semigroup property given by,

Next, we present the $\psi$-fractional integral and derivatives of a power function.

Proposition 2.5. ^[2,13] Let $\alpha \geq 0$, $\upsilon > 0$ and $t > a$. Then, $\psi$-fractional integral and derivative of a power function are given by

(i) $\mathcal{I}_{a^+}^{\alpha; \psi } \left(\psi (s)-\psi(a)\right)^{\upsilon -1}(t) = \frac{\Gamma (\upsilon)}{\Gamma (\upsilon +\alpha)} \left(\psi (t)-\psi (a) \right)^{\upsilon +\alpha -1}.$

(ii) ${^{H}\mathfrak{D}}_{a^+}^{\alpha, \rho; \psi } \left(\psi (s)-\psi (a) \right)^{\upsilon -1}(t) = \frac{\Gamma (\upsilon)}{\Gamma (\upsilon -\alpha)} {{\left(\psi (t)-\psi (a) \right)}^{\upsilon -\alpha -1}}, \quad n-1 < \alpha < n, \quad \upsilon > n.$

Lemma 2.6. ^[27] Let $m-1 < \alpha < m$, $n-1 < \beta < n$, $n, m\in\mathbb{N}$, $n \leq m$, $0\leq \rho\leq 1$ and $\alpha \geq \beta +\rho (n - \beta)$. If $f\in {\mathcal{C}^n}(J, \mathbb{R})$, then

Lemma 2.7. ^[13] If $f \in \mathcal{C}^n (J, \mathbb{R})$, $n-1 < \alpha < n$, $0 \leq \rho \leq 1$ and $\gamma = \alpha +\rho(n-\alpha)$ then

for all $t\in J$, where $f_{\psi }^{[n]}f(t): = {{\left(\frac{1}{\psi^{\prime}(t)}\frac{d}{dt} \right)}^{n}}f(t)$.

The following lemma deals with a linear variant of the system (1.2).

Lemma 2.8. Let $0 < \alpha_{1}, \alpha_{2}, p_{1}, p_{2} \leq 1,$ $0 \leq \beta_{1}, \beta_{2}, q_{1}, q_{2} \leq 1$, $\gamma_{1} = \alpha_{1} + \beta_{1}(1 - \alpha_{1})$, $\gamma_{2} = p_{1} + q_{1}(1 - p_{1})$, $\varphi_{1} = \alpha_{2} + \beta_{2}(1-\alpha_{2})$, $\varphi_{2} = p_{2} + q_{2}(1 - p_{2})$, $h_{1}$, $h_{2} \in \mathcal{S}$ and $\Omega\ne 0.$ Then the solution for the linear system of $\psi$-Hilfer fractional Langevin differential equations of the form:

is equivalent to the integral equations

and

where

Proof. Let $x \in \mathcal{S}$ be a solution of the problem (2.7). Taking the operator $\mathcal{I}_{a^+}^{\alpha_{1};\psi }$ on both sides of (2.7) and using Lemma 2.7, we have

where $c_{1}\in\mathbb{R}$. Applying the operator $\mathcal{I}_{a^+}^{p_{1};\psi }$ on both sides of (2.15) and using Lemma 2.7 again, we get

where $c_{1}$, $c_{2}$ are arbitrary constants.

In the same process, let $y \in \mathcal{S}$ be a solution of the problem (2.7). Applying the operators $\mathcal{I}_{a^+}^{\alpha_{2};\psi }$ and $\mathcal{I}_{a^+}^{p_{2};\psi }$, respectively, on both sides of the second equation in (2.7) and using Lemma 2.7, we obtain

where $d_{1}$, $d_{2}$ are arbitrary constants. Using the boundary conditions $x(a) = 0$ and $y(a) = 0$, respectively in (2.16) and (2.17), we find that $c_{2} = 0$ and $d_{2} = 0$. Hence we have

Using (2.18) and (2.19) in the conditions $x(b) = \sum_{i = 1}^{m}\eta_{i}\mathcal{I}_{a^+}^{\delta_{i}; \psi }y(\theta_{i})$ and $y(b) = \sum_{j = 1}^{n}\mu_{j}\mathcal{I}_{a^+}^{\kappa_{j}; \psi }x(\xi_{j})$, we obtain a system of equations in the unknown constants $c_{1}$ and $d_{1}$ given by

where $\Omega_{1}$, $\Omega_{2}$, $\Omega_{3}$, $\Omega_{4}$ are given by (2.10), (2.11), (2.12), (2.13), respectively, and

Solving the system (2.20)-(2.21) for $c_{1}$ and $d_{1}$, it follows that

Inserting values $c_1$ and $d_1$ in (2.18) and (2.19) respectively together with notations $\Omega_{1}$, $\Omega_{2}$, $\Omega_{3}$, $\Omega_{4}$, $A_{1}$, and $A_{2}$ lead to solutions (2.8) and (2.9).

Conversely, it is easily to shown, by a direct computation, that the solution $x(t)$ and $y(t)$ are given by (2.8) and (2.9) satisfies the problem (2.7) under the nonlocal integral boundary conditions. The proof of Lemma 2.8 is completed.

Fixed point theorems play a major role in establishing the existence theory for the system (1.2). We collect here the well-known fixed point theorems used in this paper.

Lemma 2.9. (Banach fixed point theorem) ^[28] Let $X$ be a Banach space, $D\subset X$ closed and $F: D\to D$ a strict contraction, i.e. $|Fx-Fy|\le k|x-y|$ for some $k\in (0, 1)$ and all $x, y\in D.$ Then $F$ has a unique fixed point in $D.$

Lemma 2.10. (Leray-Schauder alternative ^[29]). Let $\mathcal{K} : D \to D$ be a complete continuous operator (i.e., a map that restricted to any bounded set in $D$ is compact). Let

Then either the set $M(\mathcal{K})$ is unbounded, or $\mathcal{K}$ has at least one fixed point.

3.   Existence and uniqueness results

In this section, we discuss existence and uniqueness results to the purposed system (1.2).

Throughout this paper, the expression $\mathcal{I}_{0^+}^{q, \rho} f(s, x(s), y(s))(c)$ means that

where $u \in \{p_{1}, p_{2}, \alpha_{1}+p_{1}, \alpha_{2}+p_{2}, p_{2}+\delta_{i}, \alpha_{2}+p_{2}+\delta_{i}, p_{1}+\kappa_{j}, \alpha_{1}+p_{1}+\kappa_{j}\}$ and $c \in \{t, a, b, \theta_{i},$ $\xi_{j}\}$, $i = 1, 2, \ldots, m$, $j = 1, 2, \ldots, n$.

In view of Lemma $2.8$, we define an operator $\mathcal{Q} : \mathcal{S}\times \mathcal{S} \to \mathcal{S}\times \mathcal{S}$ by

where

and

It should be noticed that the system (1.2) has solutions if and only if $\mathcal{Q}$ has fixed points.

For the sake of convenience, we use the following notations:

3.1.   Uniqueness result via Banach's fixed point theorem

In the first result, we establish the existence and uniqueness of solutions for system (1.2), by applying Banach's fixed point theorem.

Theorem 3.1. Let $\Omega\ne 0,$ $f$, $g : [a, b]\times\mathbb{R}^{2} \to \mathbb{R}$ be continuous functions. In addition, we assume that:

($H_{1}$) There exist constants $L_{1}$, $L_{2} > 0$ such that, $\forall t \in [a, b]$ and $x_{1}$, $x_{2}$, $y_{1}$ $y_{2} \in \mathbb{R}$,

Then system (1.2) has a unique solution on $[a, b]$, provided that $\Phi_{1} < 1$, where

Proof. Firstly, we transform the system (1.2) into a fixed point problem, $(x, y)(t) = \mathcal{Q}(x, y)(t)$, where the operator $\mathcal{Q}$ is defined as in (3.1). Applying the Banach contraction mapping principle, we shall show that the operator $\mathcal{Q}$ has a unique fixed point, which is the unique solution of system (1.2).

Let $\sup_{t\in [a, b]}|f(t, 0, 0)| : = M_1 < \infty$ and $\sup_{t\in [a, b]}|g(t, 0, 0)| : = N_1 < \infty.$ Next, we set $B_{r_1} : = \{(x, y) \in \mathcal{S}\times\mathcal{S} : {\| (x, y) \|} \leq r_1\}$ with

Observe that $B_{r_1}$ is a bounded, closed, and convex subset of $\mathcal{S}$. The proof is divided into two steps:

Step Ⅰ. We show that $\mathcal{Q}B_{r_1} \subset B_{r_1}$.

For any $(x, y) \in B_{r_1}$, $t\in [a, b]$, using the condition $(H_1)$, we have

and

Then, we get

Similarly, we find that

Consequently, we have

which implies that $\mathcal{Q}B_{r_{1}} \subset B_{r_{1}}$.

Step Ⅱ. We show that $\mathcal{Q} : \mathcal{S} \to \mathcal{S}$ is a contraction.

Using condition $(H_{1})$, for any $(x_{1}, y_{1})$, $(x_{2}, y_{2}) \in \mathcal{S}\times\mathcal{S}$ and for each $t \in [a, b]$, we have

Similarly, we get that

Consequently, we get

which, by condition (3.9), implies that the operator $\mathcal{Q}$ is a contraction. Therefore, by the conclusion of Banach contraction mapping principle (Lemma 2.9), the operator $\mathcal{Q}$ has a unique fixed point. Hence, system (1.2) has a unique solution on $[a.b]$. The proof is completed.

3.2.   Existence result via Leray-Schauder alternative

In the second result, we apply the Leray-Schauder alternative to investigate the existence of solutions for the system (1.2).

For convenience, we set:

where $\Lambda_{1}(\cdot)$, $\Lambda_{2}(\cdot)$, $\Lambda_{3}(\cdot)$ and $\Lambda_{4}(\cdot)$ are given by (3.5), (3.6), (3.7) and (3.8) respectively.

Theorem 3.2. Let $f$, $g : [a, b]\times\mathbb{R}^{2} \to \mathbb{R}$ be continuous functions such that the following condition holds:

$(H_2)$ There exist real constants $K_{i}$, $\overline{K}_{i} \geq 0$ for $i = 1, 2, 3$, such that, for $x$, $y \in \mathbb{R}$,

Then, the system (1.2) has at least one solution on $[a, b]$ provided that

where $E_{1}(\cdot)$ and $E_{2}(\cdot)$ are given by (3.11) and (3.12) respectively.

Proof. The process of the proof is divided into several steps:

Firstly, we show that the operator $\mathcal{Q} : \mathcal{S} \times \mathcal{S} \to \mathcal{S} \times \mathcal{S}$ is completely continuous. Note that the operator $\mathcal{Q},$ defined by (3.1), is continuous in view of the continuity of $f$ and $g$.

Let $B_{r_{2}} \subset \mathcal{S} \times \mathcal{S}$ be a bounded set, where $B_{r_{2}} = \{(x, y) \in \mathcal{S}\times\mathcal{S} : \|(x, y)\| \leq r_{2}\}$. Then, for any $(x, y) \in B_{r_{2}}$, there exist positive real numbers $\overline{f}$ and $\overline{g}$ such that $|f(t, x(t), y(t))| \leq \overline{f}$ and $|g(t, x(t), y(t))| \leq \overline{g}.$

Thus, for each $(x, y)\in B_{r_{2}}$ we have

In similar process, we have that

Therefore, it follows that

which implies that the operator $\mathcal{Q}$ is uniformly bounded.

In the next step, we show that the operator $\mathcal{Q}$ is equicontinuous. Let $\tau_{1}$, $\tau_{2} \in [a, b]$ with $\tau_{1} < \tau_{2}$. Then, we can compute that

In the same process, we deduce that

Consequently, the operator $\mathcal{Q}(x, y)$ is equicontinuous. By Arzelá-Ascoli theorem, the operator $\mathcal{Q}(x, y)$ is completely continuous.

At last process, we show that the set $\mathcal{U} = \{(x, y) \in \mathcal{S}\times\mathcal{S} | (x, y) = \sigma\mathcal{Q}(x, y), \, 0\leq \sigma \leq 1\}$ is a bounded. Let $(x, y) \in \mathcal{U}$, with $(x, y) = \sigma \mathcal{Q}(x, y)$. For any $t\in [a, b]$, we obtain

By using condition $(H_3)$, we can calculate that

and

Hence, we have

and

From the above inequalities, we get

which implies that

where $E_{0} = \min \{1 - \Phi_{2}, \, 1 - \Phi_{3}\}$. Hence the set $\mathcal{U}$ is bounded. Thus, by Lemma $2.10$, the operator $\mathcal{Q}$ has at least one fixed point, which implies that system (1.2) has at least one solution on $[a, b]$. This completes the proof.

4.   Examples

This section is devoted to the illustration of the results derived in previous sections.

Example 4.1. Consider the following nonlocal boundary problem of the form:

Here $\alpha_k = \sqrt{e}/(k+1)$, $\beta_k = (k+4)/10$, $p_k = \sqrt[3]{e}/(k+1)$, $q_k = (3k+1)/10$, $\lambda_k = (15-5k)/100$, $k = 1, 2$, $\psi(t) = e^t/6$, $\eta_i = \ln 2/(i+1)$, $\delta_i = \ln ((6-i)/2)$, $\theta_i = (2i-1)/10$, $i = 1, 2, 3$, $\mu_j = \ln(j+1)/j$, $\kappa_j = \ln((j+3)/3)$, $\xi_j = (2j+3)/10$, $j = 1, 2$, $a = 0$ and $b = 1.2$. From (2.14) with the given data, we can compute that of

Then, $\Omega_{1} \approx 0.6261$, $\Omega_{2} \approx 0.0580$, $\Omega_{3} \approx 0.1687$, $\Omega_{4} \approx 0.8476$, and $\Omega = \Omega_{1}\Omega_{4} - \Omega_{2}\Omega_{3} \approx 0.5209 \neq 0$. In addition, Table 1 shows the numerical results of $\Omega_{i}$ for $i = 1, 2, 3, 4$, and $\Omega$ for a variety of $t \in (0, 1.2)$. These results are shown in Figure 1.

(i) To demonstrate the application of Theorem 3.1, let us take

For $x_i$, $y_i\in \mathbb{R}$, $i = 1, 2$ and $t\in [0, 1.2]$, we get the inequalities

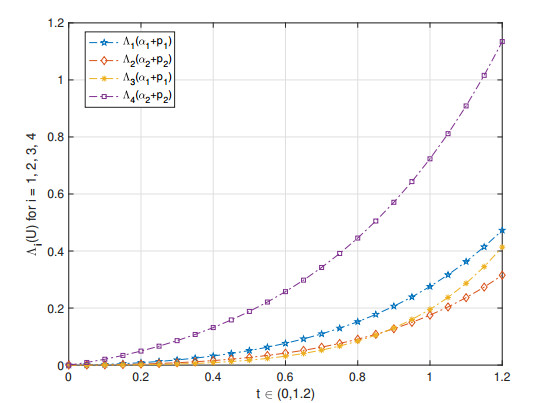
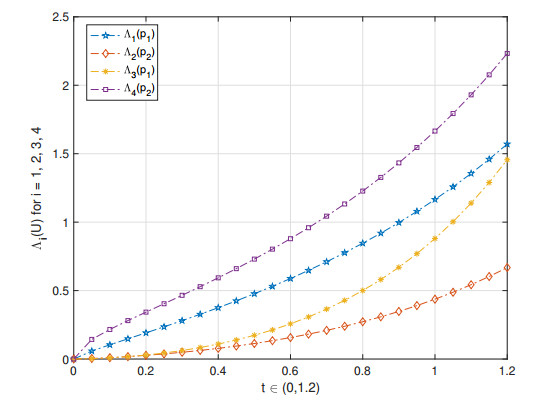
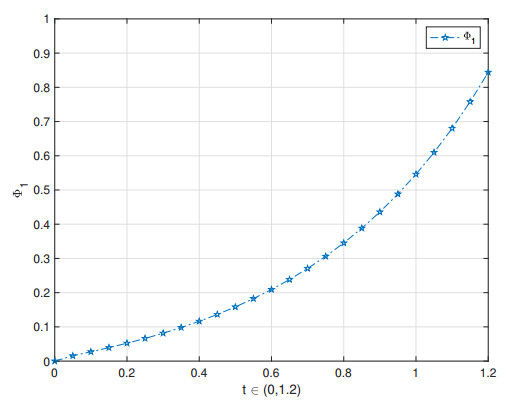
The assumption $(H_1)$ is fulfilled with $\Lambda_{1}(\alpha_{1}+p_{1}) \approx 0.3530$, $\Lambda_{2}(\alpha_{2}+p_{2}) \approx 0.0336$, $\Lambda_{3}(\alpha_{1}+p_{1}) \approx 0.0626$, $\Lambda_{4}(\alpha_{2}+p_{2}) \approx 0.7669$, $\Lambda_{1}(p_{1}) \approx 1.1549$, $\Lambda_{2}(p_{2}) \approx 0.0866$, $\Lambda_{3}(p_{1}) \approx 1.2941$, $\Lambda_{4}(p_{2}) \approx 1.4749$, $L_{1} = 3/25$ and $L_{2} = 1/5$. Also $\Phi_{1} \approx 0.4329 < 1$, and thus by Theorem 3.1, the system (4.1), with $f$ and $g$ given by (4.2), has a unique solution on $[0, 1.2]$. In addition, Tables 2 and 3 show the numerical results of $\Lambda_{i}(U)$ for $i = 1, 2, 3, 4$, where $U = \{\alpha_{1} + p_{1}, \alpha_{2} + p_{2}, p_{1}, p_{2}\}$ and $\Phi_{1}$ for a variety of $t \in (0, 1.2)$. These results are shown in Figures 2-4.

(ii) To illustrate Theorem 3.2 let

For $x$, $y\in \mathbb{R}$ and $t\in [0, 1.2]$, we have

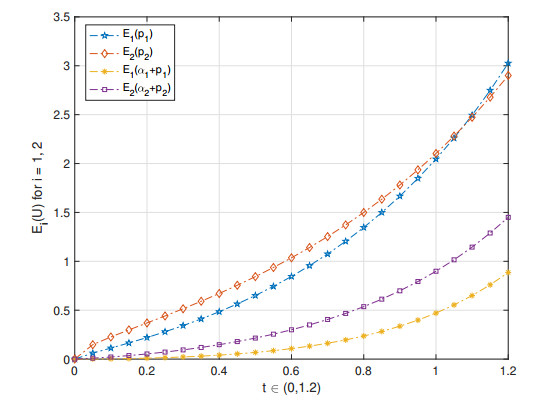
From $(3.11)$-$(3.12)$ with the given datas, we have $E_{1}(\alpha_{1}+p_{1}) \approx 0.4156$, $E_{2}(\alpha_{2}+p_{2}) \approx 0.8005$, $E_{1}(p_{1}) \approx 1.4489$, and $E_{2}(p_{2}) \approx 1.5614$. The assumption $(H_2)$ is satisfied with $K_{1} = 1/5$, $K_{2} = 3/10$, $K_{3} = 3/25$, $\overline{K}_{1} = 3/10$, $\overline{K}_{2} = 6/25$ and $\overline{K}_{3} = 4/25$. Hence, we get $\Phi_2 \approx 0.4617 < 1$ and $\Phi_3 \approx 0.2560 < 1$. Since, all the assumptions of Theorem 3.2 are fulfilled, the system (4.1), with $f$ and $g$ given by (4.3), has at least one solution on $[0, 1.2]$. In addition, Table 4 show the numerical results of $E_{i}(U)$ for $i = 1, 2$, where $U = \{\alpha_{1} + p_{1}, \alpha_{2} + p_{2}, p_{1}, p_{2}\}$ and $\Phi_{i}$ for $i = 2, 3$ for a variety of $t \in (0, 1.2)$. These results are shown in Figures 5-6.

5.   Conclusions

We have discussed the existence and uniqueness of solutions for a coupled system consisting by $\psi$-Hilfer fractional order Langevin equations supplemented with nonlocal integral boundary conditions. We proved the uniqueness of the solutions in the first case using the Banach contraction mapping principle, and we established the existence of the findings in the second case using the Leray-Schauder alternative. The results of the present paper are new and significantly contribute to the existing literature on the topic. Moreover, several new results follow as special cases of the present one.

Acknowledgments

The first author would like to thank the King Mongkut's University of Technology North Bangkok for supporting in this work. The third author would like to thank for funding this work through the Center of Excellence in Mathematics (CEM), CHE, Sri Ayutthaya Rd., Bangkok, 10400, Thailand and Burapha University.

Conflict of interest

On behalf of all authors, the corresponding author states that there is no conflict of interest.