In this paper, we study some vector fields on a contact metric manifold which are invariant under a D-homothetic deformation.
1.
Introduction
Zika infection is a kind of vector-borne disease caused and spread by the bite infected Aedes mosquitos. The Zika infection was first discovered in Uganda in 1947. In 2007, the first case of Zika virus was reported occurred in the Island of Yap (Federated States of Micronesia). After that, it spread very quickly in Asia, Africa and USA [1]. The Aides mosquitoes is the main source from which the Zika virus is spread and is also responsible for dengue infection. The transmission of virus of Zika infection to humans occurred by the bites of infected female mosquitoes from the Aedes genus. This infection can also be transmitted having unprotected sexual relations, if one partner is suffering from Zika virus. People who have infected with Zika will have mild symptom due to which they feel mild illness and get severe ailment. Zika infected people main symptoms are skin rashes, headache, mild fever, conjunctivitis, and muscle pains. Usually the symptoms last for 2–7 days but sometimes the infected individuals due to Zika virus de not developed symptoms. This infection can also affect a pregnant women to her developing fetus [2,3]. If this happened then most probably the newly born babies have abnormal brain and small head development along with muscle weakness which effects nervous system.
Epidemic models are used as powerful tool to predict the dynamics and control of various communicable diseases. These models usually consist of nonlinear differential equations describing the dynamics of the concern disease. A number of transmission models and effective possible controlling strategies have been developed in literature to explore the effective strategies for controlling of Zika infection in different regions around the globe. Kucharsk et al. [4] proposed a mathematical model and provided a detail analysis of French Polynesia Zika outbreak appeared in 2013-14. Kucharsk et al. used the total Zika infected cases between October 2013 till April 2014 which are reported in six main places of French Polynesia for model parameters estimation. Bonyah and Okosun [5] used optimal control theory to derived three different controlling strategies to reduce the spreed of this infection. The impact of bednets, used of insecticides spry and possible treatment was studied in detail in [6]. However, these models are based integer-order classical differential systems. The classical integer-order derivatives have some limitations as they are local in nature and do not posses the memory effects which are appear in most of biological systems. Secondly, classical derivative are unable to provides information about the rate of changes between two points not necessarily same. To overcome such limitations of local derivatives, various concepts on new derivatives with non-integer or fractional order were developed in recent years and can e found in [7,9,10]. The classical Caputo fractional operator [7] has been used to model many complex phenomena in different fields. For example in [11], a numerical scheme was proposed for of the diffusive fractional HBV model in Caputo sense. A numerical scheme for Caputo fractional reaction-diffusion equation and its stability analysis can be found in [12]. Also a detail stability analysis and simulations of Caputo sub-diffusion equation has been developed in [13]. The real world application of non-local and non-singular fractional operator [9] can be found in [14]. A comparative analysis Sturm-Liouville fractional problems has been carried out in [15]. Other applications of singular and non-singular fractional order operators in modeling various phenomena can be found in [18,16,19,20,17]. There is no rich literate on the modeling of Zika virus in fractional order. Only few models with fractional order has been presented in literature for Zika infection [21,22]. Keeping the above discussion in view and applicability of fractional order derivatives, in the preset investigation, a mathematical transmission model is considered in the Caputo sense in order to explore the dynamics of the Zika virus. We simulate the proposed Zika model for different values of relevant parameters and for several values of arbitrary fractional order α.
The structure of the paper is follows is as: groundwork of the fractional derivative is given in Section 2. The basic model formulation is given in Section 3. Sections 4 is devoted to explore the basic properties of the model. Sections 5 and 6 are concern to obtain the stability results of the model equilibria. Graphical analysis are given in Section 7. The whole work is summarized with a brief conclusion in Section 8.
2.
Preliminaries
The basic definitions regarding the fractional derivative in Caputo sense are as follows [7,8]:
Definition 2.1. The Caputo fractional derivative of order α∈(n−1,n) with n∈N for a function h∈Cn is stated as follow:
Clearly CDαt(h(t)) approaches to h′(t) as α→1.
Definition 2.2. The corresponding fractional integral having order α>0 of the function h:R+→R is described by the following expression
where Γ represent the Gamma function.
Definition 2.3. The constant point x∗ is said to be an equilibrium point of the following Caputo fractional dynamic system:
if and only if it observed that h(t,x∗)=0.
To present the stability analysis of nonlinear fractional systems in the Caputo sense via Lyapunov method we first recall the following necessary results from [23,24].
Theorem 2.4. Suppose x∗ be an equilibrium point for the above system (2.1) and Ω∈Rn be a domain containing x∗. Let L:[0,∞)×Ω into to R be a continuously differentiable function such that
and
∀α∈(0,1) and x∈Ω. Whereas W1(x), W2(x) and W3(x) are continuously positive definite functions on Ω. Then x∗ is uniformly asymptotically stable equilibrium point for the model (2.1).
Next we recall the following lemma from [24], which we will use in presenting the global stability via Lyapunov function.
Lemma 2.5. For a continuous and derivable function z(t)∈R+ and α∈(0,1), then for any time t≥t0 we have
3.
Mathematical model and discussion
To formulate the model, we divide the human population into two sub-classes, susceptible individuals and infected individuals. The total human population is represented by xh(t)=x1(t)+x2(t), where x1 represent susceptible and x2 represent numbers of infected human individuals. Similarly, xm is the total number of mosquitos which are further divided into susceptible mosquitos x3, and infected mosquitos x4, so that xm(t)=x3(t)+x4(t). The compartmental mathematical model is given by the following system of four ordinary differential equations to describe the mechanism of the transmission of Zika virus.
with the initial conditions
In the above proposed model Λh and Λm respectively represent the recruitment rate of human and mosquito populations. The natural death rate of the human and mosquitos are d1 and d2 respectively. The contact rate of suspectable human and infected mosquitos is β1, while β2 is the contact rate OF susceptible mosquitos and infected humans. The parameters γ1 and γ2 shows the transmission probabilities of humans and mosquitos.
4.
Existence and positivity of the solution
In order to present the non-negativity of the system solution, let
To proceeds further, first we recall the generalized mean values theorem [25].
Lemma 4.1. Let suppose that h(t)∈C[a,b] and CDαth(t)∈(a,b], then
with a≤ζ≤t,∀t∈(a,b].
Corollary 4.2. Suppose that h(t)∈C[a,b] and CDαth(t)∈(a,b], where α∈(0,1]. Then if
We are now able to give the following result.
Theorem 4.3. A unique solution y(t) of the model (3.1) exists and will remain in R4+. Further more, the solution is positive.
Proof. The exitance of the Caputo fractional Zika model can be shown with the help of theorem 3.1 from [26,27], while the uniqueness of the solution can be easily obtained by making use of the Remark 3.2 in [26] for all positive values of t. In order to explore the solution positivity, it is necessary to show that on each hyperplane bounding the positive orthant, the vector field points to R4+. Form the system (3.1), we deduced that
Hence, using the above corollary (4.2), we obtain the desired target i.e. the solution will remain in R4+ and hence biologically feasible region is constructed as:
Next we explore the equilibria and basic threshold quantity R0 of the model (3.1) in the following subsection.
4.1. Model equilibria and basic reproduction number
The equilibria of our proposed system (3.1) are obtained by solving the system below
Hence we deduced that the proposed model exhibit two type of equilibrium points. The disease free equilibrium (DFE) calculated as
and the endemic equilibrium (EE) is as evaluated as follows E1=(x∗1,x∗2,x∗3,x∗4), where
The EE E1, exist only if R0>1. The threshold quantity known as the basic reproduction number for the fractional Zika model is obtained by using the well known approach discussed in [28]. The basic reproduction number is biologically very important and determine the global dynamics of the model. The corresponding matrices F and V are given by
Further, the inverse of V is
The spectral radius ρ(FV−1) is the basic reproduction number of the model and after some simplification the reproduction number is
5.
Stability analysis of DFE
In this section we proceed to confirm the stability results in both local and global case. The Jacobian of linearization matrix of model (3.1).
Theorem 5.1. For positive integers r1 and r2 such that gcd(r1,r2)=1. Let α=(r1r2) and define N=r2, then the model DFE denoted by E0 is stable locally asymptotically provided that |arg(λ)|>π2N, where λ denotes the possible roots of the characteristic equation of the matrix JE0 given below.
Proof. By expansion of Eq. (5.1), we get the below equation in term of λ.
where the coefficients are given below:
The arguments of the roots of the equation λp1+d1=0 are as follow:
In similar pattern, it can be shown that argument of the roots of λp1+d2=0 are also greater than π2M. Further, if R0<1, then the desired condition (|arg(λ)|>π2N) is satisfied for all roots of polynomial (5.2). For R0>1, then with the help of Descartes rule of signs, there exits exactly one root of characteristic equation with |arg(λ)|<π2N. Thus the DFE is locally asymptotically stable for R0<1, otherwise unstable.
For global stability result we prove the following theorem. This subsection provide the global analysis of the model for the DF and endemic case. We have the following results.
Theorem 5.2. For arbitrary fractional order α in the interval (0, 1], and R0<1, the DFE of the proposed model is stable globally asymptotically and unstable otherwise.
Proof. To prove our result we define consider the following Lyapunov function
Where Wi, i=1,2⋯4, are arbitrary positive constants to be chosen latter. Using lemma (5.1), the time derivative of (5.4), along the solution of (3.1) is given by
Using x01=Λhd1 and x03=Λmd2, we get
Choosing the constants W1=W2=β2γ2Λmd2 and W3=W4=d1 and after simplification, we get
CDαtV(t) is negative for R0<1. Therefore, by theorem (2.4) [23,24], the DFE E0, is globally asymptotically stable in Φ.
6.
Stability of endemic equilibrium
Here, we present the global stability of the system (3.1) at E1. At the steady state the model (3.1) at E1 we obtained
Theorem 6.1. If R0>1, the EE E1 of the system (3.1) is stable globally asymptotically.
Proof. We consider the following Lyapunov function:
Using lemma (5.1), the derivative of L(t) along the solutions of (3.1) is as follows
By direct calculations, we have that:
It follows from (6.3-6.6)
Make use of arithmetical-geometrical inequality we have in equation (6.7)
Therefore, CDαtL≤0 and hence by using theorem (2.4) the EE E1 of the proposed model is globally asymptotically stable whenever R0>1.
7.
Numerical results
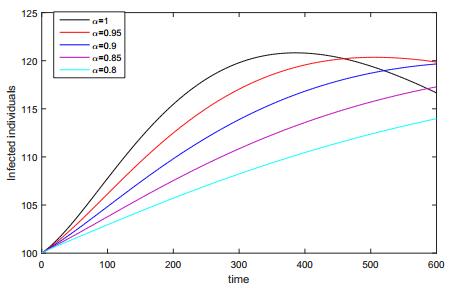
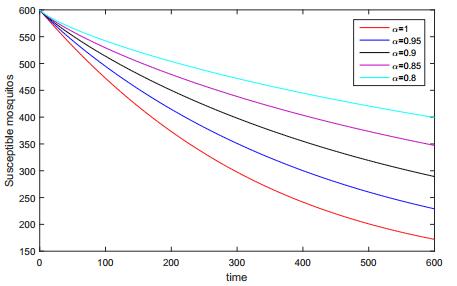
The present section is devoted to obtain the numerical results of the proposed Zika fractional order model (3.1). The Adams-type predictor-corrector method is applied to obtained the approximate solution of the model. The numerical values used in the simulations are Λh=1.2, Λm=0.3, β1=0.0004, β2=0.005, d1=0.004, d2=0.0014, γ1=0.02. γ2=0.0003. The graphical results using different five values of fractional order α=1,0.95,0.9,0.85,0.8 are presented in the Figures 1-4. From these figures we can see than the susceptible human and mosquitoes are increasing when we decreases the fractional order α. While there is a significant decrease in the graphs of infected humans and mosquitoes with decrease in α. Hence, the fractional order α has an important role in the model.
8.
Conclusion
Zika is a rapidly spreading epidemic and is one of serious health issue, specially for pregnant women. A number of deterministic models have been presented in last few year, for the possible control and eradication of this infection from the community. But, almost all of these models are based on classical or local derivative. In order to better explore the complex behavior of Zika infection, in this paper, a fractional order transmission model in Caputo sense is developed. The detail analysis such as positivity and existence of the solution, basic reproduction numberer and model equilibria of the proposed model are presented. The stability results for both local and global cases are derived in detail in fractional environment. From the numerical results we conclude that the fractional order derivative provides more information about the proposed model which are unable by classical integer-order epidemic models. Also these results ensure that by including the memory effects in the model seems very appropriate for such an investigation. In future, we will explore the proposed model using non-local and non-singular fractional derivatives presented in [9,10].
Conflict of Interest
All authors declare no conflict of interest.










 DownLoad:
DownLoad: