Generalized conformable operators: Application to the design of nonlinear observers

1.   Introduction

Since the second half of the 20th century, fractional calculus found several useful applications, as well as extensions of classical results, through the Riemann-Liouville integral and the Riemann-Liouville and Caputo derivatives; however, new definitions of fractional operators have been recently proposed, which aim to improve the modelling of many systems. Thus, several operators are now being considered in the development of the theory and the applications of fractional calculus ^{[1,2,3,4,5,6,7,8,9,10]}; some recent reviews and analysis of the most used definitions are found in ^[11,12].

However, the main disadvantage of the original fractional operators is that they fail to accomplish with some rules existing in integer-order calculus, e.g. the Leibniz product rule, the quotient rule, and the chain rule. For these reasons, R. Khalil et al. proposed a new definition, called the conformable fractional derivative, which fulfilled these properties along with some other classical calculus results ^[13]. After that, T. Abdeljawad extended the study of this derivative to other properties in calculus and linear systems ^[14]. Thenceforth, several works have developed the theory and applications of this operator ^{[15,16,17,18,19,20,21,22,23]}.

On the other hand, U. Katugampola proposed other fractional operators, which also accomplish with the classical results ^[24]. These operators are also being studied and applied ^{[25,26,27,28,29,30]}.

Furthermore, based on the tools proposed by Khalil et al. and Katugampola, Akkurt et al. proposed the so-called Generalized fractional derivative ^[31], which generalizes both operators and also satisfies the classical properties and results from integer-order calculus. This derivative is general because of the freedom to choose its kernel; however, a better nomenclature for this operator may be "generalized conformable derivative", given that it encompasses the conformable-type derivatives formerly proposed, and thus in this work we will refer to it in this way. This operator has also been considered for further studies and applications ^{[32,33,34,35]}.

Due to the level of generality and its accomplishment of the classical calculus results, the idea of developing theoretical and applied results using the generalized conformable derivative is appealing, because this would encompass results using the conformable operators. In the work ^[36], another generalized conformable operator ^[37] has been assessed, and it was observed that the results obtained with this operator outperformed the ones obtained with integer and fractional operators, presenting even finite-time stability.

On another topic, there exist several definitions and configurations of observers, which are useful for different applications. Consider e.g. the intermediate estimator ^[38], which is useful for observing states and faults simultaneously in systems with Lipschitzian nonlinearities without requiring to fulfill the observer matching condition from other adaptive observers. As another example, the interval observer in ^[39] is useful for estimation in discrete-time linear systems with unknown but bounded disturbance, which is less restrictive than other similar approaches. Therefore, in order to assess the operators studied in this paper, we will consider the design of a nonlinear quadratic regulator (NQR) ^[40] and a high-gain observer ^[41]. The NQR provides robust stability with a minimized energy-like performance index, besides being computationally efficient. Moreover, the high-gain observer is robust in the presence of external disturbances or uncertainties (like noise in the measurement), has a rapid exponential decay of the estimation error, and the speed of convergence increases by augmenting the gain of the observer. Besides, both observers are simple to implement and practical for many applications.

As it was mentioned, the generalized conformable derivative proposed by Akkurt et al. generalizes other operators and satisfies the classical calculus rules, thus, it is of great interest to develop results in theory and applications, which would enclose existing or possible results using conformable-type derivatives. For example, it may be very useful to obtain generalized results in control theory, such as modelling, control algorithms, observer design, stability proofs, among others. Therefore, in this work we use the generalized conformable derivative to design a NQR-based estimator and a high-gain observer, for a class of nonlinear systems with the generalized conformable derivative in their dynamics. Then, with the aid of a generalized exponential function, some Lyapunov-like generalized stability theorems are proven. These stability results are considered in the design of the observers, and later some numerical simulations are carried out in two systems. Finally, for evaluating the performance of the operator studied, the results obtained are compared with those obtained with the integer and the fractional-order operators.

The main contributions of this work are the following:

● A generalized conformable exponential function, which implies exponential stability for the class of systems studied.

● Some Lyapunov-like theorems to verify the stability of a class of nonlinear systems using the derivative in question, generalizing the work presented in ^[42].

● The design of stable nonlinear observers for these type of systems, which are validated by numerical simulations applied to the generalized models of the simple pendulum and the Van der Pol chaotic oscillator.

The paper is structured as follows: In Section 2, the generalized conformable derivative is presented, with some of its properties and existing results. In Section 3, the generalized conformable exponential function is presented, in order to prove some Lyapunov-like theorems for systems with the generalized conformable derivative. Section 4 shows the design of nonlinear observers for systems with this derivative, which are proven to be exponentially stable in a generalized sense. In Section 5, numerical simulations are carried out in the models mentioned, in order to evaluate the performance of the observers; moreover, they are compared with their integer and fractional-order versions. Finally, some conclusions, potential applications and future work are given in Section 6.

2.   Generalized conformable derivative

In this section, the definition of the generalized conformable derivative is presented, along with some of its properties. For the sake of comparison, consider first the original definition proposed by Khalil et al.

Definition 1 (^[13]) Given a function $f:[0, \infty)\rightarrow \mathbb{R}$, the conformable derivative of $f$ of order $\alpha$ is defined by

$\forall t > 0, \; \alpha\in(0, 1)$. If f is $\alpha$-differentiable in some $(0, a), \; a > 0$, and $\lim_{t\rightarrow 0^+}f^{(\alpha)}(t)$ exists, then define

Now, we present the definition of the generalized operator proposed by Akkurt et al.

Definition 2 (^[31]) Let $k:[a, b]\rightarrow \mathbb{R}$ be a strictly increasing, continuously differentiable nonnegative map such that $k(t), k'(t) > 0$, whenever $t > a$. Given a function $f:[a, b]\rightarrow \mathbb{R}$ and $\alpha\in(0, 1)$ a real, we say that the generalized conformable derivative of $f$ of order $\alpha$ is defined by

given that the limit exists. We say then that $f$ is $\alpha$-differentiable.

If $f$ is $\alpha$-differentiable in $(0, a)$ and $\lim_{t\rightarrow 0^+}f^{(\alpha)}(t)$ exists, then define

Henceforth, the operator $D^\alpha$ will always refer to the generalized conformable derivative (GCD), and the $\alpha$-differentiability property will refer to this operator.

Theorem 1. (^[31]) Let $f:[a, b]\rightarrow \mathbb{R}$ be a continuously differentiable function and $t > a$. Then, $f$ is $\alpha$-differentiable at $t$ and

Also, if $f'$ is continuous at $t = a$, then

Remark 1. There exist $\alpha$-differentiable functions which are not differentiable in the usual sense, and thus the order $\alpha$ and the kernel $k(t)$ may help to obtain different results between the GCD and the integer-order derivative.

Theorem 2. (^[31]) If a function $f:[a, b]\rightarrow \mathbb{R}$ is $\alpha$-differentiable at $a > 0, \alpha\in(0, 1]$, then $f$ is continuous at $a$.

Theorem 3. (^[31]) Let $\alpha\in(0, 1]$ and $f, g$ be $\alpha$-differentiable at a point $t > 0$. Then

1. $D^\alpha[cf(t)+dg(t)] = cD^\alpha f(t)+dD^\alpha g(t), \; \forall c, d\in\mathbb{R}$ (linearity).

2. $D^\alpha[t^n] = \frac{k^{1-\alpha}(t)}{k'(t)}nt^{n-1}, \; \forall n\in\mathbb{R}$.

3. $D^\alpha c = 0$, for all constant functions $f(t) = c$.

4. $D^\alpha[f(t)g(t)] = f(t)D^\alpha g(t)+g(t)D^\alpha f(t)$ (product rule).

5. $D^\alpha\left[\frac{f(t)}{g(t)}\right] = \frac{f(t)D^\alpha g(t)-g(t)D^\alpha f(t)}{\left[g(t)\right]^2}$ (quotient rule).

6. $D^\alpha[f(t)\circ g(t)] = \frac{k^{1-\alpha}(t)}{k'(t)}f'(g(t))g'(t)$ (chain rule).

Moreover, consider the following result.

Lemma 1. Let $f:[a, \infty)\rightarrow \mathbb{R}$ be $\alpha$-differentiable on $(a, \infty)$. If $D^{\alpha}f(t)\geq 0$ (respectively $\leq 0$) $\forall t\in(a, \infty)$, then $f$ is an increasing function (respectively decreasing).

Proof. This proof can be derived from the extension of the Mean Value Theorem for the operator in question ^[31].

Remark 2. Let $x:[a, \infty)\rightarrow \mathbb{R}^n$ be $\alpha$-differentiable on $(a, \infty)$. Let $P$ be a symmetric positive definite matrix. Then

On the other hand, consider the generalized conformable integral.

Definition 3. (^[31]) Let $t\geq a\geq 0$. Also, let $f$ be a function defined on $(a, t]$ and $\alpha\in\mathbb{R}$. Let $k:[a, b]\rightarrow \mathbb{R}$ be a continuous nonnegative map such that $k(t), k'(t)\neq 0$. Then, the $\alpha$-generalized conformable integral of $f$ is defined by

if the Riemann improper integral exists.

3.   Generalized conformable exponential stability

Consider the following class of nonlinear systems

where $x\in\mathbb{R}^n, \; f:\mathbb{R}^+\times\mathbb{R}^n\rightarrow \mathbb{R}^n$ is a given nonlinear function satisfying $f(t, 0) = 0, \; \forall t\geq 0$, and $\alpha\in(0, 1]$.

Throughout this paper, the function $||\cdot||$ represents the Euclidean norm.

Definition 4. The origin of system (3.1) is said to be

i) stable, if for every $\varepsilon > 0$ and $t_0\in\mathbb{R}^+, \; \exists\delta(\varepsilon, t_0)$ such that for any $x_0\in\mathbb{R}^n, \; ||x_0|| < \delta\Longrightarrow||x(t)|| < \varepsilon, \; \forall t\geq t_0$.

ii) attractive, if for any $t_0\geq 0, \; \exists c(t_0) > 0$ such that for any $x_0\in\mathbb{R}^n, \; ||x_0|| < c\Longrightarrow\lim_{t\rightarrow \infty}x(t) = 0$.

iii) asymptotically stable, if it is stable and attractive.

iv) globally asymptotically stable, if it is asymptotically stable for any $x_0\in\mathbb{R}^n$.

The next function will be the main instrument used in the stability proofs for systems involving the operator considered.

Definition 5. The generalized conformable exponential function $E_{k}^{\alpha}(\cdot)$, with parameters $\alpha, \; \gamma$ and $t_0$, and which depends on the kernel $k(t)$, is defined as

where $\alpha\in(0, 1], \; \gamma\in\mathbb{R}$ and $k(t)$ is as stated in Definition 2.

Remark 3. It is not difficult to verify that $E_{k}^{\alpha}(\gamma, t, t_0)$ is $\alpha$-differentiable, and that

Now, the notion of generalized conformable exponential stability is introduced.

Definition 6. The origin of system (3.1) is said to be generalized conformable exponentially stable (GCES) if

with $t > t_0$ and $C, \gamma > 0$.

Lemma 2. Let $g:[t_0, \infty)\rightarrow \mathbb{R}^+$ be an $\alpha$-differentiable function on $(t_0, \infty)$ such that

where $\gamma > 0$ and $\alpha\in(0, 1]$. Then

Proof. Let $h(t) = g(t)E_{k}^{\alpha}(\gamma, t, t_0)$. From Theorem 3, we have

Since $D^\alpha h(t)\leq 0$, from Lemma 1 $h(t)$ is a decreasing function. Hence, $h(t)\leq h(t_0)$, which gives the result.

The results that follow may be considered as Lyapunov-type stability theorems for the class of systems considered in this work.

Theorem 4. Let $x = 0$ be an equilibrium point for system (3.1). Let the function $V:\mathbb{R}^+\times\mathbb{R}^n\rightarrow R$ be $\alpha$-differentiable with respect to $t$ and $x$, and consider $a_i\; (i = 1, 2, 3)$ as arbitrary positive constants. If the following conditions are satisfied:

(i) $a_1||x||^2\leq V(t, x)\leq a_2||x||^2$,

(ii) $D^\alpha V(t, x)\leq-a_3||x||^2$,

then the origin of system (3.1) is GCES.

Proof. From conditions (i) and (ii) we have

Applying Lemma 2 to the last inequality, we get

Also from (i), we have

Thus

and

with $C = (a_2/a_1)^{1/2}$ and $\gamma = a_3/(2a_2)$. Therefore, the origin of system (3.1) is GCES.

Remark 4. Note that GCES implies the classical exponential stability, taking $\alpha = 1$ and $k(t) = t$.

Definition 7. A continuous function $k:\mathbb{R}^+\rightarrow \mathbb{R}^+$ is said to belong to class $\mathcal{K}$ if it is strictly increasing and $k(0) = 0$. It belongs to class $\mathcal{K}_\infty$ if $\lim_{t\rightarrow +\infty}k(t) = +\infty$.

Theorem 5. Let $x = 0$ be an equilibrium point for system (3.1). Let the function $V:\mathbb{R}^+\times\mathbb{R}^n\rightarrow R$ be $\alpha$-differentiable with respect to $t$ and $x$, and consider $k_i\; (i = 1, 2, 3)$ as functions of class $\mathcal{K}$. If the following conditions are satisfied:

(i) $k_1(||x||)\leq V(t, x)\leq k_2(||x||)$,

(ii) $D^\alpha V(t, x)\leq-k_3(||x||)$,

then the origin of system (3.1) is locally asymptotically stable.

Furthermore, if $k_i\in\mathcal{K}_\infty\; (i = 1, 2, 3)$, then the origin of system (3.1) is globally asymptotically stable.

Proof. First, from condition (ii) and the fact that $k(t)$ is strictly increasing and nonnegative, one has that $D^\alpha V(t, x)\leq0$ for almost all $t\geq t_0$. Thus, from Lemma 1 $V(t, x)$ is monotonically decreasing, but given that $V(t, x)\geq0$ from (i), this implies the existence of some real number $L = \lim_{t\rightarrow \infty}V(t, x)\geq 0$.

Moreover, condition (i) is equivalent to $k_2^{-1}(V(t, x))\leq||x||\leq k_1^{-1}(V(t, x))$, since $k_1$ and $k_2$ are monotonically increasing functions. By the same argument, one has that $k_3(||x||)\geq k_3(k_2^{-1}(V(t, x)))$. Therefore, condition (ii) implies that:

Now, suppose that $L > 0$. Furthermore, define

it is clear that $\lambda > 0$ when $L > 0$. Then,

Finally, by considering that $V(t, x) \leq V(t_0, x_0)$ (from Lemma 1), one has that

hence, from Lemma 2 one has

and consequently, $L = \lim_{t\rightarrow \infty}V(t, x) = 0$, but it was supposed that $L > 0$, which is a contradiction. Thus, $L = 0$ and from (i), one has that $\lim_{t\rightarrow \infty}x(t) = 0$ and therefore, the origin of system (3.1) is locally asymptotically stable.

Considering the case where $k_i\in\mathcal{K}_\infty\; (i = 1, 2, 3)$, it is straightforward that the origin of system (3.1) is globally asymptotically stable.

Remark 5. Note that Theorems 4 and 5 involve the generalized conformable derivative. Then, these results may serve as meta-theorems for proving generalized exponential and asymptotic stability for a more general class of dynamical systems, which consider a larger family of differential operators.

4.   Application to observer design

In this section, a pair of observers are proposed for a class of nonlinear systems with the GCD in their dynamics. Moreover, the stability results presented in the past section are used to prove that the estimation error of both observers is GCES.

Consider the following class of systems

where $0 < \alpha < 1$, $\textbf{x}\in\mathbb{R}^n$ is the state vector, $\textbf{u}\in\mathbb{R}^m$ is the input vector, $y\in\mathbb{R}$ is the output and $f:\mathbb{R}^n\times\mathbb{R}^m\rightarrow \mathbb{R}^n$ is locally Lipschitz in $\textbf{x}$ and uniformly bounded in $\textbf{u}$.

Former system may be rewritten in the following canonical form ^[43]

where $A$ is an upper shift matrix ($A:\mathbb{R}^n\rightarrow \mathbb{R}^n, \; A_{i, j} = \delta_{i+1, j}$, with $\delta_{i, j}$ the Kronecker delta), $C = [1\; 0\; 0\; \dots\; 0]$, the pair $(A, C)$ is observable, and $\Upsilon(\textbf{x, u})$ satisfies

in a region $D$. The observers will be designed for the system expressed in this canonical equivalent.

4.1.   NQR-based estimator

First, consider the following generalized conformable estimator, based on the design of a nonlinear quadratic regulator (NQR)

where $\hat{\textbf{x}}, \; \textbf{K}_i\in\mathbb{R}^n, \; 1\leq i\leq m$. For this system, consider the following result.

Lemma 3. (^[44]) Given a stable $n\times m$ matrix $\hat{A}$ and $\gamma > 0$, there exists a positive definite, symmetric matrix $P$ such that

if and only if there exists another positive definite, symmetric matrix $\hat{P}$ such that

We have the following LMI, equivalent to (4.5)

Note that, for some $\varepsilon > 0$,

Now, let $\upsilon = \Upsilon(\textbf{x}, \textbf{u})-\Upsilon(\hat{\textbf{x}}, \textbf{u})$. Considering the Lipschitz condition (4.3), the estimation error $\textbf{e} = \textbf{x}-\hat{\textbf{x}}$ and the solution $P$ of (4.6), we have the following inequality ^[44]

Remark 6. A observer is said to be generalized conformable exponentially stable if its estimation error $\textbf{e}$ is GCES.

Theorem 6. Consider system (4.2) with $(A, C)$ observable. If $\bar{A} = A-\textbf{K}_1C$ is a stable matrix and $\textbf{K}_iC > 0$, then the estimator (4.4) is a generalized conformable exponentially stable observer for system (4.1).

Proof. From (4.2) and (4.4), the fractional dynamics of the estimation error is

Since $\bar{A}$ is stable and $\varphi > 0$, from Lemma 3 there exists some $P > 0$. Consider $V = ||\textbf{e}||^2_P = \textbf{e}^TP\textbf{e}$ a candidate Lyapunov function that satisfies the Rayleigh-Ritz inequality

Moreover, from Remark 2, (4.7) and (4.8), we have

Given that, $\textbf{K}_iC$ is a positive real number for any $i$, one has that $P\textbf{K}_iC > 0$, and consequently,

Finally, from Theorem 4, (4.9) and (4.10) it follows that $\textbf{e} = 0$ is GCES and we have

with $C = \sqrt{\frac{\lambda_{max}~~(P)}{\lambda_{min}~~(P)}}, \; \gamma = \frac{\varepsilon}{2\lambda_{max}~~(P)}\; and\; e(t_0) = e_0$.

4.2.   High-gain observer

Now, consider the following generalized conformable high-gain observer (HGO)

where $F_{\infty} = \lim_{t\rightarrow \infty}F_\theta(t)$, with $F_\theta(t)$ a positive definite matrix solution of

Remark 7. Given that $F_\infty$ is a constant matrix, $D^\alpha F_\infty = 0$ and thus it can be obtained from the following algebraic equation

The coefficients of $F_\infty$ are given by

where $\alpha_{i, j}$ is a symmetric positive definite matrix independent of $\theta$ ^[41,45].

Theorem 7. The high-gain observer (4.11) is a generalized conformable exponentially stable observer for system (4.1).

Proof. Consider again the observation error $\textbf{e} = \textbf{x}-\hat{\textbf{x}}$. From (4.2) and (4.11), the fractional dynamics of the error is

with $\upsilon = \Upsilon(\textbf{x}, \textbf{u})-\Upsilon(\hat{\textbf{x}}, \textbf{u})$. Consider $V = ||\textbf{e}||^2_{F_\infty} = \textbf{e}^TF_\infty\textbf{e}$ a candidate Lyapunov function that satisfies the Rayleigh-Ritz inequality

Moreover, from Remark 2 and (4.12) we have

Thus, considering that $\textbf{e}^TF_\infty\upsilon\leq \varphi \lambda_{max}~~(F_\infty)\; ||\textbf{e}||^2$, one has that

Finally, for $\theta > 2\varphi\lambda_{max}~~(F_\infty)/\lambda_{min}~~(F_\infty)$, the system is asymptotically stable. In addition, according to Theorem 4, it follows that $\textbf{e} = 0$ is GCES and we have

with $C = \sqrt{\frac{\lambda_{max}~~(F_\infty)}{\lambda_{min}~~(F_\infty)}}, \; \gamma = \frac{\theta\lambda_{min}~~(F_\infty) - 2\varphi\lambda_{max}~~(F_\infty)}{2\lambda_{max}~~(F_\infty)}\; and\; \bf e(t_0) = \bf e_0$.

Remark 8. Note that the results of Theorems 4.1 and 4.2 involve the generalized conformable derivative, and given that this operator encompasses the conformable type derivatives, these results may serve as meta-theorems for designing exponentially stable estimators for the class of systems that consider this family of operators.

5.   Simulation results

In this part, numerical simulations of the observation scheme developed in the former section are presented. In order to assess the performance of the observers, the simulations were performed on two models. Also, these results will be compared to the ones obtained with the integer ^[40,41] and fractional-order ^[46,47] versions of the same observers. The simulations were performed using Simulink^® from MATLAB^®.

For the fractional-order dynamics, the Caputo operator was considered, given to the need of having integer-order initial conditions; to implement this operator, the ninteger fractional derivative block from D. Valério was used. Furthermore, in order to implement the GCD, the function $k(t) = 2t^2+2t+2$ was used for both systems and observers.

5.1.   Simple pendulum

Consider the simple pendulum, which has been extended and studied in its fractional version ^{[48,49,50,51]}. The model of the simple pendulum with GCD is the following:

where $x_1 = \theta$ (angular position), $x_2 = \omega$ (angular velocity), $g = 9.81\; m/s^2$ and $L = 1\; m$. Rewrite the system to its canonical form:

with $A = \left[\begin{array}{c c} 0 & 1 \\ 0 & 0 \end{array}\right], \; \Upsilon(\textbf{x}) = \left[\begin{array}{c} 0 \\ -\frac{g}{L}\sin(x_1) \end{array}\right]$ with Lipschitz constant $\varphi = \frac{g}{L}$, and $C = \left[\begin{array}{c c} 1 & 0 \end{array}\right]$.

The fractional dynamics in the model of the pendulum, as has been seen with other mechanical systems, adds the effect of damping on the trajectories ^[50,51], which represents an additional parameter which value depends on the fractional order. In the case of the dynamics with GCD, due to the freedom of choice of the order $\alpha$ and the kernel $k(t)$, the resulting model can be seen as a pendulum with some time-varying parameters (a nonautonomous system). From the graphs of the states shown in this section it can be observed, for example, a variation in the frequency of oscillation.

From (4.4), taking $m = 3$, the NQR-based estimator for system (5.1) is

where $\textbf{K}_i = [K_{i1}\; K_{i2}]^T$.

For the simulation $\alpha = 0.98$ was selected, the gains were chosen as $\textbf{K}_1 = [4.0076\; 3.1305]^T$, $\textbf{K}_2 = [4.905\; 4.905]^T$, $\textbf{K}_3 = [5\; 2.4525]^T$, and the initial conditions are $x_1(0) = \pi/2$, $x_2(0) = 0$, $\hat{x}_1(0) = \pi$, $\hat{x}_2(0) = 0$.

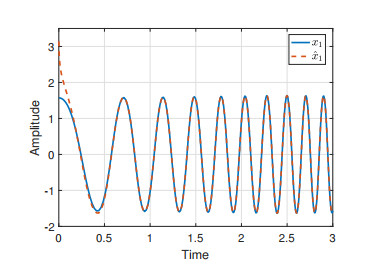
Figures 1 and 2 show the estimations of the states by the NQR-based observer with the GCD. Moreover, Figures 3 and 4 show the behaviour of the estimation errors for each state in the integer, fractional, and generalized conformable cases, for the same observer gains and initial conditions. It can be seen that, although the fractional observer performs slightly better that the integer-order one, the estimation errors obtained with the GCD converge to zero faster than the other.

Now, consider the high-gain observer (4.11). For the pendulum system, the observer takes the structure

Simulations for this observer were performed using the same initial conditions and $\theta = 25$. Figures 5 and 6 show the estimations of the states with the GCD, and Figures 7 and 8 show the behaviour of the estimation errors for each state in the integer, fractional, and generalized conformable cases. In the three versions the same gain and initial conditions have been used. It can be seen, as in the former case, that the estimation errors obtained with the GCD converge to zero faster than with the integer and fractional-order versions, which again show a similar performance with a slight advantage for the fractional case.

5.2.   Van der Pol oscillator

The subject of chaotic oscillators is a research area of great interest due to their theoretical results and applications. Particularly, the Van der Pol oscillator was proposed to study oscillations in vacuum tube circuits ^[52]. This model has also been extended and studied in its fractional-order counterpart ^{[53,54,55,56]}. Using the GCD, the model of the system is

where $\varepsilon$ is the control parameter. The system is rewritten in its canonical form:

with $A = \left[\begin{array}{c c} 0 & 1 \\ 0 & 0 \end{array}\right], \; \Upsilon(\textbf{x}) = \left[\begin{array}{c} 0 \\ -x_1-\varepsilon\left(x_1^2-1\right)x_2 \end{array}\right]$ with Lipschitz constant $\varphi = \max\left\{1+2\varepsilon|x_1||x_2|+\varepsilon+\varepsilon|x_1^2|\right\}$, and $C = \left[\begin{array}{c c} 1 & 0 \end{array}\right]$.

Hence, the Van der Pol system will perform as a master system, to which the observers designed for it will serve as slaves, looking to synchronize with it.

From (4.4) with $m = 3$, the NQR-based estimator for system (5.2) is

where $\textbf{K}_i = [K_{i1}\; K_{i2}]^T$.

For the simulation, the gains were selected as $\textbf{K}_1 = [2.3094\; 1.5166]^T$, $\textbf{K}_2 = [1.15\; 1.15]^T$, $\textbf{K}_3 = [3\; 0.575]^T$. The parameters are $\alpha = 0.9$, $\varepsilon = 0.1$ and the initial conditions were chosen as $x_1(0) = -0.25$, $x_2(0) = 1.2$, $\hat{x}_1(0) = 3$, $\hat{x}_2(0) = 1.8$. With these values, the Lipschitz constant is set as $\varphi = 2.3$.

Figures 9 and 10 show the estimations of the states by the NQR-based observer with the GCD. Moreover, Figures 11 and 12 show the behaviour of the estimation errors for each state in the integer, fractional, and generalized conformable cases, for the same observer gains and initial conditions. It can be seen that although the fractional observer performs slightly better that the integer-order one, the estimation errors obtained with the GCD converge to zero faster than the other.

Moreover, Figure 13 shows the phase portrait obtained with the estimated states $\hat{x}_1$ and $\hat{x}_2$ obtained from the NQR-based estimator with the GCD.

Now, consider the high-gain observer (4.11). For the Van der Pol oscillator, the observer takes the structure

Simulations for this observer were performed using the same initial conditions and $\theta = 1$. Figures 14 and 15 show the estimations of the states with the GCD, and Figures 16 and 17 show the behaviour of the estimation errors for each state in the integer, fractional, and generalized conformable cases. In the three versions the same gain and initial conditions have been used. It can be seen, as in the former case, that the estimation errors obtained with the GCD converge to zero faster than with the integer and fractional-order versions, which again show a similar performance with a slight advantage to the fractional case.

Finally, Figure 18 shows the phase portrait obtained with the estimated states $\hat{x}_1$ and $\hat{x}_2$ obtained from the high-gain observer with the GCD.

6.   Conclusions

Given the definition of the generalized conformable derivative, which encompasses some noninteger operators of conformable type, it may be very useful to obtain generalized results in control theory, such as modelling, control algorithms, observer design, stability proofs, among others. Thus, in this work an observation scheme, composed of a NQR-based estimator and a high-gain observer, was designed for a class of nonlinear systems which dynamics involve this derivative. With the aid of the proposed generalized conformable exponential function, it was proven that the observers were exponentially stable in a generalized way by means of some Lyapunov-like theorems. The estimators were applied to the generalized models of the simple pendulum and the Van der Pol oscillator, evaluating their performance by means of numerical simulations, and comparing these results with the ones obtained with their integer and fractional-order counterparts.

According to the simulation results, the integer and fractional-order estimators had a similar performance regarding the behaviour of the estimation error, being slightly better the fractional-order case. However, both cases were outperformed by the one with the generalized conformable derivative, which exhibited a faster speed of convergence with similar or less overshoot, for both systems. However, these results may be improved with an adequate choice of gains and the selection of the kernel $k(t)$. Furthermore, comparing the performance of each observer, both presented excellent outcomes, with slight differences that occurred due to the choice of their parameters.

To conclude, the following may be considered as future directions for this work:

● One of the limitations of this work is that the performance of the generalized conformable derivative relies on the selection of the kernel $k(t)$, which in this paper has been empirical. Thus, it may be possible to obtain optimal results with a structured selection method for the kernel.

● It may be interesting to apply this operator to other kind of estimators, like the reduced-order, algebraic, sliding-mode observers and the Kalman filter, and compare their advantages and theoretical considerations.

● Moreover, another theme of interest is the extension of the theory of the generalized conformable derivative to include time-varying and complex orders, and even real orders higher than one; besides, to consider also their discrete-time versions. It would be desired to study all these cases in order to compare the results obtained in applications, like the scheme proposed here, and in other existing systems and methodologies.

● Finally, some immediate potential applications of the generalized conformable derivative are related to the grey system. This model possesses several uses, such as the prediction of the production and consumption of combustibles and renewable energies, the calculation of wind turbine capacities, among others. Recently, the gray system has been extended to its conformable ^[57] and generalized conformable ^[58] versions; thus, there is a good amount of potential works related to these areas.

Acknowledgments

Guillermo Fernández-Anaya would like to acknowledge the support and funding provided by División de Investigación y Posgrado (DINVP) de la Universidad Iberoamericana. Universidad Iberoamericana Ciudad de México, Departamento de Física y Matemáticas, Prolongación Paseo de la Reforma 880, Colonia Lomas de Santa Fe, Álvaro Obregón, Ciudad de México 01219, México.

Conflict of interest

No potential conflict of interest was reported by the authors.