3D analysis of modified F-contractions in convex b-metric spaces with application to Fredholm integral equations

1.   Introduction and Preliminaries

Czerwik ^[1] introduced b-metric and proved fixed point theorems in it. It was further extended to partial b-metric and dislocated b-metric spaces in the past years. Chen et al. ^[2] introduced convex b-metric space and established various fixed point theorems. On the other hand, various authors generalized the metric space into many other spaces (see ^{[3,4,5,6,7,8,9,10,11]}).

Wardowski ^[12] introduced the idea of $F$-contraction which was later followed by many authors who delivered interesting results of $F$-contraction. One of them was presented by Cosentino et al. ^[13] who expanded $F$-contraction in $F$-contraction of Hardy Roger’s type. For more generalization of $F$-contraction, we refer the readers to see (^[14,15,16]).

In this article we discuss $F$-contraction in the frame of convex b-metric space using generalized Mann’s iteration algorithm. However, we have modified definition of $F$-contraction of Nadler type by eliminating two of its conditions (F3) and (F4). Cosentino et al. ^[17] have proved the results for multivalued simple F-contraction of Banach type, while our results have been proved for F-Reich type contraction for single valued mappings. As the conditions (F3) and (F4) of the mappings belonging to the set $\mathcal{F}$ have been removed, thus, our results are more generalized than the results presented by Cosentino. Further we have presented application of our results in finding a unique solution to the Fredholm integral equation of the second kind. The reader can study more about Fredholm integral equation of the second kind in the article written by Teukolsky et al. ^[18].

Some fundamental definitions related to our work are given below:

Definition 1.1 (^[17]): Let $k\ge 1$ be a real number. We denote by $\mathcal{F}$ the family of all functions $F:{R}^{+}\to R$ with the following properties:

(F1) $F$ is strictly increasing;

(F2) for each sequence $\left\{{x}_{n}\right\}\subset {R}^{+}$ of positive numbers $\lim _{n \rightarrow \infty}{a}_{n} = 0$ if and only if $\lim _{n \rightarrow \infty}F\left({a}_{n}\right) = -\infty$;

(F3) for each sequence $\left\{{x}_{n}\right\}\subset {R}^{+}$ of positive numbers with $\lim _{n \rightarrow \infty}{a}_{n} = 0$, there exists $m\in \left(\mathrm{0, 1}\right)$ such that $\lim _{n \rightarrow \infty}{\left({a}_{n}\right)}^{m}F\left({a}_{n}\right) = 0$;

(F4) for each sequence $\left({a}_{n}\right)\subset {R}^{+}$ of positive numbers such that $\tau +F\left(s{a}_{n}\right)\le F\left(s{a}_{n-1}\right)$ for all n ∈ N and some $\tau \in {R}^{+}$, then $\tau +F\left({s}^{n}{a}_{n}\right)\le F\left({s}^{n-1}{a}_{n-1}\right)$ for all n ∈ N.

Definition 1.2 (^[17]): Let $(X, d, s)$ be a b-metric space. A multivalued mapping $T : X \to CB\left(X\right)$ is called an $F$-contraction of Nadler type if there exist $F\in {\mathcal{F}}_{s}$ and $\tau \in {R}^{+}$ such that

for all $x, y\in X$ with $Tx = Ty$.

Note that, in our theorems, we will consider ${\mathcal{F}}_{b}$ as the class of functions satisfying only (F1) and (F2) which modifies the definition of $F$-contraction.

Definition 1.3 (^[1]): Assume that $E = \varnothing$ with $1\le k\in R$. If ${b}_{k}:E\times E\to [0, +\infty)$ satisfies the following axioms, for each $a, b, c\in E$:

ⅰ). ${b}_{k}\left(a, b\right) = 0$ if and only if $a = b$;

ⅱ). ${b}_{k}\left(a, b\right) = {b}_{k}(b, a)$;

ⅲ). ${b}_{k}(a, b)\le k[{b}_{k}\left(a, c\right)+{b}_{k}\left(c, b\right)]$

Then the pair $(E, {b}_{k})$ is known as b-metric space with $k\ge 1$.

Definition 1.4 (^[1]): Suppose $\left({a}_{n}\right)$ is a sequence in $E.$ Then

1) $\left({a}_{n}\right)$ is convergent to a point $a\in E$ if $\lim\limits _{n \rightarrow \infty}d\left({a}_{n}, a\right) = 0.$

2) $\left({a}_{n}\right)$ is Cauchy if $\underset{n, m\to \infty }{\mathrm{lim}}d\left({a}_{n}, {a}_{m}\right) = 0.$

3) The space $(E, {b}_{k})$ is complete if every Cauchy sequence $\left({a}_{n}\right)\subset E$ is convergent to a point $a\in E$.

Definition 1.5 (^[2]): Assume that $E = \varnothing$ and define a mapping ${b}_{k}:E\times E\to [0, +\infty)$. Let $I = \left[\mathrm{0, 1}\right]$ with a continuous mapping $\eta :E\times E\times I\to E$ such that

for each $o\in E$ and $(a, b, \gamma)\in E\times E\times I$.

Definition 1.6 (^[2]): Assume that $\eta :E\times E\times I\to E$ is a convex structure on a b-metric space $(E, {b}_{k})$. Then $\left(E, {b}_{k}, \eta \right)$ is known as convex b-metric space.

Suppose that $\left(E, {b}_{k}, \eta \right)$ is convex b-metric space with a self mapping $f$. Then for ${a}_{n}\in E$ and ${\gamma }_{n}\in \left[\mathrm{0, 1}\right],$a generalize Mann’s iteration sequence $\left\{{a}_{n}\right\}$is defined as;

2.   Fixed point results of $\mathit{F}$-Kannan contraction in convex b-metric spaces

This section evaluates $F$-Kannan contraction for the existence of unique fixed point results.

Definition 2.1: Assume that $F\in {\mathcal{F}}_{b}$, $(E, {b}_{k}, \eta)$ be a convex b-metric space with $k>1$. Then $f:E\to E$ is known as $F$-Kannan contraction if for $h:E\times E\to \left[0, \left.\frac{1}{2}\right)\right.$ the following hold:

for every $a, b\in E$.

Theorem 2.2: Suppose $(E, {b}_{k}, \eta)$ is a complete convex b-metric space with ${a}_{n} = \eta ({a}_{n-1}, f{a}_{n-1};{\gamma }_{n-1}) \; n\in N$ having ${\gamma }_{n-1}\in \left(0, \left.\frac{1}{4{k}^{2}}\right]\right.$ and $f:E\to E$ is an $F$-Kannan contraction. If $h:E\times E\to \left[0, \frac{1}{4{k}^{2}}\right],$then $f$ has a unique fixed point in $E$.

Proof. By (2.1) and hypothesis, we write

Now, Since

Therefore,

This implies that

Using (F1), we write

As

hence

Say

then

Therefore (2.3) becomes

Operating (F1) again, we write

Similarly,

Consequently, we note

taking limit $n\to \infty$, we obtain

By (F2), we get

Now, Since

therefore, we note that $\underset{n\to \infty }{\mathrm{l}\mathrm{i}\mathrm{m}}{b}_{k}\left({a}_{n}, {a}_{n+1}\right) = 0$. Next, we prove that the sequence $\left\{{a}_{n}\right\}$ is Cauchy. Suppose on the contrary that $\left\{{a}_{n}\right\}$ is not Cauchy. Then we can find subsequences $\left\{{a}_{\mu \left(u\right)}\right\}$ and $\left\{{a}_{\omega \left(u\right)}\right\}$ of $\left\{{a}_{n}\right\}$ and a positive real number ${ϵ}_{0} \; \mu \left(u\right)>\omega \left(u\right)>u$ with $\mu \left(u\right)$ as the smallest natural index such that

and

We deuce that

Now,

Since, by (2.1) we can write

Therefore,

Hence, using (F1) and (2.6), we write

Exerting limit $u\to \infty$, we obtain

This shows that

which is a contradiction. Hence, $\left\{{a}_{n}\right\}$ is a Cauchy sequence. The completeness of $E$ assure the existence of an element ${a}^{*}$ such that

Next, we prove that ${a}^{*}$ is the fixed point of $f$. For this, we know that

As

therefore,

Utilizing (F1), we obtain

Now, clearly

therefore,

Consequently,

Hence, ${b}_{k}\left({a}^{*}, f{a}^{*}\right) = 0$, i.e., ${a}^{*}$ is the fixed point of $f$. It remains to prove that ${a}^{*}$ is the only fixed point $f$. Suppose on the contrary that ${a}^{**}$ be another fixed point of $f$. Then

By hypothesis,

As a result, we get $F\left(k{b}_{k}\left({a}^{*}, f{a}_{n}\right)\right) = -\infty$. By (F2), we obtain $\lim _{n \rightarrow \infty}k{b}_{k}\left({a}^{*}, f{a}_{n}\right) = 0$. Similarly, $\lim _{n \rightarrow \infty}k{b}_{k}\left({a}^{**}, f{a}_{n}\right) = 0$. i.e., $\lim _{n \rightarrow \infty}k{b}_{k}\left({a}^{*}, f{a}_{n}\right)+\lim _{n \rightarrow \infty}k{b}_{k}\left({a}^{**}, f{a}_{n}\right) = 0$. Thus, using (F2), we obtain

Consequently, $\lim _{n \rightarrow \infty}{b}_{k}\left({a}^{*}, {a}^{**}\right) = 0$. This shows that ${a}^{*} = {a}^{**}$.

3.   Fixed point results of $\mathit{F}$-Reich contraction

This section examines $F$-Reich contraction for the possible existence of a unique fixed point. Also, an example is given to explain the proved theorem.

Definition 3.1: Assume that $F\in {\mathcal{F}}_{b}$, $(E, {b}_{k}, \eta)$ be a convex b-metric space with $k>1$. Then $f:E\to E$ is known as $F$-Reich contraction if for $g, h:E\times E\to \left[0, \left.\frac{1}{2}\right)\right.$ the following holds:

for every $a, b\in E$ provided that $\left(g+2h\right)\left(a, b\right)<1$.

Theorem 3.2: Suppose $(E, {b}_{k}, \eta)$ is a complete convex b-metric space with ${a}_{n} = \eta ({a}_{n-1}, f{a}_{n-1};{\gamma }_{n-1}) \; n\in N$ having ${\gamma }_{n-1}\in \left(0, \left.\frac{1}{4{k}^{2}}\right]\right.$ and $f:E\to E$ is an $F$-Reich contraction. If $g\left(a, b\right)+2h\left(a, b\right)\le \frac{1}{4{k}^{2}},$then $f$ has a unique fixed point in $E$.

Proof. By hypothesis and (2.1)

Now, since

therefore,

This leads to

Using (F1), we write

As,

and

hence

Say

then

Therefore (3.2) becomes

Operating (F1) again, we write

Similarly,

Consequently, we note

applying limit $n\to \infty$

By (F2), we get

Now, since

therefore, we note that $\underset{n\to \infty }{\mathrm{l}\mathrm{i}\mathrm{m}}{b}_{k}\left({a}_{n}, {a}_{n+1}\right) = 0$. It remains to prove that the sequence $\left\{{a}_{n}\right\}$ is Cauchy. Suppose on the contrary that $\left\{{a}_{n}\right\}$ is not Cauchy. Then we can find subsequences $\left\{{a}_{\mu \left(u\right)}\right\}$ and $\left\{{a}_{\omega \left(u\right)}\right\}$ of $\left\{{a}_{n}\right\}$ and a positive real number ${ϵ}_{0} \; \mu \left(u\right)>\omega \left(u\right)>u$ with $\mu \left(u\right)$ as the smallest natural index such that

and

We deduce that

Now,

Since, by (3.1)

Therefore,

Hence, using (F1) and (3.5), we write

exerting limit $u\to \infty$, we obtain

This shows that

which is a contradiction. Hence, $\left\{{a}_{n}\right\}$ is a Cauchy sequence. The completeness of $E$ assure the existence of an element ${a}^{*}$ such that

Next, we prove that ${a}^{*}$ is the fixed point of $f$.

As

therefore, using above equation and (3.6)

Utilizing (F1), we obtain

Clearly,

therefore,

consequently,

Hence, ${b}_{k}\left({a}^{*}, f{a}^{*}\right) = 0$, i.e., ${a}^{*}$ is the fixed point of $f$. It remains to prove that ${a}^{*}$ is the only fixed point $f$. Suppose on the contrary that ${a}^{**}$ be another fixed point of $f$. Then

which is a contradiction. Hence, ${b}_{k}\left({a}^{*}, {a}^{**}\right) = 0$. This shows that ${a}^{*} = {a}^{**}$.

Example 3.3: Suppose $E = \left[0, \left.+\infty \right)\right.$, $fa = \frac{a}{6}$ for all $a\in E$ and choose a mapping ${b}_{k}:E\times E\to \left[0, \left.+\infty \right)\right.$ defined as ${b}_{k}\left(a, b\right) = {(a-b)}^{2}$. Demonstrate $\mathrm{\eta }:E\times E\times \left[\mathrm{0, 1}\right]\to E$ as $\eta \left(a, b; \gamma \right) = \gamma a+\left(1-\gamma \right)b$ for all $a, b\in E$. Fix ${a}_{n} = \eta ({a}_{n-1}, f{a}_{n-1};{\gamma }_{n-1})$ and ${\gamma }_{n-1} = \frac{1}{4{k}^{2}} = \frac{1}{16}$. Now, for all $o, a, b\in E$, we write

Hence, ${(E, b}_{k}, \eta)$ is a convex b-metric space with $k = 2$. Next, define $g, h:E\times E\to \left[0, \left.\frac{1}{2}\right)\right.$ by

and fix $h(x, y) = 0$. Observe that $g\left(a, b\right)+2h\left(a, b\right)\le \frac{1}{4{k}^{2}}\cdot$ Then

i.e., $F\left(k{b}_{k}\left(fa, fb\right)\right)\le F\left[g\left(a, b\right){b}_{k}\left(a, b\right)+h\left(a, b\right)\left\{{b}_{k}\left(a, fa\right)+{b}_{k}\left(b, fb\right)\right\}\right]$. Observe that $F\left(x\right) = ln\left(x\right)$ satisfies (F1) and (F2). Thus, the inequality (3.1) is satisfied for $\tau \in \mathrm{ln}\left(\frac{4{k}^{2}+2}{4{k}^{2}+1}\right)$. Now

Similarly

Therefore, ${a}_{n} = {\left(\frac{17}{96}\right)}^{n}{a}_{0}$ and $f{a}_{n} = \frac{1}{6}{\left(\frac{17}{96}\right)}^{n}{a}_{0}$. Letting $n\to \infty,$ we get ${a}_{n}\to 0$ and $f{a}_{n}\to 0$. i.e., $0$ is the fixed point of $f$. For uniqueness, suppose on contrary that $r$ is another fixed point of $f$ then ${b}_{k}\left(0, r\right)>0$, say ${b}_{k}\left(0, r\right) = \delta$. Hence

which is a contradiction. Therefore, $0$ is the only fixed point of $f$.

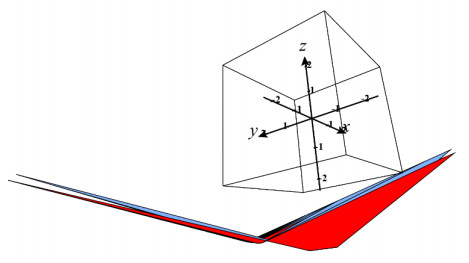
In Figure 1, the graph in blue and purple colour represent $g(a, b){b}_{k}\left(a, b\right)+h\left(a, b\right)\left\{\begin{array}{c}{b}_{k}\left(a, fa\right)\\ +{b}_{k}(b, fb)\end{array}\right\}$ while that in red and green shows $k{b}_{k}\left(a, b\right)$. The first one clearly dominates the later one, hence confirming the contractive inequality.

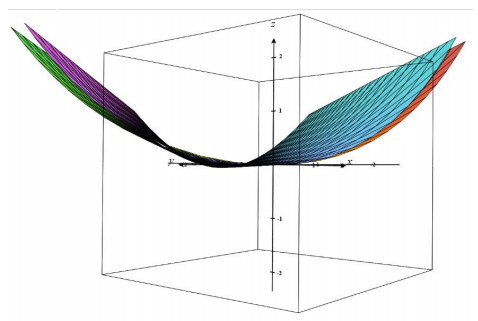
In Figure 2, the graph in red colour represent $F\left[g(a, b){b}_{k}\left(a, b\right)+h\left(a, b\right)\left\{\begin{array}{c}{b}_{k}\left(a, fa\right)\\ +{b}_{k}(b, fb)\end{array}\right\}\right]$ while that in blue shows $F\left(k{b}_{k}\left(a, b\right)\right)$. Note that the higher is the graph at z-axis, the bigger is the value of the mapping $F$. Hence, both the figures clearly demonstrate that the inequality (3.2) holds true.

In the next section, we discuss the application of our results to Fredholm integral equation of the second type.

4.   Application

Fredholm integral equation can be classified as an Equation of the first kind and Equation of the second kind. The solution of Fredholm integral equation leads to Fredholm theory. Fredholm linear equation has key importance in inverse problems, linear forward modeling, the theory of signal processing and distributions, etc. Adomian decomposition method is an effective tool for solving Fredholm integral equation. In this section we provide an application of our results to the solution of Fredholm integral equations:

Theorem 4.1: Suppose Eq (4.1) with $a\le t, \tau \le b$, $S\in E[a, b]$ and the continuous mapping $T(t, \tau)$. Let $W = \max _{a \leq t, \tau \leq b}T(t, \tau)$, $g:E[a, b]\to E[a, b]$ and $\alpha, \beta :E\left[a, b\right]\times E\left[a, b\right]\to \left[\mathrm{0, 1}\right)$ with $\alpha \left(p, q\right)+2\beta \left(p, q\right)\le \frac{1}{4{k}^{2}}$ satisfy the following condition;

for all $p, q\in E[a, b]$. Then the integral equation (4.1) has a unique solution.

Proof. Suppose $E = c[p, q]$ and ${b}_{k}:E\times E\to [0, +\infty)$ is defined by

and define a mapping $T$ by

Set

where ${\gamma }_{n-1}\in \left(0, \left.\frac{1}{4{k}^{2}}\right]\right..$ Clearly $({E, b}_{k}, \eta)$ is a convex b-metric space with $k = {2}^{p-1}$. Now

Hence, $g$ is an $F$-Reich contraction. Therefore, by Theorem (3.2) there exist a unique solution of $g\left(p\left(t\right)\right)$.

Corollary 4.2: Consider Eq (4.1) with $a\le t, \tau \le b$, $S\in E[a, b]$ and the continuous mapping $T(t, \tau)$. If $W = \max _{a \leq t, \tau \leq b}T(t, \tau)$, $g:E[a, b]\to E[a, b]$ and $\beta :E\left[a, b\right]\times E\left[a, b\right]\to [0, \left.\frac{1}{2}\right)$ with $\beta :H[a, b]\times H[a, b]\to \left(0, \left.\frac{1}{4{k}^{2}}\right]\right.$ satisfy the following condition;

for all $p, q\in E[a, b]$. Then the integral equation (4.1) has a unique solution.

5.   Conclusion

This paper has modified the definition of generalized $F$-contractions by eliminating the conditions (F3) and (F4) and thus proved some principal fixed point results in the setting of convex b-metric spaces. Throughout this research, it was observed that the elements ${a}_{n}$ are taken from the convex structure $\eta \left(a, b, \gamma \right)$. Thus, investigated fixed point for $F$-Reich contractions and $F$-Kannan contractions followed by the verification of our results with the help of example and graphs. Further, an application of our results in finding a unique solution to the Fredholm integral equation is described. The paper furthers the research already done on the topic of $F$-contractions and fixed point theory.

Conflict of interest

The authors declare that there is no conflict of interest.