Five new methods of celestial mechanics

1.   Normal forms

Let us consider the Hamiltonian system

with $n$ degrees of freedom in a vicinity of the stationary solution

If the Hamiltonian function $\gamma(\boldsymbol{\xi}, \boldsymbol{\eta})$ is analytic at the point (1.2), then it is expanded into the power series

where ${\bf{p}} = (p_1, \ldots, p_n)$, ${\bf{q}} = (q_1, \ldots, q_n)\in\mathbb{Z}^n$, ${\bf{p}}, {\bf{q}}\geqslant0$, ${{ {{ ξ}}}}^{{\bf{p}}} = \xi_1^{p_1}\xi_2^{p_2}\ldots\xi_n^{p_n}$. Here $\gamma_{{\bf{p}}{\bf{q}}}$ are constant coefficients. As the point (1.2) is stationary, than the expansion (1.3) begins from quadratic terms. They correspond to the linear part of the system (1.1). Eigenvalues of its matrix are decomposed in pairs:

Let $\boldsymbol{\lambda} = (\lambda_1, \ldots, \lambda_n)$. The canonical changes of coordinates

preserve the Hamiltonian structure of the system. Here ${\bf x} = (x_1, \dotsc, x_n)$, ${\bf{y}} = (y_1, \dotsc, y_n)$.

Theorem 1. ([4,§ 12]). There exists a formal canonical transformation (1.5), bringing the system (1.1) to the normal form

where the series

contains only resonant terms with

and the square part $g_2({\bf{x}}, {\bf{y}})$ has its own normal form (i.e. the matrix of the system is the Hamiltonian analog of the Jordan normal form).

If $\boldsymbol{\lambda}\neq0$, then the normal form (1.6) is equivalent to a system with smaller number of degrees of freedom and with additional parameters. The normalizing transformation (1.5) conserves small parameters and linear automorphisms of the initial system (1.1)

Local families of periodic solutions satisfy the system of equations

where $a$ is a free parameter. For the real initial system (1.1), the coefficients $g_{{\bf{p}}{\bf{q}}}$ of the complex normal form (1.7) satisfy to special properties of reality and after a standard canonical linear change of coordinates $({\bf{x}}, {\bf{y}})\rightarrow(\bf{X}, \bf{Y})$ the system (1.6) transforms in a real system [7,Ch. Ⅰ]. There are several methods of computation of coefficients $g_{{\bf{p}}{\bf{q}}}$ of the normal form (1.6), (1.7). The most simple method was described in the book ^[29]. Normal forms of periodic Hamiltonian systems was described in ^[9,10], see also [7,Ch. Ⅱ]. Normal forms near a periodic solution, near an invariant torus and near family of them see in [7,Chs. Ⅱ,Ⅶ,Ⅷ], [6,Part Ⅱ]. Normal form is useful in study stability, bifurcations and asymptotic behavior of solutions.

2.   Newton polyhedrons

2.1.   Truncated Hamiltonian function [8,Ch. 4]

Let ${\bf x} = (x_1, \ldots, x_n), \; {\bf{y}} = (y_1, \ldots, y_n)$ and $\boldsymbol{\mu} = (\mu_1, \ldots, \mu_s)$ be canonical variables and small parameters respectively. Let a Hamiltonian function be

where ${\bf{p}} = (p_1, \ldots, p_n)$, ${\bf x}^{ \bf p} = x_1^{p_1}\ldots x_n^{p_n}$ and $h_{ \bf p \bf q \bf r}$ are constant coefficients. To each term of sum (2.1) we put in correspondence its vectorial power exponent $Q = (\bf p, \bf q, \bf r)\in \mathbb{R}^{2n+s}$. Set ${\bf S}$ of all points $Q$ with $h_Q\neq0$ in sum (1.1) is called as support ${\bf S} = {\bf S}(f)$ of the sum (2.1). The convex hull $\Gamma({\bf S}) = \Gamma(f)$ of the support ${\bf S}$ is called as the Newton polyhedron of the sum (2.1). Its boundary consists of vertices $\Gamma_j^{(0)}$, edges $\Gamma_j^{(1)}$ and faces $\Gamma_j^{(d)}$ of dimensions $d$: $1 < d\leqslant 2n+s-1$. Intersection ${\bf S}\bigcap\Gamma_j^{(d)} = {\bf S}_j^{(d)}$ is the boundary subset of set ${\bf S}$. To each generalized face $\Gamma_j^{(d)}$ (including vertices and edges) there correspond:

● normal cone ${\bf U}_j^{(d)}$ in space $\mathbb{R}_*^{2n+s}$, which is dual to space $\mathbb{R}^{2n+s}$;

● truncated sum

It is the first approximation to the sum (2.1), when

near ${\bf U}_j^{(d)}$.

So by truncated Hamiltonian function we can describe the approximate problems.

2.2.   Restricted 3-body problem [8,Ch. 4,Section 4]

Let the two bodies ${\bf P}_1$ and ${\bf P}_2$ with masses $1-\mu$ and $\mu$ respectively turn in circular orbits around their common mass center with the period $T$. The plane circular restricted three-body problem consists in the study of the plane motion of the body ${\bf P}_3$ of infinitesimal mass under the influence of the Newton gravitation of bodies ${\bf P}_1$ and ${\bf P}_2$. In the rotating (synodical) standardized coordinate system the problem is described by the Hamiltonian system with two degrees of freedom and with one parameter $\mu$. The Hamiltonian function has the form

Here the body ${\bf P}_1 = \{X, Y:x_1 = x_2 = 0\}$ and the body ${\bf P}_2 = \{X, Y:x_1 = 1, \; x_2 = 0\}$, where $X = (x_1, x_2)$, $Y = (y_1, y_2)$. We consider the small values of the mass ratio $\mu\geqslant 0$. When $\mu = 0$ the problem turns into the two-body problem for ${\bf P}_1$ and ${\bf P}_3$. But here the points corresponding to collisions of the bodies ${\bf P}_2$ and ${\bf P}_3$ must be excluded from the phase space. The points of collisions split in parts solutions to the two-body problem for ${\bf P}_1$ and ${\bf P}_3$. For small $\mu > 0$ there is a singular perturbation of the case $\mu = 0$ near the body ${\bf P}_2$. In order to find all the first approximations to the restricted three-body problem, it is necessary to introduce the local coordinates near the body ${\bf P}_2$

and to expand the Hamiltonian function in these coordinates. After the expansion of $1/\sqrt{(\xi_1+1)^2+\xi_2^2}$ in the Maclaurin series, the Hamiltonian function (2.2) takes the form

where $f$ is the convergent power series, where the terms of order less then three are absent. Let for each term of sum (2.3) we put

Then support ${\bf S}$ of the expansion (2.3) consists of the points

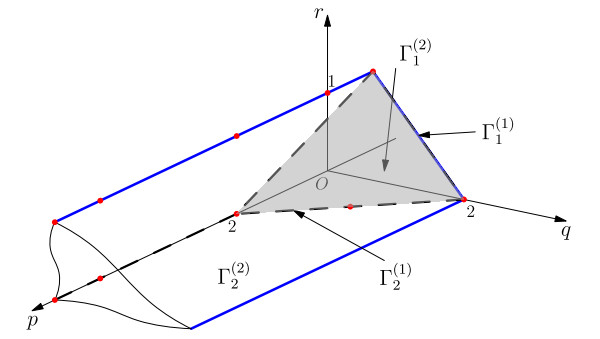
where $k = 3, 4, 5, \ldots$ The convex hull of the set ${\bf S}$ is the polyhedron $\boldsymbol{\Gamma}\subset\mathbb{R}^3$. The surface $\partial\boldsymbol{\Gamma}$ of the polyhedron $\boldsymbol{\Gamma}$ consists of faces $\boldsymbol{\Gamma}_j^{(2)}$, edges $\boldsymbol{\Gamma}_j^{(1)}$ and vertices $\boldsymbol{\Gamma}_j^{(0)}$. To each of the elements $\boldsymbol{\Gamma}_j^{(d)}$ there corresponds the truncated Hamiltonian $\hat{h}_j^{(d)}$, that is the sum of those terms of Series (2.3), the points $Q = (p, q, r)$ of which belong to $\boldsymbol{\Gamma}_j^{(d)}$. Figure 1 shows the polyhedron $\boldsymbol{\Gamma}$, which is the semi-infinite trihedral prism with an oblique base. It has four faces and six edges. Let us consider them.

The face $\boldsymbol{\Gamma}_1^{(2)}$, which is the oblique base of the prism $\boldsymbol{\Gamma}$, contains vertices

To the face there corresponds the truncated Hamiltonian function

It describes the Hill problem ^[23], which is a non-integrable one. The power transformation

reduces the corresponding Hamiltonian system to the Hamiltonian system with the Hamiltonian function of the form (2.4), where $\xi_i, \eta_i, \mu$ must be substituted by $\tilde{\xi}_i, \tilde{\eta}_i, 1$ respectively.

The face $\boldsymbol{\Gamma}_2^{(2)}$ contains points

To the face there corresponds the truncated Hamiltonian function $\hat{h}_2^{(2)}$, which is obtained from the function $h$ when $\mu = 0$. It describes the two-body problem for ${\bf P}_1$ and ${\bf P}_3$, which is an integrable one.

The edge $\boldsymbol{\Gamma}_1^{(1)}$. It includes points $(0, 2, 0)$ and $(-1, 0, 1)\subset {\bf S}$. The corresponding truncated Hamiltonian function is

It describes the two-body problem for ${\bf P}_2$ and ${\bf P}_3$. The power transformation (2.5) transforms it into the Hamiltonian system with the Hamiltonian function of the form (2.6), where $\xi_i, \eta_i, \mu$ must be substituted by $\tilde{\xi}_i, \tilde{\eta}_i, 1$ respectively.

The edge $\boldsymbol{\Gamma}_2^{(1)}$ includes points $(2, 2, 0), (1, 1, 0), (0, 2, 0)\subset {\bf S}$. To it there corresponds the truncated Hamiltonian function (2.4) with $\mu = 0$. It describes the intermediate problem (between the Hill problem and the two-body problem for ${\bf P}_1$ and ${\bf P}_3$), which is an integrable one. This first approximation was introduced by Hénon ^[20]. Thus, the first approximation to the original restricted problem with the Hamiltonian function (2.3) depends on the distance from the body ${\bf P}_2$ in the following manner:

● very close to the body ${\bf P}_2$, it is the two-body problem for bodies ${\bf P}_2$ and ${\bf P}_3$ with the Hamiltonian function (2.6);

● simply close, it is the Hill problem with Hamiltonian (2.4);

● farther from the body ${\bf P}_2$, it is the intermediate Hénon problem;

● and far from the body ${\bf P}_2$, it is the two-body problem for bodies ${\bf P}_1$ and ${\bf P}_3$.

Near the body ${\bf P}_2$, the periodic solutions to the restricted problem are either perturbations of periodic solutions to all four mentioned first approximations or they are results of the matching of the hyperbolic orbits of the two-body problem for ${\bf P}_2$ and ${\bf P}_3$ with arc-solutions to the two-body problem for ${\bf P}_1$ and ${\bf P}_3$, or to the intermediate problem. In ^{[3,24,25,26,27]} the periodic solutions to the intermediate problem were used as the generating ones in order to find quasi-satellite orbits of the restricted problem.

2.3.   Truncated systems

Now we consider the agammaegate of polynomials

To each $f_j$ there corresponds its support ${{\bf{S}}_j} = {\bf{S}}\left( {{f_j}} \right)$ and all the accompanying objects: polyhedrons $\boldsymbol{\Gamma}_j$, faces $\boldsymbol{\Gamma}_{jk_j}^{(d_j)}$, normal cones ${\bf U}_{jk_j}^{(d_j)}$, boundary subsets ${\bf S}_{jk_j}^{(d_j)}$, truncated polynomials $\hat{f}_{jk_j}^{(d_j)}$. Besides, to each non-empty intersection

there corresponds the agammaegate of truncations of the form

which is the first approximation to the agammaegate (2.7), when $\log |X|\to\infty$ near the intersection (2.8); and it is named the truncation of the agammaegate (2.7). We consider now the system of equations

corresponding to the agammaegate (2.7). To System (2.10) there correspond all objects indicated for the agammaegate (2.7), and also the truncated systems of equations

each of which corresponds to one agammaegate of truncations (2.9). We say that the truncated system (2.11) is the truncation of System (2.10) with respect to the order $P\neq0$ if the vector $P$ lies in the cone (2.8). Every truncated system (2.11) is one of the first approximations to complete system (2.10).

2.4.   Periodic solutions to periodic Hamiltonian system ^[9,10]

Normal form of a periodic Hamiltonian function with $n$ degrees of freedom near zero solution is reduced to a stationary Hamiltonian function

where ${\bf{p}}, {\bf q}\in\mathbb{Z}^n$, $\bf r\in\mathbb{Z}^s$, $m\in\mathbb{Z}$, ${\bf{p}}, {\bf q}, \bf r\geqslant0$ and

For $\boldsymbol{\mu} = 0$, expansion of $h$ (2.12) begins from terms of order 3. Local families of periodic solutions to the initial system correspond to local families of stationary points of the reduced normal form with Hamiltonian (2.12). These stationary points satisfy system of equations

To solve the system, we must to consider truncated systems and find their solutions, which gives the first approximations to solutions of the system (2.13). Other applications: the Beletskii equation for oscillation of a satellite ^[11]; the problem of periodic orbits with close approach to a planet and to Earth ^[5].

3.   Generating families of periodic solutions

3.1.   Method

Let a Hamiltonian function $H(\boldsymbol{\mu)}$ analytically depend from small parameters $\boldsymbol{\mu} = (\mu_1, \ldots, \mu_s)$ and corresponding Hamiltonian system has families $\mathcal{F}_j(\boldsymbol{\mu})$ of periodic solutions. Some of these families can have limits $\mathcal{F}_j(0)$, when $\boldsymbol{\mu}\to 0$. Families $\mathcal{F}_j(0)$ are called as generating. Their solutions are compositions of parts of solutions of the limiting Hamiltonian system with $\boldsymbol{\mu} = 0$.

If that limiting system is integrable, than generating families can be described analytically. That approach was proposed by Hénon ^[20] and was used for the Hill problem, for the restricted three-body problem ^[19,21,22,7], for the Belletskii equation ^[11].

3.2.   The Hill problem

Its Hamiltonian function is

The corresponding system

describes the motion of Moon $({\bf P}_3)$ with zero mass under attraction of Sun $({\bf P}_1)$ disposed at infinity and Earth $({\bf P}_2)$ with mass 1 disposed in origin. Hamiltonian (3.1) is analytic in

We make canonical transformation of coordinates

Then we obtain the Hamiltonian system

where

We put $\varepsilon = \sqrt{2|H|}$ and $H\to-\infty$. Then in limit we obtain system (3.2) with

It is the Hénon's problem. For $h_0$ system (3.2) is linear and hence integrable. It is enough to consider it for $h_0 = \dfrac12$. It has one regular periodic solution

If the orbit $(X_1(t), X_2(t))$ of a solution of the Hénon problem comes through the point

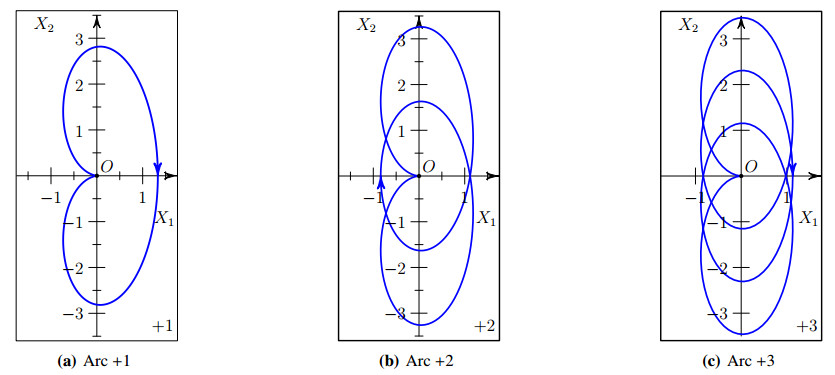
then the body $P_3$ collides with body $P_2$ and the solution cannot be continued through that collision. So solutions are divided into independent parts by the point (3.3). Hénon ^[20] found all arc-solutions, which begin and end by such collisions. They form the countable set of two types. The arc-solutions of the first type were denoted by symbol $\pm j$, $j\in\mathbb{N}$, and are epicycloids. In Figure 2 they are shown for $j = 1, 2, 3$.

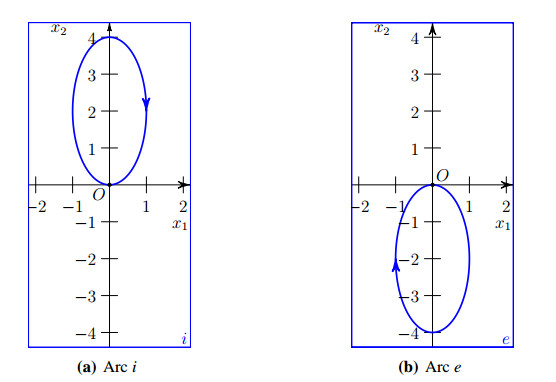
The arcs with negative values of $j$ are symmetric with respect to the axis $X_2$. The arc-solutions of the second type are denoted by symbols $i$ and $e$ and their orbits are ellipses passing through the origin (Figure 3).

A sequence of arc-solutions which does not contain two identical arcs of the second type in succession is a generating solution and it is called generating sequence for the Hill problem. All known families of periodic solutions of the Hill problem include at most one generating sequence.

4.   Generalized problems

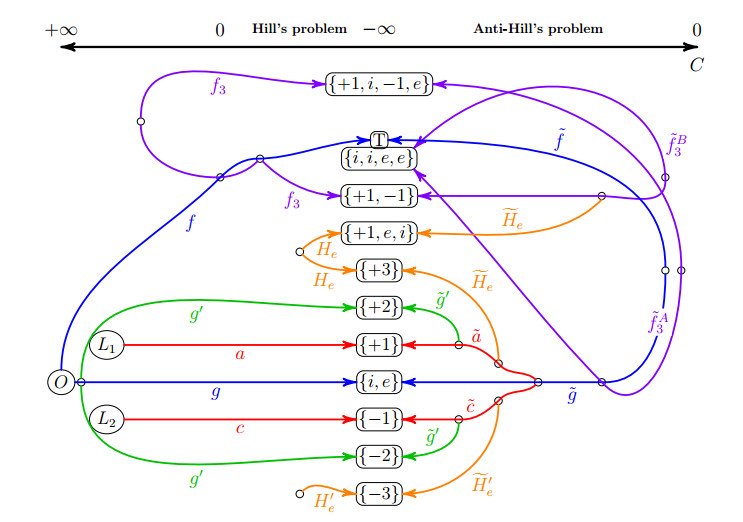
Usually in celestial mechanics we consider bodies with non-negative masses. But Batkhin ^[1] proposed to consider problems, where some masses are negative. In the Hill problem with mass of the body ${\bf P}_2$ equal to $-1$ (so-called anti-Hill problem), families of periodic solutions are continuations of families of periodic solutions of the usual Hill's problem. So computation of families of periodic solutions more convenient to make for both Hill's and anti-Hill's problems. Such approach gave new families for the Hill's problem.

Figure 4 shows diagram of connection between families of the Hill's (left part) and the anti-Hill's problems (right part). Central column gives generating sequences of the families.

5.   Sceletons

In some parts of the phase space of a Hamiltonian system there are a lot of families of periodic solutions. These families form a "skeleton" of the phase space. So computation of such families is very useful for study the structure of the phase space. Batkhin ^[2] mentioned that in systems with a finite group of symmetries, the majority of such families consists of periodic solutions, with are invariant under all symmetries of the group.

There are a lot of computed families of periodic solutions in different problems of celestial mechanics, but their number is not enough to form a skeleton. Recent results in that directions for the restricted three-body problem see in ^{[12,13,14,15,16,17,18]}.

Conflict of interest

All authors declare no conflicts of interest in this paper.