This paper reports entropy generation analysis of radiative micropolar fluid flow in porous medium. The mathematical model depicting convective boundary layer flow due to a vertically moving infinite plate bounding the porous medium on one side is solved numerically. An implicit finite difference method together with Gauss elimination method is used. The numerically computed velocity and temperature fields are employed to analyze entropy. The plots for entropy generation number for various sets of parameters are drawn. It is found that entropy generation number Ns decreases with increasing values of heat sink parameter Q and radiation parameter N whereas it increases with increasing values of Grashoff number Gr, Brinkman number Br. The Bejan number shows pronounced variations for the parameters entering into the problem.
Nomenclature: Be: Bejan number; Br: Brinkman number; cp: specific heat at constant pressure; Ec: Eckert number; g: acceleration due to gravity; Gr: Grashoff number; J: microinertia density; k: thermal conductivity; K: permeability of the porous medium; n: parameter related to microgyration vector and shear stress; N: radiation parameter; Ns: entropy generation number; Pr: Prandtl number; q: heat flux; qr: radiation heat flux; Q: suction/injection parameter; SG: local volumetric rate of entropy; SG0: characteristic entropy generation rate; T: temperature; u*, v*: components of velocities along and perpendicular to the plate, respectively; U0: scale of free stream velocity; V0: uniform normal velocity; x*, y*: distances along and perpendicular to the plate, respectively; Greek symbols: β: coefficient of volumetric thermal expansion of the fluid; γ: spin-gradient viscosity; δ: mean absorption constant; θ: dimensionless temperature; μ: fluid dynamics viscosity; μr: coefficient of vortex (microrotation) viscosity; σ: Stephan-Boltzman constant; ρ: fluid density; ϑ: fluid kinematic viscosity; ϑr: fluid kinematic rotational viscosity; w: angular velocity vector; Ω: dimensionless temperature difference; Subscripts: ∞: free stream condition; Superscripts: *: dimensional properties; ()': differentiation with respect to η
1.
Introduction
The realm of micropolar fluid theory developed by Eringen [1] is important as it deals with the fluids with microstructures. The Navier–Stokes equations address fluid flow by treating fluid, a continuum. However, when the flow system is treated on micro scale, then the classical Navier–Stokes equations may fail to fully account for fluid transport, simply because in the Navier–Stokes equations, molecular spin is not accounted for. In fact, when the flow scale is of the same order as of the molecular size, the molecules spinning alter the flow field qualitatively and quantitatively. In that case, one needs to consider microcontinuum flow. In fact, Eringen’s microcontinuum theory is a breakthrough for providing a mathematical basis to modify Navier–Stokes equations for microfluidics. In micropolar fluid theory, a micropolar fluid is considered as a continuous collection of finite-size particles, and there is an additional equation that accounts for the rotation of the microstructure. In fact, micropolar fluids are subclass of polar fluids (fluids with non-symmetric stress tensor) where the deformation of fluid elements is not taken into account. The micropolar fluid theory finds significance when the flow domain is of micro scale or nano scale. For example in channel flows, hydrodynamic quantities derived from Navier–Stokes equations do not match with experimental results, and the deviation mounts in cases of flow through narrow channels. Owing to real world applications, the microfluidics has emerged as a vibrant research domain. This includes modeling of the system as a design tool, and devising tool for solution strategies in case of mathematical models. Numerous studies have been reported on transport phenomenon of micropolar fluid in variety of configurations having technological applications. The theory of micropolar fluid and its extension to thermomicropolar fluids [2] may form suitable Non-Newtonian fluid models which can be used to analyze the behavior of exotic lubricants [3,4], shear flow [5], liquid crystals [6,7]. Kolpashchikov et al. [8] had derived a method to measure micropolar parameters experimentally. Ariman et al. [9,10] have presented a detailed review on micropolarfluid mechanics and applications. There have been some pertinent studies in different geometries pertaining to micropolar fluid [11,12,13,14,15,16,17,18,19,20,21].
Thermofluidics due to moving surface is important in understanding many natural phenomenon, and has many industrial applications. To be specific, processes such as fiber drawing, hot rolling, hot extrusion etc., involve heat transfer between moving surface and the surrounding fluid.
Given the wide array of applications, the problem of fluid flow due to a moving surface has been studied for variety of fluids. These theoretical studies cited in the ensuing text and the references contained therein offered a theoretical understanding of the phenomenon involved. This led to a want for solution strategies to handle the relevant mathematical models also.
Cess [22] examined thermal radiation with free convection. Hayday et al. [23] discussed free convection from a vertical plate with step discontinuities in surface temperature. Kao [24] examined heat transfer along a vertical plate with step jump. Cheng and Minkowycz [25] investigated heat transfer due to a vertical plate embedded in a porous medium. Raptis and Kafousias [26] reported the effect of magnetic field on heat transfer in porous medium fluid flow system bounded by an infinite vertical plate. Bianchi and Viskanta [27] investigated heat transfer on a continuous flat surface in a counter flow. Takhar et al. [28] investigated radiative MHD free convection of a gas past a semi-infinite vertical plate. Chamkha [29] discussed unsteady MHD convective heat and mass transfer due to a moving plate with heat absorption. Abdelkhalek [30] employed perturbation method to derive heat and mass transfer in convective flow due to a moving vertical surface. Makinde [31] offered similarity solution for convective flow due to a moving surface with internal heat generation and convective boundary condition. Srinivasacharya and Surender [32] derived non-similar solution for a convective nanofluid flow past a vertical plate in a doubly stratified porous medium. Khalid et al. [33] examined heat transfer in ferrofluid with cylindrical shape nanoparticles past a vertical plate subjected to ramped wall temperature embedded in a porous medium. Mishra et al. [34] examined free convective micropolar fluid flow and heat transfer over a shrinking sheet with heat source. Chen and Liu [35] employed the least square spectral collocation method for nonlinear radiative heat transfer in moving porous plate. These studies were primarily focused on first law of thermodynamics, and did not looked into second law of thermodynamics.
We know that the second law of thermodynamics offers a window to gauge inherent thermodynamic irreversibility which is related to thermodynamic efficiency. Thermodynamic efficiency is a challenging aspect for designing pertinent equipment where efficient energy usage is a primary concern. The endeavors of entropy management have gained currency in thermofluidics after the path breaking work of Bejan [36,37]. He had shown that thermofluidic systems could be treated for entropy aspects, and given a formulation for computing local volumetric rate of entropy generation. Taking a clue, theoretical entropy analysis of fluid systems has emerged as a vibrant field of research. Many relevant studies have been reported in the literature. These include various types of configurations e.g., theoretical and experimental, varied geometries for variety of fluids, different end conditions and assumptions.
Srinivasacharya and Hima Bindu [42] studied entropy generation of micropolar fluid flow through concentric cylinder annulus with slip and convective boundary conditions. In another paper, Srinivasacharya and Hima Bindu [43] reported a theoretical study on entropy generation of micropolar fluid flow in an inclined porous pipe with convective boundary conditions. Asha and Deepa [44] investigated entropy generation for peristaltic blood flow of magneto-micropolar fluid with thermal radiation with tapered asymmetric channel. Sahin [45] investigated entropy generation for a viscous fluid flow in a duct subjected to constant surface temperature. Murthy and Srinivas [46] examined entropy generation analysis for micropolar fluid between two horizontal parallel plates of a channel with constant temperature. The effect of thermal radiation on entropy generation due to micropolar fluid flow along a wavy surface was investigated by Chen et al. [47]. Many other authors [48,49,50,51,52,53,54,55,56,57] and references contained therein have reported relevant studies.
The study undertaken here may find applications in chemical, automobile and other industries where varied polymeric suspensions, lubricants, paints, colloids etc. can be viewed as micropolar fluid. In this backdrop, the study presented here is pertinent to offer an elegant solution strategy for entropy generation analysis for boundary layer flow of a micropolar fluid in porous medium.
2.
Mathematical model
Let us take a steady, laminar, incompressible 2-D radiative boundary layer flow of a micropolar fluid past a flat infinite plate moving vertically upwards embedded in a fluid saturated porous medium. The plate is perfectly attached with the porous medium bounding it on one side. The fluid is absorbing and emitting non-scattering radiation without phase change. The plate is subjected to a uniform heat flux and a uniform suction. We choose a Cartesian coordinate system to schemate the problem where x*, y*-axes are chosen along the plate and normal to it respectively.
The governing equations for the problem are (Kim [58], Raptis and Soundalgekar [59], Vyas et al. [60], Raptis [61]):
The equation of continuity (1) suggests that v∗=−V0
Consequently, the equations of momentum, rotation, and energy respectively become
The boundary conditions are:
where (u∗, v∗) denote velocity components in (x∗, y∗) directions, " ρ; the fluid density", " ϑ; the kinematic viscosity",
" ϑr; the kinematic rotational viscosity", " β∗; the coefficient of volumetric thermal expansion of the fluid", " K∗; the permeability of the porous medium", " J∗; the microinertia density", " w∗; the component of the angular velocity vector normal to the x∗y∗ -plane", " γ; the spin- gradient viscosity", "k; the thermal conductivity", "T; the temperature", " cp; specific heat at constant pressure", "q; the heat flux", " V0; the uniform normal velocity".
The boundary condition w∗=−n∂u∗∂y∗ describes the relationship between micro rotation variable w∗ and the surface stress. The parameter n is micro gyration vector associated with shear stress that takes values between 0 and 1.
The radiative heat flux qr in the energy equation is assumed to follow Rosseland approximation (Modest [41]) and is given as
Where σ and δ stand for the Stephan-Boltzman constant and the mean absorption constant respectively. We further assume that the temperature difference in the system is sufficiently small enough so that a Taylor series expansion of T4 about Tσ yields
We introduce the following non-dimensional quantities:
Furthermore, the spin-gradient viscosity γ is given by
Where β is the non-dimensional viscosity ratio.
In view of the Eq. (9), the governing Eqs. (2)–(4) together with the boundary condition (8) reduce to the following non-dimensional forms
The boundary conditions (5) in the non-dimensional form become
where
3.
Entropy generation
The local volumetric rate of entropy SG for the configuration under consideration is given as follows (Bejan [36,37], Vyas and Soni [38], Vyas and Shrivastva [39], Shrivastva, Vyas and Soni [40])
On prescribing the following characteristic quantities
SG0=q2T2σk (the characteristic entropy)
Ω=Tσ(qϑU0k) (the characteristic temperature ratio)
The entropy generation number Ns is obtained as follows
Where
H1=(1+N)(dθdη)2 stands for the heat transfer irreversibility (HTI),
H2=BrΩ[(1+β)(dudη)2+u2K+(1+β2)(dwdη)2] stands for fluid friction irreversibility (FFI),
Further, we define the Bejan Number Be as follows
4.
Solution
In order to solve the governing equations coupled with end conditions, we resorted to the numerical strategy based on finite difference method. Given the coupled nature of the equations concerned, an implicit finite difference method was a suitable choice. The essence of finite difference method is that the solution space [0, ησ] is discretized into finite number of equispaced mesh points ηj.
After having the said discretization, the derivatives appearing in the equations and/or in the end conditions are replaced by appropriate finite difference approximations. Similarly, variables are also discretized in the solution space. This discretization reduces the boundary value problem into a system of linear/nonlinear system of algebraic equations that can be handled by suitable numerical method as per the requirement.
In the present case, the employed finite difference scheme yielded a tridiagonal system of linear equations which was solved by Gauss elimination method. The working procedure has been explained as follows.
The boundary value problem [BVP] described by the Eqs. (10) and (12) is coupled and highly nonlinear. For solving the Eqs. (10) and (12), we need solution for w. We resorted to implicit finite difference numerical solution strategy by considering N+1 equispaced grid points.
The equation of angular momentum (11) is an ordinary differential equation and its solution reads
Where c and d are integration constants and to be determined by the boundary condition (13).
Since, as η→σ, w = 0, therefore d = 0
Further, when η=0, w = c. In view of Eq. (13), we get c=−n(dudη)η=0. Consequently, Eq. (19) becomes
We discretize dudη as follows
Consequently, (dudη)η=0 = (u1−u0h) leads Eq. (20) to take the following form
From the Eq. (21), we note that w would be available if u1 is known. In order to compute the unknown u1 a systematic procedure is adopted as described in the ensuing text.
In fact, the Eq. (21) is employed as in (10) and w is nothing but a finite difference approximation involving u1 with some coefficient.
The essence of the implicit finite difference scheme employed here for u and θ is that both u and θ are refined alternately in a systematic manner by choosing guess values, and the process is initiated first for θ in the solution space. This leads to some approximate solution for u. With this approximated u, the temperature θ is further refined. The process is repeated till the end conditions for both u and θ are satisfied to the prescribed error tolerance of order 10-6.
The BVP has been solved by finite difference method with Gauss elimination iteration scheme for θ. Firstly, the boundary value problem (10) with (13) is solved for u using finite difference scheme for some initial guess value of θ. Then, with this solution for u, the BVP (12) with (13) is solved for further refinement of θ by finite difference method. Here the coefficient matrix is strictly diagonally dominant which is sufficient to ensure that the iterative scheme is convergent for any initial approximation for θ.
In this procedure, the suitable guess values for θ were made by the following reasoning.
Here, we note that the boundary conditions for the temperature θ are
Hence we can go for approximating θ by taking
keeping the above end conditions for θ into account.
For solving the Eq. (10) under the associated boundary conditions, an implicit finite difference scheme has been developed. Assuming the following finite difference approximations
Then the differential Eq. (10) is discretized with the Eq. (21) as follows:
Which on rearranging terms takes the form
The end condition η→σ,u→0 is discretized as
u=[u1u2u3u4⋯⋯uN−1uN]. Consequently, uN=0 can be written as
The Eqs. (27) and (28) lead to the system of linear equations which, in matrix form reads
where
Now on employing Gauss elimination method in the matrix given by Eq. (29), we get the solution [u1u2u3u4⋯⋯⋯uN−1uN]T.
Hence, by including the given initial boundary condition for u, we obtain the complete solution for the velocity u corresponding to chosen guess value of θ as follows
u = [u0u1u2u3⋯⋯⋯uN−2uN−1uN]T.
This solution is then employed into the Eq. (12) to seek further refined value of θ that was guessed earlier.
Now we discretize the Eq. (12) by employing the finite difference approximations as follows:
Which on rearranging terms takes the form
Now from Eq. (22) we have
Then, the system of linear equations for θj's is
Which, in matrix form, is written as
where
Equation (34) is then solved by Gauss elimination method to yield θj's. With these currently approximated θj's, we now again solve Eq. (10) in the quest for better u. The process is continued until |uk+1−uk|<10−6 and |θk+1−θk|<10−6 criterion is achieved.
Here we would like to make a remark that the computational procedure was very rigorous in the sense that estimation of unknown quantities and their refinement to desired accuracy proved to be really painstaking owing to immense non-linear nature of the problem. In order to terminate the refinement process, the error tolerance was taken as ∈=10−6. These computations provided numerical solution to momentum and thermal regime which were readily used to compute entropy.
Interested readers may also refer to Altan and El-Mikkawy [62], Jiteng and Li [63] for a good insight on a new algorithm for general opposite-bordered tridiagonal (OBT) linear systems.
5.
Results and discussion
The computed quantities w, u and θ and their gradients are employed to evaluate entropy generation number to provide plots for entropy generation number and Bejan number.
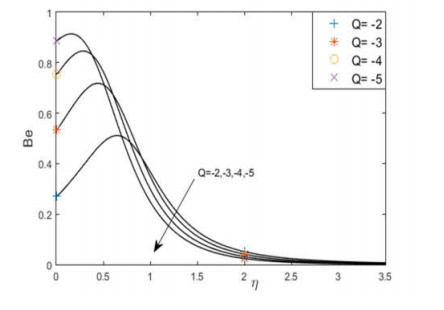
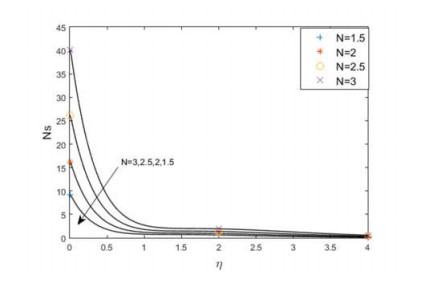
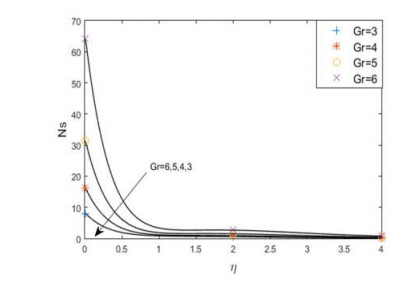
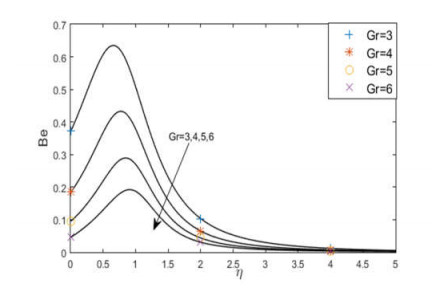
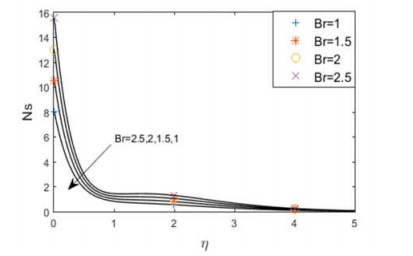
The Figure 1 depicts that NS decreases with increasing values of heat sink parameter Q. The rising values of Q indicate heat being poured out of the system. The information is of paramount application in thermal optimization in electronic devices which use heat sinks. The Figure 2 displays that the Bejan Number Be has peaks in the vicinity of the moving plate, and as we move farther away from the plate then the Bejan number tends to vanish. We also see that the Bejan number has higher peaks for larger values of heat sink parameter Q. Furthermore, the trend of Bejan number variations for varying values of Q is not uniform across the boundary layer. The Bejan number Be increases in the vicinity of the plate with increasing values of Q, however the trend is reversed at some spatial distance in the boundary layer. It may be attributed to the fact the larger values of Q amount to pronounced transfer of heat thereby causing rise in the heat transfer irreversibility. The Figure 3 shows entropy NS decreases for increasing values of radiation parameter N. The Figure 4 reveals that The Bejan number Be increases in the vicinity of the plate with increasing values of radiation parameter N, however the trend is reversed at some spatial distance in the boundary layer. The Figure 5 demonstrates that entropy number NS increases with the increasing values of Grashoff number Gr that accounts for the buoyancy. The Figure 6 depicting variations in Be shows that the Bejan number Be increases across the boundary layer for increasing values of Grashoff number Gr, and it attains peaks in the vicinity of the plate. The Figure 7 demonstrates the rise in NS for increasing values of Brinkman number. The higher values of Br stand for more pronounced frictional heat in the system there by causing a rise in entropy generation. However, the Bejan number decays for increasing Br values as is evident from the Figure 8.
6.
Conclusion
Entropy generation analysis for boundary layer flow in a porous medium saturated with micropolar fluid, was considered. The flow was assumed to be caused due to a vertically moving plate perfectly attached with porous medium and buoyancy. The mathematical model solved numerically led to a convergent system of linear equations. First the velocity, temperature and microrotation were computed, secondly these quantities and their gradients, were employed to compute entropy. The plots for entropy Ns and the Bejan number Be were drawn to give insight about the effect of the parameters entering in to the problem. it was found that the parameters have qualitative and quantitative effects on the quantities of interest. In fact, the entropy generation analysis (EGA) is an instrumental exercise for entropy generation minimization (EGM). The novelty of the problem is that it involves an efficient numerical method with a reasonably good error tolerance for wide range of parameters values. Besides the numerical procedure, the thermofluidics considered here has ample applications where in the configurations may exist as a single independent unit or may be a part of some larger setup. The study may find applications in chemical, automobile and other industries where varied polymeric suspensions, lubricants, paints, colloids, body fluids, poly-liquids, liquid foams, polymeric additives, liquid crystals, sediments etc. can be viewed as micropolar fluid. The main findings of the problem are:
1) NS decreases with increasing values of heat sink parameter Q, radiation parameter N.
2) NS increases with increasing values of Grashoff number Gr, brinkman number Br.
3) The Bejan number shows pronounced variations for the parameters entering into the problem.
4) The presented entropy generation analysis (EGA) may be instrumental for future endeavor of entropy generation minimization(EGM).
Acknowledgments
The authors are indebted to the learned reviewers for their valuable comments, which led to considerable improvement of the paper.
Conflict of interest
All authors declare no conflicts of interest in this paper.











 DownLoad:
DownLoad: