On the Fractal interpolation functions associated with Matkowski contractions

1.   Introduction

Fractal methodology provides a general frame for the understanding of real-world phenomena. In particular, fractal interpolation techniques, defined as fixed points of maps between spaces of functions using iterated function system prove to be more general than classical interpolants and provide good deterministic representations of complex phenomena. Indeed, the fractal interpolation function is not necessarily differentiable at any point, thus, it is closer to natural world phenomena and provides a more powerful tool in fitting real-world data compared to other types of interpolation techniques. In the following, we will recall the iterated function system model which is based upon the property of self-similarity which stipulates that the shape resembles the whole irrespective to the degree of magnification.

Let $(\mathbb X, d)$ be a complete metric space and let ${\mathcal H}(\mathbb X)$ be the set of nonempty compact subsets of $\mathbb X$. We define the Hausdorff metric $d_H$ by

with

The space $({\mathcal H}(\mathbb X), d_H)$ is complete, and compact whenever $\mathbb X$ is compact ^[1]. Let $N\in \mathbb N^*$, the set of positive integers, and $w_n : \mathbb X \longrightarrow \mathbb X$ be a continuous map, $n = 1, \ldots, N$. Then

is called an iterated function system (IFS in short). Now, we define the Hutchinson operator $W : {\mathcal H}(\mathbb X)\longrightarrow {\mathcal H}(\mathbb X)$ by

where $w_n(B) = \big \{w_n(x)\, ,\ x\in B \big\}$. For $k\in \mathbb N^*$, let $W^k$ denote the $k$-fold auto composition of $W$. Any set $G \in {\mathcal H}(\mathbb X)$ such that $W(G) = G$ is called an attractor for the IFS and the IFS admits always at least one attractor ^[2]. Moreover, if each $w_n$ is a contraction, i.e., if there exists $c\in[0, 1)$ such that $d(w(x), w(y))\le c \, d(x, y)$, for all $x, y\in \mathbb X$ then $\mathbb I$ is called hyperbolic. In this case the Hutchinson operator $W$ is a contraction mapping, that is,

and then admits a unique attractor $G = \lim_{k\to\infty}W^k(B)$, for an arbitrary $B\in {\mathcal H}(\mathbb X)$ ^[2]. The classical framework of IFS was studied in ^[1,3,4] as a finite set of contraction maps defined on a compact set of a Euclidean space $\mathbb R^n$. Since then, many researchers have been working on extending these results to more general spaces, generalized contractions and infinite IFSs (^[5,6,7,8,9]).

The fixed point theory plays an important role for the existence of invariant sets in different types of IFSs and this is done by considering a suitable map. In particular, fractal interpolation functions, as an alternative to classical interpolation such as polynomial interpolation, arise as fixed points of the Read-Bajraktarević operator defined on suitable function spaces. This concept was first introduced in 1986 by Barnsley ^[2] to interpolate a given set of data points. Since then, the theory of fractal interpolation has become a powerful and useful tool in applied sciences and engineering. In addition, various types of fractal interpolation functions have been constructed and some of their significant properties including calculus, dimension, smoothness, stability, perturbation error, etc, have been widely studied (^{[10,11,12,13]}). The problem of the existence of the fractal interpolation function (FIF) returns to the study of the existence (and uniqueness) of some fixed points on the fractal space. The most widely studied FIFs are based on the Banach fixed point theorem. This classical result has been extended in several ways, and recently, many researchers have studied the existence of FIFs by using different well-known fixed point results obtained in the fixed point theory ^[15,16]. In particular in ^[17] the authors ensure that the attractor of a nonlinear IFS constructed by Geraghty contractions are graphs of some continuous functions which interpolate the given data and in ^[18] the authors investigate Branciari contraction. In this paper, we investigate Matkowski contractions, introduced in ^[19].

Definition 1. Let $\varphi : [0, \infty)\longrightarrow [0, \infty)$ and $f: \mathbb X \longrightarrow \mathbb X$ be a map. We say that

1) $f$ is $\varphi$-contraction if

for all $x, y\in \mathbb X$.

2) $f$ is Matkowski contraction if it is a $\varphi$-contraction where the function $\varphi$ is non-decreasing and the $\lim\limits_{n\to \infty} \varphi^n(t) = 0$ for all $t > 0$.

In particular, each Banach contraction is a Matkowski contraction with $\varphi(t) = Ct$. In addition, we have the following result.

Theorem 1. ^[19] Let $(\mathbb X, d)$ be a complete metric space. If the function $f: \mathbb X \longrightarrow \mathbb X$ is a Matkowski contraction, then $f$ has a unique fixed point $x_0 \in \mathbb X$. Moreover, for every $x\in \mathbb X$, we have $\lim\limits_{n\to \infty} f^{n}(x) = x_0$.

This result may be seen as a generalization of the Banach theorem ^[20]. Take, for example, $\mathbb X = [0, 1]$ endowed with the Euclidean metric and consider the function $f(x) = \frac{2x}{2+x}$. Then, it is easy to see that the mapping $f$ is not a Banach contraction, indeed,

Moreover, for all $x, y \ge 0$, we have

It follows that $f$ is a Matkowski contraction with comparison function $\varphi(t) = \frac{t}{1+t}$.

In the present work, we will first construct a generalized iterated function systems (GIFS in short). The framework of GIFS was introduced by Mihail and Miculescu ^[7,21] as a natural generalization of a classical IFS. More precisely, GIFS consists of mappings $f: \mathbb X^m\longrightarrow \mathbb X$, for $m > 1$, instead of self-mappings of a metric space $\mathbb X$, where $\mathbb X^m$ is the Cartesian product of $m$ copies of $\mathbb X$. Since then, it has been the subject of study of several papers ^{[8,22,23,24,25]}. Let $J = \{1, \ldots, N\}$ and, for each $n\in J$, let $f_n$ be a Matkowski contraction. In Section 2, we give a quick proof of the fact that the GIFS $\{ \mathbb X^m\, ,\ f_n\, ,\ n\in J\}$ admits a unique attractor. Moreover, for $m = 1$, we ensure that attractors of IFSs constructed by using a Matkowski contraction are graphs of some continuous functions which interpolate the given data. This result is a generalization of the result given in ^[17] since each Geraghty contraction is a Matkowski contraction (see ^[26] for comparison on these two contractions).

Let ${\mathcal C}(I)$ be the set of continuous functions over the interval $I$. Using a fractal interpolation function through a suitable IFS, we can define a method to perturb a function $f \in {\mathcal C}(I)$. We obtain, for free parameter $\alpha$, usually called scale vector, a class of functions $f^\alpha \in {\mathcal C}(I)$ which interpolate and approximate simultaneously the function $f$. Moreover, we can select a suitable IFS so that the corresponding fractal function $f^\alpha$ shares the quality of smoothness or non-smoothness of $f$ or preserves fundamental shape properties, namely positivity, monotonicity, and convexity ^[27,28]. In Section 3, we study a new class of fractal interpolation functions which converges to $f$.

2.   Fractal interpolation function defined by Matkowski contraction

2.1.   Generalized iterated function system

Let $m\in \mathbb N^*$ and $f : \mathbb X^m \longrightarrow \mathbb X$ be a mapping, where the product space is endowed with the metric denoted also by $d$ and defined by

for all $(x_1, \ldots, x_m)$, $(y_1, \ldots, y_m)\in \mathbb X^m$. The mapping $f$ is said to be a Matkowski contraction if, for all $j\in \{1, \ldots, m\}$, $f$ is $\varphi$-contraction, that is,

for all $u = (x_1, \ldots, x_m), v = (y_1, \ldots, y_m)\in \mathbb X^m$, where the function $\varphi$ is non-decreasing and the $\lim\limits_{n\to \infty} \varphi^n(t) = 0$ for all $t > 0$. Many types of $\varphi$-contractions in the literature are considered, see for example, Rakotch contraction ^[29] and Browder contraction ^[30]. In addition, it is worth mentioning that the earlier observation shows that, among compact spaces, the notions of Matkowski, Browder and Rakotch contractions coincide ^[26].

We say that $f$ has a unique fixed point if there exists a unique $x\in \mathbb X$ such that $f(x, x, \ldots, x) = x$. Any mapping $f$ from $(\mathbb X, d)$ to itself, that is $m = 1$, satisfying (2.2) has a unique fixed point (Theorem 1). In this section we will prove that, if $f$ is a Matkowski contraction, then $f$ has a unique fixed point. Therefore, we may define a GIFS which admits a unique attractor. Our first result in this section is the following.

Proposition 1. Let $(\mathbb X, d)$ be a complete metric space and $m\in \mathbb N^*$. Assume that the mapping $f : \mathbb X^m\longrightarrow \mathbb X$ is a Matkowski contraction, satisfying (2.2), then $f$ has a unique fixed point.

Proof. Assume that $m > 1$ and let $g : \mathbb X\longrightarrow \mathbb X$ be a mapping such that $g(x) = f(x, \ldots, x)$, for all $x \in \mathbb X$. Using (2.2), we get

for all $x, y\in \mathbb X$. It follows, that $g$ is a Matkowski contraction and then, by Theorem 1, the mapping $g$ has a unique fixed point $a\in \mathbb X$. Whence $(a, \ldots, a)$ is the unique fixed point of $f$. □

Example 1. Let $m = 2$ and ${\mathtt X} = [0, 1]\cup \{4\}$ be a metric space endowed with the Euclidean metric. We define the functions

In the following we will verify the inequality (2.2). For this, we will consider five possible cases. Let $u = (x, y)\in {\mathtt X}$ and $v = (x', y')\in {\mathtt X}$. First remark that the case $y = y' = 4$ is trivial so we will assume that $(y, y')\neq (4, 4)$.

Case 1: $f(u) = x/2$ and $f(v) = x'/2$. This is the case when $u, v \in [0, 1]^2$ and then

Case 2: $f(u) = \frac12\frac{y}{1+y}$ and $f(v) = 0$. This is the case when $x = y' = 4$ and $y\neq 4$ (the case when $f(v) = \frac12\frac{y'}{1+y'}$ and $f(u) = 0$ is similar). In this case, we have

Note that the function $\phi$ is strictly increasing on $(1, +\infty)$ and

Therefore, since $|y-y'| > 2$, we obtain $d(f(u), f(v))\le \varphi(d(u, v)).$

Case 3: $f(u) = \frac12\frac{y}{1+y}$ and $f(v) = \frac12\frac{y'}{1+y'}$. This is the case when $x = x' = 4$, $y\neq 4$ and $y'\neq 4$. Then,

Case 4: $f(u) = x/2$ and $f(v) = 0$. This is the case when $x\neq 4$, $y\neq 4$ and $y' = 4$ (the case $f(v) = x'/2$ and $f(u) = 0$ is similar). It follows, using (2.3), then

Case 5: $f(u) = x/2$ and $f(v) = \frac{1}{2}\frac{y'}{1+y'}$. This is the case when $x, y\neq 4$, $x' = 4$ and $y'\neq 4$ (the case $f(v) = x'/2$ and $f(u) = \frac{1}{2}\frac{y}{1+y}$ is similar). Then,

Let, for $n = 1, \ldots, N$, $f_n : \mathbb X^m \longrightarrow \mathbb X$ be a Matkowski contraction mapping. Then

is called a GIFS. Now, we define the fractal operator $F : {\mathcal H}(\mathbb X)^m \longrightarrow {\mathcal H}(\mathbb X)$, associated with the GIFS, by

Any fixed point of the operator $F$, that is, a set $G \in {\mathcal H}(\mathbb X)$ such that $F(G, G, \ldots, G) = G$ is called an attractor for the GIFS. In the next section, we prove that any GIFS satisfying the Matkowski contraction admits a unique attractor $G$ [24, Theorem 4].

Theorem 2. Let $(\mathbb X, d)$ be a complete metric space and we define, for $n\in J$, the mappings $f_n : \mathbb X^m \longrightarrow \mathbb X$ satisfying the Matkowski contraction (2.2) with the same function $\varphi$. Then the GIFS $\{ \mathbb X^m\, ,\ f_n\, ,\ n\in J\}$ admits a unique attractor $G$.

Proof. Let $A = (A_1, \ldots, A_m)$ and $B = (B_1, \ldots, B_m) \in {\mathcal H}(\mathbb X)^m$. Choose $j\in \{1, \ldots, m\}$ and let $\varphi$ such that (2.2) is satisfied for the mappings $f_n$, $n\in J$. We only have to prove that

from which we deduce that the fractal operator $F$ is a Matkowski contraction on $({\mathcal H}(\mathbb X^m), d_H)$. Using Proposition 1, $F$ has a unique fixed point $G$ as required.

Let $z\in F(A_1, \ldots, A_m)$, then there exists $n\in J$ such that $z = f_n(x_1, \ldots, x_m)$ with $x_i\in A_i,$ for all $i\in J$. Now, since $B_j$ is compact, then there exists $y^*_j\in B_j$ such that

Therefore $d(x_j, y^*_j) \le D(A_j, B_j)\le d_H(A_j, B_j)$. It follows that

Remark that $F(A)$ is a compact set of ${\mathcal H}(\mathbb X)^m$ and $z\longmapsto d(z, F(B))$ is continuous. Therefore

Similarly, we may prove that $D\big(F(B), F(A)\big) \le \varphi\Big(d_H(A_j, B_j)\Big)$ and then (2.5). □

2.2.   Fractal interpolation function

Let $\Delta : x_0 < x_1 < \ldots < x_N$ be a partition of the real compact interval $I = [x_0, x_N]$ and consider the data set $\Big\{(x_i, y_i)\in I\times \mathbb R\; \; i = 0, 1, \ldots, N \Big\}$. Let $K$ be a suitable compact subset of $\mathbb R$ containing $y_i$, $i\in J_0 = \{0, \ldots, N\}$. We assume that the compact metric space $I \times K$ is endowed with uniform metric $d$ defined as

for all $(x_1, x_2) \in I^2$ and $(y_1, y_2)\in K^2$. Recall the set $J = \{1, \ldots, N\}$ introduced in the Section 1. For $i\in J$, we set $I_i = [x_{i-1}, x_i]$ and let $L_i : I\longrightarrow I_i$ be a contractive homeomorphism such that

for some $0\le l < 1$. We consider $N$ continuous mappings $F_i: I\times K \longrightarrow K$ satisfying

We assume also that $F_i$ is a Matkowski contraction with respect to the second variable, i.e., there exists function $\varphi_i: [0, +\infty) \to [0, +\infty)$ satisfying the condition of item (2) of Definition 1 such that

In particular, we may consider the following system,

where the real constants $a_i$ and $k_i$ and the function $F_i$ are determined by conditions (2.6) and (2.7). It is clear that if $g(y) = \frac{y}{1+y}$ then $F_i$ is not a Banach contraction but it is a Matkowski contraction with respect to the second variable.

We define also the function $w_i : I\times K \rightarrow I_i\times K$ by

for all $i \in J$. Assume that $\lim_{m\to +\infty}\varphi^m(t) = 0,$ where $\varphi = \sup_{i\in J} \varphi_i$. Using (2.8) and (2.9) we get a wide variety of systems for different approximations problems, giving more flexibility and applicability of the fractal interpolation method. In the following result we will prove the existence of the fractal interpolation function (FIF) corresponding to the IFS $\{I\times[a, b]\, ,\ w_i\, ,\ i = 1, 2, \ldots, N\}$. This result generalizes, in particular [2, Theorem 1] and the result in ^[14] since we only assume that the function $F_i$ are Matkowski contractions with respect to the second variable.

Theorem 3. The IFS $\{I\times[a, b]\, ,\ w_i\, ,\ i = 1, 2, \ldots, N\}$ defined above admits a unique attractor $G$, which is the graph of a continuous function $f : I\rightarrow [a, b]$ satisfying $f(x_i) = y_i$, for $i = 0, 1, \ldots, N$.

Proof. Let $G$ be any attractor of the IFS $\{I\times[a, b], w_i, \ i\in J\}$ and then we have

In fact, for each $i\in J$, the mapping $w_i$ may not satisfy the Matkowski contraction. But, if so, we may prove the existence and the uniqueness of the attractor $G$ using the Hutchinson operator $W$ defined in (1.1) (Theorem 2). In the following we will give the proof of Theorem 3. First, remark that the set $\widetilde I = \{x\in I, \; \exists y\in [a, b]$ with $(x, y)\in G \}$, the projection of $G$ into $I$, is equal to $I$. Indeed, since $G = \bigcup_{i = 1}^N w_i(G)$, we can deduce that $\widetilde I = \bigcup_{i = 1}^N L_i(\widetilde I)$ and on the other hand the IFS $\big\{I, L_i\, \ i\in J \big\}$ is hyperbolic having unique attractor $I$. Now, we present the proof of our result in two steps. We will prove that $G$ is the graph of a function $f$ defined on $I$ and as a consequence we obtain the uniqueness of the attractor $G$ since the union of two attractors is an attractor. In the second step we prove that $f$ is continuous by studying the fixed point of Read-Bajraktarevíc operator.

Step 1 :

Let's prove that $G$ is the graph of a function $f : I\rightarrow [a, b]$ by proving that only one $y$-value corresponds to each $x$-value. First, remark that the IFS $\big\{I, L_i\, \ i\in J \big\}$ is hyperbolic having unique attractor $I = [x_0, x_N]$. Therefore, using Eq (2.10), we obtain that for every $x\in I$ there exists $y\in [a, b]$ such that $(x, y)\in G$. In the following, we will prove that $y$ is unique. For this, we consider, for $i = 0, \ldots, N$, the set

● case $x = x_0$. Since for all $i\neq 1$, we have $w_i({\mathtt X}_0)\cap {\mathtt X}_0 = \emptyset$, $w_1({\mathtt X}_0) = {\mathtt X}_0$. Moreover,

Whence $w_1$ has a unique fixed point on the compact metric space ${\mathtt X}_0$. In addition, using Theorem 2, the IFS $\{ {\mathtt X}_0, w_1\}$ has a unique attractor ${\mathtt X}_0$ and then ${\mathtt X}_0 = \{(x_0, y_0)\}$ as required.

● case $x\in \{x_1, \ldots, x_N\}$. Similarly, we have ${\mathtt X}_N = \{(x_N, y_N)\}$ and, for $i = 1, 2, \ldots, N-1$, remark that ${\mathtt X}_i = w_{i+1}({\mathtt X}_0)\cup w_i({\mathtt X}_N) = \{(x_i, y_i)\}$.

● case $x\notin\{x_0, \ldots, x_N\}$. We will prove that, if there exist $y_x, y_x'\in [a, b]$ such that $(x, y_x), \ (x, y'_x)\in G$ then $|y_x-y_x'| = 0$. Since $G$ is compact, there exist $i\in\{1, 2, \ldots, N\}$ and $\xi\in I_i$ such that

Moreover, by the last two steps, we may assume that $\xi\in (x_{i-1}, x_i)$. Now, we may take $t, t'\in [a, b]$ such that $w_i(u) = (\xi, y_\xi)$ and $w_i(v) = (\xi, y'_\xi)$ where $u = (L_i^{-1}(\xi), t)\in G$ and $v = (L_i^{-1}(\xi), t')\in G$. It follows that

Therefore

It follows, since $\varphi^{n}(t) \to 0$ and then $\varphi(t)\le t$ for $t > 0$, that $y_\xi = y'_\xi$.

Step 2:

We will prove that $f$ is continuous. We consider the complete metric space $({\mathcal G}, \rho)$ such that

and the metric $\rho$ is defined by

Now, we define the Read-Bajraktarevíc operator $\mathtt T$ on ${\mathcal G}$ by

Assume that we have shown that, $f, g\in {\mathcal G}$, we have

then $\mathtt T$ has a unique fixed point $\widetilde g \in \mathcal{G}$. Therefore the graph of $\widetilde g$ is an attractor of the IFS $\{I\times [a, b], w_i, i = 1, 2, \ldots, N\}$ which implies that $\widetilde g = f$ and then $f$ is continuous.

Now, we will prove (2.11). Let $f, g\in {\mathcal G}$ and $i \in J$. For any $x\in I_i$, we have

Now, observe that

Then, since $I$ is compact, we get (2.11) and then $\mathtt T$ has a unique fixed point as required. □

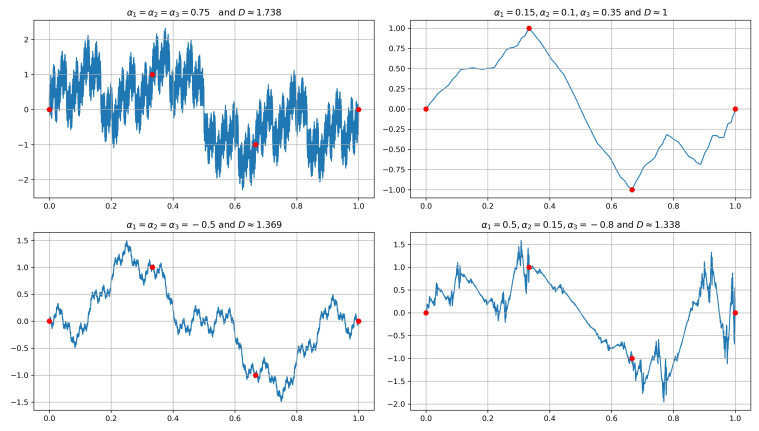
In the following example we study the affect of box dimension when we consider a nonlinear IFS.

Example 2. The box dimension is widely used to describe the complexity of certain figures and proved to be appropriate and effective method for fractal dimension estimate. The theoretical box dimension $D$ is given by

where $\mathtt N_\epsilon$ is the minimum number of $\epsilon \times \epsilon$ squares needed to cover the graph of $f$. In this example, we consider, the data set $\Delta: = \Big\{ (0, 0), (1/3, 1), (2/3, -1), (1, 0)\Big\}$ and we define

In Figure 1, we consider the case when the function $g_n$ are defined by $g_n(y) = \alpha_n y$ with free parameters $\alpha_n$ obeying $\alpha_n\in (-1, 1)$, $n = 1, 2, 3$. It is obvious that the system (2.12) satisfies (2.6), (2.7) and (2.8). The parameters $\alpha_n$ are called vertical scaling factors and have important consequences on the box dimension $D$ of the graph of the FIF, which will be denoted by $f^\alpha$, with $\alpha \in (0, 1)^3$. Indeed, since we consider equally spaced interpolation points that do not all lie on the same line, we have ^[31]

when $\sum_{n = 1}^{3} |\alpha_n| > 1$. For different values of $\alpha_n$, we determine the box dimension of the corresponding FIF in Figure 1. In particular, we obtain smooth or non-smooth fractal interpolation function depending on the choice of scaling factors. Moreover, since for each $n$ the function $F_n$ is a Banach contraction with respect to the second variable, we assert that is a special case of FIF introduced in ^[2].

In Figure 2, we consider again the system (2.12) but with different function $g$. From empirical evidence, we obtain that the function $g$ has a great impact on the box dimension. It is natural to ask whether the box dimension of FIF depends on $\|g\|_\infty$ in general. This seems to be wrong as we can see in Figure 2. Indeed, we obtain different values of the box dimension $D$ that behaves non monotonically regardless of the fact that the norms of the functions used in the system are proportional.

3.   Fractal interpolation Function, sequential approach

Let $\Delta : x_0 < x_1 < \ldots < x_N$ be a partition of the real compact interval $I = [x_0, x_N]$ and consider the data set $\Big\{(x_i, y_i)\in I\times \mathbb R\; \; i = 0, 1, \ldots, N \Big\}$. Let $K$ be a suitable compact subset of $\mathbb R$ containing $y_i$, $i\in J_0 = \{0, \ldots, N\}$. Let $f\in {\mathcal C}(I, K)$, the normed space of real-valued continuous functions on $I$ with values belonging to $K$. Assuming that ${\mathcal C}(I, K)$ is endowed with the uniform norm, we study, in this section, a new class of fractal interpolation functions which converge to $f$. Therefore, let, for $n\in \mathbb N$, $b_n : { {\mathcal C}}(I, K)\longrightarrow {\mathcal C}(I, K)$ be bounded and nonidentity linear operator such that, for every $h \in {\mathcal C}(I, K),$ we have

Let $g_i$, $i\in J$, be a differentiable function with domain ${\mathcal D}$. We assume that $K \subset {\mathcal D}$ and $\sup_K |g'_i| < 1$ for all $i\in J$. Now, let $f\in {\mathcal C}(I, K)$ that interpolates the data $\{ (x_i, y_i), \, i \in J_0\}$ and consider, for each $n\in \mathbb N$, the IFS defined through the maps

where the real constants $a_i$ and $k_i$ are determined by condition (2.6) and assume that (2.7) is satisfied. We may consider the case where $g_i(y): = g_\beta(y) = \frac{y}{1+\beta y}$, for $\beta > 0$ and $y > 0$. In this case, we have $|F_{n, i}(x, y) -F_{n, i}(x, y')|/|y-y'| = 1 /\big((1+\beta y)(1+\beta y')\big)$ for $y\neq y'$, and then the ratio $|F_{n, i}(x, y) -F_{n, i}(x, y')|/|y-y'|$ for $y\neq y'$ can be made arbitrarily close to $1$ by taking $y$ and $y'$ sufficiently close to $0$ and then $F_{n, i}$ is not a Banach contraction with respect to the second variable. Nevertheless, we can prove that $F_{n, i}$ is a Matkowski contraction. Therefore, for each $\beta > 0$ and $n\in \mathbb N$ the IFS defined by (3.2) admits a unique attractor $G_{\beta, n}$ which is the graph of a continuous function $f^\beta_n$ satisfying $f^\beta_n(x_i) = y_i$, for each $i \in J_0$. The FIF $f_n^\beta$ is referred to as $\beta$-fractal function for $f$.

The most widely studied $b_n(f)$ in the literature is the Bernstein polynomial of $f$ defined by

for all $x\in I$ and $n\in \mathbb N$. One can verify that $B_n(f, x_0) = h(x_0)$ and $B_n(f, x_N) = f(x_N)$. In addition, from classical approximation theory ^[32] we have that

where $\omega_f$ is the modulo of continuity of $f$ defined as

Since $f$ is uniformly continuous on $I$, we obtain that $\omega_f(n^{-1/2}) \to 0$ as $n\to \infty$. When the IFS is defined using the Bernstein polynomial $B_n(f, \cdot)$ and the function $g_\beta$, the fractal interpolation function $f_n^\beta$ is called Bersntein $\beta$-fractal function of order $n$ of $f$.

Example 3. Let interpolation points $\big\{(0, 1/5), (1/3, 1/2), (1/2, 1/3), (4/5, 4/5), (1, 3/5)\big\}$ be given. We consider the functions $g_i(y) = \frac{y}{1+\beta_i y}$ and $b_n(f)$ is the Bernstein polynomial $B_n(f, \cdot)$, where $f$ is the piecewise linear function passing through the interpolation points given above.

1) Let us consider a constant scaling vector $\beta = (0.6, \ldots, 0.6)$. Then the graph of the $\beta$-FIF for $f$ generated by IFS (3.2), is plotted in Figure 3(a).

2) Take a non constant scaling vector $\beta = \{\beta_1, \beta_2, \beta_3, \beta_4\}$ then the graph of the $\beta$-FIF with the above variable parameters is displayed in Figure 3(b), (c).

From Figure 3, we can notice that the self-similarity of the fractal interpolation curve shown in Figure 3(b), (c) is weaker than that of FIF in Figure 3(a). Hence, we can say that the FIFs with non constant scaling vector may have more flexibility and applicability. In fact, the FIFs generated by those IFSs with constant parameters usually have obvious self-similarity character, which could lead to the loss of flexibility, and might cause obvious errors in fitting and approximation of some complicated curves and non-stationary data that show less self-similarity.

Our first main result in this section is the following.

Theorem 4. Let $\psi \in {\mathcal C}(I, K)$ be a function providing the data $\{(x_i, y_i), i \in J_0\}$ and let $f\in {\mathcal C}(I, K)$ interpolate $\psi$ with respect to these data. Let $h: = \max_i(x_{i+1}-x_i)$ and assume, for some $r > 0$, that

Then, the sequence $\{\hat f_n\}_n$ of fractal interpolation functions, defined through the system (3.2), converges to $\psi$ as $h\to 0$ and $n\to \infty$.

Proof. We consider the complete metric space $({\mathcal G}, \rho)$ where

and $\rho$ is the uniform metric on ${\mathcal G}$. Now, for each $n\in \mathbb N$, we define the Read-Bajraktarevíc operator $\mathtt T_n : {\mathcal G}\longrightarrow {\mathcal G}$ by

In addition, for all $i\in J$, $x\in I_i$ and $h_1, h_2 \in {\mathcal G}$, we have

Now observe that

which implies that

where $\varphi = \sup_i \varphi_i$. Therefore, for $n\in \mathbb N$, $\mathtt T_n$ is also a Matkowski contraction on the complete metric space $({\mathcal G}, \rho)$ and therefore $\mathtt T_n$ possesses a unique fixed point $\hat f_n$ on ${\mathcal G}$. It follows that $\hat f_n$ satisfies the following functional equation

and then,

where $\gamma = \sup_K |g' _i| < 1$. As a consequence, we obtain

□

Example 4. In this example, we consider the operator $b_n(f)$ to be the Bernstein polynomial of $f$. First notice that, from (3.3) and (3.5), the Bersntein $\beta$-fractal function of order $n$ of $f$ satisfies

The most widely studied of fractal interpolation function has been obtained using the IFS

where the real constants $a_i$ and $k_i$ are determined by the condition (2.6), the functions $q_i$ are continuous satisfying conditions (2.7) and (2.8) and $\alpha_i$ are free parameters such that $\alpha_i \in (-1, 1)$. Therefore, the corresponding FIF will be indexed by $\alpha\in (-1, 1)^N$ and will be denoted by $f^\alpha$ named $\alpha$-fractal interpolation function (^[2,33]). As an application of Therorem 3.1, we can construct a fractal interpolation function $\hat f$, where (2.8) may be violated, and $\hat f$ is as close as we want to $f^\alpha$. In addition, we have the following consequence.

Corollary 1. Let $\alpha\in (0, 1)^N$ be a scaling vector and $f^\alpha$ be a $\alpha$-FIF interpolating data $\{(x_i, y_i), i \in J_0\}$. For every $\epsilon > 0$, there exist two approximating sequences $\{l_n\}_n$ and $\{h_n\}_n$ of fractal functions such that

for all $x\in I$ and $n\ge N_0 \in \mathbb N$.

Proof. Theorem 4 ensures the existence of a sequence $\{\hat f_n\}_n$ of fractal interpolation functions such that

Now define the fractal functions

for all $x\in I$. It follows that

Similarly, we have

□

4.   Perspective and open problem

$1)$ Let $\left\{(x_i, y_j, z_{i, j}), i = 0, 1, \ldots, N, j = 0, 1, \ldots, M\right\}\subset I\times J \times K \subset \mathbb R^3$ be a given data set. We denote $I_i, J_j, D_{i, j}$ and $D$ by $[x_{i-1}, x_i], [y_{j-1}, y_j], I_i\times J_j$ and $I\times J$ respectively. We define mappings $w_{i, j} : D\times K \rightarrow D_{i, j}\times K$ by

where $L_{i, j} : D \rightarrow D_{i, j}$ are contractive homeomorphisms and $F_{i, j}: D\times K \rightarrow K$ are Matkowski contractions. We strongly believe that the framework established in Section 3 remains true if we consider the GIFS

We find in this case that it is possible to construct a function $f : I\times J \rightarrow \mathbb R$ whose graph is the attractor of a GIFS and interpolates the given data set. Such a result would be analogous to Theorem 2 of ^[34]. The pursuit of this question is encouraged by the application of fractal surfaces in many areas such as earth sciences, surface physics, and medical sciences ^{[35,36,37,38,39]}.

$2)$ Let $(\mathbb{Y}, d)$ be a complete metric space and consider $\mathbb X = \mathbb{Y}\times \mathbb{Y}$. Recall the IFS $\left\{ \mathbb X, f_n, n = 1, \ldots, N\right\}$ defined in Section 2 which admits a unique attractor $G\in\mathcal{H}(\mathbb X)$. It is interesting to ask if the projection $G$ onto $\mathbb{Y}$ itself is an attractor of an IFS. We conject that the projection is the graph of a continuous function which is not self-similar in general.

$3)$ Let $\Omega$ be a nonempty set and let $\zeta : \Omega\times \Omega \longrightarrow [1, +\infty[$ be a function. We define $d_\zeta : \Omega\times \Omega \longrightarrow [0, +\infty[$ such that, for all $x, y, z \in \Omega$, we have

(a) $d_\zeta (x, y) = 0 \iff x = y;$

(b) $d_\zeta (x, y) = d_\zeta(y, x)$;

(c) $d_\zeta (x, y)\le \zeta (x, y)\Big[d_\zeta (x, z) + d_\zeta (z, y) \Big].$

Then, $(\Omega, d_\zeta)$ is called a $\zeta$-metric space ^[40] which extends the $b$-metric space $(\zeta(x, y) = b)$ and the metric space $(\zeta (x, y) = 1)$. We conject that the results in Section 2 remain true when we consider $\mathbb X$ to be a $\zeta$-metric space and extends in particular [42, Theorem 3.7]. This requires, the introduction of new contractive conditions of general integral type in the setting of $\zeta$-metric spaces and the definition of a generalization of the Hausdorff distance $d_\zeta$ on ${\mathcal H}(\mathbb X).$

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgments

The authors extend their appreciation to the Deanship of Scientific Research at King Khalid University for funding this work through the Small Groups Project under grant number R.G.P.1/138/44.

Conflict of interest

The authors declare there is no conflicts of interest.